Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part I: Experimental Investigation
Abstract
1. Introduction
- Long-term cycling at spatially homogeneous and steady (HS) thermal boundary conditions at defined temperature levels of the cells (reference case).
- Cell aging at spatially inhomogeneous and steady (IS) thermal boundary conditions comprising defined temperature gradients applied to the cells.
- Cell aging at homogeneous and inhomogeneous transient (HT, IT) thermal boundary conditions, periodically changing between the states defined in conditions (1) and (2).
- Discussion of the correlations between thermal boundary conditions (1)–(3) and aging during cycling.
- Discussion of different thermal management strategies based upon this.
2. Experimental Setup
2.1. Description of the Cell
2.2. Boundary Conditions
2.2.1. Electrical Parameters
2.2.2. Thermal Parameters
2.3. Verification of the Experimental Setup
2.4. Testing Procedure
3. Results and Discussion
3.1. Initial State
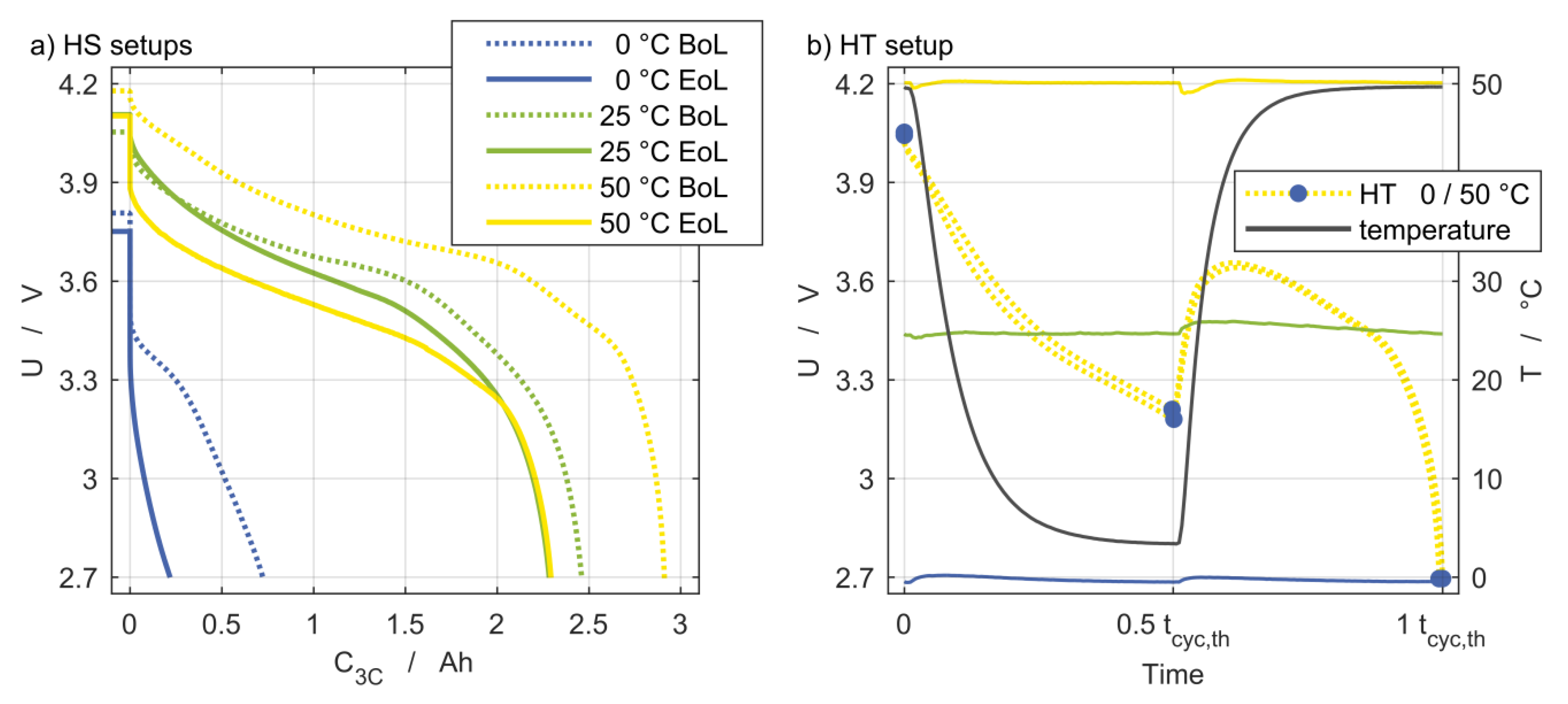
3.2. Impact of Thermal Boundary Conditions
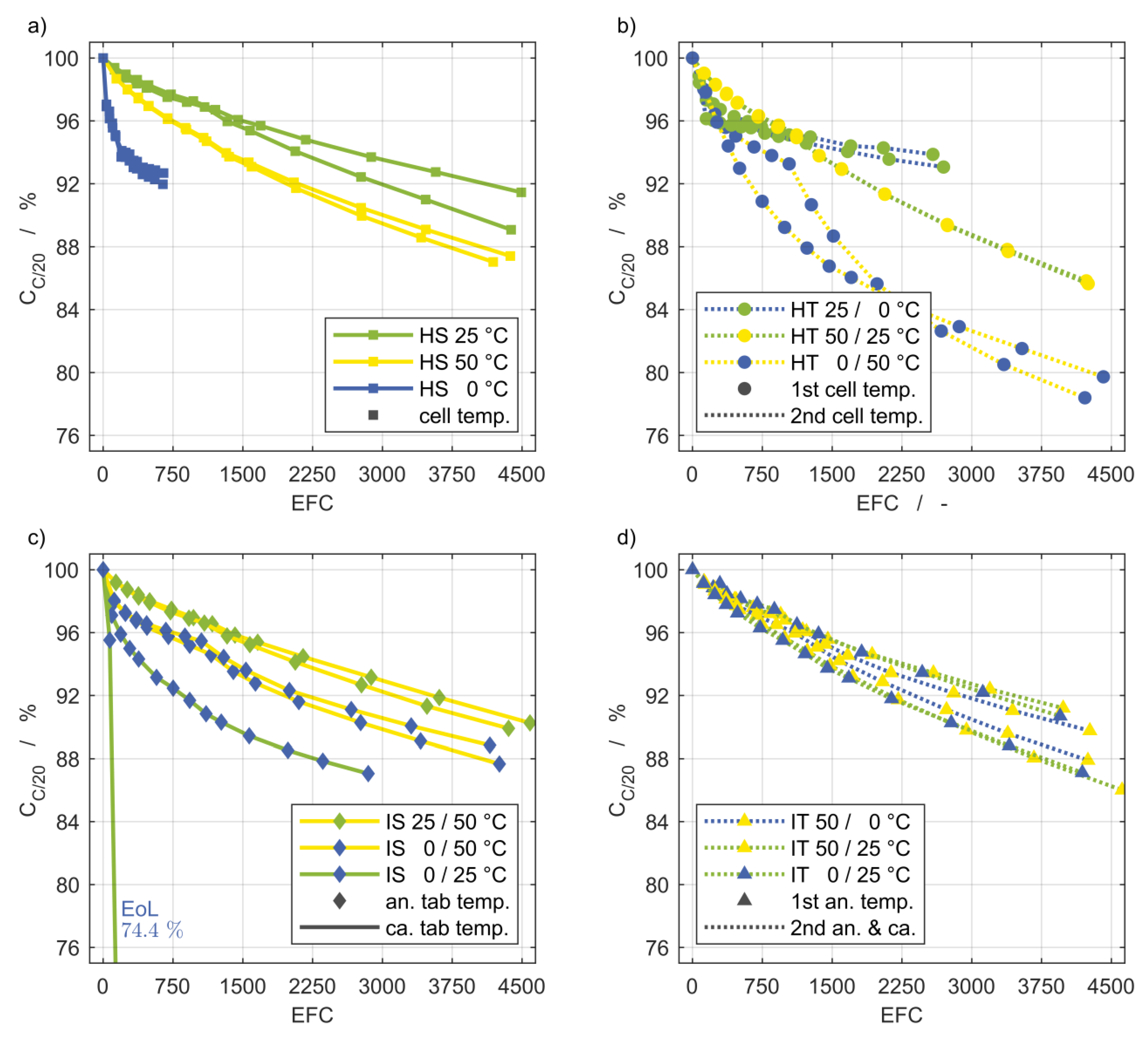
3.3. Capacity Measurement
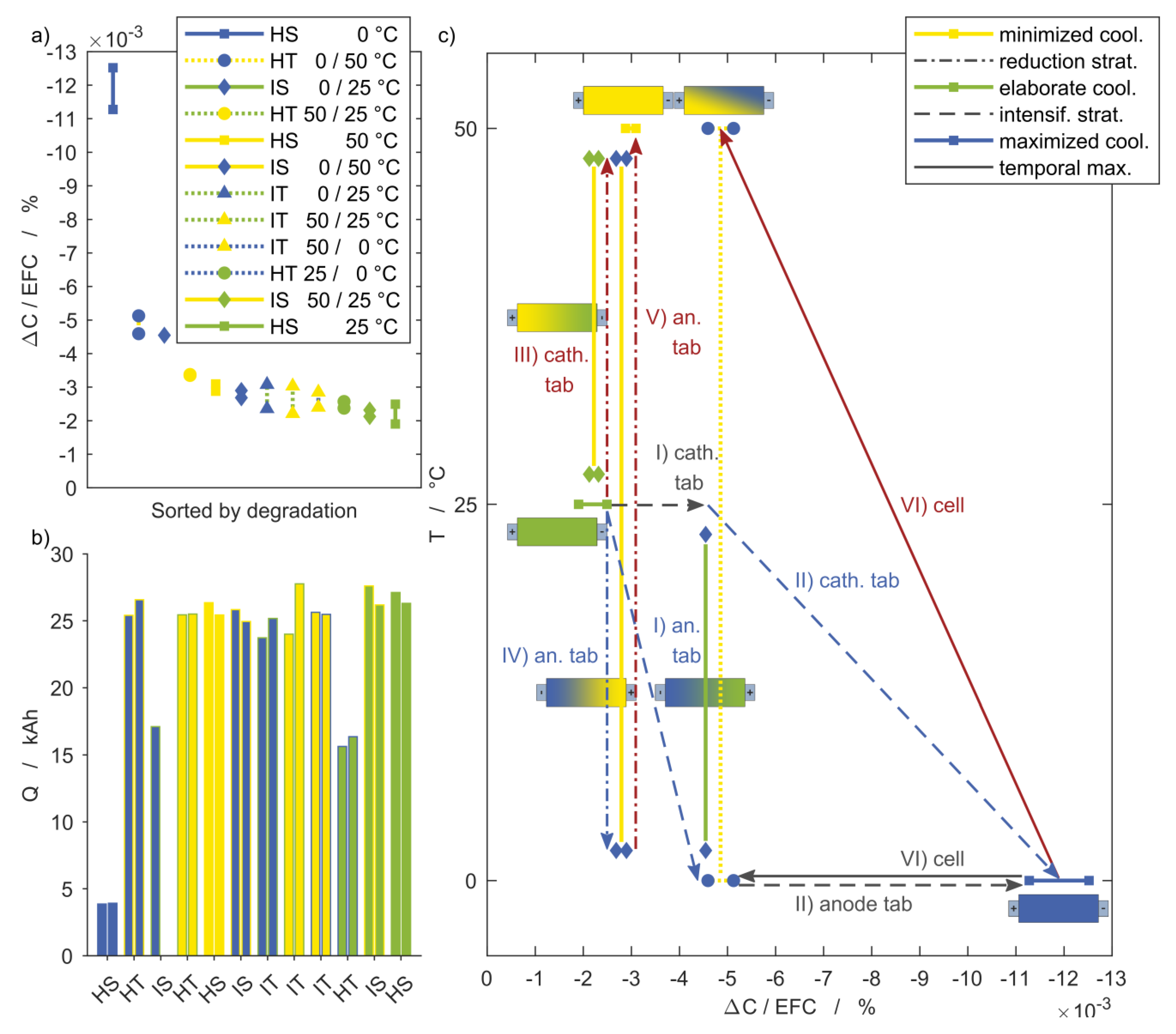
3.4. Impedance Measurement
3.5. Thermal Management Strategies
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bandhauer, T.M.; Garimella, S.; Fuller, T.F. A Critical Review of Thermal Issues in Lithium-Ion Batteries. J. Electrochem. Soc. 2011, 158, R1. [Google Scholar] [CrossRef]
- Bloom, I.D.; Cole, B.W.; Sohn, J.J.; Jones, S.A.; Polzin, E.G.; Battaglia, V.S.; Henriksen, G.L.; Motloch, C.G.; Richardson, R.A.; Unkelhaeuser, T.; et al. An accelerated calendar and cycle life study of Li-ion cells. J. Power Sources 2001, 101, 238–247. [Google Scholar] [CrossRef]
- Vetter, J.; Novák, P.; Wagner, M.R.; Veit, C.; Möller, K.-C.; Besenhard, J.O.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Maleki, H. Thermal Properties of Lithium-Ion Battery and Components. J. Electrochem. Soc. 1999, 146, 947. [Google Scholar] [CrossRef]
- Arrhenius, S. Über die Reaktionsgeschwindigkeit bei der Inversion von Rohrzucker durch Säuren. Zeitschrift Phys. Chem. 1889, 4U. [Google Scholar]
- Newman, J.; Tiedemann, W. Porous-electrode theory with battery applications. AIChE J. 1975, 21, 25–41. [Google Scholar] [CrossRef]
- Bernardi, D. A General Energy Balance for Battery Systems. J. Electrochem. Soc. 1985, 132, 5. [Google Scholar] [CrossRef]
- Mistry, A.; Fear, C.; Carter, R.; Love, C.T.; Mukherjee, P.P. Electrolyte Confinement Alters Lithium Electrodeposition. ACS Energy Lett. 2019, 4, 156–162. [Google Scholar] [CrossRef]
- Ramadass, P.; Haran, B.; White, R.; Popov, B.N. Capacity fade of Sony 18650 cells cycled at elevated temperatures Part I. Cycling performance. J. Power Sources 2002, 112, 606–613. [Google Scholar] [CrossRef]
- Ramadass, P.; Haran, B.; White, R.; Popov, B.N. Capacity fade of Sony 18650 cells cycled at elevated temperatures Part II. Capacity fade analysis. J. Power Sources 2002, 112, 614–620. [Google Scholar] [CrossRef]
- Schmalstieg, J.; Käbitz, S.; Ecker, M.; Sauer, D.U. A holistic aging model for Li(NiMnCo)O2 based 18650 lithium-ion batteries. J. Power Sources 2014, 257, 325–334. [Google Scholar] [CrossRef]
- Schuster, S.F.; Bach, T.; Fleder, E.; Müller, J.; Brand, M.; Sextl, G.; Jossen, A. Nonlinear aging characteristics of lithium-ion cells under different operational conditions. J. Energy Storage 2015, 1, 44–53. [Google Scholar] [CrossRef]
- Troxler, Y.; Wu, B.; Marinescu, M.; Yufit, V.; Patel, Y.; Marquis, A.J.; Brandon, N.P.; Offer, G.J. The effect of thermal gradients on the performance of lithium-ion batteries. J. Power Sources 2014, 247, 1018–1025. [Google Scholar] [CrossRef]
- Klein, M.; Tong, S.; Park, J.W. In-plane nonuniform temperature effects on the performance of a large-format lithium-ion pouch cell. Appl. Energy 2016, 165, 639–647. [Google Scholar] [CrossRef]
- Chen, D.; Jiang, J.; Kim, G.-H.; Yang, C.; Pesaran, A. Comparison of different cooling methods for lithium ion battery cells. Appl. Therm. Eng. 2016, 94, 846–854. [Google Scholar] [CrossRef]
- Gering, K.L.; Sazhin, S.V.; Jamison, D.K.; Michelbacher, C.J.; Liaw, B.Y.; Dubarry, M.; Cugnet, M. Investigation of path dependence in commercial lithium-ion cells chosen for plug-in hybrid vehicle duty cycle protocols. J. Power Sources 2011, 196, 3395–3403. [Google Scholar] [CrossRef]
- Carter, R.; Love, C.T. Modulation of Lithium Plating in Li-Ion Batteries with External Thermal Gradient. ACS Appl. Mater. Interfaces 2018, 10, 26328–26334. [Google Scholar] [CrossRef]
- Atkinson, R.W.; Carter, R.; Love, C.T. Operational strategy to stabilize lithium metal anodes by applied thermal gradient. Energy Storage Mater. 2019, 22, 18–28. [Google Scholar] [CrossRef]
- Hunt, I.A.; Zhao, Y.; Patel, Y.; Offer, J. Surface Cooling Causes Accelerated Degradation Compared to Tab Cooling for Lithium-Ion Pouch Cells. J. Electrochem. Soc. 2016, 163, A1846–A1852. [Google Scholar] [CrossRef]
- ISO International Organization for Standardization Electrically propelled road vehicles – Test specification for Lithium-ion traction battery packs and systems – Part 1: high-power applications; International Organization for Standardization: Geneva, Switzerland, 2011.
- Loges, A.; Herberger, S.; Werner, D.; Wetzel, T. Thermal characterization of Li-ion cell electrodes by photothermal deflection spectroscopy. J. Power Sources 2016, 325, 104–115. [Google Scholar] [CrossRef]
- Cannarella, J.; Arnold, C.B. Stress evolution and capacity fade in constrained lithium-ion pouch cells. J. Power Sources 2014, 245, 745–751. [Google Scholar] [CrossRef]
- Wünsch, M.; Kaufman, J.; Sauer, D.U. Investigation of the influence of different bracing of automotive pouch cells on cyclic liefetime and impedance spectra. J. Energy Storage 2019, 21, 149–155. [Google Scholar] [CrossRef]
- Schmidt, J.P.; Arnold, S.; Loges, A.; Werner, D.; Wetzel, T.; Ivers-Tiffée, E. Measurement of the internal cell temperature via impedance: Evaluation and application of a new method. J. Power Sources 2013, 243, 110–117. [Google Scholar] [CrossRef]
- Werner, D.; Loges, A.; Becker, D.J.; Wetzel, T. Thermal conductivity of Li-ion batteries and their electrode configurations – A novel combination of modelling and experimental approach. J. Power Sources 2017, 364, 72–83. [Google Scholar] [CrossRef]
- Gnielinski, V. On heat transfer in tubes. Int. J. Heat Mass Transf. 2013, 63, 134–140. [Google Scholar] [CrossRef]
- Oertel, H.; Antmann, S.S.; Marsden, J.E.; Sirovich, L. (Eds.) Prandtl-Essentials of Fluid Mechanics, 3rd ed.; Springer New York: New York, NY, USA, 2010; Volume 158. [Google Scholar]
- Bloom, I.; Jansen, A.N.; Abraham, D.P.; Knuth, J.; Jones, S.A.; Battaglia, V.S.; Henriksen, G.L. Differential voltage analyses of high-power, lithium-ion cells. J. Power Sources 2005, 139, 295–303. [Google Scholar] [CrossRef]
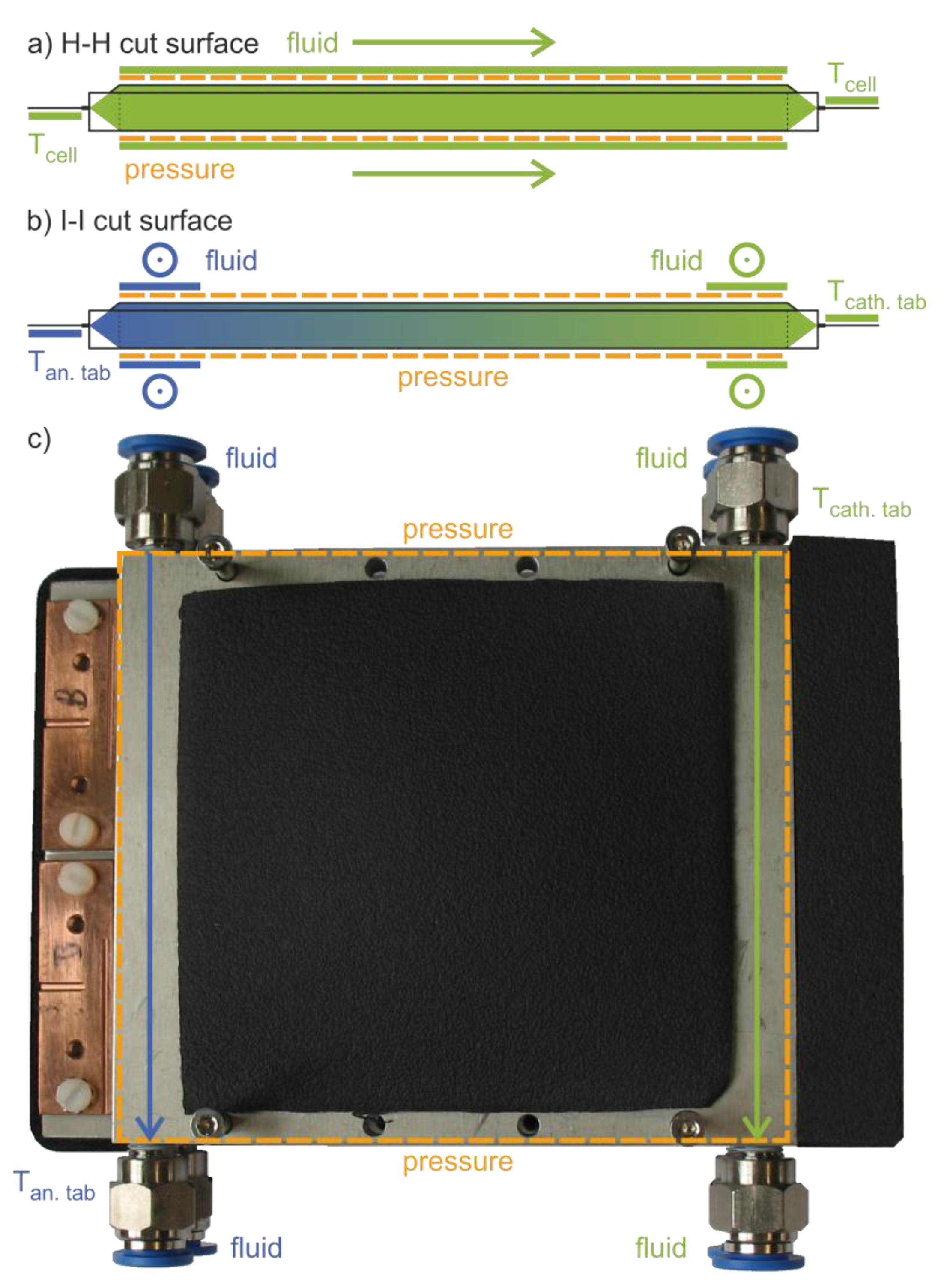
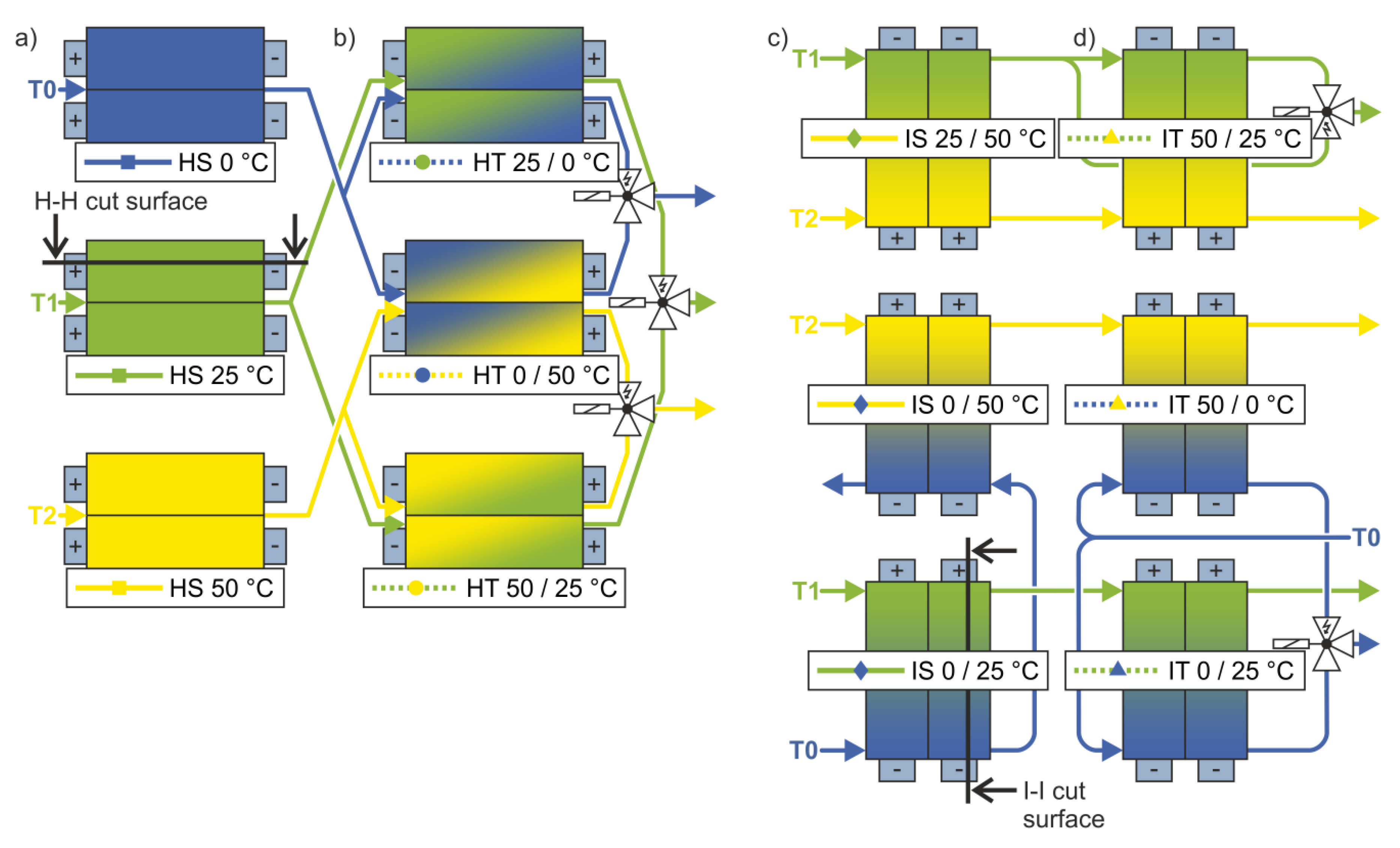
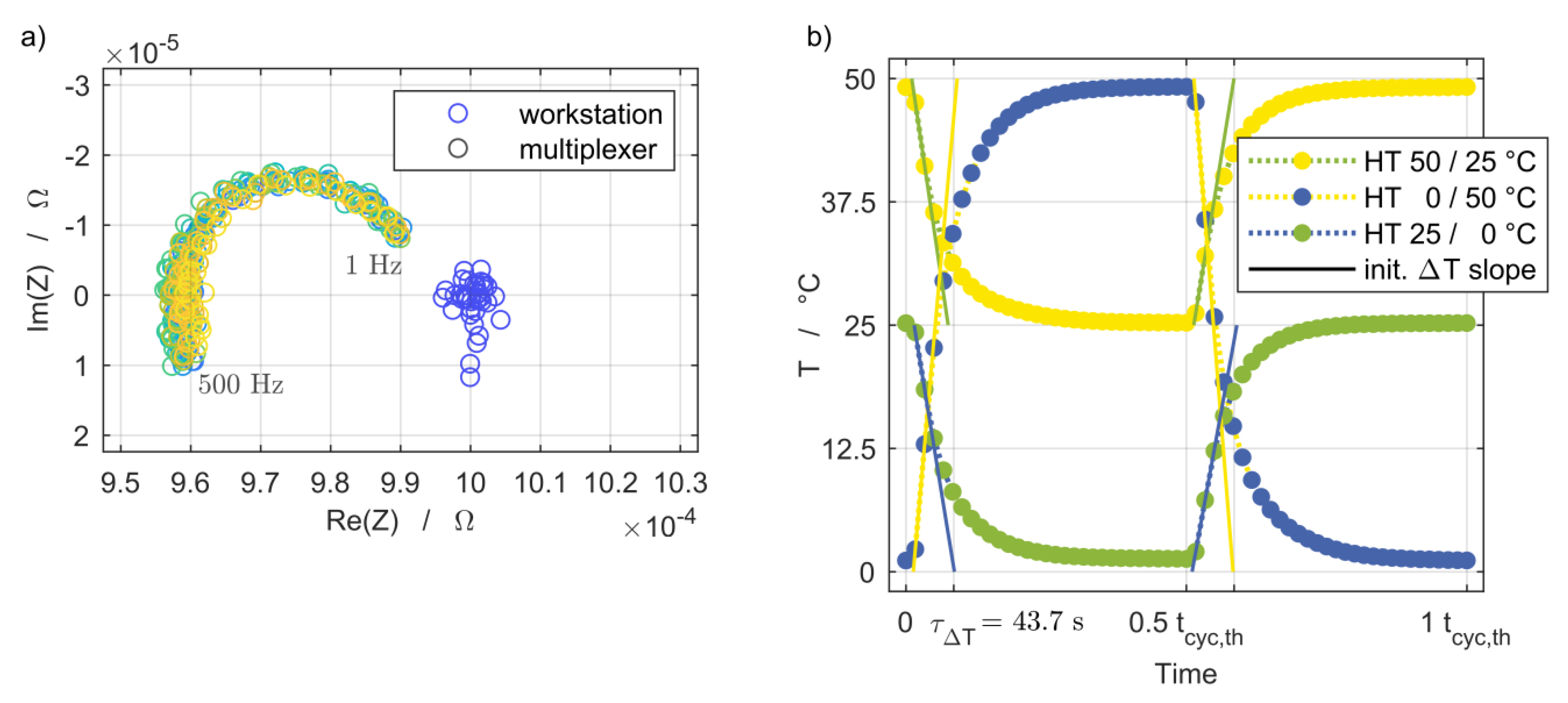

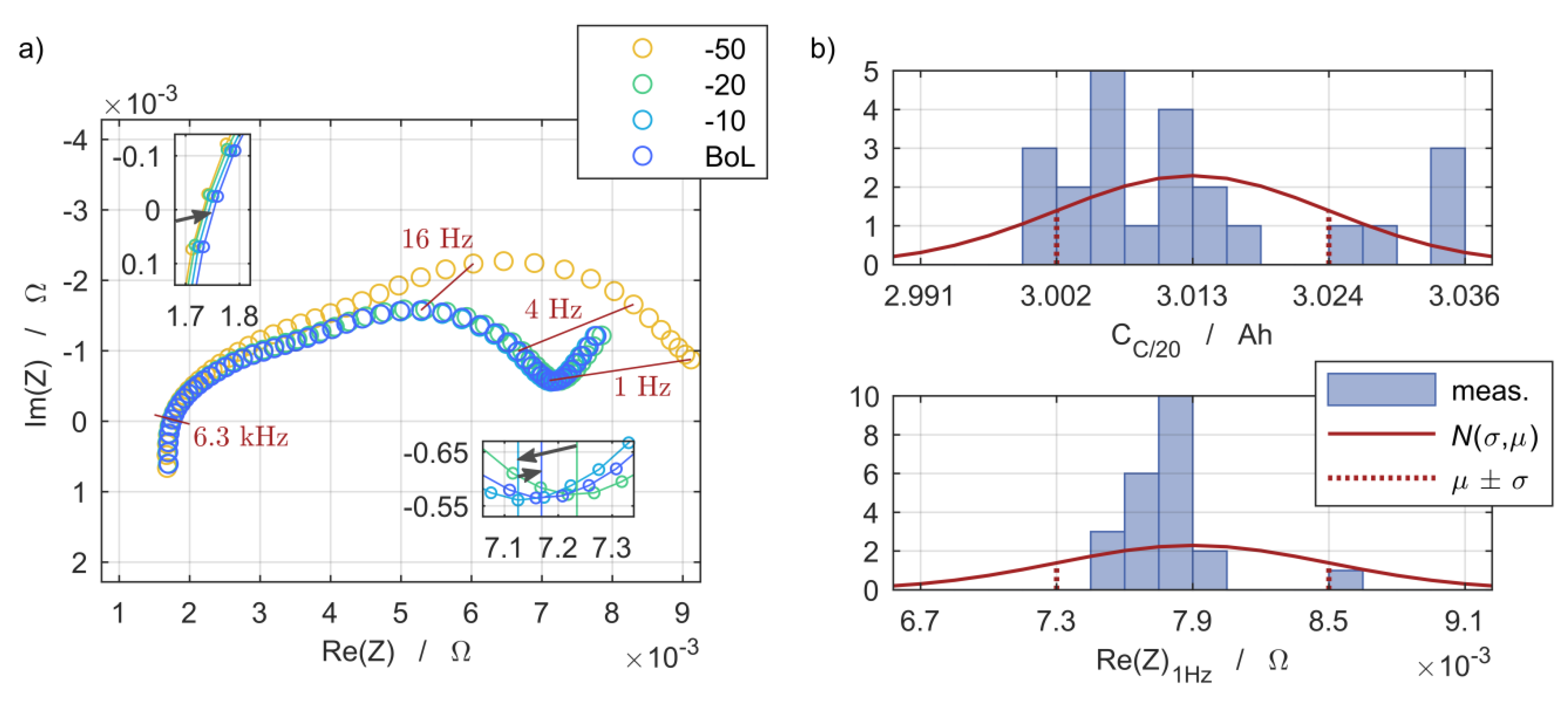

| Parameter | Value |
|---|---|
| Nominal capacity | 3.2 Ah |
| Typical capacity for applied charge and discharge protocol | 3.0 Ah |
| Nominal voltage | 3.7 V |
| Charge condition, continuous current | 6.4 A |
| Charge condition, voltage | 4.2 V |
| Discharge condition, continuous current | 96 A |
| Discharge condition, peak current | 160 A |
| Discharge condition, voltage | 2.7 V |
| Parameter | T0 | T1 | T2 |
|---|---|---|---|
| Temperature | 0 °C | 25 °C | 50 °C |
| Fluid | water + ethylene glycol 5% (v/v) | water | water |
| Fluid heat transfer coefficient homogeneous temperature control * | 6500 W m−² K−1 ≙ 26 W K−1 | ||
| Volume flow | 7.3 L min−1 | 3.6 L min−1 | 2.7 L min−1 |
| Fluid heat transfer coefficient inhomogeneous temperature control * | 5200 W m−² K−1 ≙ 19 W K−1 | ||
| Volume flow | 7.4 L min−1 | 3.7 L min−1 | 2.6 L min−1 |
| 500 Cycles | T0 ↔ T1 | T0 ↔ T2 | T1 ↔ T2 | |||
|---|---|---|---|---|---|---|
| T reference | 0 °C | 25 °C | 0 °C | 50 °C | 25 °C | 50 °C |
| Label acc. Figure 2 | T0 | T1 | T0 | T2 | T1 | T2 |
| Ø T | 1.2 °C | 25.2 °C | 1.1 °C | 49.2 °C | 25.2 °C | 49.1 °C |
| Ø ΔT | 0.03 K | 0.01 K | 0.02 K | 0.01 K | 0.01 K | 0.01 K |
| Setup Legend | Thermal Boundary Condition | Temperature Levels | (i) Design Complexity | (ii) Effort for Coolant System | (iii) Impact on Capacity Degradation |
|---|---|---|---|---|---|
| HS 0 °C * | Hom. Stat. | 0 °C | High | High | Very high |
| HT 0/50 °C* | Hom. Trans. | 0 °C ↔ 50 °C | High | High | High |
| IS 0/25 °C * | Inhom. Stat. | 0 °C/25 °C | Moderate | High | High |
| HT 50/25 °C | Hom. Trans. | 50 °C ↔ 25 °C | High | Low | High |
| HS 50 °C * | Hom. Stat. | 50 °C | High | Low | Moderate |
| IS 0/50 °C * | Inhom. Stat. | 0 °C/50 °C | Low | High | Moderate |
| IT 0/25 °C | Inhom. Trans. | (0 °C) **/25 °C | Moderate | High | Moderate |
| IT 50/25 °C | Inhom. Trans. | 50 °C/(25 °C) ** | Moderate | Low | Moderate |
| IT 50/0 °C | Inhom. Trans. | 50 °C/(0 °C) ** | Low | High | Moderate |
| HT 25/0 °C | Hom. Trans. | 25 °C ↔ 0 °C | High | High | Low |
| IS 50/25 °C * | Inhom. Stat. | 50 °C/25 °C | Moderate | Low | Low |
| HS 25 °C * | Hom. Stat. | 25 °C | High | Moderate | Low |
| 500 Cycles | T1 ↔ T2 | T0 ↔ T2 | T0 ↔ T1 | |||
|---|---|---|---|---|---|---|
| T reference | 37.5 °C | 50 °C | 25 °C | 50 °C | 12.5 °C | 25 °C |
| Label acc. Figure 2 | T1/T2 | T2 | T2/T0 | T2 | T1/T0 | T1 |
| Ø T | 37.5 °C | 43.1 °C | 25.7 °C | 39.2 °C | 14.3 °C | 21.9 °C |
| Ø ΔT | 0.01 K | 0.01 K | 0.19 K | 0.10 K | 0.02 K | 0.02 K |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Werner, D.; Paarmann, S.; Wiebelt, A.; Wetzel, T. Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part I: Experimental Investigation. Batteries 2020, 6, 13. https://doi.org/10.3390/batteries6010013
Werner D, Paarmann S, Wiebelt A, Wetzel T. Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part I: Experimental Investigation. Batteries. 2020; 6(1):13. https://doi.org/10.3390/batteries6010013
Chicago/Turabian StyleWerner, Daniel, Sabine Paarmann, Achim Wiebelt, and Thomas Wetzel. 2020. "Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part I: Experimental Investigation" Batteries 6, no. 1: 13. https://doi.org/10.3390/batteries6010013
APA StyleWerner, D., Paarmann, S., Wiebelt, A., & Wetzel, T. (2020). Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part I: Experimental Investigation. Batteries, 6(1), 13. https://doi.org/10.3390/batteries6010013






