Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part II: Analysis and Correlation
Abstract
1. Introduction
- Evaluation of the electrochemical impedance spectra and the individual contributions to the overall impedance for complex thermal boundary conditions.
- Introduction of an equivalent aging temperature (EAT) as a characteristic value, unifying most of the investigated complex thermal boundary conditions to a single temperature value.
- Development of aging correlations for different evaluated impedance quantities, relating them to the EAT.
- Identification of different aging mechanisms at low and high temperatures.
2. Experimental Setup
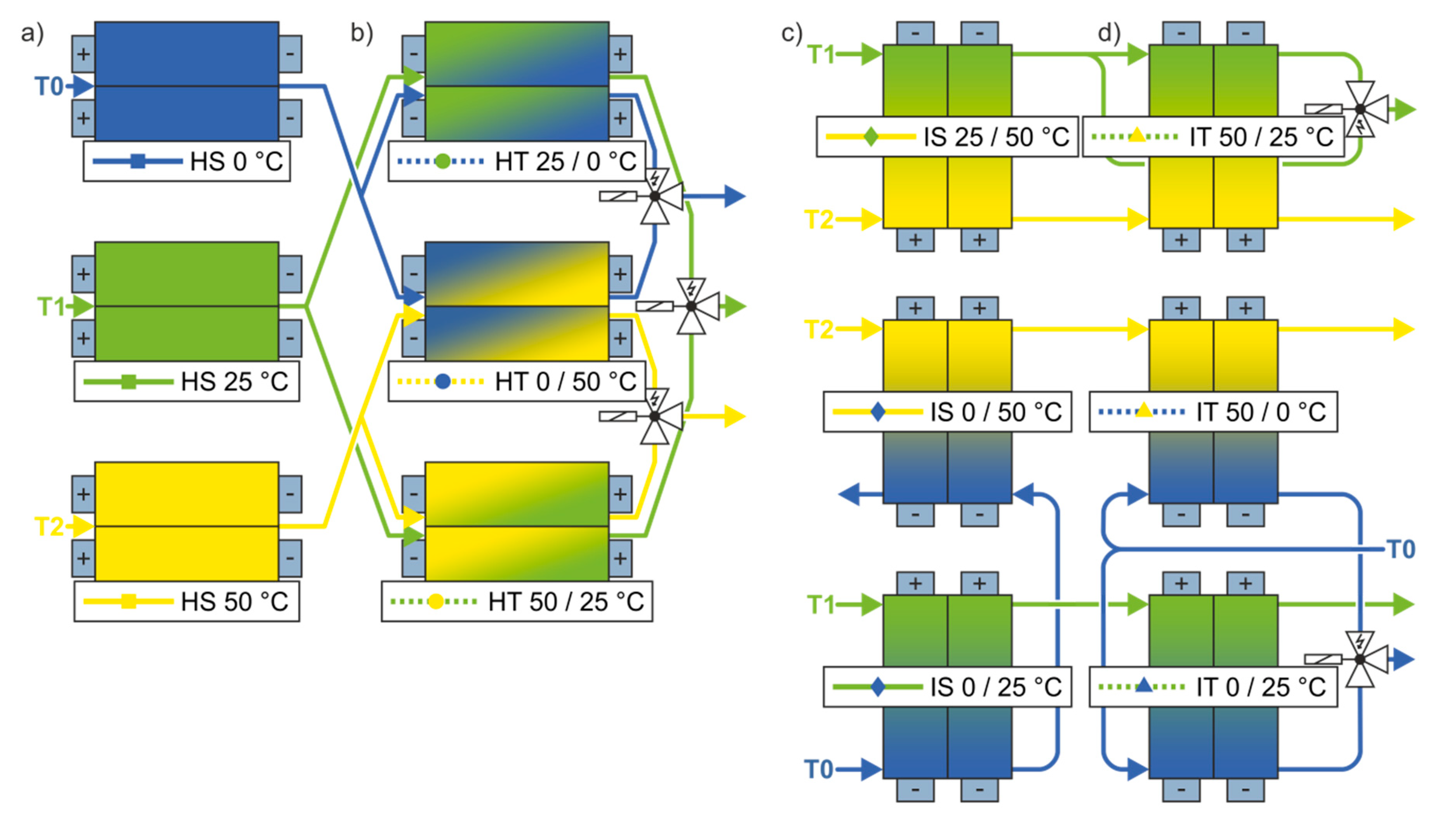
2.1. Boundary Condition and Testing Procedure
2.2. Evaluation Procedure
3. Results and Discussion
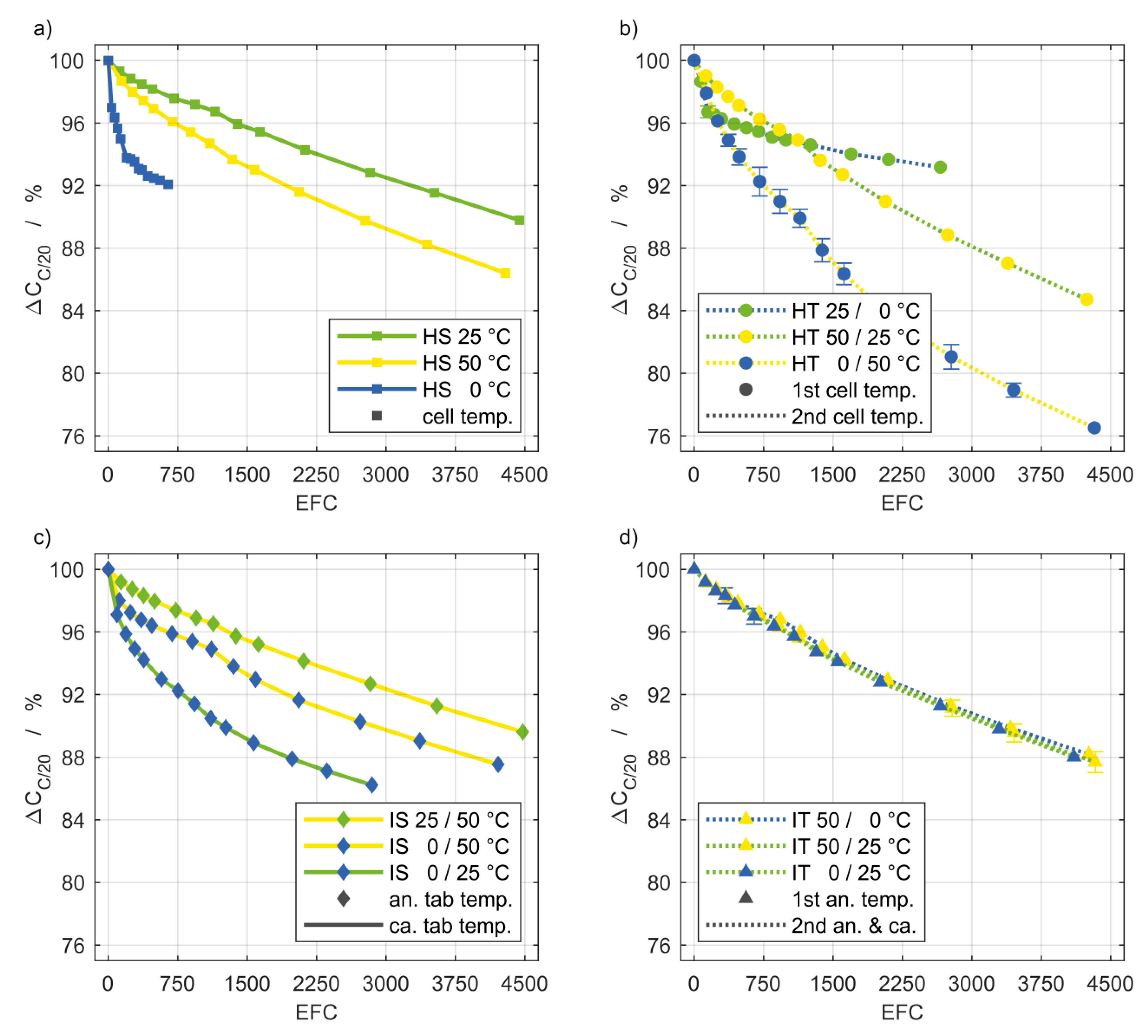
3.1. Capacity Measurements
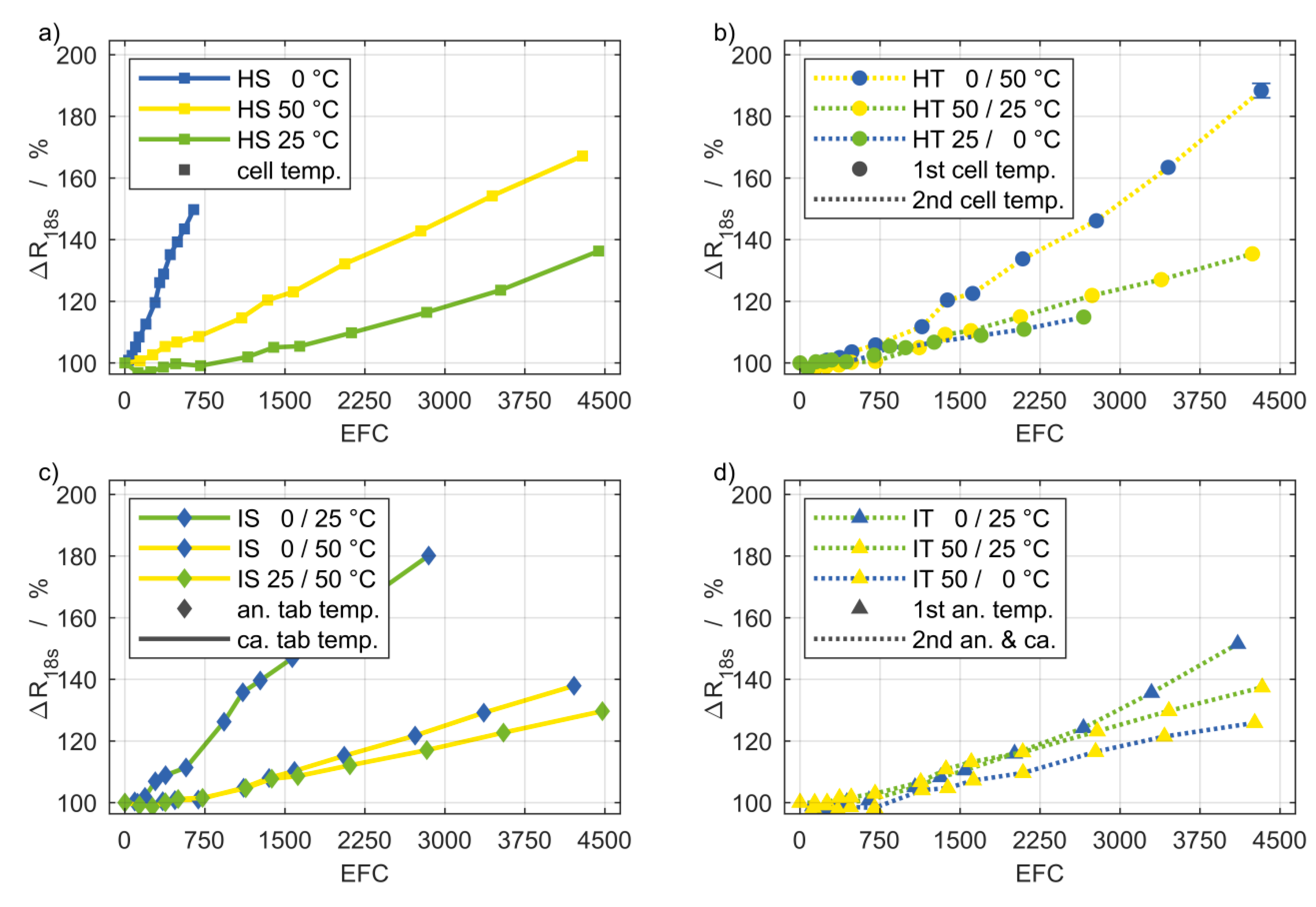
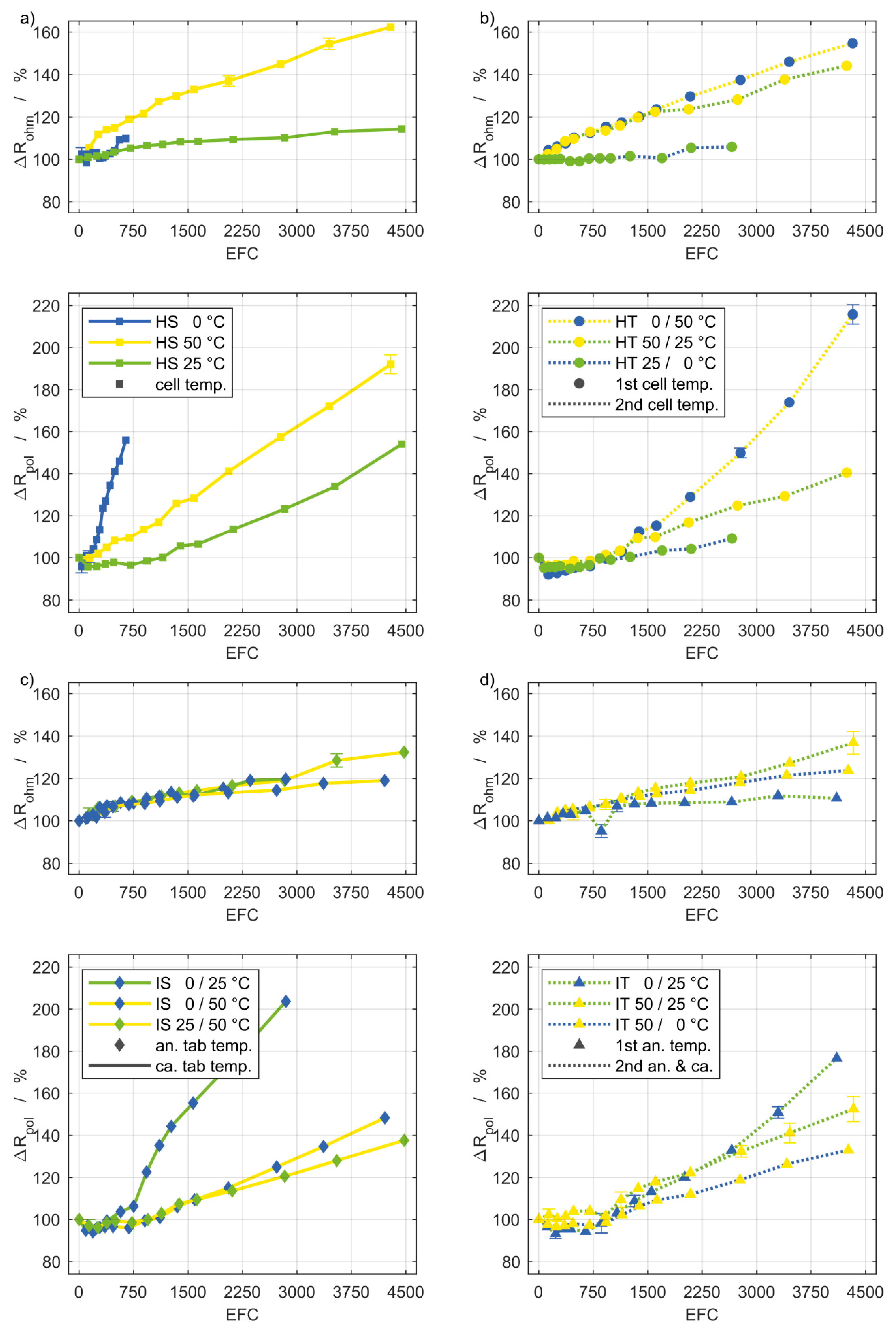
3.2. Impedance Measurements
3.3. Impedance Versus Capacity Degradation
3.4. Equivalent Aging Temperature
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Averaging and Reproducibility
| Setup | ∑NEFC | ∑σEFC |
|---|---|---|
| HS 0 °C | 645.2 | 5.9 |
| HS 25 °C | 4441.5 | 7.6 |
| HS 50 °C | 4289.5 | 16.1 |
| HT 25/0 °C | 2659.6 | 4.3 |
| HT 0/50 °C | 4323.6 | 13.8 |
| HT 50/25 °C | 4240.9 | 8.5 |
| IS 25/50 °C | 4476.5 | 11.6 |
| IS 0/50 °C | 4210.8 | 26.9 |
| IS 0/25 °C | 2847.8 | - |
| IT 50/0 °C | 4262.3 | 6.8 |
| IT 50/25 °C | 4333.1 | 6.6 |
| IT 0/25 °C | 4103.0 | 11.3 |
References
- Werner, D.; Paarmann, S.; Wiebelt, A.; Wetzel, T. Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-ion Cells. Part I: Experimental Investigation. Batteries 2020, 6, 13. [Google Scholar] [CrossRef]
- Baghdadi, I.; Briat, O.; Delétage, J.-Y.; Gyan, P.; Vinassa, J.-M. Lithium battery aging model based on Dakin’s degradation approach. J. Power Sources 2016, 325, 273–285. [Google Scholar] [CrossRef]
- Waldmann, T.; Wilka, M.; Kasper, M.; Fleischhammer, M.; Wohlfahrt-Mehrens, M. Temperature dependent ageing mechanisms in Lithium-ion batteries—A Post-Mortem study. J. Power Sources 2014, 262, 129–135. [Google Scholar] [CrossRef]
- Schuster, S.F.; Bach, T.; Fleder, E.; Müller, J.; Brand, M.; Sextl, G.; Jossen, A. Nonlinear aging characteristics of lithium-ion cells under different operational conditions. J. Energy Storage 2015, 1, 44–53. [Google Scholar] [CrossRef]
- Käbitz, S.; Gerschler, J.B.; Ecker, M.; Yurdagel, Y.; Emmermacher, B.; André, D.; Mitsch, T.; Sauer, D.U. Cycle and calendar life study of a graphite|LiNi1/3Mn1/3Co1/3O2 Li-ion high energy system. Part A: Full cell characterization. J. Power Sources 2013, 239, 572–583. [Google Scholar] [CrossRef]
- Jalkanen, K.; Karppinen, J.; Skogström, L.; Laurila, T.; Nisula, M.; Vuorilehto, K. Cycle aging of commercial NMC/graphite pouch cells at different temperatures. Appl. Energy 2015, 154, 160–172. [Google Scholar] [CrossRef]
- Gering, K.L.; Sazhin, S.V.; Jamison, D.K.; Michelbacher, C.J.; Liaw, B.Y.; Dubarry, M.; Cugnet, M. Investigation of path dependence in commercial lithium-ion cells chosen for plug-in hybrid vehicle duty cycle protocols. J. Power Sources 2011, 196, 3395–3403. [Google Scholar] [CrossRef]
- Hunt, I.A.; Zhao, Y.; Patel, Y.; Offer, J. Surface Cooling Causes Accelerated Degradation Compared to Tab Cooling for Lithium-Ion Pouch Cells. J. Electrochem. Soc. 2016, 163, A1846–A1852. [Google Scholar] [CrossRef]
- Carter, R.; Love, C.T. Modulation of Lithium Plating in Li-Ion Batteries with External Thermal Gradient. ACS Appl. Mater. Interfaces 2018, 10, 26328–26334. [Google Scholar] [CrossRef]
- Jaguemont, J.; Boulon, L.; Venet, P.; Dube, Y.; Sari, A. Lithium-Ion Battery Aging Experiments at Subzero Temperatures and Model Development for Capacity Fade Estimation. IEEE Trans. Veh. Technol. 2016, 65, 4328–4343. [Google Scholar] [CrossRef]
- International Organization for Standardization (ISO). Electrically Propelled Road Vehicles—Test Specification for Lithium-ion Traction Battery Packs and Systems—Part 1: High-Power Applications; International Organization for Standardization: Geneva, Switzerland, 2011. [Google Scholar]
- Jacobsen, T.; West, K. Diffusion impedance in planar, cylindrical and spherical symmetry. Electrochim. Acta 1995, 40, 255–262. [Google Scholar] [CrossRef]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy, 2nd ed.; John Wiley Sons, Inc.: Hoboken, NJ, USA, 2005; ISBN 9780471716242. [Google Scholar]
- Gantenbein, S.; Weiss, M.; Ivers-Tiffée, E. Impedance based time-domain modeling of lithium-ion batteries: Part I. J. Power Sources 2018, 379, 317–327. [Google Scholar] [CrossRef]
- Liu, W.; Delacourt, C.; Forgez, C.; Pelissier, S. Study of graphite/NCA Li-ion cell degradation during accelerated aging tests—Data analysis of the SIMSTOCK project. In Proceedings of the 2011 IEEE Vehicle Power and Propulsion Conference, Chicago, IL, USA, 6–9 September 2011; pp. 1–6. [Google Scholar]
- Spotnitz, R. Simulation of capacity fade in lithium-ion batteries. J. Power Sources 2003, 113, 72–80. [Google Scholar] [CrossRef]
- Sarasketa-Zabala, E.; Gandiaga, I.; Rodriguez-Martinez, L.M.; Villarreal, I. Calendar ageing analysis of a LiFePO4/graphite cell with dynamic model validations: Towards realistic lifetime predictions. J. Power Sources 2014, 272, 45–57. [Google Scholar] [CrossRef]
- Viswanathan, V.V.V.; Choi, D.; Wang, D.; Xu, W.; Towne, S.; Williford, R.E.; Zhang, J.-G.; Liu, J.; Yang, Z. Effect of entropy change of lithium intercalation in cathodes and anodes on Li-ion battery thermal management. J. Power Sources 2010, 195, 3720–3729. [Google Scholar] [CrossRef]
- Park, M.; Zhang, X.; Chung, M.; Less, G.B.; Sastry, A.M. A review of conduction phenomena in Li-ion batteries. J. Power Sources 2010, 195, 7904–7929. [Google Scholar] [CrossRef]
- Loges, A.; Herberger, S.; Werner, D.; Wetzel, T. Thermal characterization of Li-ion cell electrodes by photothermal deflection spectroscopy. J. Power Sources 2016, 325, 104–115. [Google Scholar] [CrossRef]
- Loges, A.; Herberger, S.; Seegert, P.; Wetzel, T. A study on specific heat capacities of Li-ion cell components and their influence on thermal management. J. Power Sources 2016, 336, 341–350. [Google Scholar] [CrossRef]
- Werner, D.; Loges, A.; Becker, D.J.; Wetzel, T. Thermal conductivity of Li-ion batteries and their electrode configurations—A novel combination of modelling and experimental approach. J. Power Sources 2017, 364, 72–83. [Google Scholar] [CrossRef]
- Wang, J.; Purewal, J.; Liu, P.; Hicks-Garner, J.; Soukazian, S.; Sherman, E.; Sorenson, A.; Vu, L.; Tataria, H.; Verbrugge, M.W. Degradation of lithium ion batteries employing graphite negatives and nickel-cobalt-manganese oxide + spinel manganese oxide positives: Part 1, aging mechanisms and life estimation. J. Power Sources 2014, 269, 937–948. [Google Scholar] [CrossRef]
- Purewal, J.; Wang, J.; Graetz, J.; Soukiazian, S.; Tataria, H.; Verbrugge, M.W. Degradation of lithium ion batteries employing graphite negatives and nickel-cobalt-manganese oxide + spinel manganese oxide positives: Part 2, chemical-mechanical degradation model. J. Power Sources 2014, 272, 1154–1161. [Google Scholar] [CrossRef]
- Ecker, M.; Tran, T.K.D.; Dechent, P.; Käbitz, S.; Warnecke, A.; Sauer, D.U. Parameterization of a Physico-Chemical Model of a Lithium-Ion Battery I. Determination of Parameters. J. Electrochem. Soc. 2015, 162, A1836–A1848. [Google Scholar] [CrossRef]
- Ecker, M.; Käbitz, S.; Laresgoiti, I.; Sauer, D.U. Parameterization of a Physico-Chemical Model of a Lithium-Ion Battery II. Model Validation. J. Electrochem. Soc. 2015, 162, A1849–A1857. [Google Scholar] [CrossRef]
- Von Srbik, M.-T.; Marinescu, M.; Martinez-Botas, R.F.; Offer, G.J. A physically meaningful equivalent circuit network model of a lithium-ion battery accounting for local electrochemical and thermal behaviour, variable double layer capacitance and degradation. J. Power Sources 2016, 325, 171–184. [Google Scholar] [CrossRef]
- Arrhenius, S. Über die Reaktionsgeschwindigkeit bei der Inversion von Rohrzucker durch Säuren. Z. Phys. Chem. 1889, 4, 226–248. [Google Scholar] [CrossRef]
- Schmalstieg, J.; Käbitz, S.; Ecker, M.; Sauer, D.U. A holistic aging model for Li(NiMnCo)O2 based 18650 lithium-ion batteries. J. Power Sources 2014, 257, 325–334. [Google Scholar] [CrossRef]
- Liaw, B.Y.; Roth, E.P.; Jungst, R.G.; Nagasubramanian, G.; Case, H.L.; Doughty, D.H. Correlation of Arrhenius behaviors in power and capacity fades with cell impedance and heat generation in cylindrical lithium-ion cells. J. Power Sources 2003, 119–121, 874–886. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, C.-Y. Cycle-Life Characterization of Automotive Lithium-Ion Batteries with LiNiO2 Cathode. J. Electrochem. Soc. 2009, 156, A527. [Google Scholar] [CrossRef]

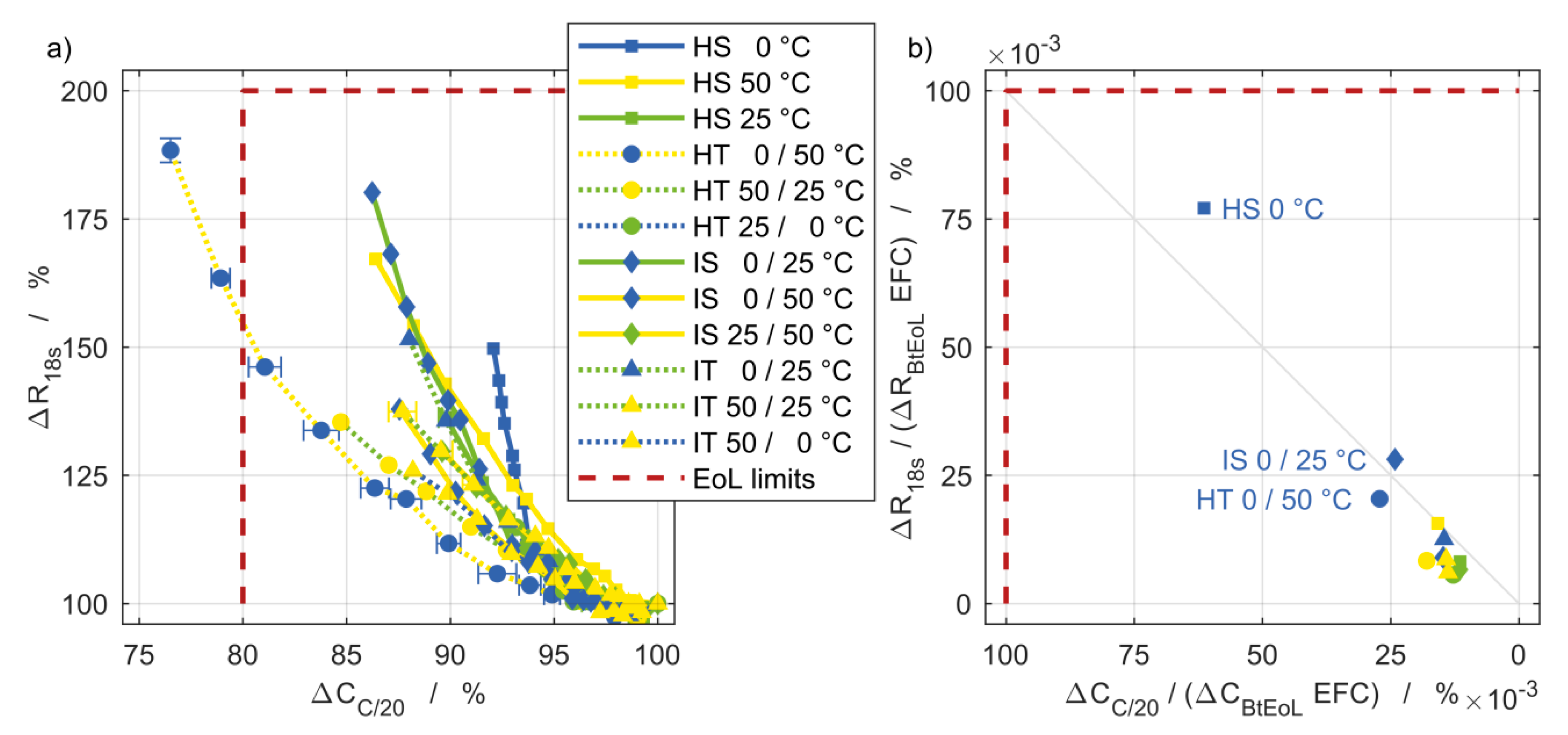
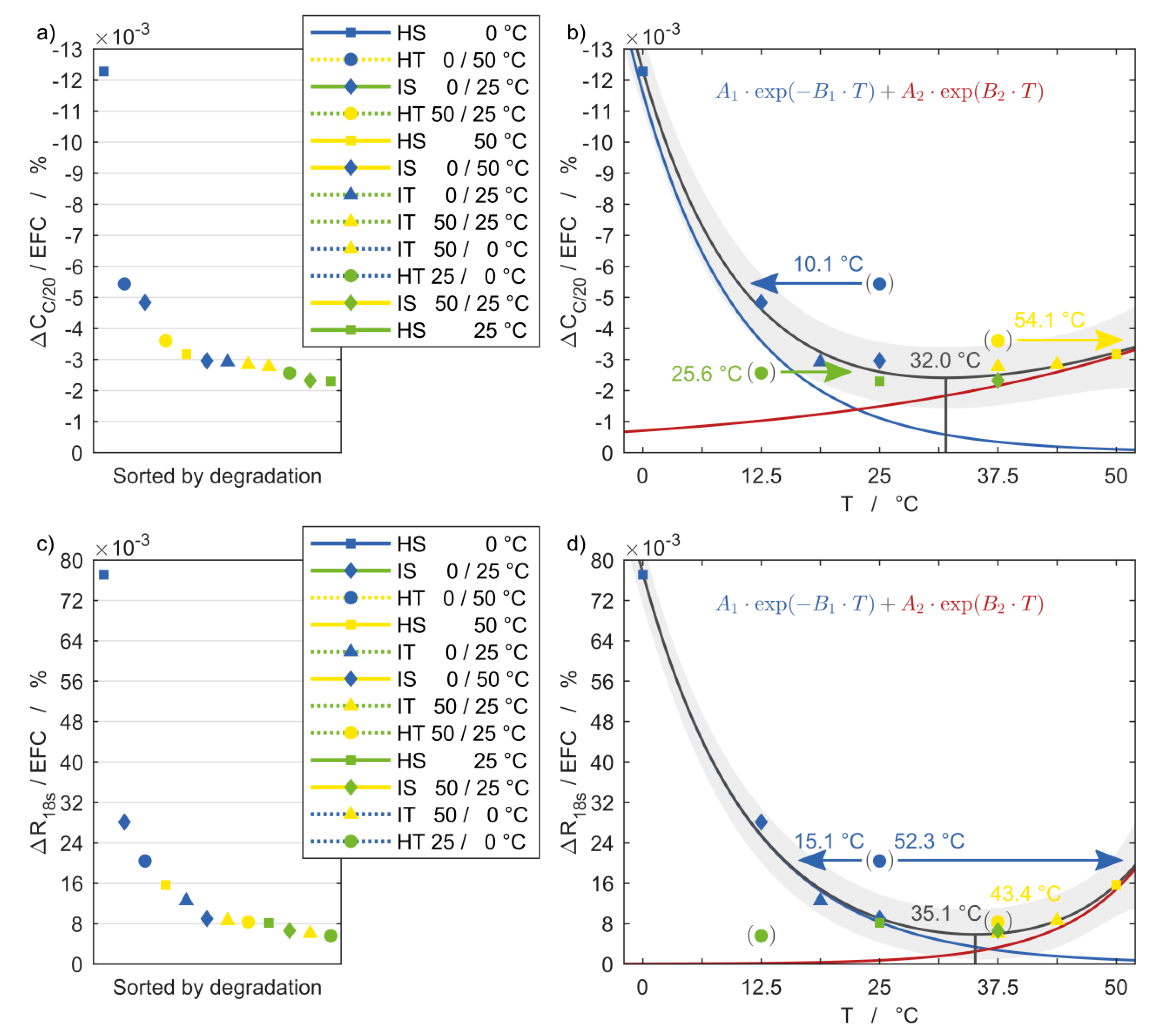
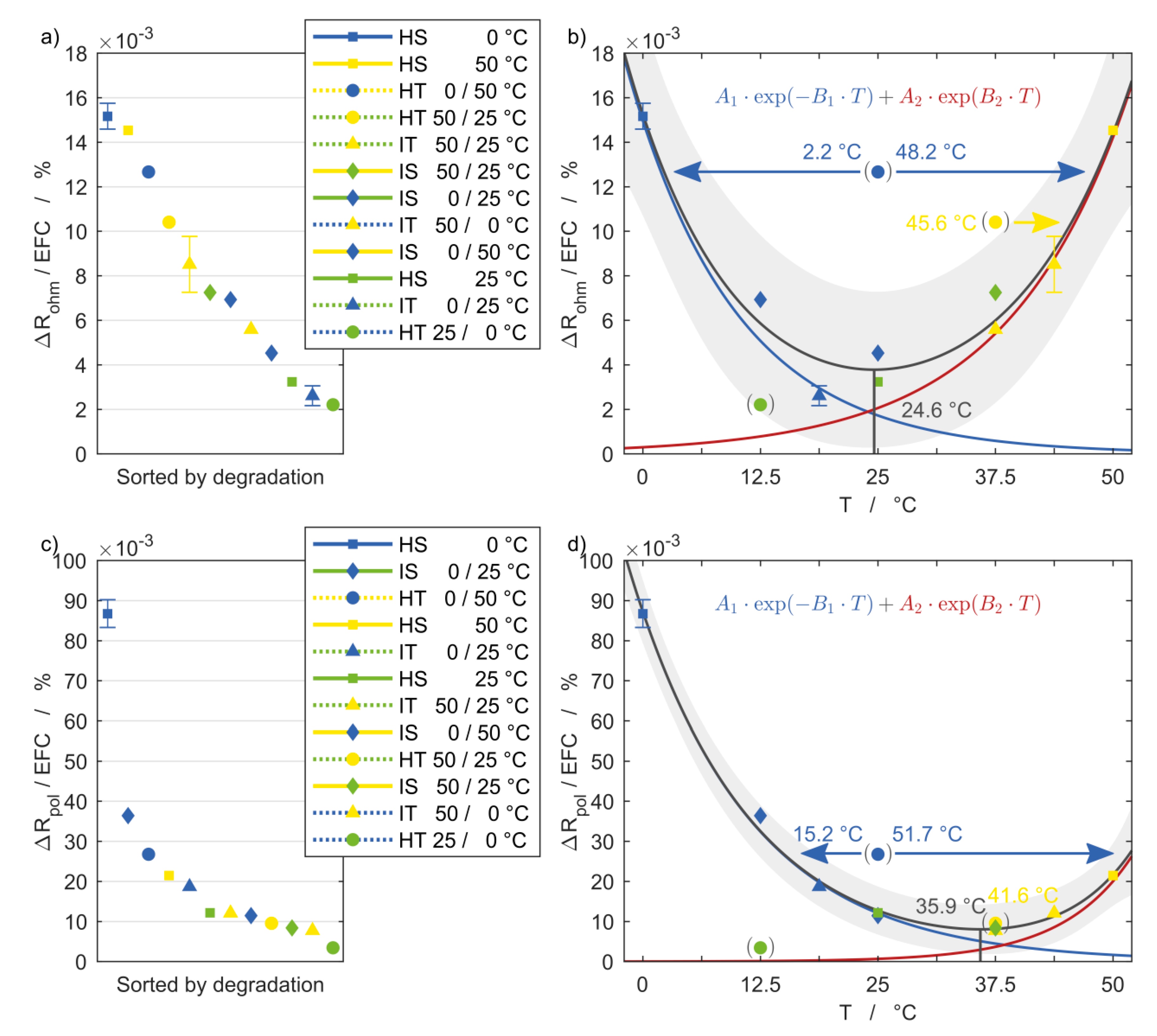
| Parameter | Value |
|---|---|
| CC/20 | (3.013 ± 0.011) Ah |
| R18s | (12.98 ± 0.028) mΩ |
| Rohm | (1.552 ± 0.080) mΩ |
| Rpol | (6.269 ± 0.148) mΩ |
| LT | HT | Fit | ||||
|---|---|---|---|---|---|---|
| Quantity | A1/- | EA1/eV | A2/- | EA2/eV | LSS/(%)2 | RSQ |
| ΔCC/20 | 6.515 10−13 | 0.6102 | 820.6 | 0.2845 | 0.4897 | 0.9927 |
| ΔR18s | 21.52 10−13 | 0.6262 | 9.099 ∙ 108 | 0.6339 | 34.67 | 0.9877 |
| ΔRohm | 12.08 10−13 | 0.6014 | 9.206 ∙ 108 | 0.6298 | 7.107 | 0.8658 |
| ΔRpol | 126.9 10−13 | 0.5894 | 9.973 ∙ 108 | 0.6293 | 135.7 | 0.9795 |
| LT | HT | Fit | ||||
|---|---|---|---|---|---|---|
| Quantity | A1/- | B1/°C−1 | A2/- | B2/°C−1 | LSS/(%)2 | RSQ |
| ΔCC/20 | 0.1159 | 0.09366 | 71.05 10−4 | 0.02962 | 0.4752 | 0.9929 |
| ΔR18s | 0.7735 | 0.08918 | 3.712 10−4 | 0.1198 | 13.44 | 0.9963 |
| ΔRohm | 0.1496 | 0.08642 | 29.86 10−4 | 0.07726 | 6.735 | 0.9518 |
| ΔRpol | 0.8720 | 0.07913 | 2.279 10−4 | 0.1356 | 19.89 | 0.9953 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Werner, D.; Paarmann, S.; Wiebelt, A.; Wetzel, T. Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part II: Analysis and Correlation. Batteries 2020, 6, 12. https://doi.org/10.3390/batteries6010012
Werner D, Paarmann S, Wiebelt A, Wetzel T. Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part II: Analysis and Correlation. Batteries. 2020; 6(1):12. https://doi.org/10.3390/batteries6010012
Chicago/Turabian StyleWerner, Daniel, Sabine Paarmann, Achim Wiebelt, and Thomas Wetzel. 2020. "Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part II: Analysis and Correlation" Batteries 6, no. 1: 12. https://doi.org/10.3390/batteries6010012
APA StyleWerner, D., Paarmann, S., Wiebelt, A., & Wetzel, T. (2020). Inhomogeneous Temperature Distribution Affecting the Cyclic Aging of Li-Ion Cells. Part II: Analysis and Correlation. Batteries, 6(1), 12. https://doi.org/10.3390/batteries6010012






