Enhancing Battery Pack Cooling Efficiency Through Graphite-Integrated Hybrid-Battery Thermal Management Systems
Abstract
1. Introduction
2. Problem Description
3. Governing Equations
3.1. Porous Domain
3.2. Battery
3.3. Equivalent Circuit Model (ECM)
3.4. Assumptions
- All batteries are identical and have the same initial state of charge at the start of the simulations.
- The porous media between batteries is assumed to be homogeneous.
- The boundary condition in outlets is ‘pressure outlet’ and in inlets is ‘velocity inlet’.
- Natural convection is considered on the battery box, and the heat transfer coefficient is 5 W/m·K.
4. Grid Study
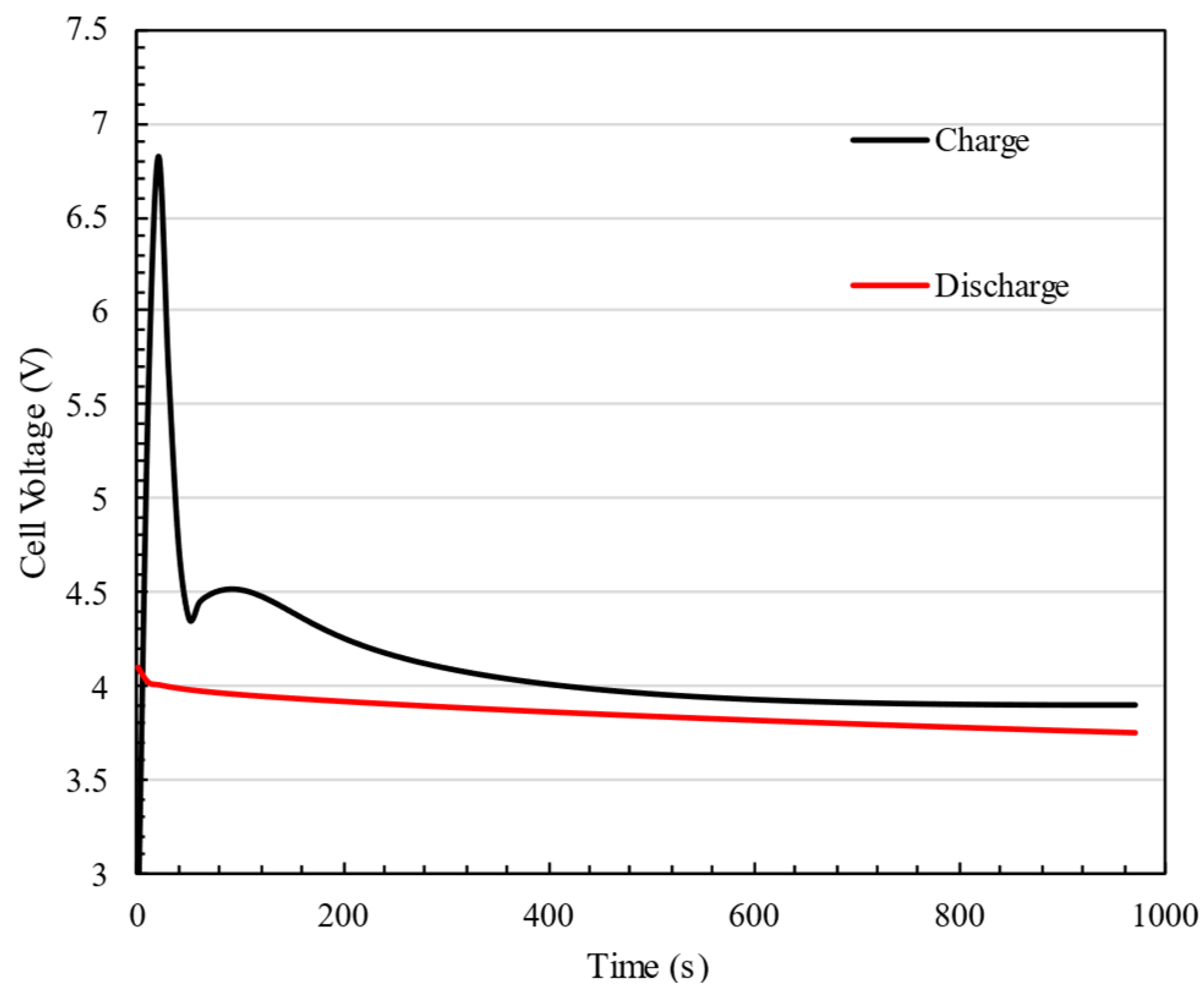
5. Validation
6. Results
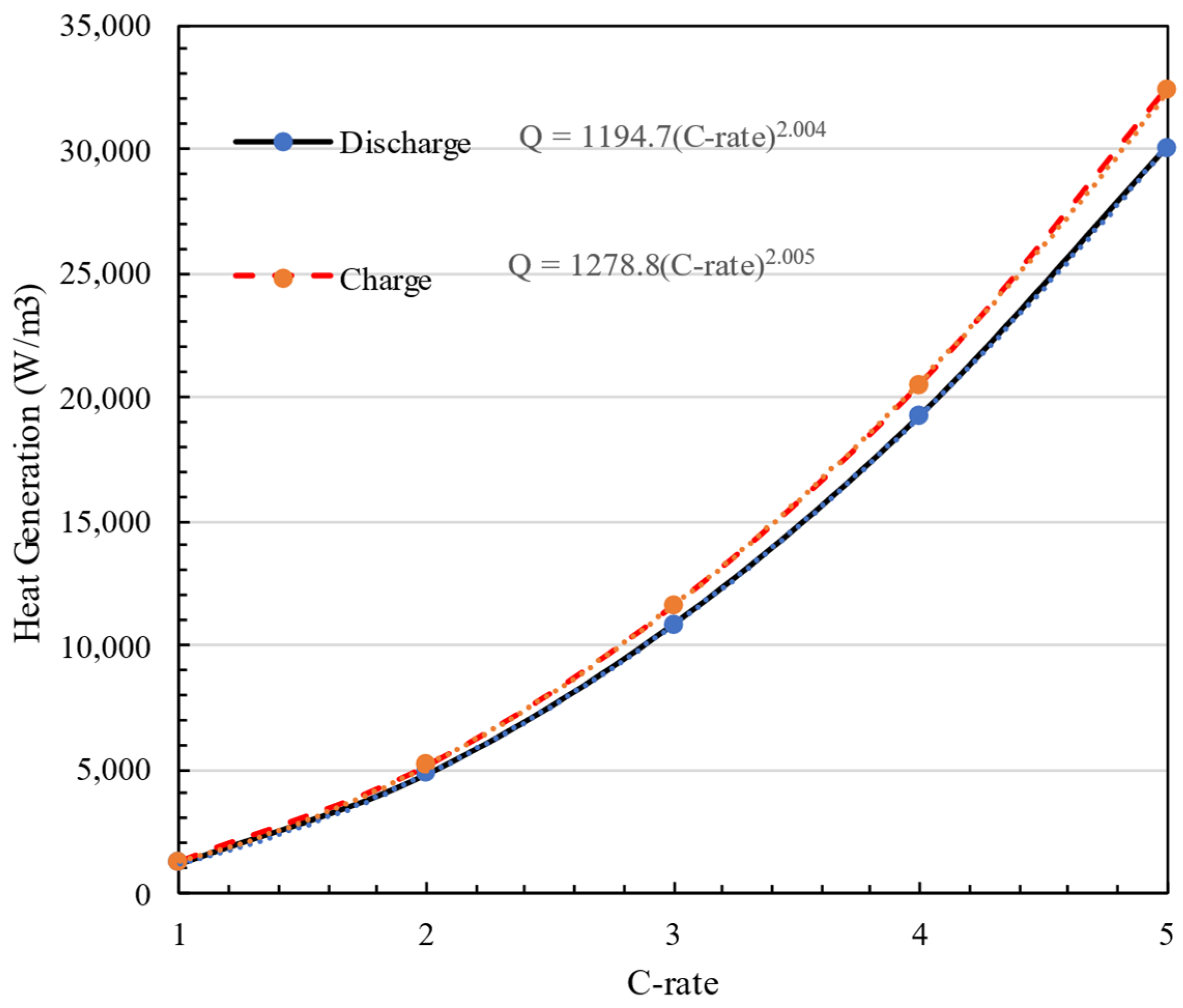
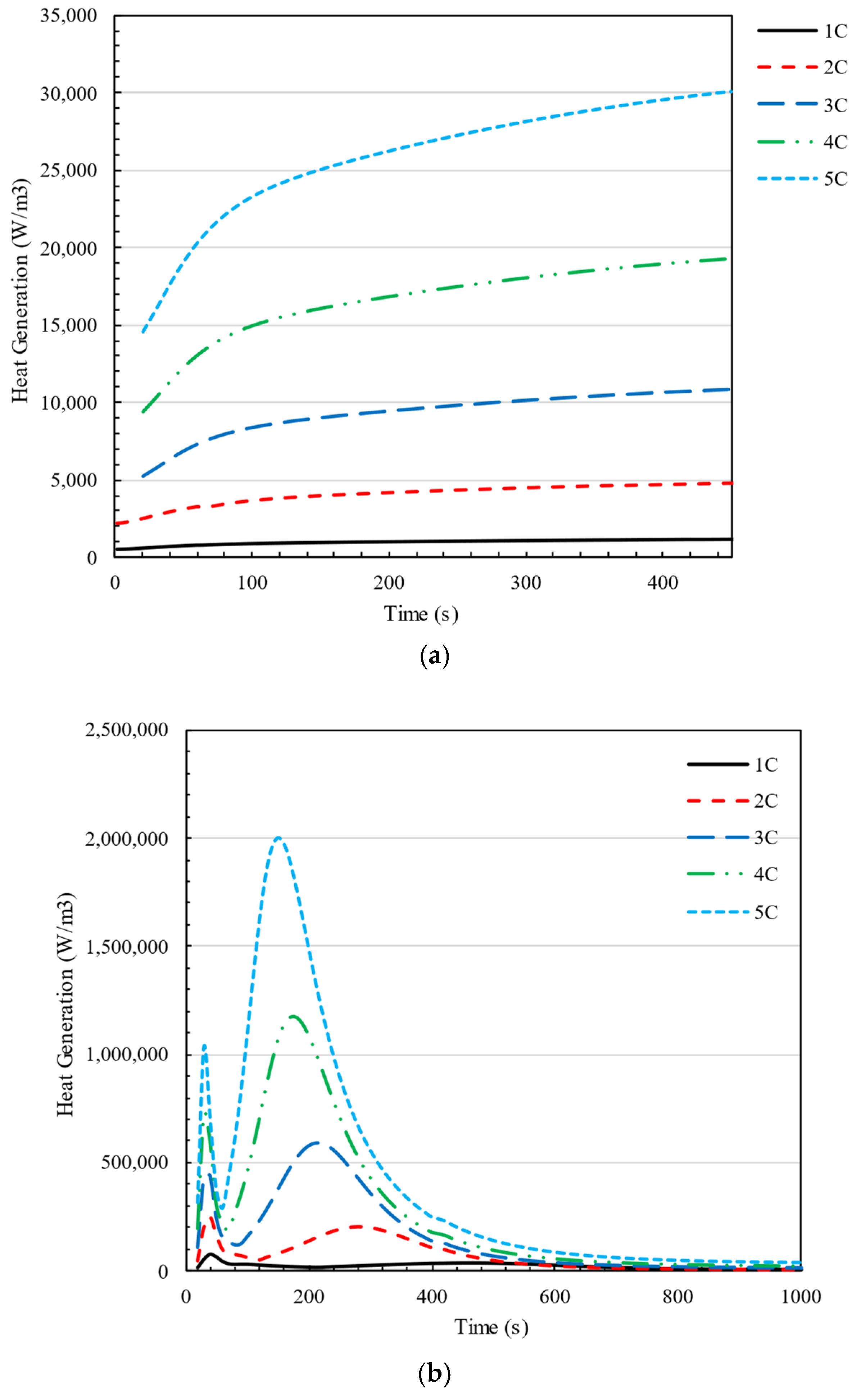
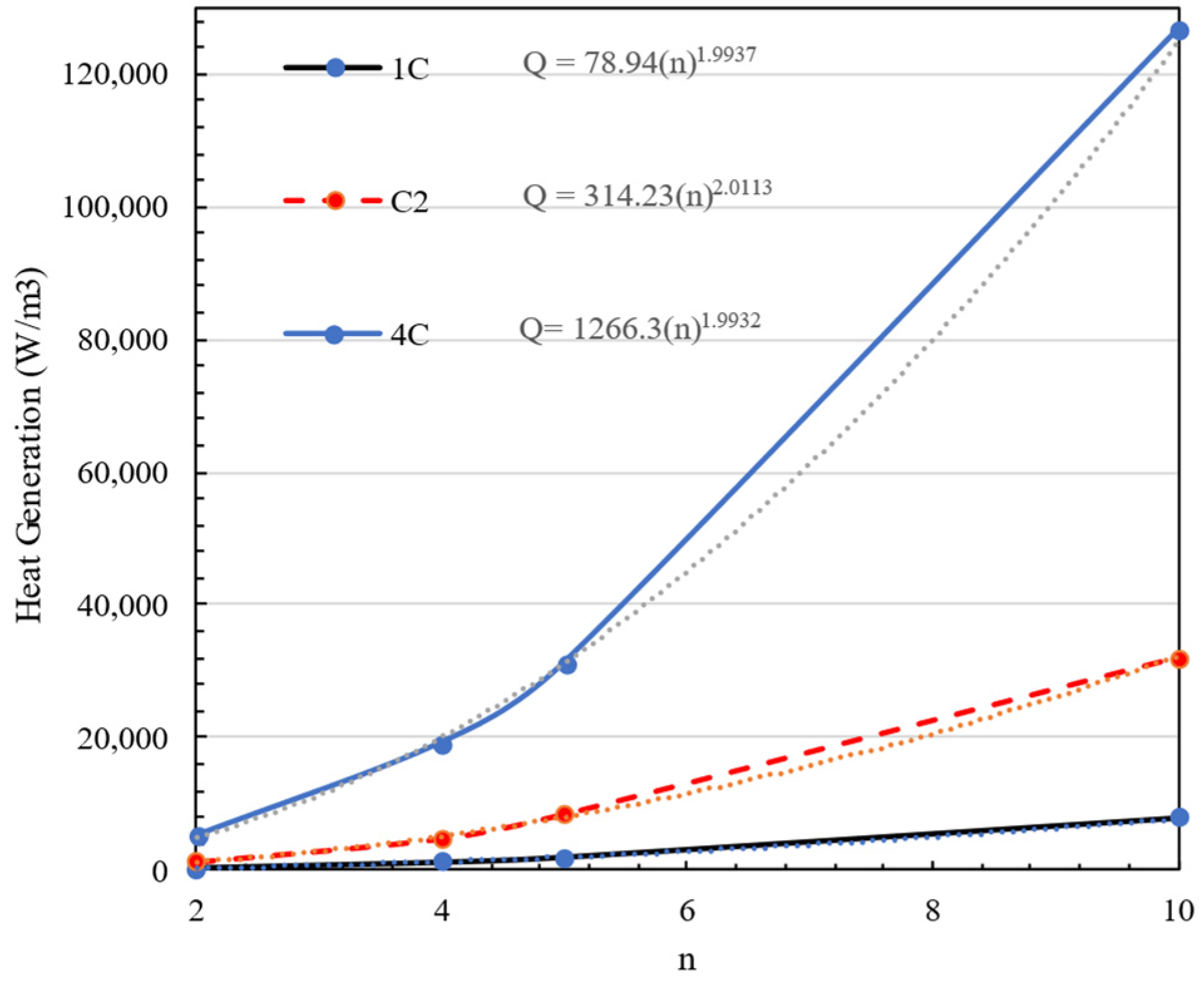
6.1. Heat Generation in Battery Pack
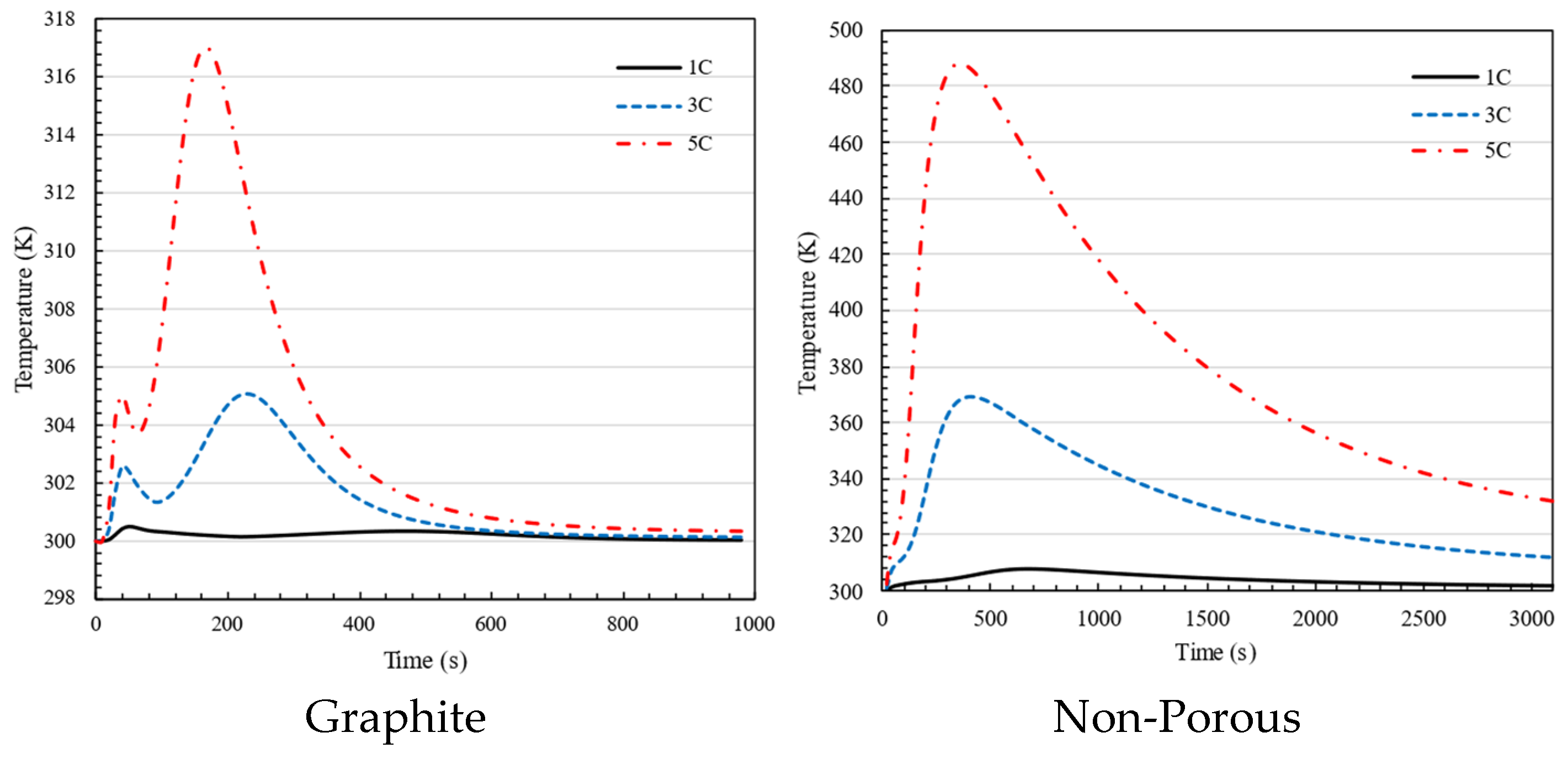
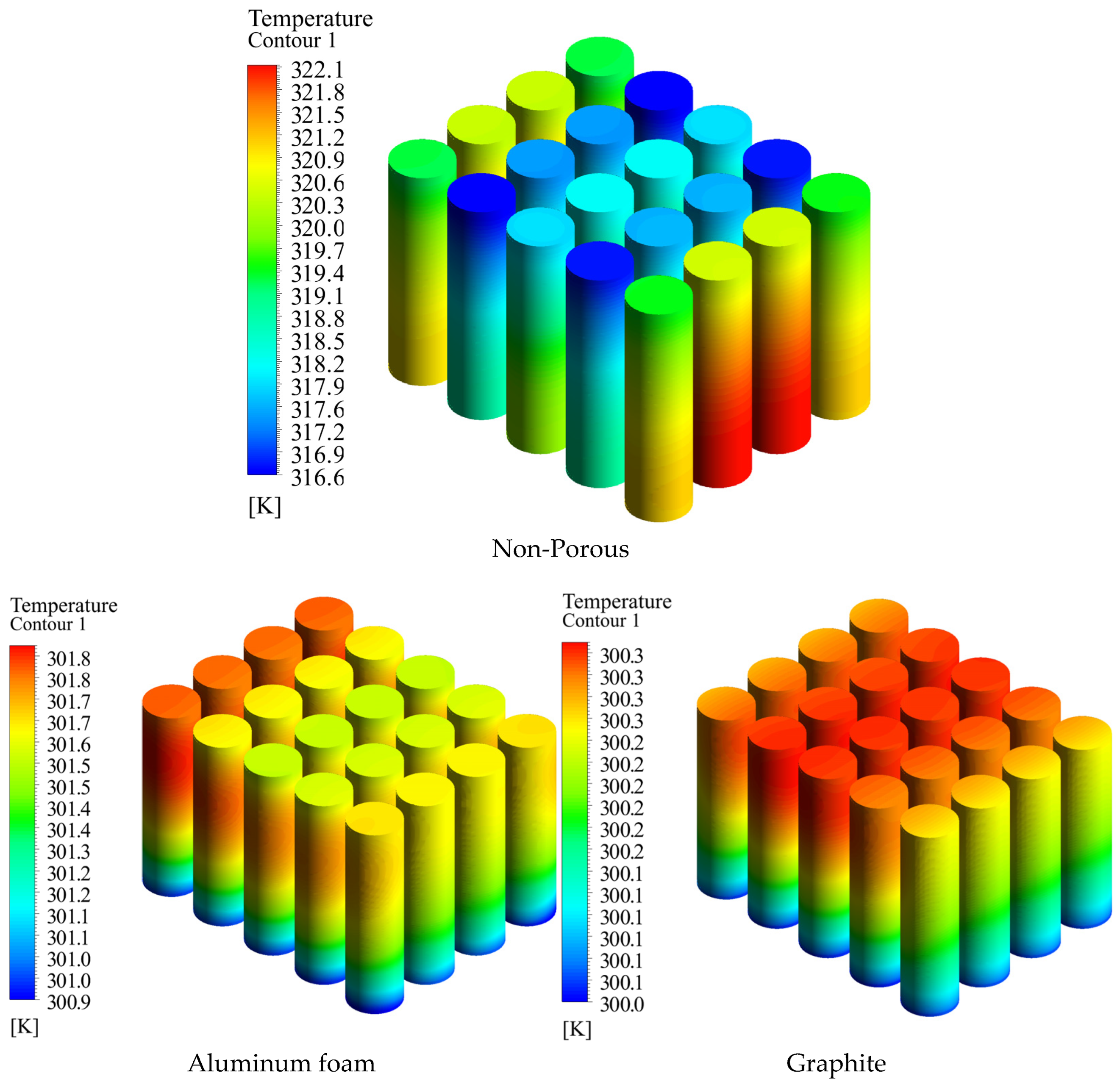
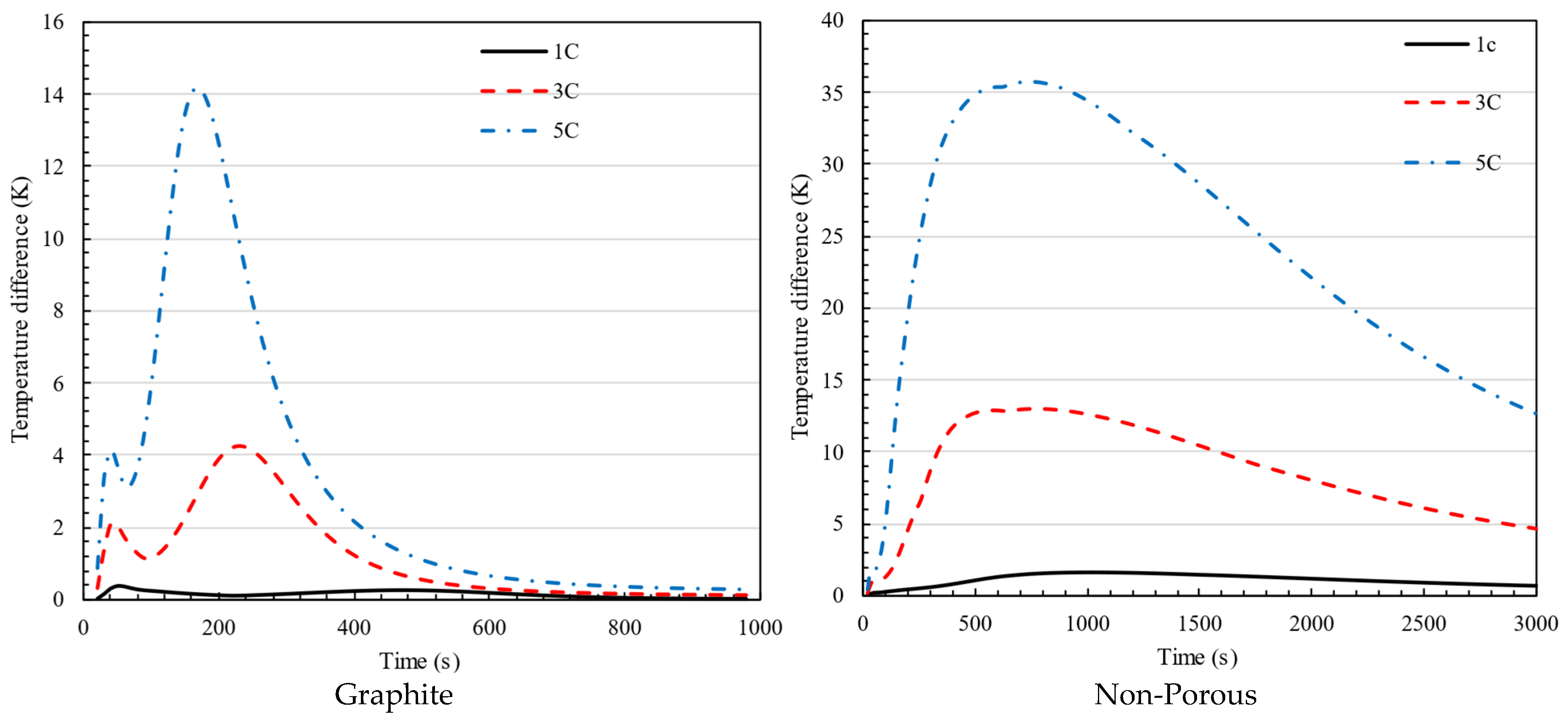
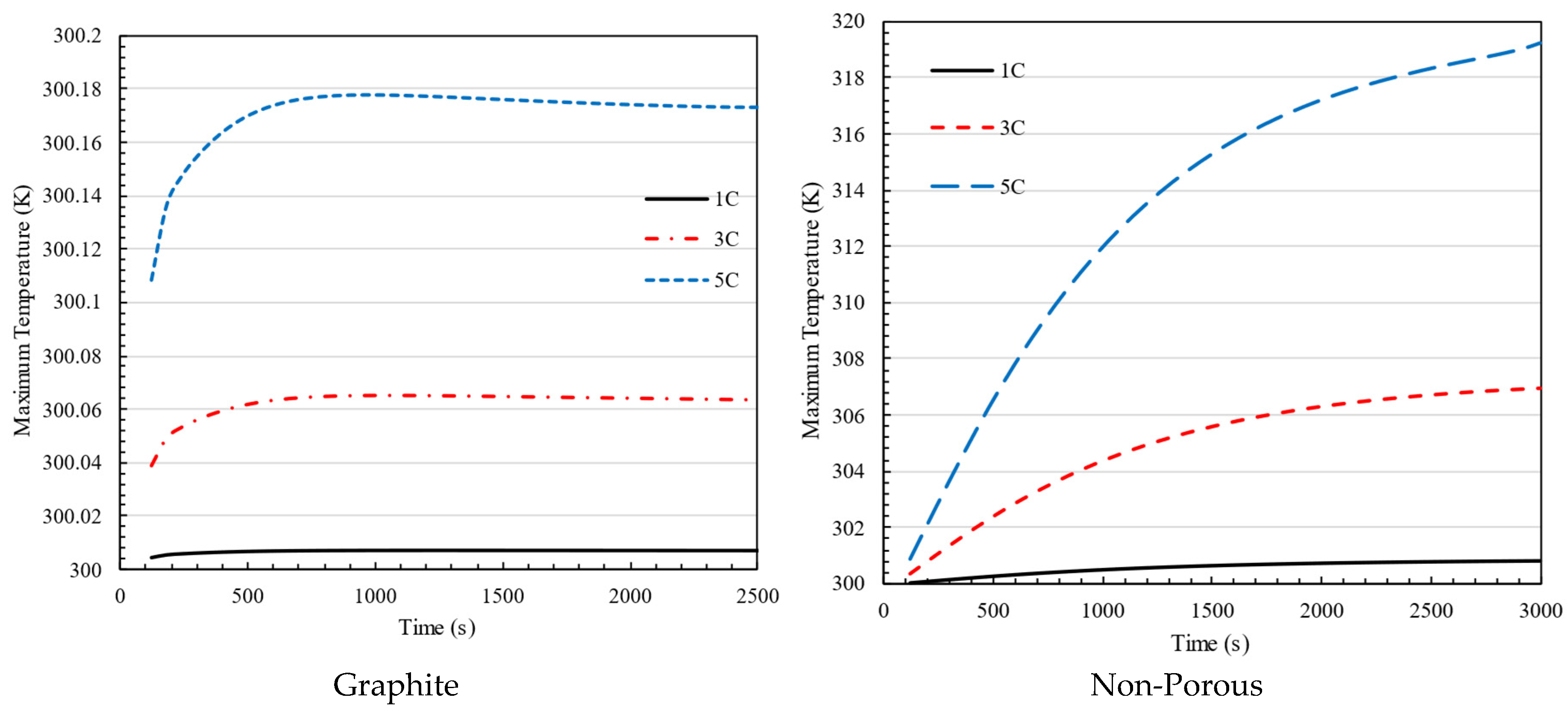
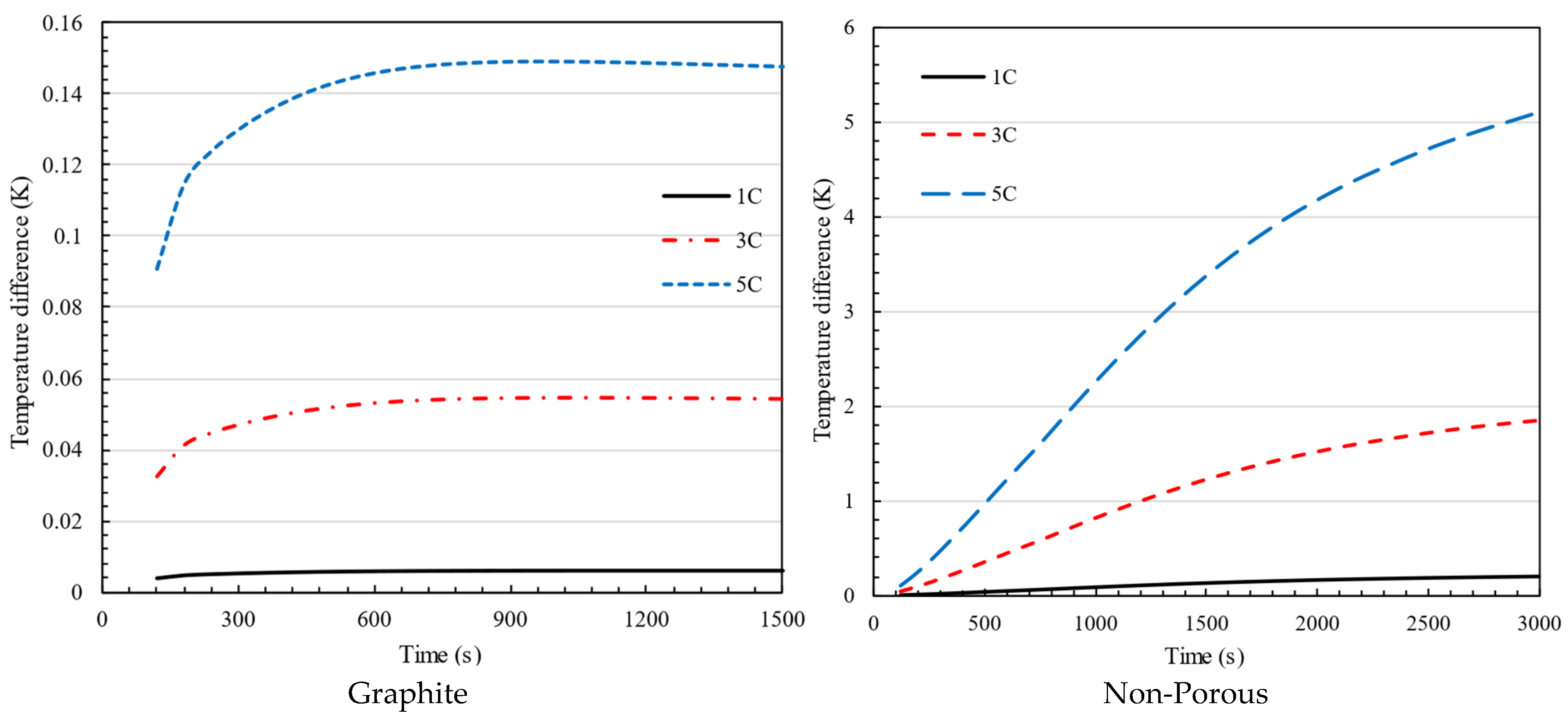
6.2. Effectiveness of Incorporating Porous Materials
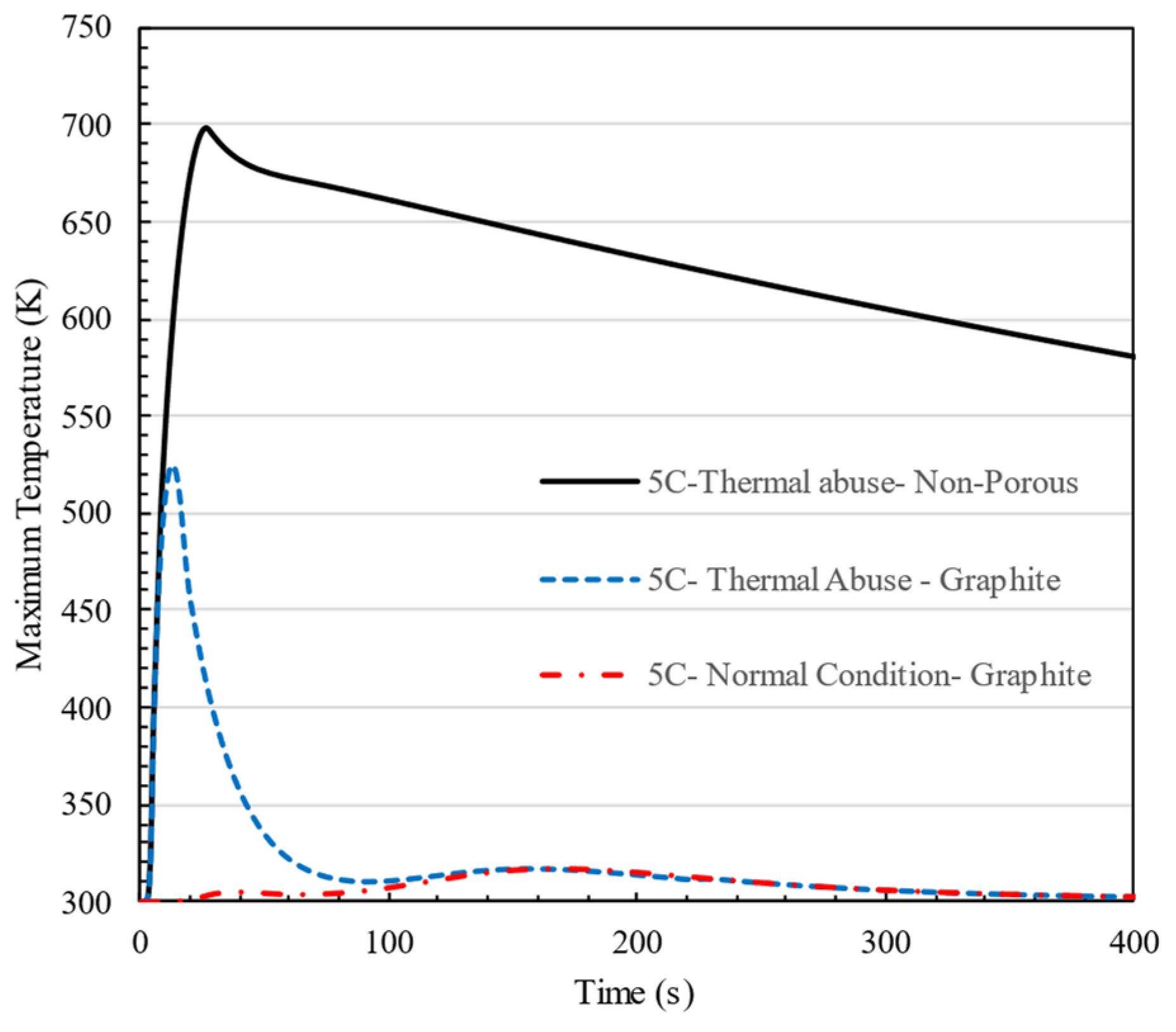
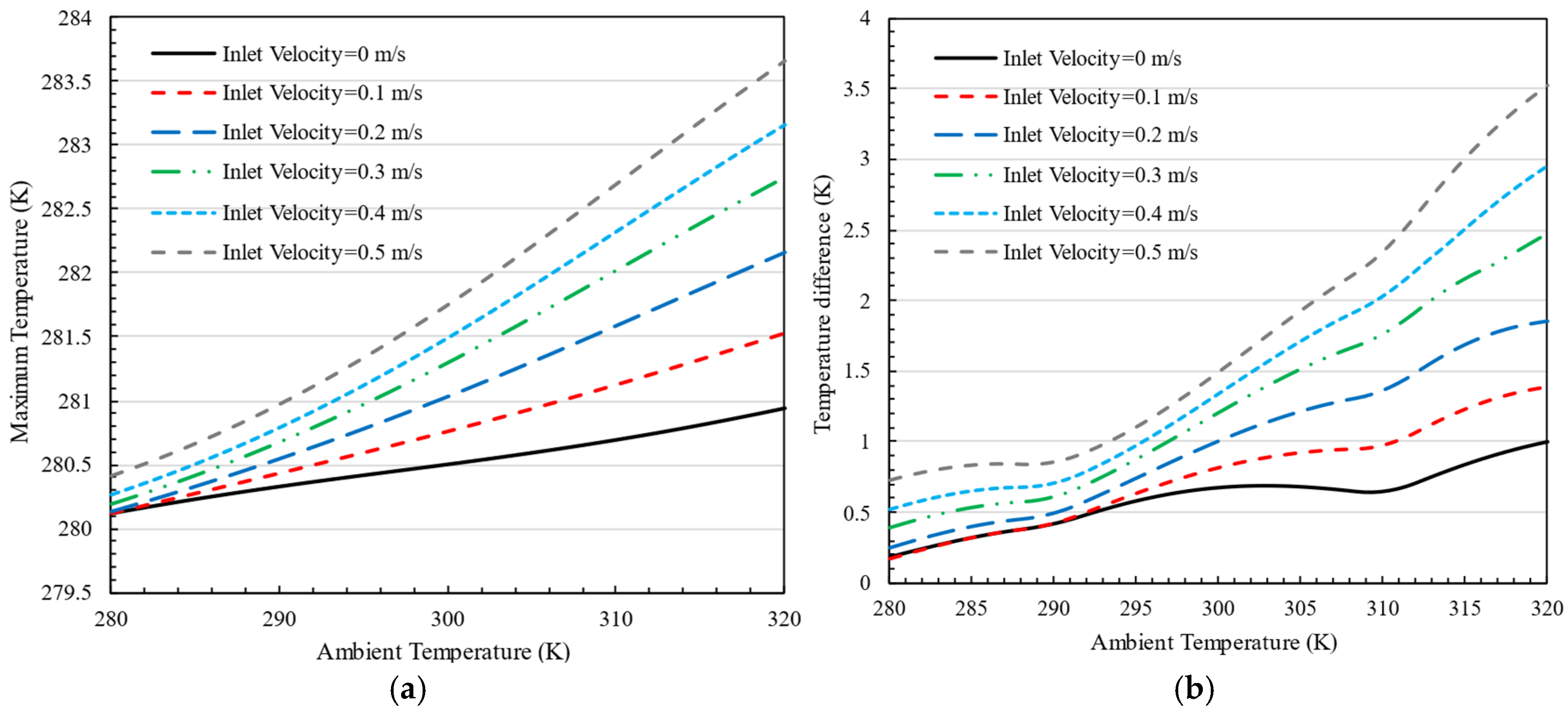
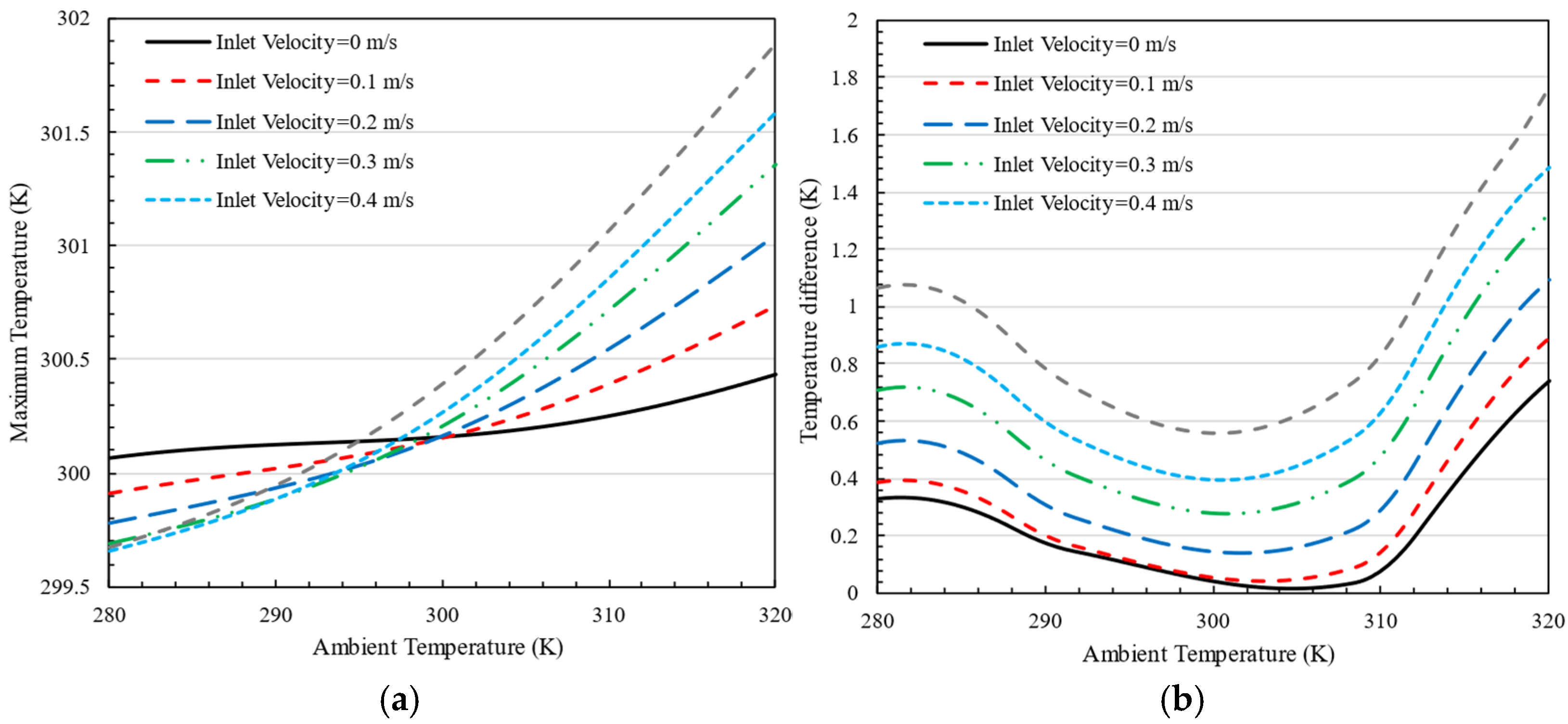
6.3. Battery Pack Performance at Different Conditions
7. Conclusions
- The heat produced during charging and discharging is directly proportional to the square of the C-rate, applicable to all battery pack configurations.
- The heat produced in a battery pack increases in direct proportion to the square of the number of batteries connected in series.
- Five distinct porous materials, including aluminum foam, copper foam, silicon carbide, aluminum oxide, and graphite, are analyzed. The results indicate that graphite demonstrates superior performance in maintaining the maximum cell temperature and temperature difference within an acceptable range throughout both the charging and discharging of the battery pack.
- The suggested battery pack can maintain cells within the permissible range during charging and discharging at rates up to 5C.
- Porous materials help maintain a stable temperature in the battery pack within 10 min of the battery beginning to charge or discharge. In the scenario where a porous material is not utilized, the temperature stabilizes after one hour.
- Analyzing temperature contours of a battery pack reveal improved temperature dispersion during both the charging and draining processes.
- The suggested BTMS is evaluated under various conditions. BTMS performance is consistently satisfactory in all scenarios during the 5C charging of the battery pack.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Unit | Description | |
| A | m2 | Area |
| F | Capacitor | |
| j/kg·K | Specific heat capacity | |
| m | Pore diameter | |
| ampere | Current | |
| Volumetric current transfer rate | ||
| W/m·K | PCM thermal conductivity | |
| W/m·K | TEC material thermal conductivity | |
| J/kg | Latent heat | |
| W | Heat generation | |
| Qc | W | Heat absorption |
| QH | W | Heat release |
| ohm | Resistor | |
| ohm | Internal resistance | |
| Electrical resistance | ||
| t | s | Time |
| K | Temperature | |
| K | Cold temperature | |
| K | Hot temperature | |
| v | m/s | Velocity |
| V | volt | Voltage |
| volt | Open-circuit voltage | |
| Seebeck coefficient | ||
| - | Fluid fraction | |
| - | Porosity | |
| Ohm·m | Effective electric conductivity for positive electrode | |
| Ohm·m | Effective electric conductivity for negative electrode | |
| Joule/kg | Phase potential for positive electrode | |
| Joule/kg | Phase potential for negative electrode | |
| density | ||
| Battery thermal management system | ||
| CFD | Computational fluid dynamics | |
| COP | Coefficient of performance | |
| SOC | State of charge | |
References
- Ravi, S.S.; Brace, C.; Larkin, C.; Aziz, M.; Leach, F.; Turner, J.W. On the pursuit of emissions-free clean mobility—Electric vehicles versus e-fuels. Sci. Total Environ. 2023, 875, 162688. [Google Scholar] [CrossRef] [PubMed]
- Hu, H.; Xu, X.; Sun, X.; Li, R.; Zhang, Y.; Fu, J. Numerical study on the inhibition control of lithium-ion battery thermal runaway. ACS Omega 2020, 5, 18254–18261. [Google Scholar] [CrossRef]
- Zhao, J.; Song, C.; Li, G. Fast-charging strategies for lithium-ion batteries: Advances and perspectives. ChemPlusChem 2022, 87, e202200155. [Google Scholar] [CrossRef] [PubMed]
- Su, Y.D.; Preger, Y.; Burroughs, H.; Sun, C.; Ohodnicki, P.R. Fiber optic sensing technologies for battery management systems and energy storage applications. Sensors 2021, 21, 1397. [Google Scholar] [CrossRef]
- Rahmani, A.; Dibaj, M.; Akrami, M. Recent advancements in battery thermal management systems for enhanced performance of Li-ion batteries: A comprehensive review. Batteries 2024, 10, 265. [Google Scholar] [CrossRef]
- Saavedra, J.; Gonzalez Cuadrado, D. Thermal Sensor Allocation for Effective and Efficient Heat Transfer Measurements in Transportation Systems. Sensors 2023, 23, 2803. [Google Scholar] [CrossRef] [PubMed]
- Afzal, A.; Abdul Razak, R.K.; Mohammed Samee, A.D.; Kumar, R.; Ağbulut, Ü.; Park, S.G. A critical review on renewable battery thermal management system using heat pipes. J. Therm. Anal. Calorim. 2023, 148, 8403–8442. [Google Scholar] [CrossRef]
- Rahmani, A.; Dibaj, M.; Akrami, M. A study on a battery pack in a hybrid battery thermal management system integrating with thermoelectric cooling. Case Stud. Therm. Eng. 2024, 61, 104856. [Google Scholar] [CrossRef]
- Mariam, E.; Ramasubramanian, B.; Reddy, V.S.; Dalapati, G.K.; Ghosh, S.; Sherin, T.; Chakrabortty, S.; Motapothula, M.R.; Kumar, A.; Ramakrishna, S.; et al. Emerging trends in cooling technologies for photovoltaic systems. Renew. Sustain. Energy Rev. 2024, 192, 114203. [Google Scholar] [CrossRef]
- Sharif, M.; Seker, H. Smart EV charging with context-awareness: Enhancing resource utilization via deep reinforcement learning. IEEE Access 2024, 12, 7009–7027. [Google Scholar] [CrossRef]
- Wu, Y.; Huang, Z.; Li, D.; Li, H.; Peng, J.; Stroe, D.; Song, Z. Optimal battery thermal management for electric vehicles with battery degradation minimization. Appl. Energy 2024, 353, 122090. [Google Scholar] [CrossRef]
- Lin, X.W.; Li, Y.B.; Wu, W.T.; Zhou, Z.F.; Chen, B. Advances on two-phase heat transfer for lithium-ion battery thermal management. Renew. Sustain. Energy Rev. 2024, 189, 114052. [Google Scholar] [CrossRef]
- Shahzad, K.; Cheema, I.I. Low-carbon technologies in automotive industry and decarbonizing transport. J. Power Sources 2024, 591, 233888. [Google Scholar] [CrossRef]
- Zhang, S.B.; Nie, F.; Cheng, J.P.; Yang, H.; Gao, Q. Optimizing the air flow pattern to improve the performance of the air-cooling lithium-ion battery pack. Appl. Therm. Eng. 2024, 236, 121486. [Google Scholar] [CrossRef]
- Kumar, R.; Singh, L.K.; Gupta, A.K. Evaluating air-cooling performance of lithium-ion-battery module with various cell arrangements. Energy Technol. 2024, 12, 2301061. [Google Scholar] [CrossRef]
- Chen, K.; Zhang, Z.; Wu, B.; Song, M.; Wu, X. An air-cooled system with a control strategy for efficient battery thermal management. Appl. Therm. Eng. 2024, 236, 121578. [Google Scholar] [CrossRef]
- Moosavi, A.; Ljung, A.L.; Lundström, T.S. A study on the effect of cell spacing in large-scale air-cooled battery thermal management systems using a novel modeling approach. J. Energy Storage 2023, 72, 108418. [Google Scholar] [CrossRef]
- Oyewola, O.M.; Idowu, E.T. Effects of step-like plenum, flow pattern and inlet flow regime on thermal management system. Appl. Therm. Eng. 2024, 243, 122637. [Google Scholar] [CrossRef]
- Lan, X.; Li, X.; Ji, S.; Gao, C.; He, Z. Design and optimization of a novel reverse layered air-cooling battery management system using U and Z type flow patterns. Int. J. Energy Res. 2022, 46, 14206–14226. [Google Scholar] [CrossRef]
- Wang, Y.; Yu, Y.; Jing, Z.; Wang, C.; Zhou, G.; Zhao, W. Thermal performance of lithium-ion batteries applying forced air cooling with an improved aluminium foam heat sink design. Int. J. Heat Mass Transf. 2021, 167, 120827. [Google Scholar] [CrossRef]
- Zhang, S.B.; He, X.; Long, N.C.; Shen, Y.J.; Gao, Q. Improving the air-cooling performance for lithium-ion battery packs by changing the air flow pattern. Appl. Therm. Eng. 2023, 221, 119825. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, P.; He, Y.; Li, S. Cooling performance optimization of air cooling lithium-ion battery thermal management system based on multiple secondary outlets and baffle. J. Energy Storage 2022, 52, 104678. [Google Scholar] [CrossRef]
- Kang, D.; Lee, P.Y.; Yoo, K.; Kim, J. Internal thermal network model-based inner temperature distribution of high-power lithium-ion battery packs with different shapes for thermal management. J. Energy Storage 2020, 27, 101017. [Google Scholar] [CrossRef]
- Shahid, S.; Agelin-Chaab, M. Development and analysis of a technique to improve air-cooling and temperature uniformity in a battery pack for cylindrical batteries. Therm. Sci. Eng. Prog. 2018, 5, 351–363. [Google Scholar] [CrossRef]
- Oyewola, O.M.; Awonusi, A.A.; Ismail, O.S. Design optimization of Air-Cooled Li-ion battery thermal management system with Step-like divergence plenum for electric vehicles. Alex. Eng. J. 2023, 71, 631–644. [Google Scholar] [CrossRef]
- Jiang, W.; Feng, G.; Wang, H.; Chang, Z.; Tan, X.; Ji, J.; Zhang, Y.; Zhou, J. Analysis of hybrid active-passive prismatic Li-ion battery thermal management system using phase change materials with porous-filled mini-channels. J. Energy Storage 2024, 80, 110144. [Google Scholar] [CrossRef]
- Verma, S.P.; Saraswati, S. Numerical and experimental analysis of air-cooled Lithium-ion battery pack for the evaluation of the thermal performance enhancement. J. Energy Storage 2023, 73, 108983. [Google Scholar] [CrossRef]
- Zhao, G.; Wang, X.; Negnevitsky, M.; Li, C. An up-to-date review on the design improvement and optimization of the liquid-cooling battery thermal management system for electric vehicles. Appl. Therm. Eng. 2023, 219, 119626. [Google Scholar] [CrossRef]
- Maknikar, S.K.; Pawar, A.M. Application of phase change material (PCM) in battery thermal management system (BTMS): A critical review. Mater. Today Proc. 2023; in press. [Google Scholar] [CrossRef]
- Kavasoğullari, B.; Karagöz, M.E.; Yildiz, A.S.; Biçer, E. Numerical investigation of the performance of a hybrid battery thermal management system at high discharge rates. J. Energy Storage 2023, 73, 108982. [Google Scholar] [CrossRef]
- Jiang, Z.; Li, H.; Sun, Z.; Qu, Z. Experimental study on 18650 lithium-ion battery-pack cooling system composed of heat pipe and reciprocating air flow with water mist. Int. J. Heat Mass Transf. 2024, 222, 125171. [Google Scholar] [CrossRef]
- Sharma, D.K.; Agarwal, P.; Prabhakar, A. Effect of fin design and continuous cycling on thermal performance of PCM-HP hybrid BTMS for high ambient temperature applications. J. Energy Storage 2023, 74, 109360. [Google Scholar] [CrossRef]
- Asli, M.; König, P.; Sharma, D.; Pontika, E.; Huete, J.; Konda, K.R.; Mathiazhagan, A.; Xie, T.; Höschler, K.; Laskaridis, P. Thermal management challenges in hybrid-electric propulsion aircraft. Prog. Aerosp. Sci. 2024, 144, 100967. [Google Scholar] [CrossRef]
- Moaveni, A.; Siavashi, M.; Mousavi, S. Passive and hybrid battery thermal management system by cooling flow control, employing nano-PCM, fins, and metal foam. Energy 2024, 288, 129809. [Google Scholar] [CrossRef]
- Rahmani, A.; Dibaj, M.; Akrami, M. Computational investigation of magnetohydrodynamic flow and melting process of phase change material in a battery pack using the lattice Boltzmann method. J. Energy Storage 2024, 78, 110046. [Google Scholar] [CrossRef]
- Pakrouh, R.; Hosseini, M.J.; Ranjbar, A.A.; Rahimi, M. A novel liquid-based battery thermal management system coupling with phase change material and thermoelectric cooling. J. Energy Storage 2023, 64, 107098. [Google Scholar] [CrossRef]
- Yang, M.; Mathew, G.; Nemati, H.; Moghimi, M.A. A novel approach for active cooling of a battery at cell level: Air-cooled mini-channel heat sink, enhanced with intermittent metal foam. J. Energy Storage 2024, 81, 110374. [Google Scholar] [CrossRef]
- Sutheesh, P.M.; Atul, A.P.; Rohinikumar, B. Numerical and experimental investigations of thermal performance of lithium-ion battery with hybrid cooling system under dry-out condition. J. Energy Storage 2024, 84, 110889. [Google Scholar] [CrossRef]
- Rahmani, A.; Dibaj, M.; Akrami, M. Enhancing Heat Storage Cooling Systems via the Implementation of Honeycomb-Inspired Design: Investigating Efficiency and Performance. Energies 2024, 17, 351. [Google Scholar] [CrossRef]
- Bargal, M.H.; Abdelkareem, M.A.; Tao, Q.; Li, J.; Shi, J.; Wang, Y. Liquid cooling techniques in proton exchange membrane fuel cell stacks: A detailed survey. Alex. Eng. J. 2020, 59, 635–655. [Google Scholar] [CrossRef]
- Kwon, K.H.; Shin, C.B.; Kang, T.H.; Kim, C.S. A two-dimensional modeling of a lithium-polymer battery. J. Power Sources 2006, 163, 151–157. [Google Scholar] [CrossRef]
- Vanaclocha Hervas, C. Comparative Study of Three Electrochemical Cell Models for the CFD Simulation of a Battery Module. Ph.D. Thesis, Universitat Politècnica de València, Valencia, Spain, 2021. [Google Scholar]
- Yetik, O.; Karakoc, T.H. A numerical study on the thermal performance of prismatic Li-ion batteries for hibrid electric aircraft. Energy 2020, 195, 117009. [Google Scholar] [CrossRef]
- Yetik, O.; Karakoc, T.H. Estimation of thermal effect of different busbars materials on prismatic Li-ion batteries based on artificial neural networks. J. Energy Storage 2021, 38, 102543. [Google Scholar] [CrossRef]
- Yetik, O.; Karakoc, T.H. Thermal and electrical analysis of batteries in electric aircraft using nanofluids. J. Energy Storage 2022, 52, 104853. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, T.; Hua, Y.; Gao, Q.; Han, Z.; Yang, K.; Xu, Y.; Liu, X.; Xu, X.; Wang, S. Simulation study on the interaction between the battery module and busbar under typical driving conditions of electric vehicles. Case Stud. Therm. Eng. 2023, 45, 103006. [Google Scholar] [CrossRef]
- He, H.; Zhang, X.; Xiong, R.; Xu, Y.; Guo, H. Online model-based estimation of state-of-charge and open-circuit voltage of lithium-ion batteries in electric vehicles. Energy 2012, 39, 310–318. [Google Scholar] [CrossRef]
- Hossein Bashiri, A.; Sangtarash, A.; Zamani, M. The effect of the porous media on thermal management of lithium-ion battery pack; a comparative and numerical study. Therm. Sci. Eng. Prog. 2022, 34, 101427. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Fluent Theory Guide, Release 2023 R1; ANSYS Inc.: Canonsburg, PA, USA, 2023; Available online: https://www.ansys.com (accessed on 20 December 2024).
- Li, C.; Cui, N.; Chang, L.; Cui, Z.; Yuan, H.; Zhang, C. Effect of parallel connection topology on air-cooled lithium-ion battery module: Inconsistency analysis and comprehensive evaluation. Appl. Energy 2022, 313, 118758. [Google Scholar] [CrossRef]
- Plett, G.L. Battery Management Systems, Volume II: Equivalent-Circuit Methods; Artech House: London, UK, 2015. [Google Scholar]
- Rashidi, S.; Esfahani, J.A.; Karimi, N. Porous materials in building energy technologies—A review of the applications, modelling and experiments. Renew. Sustain. Energy Rev. 2018, 91, 229–247. [Google Scholar] [CrossRef]
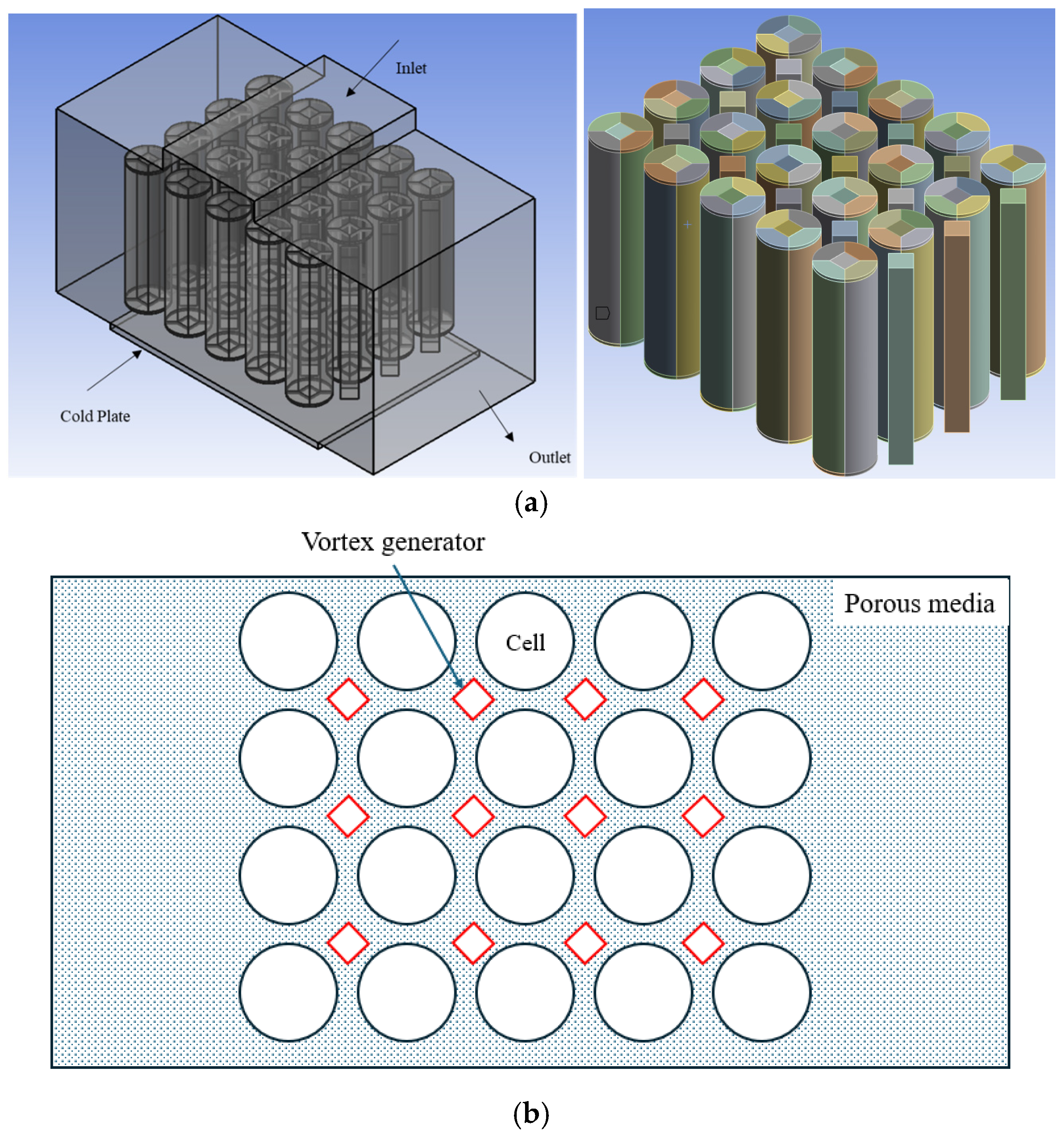

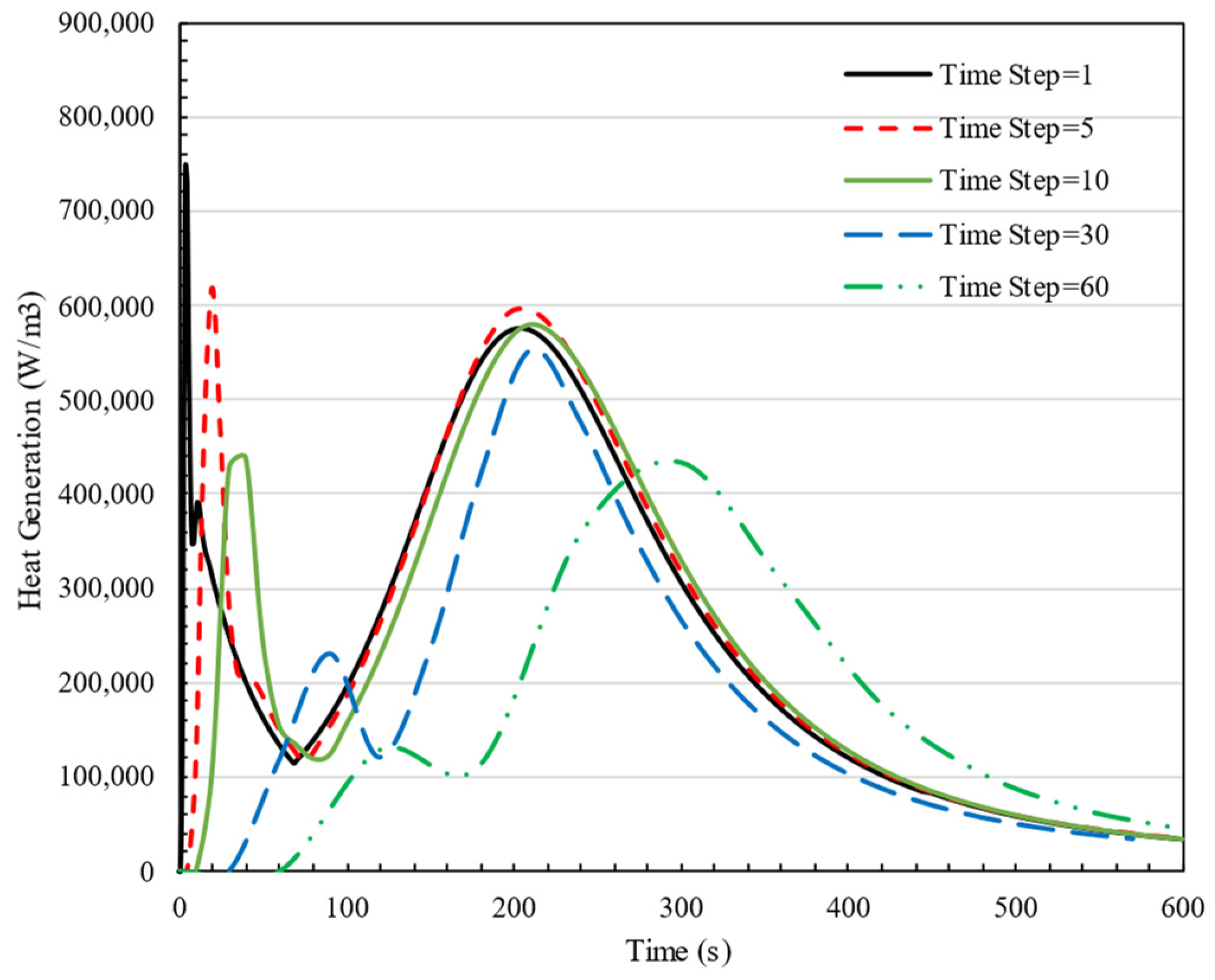
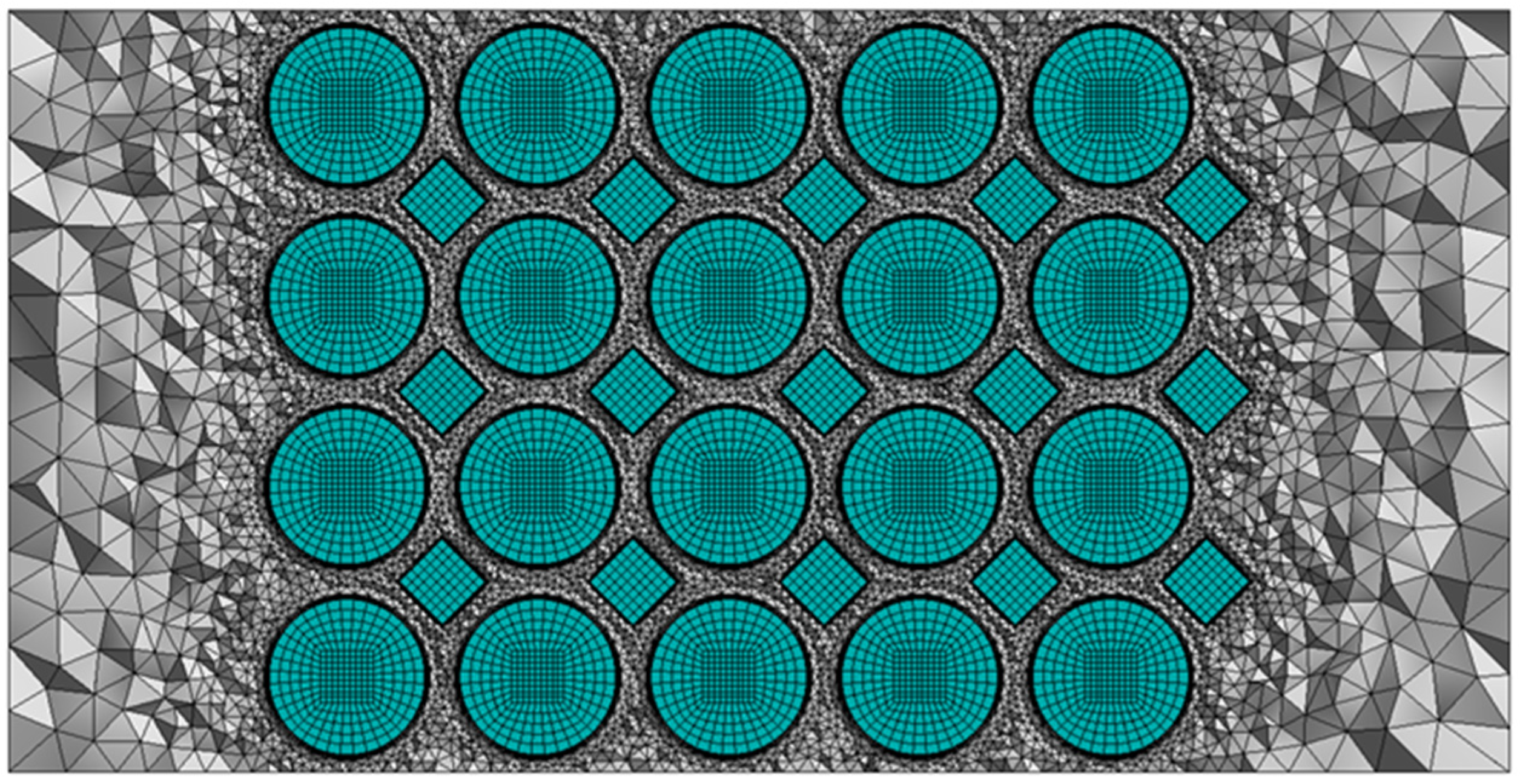
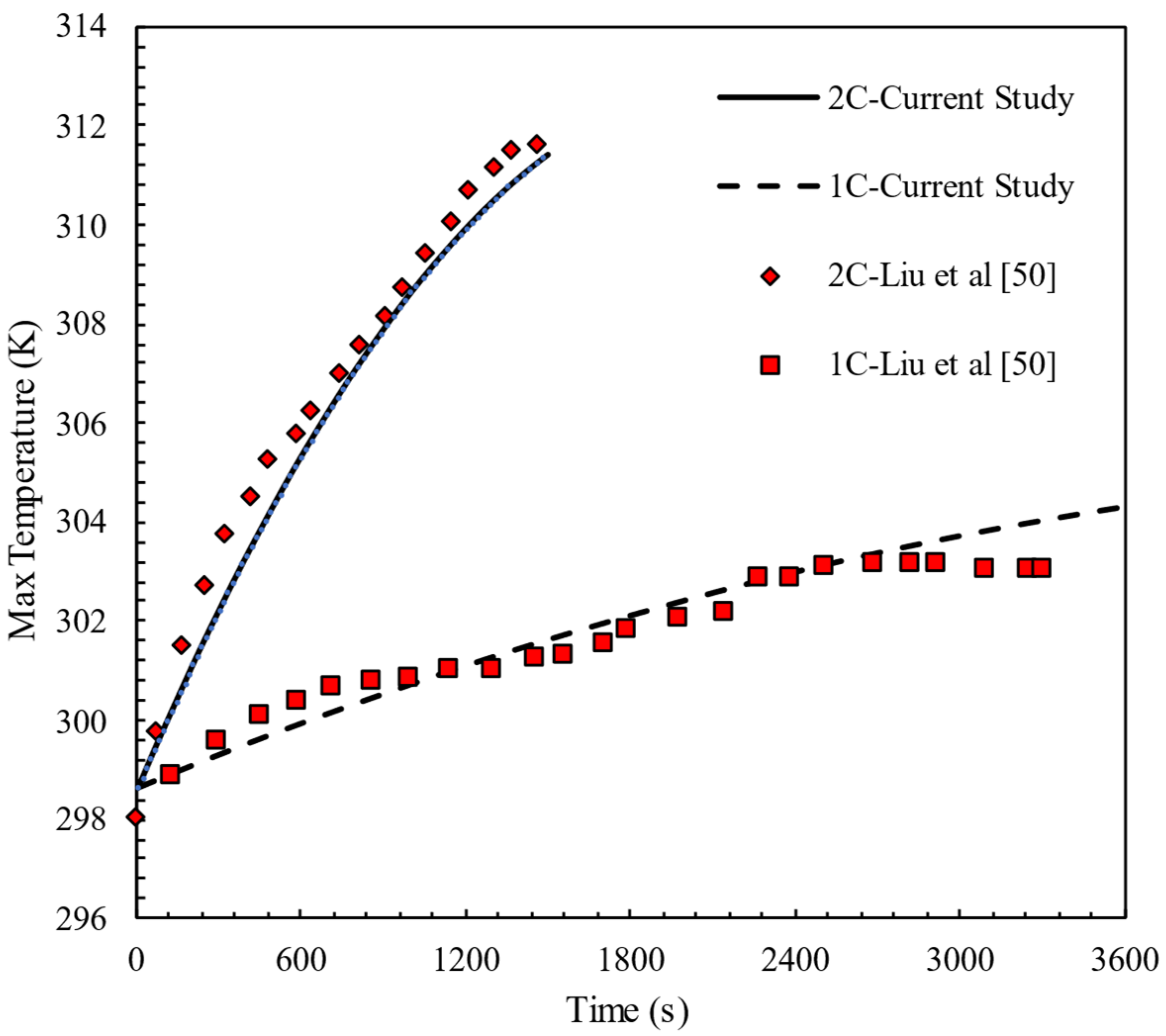


| Properties | Unit | Value |
|---|---|---|
| Cell diameter | mm | 18 |
| Cell length | mm | 65 |
| Nominal capacity | mAh | 3500 |
| Nominal voltage | V | 3.56 |
| 2092 | ||
| j/kg·K | 678 | |
| W/m·K | 18.2 | |
| Operating temperature | K | Charge: 273–318 K Discharge: 253–333 K |
| Parameter | Unit | Value |
|---|---|---|
| - | ||
| A | ||
| HW | ||
| E | J/mol | −15,253 |
| R | 8.314 | |
| m | - | 0.9404 |
| n | - | 0.425 |
| Number of Total Elements | Maximum Peak Temperature | Error |
|---|---|---|
| 563,156 | 313.67 | - |
| 991,325 | 315.34 | 1.67 K |
| 1,511,644 | 316.75 | 1.41 K |
| 2,753,472 | 316.98 | 0.23 K |
| 14,165,808 | 317.06 | 0.08 K |
| Material | Permeability | ||||
|---|---|---|---|---|---|
| Aluminum foam | 2719 | 871 | 202.4 | 0.958 | |
| Copper foam | 8978 | 386 | 398 | 0.944 | |
| Silicon Carbide | 3210 | 700 | 430 | 0.890 | |
| Aluminum oxide | 3891 | 765 | 35 | 0.878 | |
| Graphite | 2130 | 700 | 1700 | 0.728 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahmani, A.; Dibaj, M.; Akrami, M. Enhancing Battery Pack Cooling Efficiency Through Graphite-Integrated Hybrid-Battery Thermal Management Systems. Batteries 2025, 11, 113. https://doi.org/10.3390/batteries11030113
Rahmani A, Dibaj M, Akrami M. Enhancing Battery Pack Cooling Efficiency Through Graphite-Integrated Hybrid-Battery Thermal Management Systems. Batteries. 2025; 11(3):113. https://doi.org/10.3390/batteries11030113
Chicago/Turabian StyleRahmani, Amin, Mahdieh Dibaj, and Mohammad Akrami. 2025. "Enhancing Battery Pack Cooling Efficiency Through Graphite-Integrated Hybrid-Battery Thermal Management Systems" Batteries 11, no. 3: 113. https://doi.org/10.3390/batteries11030113
APA StyleRahmani, A., Dibaj, M., & Akrami, M. (2025). Enhancing Battery Pack Cooling Efficiency Through Graphite-Integrated Hybrid-Battery Thermal Management Systems. Batteries, 11(3), 113. https://doi.org/10.3390/batteries11030113








