Sequential Multi-Scale Modeling Using an Artificial Neural Network-Based Surrogate Material Model for Predicting the Mechanical Behavior of a Li-Ion Pouch Cell Under Abuse Conditions
Abstract
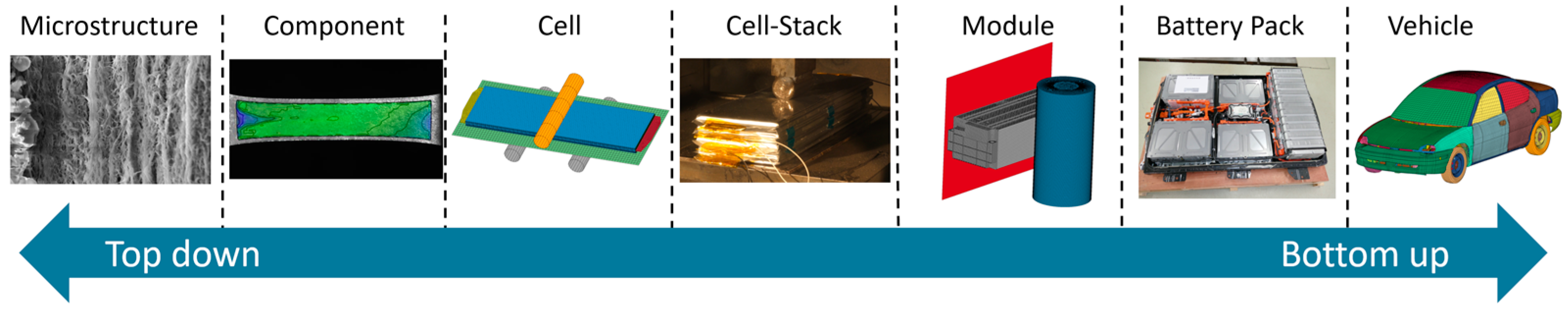
:1. Introduction
2. Materials and Methods
2.1. Cell Under Study
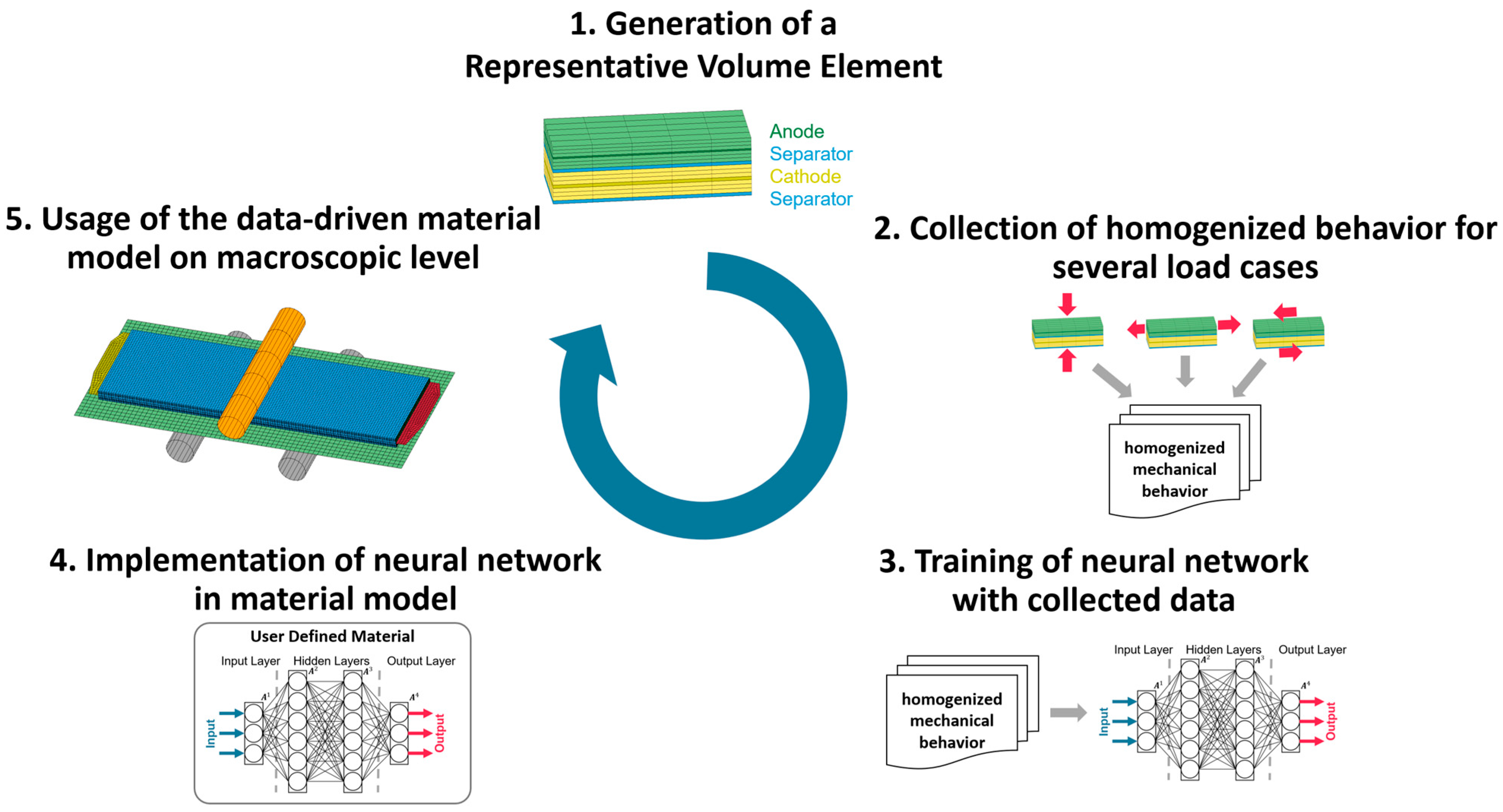
2.2. Concept
- The selection and modeling of a representative volume element.
- The generation of training data in appropriate load cases (homogenized mechanical behavior).
- The training of a neural network.
- The implementation of the trained neural network in a commercial FEA solver.
- The application of the data-driven material model on a macroscopic cell level.
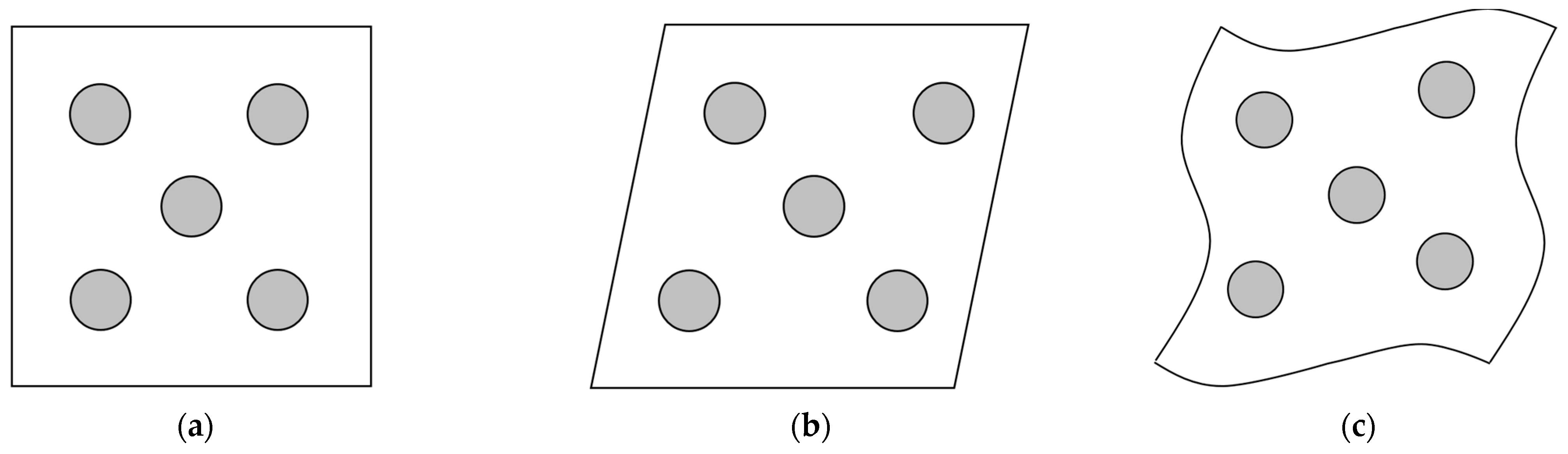
2.3. Representative Volume Element (RVE)
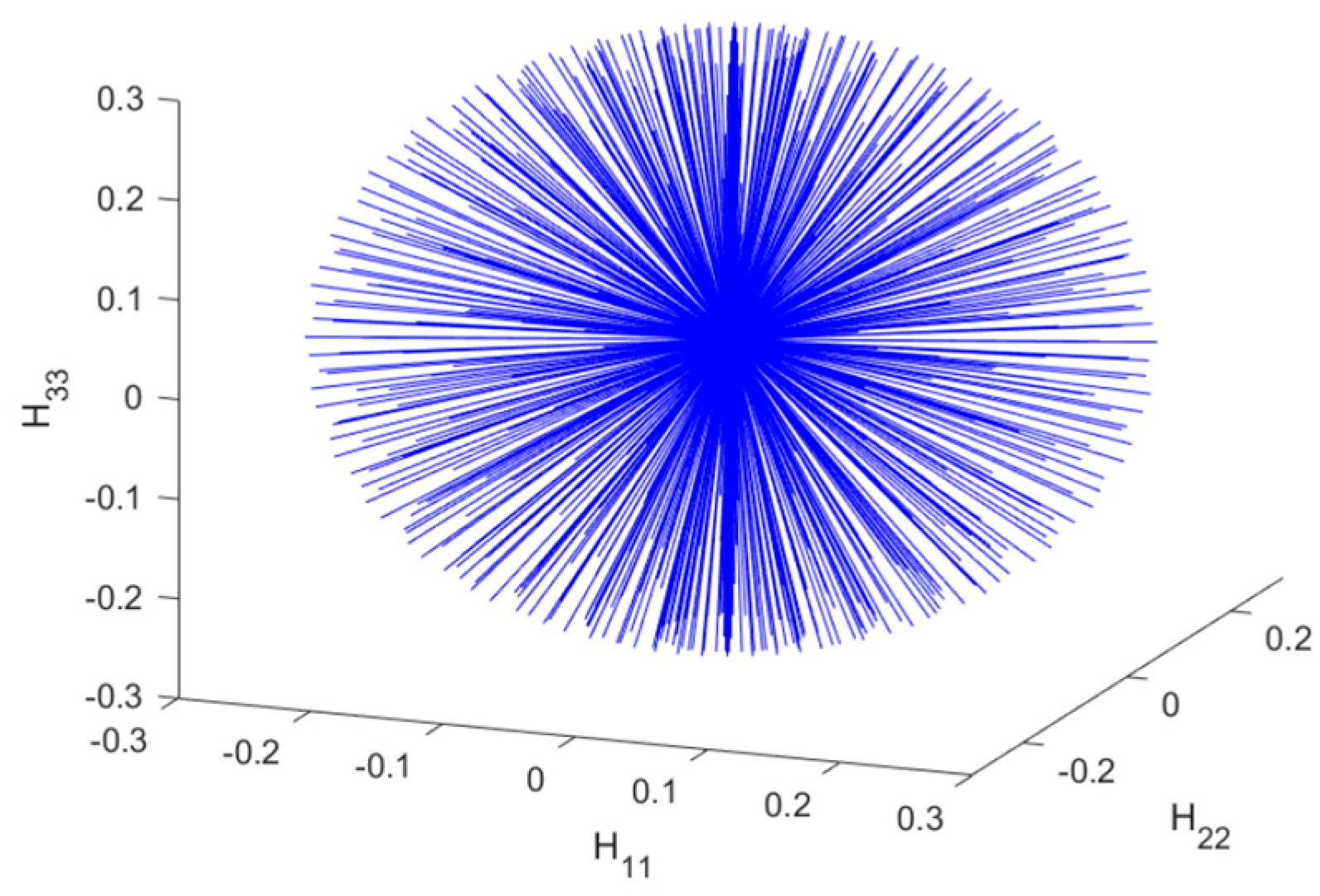
2.4. Homogenization
2.5. Artificial Neural Network
2.6. Consideration of Component Failure
2.7. Implementation in LS-DYNA
2.8. Cell Model
2.9. Experiments for Characterization and Vaildation
2.9.1. In-Plane Characterization of Components
2.9.2. Out-of-Plane Characterization of Components
2.9.3. Cell Tests for Calibration and Validation
3. Results
3.1. In-Plane Characterization of Components
3.2. Out-of-Plane Characterization of Components
3.3. Verification of Data-Driven Material Model
3.4. Validation Through Cell Tests
3.5. Influence of Network Size
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fleischmann, J.; Hanicke, M.; Horetsky, E.; Ibrahim, D.; Jautelat, S.; Linder, M.; Schaufuss, P.; Torscht, L.; Van de Rijt, A. Battery 2030: Resilient, Sustainable and Circular: Battery Demand Is Growing—and so Is the Need for Better Solutions Along the Value Chain; McKinsey & Company: New York, NY, USA, 2023. [Google Scholar]
- Chombo, P.V.; Laoonual, Y.; Wongwises, S. Lessons from the Electric Vehicle Crashworthiness Leading to Battery Fire. Energies 2021, 14, 4802. [Google Scholar] [CrossRef]
- Li, G.; Fu, X.; Yang, Y. Anti-vibration safety performance research of battery pack based on finite element method in electric vehicle. In Proceeding of the 36th Chinese Control Conference (CCC), Dalian, Chian, 26–28 July 2017; pp. 10281–10285. [Google Scholar] [CrossRef]
- Fasching, M.; Grollitsch, S.; Höschele, P.; Schmid, A.; Ellersdorfer, C. Investigating the mechanical in-plane characteristics of lithium-ion pouch cells under crush loads. J. Energy Storage 2024, 89, 111581. [Google Scholar] [CrossRef]
- Sahraei, E.; Campbell, J.; Wierzbicki, T. Modeling and short circuit detection of 18650 Li-ion cells under mechanical abuse conditions. J. Power Sources 2012, 220, 360–372. [Google Scholar] [CrossRef]
- Sievers, M.; Sievers, U.; Mao, S.S. Thermal modelling of new Li-ion cell design modifications. Forsch. Ingenieurwes 2010, 74, 215–231. [Google Scholar] [CrossRef]
- Ramadesigan, V.; Northrop, P.W.C.; De, S.; Santhanagopalan, S.; Braatz, R.D.; Subramanian, V.R. Modeling and Simulation of Lithium-Ion Batteries from a Systems Engineering Perspective. J. Electrochem. Soc. 2012, 159, R31–R45. [Google Scholar] [CrossRef]
- Lin, N.; Röder, F.; Krewer, U. Multiphysics Modeling for Detailed Analysis of Multi-Layer Lithium-Ion Pouch Cells. Energies 2018, 11, 2998. [Google Scholar] [CrossRef]
- Allu, S.; Kalnaus, S.; Simunovic, S.; Nanda, J.; Turner, J.A.; Pannala, S. A three-dimensional meso-macroscopic model for Li-Ion intercalation batteries. J. Power Sources 2016, 325, 42–50. [Google Scholar] [CrossRef]
- Zhu, J.; Wierzbicki, T.; Li, W. A review of safety-focused mechanical modeling of commercial lithium-ion batteries. J. Power Sources 2018, 378, 153–168. [Google Scholar] [CrossRef]
- Zhu, L.; Xia, Y.; Liu, Y.; Ge, Y.; Wang, L.; Zhang, L. Extending a Homogenized Model for Characterizing Multidirectional Jellyroll Failure in Prismatic Lithium-Ion Batteries. Energies 2021, 14, 3444. [Google Scholar] [CrossRef]
- Xu, J.; Liu, B.; Wang, X.; Hu, D. Computational model of 18650 lithium-ion battery with coupled strain rate and SOC dependencies. Appl. Energy 2016, 172, 180–189. [Google Scholar] [CrossRef]
- Schmid, A.; Pasquale, A.; Ellersdorfer, C.; Raffler, M.; Champaney, V.; Ziane, M.; Chinesta, F.; Feist, F. Mechanical Characterization of Li-Ion Cells and the Calibration of Numerical Models Using Proper Generalized Decomposition. In Proceeding of the ASME International Mechanical Engineering Congress and Exposition, New Orleans, LA, USA, 29 October–2 November 2023; Volume 7. [Google Scholar] [CrossRef]
- Schmid, A.; Pasquale, A.; Ellersdorfer, C.; Champaney, V.; Raffler, M.; Guévelou, S.; Kizio, S.; Ziane, M.; Feist, F.; Chinesta, F. PGD based meta modelling of a lithium-ion battery for real time prediction. Front. Mater. 2023, 10, 1245347. [Google Scholar] [CrossRef]
- Raffler, M.; Sevarin, A.; Ellersdorfer, C.; Heindl, S.F.; Breitfuss, C.; Sinz, W. Finite element model approach of a cylindrical lithium ion battery cell with a focus on minimization of the computational effort and short circuit prediction. J. Power Sources 2017, 360, 605–617. [Google Scholar] [CrossRef]
- Qin, D.; Wang, P.; Wang, T.; Chen, J. Modeling and Dynamic Impact Analysis of Prismatic Lithium-Ion Battery. Sustainability 2023, 15, 8414. [Google Scholar] [CrossRef]
- Kisters, T.; Gilaki, M.; Nau, S.; Sahraei, E. Modeling of Dynamic Mechanical Response of Li-Ion cells with Homogenized Electrolyte-Solid Interactions. J. Energy Storage 2022, 49, 104069. [Google Scholar] [CrossRef]
- Beaumont, R.; Masters, I.; Das, A.; Lucas, S.; Thanikachalam, A.; Williams, D. Methodology for Developing a Macro Finite Element Model of Lithium-Ion Pouch Cells for Predicting Mechanical Behaviour under Multiple Loading Conditions. Energies 2021, 14, 1921. [Google Scholar] [CrossRef]
- Zhu, J. Standardizing mechanical tests on li-ion batteries to develop a useful cell-level model under extreme mechanical loads. J. Energy Storage 2023, 65, 107320. [Google Scholar] [CrossRef]
- Sinz, W.; Feist, F.; Gstrein, G.; Gugler, J.; Tomasch, E.; Breitfuss, C.; Luttenberger, P.; Steffan, H.; Gollob, P.; Hennige, V. Concepts for Mechanical Abuse Testing of High-Voltage Batteries; SAE Technical Paper Series; SAE International: Warrendale, PA, USA, 2012. [Google Scholar]
- Ellersdorfer, C.; Höschele, P.; Heider, E.; Kovachev, G.; Gstrein, G. Safety Assessment of High Dynamic Pre-Loaded Lithium Ion Pouch Cells. Batteries 2023, 9, 71. [Google Scholar] [CrossRef]
- Goodman, J.K.S.; Miller, J.T.; Kreuzer, S.; Forman, J.; Wi, S.; Choi, J.-M.; Oh, B.; White, K. Lithium-ion cell response to mechanical abuse: Three-point bend. J. Energy Storage 2020, 28, 101244. [Google Scholar] [CrossRef]
- Raffler, M.; Sinz, W.; Erker, S.; Brunnsteiner, B.; Ellersdorfer, C. Influence of loading rate and out of plane direction dependence on deformation and electro-mechanical failure behavior of a lithium-ion pouch cell. J. Energy Storage 2022, 56, 105906. [Google Scholar] [CrossRef]
- Kisters, T.; Sahraei, E.; Wierzbicki, T. Dynamic impact tests on lithium-ion cells. Int. J. Impact Eng. 2017, 108, 205–216. [Google Scholar] [CrossRef]
- Breitfuss, C.; Sinz, W.; Feist, F.; Gstrein, G.; Lichtenegger, B.; Knauder, C.; Ellersdorfer, C.; Moser, J.; Steffan, H.; Stadler, M.; et al. A ‘Microscopic’ Structural Mechanics FE Model of a Lithium-Ion Pouch Cell for Quasi-Static Load Cases. SAE Int. J. Passeng. Cars-Mech. Syst. 2013, 6, 1044–1054. [Google Scholar] [CrossRef]
- Schaufelberger, B.; Altes, A.; Matura, P. Modeling the Mechanical Behaviour of a Li-Ion Pouch Cell under Three-Point Bending. In Proceeding of the 13th European LS-DYNA Conference, Ulm, Germany, 5–7 October 2021. [Google Scholar]
- Schmid, A.; Ellersdorfer, C.; Raffler, M.; Karajan, N.; Feist, F. An efficient detailed layer model for prediction of separator damage in a Li-Ion pouch cell exposed to transverse compression. J. Power Sources 2023, 581, 233510. [Google Scholar] [CrossRef]
- Wang, L.; Yin, S.; Xu, J. A detailed computational model for cylindrical lithium-ion batteries under mechanical loading: From cell deformation to short-circuit onset. J. Power Sources 2019, 413, 284–292. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, J.; Cao, L.; Wu, Z.; Santhanagopalan, S. Constitutive behavior and progressive mechanical failure of electrodes in lithium-ion batteries. J. Power Sources 2017, 357, 126–137. [Google Scholar] [CrossRef]
- Schmid, A.; Pasquale, A.; Ellersdorfer, C.; Ziane, M.; Raffler, M.; Champaney, V.; Feist, F.; Chinesta, F. Application of PGD separation of space to create a reduced-order model of a lithium-ion cell structure. Front. Mater. 2023, 10, 1212400. [Google Scholar] [CrossRef]
- Cannarella, J.; Liu, X.; Leng, C.Z.; Sinko, P.D.; Gor, G.Y.; Arnold, C.B. Arnold, Mechanical Properties of a Battery Separator under Compression and Tension. J. Electrochem. Soc. 2014, 161, F3117–F3122. [Google Scholar] [CrossRef]
- Wang, L.; Yin, S.; Zhang, C.; Huan, Y.; Xu, J. Mechanical characterization and modeling for anodes and cathodes in lithium-ion batteries. J. Power Sources 2018, 392, 265–273. [Google Scholar] [CrossRef]
- Li, Z.; Chen, J.; Lan, F.; Li, Y. Constitutive Behavior and Mechanical Failure of Internal Configuration in Prismatic Lithium-Ion Batteries under Mechanical Loading. Energies 2021, 14, 1219. [Google Scholar] [CrossRef]
- Sahraei, E.; Bosco, E.; Dixon, B.; Lai, B. Microscale failure mechanisms leading to internal short circuit in Li-ion batteries under complex loading scenarios. J. Power Sources 2016, 319, 56–65. [Google Scholar] [CrossRef]
- Ali, M.Y.; Lai, W.-J.; Pan, J. Computational models for simulations of lithium-ion battery cells under constrained compression tests. J. Power Sources 2013, 242, 325–340. [Google Scholar] [CrossRef]
- Ali, M.Y.; Lai, W.-J.; Pan, J. Computational models for simulation of a lithium-ion battery module specimen under punch indentation. J. Power Sources 2015, 273, 448–459. [Google Scholar] [CrossRef]
- Budiman, B.A.; Rahardian, S.; Saputro, A.; Hidayat, A.; Nurprasetio, I.P.; Sambegoro, P. Structural integrity of lithium-ion pouch battery subjected to three-point bending. Eng. Fail. Anal. 2022, 138, 106307. [Google Scholar] [CrossRef]
- Kermani, G.; Keshavarzi, M.M.; Sahraei, E. Deformation of lithium-ion batteries under axial loading: Analytical model and Representative Volume Element. Energy Rep. 2021, 7, 2849–2861. [Google Scholar] [CrossRef]
- Geers, M.G.D.; Kouznetsova, V.G.; Brekelmans, W.A.M. Multi-scale computational homogenization: Trends and challenges. J. Comput. Appl. Math. 2010, 234, 2175–2182. [Google Scholar] [CrossRef]
- Vernerey, F.; Liu, W.K.; Moran, B. Multi-scale micromorphic theory for hierarchical materials. J. Mech. Phys. Solids 2007, 55, 2603–2651. [Google Scholar] [CrossRef]
- Schmitz, A.; Horst, P. A finite element unit-cell method for homogenised mechanical properties of heterogeneous plates. Compos. Part. A: Appl. Sci. Manuf. 2014, 61, 23–32. [Google Scholar] [CrossRef]
- Tikarrouchine, E.; Chatzigeorgiou, G.; Praud, F.; Piotrowski, B.; Chemisky, Y.; Meraghni, F. Three-dimensional FE2 method for the simulation of non-linear, rate-dependent response of composite structures. Compos. Struct. 2018, 193, 165–179. [Google Scholar] [CrossRef]
- Hashash, Y.M.A.; Jung, S.; Ghaboussi, J. Numerical implementation of a neural network based material model in finite element analysis. Int. J. Numer. Meth. Engng. 2004, 59, 989–1005. [Google Scholar] [CrossRef]
- Gorji, M.B.; Mozaffar, M.; Heidenreich, J.N.; Cao, J.; Mohr, D. On the potential of recurrent neural networks for modeling path dependent plasticity. J. Mech. Phys. Solids 2020, 143, 103972. [Google Scholar] [CrossRef]
- Tancogne-Dejean, T.; Gorji, M.B.; Zhu, J.; Mohr, D. Recurrent neural network modeling of the large deformation of lithium-ion battery cells. Int. J. Plast. 2021, 146, 103072. [Google Scholar] [CrossRef]
- Liu, Z.; Wu, C.T.; Ren, B.; Liu, K.W.; Grimes, R. Multiscale Simulations of Material with Heterogeneous Structures Based on Representative Volume Element Techniques. In Proceeding of the 15th International LS-DYNA Users Conference, Detroit, MI, USA, 10 June 2018. [Google Scholar]
- Hill, R. Elastic properties of reinforced solids: Some theoretical principles. J. Mech. Phys. Solids 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Livermore Software Technology (LST). LS-DYNA® Keyword User’s Manual Volume I R13; Livermore Software Technology Corporation: Livermore, CA, USA, 2021; pp. 3518–3527. [Google Scholar]
- MacKay, D.J. Bayesian Interpolation. Neural Comput. 1992, 4, 415–447. [Google Scholar] [CrossRef]
- The MathWorks, Inc. Documentation: Bayesian Regularization Backpropagation. Available online: https://www.mathworks.com/help/deeplearning/ref/trainbr.html (accessed on 23 September 2024).
- Joseph, V.R. Optimal ratio for data splitting. Stat. Anal. 2022, 15, 531–538. [Google Scholar] [CrossRef]
- Erhart, T. User Defined Materials in LS-DYNA; Dynamore GmbH: Stuttgart, Germany, 2015. [Google Scholar]
- Qu, Y.; Xing, B.; Wang, C.; Xia, Y. Simplified layered model of pouch cell for varied load cases: An indentation and three-point bending study. J. Energy Storage 2023, 59, 106476. [Google Scholar] [CrossRef]
- Rezaei, S.; Moeineddin, A.; Harandi, A. Learning solution of nonlinear constitutive material models using physics-informed neural networks: COMM-PINN. arXiv 2023, arXiv:2304.06044. [Google Scholar]
- Kalina, K.A.; Linden, L.; Brummund, J.; Kästner, M. FE ANN: An efficient data-driven multiscale approach based on physics-constrained neural networks and automated data mining. Comput. Mech. 2023, 71, 827–851. [Google Scholar] [CrossRef]
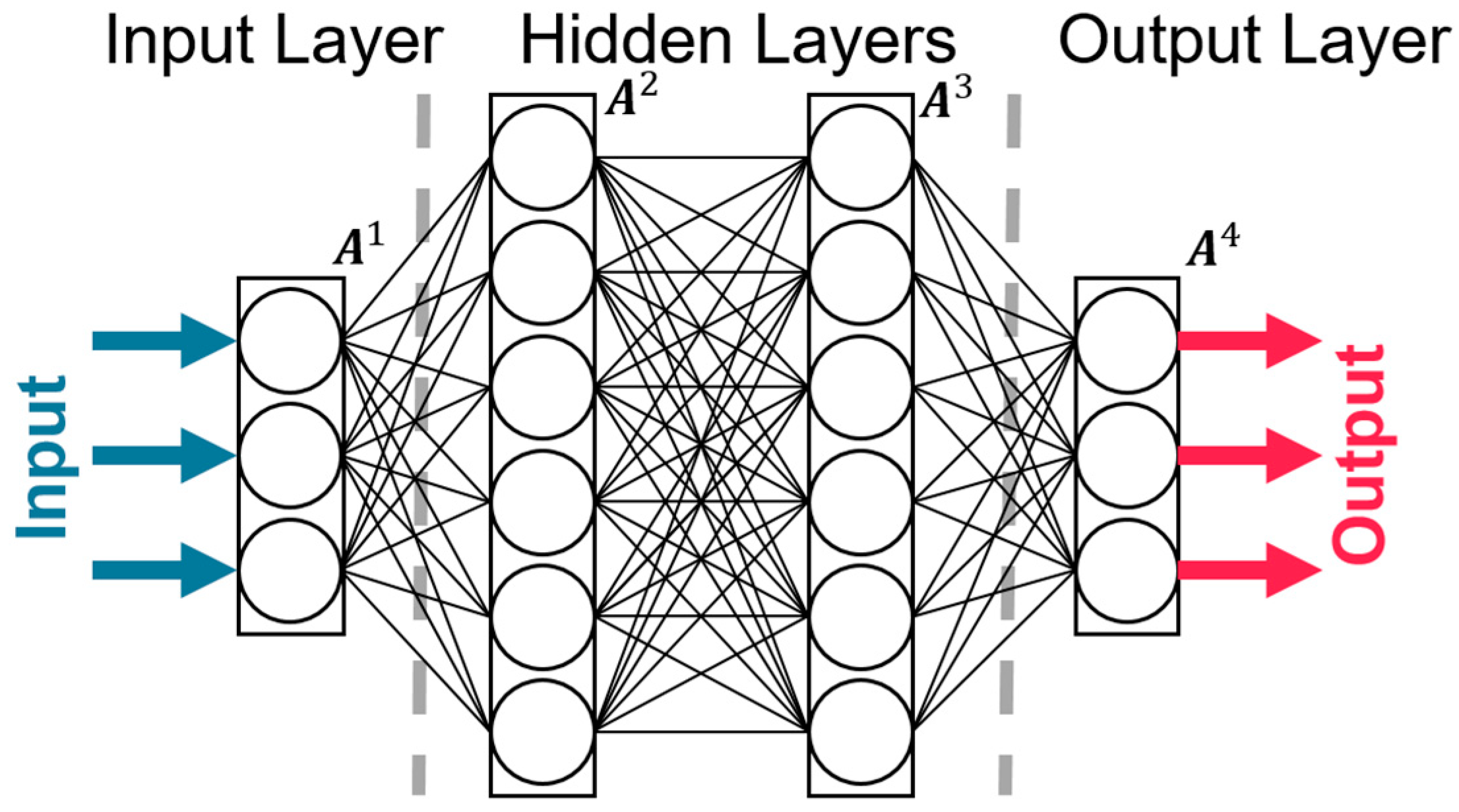

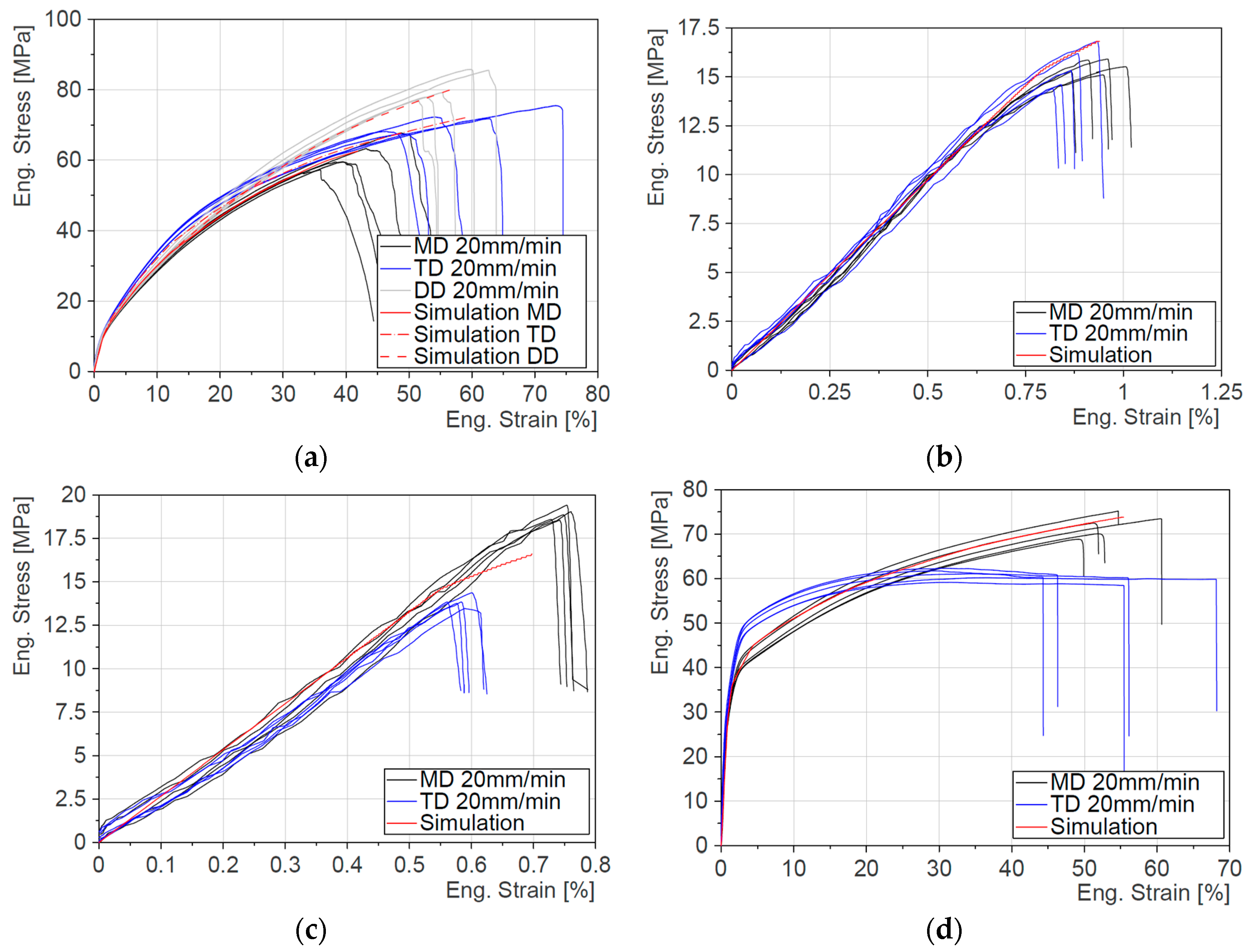
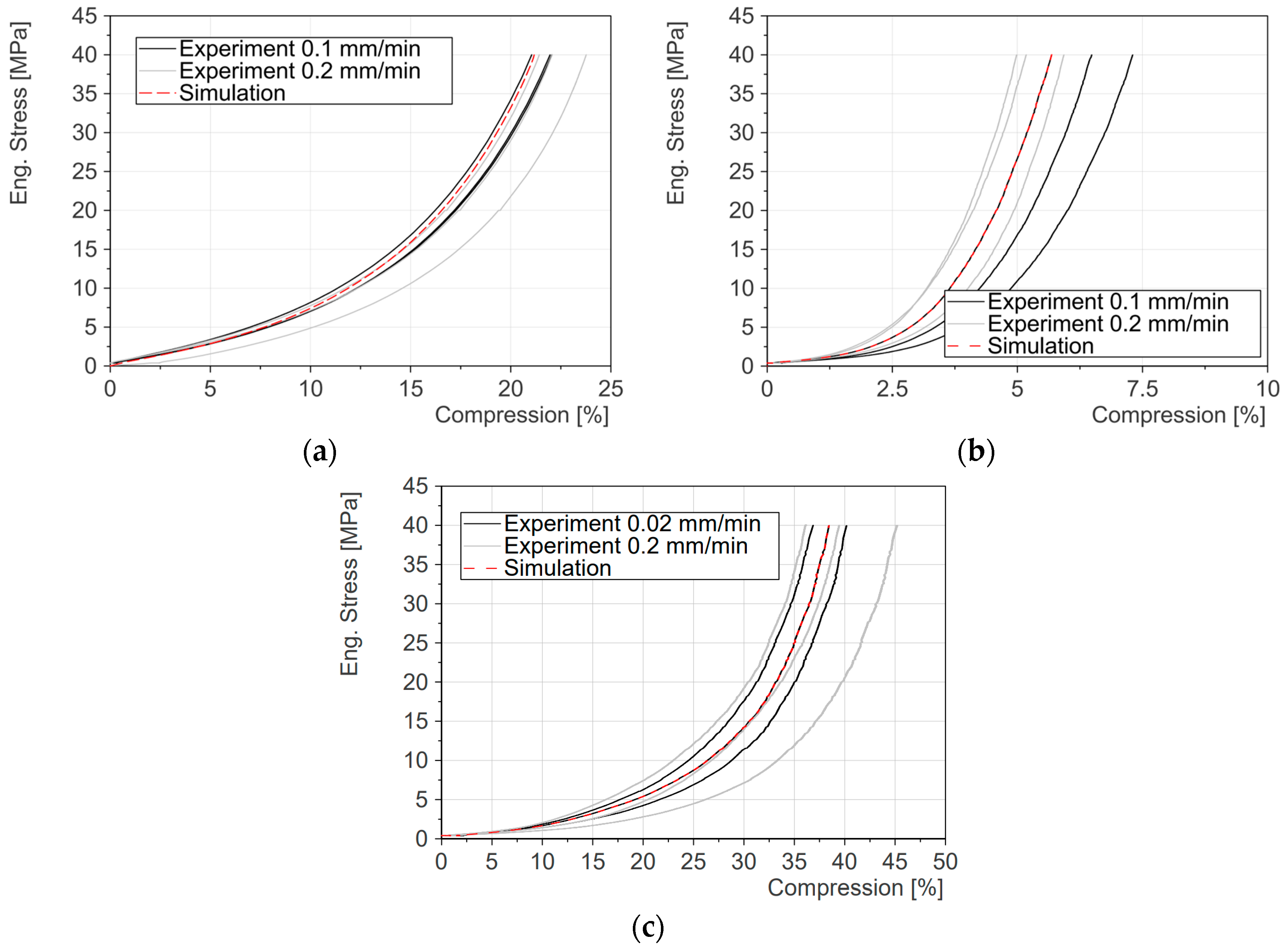

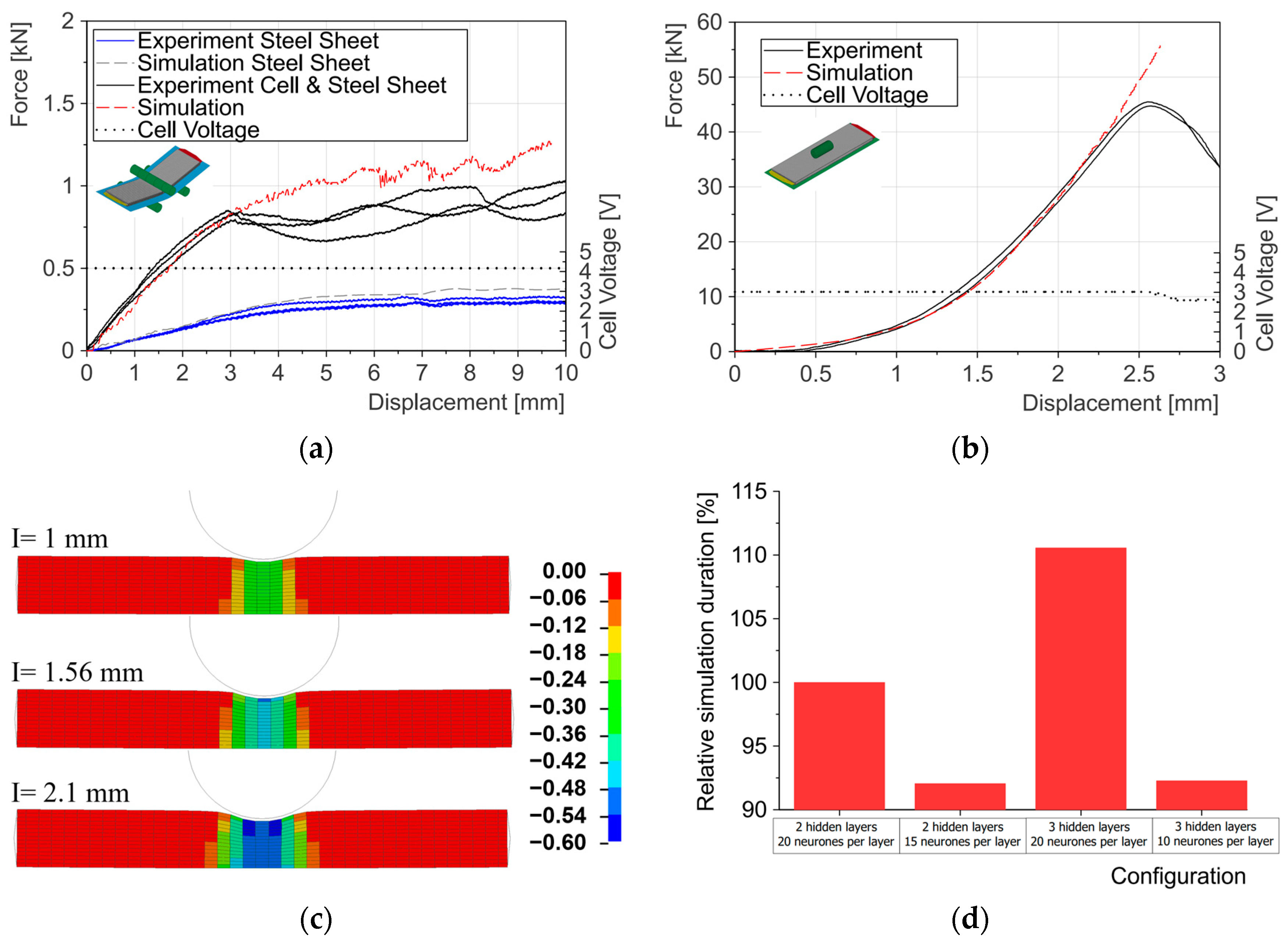
| Component | Material | Thickness [µm] | Number of Layers | Total Thickness [mm] |
|---|---|---|---|---|
| Anode | 166 | 33 | 5.478 | |
| Active Material | Graphite and SiO | 80 | ||
| Current Collector | Copper | 6 | ||
| Cathode | 142 | 32 | 4.544 | |
| Active Material | NMC | 65.15 | ||
| Current Collector | Aluminum | 11.7 | ||
| Separator | Polyolefin | 14 | 66 | 0.924 |
| Pouch | Aluminum and PET | 153 | 2 | 0.306 |
| Sum | 11.2502 | |||
| Cell | 11.25 |
| Parameter | Description |
|---|---|
| cm (*) | Material constants’ array |
| eps (6) | Strain increments |
| sig (6) | Stresses in previous time step |
| hsv (*) | History variables in previous time step |
| Component | Young’s Modulus [GPa] | Poisson Ratio | Yield Stress [MPa] | Failure Strain [%] |
|---|---|---|---|---|
| Separator | 1.5 | 0.01 | 4 | 42 (MD), 58 (TD), 55 (DD) |
| Anode (Current Collector) | 52.5 | 0.35 | 420 | 0.9 |
| Cathode (Current Collector) | 32 | 0.33 | 180 | 0.7 |
| Pouch | 3.3 | 0.35 | 30 | 55 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schmid, A.; Ellersdorfer, C.; Ewert, E.; Feist, F. Sequential Multi-Scale Modeling Using an Artificial Neural Network-Based Surrogate Material Model for Predicting the Mechanical Behavior of a Li-Ion Pouch Cell Under Abuse Conditions. Batteries 2024, 10, 425. https://doi.org/10.3390/batteries10120425
Schmid A, Ellersdorfer C, Ewert E, Feist F. Sequential Multi-Scale Modeling Using an Artificial Neural Network-Based Surrogate Material Model for Predicting the Mechanical Behavior of a Li-Ion Pouch Cell Under Abuse Conditions. Batteries. 2024; 10(12):425. https://doi.org/10.3390/batteries10120425
Chicago/Turabian StyleSchmid, Alexander, Christian Ellersdorfer, Eduard Ewert, and Florian Feist. 2024. "Sequential Multi-Scale Modeling Using an Artificial Neural Network-Based Surrogate Material Model for Predicting the Mechanical Behavior of a Li-Ion Pouch Cell Under Abuse Conditions" Batteries 10, no. 12: 425. https://doi.org/10.3390/batteries10120425
APA StyleSchmid, A., Ellersdorfer, C., Ewert, E., & Feist, F. (2024). Sequential Multi-Scale Modeling Using an Artificial Neural Network-Based Surrogate Material Model for Predicting the Mechanical Behavior of a Li-Ion Pouch Cell Under Abuse Conditions. Batteries, 10(12), 425. https://doi.org/10.3390/batteries10120425







