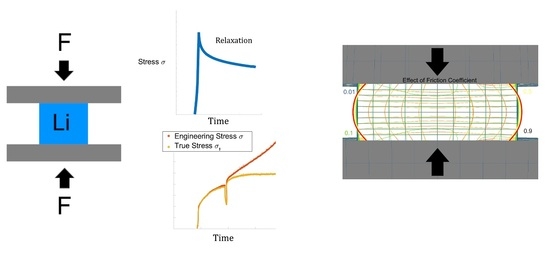
Lithium Metal under Static and Dynamic Mechanical Loading
Abstract
1. Introduction
2. Methods
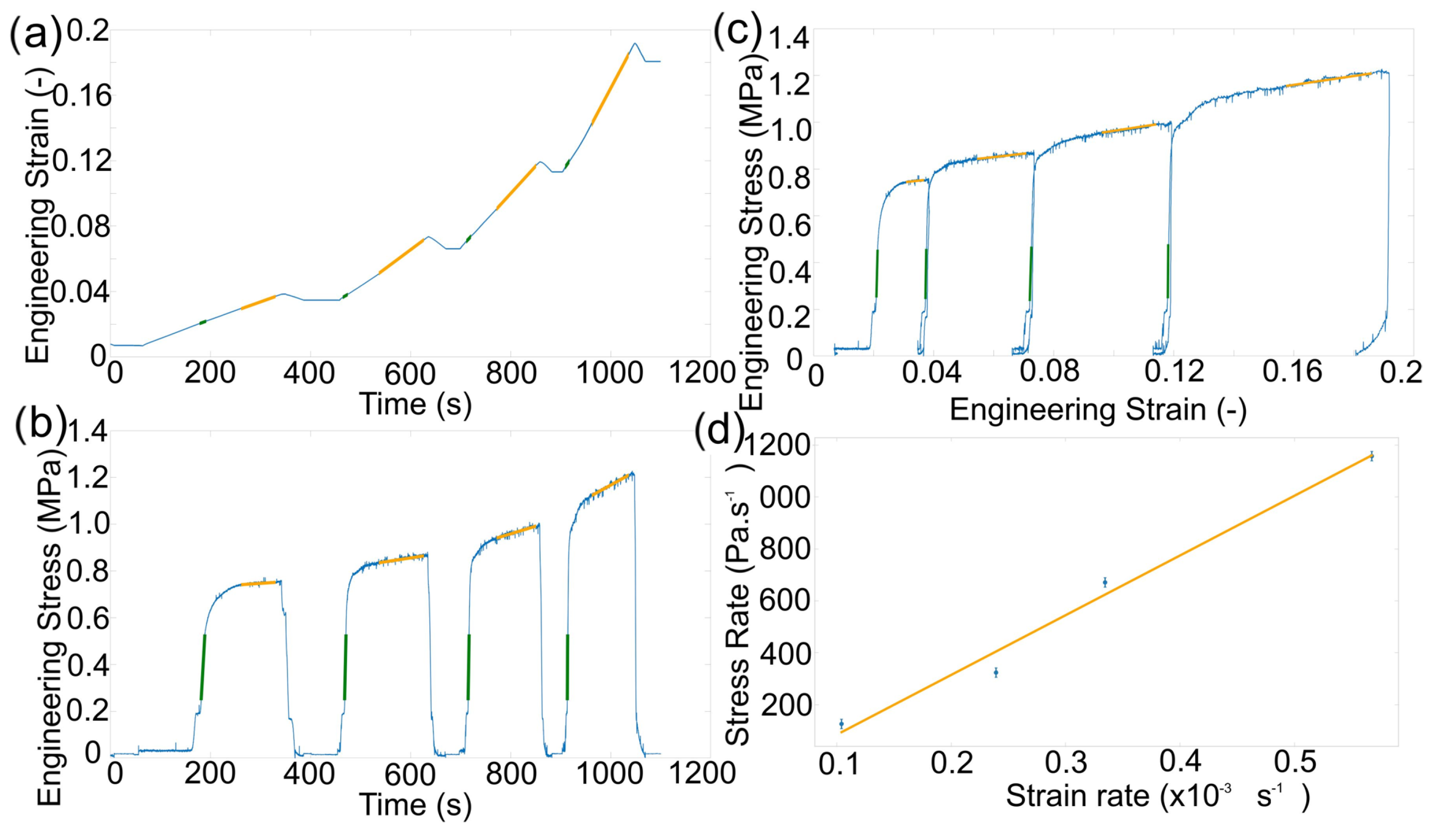
2.1. Compressive Forging and Strain Rate Observations
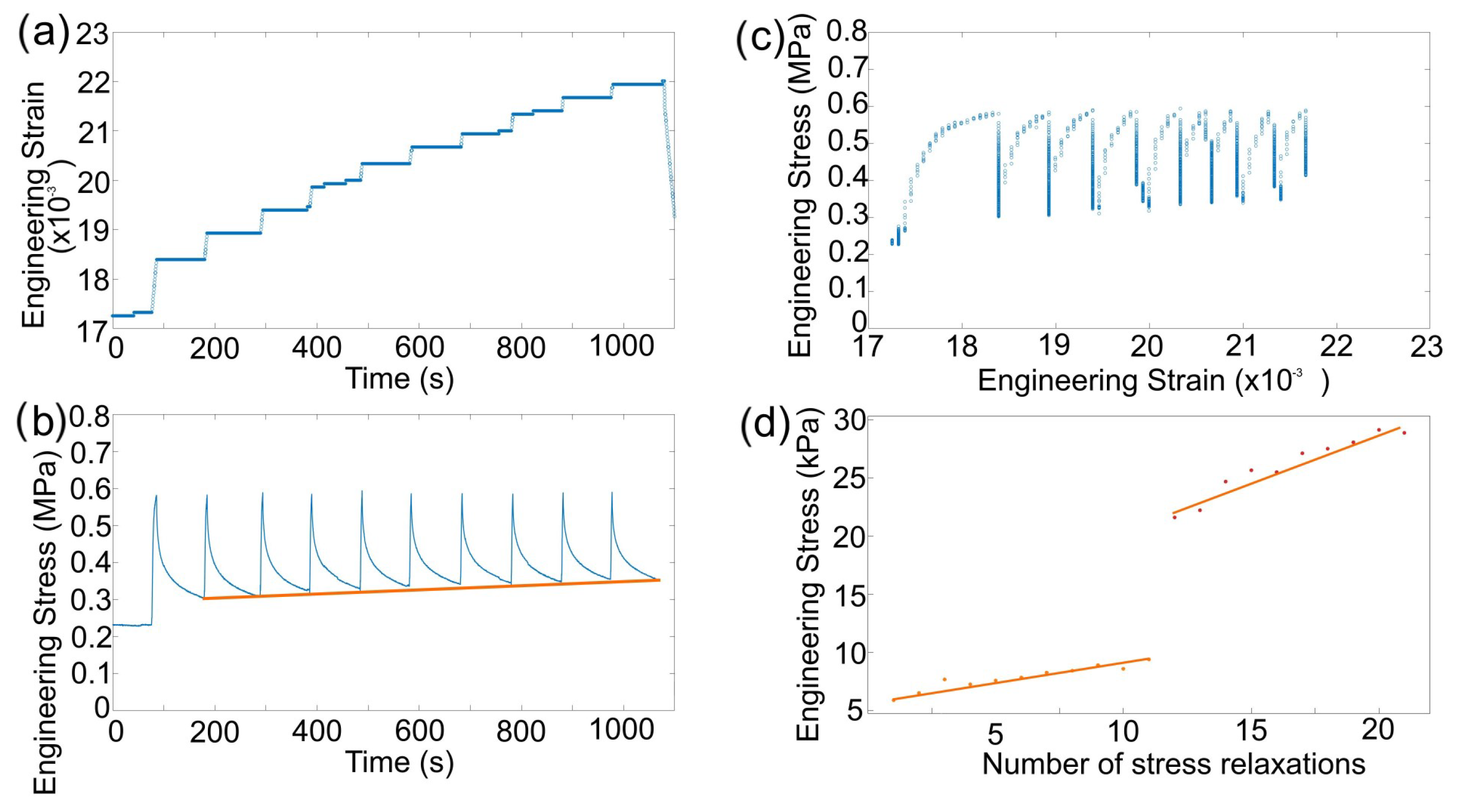
2.2. Stress Relaxation Observations
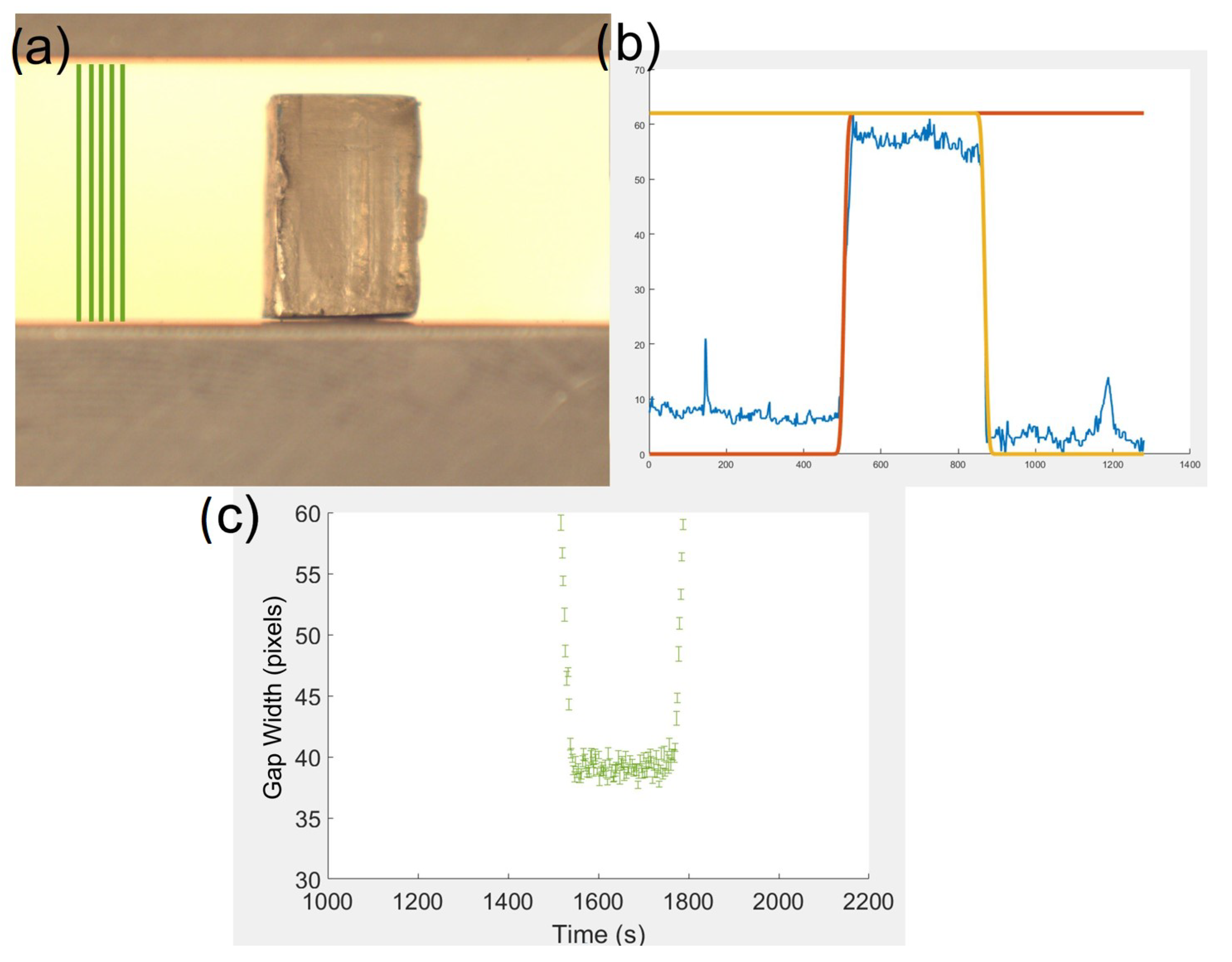
2.3. In-Situ Observation of Compression
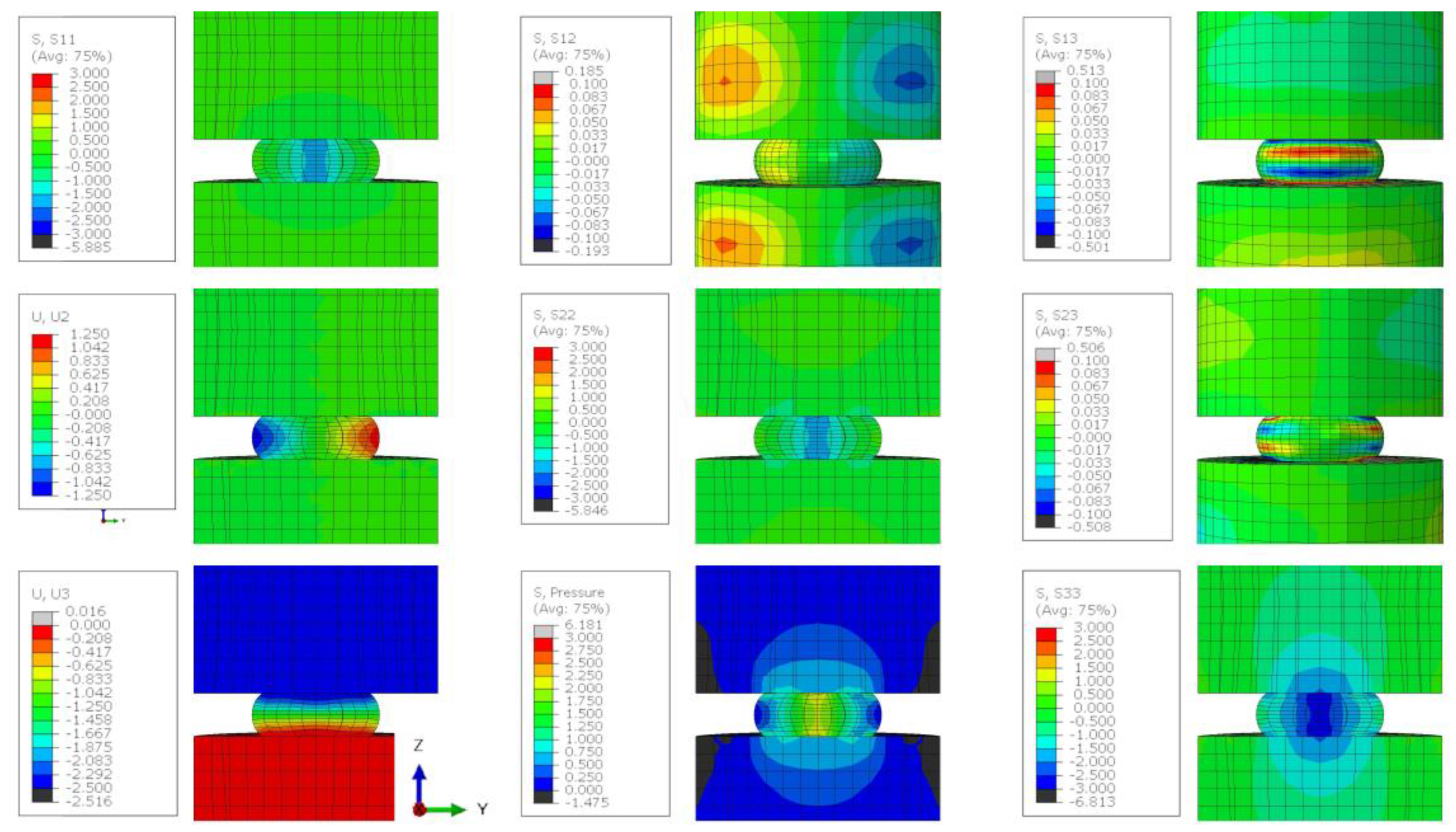
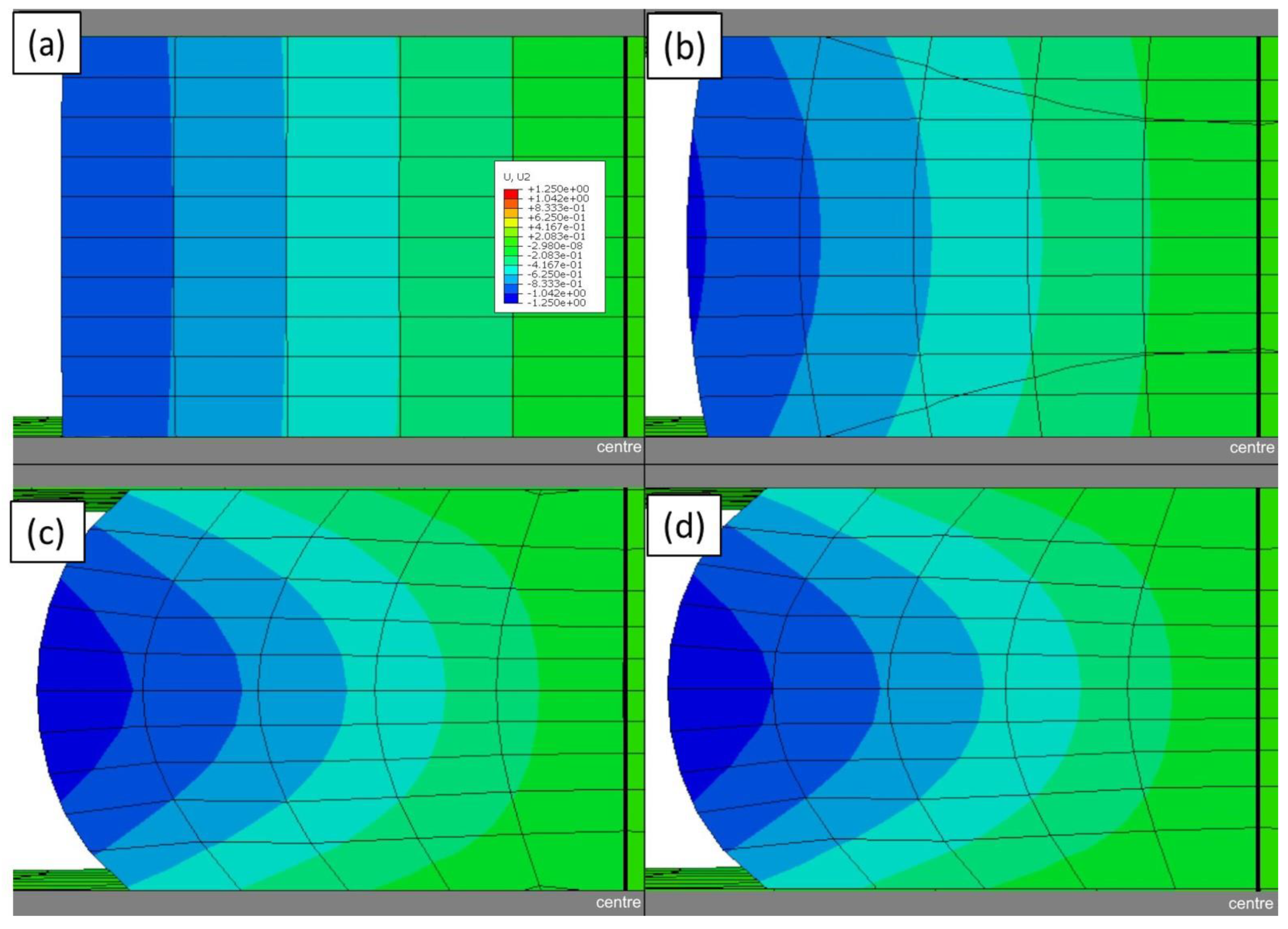
2.4. Finite Element Analysis
3. Results
3.1. Compressive Forging and Strain Rate Observations
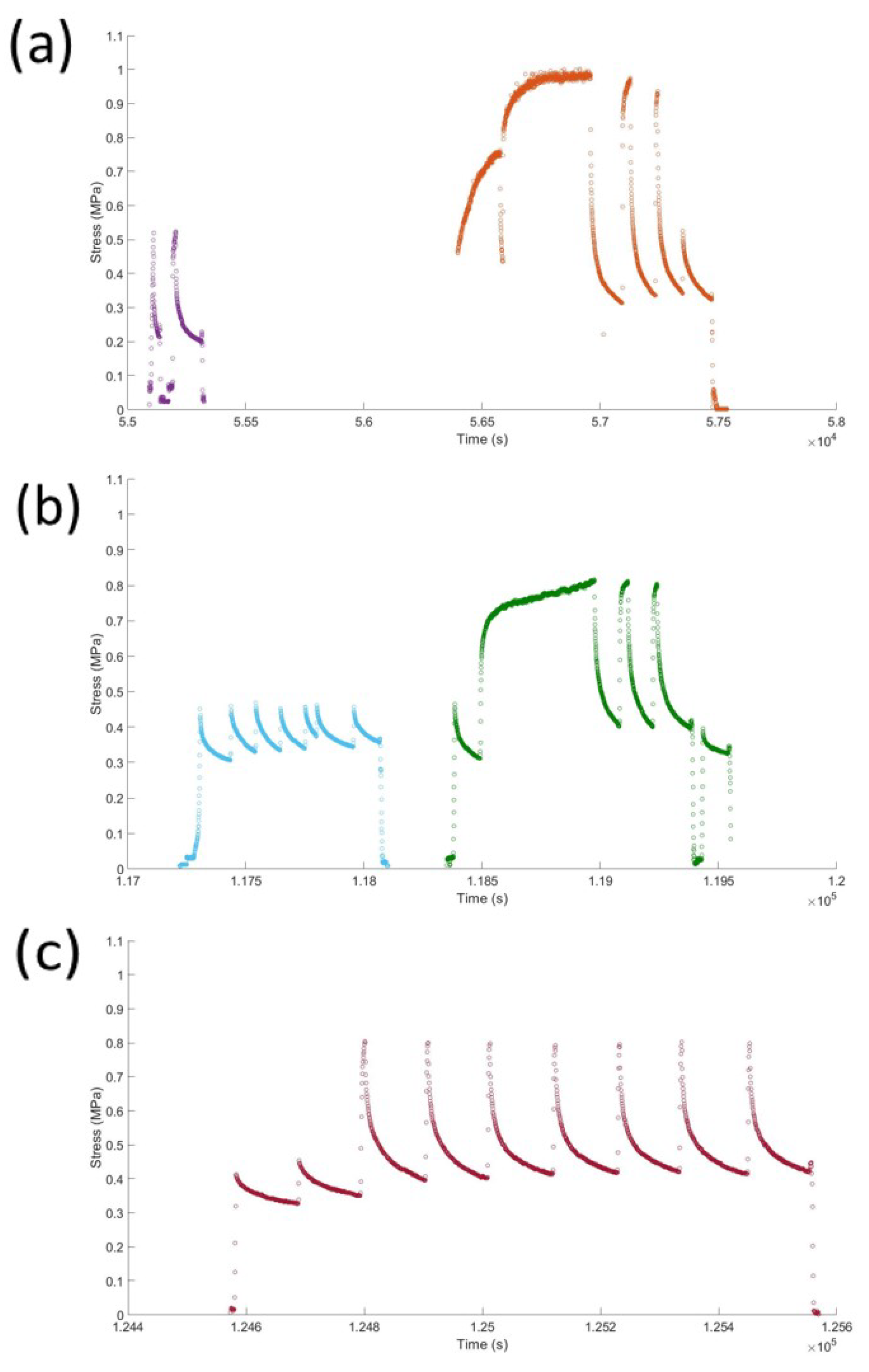
3.2. Stress Relaxation Observations
3.3. In Situ Observation of Compression
4. Discussion
4.1. Stack Pressure Effect on Thin Lithium Anodes
4.2. Producing Thin Lithium Anodes
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Effect of Sample Geometry on Forging
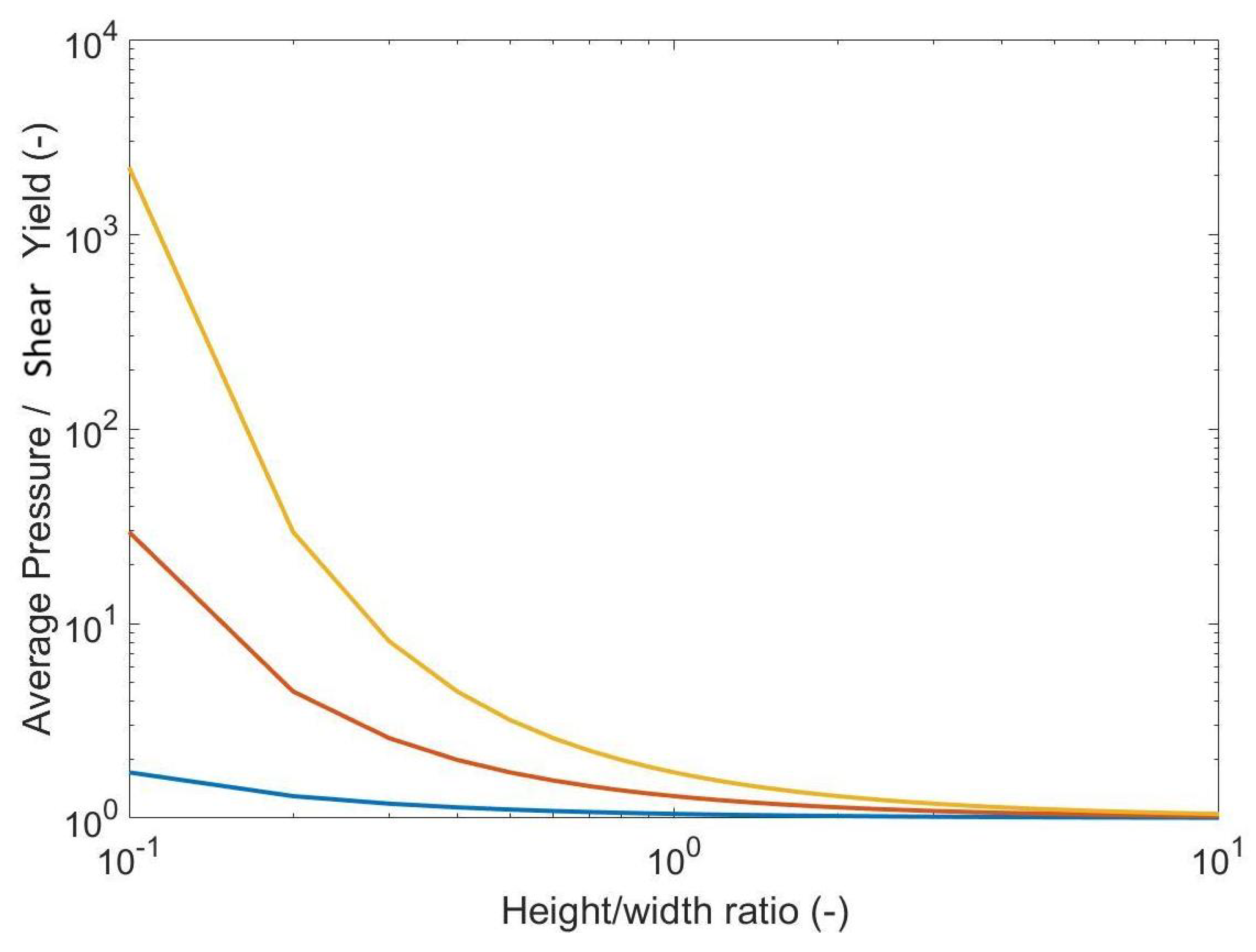
Appendix A.2. Pressure during Rolling
Appendix A.3. MATLAB Geometry Extraction
Appendix A.4. Further Data on Material Tested
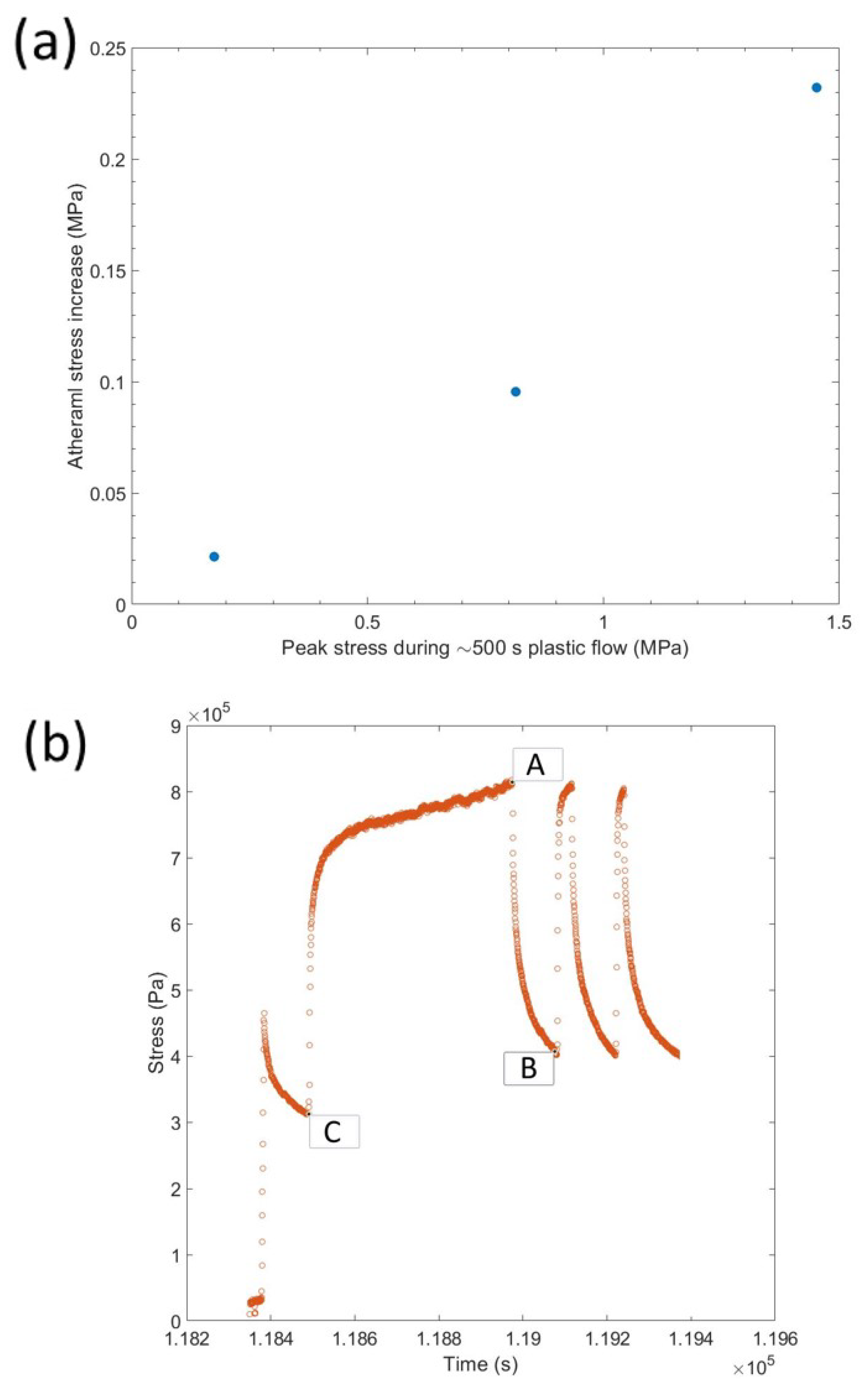
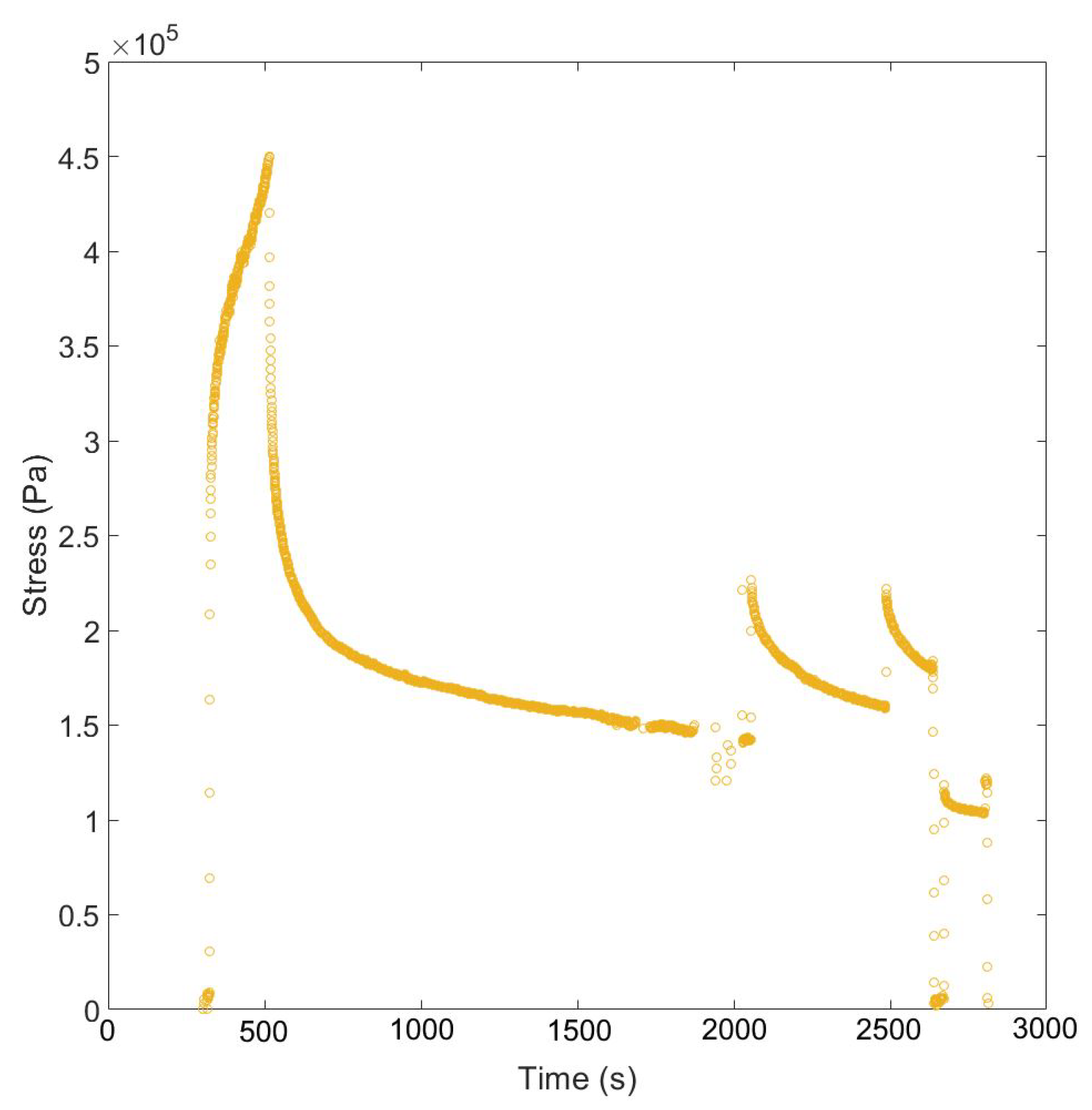
Appendix A.5. Further Finite Element Analysis Outputs
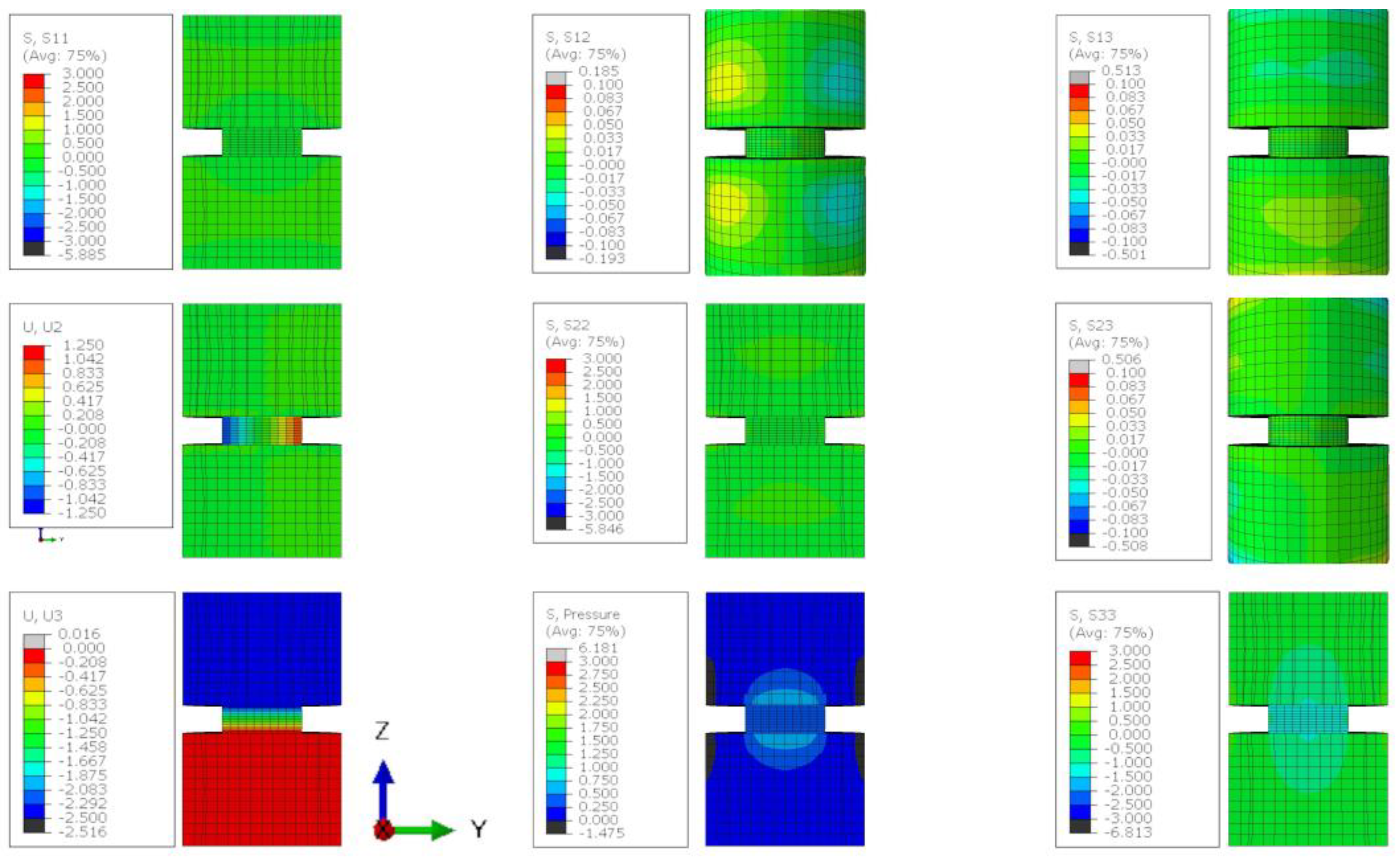
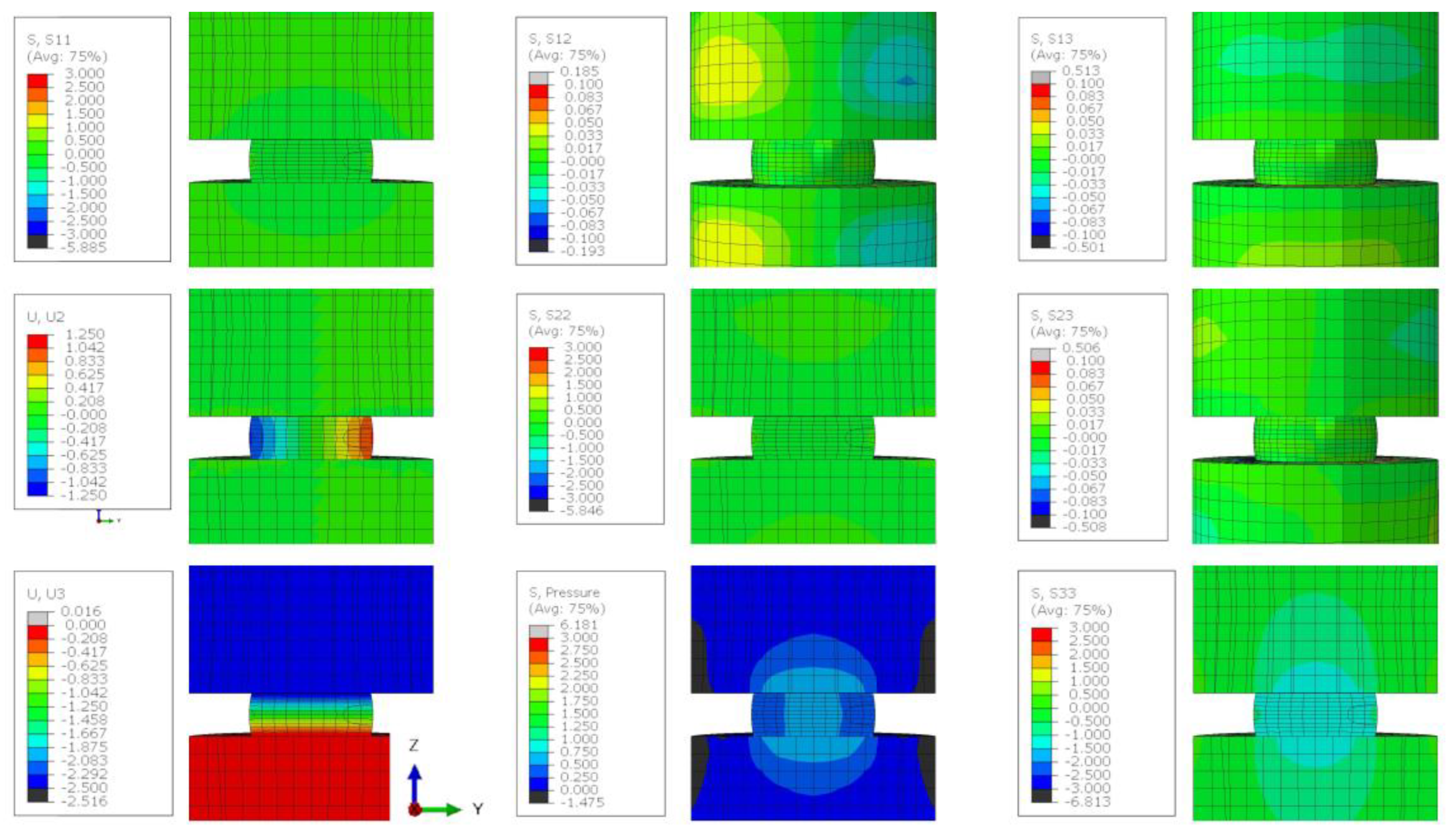


References
- Yoon, K.; Lee, S.; Oh, K.; Kang, K.; Yoon, K.; Lee, S.; Oh, K.; Kang, K. Challenges and Strategies towards Practically Feasible Solid-State Lithium Metal Batteries. Adv. Mater. 2022, 34, 2104666. [Google Scholar] [CrossRef] [PubMed]
- Pasta, M.; Armstrong, D.; Brown, Z.L.; Bu, J.; Castell, M.R.; Chen, P.; Cocks, A.; Corr, S.A.; Cussen, E.J.; Darnbrough, E.; et al. 2020 roadmap on solid-state batteries. J. Phys. Energy 2020, 2, 032008. [Google Scholar] [CrossRef]
- Hatzell, K.B.; Chen, X.C.; Cobb, C.L.; Dasgupta, N.P.; Dixit, M.B.; Marbella, L.E.; McDowell, M.T.; Mukherjee, P.P.; Verma, A.; Viswanathan, V.; et al. Challenges in Lithium Metal Anodes for Solid-State Batteries. ACS Energy Lett. 2020, 5, 922–934. [Google Scholar] [CrossRef]
- Wang, M.J.; Kazyak, E.; Dasgupta, N.P.; Sakamoto, J. Transitioning solid-state batteries from lab to market: Linking electro-chemo-mechanics with practical considerations. Joule 2021, 5, 1371–1390. [Google Scholar] [CrossRef]
- Sakamoto, J. More pressure needed. Nat. Energy 2019, 4, 827–828. [Google Scholar] [CrossRef]
- Kasemchainan, J.; Zekoll, S.; Spencer Jolly, D.; Ning, Z.; Hartley, G.O.; Marrow, J.; Bruce, P.G. Critical stripping current leads to dendrite formation on plating in lithium anode solid electrolyte cells. Nat. Mater. 2019, 18, 1105–1111. [Google Scholar] [CrossRef]
- Ning, Z.; Li, G.; Melvin, D.L.; Chen, Y.; Bu, J.; Spencer-Jolly, D.; Liu, J.; Hu, B.; Gao, X.; Perera, J.; et al. Dendrite initiation and propagation in lithium metal solid-state batteries. Nature 2023, 618, 287–293. [Google Scholar] [CrossRef]
- Darnbrough, E.; Aspinall, J.; Pasta, M.; Armstrong, D.E. Elastic and Plastic Mechanical Properties of Lithium measured by nanoindentation. Mater. Des. 2023, 233, 112200. [Google Scholar] [CrossRef]
- Masias, A.; Felten, N.; Garcia-Mendez, R.; Wolfenstine, J.; Sakamoto, J. Elastic, plastic, and creep mechanical properties of lithium metal. J. Mater. Sci. 2019, 54, 2585–2600. [Google Scholar] [CrossRef]
- Masias, A.; Felten, N.; Sakamoto, J. Characterizing the mechanical behavior of lithium in compression. J. Mater. Res. 2021, 36, 729–739. [Google Scholar] [CrossRef]
- Fincher, C.D.; Ojeda, D.; Zhang, Y.; Pharr, G.M.; Pharr, M. Mechanical properties of metallic lithium: From nano to bulk scales. Acta Mater. 2020, 186, 215–222. [Google Scholar] [CrossRef]
- Fan, H.; Wang, Q.; El-Awady, J.A.; Raabe, D.; Zaiser, M. Strain rate dependency of dislocation plasticity. Nat. Commun. 2021, 12, 1845. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Ahmad, Z.; Aryanfar, A.; Viswanathan, V.; Greer, J.R. Enhanced strength and temperature dependence of mechanical properties of Li at small scales and its implications for Li metal anodes. Proc. Natl. Acad. Sci. USA 2017, 114, 57–61. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Yang, T.; Du, C.; Liu, Q.; Tang, Y.; Zhao, J.; Wang, B.; Chen, T.; Sun, Y.; Jia, P.; et al. Lithium whisker growth and stress generation in an in situ atomic force microscope–environmental transmission electron microscope set-up. Nat. Nanotechnol. 2020, 15, 94–98. [Google Scholar] [CrossRef] [PubMed]
- Ding, S.; Fairgrieve-Park, L.; Sendetskyi, O.; Fleischauer, M.D. Compressive creep deformation of lithium foil at varied cell conditions. J. Power Sources 2021, 488, 229404. [Google Scholar] [CrossRef]
- Haslam, C.G.; Wolfenstine, J.B.; Sakamoto, J. The effect of aspect ratio on the mechanical behavior of Li metal in solid-state cells. J. Power Sources 2022, 520, 230831. [Google Scholar] [CrossRef]
- Ledbetter, H.M.; Frederick, N.V.; Austin, M.W. Elastic-constant variability in stainless-steel 304. J. Appl. Phys. 1980, 51, 305–309. [Google Scholar] [CrossRef]
- Aspinall, J.; Armstrong, D.E.; Pasta, M. EBSD-coupled indentation: Nanoscale mechanics of lithium metal. Mater. Today Energy 2022, 30, 101183. [Google Scholar] [CrossRef]
- Caillard, D.; Martin, J.L. Thermally Activated Mechanisms in Crystal Plasticity; Elsevier: Amsterdam, The Netherlands, 2003; p. 433. [Google Scholar]
- LePage, W.S.; Chen, Y.; Kazyak, E.; Chen, K.H.; Sanchez, A.J.; Poli, A.; Arruda, E.M.; Thouless, M.D.; Dasgupta, N.P. Lithium Mechanics: Roles of Strain Rate and Temperature and Implications for Lithium Metal Batteries. J. Electrochem. Soc. 2019, 166, A89–A97. [Google Scholar] [CrossRef]
- Khoddam, S.; Hodgson, P.D. Advancing mechanics of Barrelling Compression Test. Mech. Mater. 2018, 122, 1–8. [Google Scholar] [CrossRef]
- Ebrahimi, R.; Najafizadeh, A. A new method for evaluation of friction in bulk metal forming. J. Mater. Process. Technol. 2004, 152, 136–143. [Google Scholar] [CrossRef]
- Hosford, W.F.; Caddell, R.M. Metal Forming: Mechanics and Metallurgy; Cambridge University Press: New York, NY, USA, 2011. [Google Scholar]
- Stallard, J.; Vema, S.; Grey, C.; Deshpande, V.; Fleck, N. The strength of a constrained lithium layer. Acta Mater. 2023, 260, 119313. [Google Scholar] [CrossRef]
- Krauskopf, T.; Hartmann, H.; Zeier, W.G.; Janek, J. Toward a Fundamental Understanding of the Lithium Metal Anode in Solid-State Batteries—An Electrochemo-Mechanical Study on the Garnet-Type Solid Electrolyte Li6.25Al0.25La3Zr2O12. ACS Appl. Mater. Interfaces 2019, 11, 14463–14477. [Google Scholar] [CrossRef] [PubMed]
- Doux, J.M.J.M.; Nguyen, H.; S Tan, D.H.; Banerjee, A.; Wang, X.; Wu, E.A.; Jo, C.; Yang, H.; Shirley Meng, Y.; Doux, J.M.J.M.; et al. Stack Pressure Considerations for Room-Temperature All-Solid-State Lithium Metal Batteries. Adv. Energy Mater. 2020, 10, 1903253. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Q.J.; Harrison, K.L.; Jungjohann, K.; Boyce, B.L.; Roberts, S.A.; Attia, P.M.; Harris, S.J. Rethinking How External Pressure Can Suppress Dendrites in Lithium Metal Batteries. J. Electrochem. Soc. 2019, 166, A3639–A3652. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Q.J.; Harrison, K.L.; Roberts, S.A.; Harris, S.J. Pressure-Driven Interface Evolution in Solid-State Lithium Metal Batteries. Cell Rep. Phys. Sci. 2020, 1, 100012. [Google Scholar] [CrossRef]
- Acebedo, B.; Morant-Miñana, M.C.; Gonzalo, E.; Ruiz de Larramendi, I.; Villaverde, A.; Rikarte, J.; Fallarino, L.; Acebedo, B.; Morant-Miñana, M.C.; Gonzalo, E.; et al. Current Status and Future Perspective on Lithium Metal Anode Production Methods. Adv. Energy Mater. 2023, 13, 2203744. [Google Scholar] [CrossRef]
- Duan, J.; Wu, W.; Nolan, A.M.; Wang, T.; Wen, J.; Hu, C.; Mo, Y.; Luo, W.; Huang, Y.; Duan, J.; et al. Lithium–Graphite Paste: An Interface Compatible Anode for Solid-State Batteries. Adv. Mater. 2019, 31, 1807243. [Google Scholar] [CrossRef]
- Schönherr, K.; Schumm, B.; Hippauf, F.; Lissy, R.; Althues, H.; Leyens, C.; Kaskel, S. Liquid lithium metal processing into ultrathin metal anodes for solid state batteries. Chem. Eng. J. Adv. 2022, 9, 100218. [Google Scholar] [CrossRef]
- Schmuch, R.; Wagner, R.; Hörpel, G.; Placke, T.; Winter, M. Performance and cost of materials for lithium-based rechargeable automotive batteries. Nat. Energy 2018, 3, 267–278. [Google Scholar] [CrossRef]
- Shi, F.; Pei, A.; Vailionis, A.; Xie, J.; Liu, B.; Zhao, J.; Gong, Y.; Cui, Y. Strong texturing of lithium metal in batteries. Proc. Natl. Acad. Sci. USA 2017, 114, 12138–12143. [Google Scholar] [CrossRef] [PubMed]
- Spencer Jolly, D.; Ning, Z.; Hartley, G.O.; Liu, B.; Melvin, D.L.; Adamson, P.; Marrow, J.; Bruce, P.G. Temperature Dependence of Lithium Anode Voiding in Argyrodite Solid-State Batteries. ACS Appl. Mater. Interfaces 2021, 13, 22708–22716. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Darnbrough, E.; Armstrong, D.E.J. Lithium Metal under Static and Dynamic Mechanical Loading. Batteries 2024, 10, 20. https://doi.org/10.3390/batteries10010020
Darnbrough E, Armstrong DEJ. Lithium Metal under Static and Dynamic Mechanical Loading. Batteries. 2024; 10(1):20. https://doi.org/10.3390/batteries10010020
Chicago/Turabian StyleDarnbrough, Ed, and David E. J. Armstrong. 2024. "Lithium Metal under Static and Dynamic Mechanical Loading" Batteries 10, no. 1: 20. https://doi.org/10.3390/batteries10010020
APA StyleDarnbrough, E., & Armstrong, D. E. J. (2024). Lithium Metal under Static and Dynamic Mechanical Loading. Batteries, 10(1), 20. https://doi.org/10.3390/batteries10010020