Magnetic and Magnetocaloric Properties of Nano- and Polycrystalline Bulk Manganites La_0.7Ba_(0.3−x)Ca_xMnO₃ (x ≤ 0.25)

Abstract

Here we report the synthesis and investigation of bulk and nano-sized La_0.7Ba_0.3−xCa_xMnO₃ (x = 0, 0.15, 0.2 and 0.25) compounds that are promising candidates for magnetic refrigeration applications. We compare the structural and magnetic properties of bulk and nano-scale polycrystalline La_0.7Ba_0.3−xCa_xMnO₃ for potential use in magnetic cooling systems. Solid-state reactions were implemented for bulk materials, while the sol–gel method was used for nano-sized particles. Structurally and morphologically, the samples were investigated by X-ray diffraction (XRD), optical microscopy and transmission electron microscopy (TEM). Oxygen stoichiometry was investigated by iodometry. Bulk compounds exhibit oxygen deficiency, while nano-sized particles show excess oxygen. Critical magnetic behavior was revealed for all samples using the modified Arrott plot (MAP) method and confirmed by the Kouvel–Fisher (KF) method. The bulk polycrystalline compound behavior was better described by the tricritical field model, while the nanocrystalline samples were governed by the mean-field model. Resistivity in bulk material showed a peak at a temperature T_p1 attributed to grain boundary conditions and at T_p2 associated with a Curie temperature of T_c. Parent polycrystalline sample La_0.7Ba_0.3MnO₃ has T_c at 340 K. Substitution of x = 0.15 of Ca brings T_c to 308 K, and x = 0.2 brings it to 279 K. Nanocrystalline samples exhibit a very wide effective temperature range in the magnetocaloric effect, up to 100 K. Bulk compounds exhibit a high and sharp peak in magnetic entropy change, up to 7 J/kgK at 4 T at T_c for x = 0.25. To compare the magnetocaloric performances of the studied compounds, both relative cooling power (RCP) and temperature-averaged entropy change (TEC) figures of merit were used. RCP is comparable for bulk polycrystalline and nano-sized samples of the same substitution level, while TEC shows a large difference between the two systems. The combination of bulk and nanocrystalline materials can contribute to the effectiveness and improvement of magnetocaloric materials.

1. Introduction

Magnetism is not just a metaphor for love used by poets. As it turns out, magnetism can also help levitate trains [1] full of men hurrying to their daily lives. It can also help create numerous devices that help them communicate. But laws of physics cause the same devices to overheat, putting limits on our desire for unlimited communications and information. Men have tried to cope with the problem, some with liquids and some with gases, but efficiency was not very high and was even harmful to the environment [1]. In recent years, scientific “eyes” have turned back to this mysterious quality called magnetism. Through methodical investigation, safe and efficient materials have revealed their potential [2].

In the last couple of decades, manganites of the type A_1−xB_xMnO₃ (where A is a trivalent rare earth cation and B is a divalent alkaline earth cation) have garnered attention for their high magnetic entropy change at T_c (when magnetic field intensity is changed) as well as for their colossal magnetoresistance (CMR) [3].

Compounds such as La_1−xSr_xMnO₃ and Pr_1−xBa_xMnO₃ [4,5] have exhibited large magnetic entropy changes, which are achieved by doping divalent Sr and Ba in place of trivalent La and Pr. Such actions create a mismatch in neighboring orbitals of manganese (Mn). This Mn³⁺-O-Mn⁴⁺ arrangement allows for ferromagnetic behavior in a process called “double exchange” [6,7]. The best ratio of doping is found to be about x = 0.3 of Mn⁴⁺ ions; a lower amount does not result in strong ferromagnetism, and doping closer to 0.5 can result in an anti-ferromagnetic change ordered state [7]. Further addition of divalent but different-sized atoms to the structure will change the structure’s shape and volume, leading to changes in properties such as T_c [7,8]. In addition, changing materials from bulk to nanocrystalline states can greatly affect properties such as magnetic behavior. Furthermore, the size of the nano-particles can affect the same properties [9], which opens many more avenues for research.

In this paper, we investigate the magnetic and electrical properties along with the critical behavior of bulk and nano-sized La_0.7Ba_0.3−xCa_xMnO₃ (x = 0.15, 0.2 and 0.25). Interesting and sometimes promising research conducted recently [9,10,11,12] on similar compounds showing high values in magnetization, CMR and magnetocaloric effect calls for further investigation in the search for possible improvements. While the parent samples La_0.7Ba_0.3MnO₃ exhibit T_c at 340 K and 263 K for bulk and nano-particle samples, respectively, the substitution of smaller calcium (Ca) atoms in place of barium (Ba) leads to increased disorder and smaller cell volume, resulting in lower values of T_c (which can be of interest for technical applications, such as magnetic refrigeration). Both systems were investigated by XRD analysis, and they were found to show a change in symmetry from the Rhombohedral (R-3c) to the Orthorhombic (pbnm) group for x = 0.25. Critical magnetic behavior was found to be closer to the tricritical mean-field model for bulk samples and the mean-field model for the nano-particles. Bulk samples exhibit a high magnetic entropy change ΔS_M for all samples at T_c. Nano-particles span a wide range of effective temperatures for ΔS_M (up to 100 K). All bulk samples exhibit transition temperatures near room temperature, with x = 0.2 being the closest at 279 K.

This paper is organized as follows: in Section 2, we describe the precursors, equipment and preparation methods, as well as all the characterization methods we used from structural, morphological, oxygen stoichiometric, electrical, and magnetic perspectives. In Section 3, we present the results of our investigation and analysis of data, and we discuss the critical behavior and electrical and magnetic properties of our samples. In the end, we summarize our results in Section 4.

2. Materials and Methods

2.1. Materials

Precursors for bulk materials, bought from Alfa Aesar (Heysham, UK), consist of oxides La₂O₃ (99.9%), MnO₂ (99.9%), and carbonates BaCO₃ (99.9%) and CaCO₃ (99.99%).

Precursors for nanocrystalline samples: nitrates La₂(NO₃)₃·6H₂O, Ca(NO₃)₂·4H₂O, Ba(NO₃)₂, Mn(NO₃)₂·6H₂O

2.2. Preparation

All bulk samples were prepared by conventional solid-state reactions. Precursors were mixed by hand in an agate mortar for 3 h and calcinated for 24 h in the air at 1100 °C. Next, the samples were pressed into pellets at 3 tons and sintered at 1350 °C for 30 h.

Nano-sized samples were prepared using the sol–gel method. Precursors were mixed in 150 mL of pure water (18.2 MΩ × cm at 25 °C) for 45–60 min at 60 °C. About 10 g of sucrose was added, and the solution was mixed further for 45 min. This allowed positive ions to attach themselves to the sucrose chain. Next, the mixture was cooled, and 2 g of pectin was added in order to expand the xerogel. It was stirred for 20 min. The final mixture was dried in the air at 200 °C for 24 h and burned in the oven at 1000 °C for 2 h.

2.3. Structural Characterization

Samples were structurally categorized using X-ray diffraction (XRD). Data from XRD was analyzed by the FULLPROF Rietveld refinement technique. Optical microscopy was used on bulk samples to determine grain size, and transmission electron microscopy (TEM) was used to determine the size of nano-sized particles. In addition, the Williamson–Hall method was used to confirm and compare the experimental results.

Oxygen stoichiometry was determined by iodometric analytical titration. In iodometry, a small amount of the sample is placed in hydrochloric (HCl) acid, in which Mn⁺ reacts with ions of Cl⁻ to produce Cl₂. The Cl₂ is then pushed by an inert gas into another vessel containing potassium iodide (KI). The resulting iodine molecules in the solution are titrated with sodium thiosulfate. Then, the ratio of Mn³⁺ and Mn⁴⁺ is calculated through balanced chemical equations [13].

2.4. Electrical and Magnetic Properties

Electrical properties of the bulk samples were determined using the four-point technique in a cryogen-free superconducting setup. Four-point chips, which measure voltage and current separately, were placed in a temperature range of between 10 K and 290 K in applied magnetic fields of up to 7 T and recorded the resistance of the samples.

Magnetic measurements were made using a Vibrating Sample Magnetometer (VSM) in the range of 4–300 K and in magnetic fields of up to 4 T.

3. Results

3.1. Structural Analysis

Figure 1 presents stacked XRD patterns and Rietveld refinement results for both systems. Visual inspection of the graphs shows single-phase samples and wider peaks for nanocrystalline samples. A gradual shift to the right with an increasing level of substitution, except for x = 0.25, which shifts slightly to the left, can be observed more clearly in the Supplementary Materials in the XRD graphs. This is indicative of possible diminishing cell dimensions and a change in symmetry for x = 0.25 [14].

Rietveld refinement analysis confirms the Rhombohedral (R-3c) no. 167 space group for the parent and all samples with x = 0.15, 0.2. Compounds with x = 0.25 for bulk and nano-sized systems belong to the Orthorhombic (pbnm) no. 62 space group. Lattice parameters and cell volume diminish with increased substitution as a result of smaller Ca²⁺ ions having a smaller crystal radius (1.26 Å) than Ba²⁺ ions (1.56 Å). This changes the Mn-O-Mn angle and Mn-O bond length, creating distortion in the Mn-O octahedral [7,15]. The results are presented in

Table 1. Calculated lattice parameters, cell volume, goodness of fit and space groups for both systems calculated using Rietveld refinement analysis.

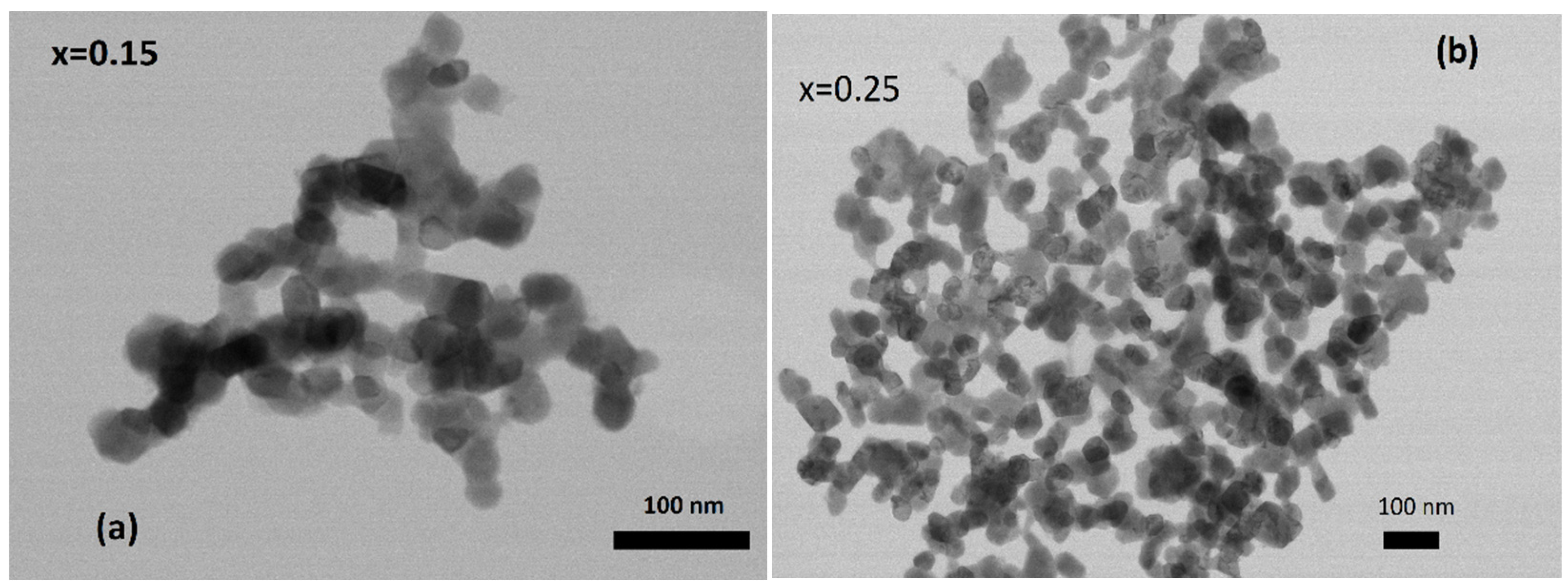
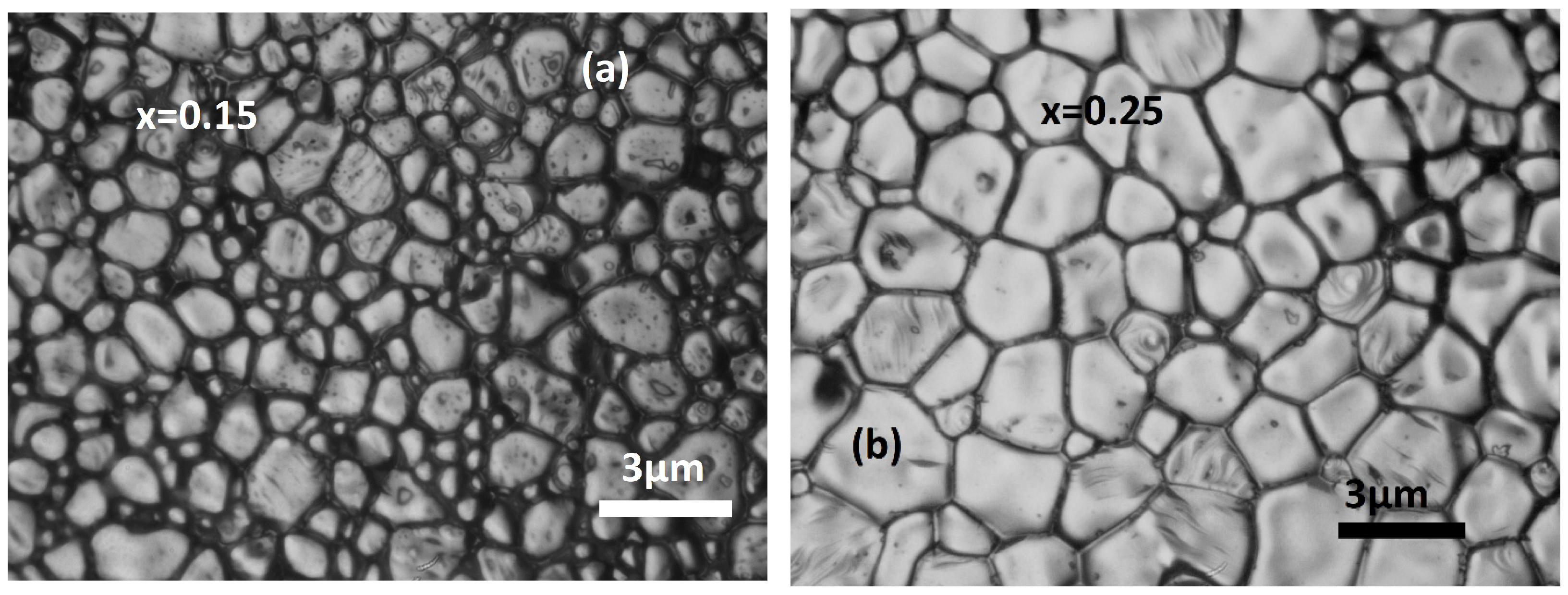
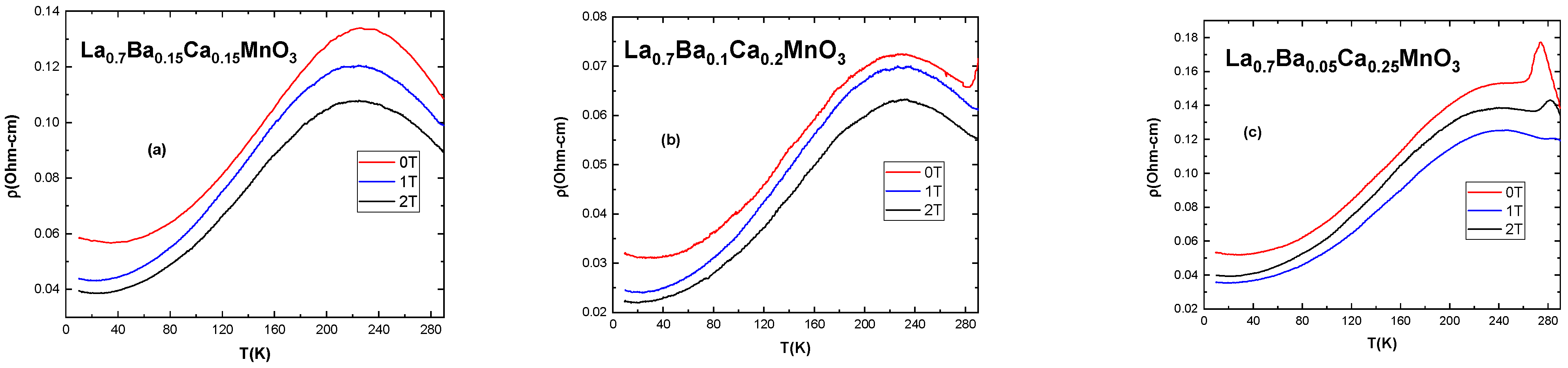
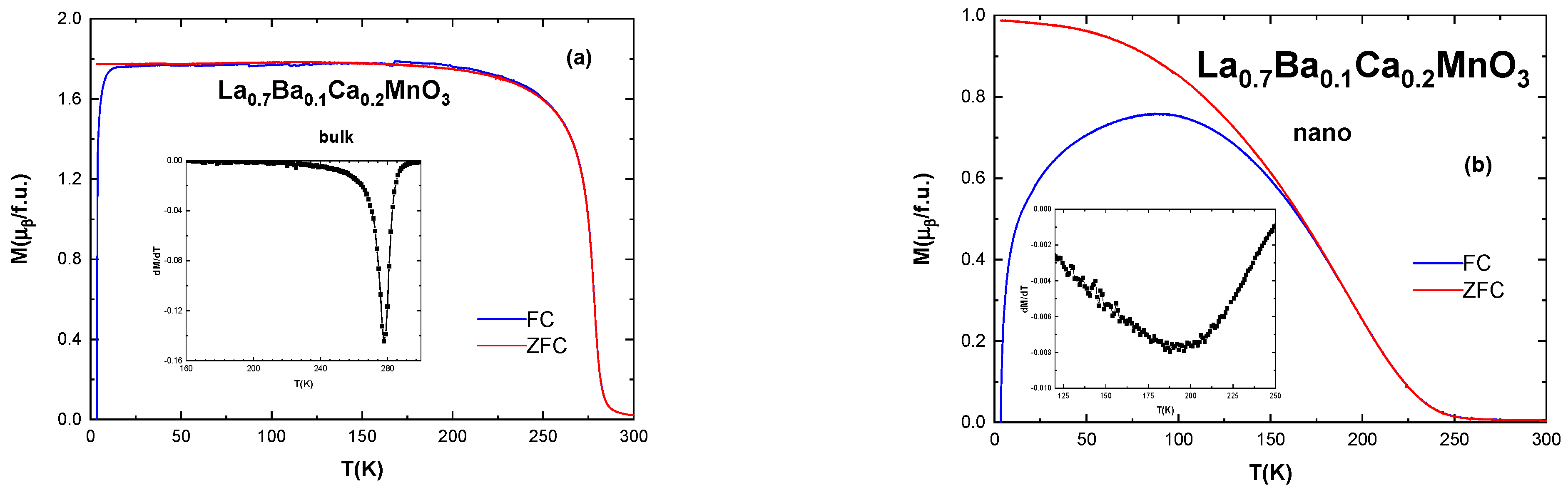
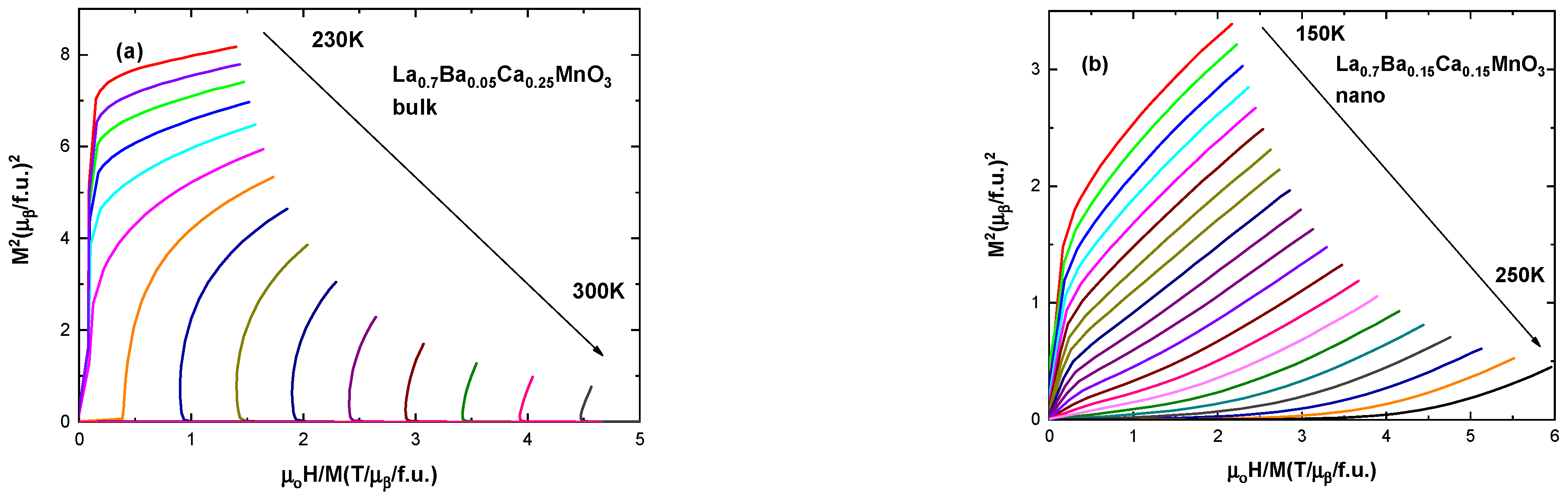
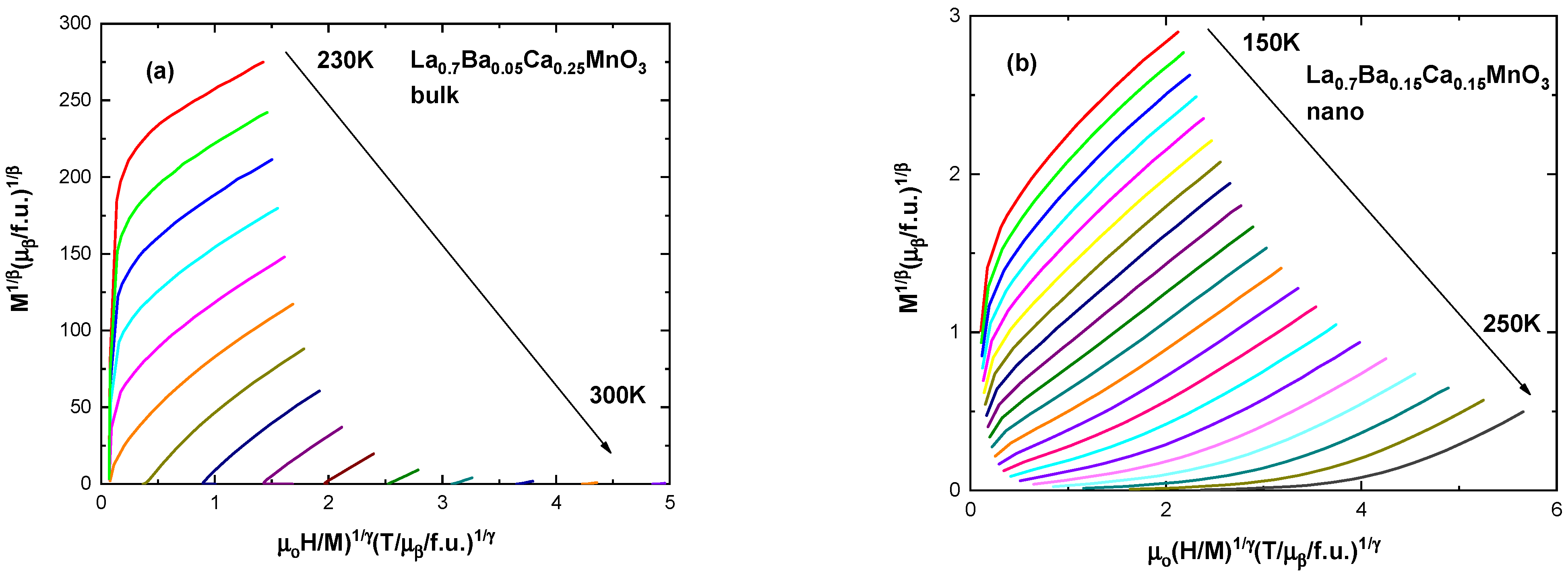
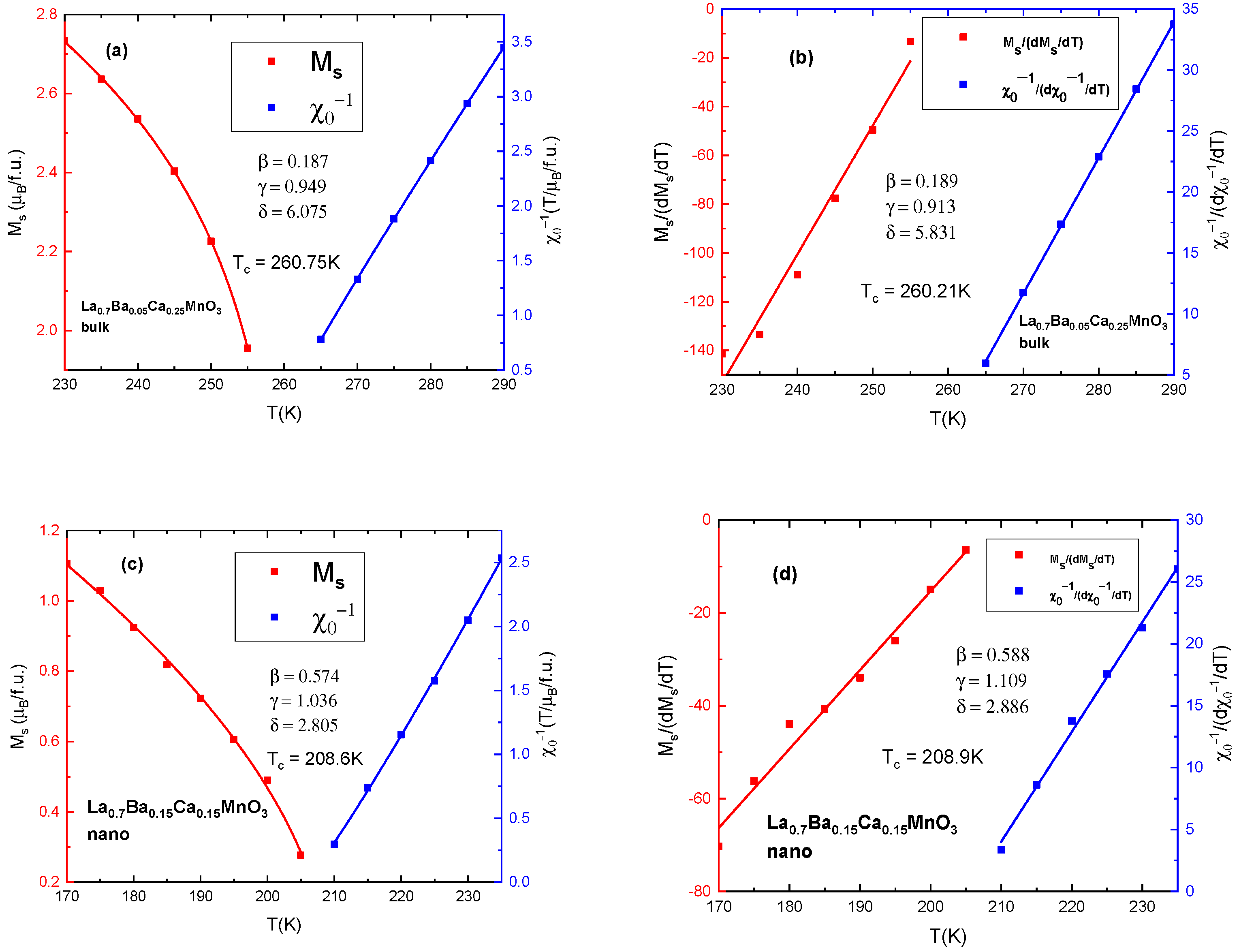
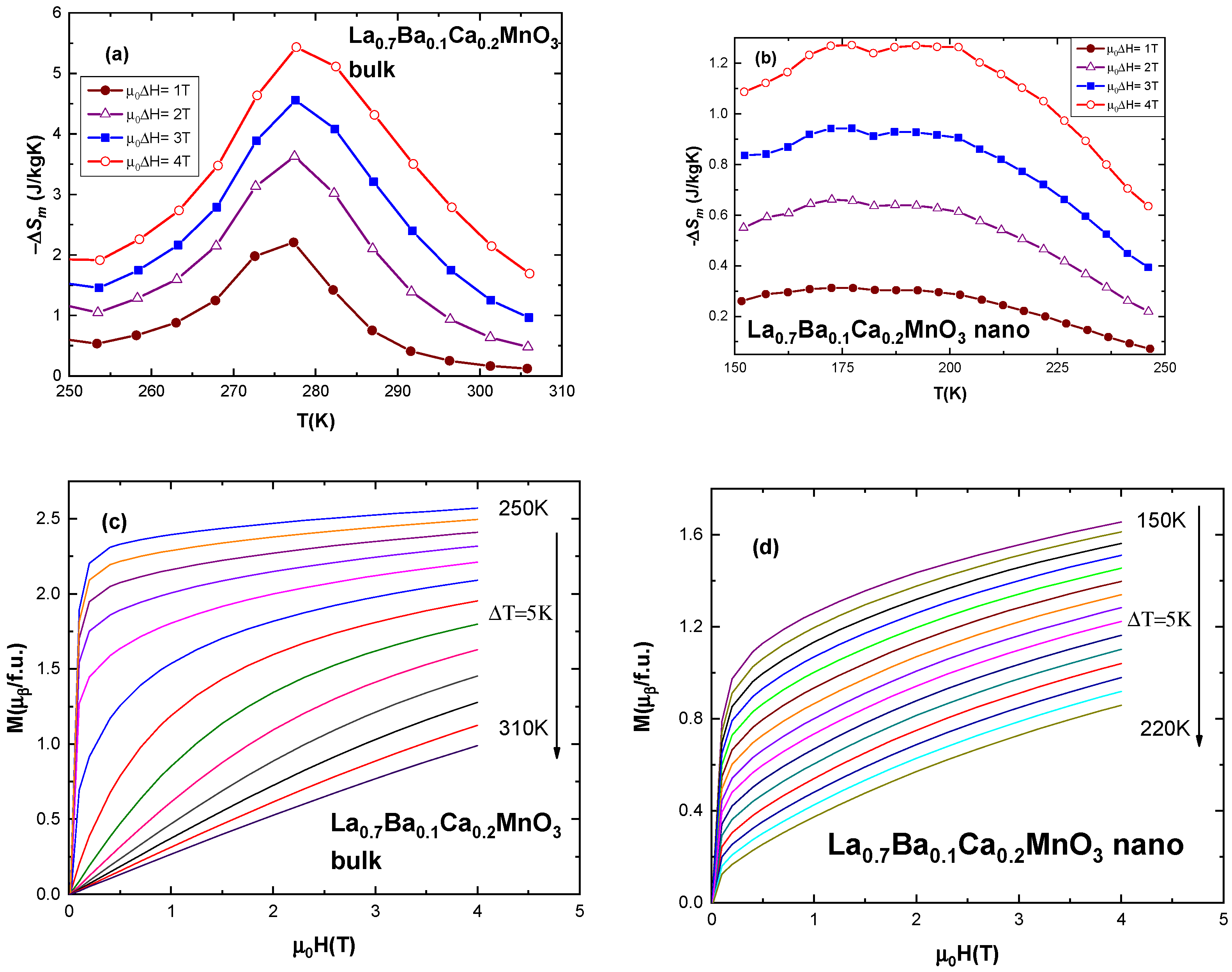
Ca Content	Bulk Samples				Fit (Χ2)	Nanocrystalline Samples				Fit (Χ2)	Space Group
	a (Å)	b (Å)	c (Å)	V (Å3)		a (Å)	b (Å)	c (Å)	V (Å3)
X = 0	5.533(2)	5.533(2)	13.519(6)	358.3(2)	1.54	5.526(3)	5.526(3)	13.48(12)	356.6(5)	1.9	R-3c
X = 0.15	5.509(4)	5.509(4)	13.42(14)	352.8(6)	1.54	5.502(9)	5.502(9)	13.44(27)	352.4(9)	1.53	R-3c
X = 0.2	5.499(5)	5.499(5)	13.41(2)	351.2(6)	1.67	5.487(8)	5.487(8)	13.41(24)	349.8(9)	1.64	R-3c
X = 0.25	5.492(4)	5.467(5)	7.727(8)	232.0(4)	1.64	5.509(3)	5.505(2)	7.802(3)	236.(157)	1.68	Pbnm

,

Table 2. Calculated tolerance factors, Mn-O lengths, Mn-O-Mn angle (°) and crystallite sizes for polycrystalline bulk samples using the Williamson–Hall and Rietveld methods, including grain diameters from optical microscopy.

Ca Content (Bulk)	t (Tolerance Factor)	Mn-O1 (Mn-O2) (Å)	Mn-O1-Mn (Mn-O2-Mn) (°)	Average Particle Diameter (μm)	Williamson–Hall Size (nm)	Average Rietveld Size (nm)	Strain
x = 0	0.926	1.9643 (4)	168.36 (3)	1.1	110.05	111.1	0.0018
x = 0.15	0.91	1.9531 (5)	169.31 (3)	1	83.15	91.18	0.0019
x = 0.2	0.905	1.9515 (5)	168.35 (6)	0.9	62.81	89.99	0.0016
x = 0.25	0.9	1.9327 (6) (1.9473 (6))	180(168.61 (6))	1.5	120.95	132.45	0.0019

and

Table 3. Calculated, Mn-O lengths, Mn-O-Mn angle (°) and crystallite sizes for nanocrystalline samples using the Williamson–Hall and Rietveld methods, including particle diameters from TEM.

Ca Content (Nano)	Mn-O1 (Mn-O2) (Å)	Mn-O1-Mn (Mn-O2-Mn) (°)	Average Particle Diameter (nm)	Williamson–Hall Size (nm)	Average Rietveld Size (nm)	Strain
x = 0	1.9616 (6)	168.35 (9)	46.6	36.23	18.14	0.0022
x = 0.15	1.9687 (4)	161.98 (5)	35.6	27.98	16.41	0.0022
x = 0.2	1.9632 (4)	161.98 (8)	45.9	32.67	17.65	0.0019
x = 0.25	1.9514 (7) (1.9577 (7))	180 (168.58 (8))	55.4	54.6	23.28	0.0024

. A figure in the Supplementary Materials illustrated a sudden change in cell parameter and cell volume for x = 0.25.

A useful parameter for understanding the stability of perovskite structures is the Goldschmidt tolerance factor. It was calculated using the following relationship [16]:

$$\mathrm{t}=\frac{{\mathrm{R}}_{\mathrm{A}}+{\mathrm{R}}_0}{\sqrt{2}\left({\mathrm{R}}_{\mathrm{B}}+{\mathrm{R}}_0\right)}$$

(1)

where R_A is the radius of the A cation, R_B is the radius of the B cation, and R₀ is the radius of the anion.

Smaller radii of Ca ions cause the tolerance factor to diminish slightly with each additional substitution, as seen in Table 2, but all samples fall within Rhombohedral/Orthorhombic limits of 0.7–0.96 [17]. Bond length plays a major role in “double exchange” [7], and as can be seen in Table 2 and Table 3, it shortens for samples in the Rhombohedral group before changing into the Orthorhombic space group.

The Williamson–Hall (W–H) method is widely used for approximating crystallite size. Compared to the Scherrer method, it accounts for strain and is thus closer to real values [18]. Table 2 includes calculated values for nanocrystalline samples using the W–H method and results from Rietveld refinement analysis compared to values of particle sizes taken from TEM. It can be observed that TEM revealed a range of particle sizes of 30–60 nm, which is closer to W–H values. Selected TEM pictures are presented in Figure 2. Previous work has shown similar results for nanocrystalline samples made via the sol–gel method [19]. Results for the bulk samples, presented in Table 3, show a different correlation. Calculated values from Rietveld analysis are closer to values procured by optical microscopy, which show average grain sizes of 0.9–1.5 μm. Examples of grain sized can be seen in Figure 3. This discrepancy between calculated and experimental values can be attributed to a single grain containing many crystallites.

3.2. Oxygen Content

The preparation method can affect the properties of the samples. In particular, it can cause accidental vacancies in the A-site occupancy and oxygen deficiency or excess. While the former is beyond the scope of this experiment and cannot be quantified, the latter is easily investigated with relatively inexpensive methods.

Iodometric titration was implemented in calculating the oxygen content of all samples. Iodometry is a comparatively accessible and reliable method of determining oxygen content in manganites [13,20]. It consists of measuring the ratio of Mn³⁺ to Mn⁴⁺ by dissolving an amount of the sample in hydrochloric acid. The resulting ratio of 1/2Cl₂ and Cl₂ is sent into another vessel to react with potassium iodine (KI), which is then titrated with sodium thiosulfate [13]. In this experiment, all bulk compounds reveal oxygen deficiency. For example, a sample with an average of 78% Mn³⁺, as is the case for bulk x = 0.15, results in an average oxygen content of O_2.96 in the range of 2.95–2.97. This can be attributed to the lack of oxygen flow during calcination. These results would affect the ratio of Mn³⁺/Mn⁴⁺, thus changing the magnetic and electrical properties of the studied compounds [20].

Interestingly, all nano-sized samples showed an excess of oxygen. For example, a sample with 67% Mn³⁺ results in O_3.02, as with x = 0.15. Such an outcome should be attributed to the high surface-to-volume ratio of the particles. We suggest that, at the surface, broken bonds of positive ions would attract an excess of oxygen atoms. These results would increase Mn⁴⁺ and lower the magnetic and electrical properties of the compounds.

Standard deviation and relative standard deviation fall within acceptable deviations from the “mean”. A maximum of 4% relative standard deviation shows reliable conclusions. All results are presented in

Table 4. Average oxygen content calculated using iodometry for bulk and nanocrystalline samples.

Ca content	Average Mn3+ Ratio	Standard Deviation	Relative Standard Deviation (%)	Average Oxygen Content
x = 0 bulk	0.7306	0.0159	2.18	O2.98±0.02
x = 0.15 bulk	0.7817	0.015	1.92	O2.96±0.01
x = 0.2 bulk	0.7616	0.0306	4.01	O2.97±0.02
x = 0.25 bulk	0.7852	0.006	0.76	O2.96±0.01
x = 0 nano	0.6813	0.0086	1.26	O3.01±0.01
x = 0.15 nano	0.6707	0.0178	2.65	O3.02±0.02
x = 0.2 nano	0.6766	0.009	1.33	O3.01±0.01
x = 0.25 nano	0.6689	0.0207	3.09	O3.02±0.02

.

3.3. Electrical Measurements

The investigation of electrical behavior was carried out by the cryogenic four-point probe method. Magnetoresistivity (MR) was calculated using the following formula [21].

MR% = [(ρ(H) − ρ(0))/ρ(0)] × 100,

(2)

where ρ(H) and ρ(0) are resistivities in an external magnetic field and in its absence, respectively.

Figure 4 shows graphs of the samples with Ca substitution, and it can be observed that all three plots exhibit a peak at T_p1 at about 226–230 K. This value, being far away from T_c for all samples, can be attributed to grain boundary conditions [21,22,23], where substitution of smaller Ca ions results in increased disorder, which tends to shift toward the boundaries in order to conserve energy [24,25]. According to previous works [26,27], the parent compound does not exhibit such a peak; hence, it can be concluded that the effect is mainly the result of substitution. Additionally, there is another peak at T_p2 for the sample with x = 0.25, most pronounced at 0 T. It becomes smaller at 1 T and almost disappears at 2 T. This peak should be attributed to the ferromagnetic–paramagnetic transition at T_c. The sample with x = 0.2 exhibits an increase in resistivity at about 280 K for 0 T, but the peak resides beyond the experiment’s temperature range.

Attention should be paid to the behavior of resistivity at low temperatures. A minimum is observed at around 30 K. The upturn in resistivity at temperatures below the minima is a consequence of both intrinsic (intragrain) effects and extrinsic grain boundary scattering/tunneling effects [25].

No new features are expected in ρ(T) curves when the measurements are conducted above 2 T. In higher applied magnetic fields, the order of the manganese spin ions increases and the magnitude of the resistivity decreases, in agreement with the double exchange theory [3,7]. In addition, the position of the peaks of the ρ(T) curves shifts to slightly higher temperatures. The behavior of the electrical resistance R (as well as magnetoresistance MR) with increasing magnetic fields, up to 7 T, is typical for polycrystalline CMR manganites [7], and some examples (for x = 0.15, 0.2 and 0.25) are displayed in Figure S3 (Supplementary Materials). At low temperatures, a sharp drop in resistance in low magnetic fields (below 50 mT) and a nearly linear dependence of R in higher magnetic fields can be seen. The maximum value of this decrease takes place at the lowest temperature (10 K), while in the case of intrinsic magnetoresistance, it was found to have a maximum close to the Curie temperature and small values at low temperatures [7]. The low-field magnetic behavior of MR in polycrystalline CMR manganites was attributed to the spin-polarized tunneling between misaligned neighboring grains [7,16]. For the high-field magnetoresistance, the alignment of spins from a disordered region close to the grain boundaries seems to be responsible [7,16].

Plots of resistance R vs. applied field H, presented in Supplementary Materials in Figure S3, reveal a smooth drop in R with the application of a magnetic field. Isothermal lines at 200 K, 250 K and 270 K, where grain boundary conditions play a leading role, show a steeper slope over higher values of the magnetic field [7].

The magnetoresistivity is negative, where the application of an external field lowers the resistance, for all the investigated samples. An interesting observation to be made is the comparison between magnetoresistivity at T_p and at 10 K, where resistivity takes an upturn. While the values of MR at T_p1 are varied, magnetoresistivity at 10 K is relatively constant and is higher than at any other temperature point. This suggests the addition of an effect of spin-polarized electron tunneling/scattering between domains [28]. This can be observed in the MR vs. T graphs available in Supplementary Materials, Figure S4. MR is highest at low temperatures for all samples and shows a dramatic jump at around T_c for x = 0.25. All of the above, including plots of R vs. H and MR vs. T, show Colossal Magnetoresistance (CMR) and its dominance by the grain boundaries. Values for T_p and MR are presented in

Table 5. Experimental values for La_0.7Ba_0.3−x Ca_xMnO₃ bulk materials: electrical properties.

Compound (Bulk)	Tc (K)	Tp1 (K)	Tp2 (K)	ρpeak (Ωcm) in 0 T	MRMax (%) (1 T) at Tp	MRMax (%) (2 T) at Tp	MRMax (%) (1 T) at 10K	MRMax (%) (2 T) at 10K
La0.7Ba0.3MnO3 [21]	340	295		0.693	5.8	12.9	27.03	32.11
La0.7Ba0.15Ca0.15MnO3	308	226		0.134	10.18	19.72	25.12	32.59
La0.7Ba0.1Ca0.2MnO3	279	226		0.073	3.42	12.99	23.78	30.97
La0.7Ba0.05Ca0.25MnO3	261	230	274	0.177	9.2 (22.04)	18.1 (31.94)	24.94	33.05

(MR for x = 0.25 at T_p2 is shown in the brackets).

3.4. Magnetic Properties

Ferromagnetic–paramagnetic transition was observed in all samples by studying their magnetization behavior in an external field of 0.05 T in the temperature range of 4–360 K. Plots of selected zero-field cooled/field cooled (ZFC–FC) data are presented in Figure 5. T_c was estimated by taking a derivative of magnetization vs. temperature, with the inflection point representing T_c, as shown in the insets of the graphs.

A lower Curie temperature caused by the introduction of Ca ions is observed for all samples. Bulk compounds with x = 0.2 and 0.15 exhibit T_c close to room temperature at 279 K and 308 K, respectively. The parent nano-sample exhibits T_c = 263 K [26]. Nanocrystalline samples reveal lower Curie temperatures relative to their bulk counterparts. This is explained by the size of the particles and defects on the surface, including broken chemical bonds. In addition, canting of spins on the surface leads to reduced magnetic moments [29,30]. The slope of plots of magnetization vs. temperature (M vs. T) for nano-sized samples is lower than those for bulk samples. This is attributed to the distribution of nano-sizes in the compounds, which will result in a distribution of Curie temperatures and consequently a broadening of the magnetic phase transition. Particles of different sizes will change phase at different temperatures. In general, bulk samples have higher magnetization values, while nano-particles have a wide range of magnetic phase transitions.

The Banerjee criterion is a convenient guide for determining the order of a phase transition. It states that the positive slope of the Arrott plot (M² vs. H/M) represents a second-order magnetic phase transition and the negative slope represents a first-order transition [31]. We constructed Arrott plots for all samples and confirmed second-order transitions for most of the samples. An interesting observation is to be made with the x = 0.25 bulk sample, which exhibits a negative slope in the initial part of the plot for temperatures above T_c (Figure 6), suggesting the existence of a first-order transition. Selected graphs are shown in Figure 6.

Landau’s mean-field theory [32] describes Gibbs free energy around a critical point and is defined as:

G (T,M) = G_O + MH + aM² + bM⁴ +…,

(3)

where a and b are coefficients that depend on temperature. Minimizing Gibbs free energy with respect to magnetization leads to an equation relating the Arrott plot axis:

H/M = 2a + 4bM²,

(4)

According to mean field theory, the isotherm lines (M² vs. H/M), if correct, are parallel and straight [33], but that is not observed in Arrott plots for our samples. The problem lies in the inexactness of the critical exponents [34]. Observation of Arrott plots for nanocrystalline samples suggests exponents close to mean field theory, while the curvature of the plots for bulk samples suggests one of the other models. An exponent β relates to spontaneous magnetization below T_c and, in mean field theory, has a value of ½, while an exponent $\gamma$ is related to the inverse of susceptibility χ above T_c and has a value of one. An additional exponent, δ = 3, relates magnetization to the external field at T_c [35].

These relations can be generalized around the critical point as follows [34]:

M_S(T) = M₀ (−ε)^β, T < T_C,

(5)

$${\chi }^{-1}\left(T\right)=\left(\frac{h_0}{M_0}\right){\epsilon }^{\gamma },T>T_C$$

(6)

M = D (μ₀H^1/δ), T = T_C,

(7)

where ε is the reduced temperature (T − T_c)/T_c and M₀, h₀/M₀, and D are critical amplitudes.

The values of the exponents describe the range of the interactions and are related to the exchange interaction J(r), spin, and system dimensionality [36,37]. According to renormalization group theory [30], J(r) = 1/r^d+σ (d—dimensionality of the system; σ—range of interaction) [36]. For σ greater than 2, the 3D Heisenberg model is valid: β = 0.355, γ = 1.366 and δ = 4.8. For σ < 3/2, the mean-field theory of long-range interaction is valid. Additionally, for the tricritical point, the critical exponents are universal: β = 0.25, γ = 1 and δ = 5 [7], and set a boundary between two different ranges of order phase transitions.

There are several different methods for calculating critical exponents. In this work, we used the modified Arrott plot (MAP) method, and to confirm the results of MAP, we applied the Kouvel–Fisher (KF) method. The construction of a modified Arrott plot is an iterative method [34]. The first stage includes the construction of an Arrott–Noakes plot (M^1/β vs. μ₀H/M^1/γ) by approaching T_c on both sides with straight lines that cross the axis at the appropriate values. The second stage requires finding intercepts of isotherms. Below T_c, on the ordinate, find values of M_s and above T_c, on the abscissa, find values of χ₀⁻¹. Finally, these are plotted against temperature and fitted for Equations (5) and (6). Iteration of these steps, until results stabilize, provides the true critical exponents. The Widom scaling relation β + γ = β δ [5] gives the value of δ. The full results of MAP are presented in

Table 6. Critical exponent values for all samples from the modified Arrott plot method.

Compound		γ	β	δ	Tc (K)
x = 0	bulk	1.065	0.288	4.69	340 [26]
x = 0.15	bulk	0.958	0.238	5.025	308
x = 0.2	bulk	0.979	0.245	4.996	279
x = 0.25	bulk	0.949	0.187	6.075	261
x = 0	nano	1.823	0.493	4.698	263 [26]
x= 0.15	nano	1.036	0.574	2.805	210
x = 0.2	nano	1.022	0.555	2.838	185
x = 0.25	nano	0.985	0.623	2.581	130
Mean-field model		1	0.5	3
3D Heisenberg model		1.366	0.355	4.8
Ising model		1.24	0.325	4.82
Tricritical mean-field model		1	0.25	5

.

Interestingly, it can be observed that bulk samples are governed by the tricritical mean-field model. The sample with x = 0.25 has exponents furthest from theoretical values at β = 0.187, γ = 0.949 and δ = 6.075. Its MAP is presented in Figure 7a. All nanocrystalline samples are represented by the mean-field model, as observation of Arrott plots had predicted. A selected MAP of a nanocrystalline sample is shown in Figure 7b.

To confirm the results obtained from the MAP method, the Kouvel–Fisher (KF) method was implemented, as it is a reliable tool for determining critical exponents [38,39,40]. KF is also an iterative method. It requires the construction of an Arrott–Noakes plot and finding the intercepts on the ordinate and abscissa. Finally, these values are used in the following equations [38,40]:

M_s {dM_s/dT}⁻¹ = (T − T_c)/β,

(8)

χ₀⁻¹ {d χ₀⁻¹/dT}⁻¹ = (T − T_c)/γ,

(9)

The plot of M_s {dM_s/dT}⁻¹ vs. T should be a straight line, and its slope is 1/β, while the intercept gives the T_c. The same logic follows the plot of χ₀⁻¹ {d χ₀⁻¹/dT}⁻¹ vs. T, which gives a slope equal to 1/γ. Figure 8 shows the comparison between MAP and KF results for two of the samples. It can be seen from Figure 8b that the results from KF are close to the results from MAP for bulk sample x = 0.25 in Figure 8a, thus confirming the tricritical field model assumption. Similarly, the KF result for nano-sized particles x = 0.15 in Figure 8d exhibits exponents that also fall within the mean-field model, as do the results from MAP for x = 0.15 in Figure 8c.

Hysteresis loops measured at 4 K show very narrow coercive fields for all samples. A compound with x = 0.15 exhibits the largest coercive field of 150 Oe in bulk. The largest nano-sized sample coercivity is 710 Oe for x = 0.25. Generally, nano-sized multi-domain particles produce a larger coercive field. If nano-particles were to be made smaller, their field would increase until single domain size. It becomes zero in the superparamagnetic state [41].

Magnetic entropy change was calculated from magnetization M(μ₀H,T) isotherm data at external fields of 1 T, 2 T, 3 T and 4 T using the following formula [42,43]:

$${\mathsf{\Delta }{S}_m(T,H_0)=S}_m(T,H_0)-S_m(T,0)=\frac{1}{\mathsf{\Delta }T}{\int }_0^{H_0}\left[M(T+\Delta T,H)-M(T,H)\right]dH$$

(10)

Furthermore, we constructed plots of −ΔS_m vs. T (temperature) in order to better estimate the magnetocaloric effect. Selected samples are shown in Figure 9.

Although relative cooling power RCP should be taken with “a pinch of salt” [44], it has been a widely used reference for the applicability of the material. It is calculated from the combination of the highest entropy change and its temperature range applicability [43,45]:

$$RCP\left(S\right)=-{\mathsf{\Delta }{S}_m}^{max}\left(T,H\right)\times \delta T_{FWHM}$$

(11)

where –ΔS_m ^max is the maximum inverse magnetic entropy change value and δT_FWHM is the full width at half maximum of the magnetic entropy curve.

It is noteworthy that both systems show broadly similar values of RCP for samples x = 0.15 and x = 0.2, whose T_c is close to room temperature. All samples show values for entropy change and RCP comparable to or higher than those reported in the literature [45,46,47]. For example, bulk x = 0.2 has an RCP of 41 J/kg at μ₀ΔH =1 T, and nano-sized particles have an RCP of 35 J/kg in the same magnetic field variation. Despite the fact that bulk samples usually exhibit a higher maximum entropy change as a result of higher magnetization and a narrower phase transition, nano-sized samples show a wide temperature range phase transition due to size distribution and separation. Bulk sample x = 0.2 has |ΔS_M| = 2.29 J/5 556 bkgK, close to T_c but $\delta T_{FWHM}$ of only 18 K at μ₀ΔH =1 T, while the same nano-sized sample has a temperature range of approximately 100 K and lower |ΔS_m|. This can be observed in Figure 9.

In recent events, RCP has been discredited as a reliable figure of merit. RCP overestimates the quality of the materials with a very large δT_FWHM and a small entropy change [44,48]. Materials with the same cooling power can behave differently [48,49] when tested in real magnetic refrigerators. Active magnetic regenerative refrigeration (AMRR) systems point to heat capacity, heat conductivity and resistivity, and chemical and mechanical stability as important properties in addition to high magnetic entropy change. A wide temperature range is neglected as a worthwhile figure, and even books on magnetocaloric effects omit $\delta T_{FWHM}$ completely [50]. For this purpose, temperature-averaged entropy change (TEC) is seen by many as a more suitable figure-of-merit [51,52]:

$$TEC=\frac{1}{\mathsf{\Delta }{T}_{H-C}}\max \left\{{\int }_{T_{mid-\frac{\mathsf{\Delta }{T}_{H-C}}{2}}}^{T_{mid+\frac{\mathsf{\Delta }{T}_{H-C}}{2}}}\left|∆S\right|dT\right\}$$

(12)

Here, ΔT_H-C is the temperature span that would be used in a device. We have investigated a temperature range of 5 K to 50 K with a step of 5 K. T_mid is the temperature that maximizes the integration, or, in other words, TEC, for a given temperature span T_H-C. Figure 10 presents the values of TEC for polycrystalline and nanocrystalline samples.

Initial observation reveals that the value for TEC for all polycrystalline samples diminishes with increasing temperature span ΔT_H-C. This tendency has been reported in previous research [51,52,53] and is expected, as the graphs for magnetic entropy change in bulk have a smooth Gaussian-like distribution. Nano-sized samples, on the other hand, show a small fluctuation around a mean value, which also diminishes, but very slowly, with increasing ΔT_H-C. This is explained by the existence of local minima and maxima on the magnetic entropy change graphs. At a second glance, another observation can be made: increasing levels of substitution by Ca ions cause an increase in TEC in polycrystalline samples. The opposite effect is seen for nanocrystalline samples, as seen in Figure 10b; they tend to decrease their value of TEC with increasing substitution due to lowering values of entropy change.

The values of maximum entropy change (ΔS_M), RCP, M_s and H_ci for both polycrystalline and nanocrystalline samples are presented in

Table 7. Experimental values for La_0.7Ba_0.3−xCa_xMnO₃ bulk materials: magnetic measurements.

Compound (Bulk)	TC (K)	Ms (μB/f.u.)	Hci (Oe)	|ΔSM| (J/kgK) μ0ΔH = 1 T	|ΔSM| (J/kgK) μ0ΔH = 4 T	RCP (S) (J/kg) μ0ΔH = 1 T	RCP (S) (J/kg) μ0ΔH = 4 T	Refs
La0.7Ba0.3MnO3	340	4.04	200	1.33	3.5	53.7	158.4	[26,27]
La0.7Ba0.15Ca0.15MnO3	308	3.612	150	2.04	4.37	38.74	140.43	This work
La0.7Ba0.1Ca0.2MnO3	279	3.676	110	2.29	5.43	41.26	184.69	This work
La0.7Ba0.05Ca0.25MnO3	261	3.758	100	3.66	7.01	40.24	182.37	This work
La0.7Ca0.3MnO3	256			1.38		41		[5]
La0.7Sr0.3MnO3	365			-	4.44 (5 T)		128 (5 T)	[5]
La0.6Nd0.1Ca0.3MnO3	233			1.95		37		[5]
Gd5Si2Ge2	276			-	18 (5 T)	-	535 (5 T)	[5]
Gd	293			2.8		35		[5]

and

Table 8. Experimental values for La_0.7Ba_0.3−xCa_xMnO₃ nano-sized materials.

Compound (Nano)	Tc (K)	Ms (μB/f.u.)	Hci (Oe)	|ΔSM| (J/kgK) μ0ΔH = 1 T	|ΔSM| (J/kgK) μ0ΔH = 4 T	RCP(S) (J/kg) μ0ΔH = 1 T	RCP(S) (J/kg) μ0ΔH = 4 T	Refs
La0.7Ba0.3MnO3	263	2.79	4800	1.04	1.37	105.4	130.1	[26,27]
La0.7Ba0.15Ca0.15MnO3	210	2.547	370	0.33	1.31	33.7	144.1	This work
La0.7Ba0.1Ca0.2MnO3	185	2.389	440	0.32	1.28	35.2	153.6	This work
La0.7Ba0.05Ca0.25MnO3	130		710	0.05	0.27	6.1	40.5	This work
La0.67Ca0.33MnO3	260			-	0.97 (5 T)		27 (5 T)	[54]
Pr0.65(Ca0.6Sr0.4)0.35MnO3	220			0.75		21.8		[55]
La0.6Sr0.4MnO3	365			1.5		66		[14]

, respectively. It can be seen that the nanocrystalline sample x = 0.25 does not reach magnetic saturation at 4 T.

4. Conclusions

Bulk compounds of La_0.7Ba_0.3−xCa_xMnO₃ (0, 0.15, 0.2, 0.25) were prepared by solid-state reactions. Morphology was investigated by optical microscopy and revealed an average grain size of approximately 1000 nm. Nano-sized compounds were synthesized via the sucrose-based sol–gel method. TEM was implemented for size determination, showing an average size of 30–70 nm. Both systems were investigated by Rietveld refinement analysis on XRD patterns. All samples are single-phase. Compounds with a substitution level of x ≤ 0.2 for both systems retain Rhombohedral (R-3c) lattice symmetry. Samples with x = 0.25 change symmetry to Orthorhombic (Pbnm) for bulk and nanocrystalline samples. The bond length of Mn-O progressively diminishes with the addition of Ca. Iodometric analysis showed an oxygen deficiency in bulk material and a slight oxygen excess in nano-sized particles, attributed to surface properties. Derivatives of M(T) curves reveal T_c values: bulk x = 0.15 and x = 0.2 experience close to room temperature ferromagnetic–paramagnetic transitions at 308 K and 279 K, respectively. Nano-sized sample with x = 0.15 drops the T_c of the parent sample (263 K) to 210 K. Very low coercivity was observed in hysteresis loops for all samples. All bulk samples exhibit a metallic–insulator transition at T_p1, attributed to grain size and boundary conditions at around the same temperature (226 K–230 K). A second transition peak at T_p2 was observed for the x = 0.25 sample in μ₀H = 0 T. This peak is associated with the magnetic phase transition T_c. Negative magnetoresistance (MR) is observed for all bulk samples. Arrott plots confirm a second-order magnetic phase transition for all samples. Modified Arrott plot MAP analysis revealed the critical exponents for bulk samples to be in the tricritical mean-field model range and in the mean-field model range for nanocrystalline samples. The Kouvel–Fisher method confirmed the results obtained from MAP. Bulk compounds show a sharp magnetic entropy change |ΔS_m(μ₀H,T)| with high values. Nanocrystalline samples exhibit a lower peak entropy change but a very wide effective temperature range δT_fwhm. Relative cooling power (RCP) is comparable for bulk and nano-sized samples with the same substitution level. Temperature-averaged entropy change (TEC) increases with increasing substitution by Ca ions for the bulk samples, with high values of 5 J/kgK (4 T) and 2 J/kgK (1 T) at ΔT_H-C = 10 K for the x = 0.15 sample, while they are much lower for the nano-sized samples. TEC values decrease with substitution by Ca ions in nanocrystalline samples but possess respectable values of 1.3 J/KgK (4T) at ΔT_H-C = 10 K for the x = 0.15 sample. Further substitution lowers the TEC drastically for nano-sized compounds. Both bulk and nanocrystalline compounds exhibit properties useful for magnetic refrigeration. Polycrystalline bulk samples exhibit higher peak entropy changes and show the room temperature T_c in addition to their CMR. While nanocrystalline samples possess lower values of T_c and lower values of entropy change, they are flexible in application and temperature range. They can be used separately or, possibly, together in multistep refrigeration processes. The flexibility of the use of nanocrystalline samples could be combined with the high entropy change of bulk samples.