1. Introduction
Accounting for solvation may be of crucial importance in the calculation of molecular properties. Solvation effects caused by, e.g., hydrogen-bonding or polarity effects may lead to geometrical changes in the molecule (e.g., changes in bond lengths, bond angles, etc.) but may also directly perturb the electronic distribution in the molecule, leading to a change in molecular properties. The former of these two contributions to the effect of solvation is generally referred to as an indirect effect, while the latter is referred to as a direct solvent effect. Two examples of molecular properties that may be significantly affected by a solvent are the chemical shift and spin–spin coupling constant (SSCC) [
1,
2,
3,
4,
5]. These molecular properties represent NMR parameters that, together, provide important structural information. For example, the SSCC describes how a nucleus experiences a change in the magnetic field due to other nuclei with spin. In an NMR spectrum, they are the distance between the peaks in a multiplet, which is a signal containing more than one peak [
1]. Solvent effects have been a topic of much discussion, both experimentally and computationally, for a long time. Experimentally, the effects of different solvents on chemical properties have frequently been investigated. Multiple articles have reported NMR spin–spin coupling constant for a given molecule in more than one solvent. Some of these [
6,
7,
8,
9,
10,
11,
12,
13,
14,
15,
16,
17,
18,
19] will be used as experimental reference values in this work.
Computationally, different solvent models have been formulated to improve calculations. In broad terms, solvent models may be classified as continuum solvent models or models with explicit solvent molecules treated at a lower level of theory, e.g., via a QM/MM approach. Several authors have previously combined such solvent models with the second-order polarization propagator approximation (SOPPA) for the calculation of excitation energies and linear response properties [
20,
21]. Most recently, Eriksen et al. [
22,
23] presented the PCM-SOPPA/RPA solvent model (described in
Section 2.2) and applied it to the calculation of excitation energies in a water environment. The results showed that the PCM-SOPPA/RPA calculations had a tendency to underestimate the excitation energies compared with experimental values, as is also observed in gas phase SOPPA calculations [
24,
25]. Different solvent models have also been combined with density functional theory (DFT) for calculations of spin–spin coupling constants. An extension of a polarizable continuum model (PCM) was presented by Ruud et al. [
26], and a QM/MM implementation was presented by Møgelhøj et al. [
27]. Both models gave values of SSCCs that are in good agreement with experimental observations.
For the present study, the polarizable continuum model (PCM) has now also been interfaced with the atomic orbital SOPPA module in the Dalton program. With this new implementation, it is now possible to carry out not only SOPPA but also HRPA(D) calculations of different response properties in a solvent environment. In this work, the solvent implementation has been used to calculate the NMR spin–spin coupling constants for 20 small organic molecules (
Figure 1) in order to investigate whether the solvent calculations are more in agreement with experimental values than a typical vacuum calculation. Our benchmark set was chosen based on the availability of good experimental data and keeping the size of the selected molecules small to reduce the computational effort. For the SSCC calculations, five different methods were used: SOPPA [
28,
29,
30,
31], HRPA(D) [
32] and DFT with the functionals B3LYP [
33,
34], BHandH [
34,
35], and PBE0 [
36,
37].
3. Computational Details
For all 20 molecules, initial coordinates were generated with ChemDraw3D. Subsequently, geometry optimizations were carried out using the Gaussian16 [
44] program. Geometry optimizations were carried out both with a continuum solvation model and in a vacuum. To save time, an initial second-order Møller–Plesset (MP2) [
45,
46] geometry optimization with the basis set pcseg-1 [
47] was performed first.
This geometry was then used as a starting point for a second geometry optimization with the larger basis set pcseg-2 [
47]. The methods used in the second geometry optimization depend on the methods used in the SSCC calculations. The MP2 method was used for the second geometry optimization for the SOPPA [
28,
29,
30] and HRPA(D) [
32] SSCC calculations. Because it is possible for the DFT calculations to use the same functional in both the second geometry optimization and the SSCC, this was implemented to minimize errors due to using different functionals, as illustrated by Giovanetti et al. [
48].
For the SSCC calculations, the publicly available 2022 development version of the Dalton [
49,
50] program was used, in which the new PCM-HRPA(D)/RPA model has been implemented. All the SSCC calculations were carried out with the same basis set, pcJ-2 [
51], which was made specifically to reduce basis set errors for the calculation of NMR spin–spin coupling constants. For each of the molecules (and solvents), we considered the inclusion of solvation correction (or not) both in the geometry and the final SSCC calculation. This leads to four combinations, denoted vacuum–vacuum, solvent–solvent, vacuum–solvent, and solvent–vacuum, where the first term refers to the geometry treatment and the second term refers to the SSCC calculation treatment. These four combinations make it possible to see where the solvent effect is greatest; in the solvent geometry optimization or the solvent SSCC calculation.
The solvent model used for the SOPPA and HRPA(D) SSCC calculations is the PCM-SOPPA/RPA(as described in
Section 2.2). For the DFT calculations, the solvent models are the PCM-B3LYP, PCM-BHandH, and PCM-PBE0.
Vibrational corrections [
52] are not included in the present study, as we want to be able to see the pure effect of the solvent corrections. However, this implies that temperature effects are also not included, and that perfect agreement with experimental values should not be expected.
4. Results
For the 20 molecules, 242 different spin–spin coupling constants were calculated with these five methods and four solvent treatment options. All of the SSCCs, including the experimental values, can be found in the table in the Supplementary Material. Some SSCCs in the table are reported as a mean value between two or three SSCCs, because they are indistinguishable in the experimental NMR spectrum. This can happen, e.g., for a methyl group, where the rotation of the hydrogens is too fast for an NMR instrument to resolve. Different types of spin–spin coupling constants were calculated depending on which experimental data were available. Of the 242 calculated SSCCs, 89 are SSCCs (from 10 molecules), 29 are SSCCs (from four molecules), and 51 are SSCCs (from nine molecules). Furthermore, there are 2 , 2 , 1 , 3 , 12 , 7 , 10 , 7 , 1 , 6 , 15 and 7 SSCCs.
Examining
Table S1 in the supplementary material, two trends stand out: (i) the indirect solvent effects via the geometry optimization and direct solvent effects in the SSCC calculations are approximately additive, i.e.,
; and (ii) the solvent effects for adding solvation are greater in the SSCC calculations than in the geometry optimization, where they are found to be over twice as large (with few exceptions) and sometimes over ten times greater. In
Table 1, the averaged magnitude of the solvent effects on the calculated SSCCs is reported, where we define the solvent effect as the difference
with
corresponding to a solvent–vacuum, vacuum–solvent, or solvent–solvent type of calculation. The magnitude of the solvent effect on the SSCCs is only weakly dependent on the choice of computational method, as is reflected in the very similar mean- and mean-absolute deviations obtained with both the wave function and DFT methods. It is also apparent that the impact of the direct solvent effect is typically more substantial than the indirect (geometry) effect. For example, the mean absolute deviation for SOPPA is 0.20 Hz for the indirect effect alone, compared with 1.07 Hz for the direct effect alone. The total solvent effects are also highly correlated between methods. For instance, comparing SOPPA and PBE0, we find a coefficient of determination
. This suggests that, to estimate SSCCs that include solvation effects, one could employ composite methods which compute the effects of solvation with an affordable method, while the main SSCC is calculated using a more accurate and expensive wave function method in a vacuum.
In the following sections, a statistical analysis of the deviation from the experimental values is presented, including the mean absolute deviation (Mean Abs Dev), the mean signed deviation (Mean Dev), the standard deviation (Std Dev), and the maximum absolute deviation (Max Abs Dev).
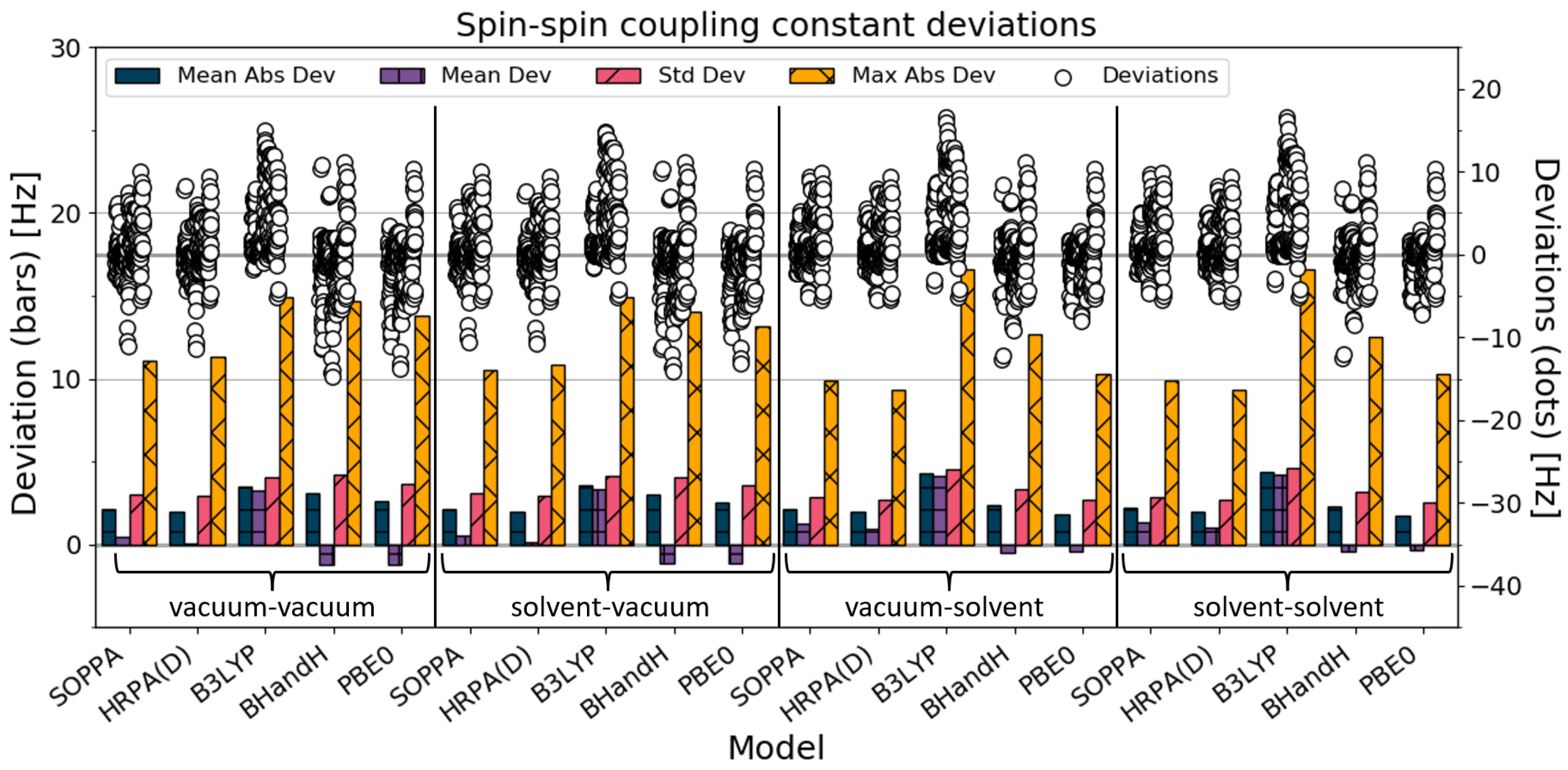
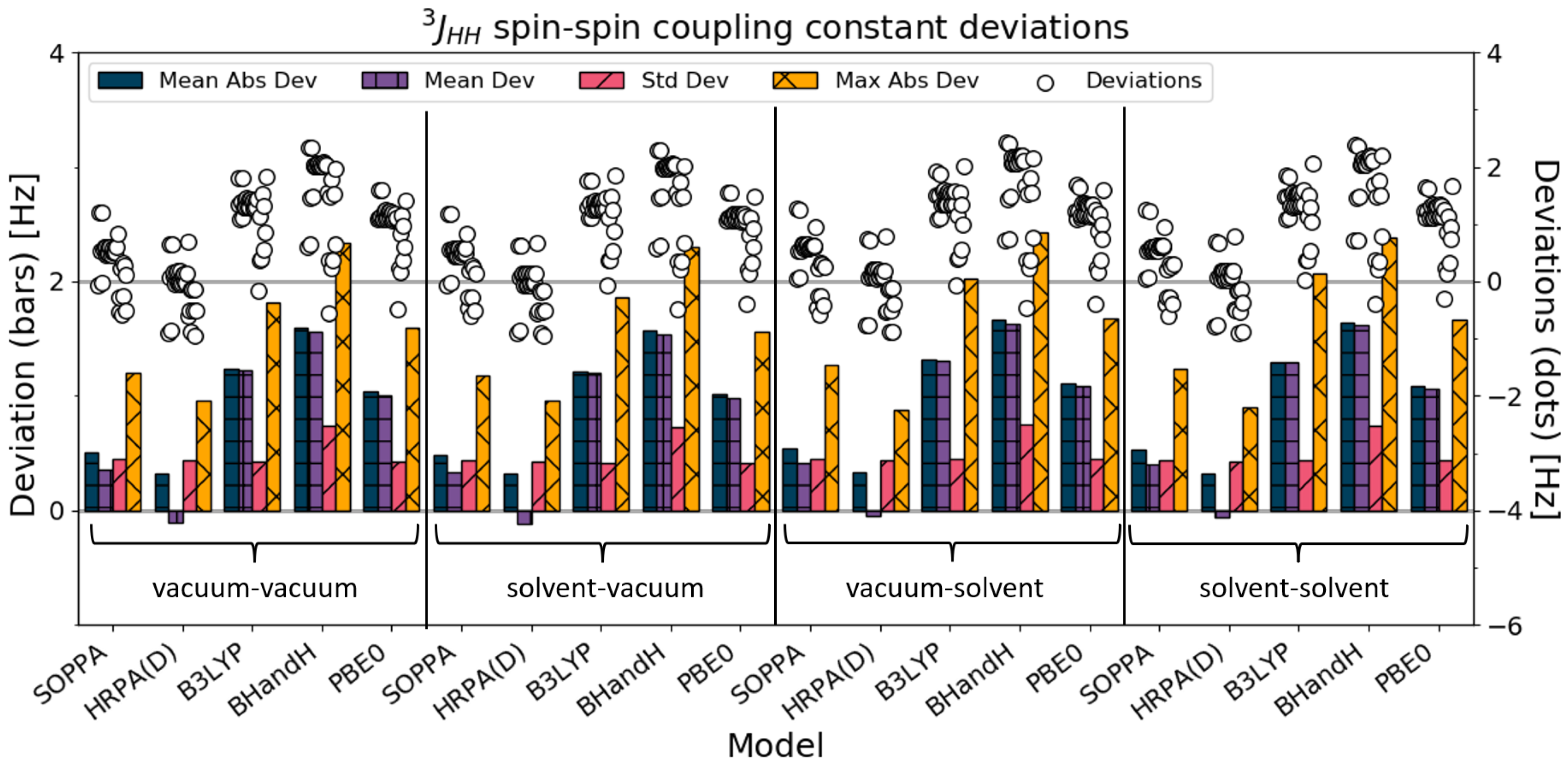
4.1. All SSCC
Statistical descriptors of deviations from the experimental data for the 242 calculated spin–spin coupling constants are presented in
Figure 2 and
Table 2. In terms of the mean absolute deviations, it is clear that the PBE0 solvent-solvent SSCC calculations perform best. Considering the vacuum–vacuum calculations, the HRPA(D) SSCC calculations perform better than the DFT calculations. The BHandH and PBE0 SSCC calculations are the only ones for which the SSCCs improve by going from vacuum–vacuum to solvent–solvent, whereas the B3LYP SSCC calculations worsen. The SSCC calculations for SOPPA and HRPA(D) are changed negligibly by the addition of solvation, with only a slightly worsened quality of the solvent–solvent result compared with the vacuum–vacuum results.
The standard deviation describes the consistency of each set of calculations, meaning the precision. For all the methods except B3LYP, consistency decreased when solvation was added in the SSCC calculations (i.e., the standard deviation increased).
The maximum absolute deviation decreases when solvation is added for all the methods except B3LYP, for which it increases. The calculated SSCCs farthest from the experimental values are the coupling constants of imidazole (in chloroform) with the B3LYP solvent–solvent/vacuum–solvent combination.
In general, the SSCCs for PBE0 and BHandH improve by going from the vacuum–vacuum to the solvent–solvent combination, whereas the results of B3LYP worsened.
For SOPPA and HRPA(D), the accuracy (Mean Abs Dev) hardly changed when solvation was added to the calculations. Meanwhile, the consistency improved, which is reflected in a lower standard deviation and maximum deviation. When comparing SOPPA and HRPA(D) SSCCs, it is noticeable that HRPA(D) performs slightly better for each combination.
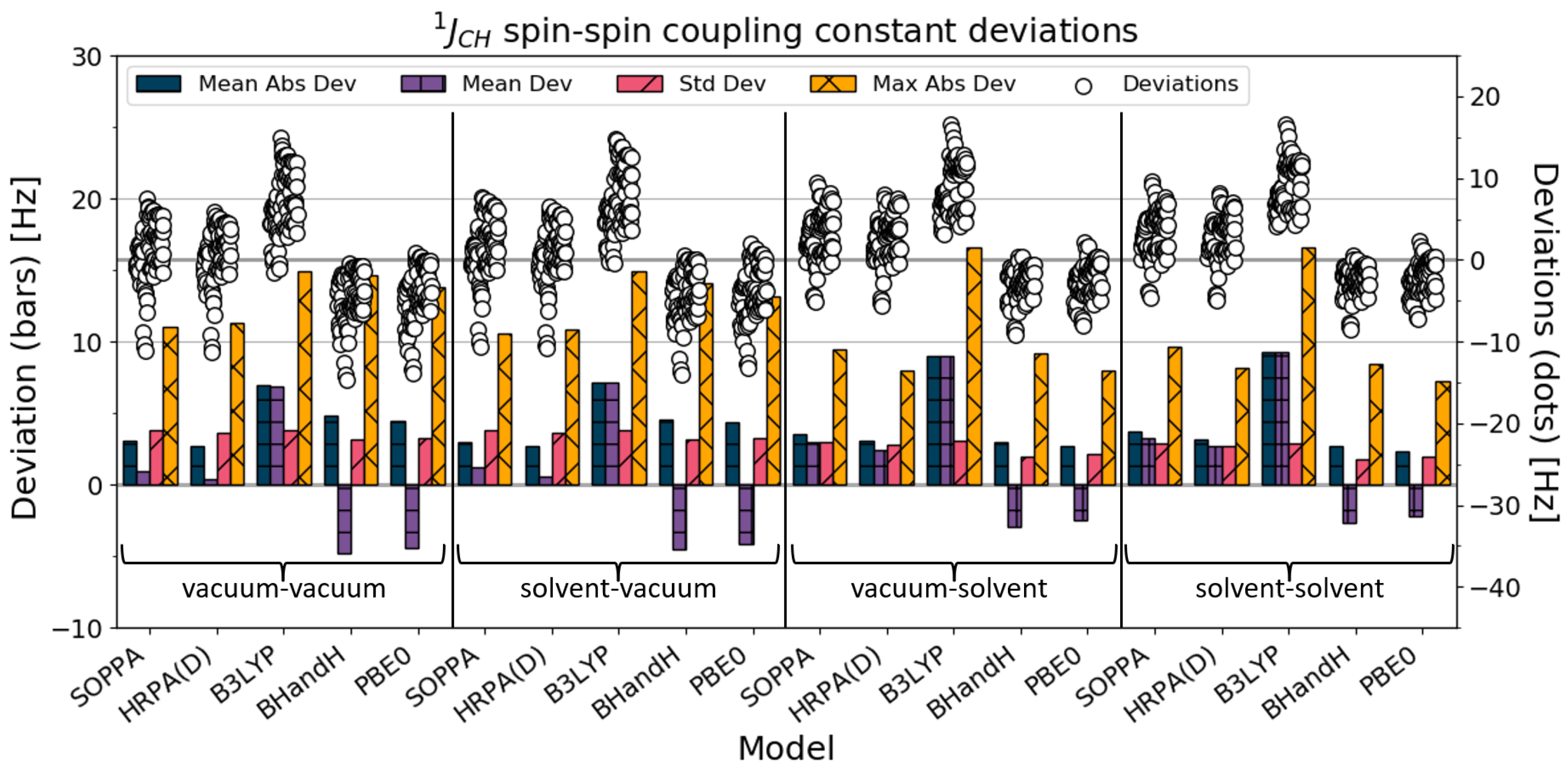
4.2. J
For all of the 89 one-bond spin–spin coupling constants between a carbon atom and a hydrogen atom, the results of the statistical analysis are illustrated in
Figure 3 and
Table 3.
For PBE0 and BHandH, the calculated coupling constants improved with the solvent–SSCC calculations. The solvent–solvent SSCC calculations for PBE0 were closest to the experimental values. The B3LYP SSCCs differ most from the experimental values, and the coupling constants significantly worsen by going from a vacuum–vacuum to solvent–solvent calculation. The accuracy of the SSCC calculations for SOPPA and HRPA(D) were increased slightly when solvation was added in the calculations. The HRPA(D) method (followed by SOPPA) performs better in vacuum–vacuum and solvent–vacuum calculations than the other methods.
The mean absolute deviation for the SSCCs is larger than for all the other SSCCs. This is not surprising because the magnitude of a coupling constant is rather large (above 100 Hz), and therefore the mean absolute deviation is larger, even if the percentage error is small. The standard deviation improves with adding solvation in the calculations for all the methods (including B3LYP). The method that has the best consistency in the calculated SSCCs is BHandH with the solvent–solvent combination.
For PBE0 and BHandH, all SSCC calculations improve when solvation is added to the calculations, as was the case for all the SSCCs (
Section 4.1). The B3LYP SSCC calculations with solvation deviate further from the experimental values, but their consistency improved. For SOPPA and HRPA(D), the mean absolute deviation increased slightly with solvation, while the standard deviation and the maximum absolute deviation improved. As for all the SSCCs, the HRPA(D) SSCC calculations were slightly better than the SOPPA calculations for the
SSCCs.
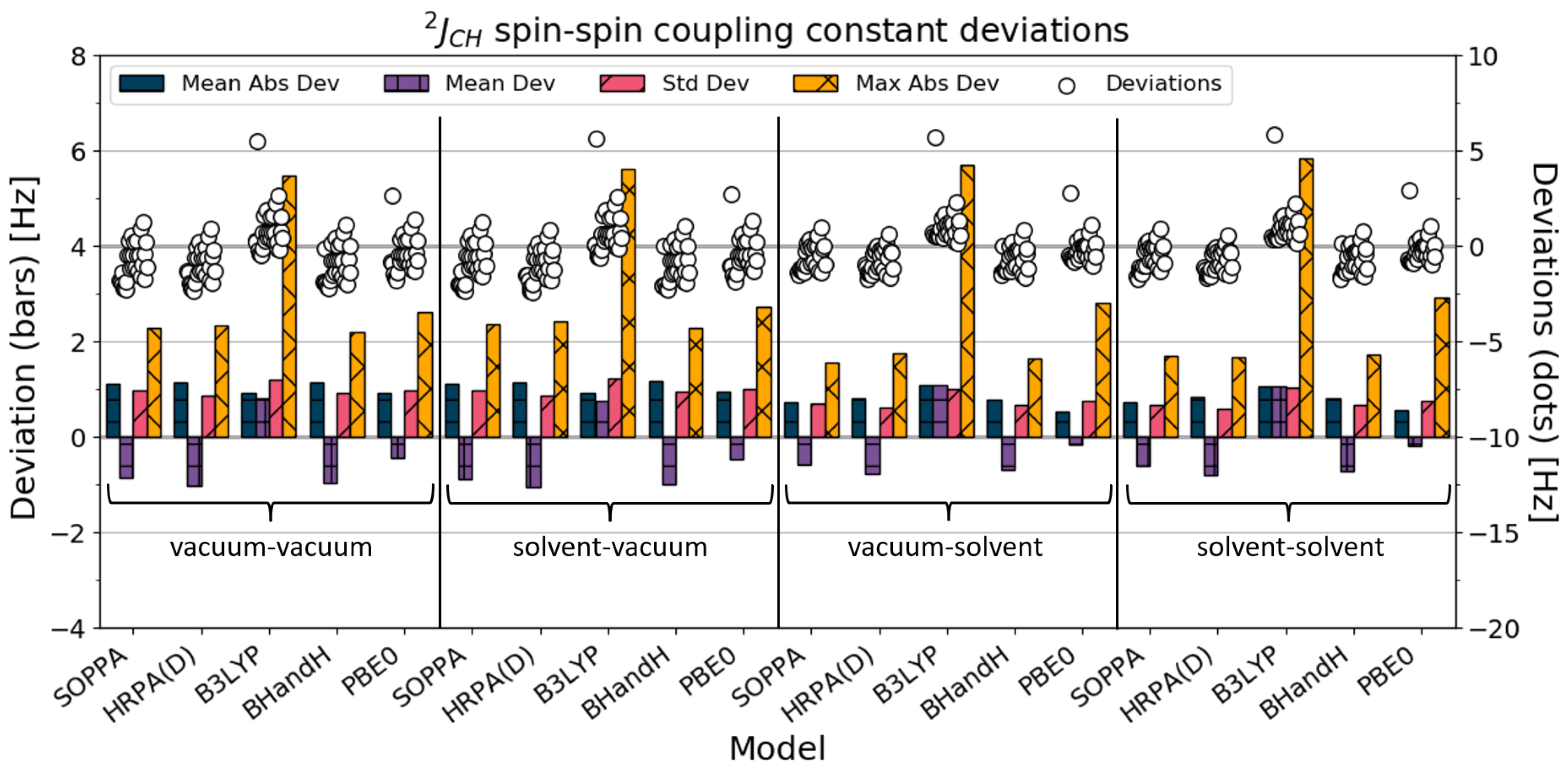
4.3. J
is the two-bond coupling constant between a carbon atom and a hydrogen atom. The statistical analysis of the 29
SSCCs is illustrated in
Figure 4. The numerical values can be found in
Table 4.
The mean absolute deviation improved for all the methods, except B3LYP, when solvation was added in the SSCC calculation, which means that the calculated SSCCs were closer to experimental values. The vacuum–solvent combinations give the best results for these four methods, followed closely by the solvent–solvent calculation. PBE0 gives the best results for both the vacuum–vacuum and the vacuum–solvent SSCCs. The B3LYP SSCCs calculations worsen slightly with solvation, but in comparison to all the SSCCs (
Section 4.1), the magnitude of this change is smaller.
The consistency of the SSCC calculations is quite good for all the methods, especially when compared with all SSCCs (
Section 4.1). The standard deviations were all improved with solvation, and the HRPA(D) solvent-solvent calculations showed the best value. The maximum absolute deviation improved for SOPPA, HRPA(D), and BHandH with solvation, whereas it slightly worsened for B3LYP and PBE0. The maximum absolute deviations for the B3LYP calculations are more than twice as large as those of all the other methods, independent of the solvent model. The reason for this is a large degree of error occurring in the calculations on 1,2-dichloroethene.
The agreement with experimental results improved with the addition of solvation in the SSCC calculation for all the methods with the exception of B3LYP, and the consistency of the results also improved. For B3LYP, the mean absolute deviation worsened slightly with solvation, while the standard deviation improved. For this type of coupling constant, the SOPPA calculations are slightly better than the HRPA(D) SSCC calculations.
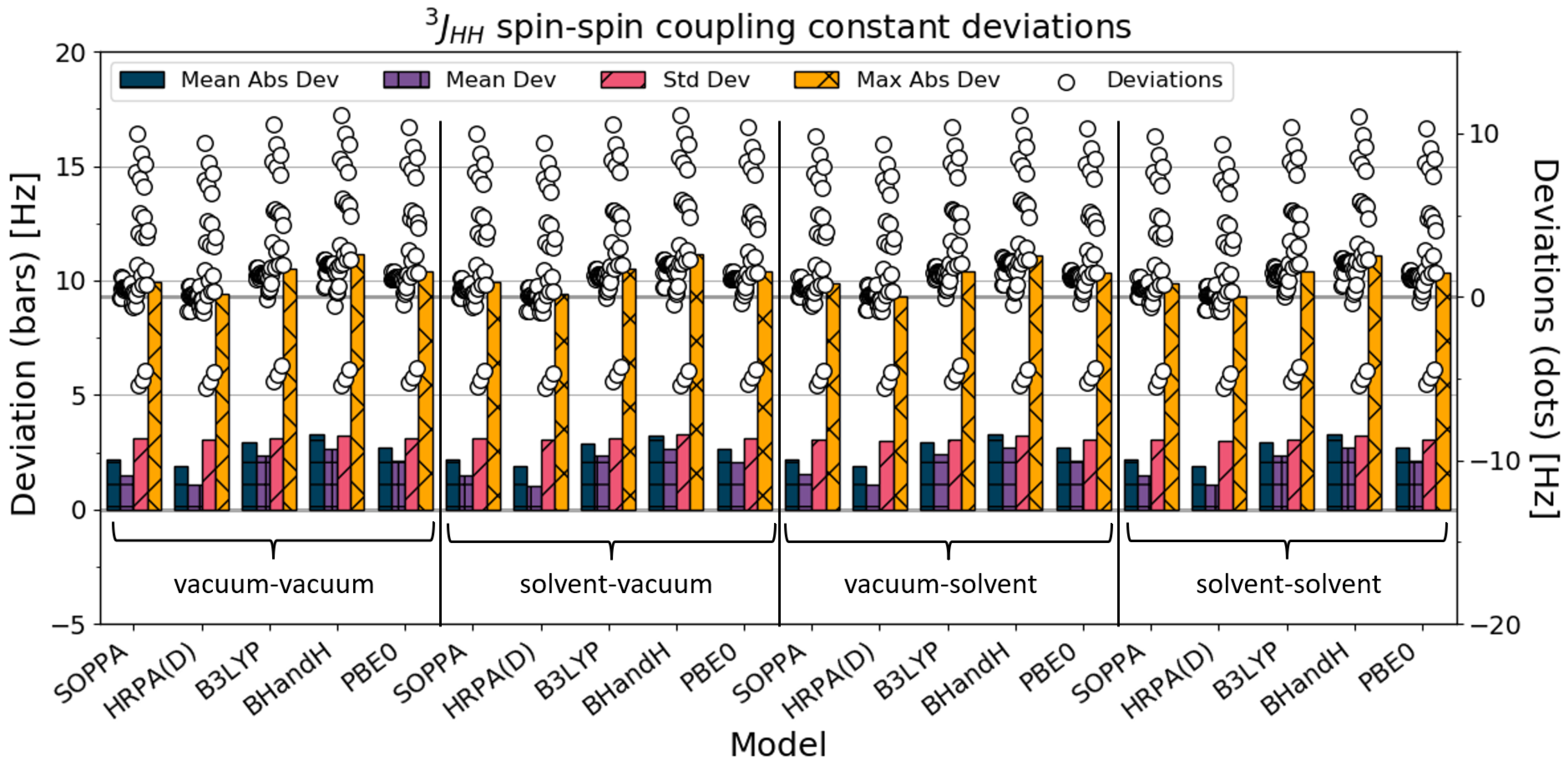
4.4. J
The
SSCC is the vicinal coupling between two hydrogens. A statistical analysis was made for all 51
; this can be found in
Figure 5 and
Table 5. The spin–spin coupling constants barely changed when solvation was added to the calculations, and the HRPA(D) results are found to be closest to the experimental values.
Figure 5 shows that some of the calculated SSCCs deviate considerably from the experimental values (both by underestimating and overestimating). This deviation follows the same trend through every method and combination. We considered in more detail which molecule(s) these coupling constants belonged to, and found that the outliers are all from the vicinal transcoupling of the molecule trans-1,2-dichlorocyclopentane. To get a better impression of how the methods work for all the other couplings, a statistical analysis that excludes the SSCCs for trans-1,2-dichlorocyclopentane is therefore presented in
Figure 6 and
Table 6.
The results barely changed from the vacuum–vacuum calculations to the solvent–solvent calculations. The SSCCs slightly worsen with solvation, except for HRPA(D), where there is no difference relating to the inclusion of solvation. For this type of spin–spin coupling constant, the HRPA(D) calculations are closest to the experimental values, followed closely by the SOPPA calculations. The BHandH SSCC results are farthest from the experimental values.
In general, the calculations of the
SSCCs are relatively close to the experimental values. The standard deviations are similar for all the methods except for BHandH, with a small decline with the inclusion of solvation. BHandH has a standard deviation of around twice the magnitude of the other methods. In general, the standard deviations are rather small for the
SSCCs compared with all the SSCCs (
Section 4.1).
5. Discussion
We expected the SOPPA or HRPA(D) calculations to perform better relative to experimental values than the DFT calculations, but the PBE0 SSCCs were overall closest to the experimental values with the solvent–solvent and vacuum–solvent combinations, except for the coupling constants where HRPA(D) performed best. For the vacuum–vacuum (and solvent–vacuum) calculations, SOPPA and HRPA(D) generally performed better than DFT, except for where PBE0 performed best, closely followed by B3LYP.
One potential explanation for why DFT performs better may be the basis set selection: pcseg-2 and pcJ-2 are optimized for good performance with DFT methods, and this improved performance might not be transferable to wave function methods. Another reason may be found in the solvent model. The solvent environment is modeled with a continuum model, and more advanced (atomistic) solvent models could improve the quality of the results. Additionally, in the SOPPA and HRPA(D) calculations, the dynamic response solvent coupling is evaluated only at the RPA level. Therefore, the SOPPA and HRPA(D) results might improve if the solvent model is changed to a full PCM-SOPPA treatment, where both the static and dynamic response effects are evaluated at a correlated level. A third reason could be that neither temperature nor vibrational corrections [
52] were included in this study, while the experimental spectra are typically recorded at ambient temperatures. However, previous calculations of the temperature dependence of NMR spin–spin coupling constants showed that, for simple systems such as CH
, SiH
and H
O [
53,
54,
55], the temperature dependence due to vibrational corrections is less than 1 Hz. This might of course be different if the temperature dependence is due to a change in an conformational equilibrium.
6. Conclusions
This work aimed to investigate the effect of adding solvation in the calculation of SSCCs, both indirectly in the geometry optimization part and directly in the SSCC part of such calculations. We found that the direct and indirect solvent effects are additive for all the methods studied here, and that the direct solvent effect for the SSCC calculations is greater than the indirect effect via geometry optimization. Furthermore, the averaged solvent effects are almost independent of the employed computational method, i.e., DFT or wave function methods. For the individual couplings, the indirect solvent effects via the DFT or MP2 geometry optimization are very similar, while the direct solvent effect can vary substantially depending on the method.
In comparison with the experimental values, we find that PBE0 gives the best results for most SSCC types, except for the SSCCs, where HRPA(D) performs best. The PBE0 and BHandH calculations improve when solvation is added to the spin–spin coupling constant, meaning that the vacuum–solvent and solvent–solvent combinations perform best for these methods. Adding solvation, on average, barely improved the agreement with experimental values for the SOPPA and HRPA(D) calculations. For the B3LYP calculations, the SSCCs were mostly further away from the experimental values when solvation was added to the calculation, which means that the vacuum–vacuum and solvent–vacuum combinations performed best for this method.