Correlation between Slow Magnetic Relaxations and Molecular Structures of Dy(III) Complexes with N5O4 Nona-Coordination
Abstract
1. Introduction
2. Results
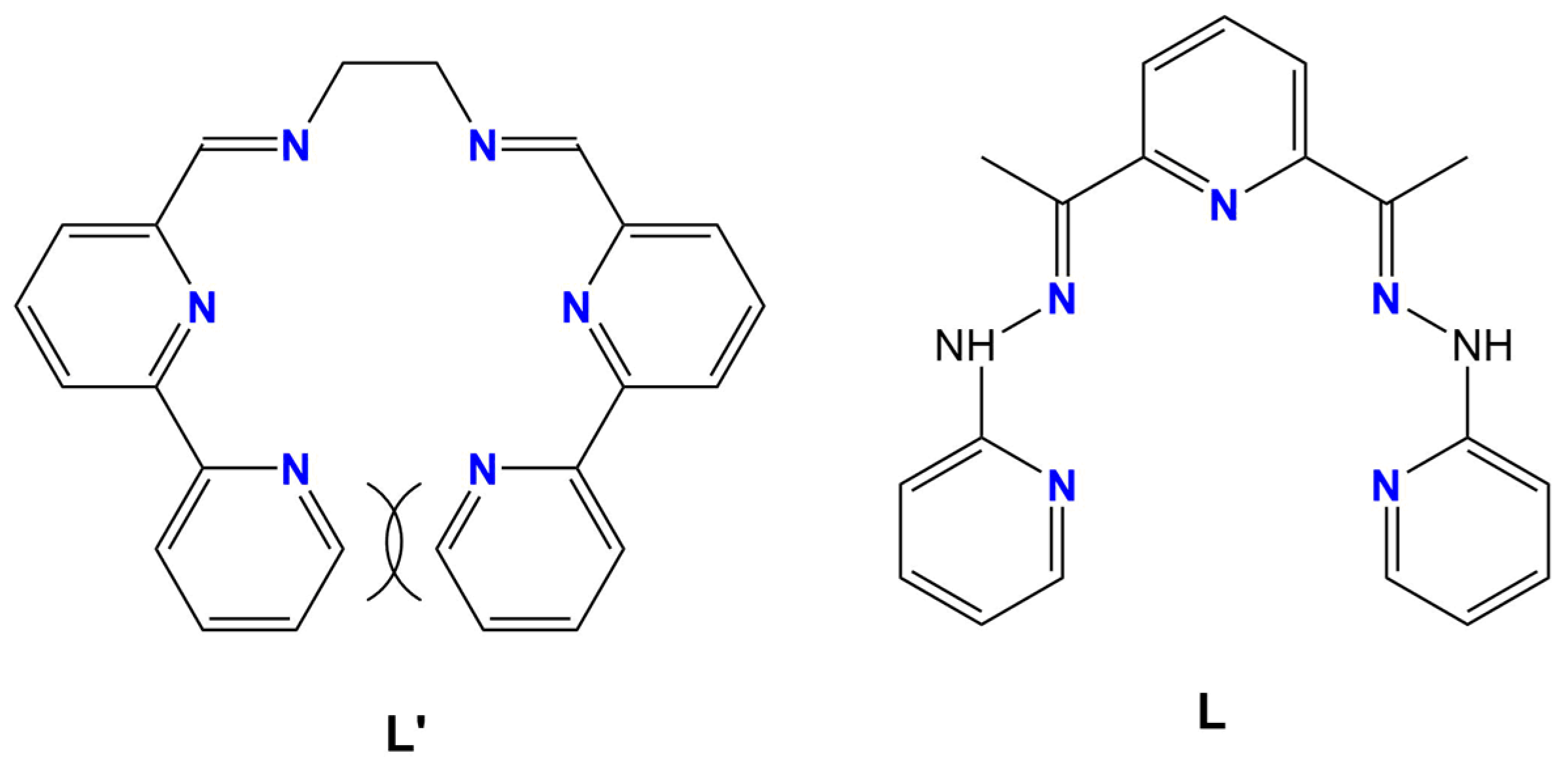
2.1. Synthesis and Characterization
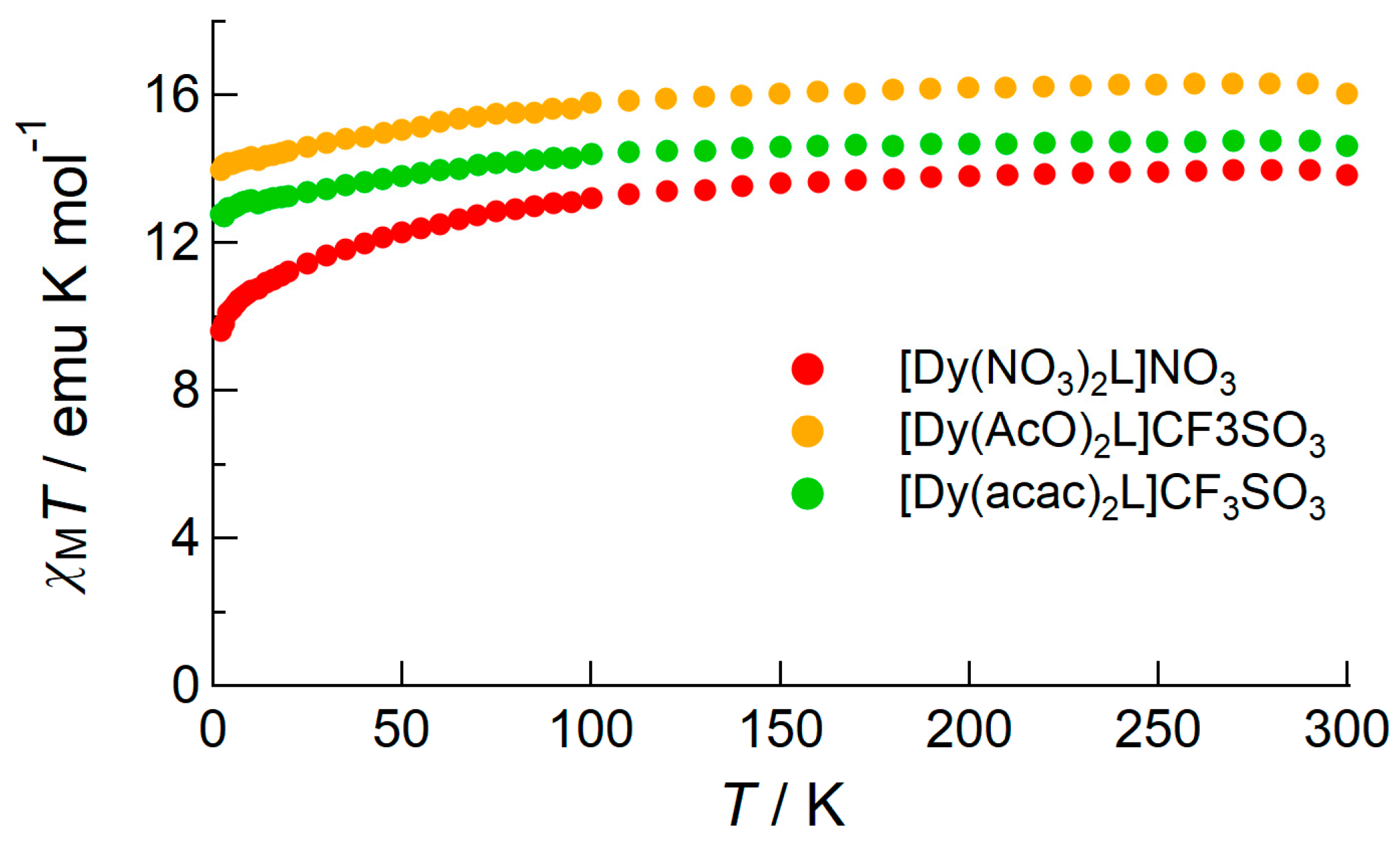
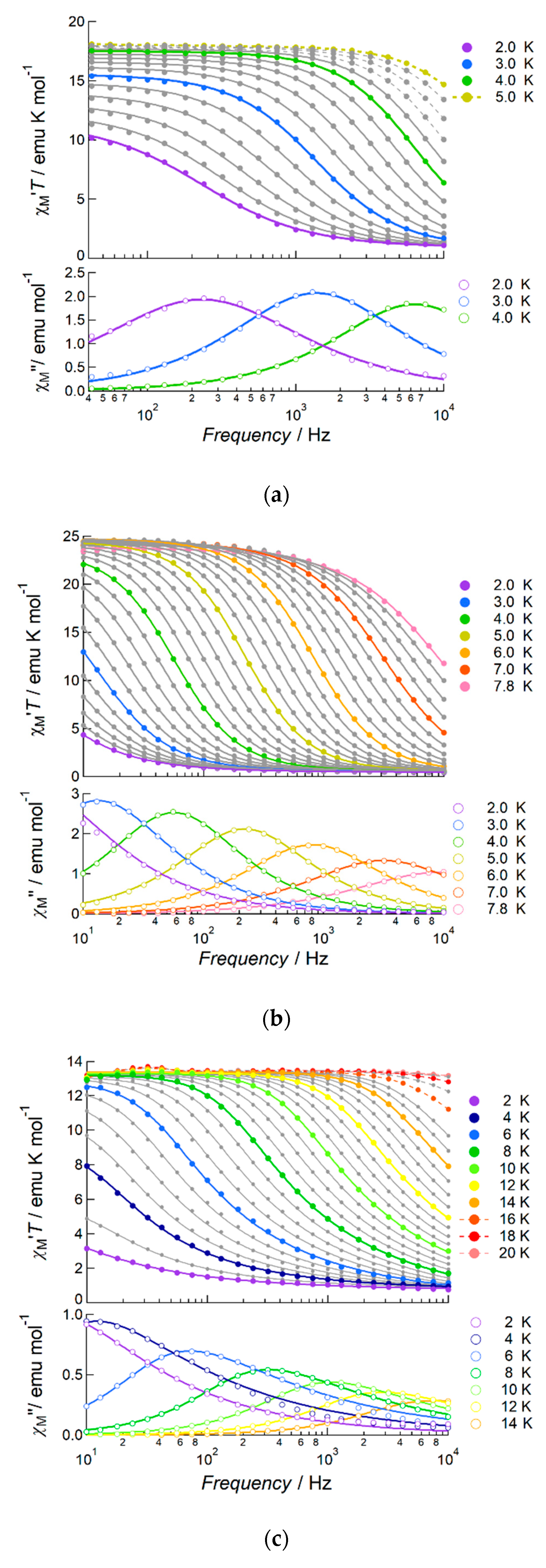
2.2. Magnetic Properties of the Complexes
2.2.1. DC Susceptibility of the Complexes
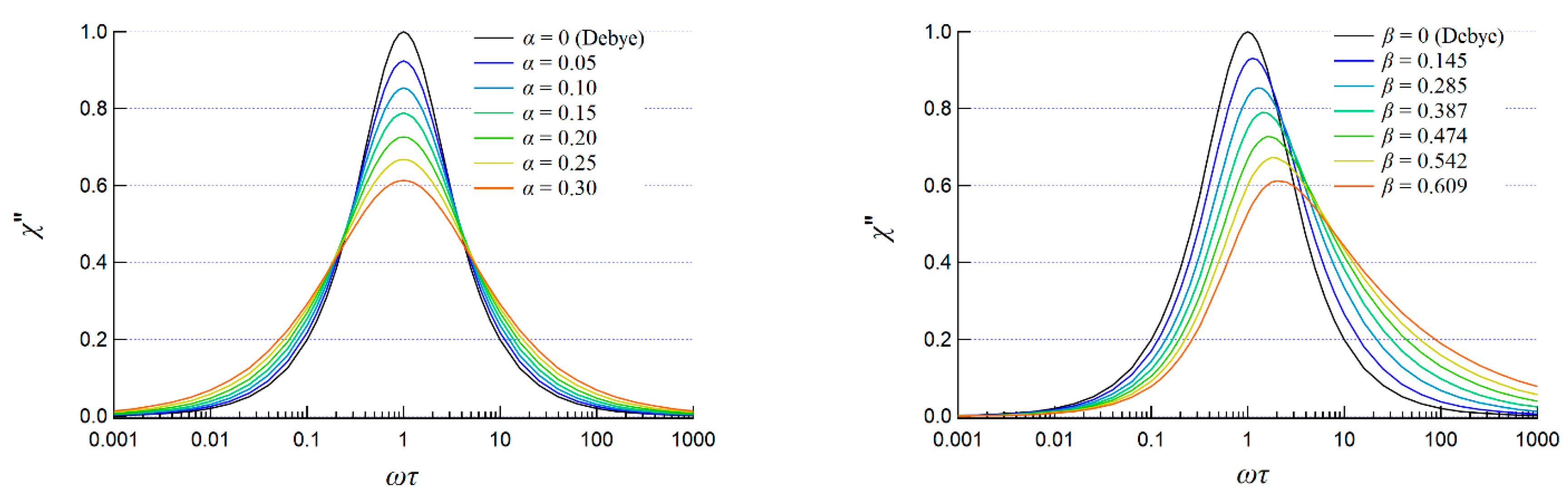
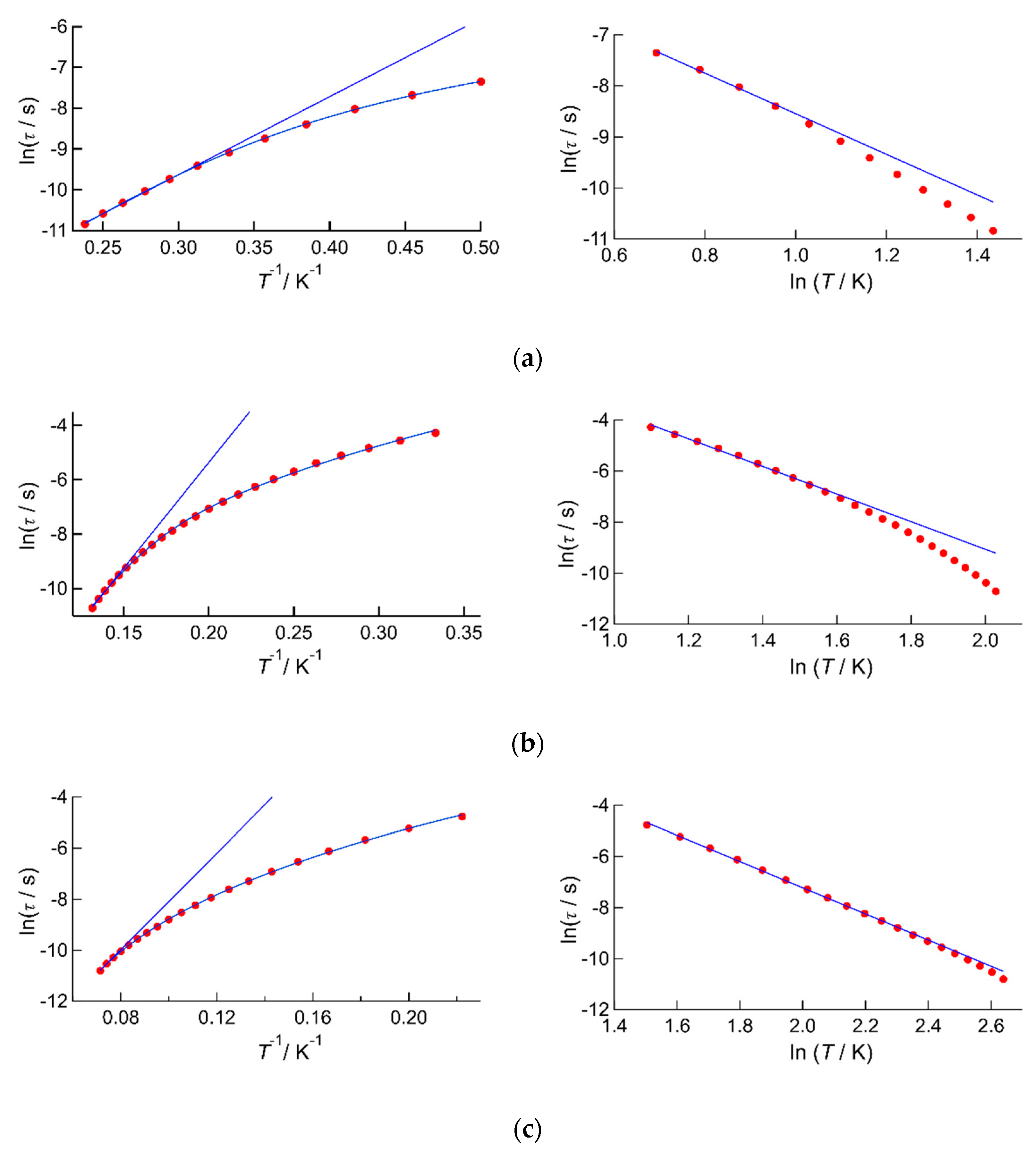
2.2.2. AC Susceptibility of the Complexes
2.3. Molecular Orbital Analyses
3. Materials and Methods
3.1. General Procedures and Methods
3.2. Synthesis of Complexes
3.3. Crystallography
3.4. DFT Calculation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| ORTEP | Oak Ridge Thermal-Ellipsoid Plot program |
References
- Gatteschi, D.; Sessoli, R.; Villain, J. Molecular Nanomagnets; Oxford University Press: New York, NY, USA, 2006. [Google Scholar]
- Layfield, R.A. Organometallic Single-Molecule Magnets. Organometallics 2014, 33, 1084–1099. [Google Scholar] [CrossRef]
- Madhu, N.T.; Tang, J.-K.; Hewitt, I.J.; Clérac, R.; Wernsdorfer, W.; van Slageren, J.; Anson, C.E.; Powell, A.K. What makes a single molecule magnet? Polyhedron 2005, 24, 2864–2869. [Google Scholar] [CrossRef]
- Glaser, T. Rational design of single-molecule magnets: A supramolecular approach. Chem. Commun. 2011, 47, 116–130. [Google Scholar] [CrossRef]
- Woodruff, D.N.; Winpenny, R.E.; Layfield, R.A. Lanthanide single-molecule magnets. Chem. Rev. 2013, 113, 5110–5148. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Guo, Y.-N.; Tang, J. Recent advances in dysprosium-based single molecule magnets: Structural overview and synthetic strategies. Coord. Chem. Rev. 2013, 257, 1728–1763. [Google Scholar] [CrossRef]
- Feltham, H.L.C.; Brooker, S. Review of purely 4f and mixed-metal nd-4f single-molecule magnets containing only one lanthanide ion. Coord. Chem. Rev. 2014, 276, 1–33. [Google Scholar] [CrossRef]
- Liddle, S.T.; van Slageren, J. Improving f-element single molecule magnets. Chem. Soc. Rev. 2015, 44, 6655–6669. [Google Scholar] [CrossRef]
- Meng, Y.S.; Jiang, S.D.; Wang, B.W.; Gao, S. Understanding the Magnetic Anisotropy toward Single-Ion Magnets. Acc. Chem. Res. 2016, 49, 2381–2389. [Google Scholar] [CrossRef] [PubMed]
- Day, B.M.; Guo, F.S.; Layfield, R.A. Cyclopentadienyl Ligands in Lanthanide Single-Molecule Magnets: One Ring to Rule Them All? Acc. Chem. Res. 2018, 51, 1880–1889. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.C.; Liu, J.L.; Ungur, L.; Liu, J.; Li, Q.W.; Wang, L.F.; Ni, Z.P.; Chibotaru, L.F.; Chen, X.M.; Tong, M.L. Symmetry-Supported Magnetic Blocking at 20 K in Pentagonal Bipyramidal Dy(III) Single-Ion Magnets. J. Am. Chem. Soc. 2016, 138, 2829–2837. [Google Scholar] [CrossRef]
- Goodwin, C.A.P.; Ortu, F.; Reta, D.; Chilton, N.F.; Mills, D.P. Molecular magnetic hysteresis at 60 kelvin in dysprosocenium. Nature 2017, 548, 439–442. [Google Scholar] [CrossRef]
- Yu, S.; Hu, Z.; Chen, Z.; Li, B.; Zhang, Y.Q.; Liang, Y.; Liu, D.; Yao, D.; Liang, F. Two Dy(III) Single-Molecule Magnets with Their Performance Tuned by Schiff Base Ligands. Inorg. Chem. 2019. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.W.; Tian, Y.M.; Tao, J.; Chen, P.; Li, H.F.; Zhang, Y.Q.; Yan, P.F.; Sun, W.B. Modulation of the Coordination Environment around the Magnetic Easy Axis Leads to Significant Magnetic Relaxations in a Series of 3d-4f Schiff Complexes. Inorg. Chem. 2018, 57, 8065–8077. [Google Scholar] [CrossRef] [PubMed]
- Shen, F.X.; Li, H.Q.; Miao, H.; Shao, D.; Wei, X.Q.; Shi, L.; Zhang, Y.Q.; Wang, X.Y. Heterometallic M(II)Ln(III) (M = Co/Zn; Ln = Dy/Y) Complexes with Pentagonal Bipyramidal 3d Centers: Syntheses, Structures, and Magnetic Properties. Inorg. Chem. 2018. [Google Scholar] [CrossRef] [PubMed]
- Ehama, K.; Ohmichi, Y.; Sakamoto, S.; Fujinami, T.; Matsumoto, N.; Mochida, N.; Ishida, T.; Sunatsuki, Y.; Tsuchimoto, M.; Re, N. Synthesis, structure, luminescent, and magnetic properties of carbonato-bridged Zn(II)2Ln(III)2 complexes [(m4-CO3)2{Zn(II)L(n)Ln(III)(NO3)}2] (Ln(III) = Gd(III), Tb(III), Dy(III); L(1) = N,N′-bis(3-methoxy-2-oxybenzylidene)-1,3-propanediaminato, L(2) = N,N′-bis(3-ethoxy-2-oxybenzylidene)-1,3-propanediaminato). Inorg. Chem. 2013, 52, 12828–12841. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Basalov, I.V.; Forosenko, N.V.; Lyssenko, K.A.; Mamontova, E.; Cherkasov, A.V.; Damjanovic, M.; Chibotaru, L.F.; Guari, Y.; Larionova, J.; et al. Dysprosium Single-Molecule Magnets with Bulky Schiff Base Ligands: Modification of the Slow Relaxation of the Magnetization by Substituent Change. Chemistry 2019, 25, 474–478. [Google Scholar] [CrossRef]
- Bar, A.K.; Kalita, P.; Sutter, J.P.; Chandrasekhar, V. Pentagonal-Bipyramid Ln(III) Complexes Exhibiting Single-Ion-Magnet Behavior: A Rational Synthetic Approach for a Rigid Equatorial Plane. Inorg. Chem. 2018, 57, 2398–2401. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Tolpygin, A.O.; Cherkasov, A.V.; Lyssenko, K.A.; Guari, Y.; Larionova, J.; Trifonov, A.A. Single-Molecule Magnet Behavior in Dy3+ Half-Sandwich Complexes Based on Ene-Diamido and Cp* Ligands. Organometallics 2019, 38, 748–752. [Google Scholar] [CrossRef]
- Maxwell, L.; Amoza, M.; Ruiz, E. Mononuclear Lanthanide Complexes with 18-Crown-6 Ether: Synthesis, Characterization, Magnetic Properties, and Theoretical Studies. Inorg. Chem. 2018, 57, 13225–13234. [Google Scholar] [CrossRef]
- Wada, H.; Ooka, S.; Iwasawa, D.; Hasegawa, M.; Kajiwara, T. Slow Magnetic Relaxation of Lanthanide(III) Complexes with a Helical Ligand. Magnetochemistry 2016, 2, 43. [Google Scholar] [CrossRef]
- Ji, C.-L.; Jiang, Y.-X.; Zhang, J.-C.; Qi, Z.-Y.; Kong, J.-J.; Huang, X.-C. Field-induced Slow Magnetic Relaxation Behavior in a Mononuclear Dy(III) Complex based on 8-Hydroxyquinoline Derivate Ligand. Zeitschrift für Anorganische und Allgemeine Chemie 2018, 644, 1635–1640. [Google Scholar] [CrossRef]
- Then, P.L.; Takehara, C.; Kataoka, Y.; Nakano, M.; Yamamura, T.; Kajiwara, T. Structural switching from paramagnetic to single-molecule magnet behaviour of LnZn2 trinuclear complexes. Dalton Trans. 2015, 44, 18038–18048. [Google Scholar] [CrossRef] [PubMed]
- Hino, S.; Maeda, M.; Yamashita, K.; Kataoka, Y.; Nakano, M.; Yamamura, T.; Nojiri, H.; Kofu, M.; Yamamuro, O.; Kajiwara, T. Linear trinuclear Zn(II)-Ce(III)-Zn(II) complex which behaves as a single-molecule magnet. Dalton Trans. 2013, 42, 2683–2686. [Google Scholar] [CrossRef] [PubMed]
- Takehara, C.; Then, P.L.; Kataoka, Y.; Nakano, M.; Yamamura, T.; Kajiwara, T. Slow magnetic relaxation of light lanthanide-based linear LnZn2 trinuclear complexes. Dalton Trans. 2015, 44, 18276–18283. [Google Scholar] [CrossRef] [PubMed]
- Wada, H.; Ooka, S.; Yamamura, T.; Kajiwara, T. Light Lanthanide Complexes with Crown Ether and Its Aza Derivative Which Show Slow Magnetic Relaxation Behaviors. Inorg. Chem. 2017, 56, 147–155. [Google Scholar] [CrossRef]
- Hasegawa, M.; Ohtsu, H.; Kodama, D.; Kasai, T.; Sakurai, S.; Ishii, A.; Suzuki, K. Luminescence behaviour in acetonitrile and in the solid state of a series of lanthanide complexes with a single helical ligand. New J. Chem. 2014, 38, 1225–1234. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and Absorption in Dielectrics I. Alternating Current Characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- Davidson, D.W.; Cole, R.H. Dielectric Relaxation in Glycerol, Propylene Glycol, andn-Propanol. J. Chem. Phys. 1951, 19, 1484–1490. [Google Scholar] [CrossRef]
- Abragam, A.; Bleaney, B. Electron Paramagnetic Resonance of Transition Ions; Oxford University Press: Oxford, UK, 1970. [Google Scholar]
- Carlin, R.L. Magnetochemistry; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Sakamoto, M.; Matsumoto, N.; Okawa, H. Synthesis and Molecular Structure of Europium(III) Complex with 2,6-Diacetylpyridine Bis(2-pyridylhydrazone). Bull. Chem. Soc. Jpn. 1991, 64, 691–693. [Google Scholar] [CrossRef]
- Crystal Clear, Operating Software for the CCD Detector System; Version 1.3.5; Rigaku and Molecular Structure Corp.: Tokyo, Japan; The Woodlands, TX, USA, 2003.
- Altomare, A.; Burla, M.C.; Camalli, M.; Cascarano, G.L.; Giacovazzo, C.; Guagliardi, A.; Moliterni, A.G.G.; Polidori, G.; Spagna, R. SIR97: A new tool for crystal structure determination and refinement. J. Appl. Crystallogr. 1999, 32, 115–119. [Google Scholar] [CrossRef]
- Sheldrick, G. Crystal structure refinement with SHELXL. Acta Crystallogr. Sect. C Struct. Chem. 2015, 71, 3–8. [Google Scholar] [CrossRef] [PubMed]
- Becke, A. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Andrae, D.; Häußermann, U.; Dolg, M.; Stoll, H.; Preuß, H. Energy-adjustedab initio pseudopotentials for the second and third row transition elements. Theor. Chim. Acta 1990, 77, 123–141, Note that this basis set is quasi-relativistic and sometimes called MWB28 or SDD. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09; Revision C01; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]



| Complex | 1 | 2 | 3 |
|---|---|---|---|
| ΔE kB‒1/K | 24.1(6) | 85(3) | 140(15) |
| τ0/s | 9.0(9) × 10−8 | 5(2) × 10−10 | 3(3) × 10−9 |
| n | 3.09(15) | 5.4(1) | 5.06(4) |
| C/s‒1 K‒n | 174(18) | 0.17(2) | 0.054(4) |
| Orbitals | m1 | m2 | m3 |
|---|---|---|---|
| f0 | 0.49 | 0.43 | 0.80 |
| f+1 | 0.98 | 0.72 | 0.64 |
| f−1 | 0.88 | 0.84 | 0.35 |
| f+2 | 0.51 | 0.86 | 0.73 |
| f−2 | 0.55 | 0.21 | 0.72 |
| f+3 | 0.61 | 0.85 | 0.74 |
| f−3 | 0.93 | 0.51 | 0.44 |
| Sum of f orbitals | 4.96 | 4.42 | 4.41 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kobayashi, K.; Harada, Y.; Ikenaga, K.; Kitagawa, Y.; Nakano, M.; Kajiwara, T. Correlation between Slow Magnetic Relaxations and Molecular Structures of Dy(III) Complexes with N5O4 Nona-Coordination. Magnetochemistry 2019, 5, 27. https://doi.org/10.3390/magnetochemistry5020027
Kobayashi K, Harada Y, Ikenaga K, Kitagawa Y, Nakano M, Kajiwara T. Correlation between Slow Magnetic Relaxations and Molecular Structures of Dy(III) Complexes with N5O4 Nona-Coordination. Magnetochemistry. 2019; 5(2):27. https://doi.org/10.3390/magnetochemistry5020027
Chicago/Turabian StyleKobayashi, Kaede, Yukina Harada, Kazuki Ikenaga, Yasutaka Kitagawa, Masayoshi Nakano, and Takashi Kajiwara. 2019. "Correlation between Slow Magnetic Relaxations and Molecular Structures of Dy(III) Complexes with N5O4 Nona-Coordination" Magnetochemistry 5, no. 2: 27. https://doi.org/10.3390/magnetochemistry5020027
APA StyleKobayashi, K., Harada, Y., Ikenaga, K., Kitagawa, Y., Nakano, M., & Kajiwara, T. (2019). Correlation between Slow Magnetic Relaxations and Molecular Structures of Dy(III) Complexes with N5O4 Nona-Coordination. Magnetochemistry, 5(2), 27. https://doi.org/10.3390/magnetochemistry5020027







