Slow Relaxation of the Magnetization in Bis-Decorated Chiral Helicene-Based Coordination Complexes of Lanthanides

Abstract

The complexes [Ln₂(hfac)₆(L)]·nC₆H₁₄ (Ln = Dy (1) n = 0, Yb (2) n = 1) with the L chiral 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene ligand (hfac⁻ = 1,1,1,5,5,5-hexafluoroacetylacetonate) have been synthesized in their racemic form and structurally and magnetically characterized. Both complexes behave as field-induced single molecule magnets in the crystalline phase. These magnetic properties were rationalized by ab initio calculations.

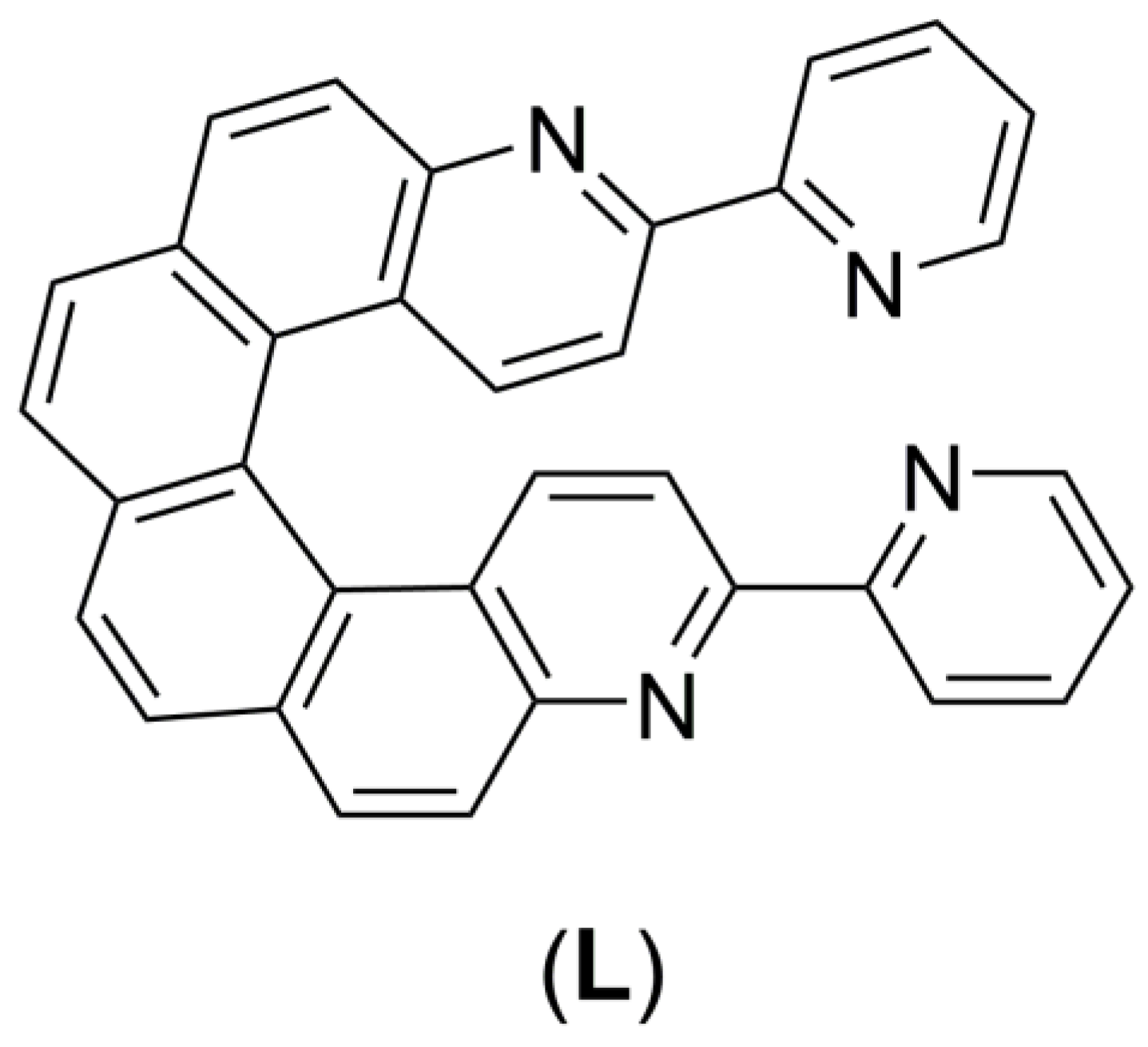
1. Introduction

The development of nanomaterials for high density data storage, the development of molecular Qubits/Qudits for quantum computing and spintronics is very active in the field of molecular magnetism, and both chemists and physicists communities are working hand-in-hand to make rapid progress in this field [1,2,3,4,5,6,7]. In this context, lanthanide-based complexes are currently intensively studied, especially those displaying Single-Molecule Magnet (SMM) behavior. The interest in such species is due to the intrinsic strong magnetic anisotropy and high magnetic moment of the lanthanides [8,9,10,11,12,13,14,15]. The electronic density distribution leads to two classes of lanthanides i.e., prolate ions (axial distribution) and oblate ions (planar distribution) [16]. The most popular ions for each class are the Yb^III and Dy^III ions, respectively. The coordination sphere plays a crucial role for tuning adequate electronic distribution and energy splitting of the ground multiplet state leading to the observation of SMM behavior. For the oblate Dy^III ion, the association of the β-diketonate anions and bis-chelating nitrogenated ligand (N2O6 coordination sphere) often permits the detection of slow magnetic relaxation, which is not the case for the prolate Yb^III ion [17,18,19,20,21,22,23].

In addition, another challenge is to combine one more property to the SMM behavior. In this framework, a hot topic is the design of chiral SMMs [24,25,26]. In our group, special attention is devoted to the use of high optical activity ligands such as the [n]-helicene ligand. By studying the complexes [Dy(hfac)₃(bpyhelicene)] and [Dy(tta)₃(bpyhelicene)] where bpyhelicene = 3-(2-pyridyl)-4-aza[6]-helicene ligand, some of us demonstrated that the different X-ray structures between the racemic and enantiomerically pure forms could lead to drastic changes of magnetic behavior due to the change of dipolar interaction intensity or nature (ferro versus antiferromagnetic) [27,28].

In the present paper, we proposed to go one step forward by functionalizing the [6]-helicene core with two 2,2-bipyridine moieties. The resulting 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene ligand (L) (Scheme 1) was coordinated to Ln(hfac)₃ units (Ln = Dy and Yb) giving rise to the dinuclear complexes of formula [Ln₂(hfac)₆(L)]·C₆H₁₄. The two compounds were structurally characterized by X-ray diffraction and their magnetic properties studied by static and dynamic measurements.

2. Results and Discussion

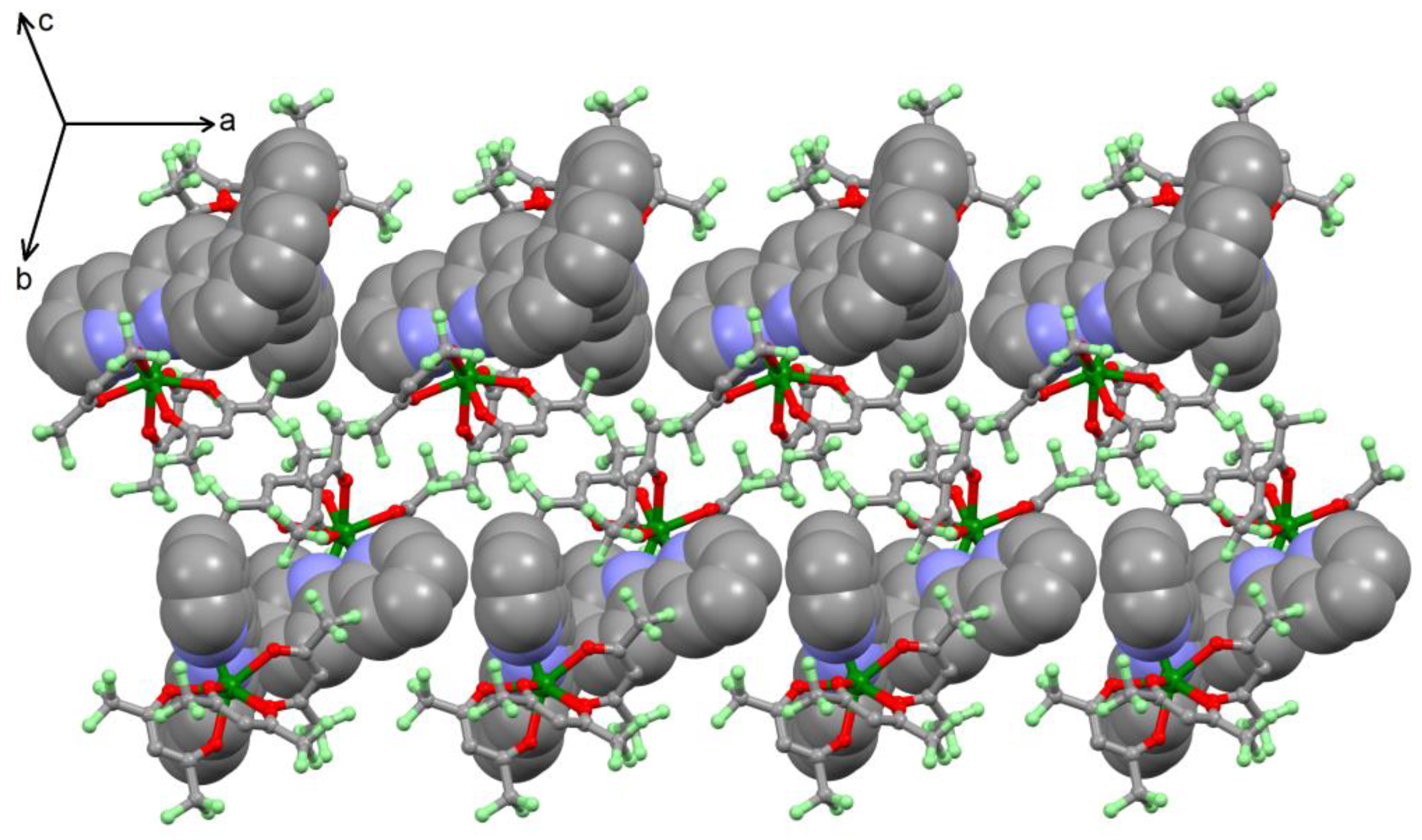
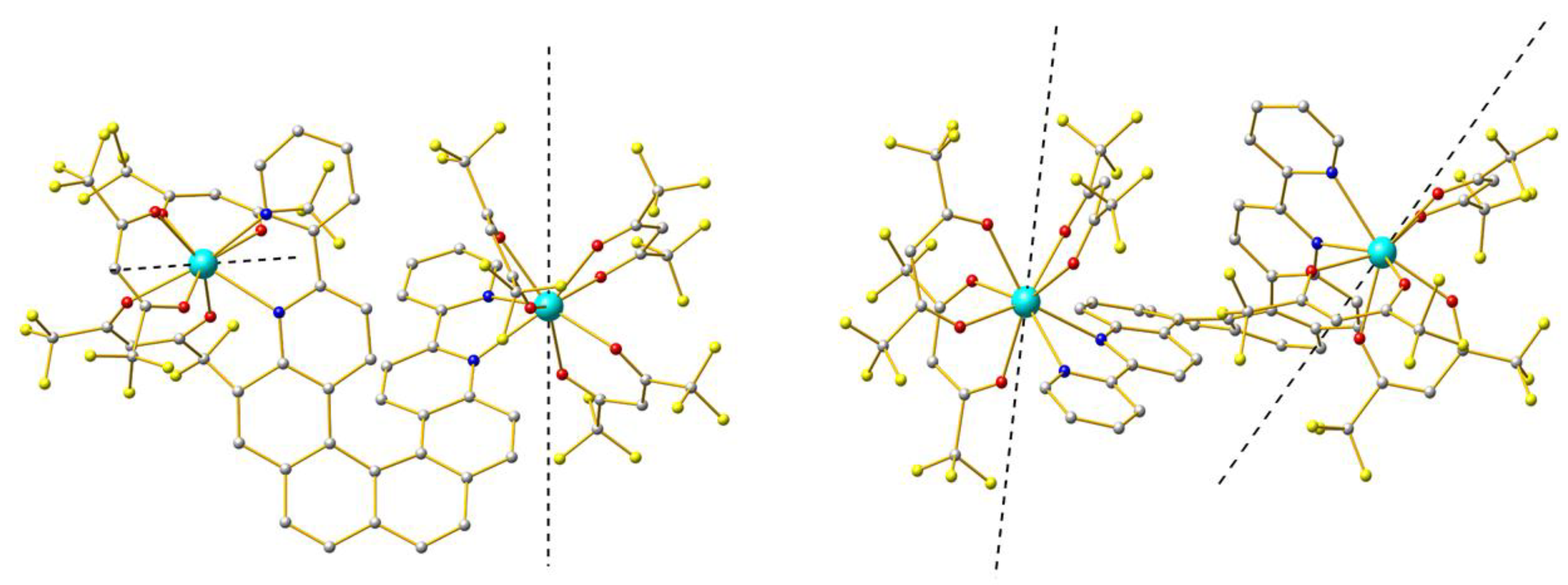
2.1. X-ray Structures

The coordination reaction of the chiral 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene ligand (L) and tris(1,1,1,5,5,5-hexafluoroaacethylacetonate)bis(aqueous)Ln^III in CH₂Cl₂ led to the formation of both complexes 1 (Ln = Dy) and 2 (Ln = Yb). Both compounds crystallized in the triclinic centrosymmetric space group P-1 (Figure 1 and Figure S1 and Figure S2, Table S1). The asymmetric unit is composed by a [Ln₂(hfac)₆(L)] dinuclear species and one n-hexane molecule of crystallization for 2. The two 2,2′-bipyridine coordination sites are occupied by a Ln(hfac)₃ (hfac⁻ = 1,1,1,5,5,5-hexafluoroacethyacetonate) moiety. Thus each Ln^III ion is surrounded by two nitrogen atoms and six oxygen atoms coming from the three hfac⁻ anions and the L ligand. Nevertheless the two resulting N₂O₆ coordination polyhedrons have distinct symmetries, as shown by the deviations from ideal symmetries determined from the SHAPE program (Table S2) [29]. Since distinct symmetries lead to different electronic distributions, a significant impact on the magnetic properties could be expected (see magnetic section).

Both enantiomers are present in the cell since the reaction is performed starting from the racemic mixture of L. The two planes represented by the two 2,2′-bipyridine moieties form an angle of 55.3(1)° and 61.0(1)° and the conformation of the ligand leads to an intramolecular Ln–Ln distance of 9.320 Å and 9.890 Å for 1 and 2, respectively.

Remarkably, even if on the molecular level the two compounds are very similar, the two crystal packings are drastically different. For 1, the crystal packing reveals that head-to-tail heterochiral dimers are formed with the presence of π–π interactions between the 2,2-bipyridyl terminal moiety and the helicenic core (Figure 2). An organic network runs along the a axis thanks to π–π interactions between the helicenic cores of neighboring dimers (Figure 2).

For 2, the crystal packing reveals that the homochiral dinuclear complexes arrange themselves along the a axis while the dinuclear complexes with the other enantiomer of L form the neighboring arrangement (Figure 3), thus yielding alternated arrays of homochiral columns. Surprisingly the organic network is not built through π–π stacking as observed for 1 and for the mononuclear complexes involving the 3-(2-pyridyl)-4-aza[6]-helicene ligand [27] but through CH–π interactions between the 2,2′-bipyridine moieties.

The shortest Ln–Ln intermolecular distance is 8.179 Å and 8.956 Å for 1 and 2, respectively, which is similar to the distances measured in the mononuclear complexes involving both Dy(hfac)₃ and Dy(tta)₃ metallo-precursor [27,28].

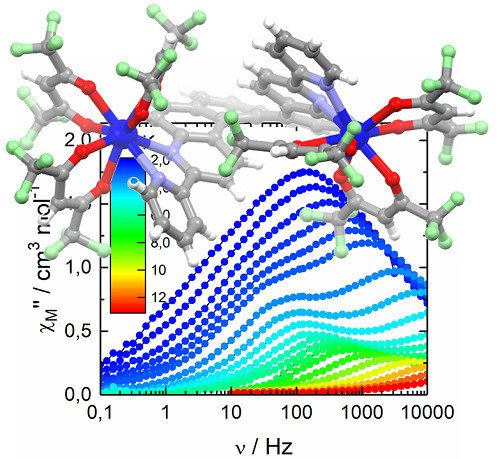
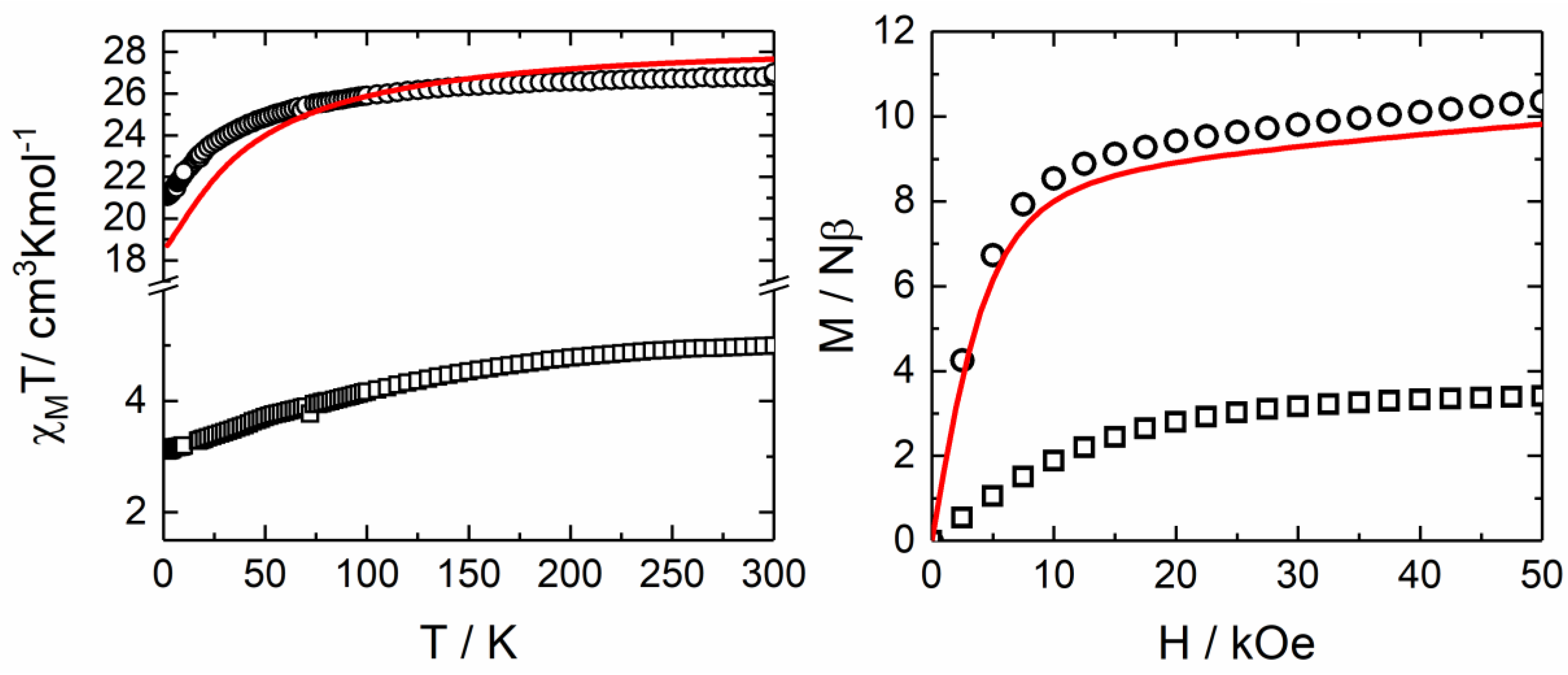
2.2. Magnetic Properties

2.2.1. Static magnetic Measurements

The temperature dependence of χ_MT for the samples 1 and 2 are represented in Figure 4. The room temperature values are 26.82 cm³·K·mol⁻¹ and 5.00 cm³·K·mol⁻¹, respectively, for 1 and 2 which are in good agreement with the expected value of 28.34 cm³·K·mol⁻¹ and 5.14 cm³·K·mol⁻¹ for two isolated Dy^III ions (⁶H_15/2 ground state multiplet) and Yb^III (²F_7/2 ground state multiplet), respectively [30]. Upon cooling, χ_MT decreases monotonically down to 21.10 cm³·K·mol⁻¹ for 1 and 3.11 cm³·K·mol⁻¹ for 2 due to the thermal depopulation of the M_J states. The field dependence of the magnetization measured at 2.0 K reaches the value of 10.36 µ_B for 1 and 3.41 µ_B for 2 under a magnetic field of 50 kOe, which is far from the expected saturated values of 20 µ_B for 1 and 4 µ_B for 2 (Figure 4) [30].

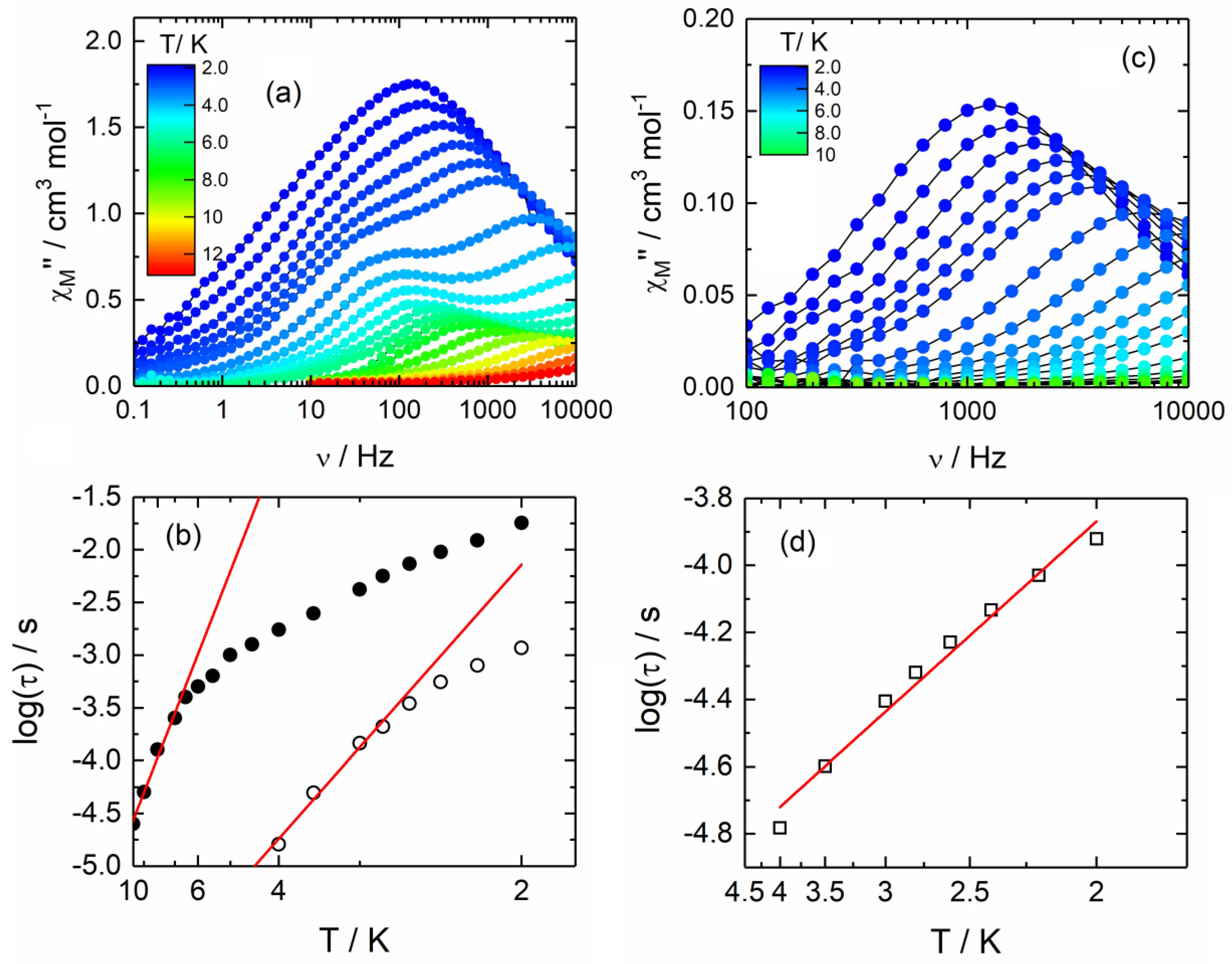
2.2.2. Dynamic Magnetic Measurements

The out-of-phase component of the ac susceptibility (χ_M”) for both compounds 1 and 2 were measured using immobilized crunched single crystals. For both compounds, no out-of-phase signal was detected in zero magnetic field. In order to suppress the fast magnetic relaxation through quantum tunneling of the magnetization (QTM), the field dependence of the magnetic susceptibility is studied. For both compounds, the application of a small magnetic field led to the appearance of an out-of-phase component of the magnetic susceptibility. Nevertheless for 1, two contributions are observed whatever the value of the magnetic field (Figure S3) and the value of 800 Oe is selected (value at which the fast relaxation contribution is centered at the lowest frequency i.e., 152 Hz at 2 K), while for 2, the maximum of the out-of-phase component remains up to 1000 Hz (Figure S4). Thus the frequency dependence of the magnetic susceptibility for 1 and 2 has been measured up to 10,000 Hz.

Under an applied field of 800 Oe, 1 highlighted a frequency dependence of the out-of-phase signal of the magnetization (Figure 5a and Figure S5). We manually selected the frequency maxima to plot the temperature dependence of the relaxation time (log(τ) vs T for both high (HF, open circles) and low frequency (LF, full circles) contributions (Figure 5b, Table S3). The relaxation time follows the Arrhenius law τ = τ₀ exp(Δ/kT) at high temperature with τ₀ = 4.6(3) × 10⁻⁸ s and Δ = 16.6(1) cm⁻¹ for HF and τ₀ = 1.2(2) × 10⁻⁷ s and Δ = 37.7(5) cm⁻¹ for LF (Figure 5b), while other magnetic relaxation pathways are observed at lower temperatures such as Raman and Direct processes. The two different relaxation times could be attributed to the two crystallographically independent Dy centers.

Under an applied field of 1000 Oe, 2 highlighted a frequency dependence of the out-of-phase signal of the magnetization (Figure 5c and Figure S6) which can be also analyzed in the framework of the extended Debye model [31,32]. The temperature dependence of the relaxation time was extracted and depicted in Figure 5d (Table S3). The relaxation time follows the Arrhenius law τ = τ₀ exp(Δ/kT) between 2 and 4 K with τ₀ = 2.7(4) × 10⁻⁶ s and Δ = 5.5(3) cm⁻¹ (Figure 5d). Even if the magnetic performances of 2 are modest, the observation of a field-induced SMM for an Yb^III ion in a N₂O₆ coordination site has unprecedented example.

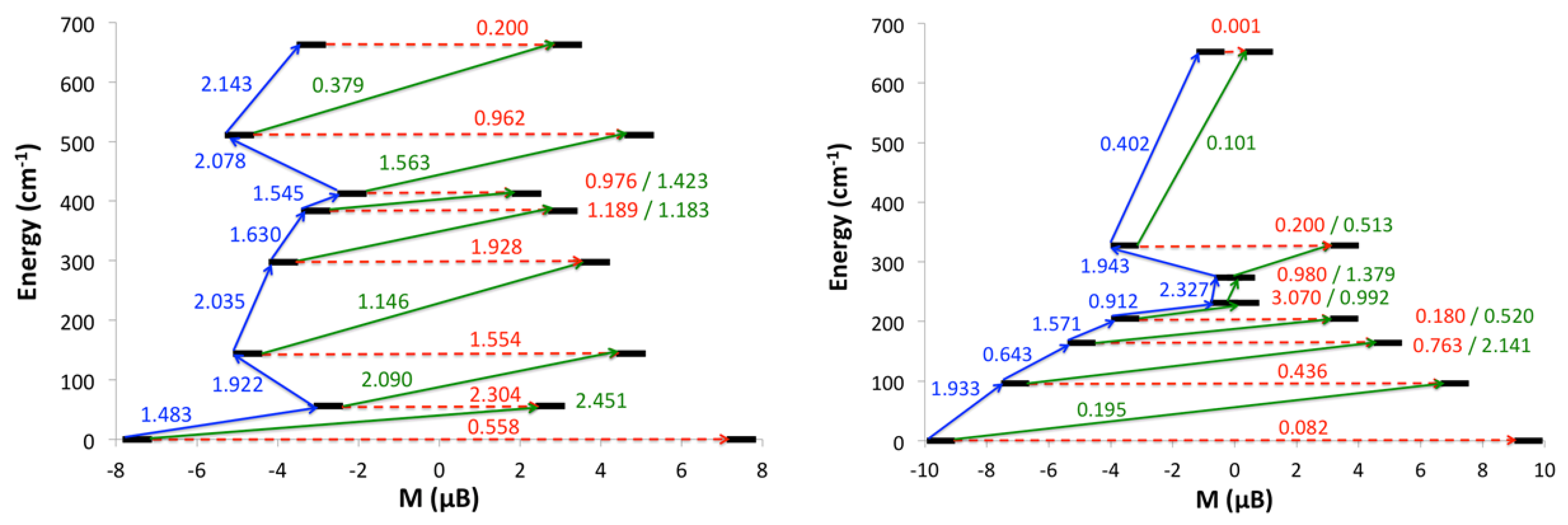
2.2.3. Ab Initio Calculations

SA-CASSCF/RASSI-SO calculations were performed for 1 to rationalize the observed magnetic properties (see computational details). The calculated χ_MT vs T and M vs H (Figure 4) curves fairly well reproduce the experimental curves. Dy1 presents a strongly mixed ground state (30% M_J = |±13/2>, 27% M_J = |±11/2>, 23% M_J = |±15/2>, and 11% M_J = |±7/2>, Table S4) defined by a g-tensor with a main component g_Z = 14.94 and exhibiting non-negligible transversal components with g_X = 1.34 and g_Y = 2.01 confirming the low anisotropy character of the ground state (for a pure M_J = |±15/2> ground state, the fully axial, Ising-type, g-tensor expected possess g_X = g_Y = 0.0 and g_Z = 20.0). For Dy2, the ground state is calculated to be mainly M_J = |±15/2> (86% M_J = |±15/2> and 11% M_J = |±11/2>, Table S5) with a g-tensor showing a stronger anisotropy character than Dy1, with g_Z = 18.97 and lower transversal components (g_X = 0.17 and g_Y = 0.33). At this point the Dy1 and Dy2 magnetic relaxations could be attributed to the HF and LF contributions, respectively. The main component of the ground state g-tensor of each Dy^III center is represented in Figure 6. For both magnetic centers, the main magnetic axis appears perpendicular to the plane containing the N atoms from the helicene ligand, as expected for an oblate ion in this coordination sphere [33,34].

The deviations observed between the two magnetic centers may be related to the differences in the coordination spheres. In fact, it is well-known that to one electronic distribution is associated one magnetic behavior, and consequently when more than one crystallographically lanthanide center is identified in a polynuclear SMM, a multi-magnetic relaxation is expected [35,36,37,38,39,40,41]. In order to give more insights into the relaxation mechanisms observed for the two Dy^III centers, we computed the transversal magnetic moments between the Stark levels of each Kramers ion (Figure 7). The two magnetic centers relax slightly differently with probable main relaxation pathways through the 1st excited state. However, the major relaxation for Dy1 seems to proceed with large quantum-tunneling elements (0.558 μ_B and 2.304 μ_B for the ground and first excited states, respectively) while Dy2 displays much weaker QTM values. These differences are in the trend of the experimental results with a faster relaxation of the magnetization for Dy1 arising from the large QTM values compared to Dy2 which shows a slower relaxation (small QTM values between the first KDs). The difference between the calculated energy barrier (Δ = 55.9 cm⁻¹ and 96 cm⁻¹) and the experimental barriers (Δ = 16.6 cm⁻¹ and 37.7 cm⁻¹), while the trend is conserved, is common in the literature [42,43,44] and may be explained because the coupling of spin-phonon degrees of freedom in the SMM relaxation is not taken into account in the ab initio model [45,46].

A qualitative comparison with the literature highlighted a classic magnetic behavior for the LF Dy magnetic relaxation while the HF Dy magnetic relaxation displayed a faster relaxation of the magnetization than the classical Dy^III in N2O6 coordination environment [17,18,19,20,21,22,23]. Such an observation could be due to the specific electronic distribution caused by the bulky 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene ligand.

3. Conclusions

In this article, the [6]-helicene-based lanthanide SMM family was extended starting from the 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene ligand. The latter is composed of two 2,2′-bipyridine coordination sites able to coordinate two Ln(hfac)₃ units (hfac⁻ = 1,1,1,5,5,5-hexafluoroacetylacetonate) to give the dinuclear complexes of formula [Ln₂(hfac)₆(L)]·nC₆H₁₄ (Ln = Dy (1) n = 0, Yb (2) n = 1). Both compounds have been characterized by X-ray diffraction and displayed field-induced Single-Molecule Magnet behavior. The magnetic properties of 1 were rationalized by ab initio calculations. It is worth noting that 2 is the first N2O6 Yb^III field-induced SMM. These systems are still under investigation by our group to study the optical and magnetic properties of their enantiomerically pure forms.

4. Materials and Methods

4.1. Synthesis General Procedures and Materials

The precursor Dy(hfac)₃·2H₂O (hfac⁻ = 1,1,1,5,5,5-hexafluoroacetyacetonate anion) was synthesized following previously reported methods [47]. All other reagents were commercially available and used without further purification.

4.2. Synthesis of Complex [Ln₂(hfac)₆(L)]·nC₆H₁₄ (Ln = Dy (1) n = 0, Yb (2) n = 1)

Ln(hfac)₃·2H₂O (32.8 mg for Ln = Dy and 33.2 mg for Ln = Yb, 0.04 mmol) were dissolved in 5 mL of CH₂Cl₂ and then added to a solution of 5 mL of CH₂Cl₂ containing 9.7 mg of L (0.02 mmol). After 20 min of stirring, 30 mL of n-hexane was layered at room temperature in the dark. Slow diffusion following by slow evaporation lead to yellow single crystals that were suitable for X-ray studies. Yield: 17.7 mg (41%) for 1 and 21.4 mg (49%) for 2. Anal. Calcd (%) for C₆₄H₂₆Dy₂F₃₆N₄O₁₂: C 37.43, H 1.27, N 2.73; found: C 37.39, H 1.33 N, 2.75. Anal. Calcd (%) for C₇₀H₄₀Yb₂F₃₆N₄O₁₂: C 38.53, H 1.83, N 2.57; found: C 38.47, H 1.99 N, 2.50.

4.3. Crystallography

Single crystals of 1 and 2 were mounted on a APEXIII D8 VENTURE Bruker-AXS diffractometer for data collection (MoK_α radiation source, λ = 0.71073 Å), from the Centre de Diffractométrie (CDIFX), Université de Rennes 1, France (Table S1). The structure was solved with a direct method using the SHELXT program [48] and refined with a full matrix least-squares method on F² using the SHELXL-14/7 program [49]. Complete crystal structure results as a CIF file including bond lengths, angles, and atomic coordinates are deposited as Supporting Information. CCDC number is 1854828 and 1854829 for compounds 1 and 2, respectively.

4.4. Physical Measurements

The elemental analyses of the compounds were performed at the Centre Régional de Mesures Physiques de l’Ouest, Rennes. The dc magnetic susceptibility measurements were performed on solid polycrystalline samples with a Quantum Design MPMS-XL SQUID magnetometer between 2 and 300 K in an applied magnetic field of 0.2 kOe for temperatures of 2–20 K, 2 kOe between 20 and 80 K and 10 kOe above. These measurements were all corrected for the diamagnetic contribution as calculated by Pascal’s constants. The ac magnetic susceptibility measurements were performed on both Quantum Design MPMS-XL SQUID magnetometer and Quantum Design PPMS system equipped with ac/dc probe.

4.5. Computational Details

The atomic positions were extracted from the X-ray diffraction crystal structure of the [Dy₂(hfac)₆(L)] system (L = 3,14-di-(2-pyridyl)-4,13-diaza[6]helicene). A calculation is performed on each magnetic center while the other Dy^III ion is replaced by the diamagnetic Y^III ion.

All ab initio calculations were performed using the State-Averaged Complete Active Space Self-Consistent Field approach with the restricted-active-space-state-interaction method (SA-CASSCF/RASSI-SO), as implemented in the MOLCAS quantum-chemistry package (version 8.0) [50]. The relativistic effects are treated in two steps on the basis of the Douglas–Kroll Hamiltonian. The scalar terms were included in the basis-set generation and were used to determine the CASSCF wavefunctions and energies [51]. Spin-orbit coupling was then added within the RASSI-SO method, which mixes the calculated CASSCF wavefunctions [52,53]. The resulting spin-orbit wavefunctions and energies were used to compute the magnetic properties and g-tensors of the ground state multiplet following the pseudospin S = 1/2 formalism, as implemented in the SINGLE-ANISO routine [43,46]. Cholesky decomposition of the bielectronic integrals was employed to save disk space and to speed up the calculations [54].

The active space considered in the calculations consisted of the nine 4f electrons of the Dy(III) ion, spanning the seven 4f orbitals; that is, CAS(9,7)SCF. State-averaged CASSCF calculations were performed for all of the sextets (21 roots), all of the quadruplets (224 roots), and 300 out of the 490 doublets of the Dy^III ion. Twenty-one sextets, 128 quadruplets, and 107 doublets were mixed through spin–orbit coupling in RASSI-SO. All atoms were described with ANO-RCC basis sets with the following contractions [8s7p4d3f2g1h] for Dy; [7s6p4d2f] for Y; [4s3p2d] for the O and N atoms; [3s2p1d] for C of the first coordination sphere and [3s2p] for the other C atoms; [2s1p] for F and [2s] for the H atoms [55,56].