Analysis of the Anisotropic Magnetocaloric Effect in RMn2O5 Single Crystals
Abstract
:1. Introduction
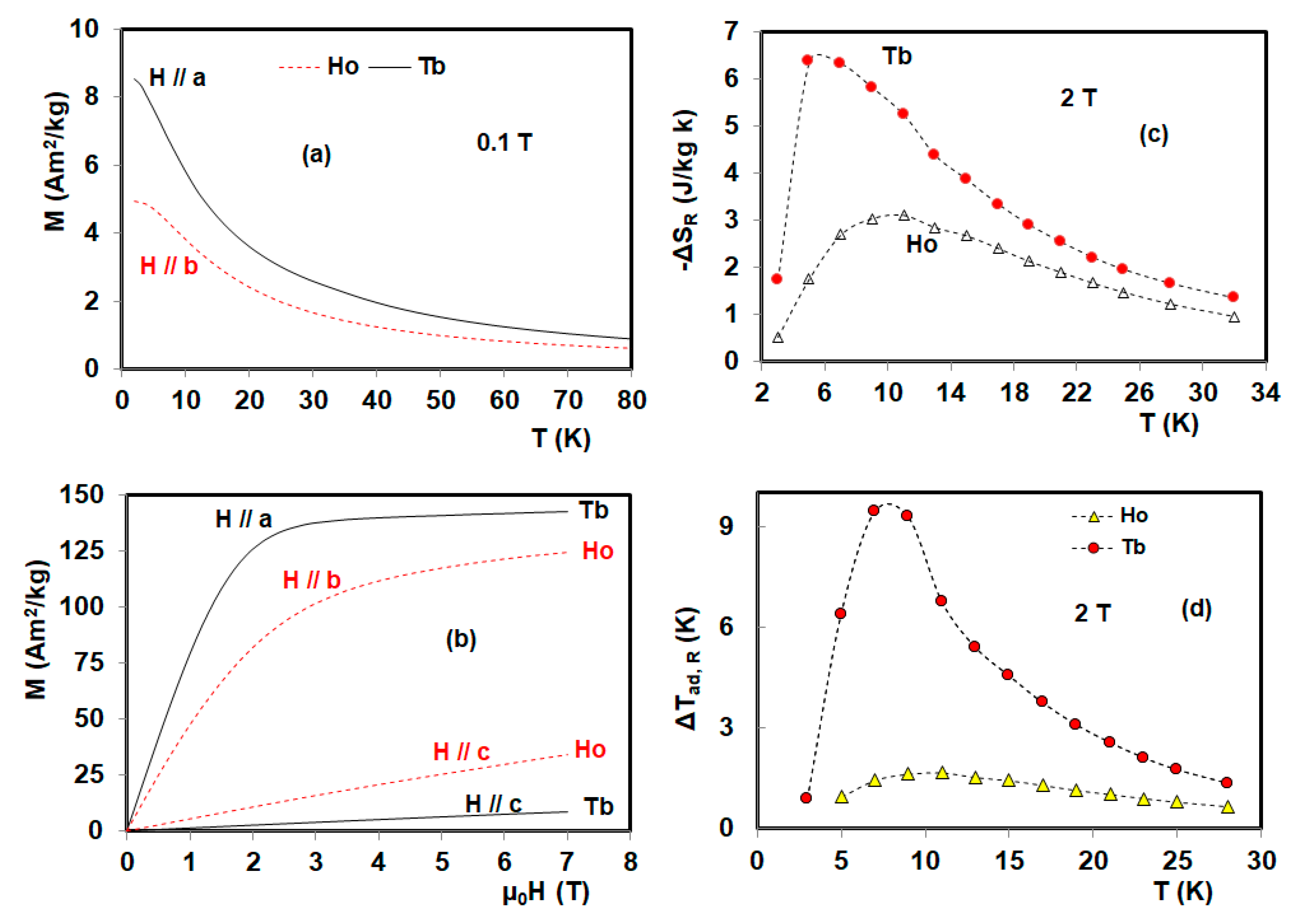
2. RMCE in RMn2O5 (R = Tb and Ho): Comparative Study
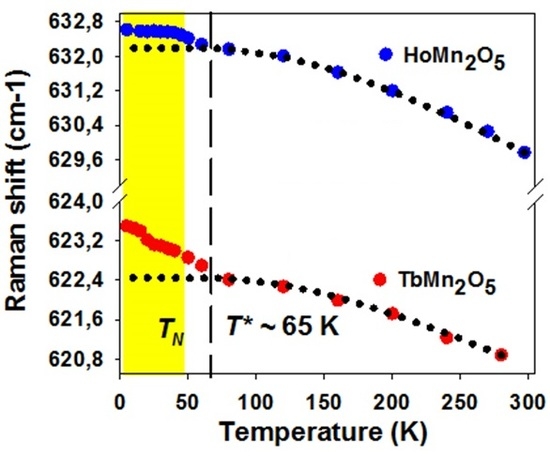
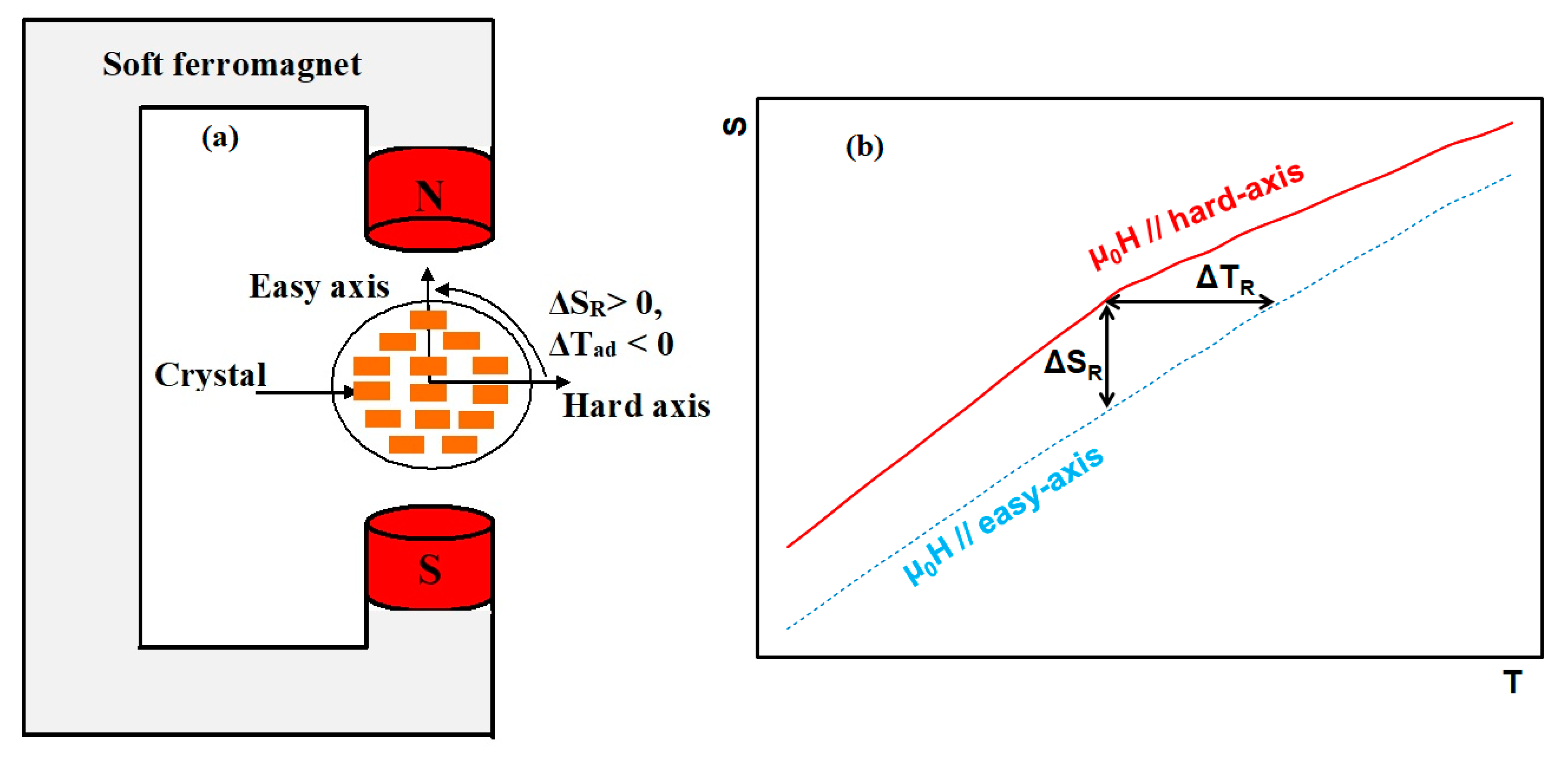
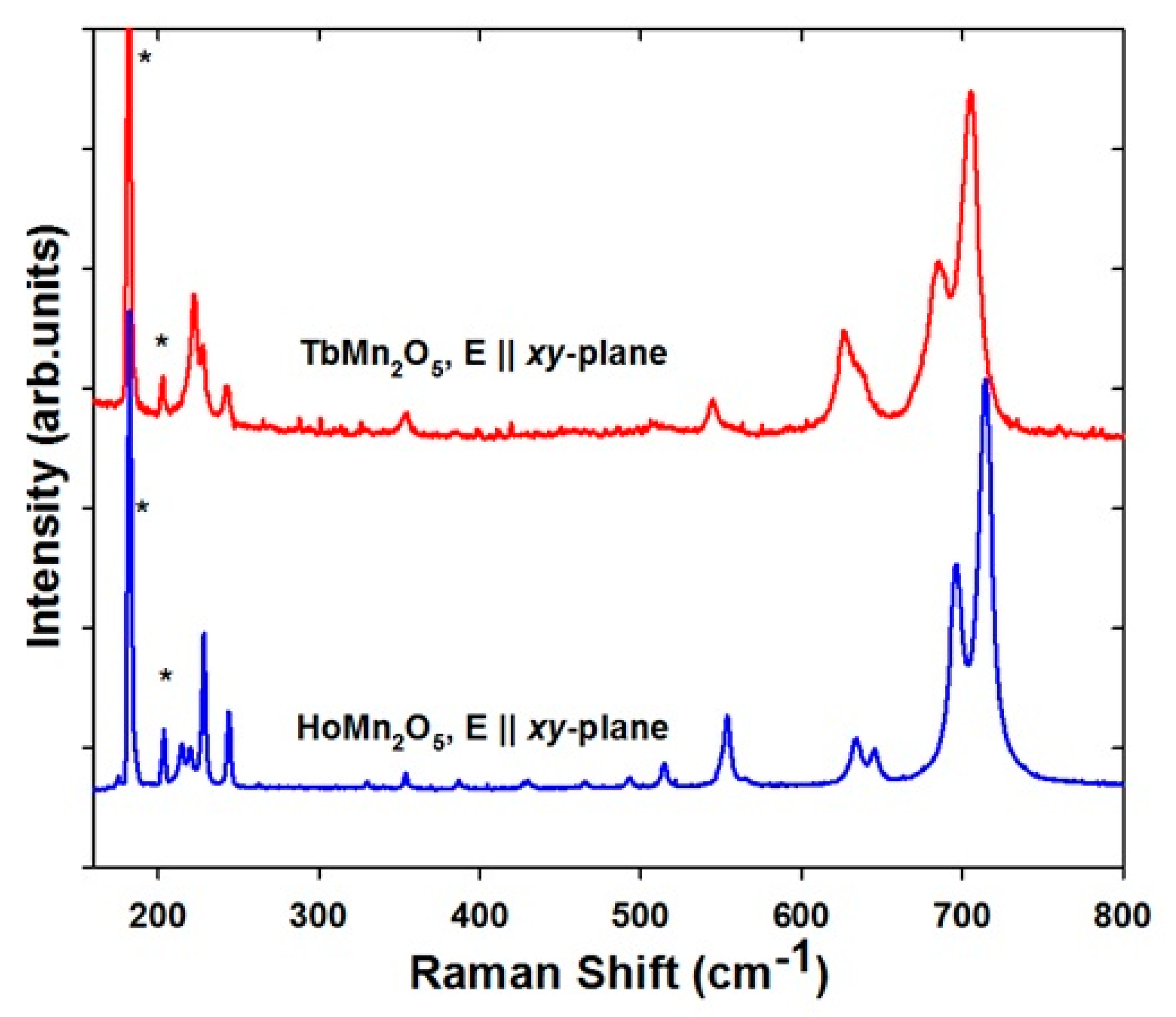
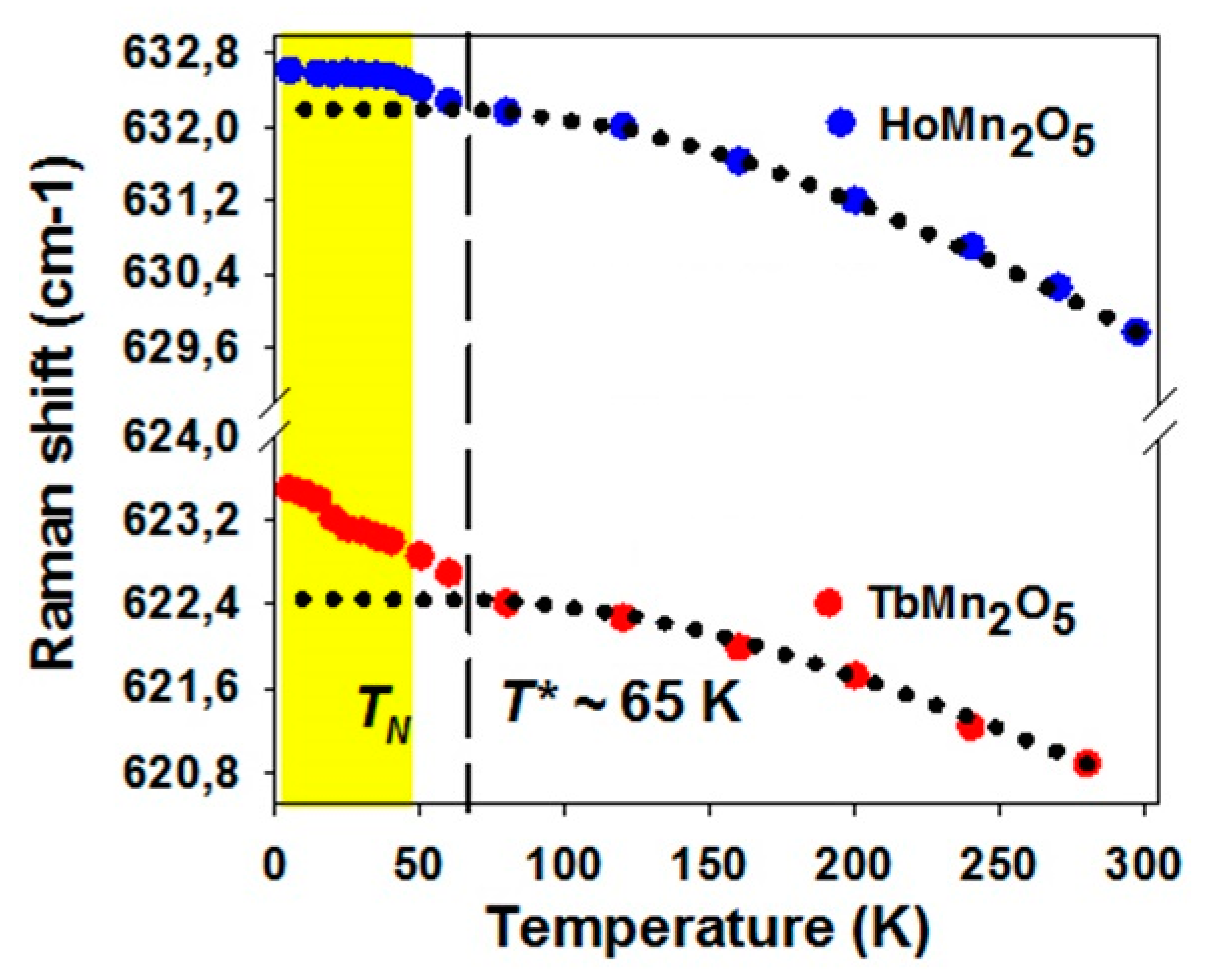
3. Distinguished Features of the RMCE in RMn2O5: Hypothesis
4. Materials and Methods
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Balli, M.; Jandl, S.; Fournier, P.; Kedous-Lebouc, A. Advanced materials for magnetic cooling: Fundamentals and practical aspects. Appl. Phys. Rev. 2017, 4, 021305. [Google Scholar] [CrossRef]
- Sari, O.; Balli, M. From conventional to magnetic refrigerator technology. Int. J. Refrig. 2014, 37, 8–15. [Google Scholar] [CrossRef]
- Balli, M.; Sari, O.; Zamni, L.; Mahmed, C.; Forchelet, J. Implementation of La (Fe, Co)13-xSix materials in magnetic refrigerators: Practical aspects. Mater. Sci. Eng. B 2012, 177, 629–634. [Google Scholar] [CrossRef]
- Moya, X.; Kar-Narayan, S.; Mathur, N.D. Caloric materials near ferroic phase transitions. Nat. Mater. 2014, 13, 439–450. [Google Scholar] [CrossRef] [PubMed]
- Pecharsky, V.K.; Gschneidner, K.A., Jr. Giant Magnetocaloric Effect in Gd5Ge2Si2. Phys. Rev. Lett. 1997, 78, 4494. [Google Scholar] [CrossRef]
- Wada, H.; Tanabe, Y. Giant magnetocaloric effect of MnAs1-xSbx. Appl. Phys. Lett. 2001, 79, 3302. [Google Scholar] [CrossRef]
- Balli, M.; Fruchart, D.; Gignoux, D.; Dupuis, C.; Kedous-Lebouc, A.; Zach, R. Giant magnetocaloric effect in Mn1-x(Ti0.5V0.5)xAs: Experiments and calculations. J. Appl. Phys. 2008, 103, 103908. [Google Scholar] [CrossRef]
- Balli, M.; Fruchart, D.; Gignoux, D.; Tobola, J.; Hlil, E.K.; Wolfers, P.; Zach, R. Magnetocaloric effect in ternary metal phosphides (Fe1-xNix)2 P. J. Magn. Magn. Mater. 2007, 316, 358–360. [Google Scholar] [CrossRef]
- Tegus, O.; Brück, E.; Buschow, K.H.J.; De Boer, F.R. Transition-metal-based magnetic refrigerants for room temperature applications. Nature 2002, 415, 150–152. [Google Scholar] [CrossRef] [PubMed]
- Fujita, A.; Fujieda, S.; Hasegawa, Y.; Fukamichi, K. Itinerant-electron metamagnetic transition and large magnetocaloric effects in La(FexSi1-x)13 compounds and their hydrides. Phys. Rev. B 2003, 67, 104416. [Google Scholar] [CrossRef]
- Hu, F.X.; Shen, B.G.; Sun, J.R.; Wang, G.J.; Cheng, Z.H. Very large magnetic entropy change near room temperature in LaFe11.2Co0.7Si1.1. Appl. Phys. Lett. 2002, 80, 826–828. [Google Scholar] [CrossRef]
- Balli, M.; Fruchart, D.; Gignoux, D. The LaFe11.2Co0.7Si1.1Cx carbides for magnetic refrigeration close to room temperature. Appl. Phys. Lett. 2008, 92, 232505. [Google Scholar] [CrossRef]
- Balli, M.; Fruchart, D.; Gignoux, D. Optimization of La (Fe, Co)13-xSix based compounds for magnetic refrigeration. J. Phys. Condens. Matter 2007, 19, 236230. [Google Scholar] [CrossRef]
- Matsumoto, K.; Kondo, T.; Yoshioka, S.; Kamiya, K.; Numazawa, T. Magnetic refrigerator for hydrogen liquefaction. J. Phys. Conf. Ser. 2009, 150, 012028. [Google Scholar] [CrossRef]
- Balli, M.; Jandl, S.; Fournier, P.; Gospodinov, M.M. Anisotropy-enhanced giant reversible rotating magnetocaloric effect in HoMn2O5 single crystals. Appl. Phys. Lett. 2014, 104, 232402. [Google Scholar] [CrossRef]
- Balli, M.; Jandl, S.; Fournier, P.; Dimitrov, D.Z. Giant rotating magnetocaloric effect at low magnetic fields in multiferroic TbMn2O5 single crystals. Appl. Phys. Lett. 2016, 108, 102401. [Google Scholar] [CrossRef]
- Jin, J.L.; Zhang, X.Q.; Li, G.K.; Cheng, Z.H.; Zheng, L.; Lu, Y. Giant anisotropy of magnetocaloric effect in TbMnO3 single crystals. Phys. Rev. B 2011, 83, 184431. [Google Scholar] [CrossRef]
- Balli, M.; Jandl, S.; Fournier, P.; Mansouri, S.; Mukhin, A.; Ivanov, Y.V.; Balbashov, A.M. On the magnetocaloric effect in the multiferroic hexagonal DyMnO3 single crystals. J. Magn. Magn. Mater. 2015, 374, 252–257. [Google Scholar] [CrossRef]
- Jin, J.L.; Zhang, X.Q.; Ge, H.; Cheng, Z.H. Rotating field entropy change in hexagonal TmMnO3 single crystal with anisotropic paramagnetic response. Phys. Rev. B 2012, 85, 214426. [Google Scholar] [CrossRef]
- Balli, M.; Mansouri, S.; Jandl, S.; Fournier, P.; Dimitrov, D.Z. Large rotating magnetocaloric effect in the orthorhombic DyMnO3 single crystal. Solid State Commun. 2016, 239, 9–13. [Google Scholar] [CrossRef]
- Li, L.; Namiki, T.; Huo, D.; Qian, Z.; Nishimura, K. Two successive magnetic transitions induced large refrigerant capacity in HoPdIn compound. Appl. Phys. Lett. 2013, 103, 222405. [Google Scholar] [CrossRef]
- Midya, A.; Das, S.N.; Mandal, P.; Pandya, S.; Ganesan, V. Anisotropic magnetic properties and giant magnetocaloric effect in antiferromagnetic RMnO3 crystals (R = Dy, Tb, Ho, and Yb). Phys. Rev. B 2011, 84, 235127. [Google Scholar] [CrossRef]
- Balli, M.; Roberge, B.; Vermette, J.; Jandl, S.; Fournier, P.; Gospodinov, M.M. Magnetocaloric properties of the hexagonal HoMnO3 single crystal revisited. Phys. B 2015, 478, 77–83. [Google Scholar] [CrossRef]
- Balli, M.; Roberge, B.; Jandl, S.; Fournier, P.; Palstra, T.T.M.; Nugroho, A.A. Observation of large refrigerant capacity in the HoVO3 vanadate single crystal. J. Appl. Phys. 2015, 118, 073903. [Google Scholar] [CrossRef]
- Noda, Y.; Kimura, H.; Fukunaga, M.; Kobayashi, S.; Kagomiya, I.; Kohn, K. Magnetic and ferroelectric properties of multiferroic RMn2O5. J. Phys. Condens. Matter 2008, 20, 434206. [Google Scholar] [CrossRef]
- Blake, G.R.; Chapon, L.C.; Radaelli, P.G.; Park, S.; Hur, N.; Cheong, S.W.; Rodriguez-Carvajal, J. Spin structure and magnetic frustration in multiferroic RMn2O5 (R = Tb, Ho, Dy). Phys. Rev. B 2005, 71, 214402. [Google Scholar] [CrossRef]
- Hur, N.; Park, S.; Sharma, P.A.; Ahn, J.S.; Guha, S.; Cheong, S.W. Electric polarization reversal and memory in a multiferroic material induced by magnetic fields. Nature 2004, 429, 392–395. [Google Scholar] [CrossRef] [PubMed]
- Hur, N.; Park, S.; Sharma, P.A.; Guha, S.; Cheong, S.W. Colossal Magnetodielectric Effects in DyMn2O5. Phys. Rev. Lett. 2004, 93, 107207. [Google Scholar] [CrossRef] [PubMed]
- Mihailova, B.; Gospodinov, M.M.; Güttler, B.; Yen, F.; Litvinchuk, A.P.; Iliev, M.N. Temperature-dependent Raman spectra of HoMn2O5 and TbMn2O5. Phys. Rev. B 2005, 71, 172301. [Google Scholar] [CrossRef]
- Nikitin, S.A.; Skokov, K.P.; Koshkid’ko, Y.S.; Pastushenkov, Y.G.; Ivanova, T.I. Giant rotating magnetocaloric effect in the region of spin-reorientation transition in the NdCo5 single crystal. Phys. Rev. Lett. 2010, 105, 137205. [Google Scholar] [CrossRef] [PubMed]
- Kuz’min, M.D.; Tishin, A.M. Magnetic refrigerants for the 4.2-20 K region: Garnets or perovskites? J. Phys. D Appl. Phys. 1991, 24, 2039. [Google Scholar] [CrossRef]
- Balli, M.; Fruchart, D.; Gignoux, D.; Zach, R. The “colossal” magnetocaloric effect in Mn1-xFexAs: What are we really measuring? Appl. Phys. Lett. 2009, 95, 072509. [Google Scholar] [CrossRef]
- Balli, M.; Sari, O.; Fruchart, D.; Forchelet, J. Influence of the materials magnetic state on the accurate determination of the magnetocaloric effect. Eur. Phys. J. Web Conf. 2012, 29, 00005. [Google Scholar] [CrossRef]
- Balli, M.; Sari, O.; Mahmed, C.; Besson, C.; Bonhote, P.; Duc, D.; Forchelet, J. A pre-industrial magnetic cooling system for room temperature application. Appl. Energy 2012, 98, 556–561. [Google Scholar] [CrossRef]
- Sari, O.; Balli, M.; Trottet, G.; Bonhote, P.; Egolf, P.W.; Muller, C.; Heitzler, J.C.; Bour, S. Initial results of a test-bed magnetic refrigeration machine with practical running conditions. In Proceedings of the 3rd International Conference on Magnetic Refrigeration at Room Temperature, Des Moines, IA, USA, 11–15 May 2009; pp. 371–380. [Google Scholar]
- Goodenough, J.B. Theory of the Role of Covalence in the Perovskite-Type Manganites [La, M(II)]MnO3. Phys. Rev. 1955, 100, 564. [Google Scholar] [CrossRef]
- Kanamori, J. Superexchange interaction and symmetry properties of electron orbitals. J. Phys. Chem. Solids 1959, 10, 87–98. [Google Scholar] [CrossRef]
- Anderson, P.W. Theory of magnetic exchange interactions: Exchange in insulators and semiconductors. Solid State Phys. 1963, 14, 99–214. [Google Scholar]
- Garcia-Flores, A.F.; Granado, E.; Martinho, H.; Urbano, R.R.; Rettori, C.; Golovenchits, E.I.; Sanina, V.A.; Oseroff, S.B.; Park, S.; Cheong, S.-W. Anomalous phonon shifts in the paramagnetic phase of multiferroic RMn2O5 (R = Bi, Eu, Dy): Possible manifestations of unconventional magnetic correlations. Phys. Rev. B 2006, 73, 104411. [Google Scholar] [CrossRef]
- Chapon, L.C.; Blake, G.R.; Gutmann, M.J.; Park, S.; Hur, N.; Radaelli, P.G.; Cheong, S.W. Structural Anomalies and Multiferroic Behavior in Magnetically Frustrated TbMn2O5. Phys. Rev. Lett. 2004, 93, 177402. [Google Scholar] [CrossRef] [PubMed]
- Granado, E.; Eleotério, M.S.; García-Flores, A.F.; Souza, J.A.; Golovenchits, E.I.; Sanina, V.A. Magnetoelastic and thermal effects in the BiMn2O5 lattice: A high-resolution x-ray diffraction study. Phys. Rev. B 2008, 77, 134101. [Google Scholar] [CrossRef]
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balli, M.; Mansouri, S.; Jandl, S.; Fournier, P.; Dimitrov, D.Z. Analysis of the Anisotropic Magnetocaloric Effect in RMn2O5 Single Crystals. Magnetochemistry 2017, 3, 36. https://doi.org/10.3390/magnetochemistry3040036
Balli M, Mansouri S, Jandl S, Fournier P, Dimitrov DZ. Analysis of the Anisotropic Magnetocaloric Effect in RMn2O5 Single Crystals. Magnetochemistry. 2017; 3(4):36. https://doi.org/10.3390/magnetochemistry3040036
Chicago/Turabian StyleBalli, Mohamed, Saber Mansouri, Serge Jandl, Patrick Fournier, and Dimitre Z. Dimitrov. 2017. "Analysis of the Anisotropic Magnetocaloric Effect in RMn2O5 Single Crystals" Magnetochemistry 3, no. 4: 36. https://doi.org/10.3390/magnetochemistry3040036
APA StyleBalli, M., Mansouri, S., Jandl, S., Fournier, P., & Dimitrov, D. Z. (2017). Analysis of the Anisotropic Magnetocaloric Effect in RMn2O5 Single Crystals. Magnetochemistry, 3(4), 36. https://doi.org/10.3390/magnetochemistry3040036