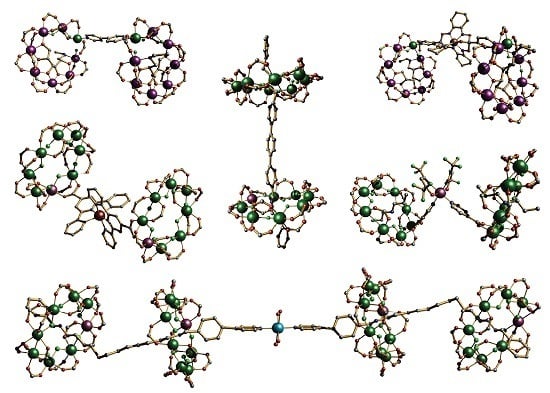
Cr7Ni Wheels: Supramolecular Tectons for the Physical Implementation of Quantum Information Processing
Abstract
:1. Introduction: Molecules as Qubits
2. Individual {Cr7Ni} Wheels as Single Qubits
3. Dimeric Assemblies of {Cr7Ni} Wheels as Double Qubit-Based Quantum Gates
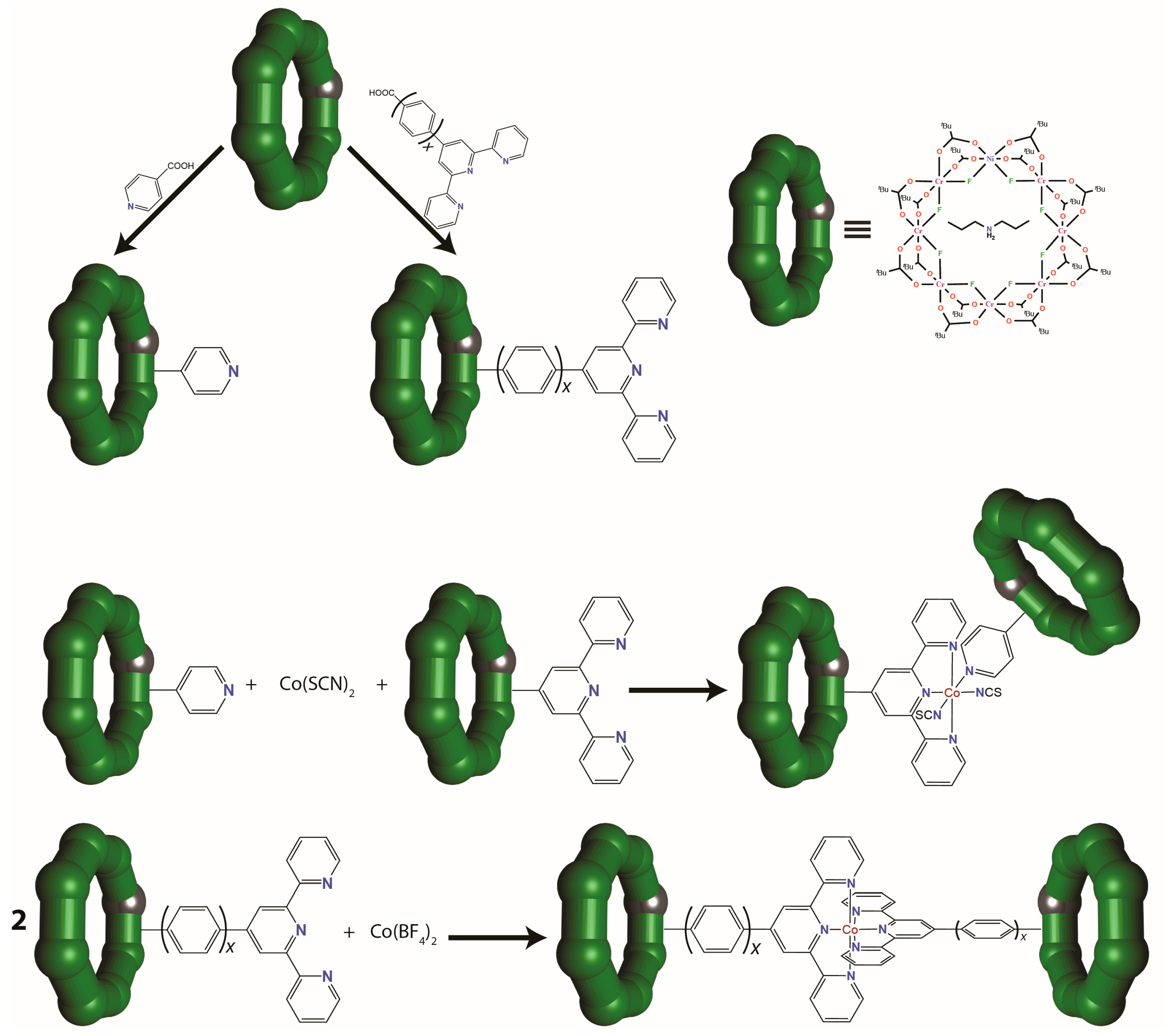
3.1. {Cr7Ni} Wheels with Open Metal Sites as Metal Complexes
3.2. {Cr7Ni} Wheels as Metalloligands
- CNOT: ; ; ;
- SWAP: ;
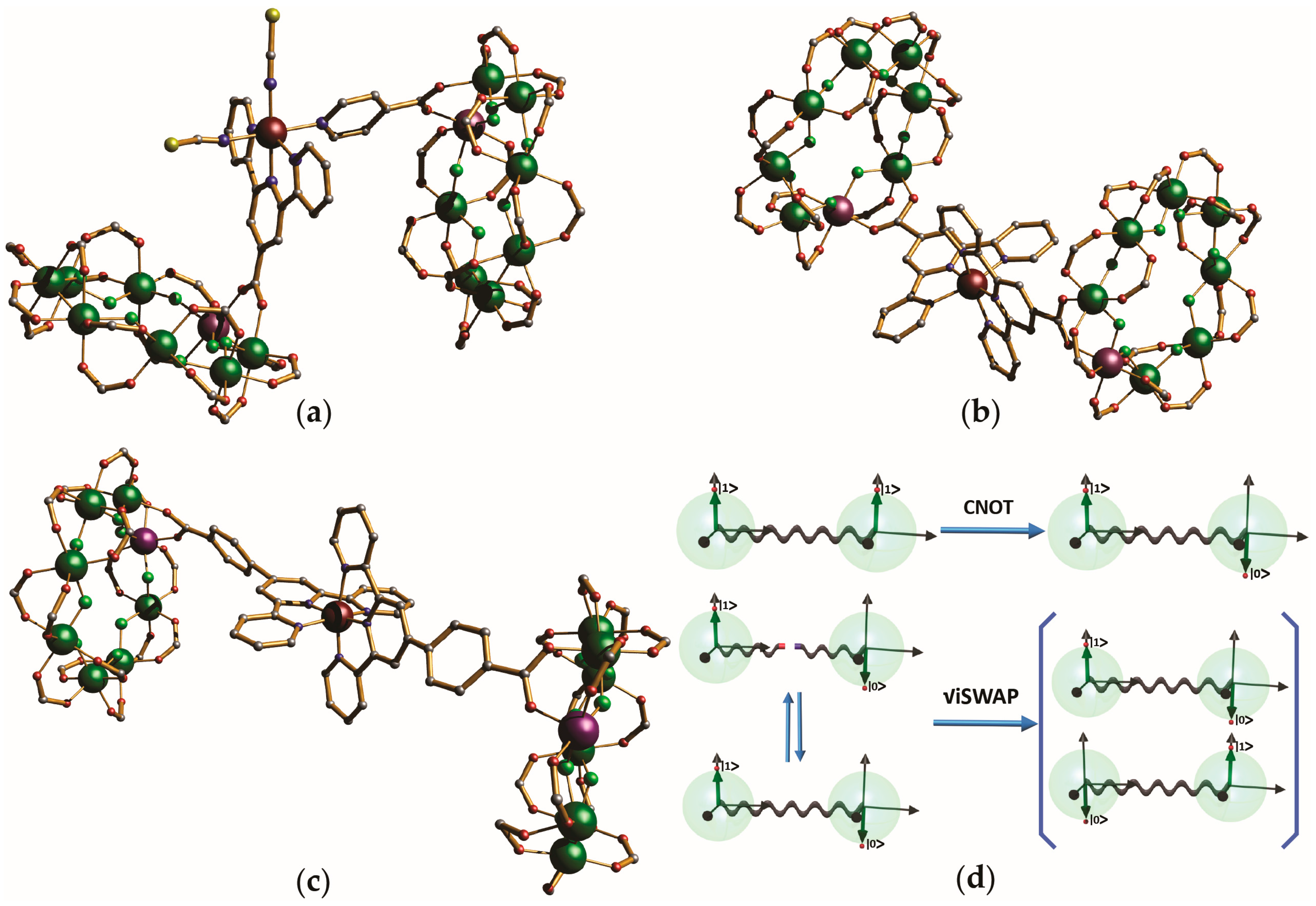
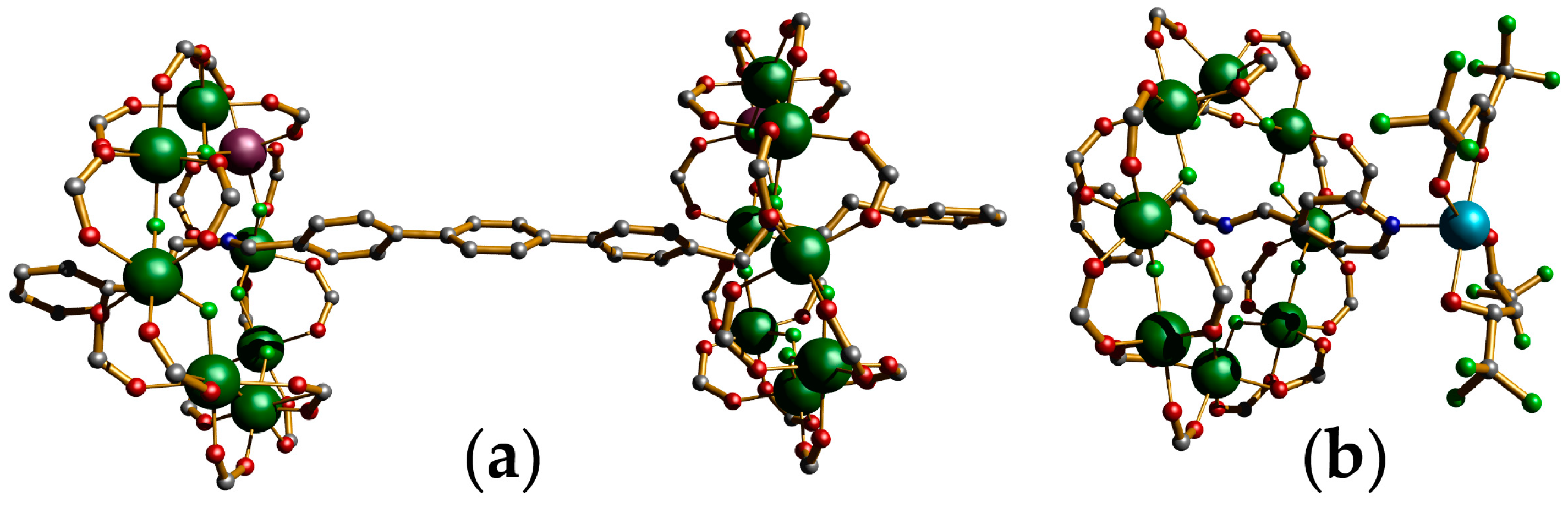
3.3. {Cr7Ni} Wheels as Inorganic Macrocyclic Subunits of Hybrid Rotaxanes
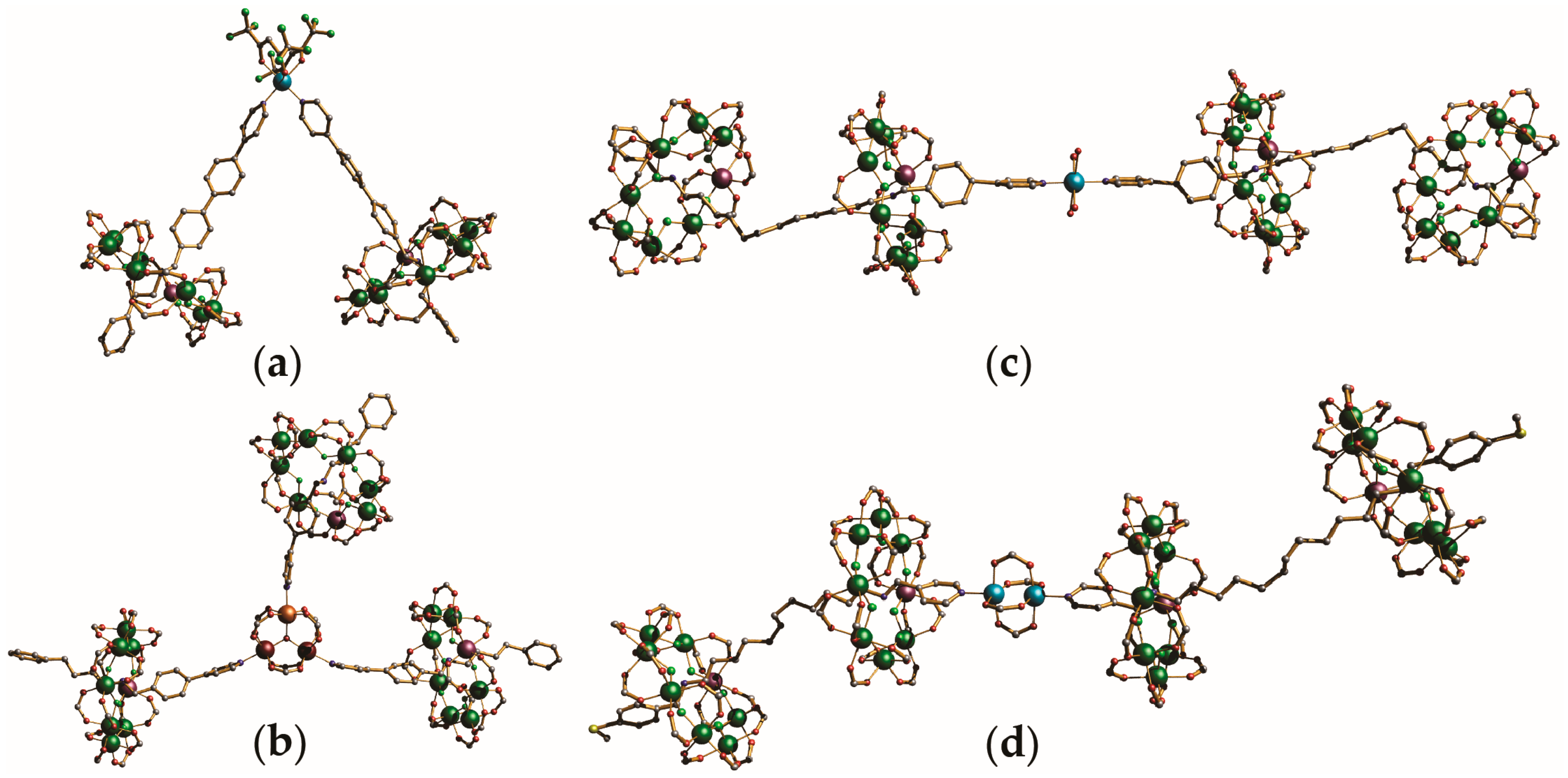
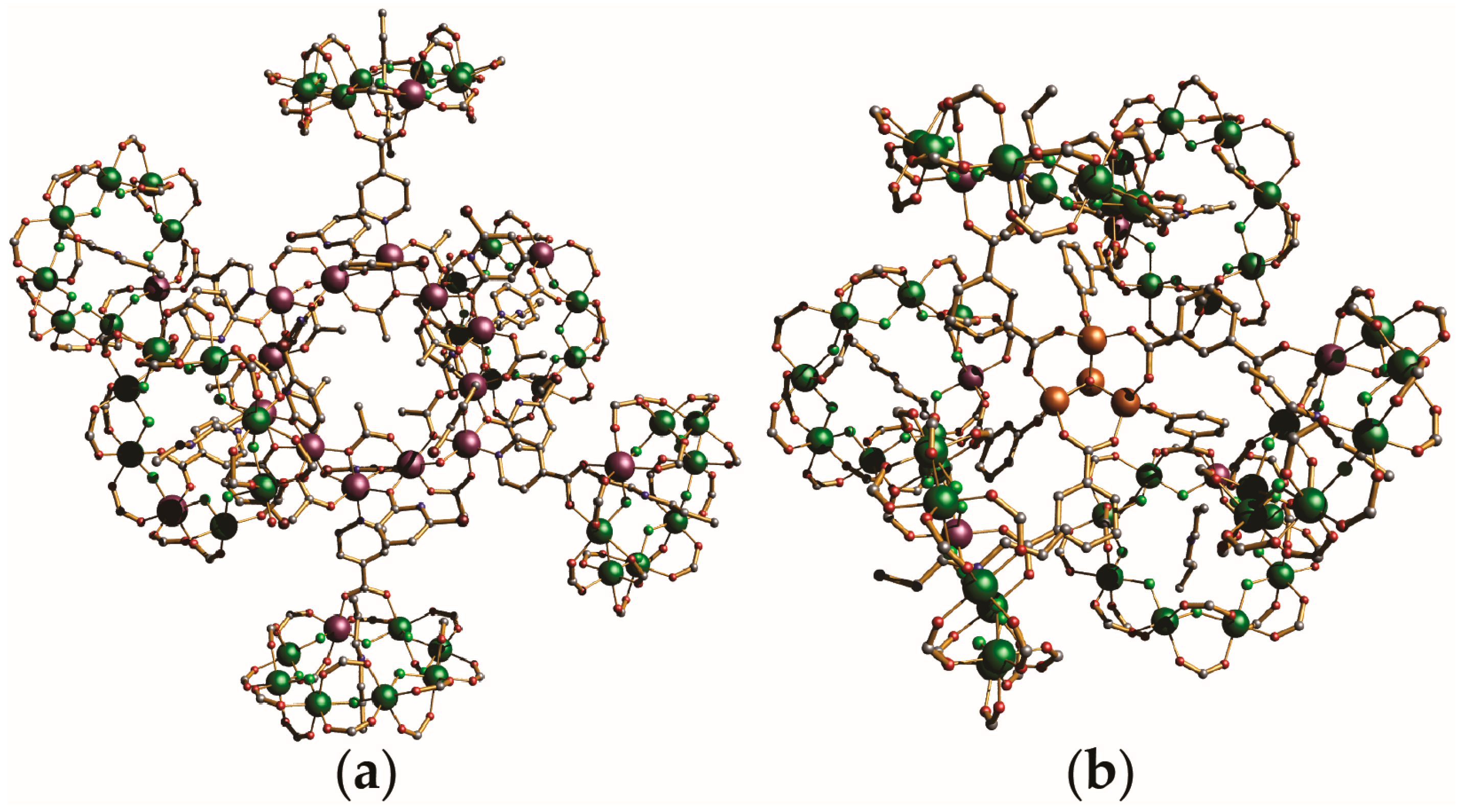
4. Oligomeric Arrays of {Cr7Ni} Wheels as Multiple Qubits
5. Conclusions and Perspectives
Acknowledgments
Conflicts of Interest
References
- Feynman, R.P. Simulating physics with computers. Int. J. Theor. Phys. 1982, 21, 467–488. [Google Scholar] [CrossRef]
- Lloyd, S. Universal quantum simulators. Science 1996, 273, 1073–1078. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: New York, NY, USA, 2000. [Google Scholar]
- Ladd, T.D.; Jelezko, F.; Laflamme, R.; Nakamura, Y.; Monroe, C.; O’Brien, J.L. Quantum computers. Nature 2010, 464, 45–53. [Google Scholar] [CrossRef] [PubMed]
- Shor, P.W. Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM J. Comput. 1997, 26, 1484–1509. [Google Scholar] [CrossRef]
- Grover, L.K. Quantum computers can search arbitrarily large databases by a single query. Phys. Rev. Lett. 1997, 79, 4709–4712. [Google Scholar] [CrossRef]
- Leuenberger, M.; Loss, D. Simulating physics with computers. Nature 2001, 410, 789–793. [Google Scholar] [CrossRef] [PubMed]
- Meier, F.; Levy, J.; Loss, D. Quantum computing with spin cluster qubits. Phys. Rev. Lett. 2003, 90, 47901–47904. [Google Scholar] [CrossRef] [PubMed]
- Troiani, F.; Affronte, M.; Carretta, S.; Santini, P.; Amoretti, G. Proposal for quantum gates in permanently coupled antiferromagnetic spin rings without need of local fields. Phys. Rev. Lett. 2005, 94, 190501. [Google Scholar] [CrossRef] [PubMed]
- Lehmann, J.; Gaita-Ariño, A.; Coronado, E.; Loss, D. Spin qubits with electrically gated polyoxometalate molecules. Nat. Nanotechnol. 2007, 2, 312–317. [Google Scholar] [CrossRef] [PubMed]
- Ardavan, A.; Rival, O.; Morton, J.J.L.; Blundell, S.J.; Tyryshkin, A.M.; Timco, G.A.; Winpenny, R.E.P. Will spin-relaxation times in molecular magnets permit quantum information processing? Phys. Rev. Lett. 2007, 98, 057201. [Google Scholar] [CrossRef] [PubMed]
- Kitagawa, M.; Nakasuji, K.; Takui, T. Triple-stranded metallo-helicates addressable as Lloyd’s electron spin qubits. J. Am. Chem. Soc. 2010, 132, 6944–6946. [Google Scholar]
- Nakazawa, S.; Nishida, S.; Ise, T.; Yoshino, T.; Mori, N.R.; Rahimi, D.; Sato, K.; Morita, Y.; Toyota, K.; Shiomi, D.; et al. A synthetic two-spin quantum bit: g-engineered exchange-coupled biradical designed for controlled-NOT gate operations. Angew. Chem. Int. Ed. 2012, 51, 9860–9864. [Google Scholar] [CrossRef] [PubMed]
- Wedge, C.J.; Timco, G.A.; Spielberg, E.T.; George, R.E.; Tuna, F.; Rigby, S.; McInnes, E.J.L.; Winpenny, R.E.P.; Blundell, S.J.; Ardavan, A. Chemical engineering of molecular qubits. Phys. Rev. Lett. 2012, 108, 107204. [Google Scholar] [CrossRef] [PubMed]
- Aromí, G.; Aguilà, D.; Gamez, P.; Luis, F.; Roubeau, O. Design of magnetic coordination complexes for quantum computing. Chem. Soc. Rev. 2012, 41, 537–546. [Google Scholar]
- Warner, M.; Din, S.; Tupitsyn, I.S.; Morley, G.W.; Stoneham, A.; Gardener, J.A.; Wu, Z.; Fisher, A.J.; Heutz, S.; Kay, C.W.M.; et al. Potential for spin-based information processing in a thin-film molecular semiconductor. Nature 2013, 503, 504–508. [Google Scholar] [CrossRef] [PubMed]
- Graham, M.J.; Zadrozny, J.M.; Shiddiq, M.; Anderson, J.S.; Fataftah, M.S.; Hill, S.; Freedman, D.E. Influence of electronic spin and spin–orbit coupling on decoherence in mononuclear transition metal complexes. J. Am. Chem. Soc. 2014, 136, 7623–7626. [Google Scholar] [CrossRef] [PubMed]
- Bader, K.; Dengler, D.; Lenz, S.; Endeward, B.; Jiang, S.D.; Neugebauer, P.; Slageren, J. Room temperature quantum coherence in a potential molecular qubit. Nat. Commun. 2014, 5, 5304. [Google Scholar] [CrossRef] [PubMed]
- Aguilà, D.; Barrios, L.A.; Velasco, V.; Roubeau, O.; Repollés, A.; Alonso, P.J.; Sesé, J.; Teat, S.J.; Luis, F.; Aromí, G. Heterodimetallic [LnLn′] Lanthanide complexes: Toward a chemical design of two-qubit molecular spin quantum gates. J. Am. Chem. Soc. 2014, 136, 14215–14222. [Google Scholar] [CrossRef] [PubMed]
- Walsh, J.P.S.; Meadows, S.B.; Ghirri, A.; Moro, F.; Jennings, M.; Smith, W.F.; Graham, D.M.; Kihara, T.; Nojiri, H.; Vitorica-Yrezabal, I.; et al. Electronic structure of a mixed-metal fluoride-centered triangle complex: A potential qubit component. Inorg. Chem. 2015, 54, 12019–12026. [Google Scholar] [CrossRef] [PubMed]
- Zadrozny, J.M.; Niklas, J.; Poluektov, O.G.; Freedman, D.E. Millisecond coherence time in a tunable molecular electronic spin qubit. ACS Cent. Sci. 2015, 1, 488–492. [Google Scholar] [CrossRef] [PubMed]
- Atzori, M.; Tesi, L.; Morra, E.; Chiesa, M.; Sorace, L.; Sessoli, R. Room-temperature quantum coherence and rabi oscillations in vanadyl phthalocyanine: Toward multifunctional molecular spin qubits. J. Am. Chem. Soc. 2016, 138, 2154–2157. [Google Scholar] [CrossRef] [PubMed]
- Pedersen, K.S.; Ariciu, A.M.; McAdams, S.; Weihe, H.; Bendix, J.; Tuna, F.; Piligkos, S. Toward molecular 4f single-ion magnet qubits. J. Am. Chem. Soc. 2016, 138, 5801–5804. [Google Scholar] [CrossRef] [PubMed]
- Shiddiq, M.; Komijani, D.; Duan, Y.; Gaita-Ariño, A.; Coronado, E.; Hill, S. Enhancing coherence in molecular spin qubits via atomic clock transitions. Nature 2016, 531, 348–351. [Google Scholar] [CrossRef] [PubMed]
- Burkard, G.; Loss, D.; DiVincenzo, D.P. Coupled quantum dots as quantum gates. Phys. Rev. B 1999, 59, 2070–2078. [Google Scholar] [CrossRef]
- Hanson, R.; Awschalom, D.D. Coherent manipulation of single spins in semiconductors. Nature 2008, 453, 1043–1049. [Google Scholar] [CrossRef] [PubMed]
- Maurer, P.C.; Kucsko, G.; Latta, C.; Jiang, L.; Yao, N.Y.; Bennett, S.D.; Pastawski, F.; Hunger, D.; Chisholm, N.; Markham, M.; et al. Room-temperature quantum bit memory exceeding one second. Science 2012, 336, 1283–1286. [Google Scholar] [CrossRef] [PubMed]
- Knowles, H.S.; Kara, D.M.; Atatüre, M. Observing bulk diamond spin coherence in high-purity nanodiamonds. Nat. Mater. 2014, 13, 21–25. [Google Scholar] [CrossRef] [PubMed]
- Georgescu, I.M.; Ashhab, S.; Nori, F. Quantum simulation. Rev. Mod. Phys. 2014, 86, 153–185. [Google Scholar] [CrossRef]
- Lehn, J.M. Supramolecular Chemistry: Concepts and Perspectives; Wiley-VCH: Weinheim, Germany, 1995. [Google Scholar]
- Fujita, M.; Tominaga, M.; Hori, A.; Therrien, B. Coordination assemblies from a Pd(II)-cornered square complex. Acc. Chem. Res. 2005, 38, 371–380. [Google Scholar] [CrossRef] [PubMed]
- Pluth, M.D.; Bergman, R.G.; Raymond, K.N. Supermolecules by design. Acc. Chem. Res. 2009, 42, 1650–1659. [Google Scholar] [CrossRef] [PubMed]
- Chakrabarty, R.; Mukherjee, P.S.; Stang, P.J. Supramolecular coordination: Self-assembly of finite two- and three-dimensional ensembles. Chem. Rev. 2011, 111, 6810–6918. [Google Scholar] [CrossRef] [PubMed]
- Ward, M.D.; Raithby, P.R. Functional behaviour from controlled self-assembly: Challenges and prospects. Chem. Soc. Rev. 2013, 42, 1619–1636. [Google Scholar] [CrossRef] [PubMed]
- Schweiger, A.; Jeschke, G. Principles of Pulse Electron Paramagnetic Resonance; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Affonte, M.; Carretta, S.; Timco, G.A.; Winpenny, R.E.P. A ring cycle: Studies of heterometallic wheels. Chem. Commun. 2007, 1789–1797. [Google Scholar] [CrossRef] [PubMed]
- Timco, G.A.; Faust, T.B.; Tuna, F.; Winpenny, R.E.P. Linking heterometallic rings for quantum information processing and amusement. Chem. Soc. Rev. 2011, 40, 3067–3075. [Google Scholar] [CrossRef] [PubMed]
- McInnes, E.J.L.; Timco, G.A.; Whitehead, G.F.S.; Winpenny, R.E.P. Heterometallic rings: Their physics and use as supramolecular building blocks. Angew. Chem. Int. Ed. 2015, 54, 14244–14269. [Google Scholar] [CrossRef] [PubMed]
- Candini, A.; Lorusso, G.; Troiani, F.; Ghirri, A.; Carretta, S.; Santini, P.; Amoretti, G.; Muryn, C.; Tuna, F.; Timco, G.; et al. Entanglement in supramolecular spin systems of two weakly coupled antiferromagnetic rings (Purple-Cr7Ni). Phys. Rev. Lett. 2010, 104, 037203. [Google Scholar] [CrossRef] [PubMed]
- Kaminski, D.; Webber, A.L.; Wedge, C.J.; Liu, J.; Timco, G.A.; Vitorica-Yrezabal, I.J.; McInnes, E.J.L.; Winpenny, R.E.P.; Ardavan, A. Quantum spin coherence in halogen-modified Cr7Ni molecular nanomagnets. Phys. Rev. B 2014, 90, 184419. [Google Scholar] [CrossRef]
- Ardavan, A.; Bowen, A.; Fernandez, A.; Fielding, A.; Kaminski, D.; Moro, F.; Muryn, C.A.; Wise, M.D.; Ruggi, A.; McInnes, E.J.L.; et al. Engineering coherent interactions in molecular nanomagnet dimers. NPJ Quantum Inf. 2015, 1, 15012. [Google Scholar] [CrossRef]
- Timco, G.A.; McInnes, E.J.L.; Pritchard, R.G.; Tuna, F.; Winpenny, R.E.P. Heterometallic rings made from chromium stick together easily. Angew. Chem. Int. Ed. 2008, 47, 9681–9684. [Google Scholar] [CrossRef] [PubMed]
- Faust, T.B.; Bellini, V.; Candini, A.; Carretta, S.; Lorusso, G.; Allan, D.R.; Carthy, L.; Collison, D.; Docherty, R.J.; Kenyon, J.; et al. Chemical control of spin propagation between heterometallic rings. Chem. Eur. J. 2011, 17, 14020–14030. [Google Scholar] [CrossRef] [PubMed]
- Bellini, V.; Lorusso, G.; Candini, A.; Wernsdorfer, W.; Faust, T.B.; Timco, G.A.; Winpenny, R.E.P.; Affronte, M. Propagation of spin information at the supramolecular scale through heteroaromatic linkers. Phys. Rev. Lett. 2011, 106, 227205. [Google Scholar] [CrossRef] [PubMed]
- Faust, T.B.; Tuna, F.; Timco, G.A.; Affronte, M.; Bellini, V.; Wernsdorfer, W.; Winpenny, R.E.P. Controlling magnetic communication through aromatic bridges by variation in torsion angle. Dalton Trans. 2012, 41, 13626–13631. [Google Scholar] [CrossRef] [PubMed]
- Timco, G.A.; Carretta, S.; Troiani, F.; Tuna, F.; Pritchard, R.J.; Muryn, C.A.; McInnes, E.J.L.; Ghirri, A.; Candini, A.; Santini, P.; et al. Engineering the coupling between molecular spin qubits by coordination chemistry. Nat. Nanotechnol. 2008, 4, 173–178. [Google Scholar] [CrossRef] [PubMed]
- Sessoli, R.; Tsai, H.L.; Schake, A.R.; Wang, S.; Vincent, J.B.; Folting, K.; Gatteschi, D.; Christou, G.; Hendrickson, D.N. High-spin molecules: [Mn12O12(O2CR)16(H2O)4]. J. Am. Chem. Soc. 1993, 115, 1804–1816. [Google Scholar] [CrossRef]
- Soler, M.; Artus, P.; Folting, K.; Huffman, J.C.; Hendrickson, D.N.; Christou, G. Single-molecule magnets: Preparation and properties of mixed-carboxylate complexes [Mn12O12(O2CR)8(O2CR′)8(H2O)4]. Inorg. Chem. 2001, 40, 4902–4912. [Google Scholar] [CrossRef] [PubMed]
- Whitehead, G.F.S.; Moro, F.; Timco, G.A.; Wernsdorfer, W.; Teat, S.J.; Winpenny, R.E.P. A ring of rings and other multicomponent assemblies of cages. Angew. Chem. Int. Ed. 2013, 52, 9932–9935. [Google Scholar] [CrossRef] [PubMed]
- Chiesa, A.; Whitehead, G.F.S.; Carretta, S.; Timco, G.A.; Carthy, L.; Teat, S.J.; Amoretti, G.; Pavarini, E.; Winpenny, R.E.P.; Santini, P. Molecular nanomagnets with switchable coupling for quantum simulation. NPG Sci. Rep. 2014, 4, 7423. [Google Scholar] [CrossRef] [PubMed]
- Whitehead, G.F.S.; Ferrando-Soria, J.; Carthy, L.; Pritchard, R.G.; Teat, S.J.; Timco, G.A.; Winpenny, R.E.P. Synthesis and reactions of N-heterocycle functionalised variants of heterometallic {Cr7Ni} rings. Dalton Trans. 2016, 45, 1638–1647. [Google Scholar] [CrossRef] [PubMed]
- Ferrando-Soria, J.; Pineda, E.M.; Chiesa, A.; Fernandez, A.; Magee, S.A.; Carretta, S.; Santini, P.; Vitorica-Yrezabal, I.J.; Tuna, F.; Timco, G.A.; et al. A modular design of molecular qubits to implement universal quantum gates. Nat. Commun. 2016, 7, 11377. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.-F.; Leigh, D.A.; Pritchard, R.G.; Schultz, D.; Teat, S.J.; Timco, G.A.; Winpenny, R.E.P. Hybrid organic-inorganic rotaxanes and molecular shuttles. Nature 2009, 458, 314–318. [Google Scholar] [CrossRef] [PubMed]
- Ballesteros, B.; Faust, T.B.; Lee, C.-F.; Leigh, D.A.; Muryn, C.A.; Pritchard, R.G.; Schultz, D.; Teat, S.J.; Timco, G.A.; Winpenny, R.E.P. Synthesis, structure and dynamic properties of hybrid organic-inorganic rotaxanes. J. Am. Chem. Soc. 2010, 132, 15435–15444. [Google Scholar] [CrossRef] [PubMed]
- Whitehead, G.F.S.; Cross, B.; Carthy, L.; Milway, V.A.; Rath, H.; Fernandez, A.; Heath, S.L.; Muryn, C.A.; Pritchard, R.G.; Teat, S.J.; et al. Rings and threads as linkers in metal-organic frameworks and poly-rotaxanes. Chem. Commun. 2013, 49, 7195–7197. [Google Scholar] [CrossRef] [PubMed]
- Fernandez, A.; Moreno Pineda, E.; Muryn, C.A.; Sproules, S.; Moro, F.; Timco, G.A.; McInnes, E.J.L.; Winpenny, R.E.P. g-Engineering in hybrid rotaxanes to create AB and AB2 electron spin systems: EPR spectroscopic studies of weak interactions between dissimilar electron spin qubits. Angew. Chem. Int. Ed. 2015, 54, 10858–10861. [Google Scholar] [CrossRef] [PubMed]
- Fernandez, A.; Ferrando-Soria, J.; Pineda, E.M.; Tuna, F.; Vitorica-Yrezabal, I.J.; Knappke, C.; Ujma, J.; Muryn, C.A.; Timco, G.A.; Barran, P.E.; et al. Making hybrid [n]-rotaxanes as supramolecular arrays of molecular electron spin qubits. Nat. Commun. 2016, 7, 10240. [Google Scholar] [CrossRef] [PubMed]
- Whitehead, G.F.S.; Ferrando-Soria, J.; Christie, L.G.; Chilton, N.F.; Timco, G.A.; Moro, F.; Winpenny, R.E.P. The acid test: The chemistry of carboxylic acid functionalised {Cr7Ni} rings. Chem. Sci. 2014, 5, 235–239. [Google Scholar] [CrossRef]
- Ferrando-Soria, J.; Fernandez, A.; Pineda, E.M.; Varey, S.A.; Adams, R.W.; Vitorica-Yrezabal, I.J.; Tuna, F.; Timco, G.A.; Muryn, C.A.; Winpenny, R.E.P. Controlled synthesis of nanoscopic metal cages. J. Am. Chem. Soc. 2015, 137, 7644–7647. [Google Scholar] [CrossRef] [PubMed]
- Bader, K.; Winkler, M.; van Slageren, J. Tuning of molecular qubits: Very long coherence and spin-lattice relaxation times. Chem. Commun. 2016, 52, 3623–3626. [Google Scholar] [CrossRef] [PubMed]
- Atzori, M.; Morra, E.; Tesi, L.; Albino, A.; Chiesa, M.; Sorace, L.; Sessoli, R. Quantum coherence times enhancement in vanadium(IV)-based potential molecular qubits: The key role of the vanadyl moiety. J. Am. Chem. Soc. 2016, 138, 11234–11244. [Google Scholar] [CrossRef] [PubMed]
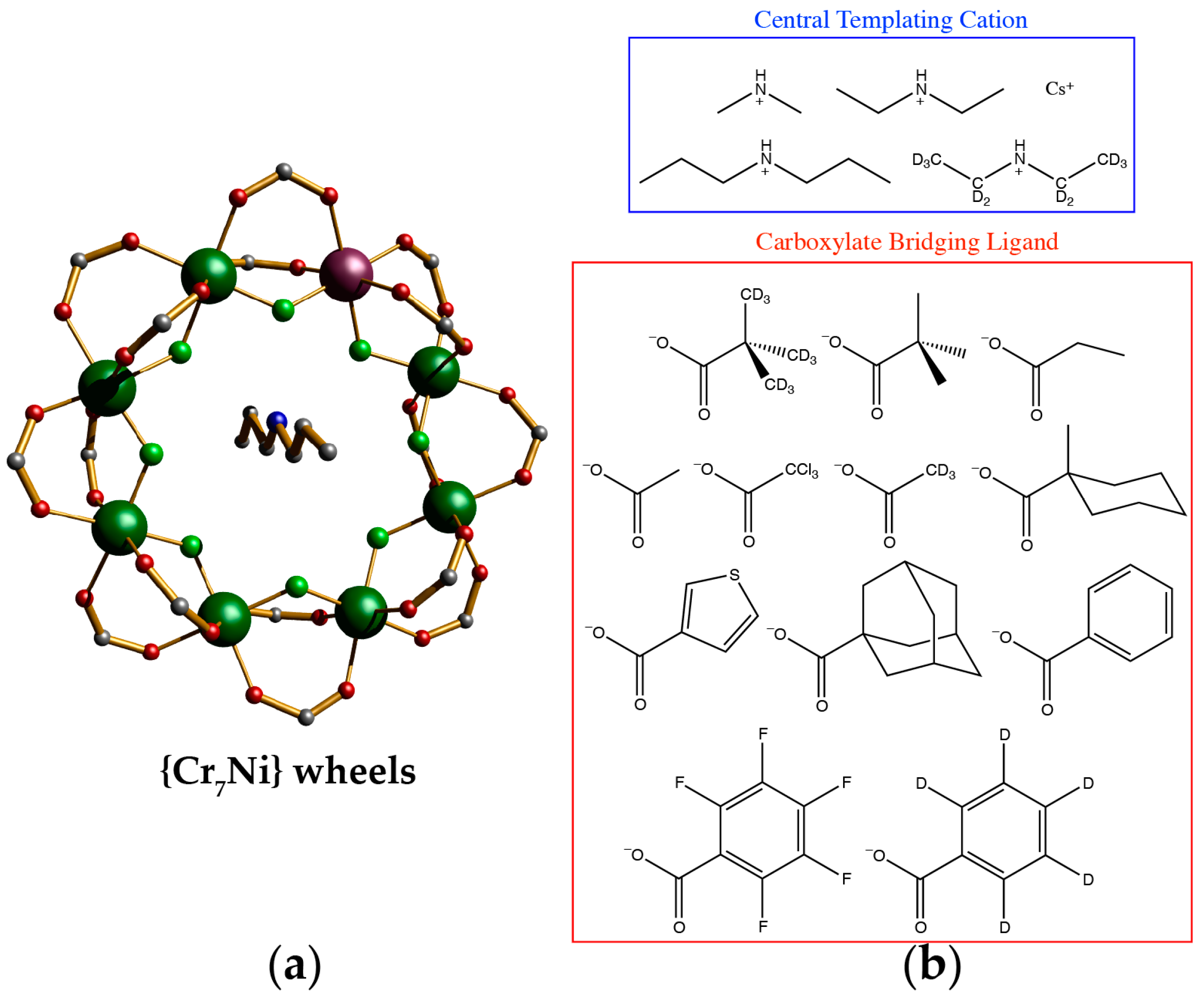




| Compound a | T/K | TM b/μs |
|---|---|---|
| Me2NH2[CrIII7NiIIF8(piv)16] c | 5.0 | 0.38 |
| 1.8 | 0.55 | |
| d-Me2NH2[CrIII7NiIIF8(d-piv)16] c | 1.8 | 3.8 |
| Et2NH2[CrIII7NiIIF8(piv)16] c | 5.0 | 0.73 |
| Et2NH2[CrIII7NiIIF8(d-piv)16] c | 5.0 | 0.93 |
| d-Et2NH2[CrIII7NiIIF8(d-piv)16] c | 5.0 | 0.93 |
| Pr2NH2[CrIII7NiIIF8(piv)16] c | 5.0 | 0.62 |
| Cs[CrIII7NiIIF8(piv)16] c | 5.0 | 0.74 |
| Cs[CrIII7NiIIF8(d-piv)16] c | 5.0 | 0.89 |
| Cs[CrIII7NiIIF8(d-piv)16] d | 5.0 | 15.3 |
| Pr2NH2[CrIII7NiIIF8(prop)16] c | 5.0 | 0.34 |
| Pr2NH2[CrIII7NiIIF8(ac)16] c | 5.0 | 0.44 |
| Pr2NH2[CrIII7NiIIF8(bz)16] d | 5.0 | 1.0 |
| Pr2NH2[CrIII7NiIIF8(d-bz)16] d | 5.0 | 0.90 |
| Pr2NH2[CrIII7NiIIF8(pfbz)16] d | 5.0 | 0.40 |
© 2016 by the author; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferrando-Soria, J. Cr7Ni Wheels: Supramolecular Tectons for the Physical Implementation of Quantum Information Processing. Magnetochemistry 2016, 2, 36. https://doi.org/10.3390/magnetochemistry2030036
Ferrando-Soria J. Cr7Ni Wheels: Supramolecular Tectons for the Physical Implementation of Quantum Information Processing. Magnetochemistry. 2016; 2(3):36. https://doi.org/10.3390/magnetochemistry2030036
Chicago/Turabian StyleFerrando-Soria, Jesus. 2016. "Cr7Ni Wheels: Supramolecular Tectons for the Physical Implementation of Quantum Information Processing" Magnetochemistry 2, no. 3: 36. https://doi.org/10.3390/magnetochemistry2030036
APA StyleFerrando-Soria, J. (2016). Cr7Ni Wheels: Supramolecular Tectons for the Physical Implementation of Quantum Information Processing. Magnetochemistry, 2(3), 36. https://doi.org/10.3390/magnetochemistry2030036