Wideband Radar Absorbing Structure Using Polyaniline-Graphene Nanocomposite
Abstract
1. Introduction
2. Choice of Conducting Polymers
3. Design of the Proposed Wideband Non-Resonant Absorber
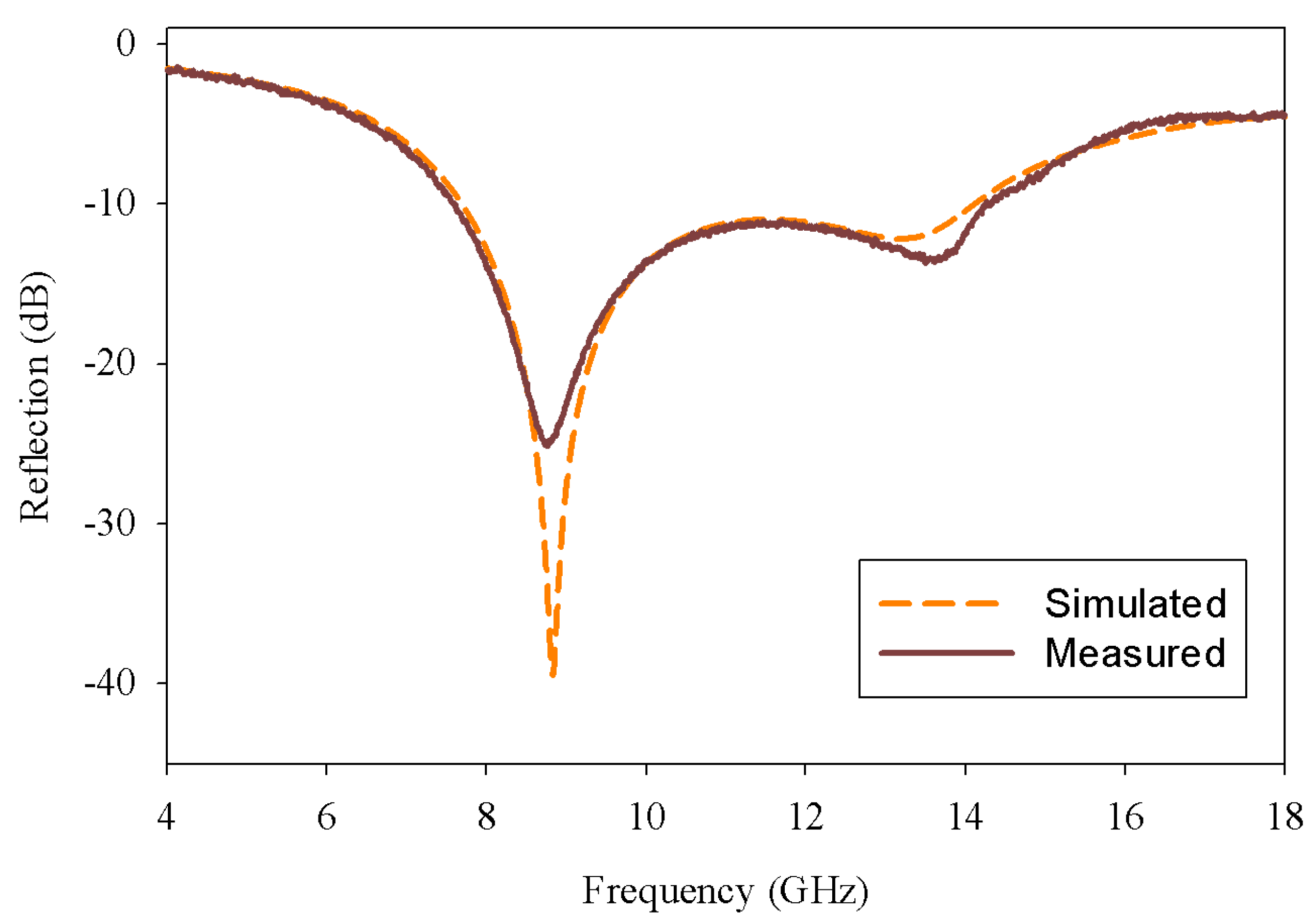
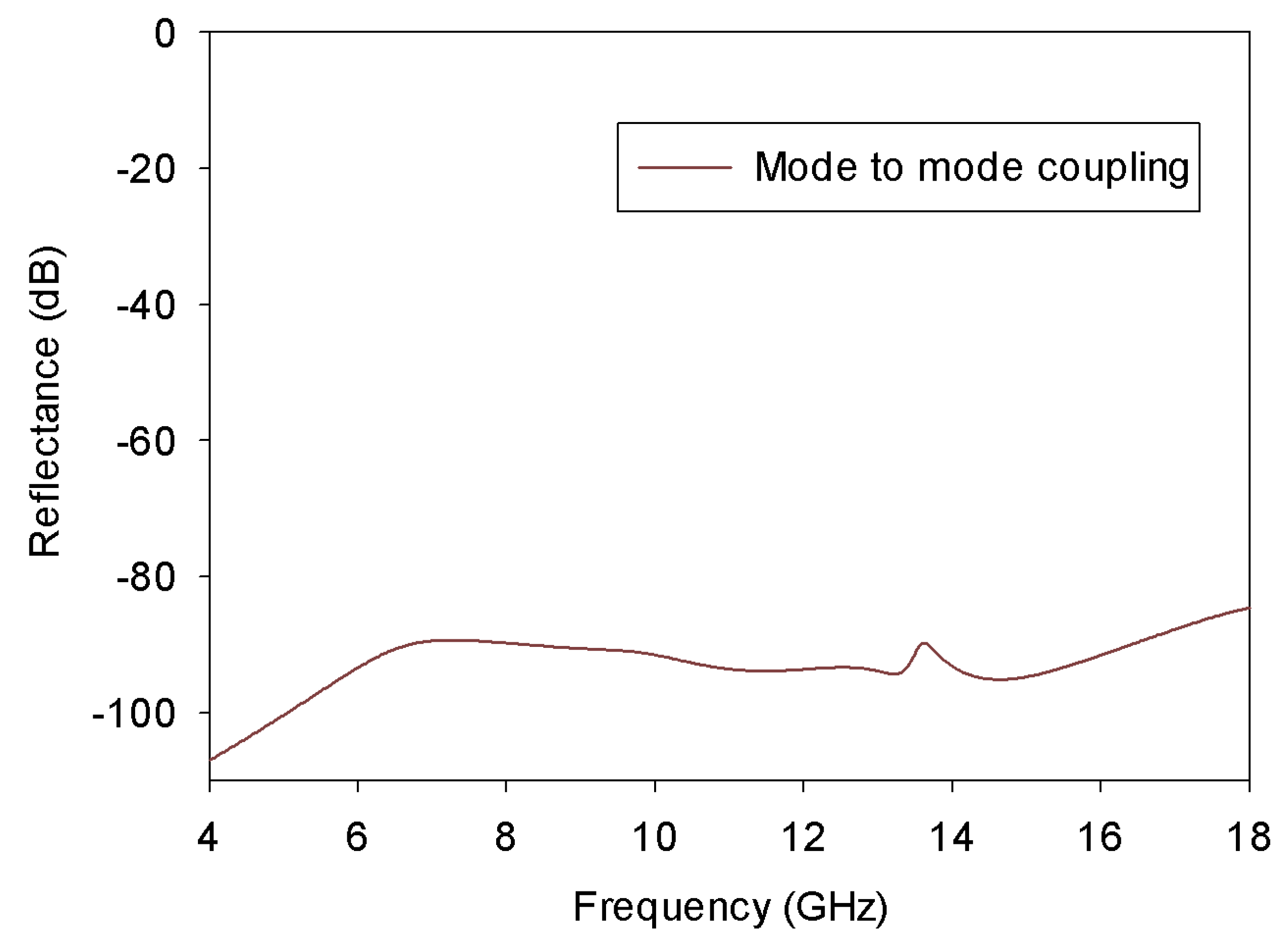
4. Results and Discussion
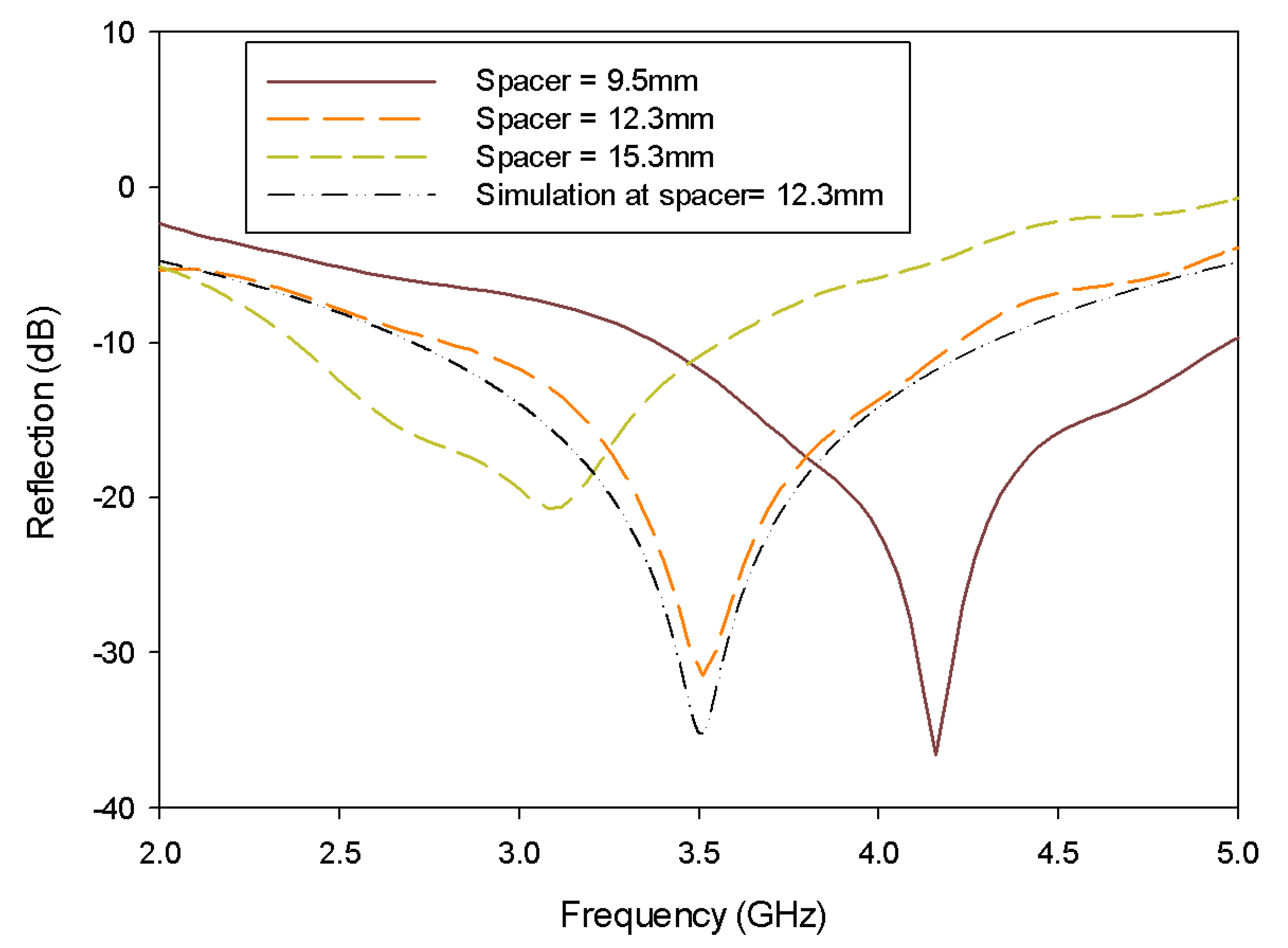
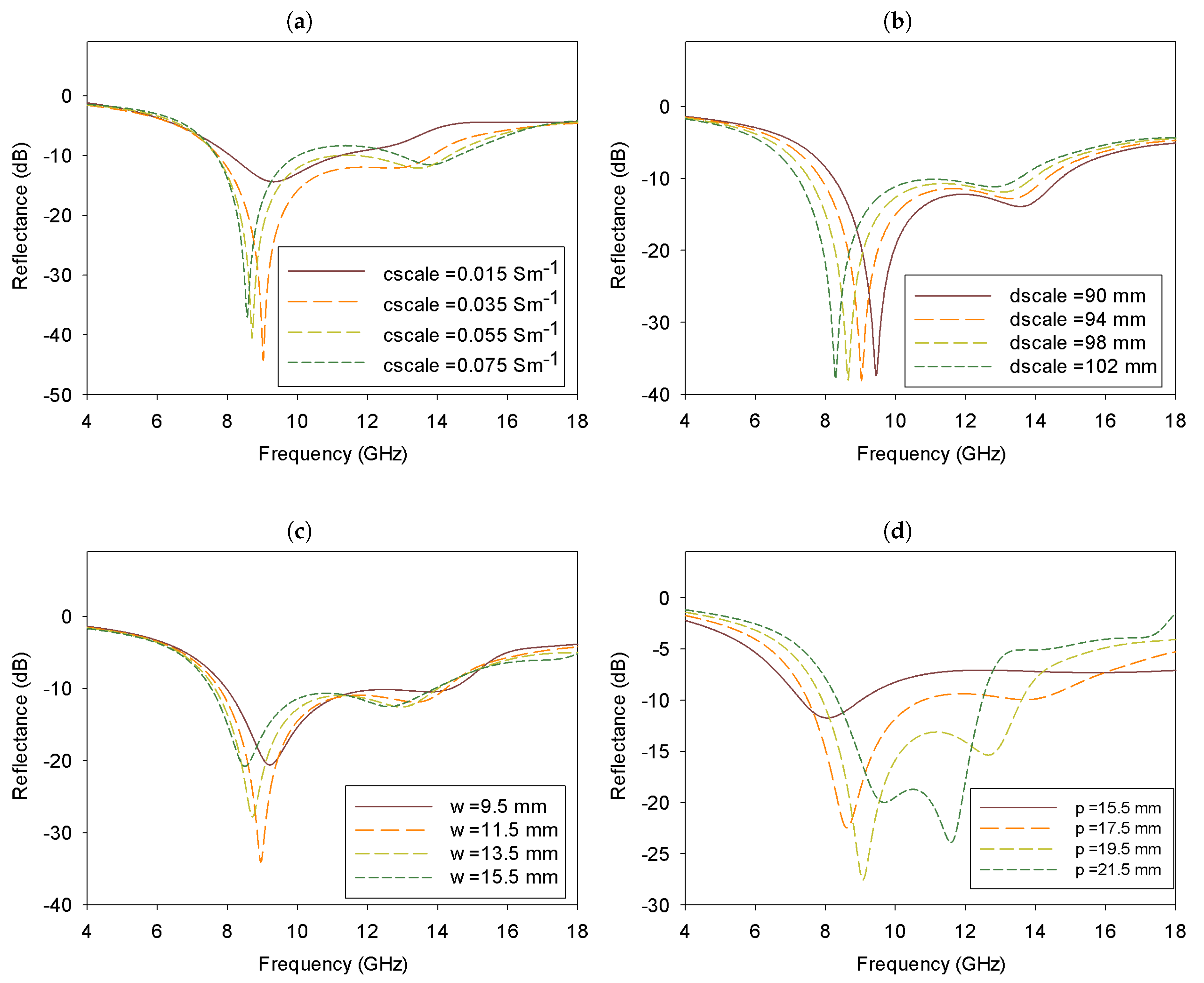
4.1. Parametrics
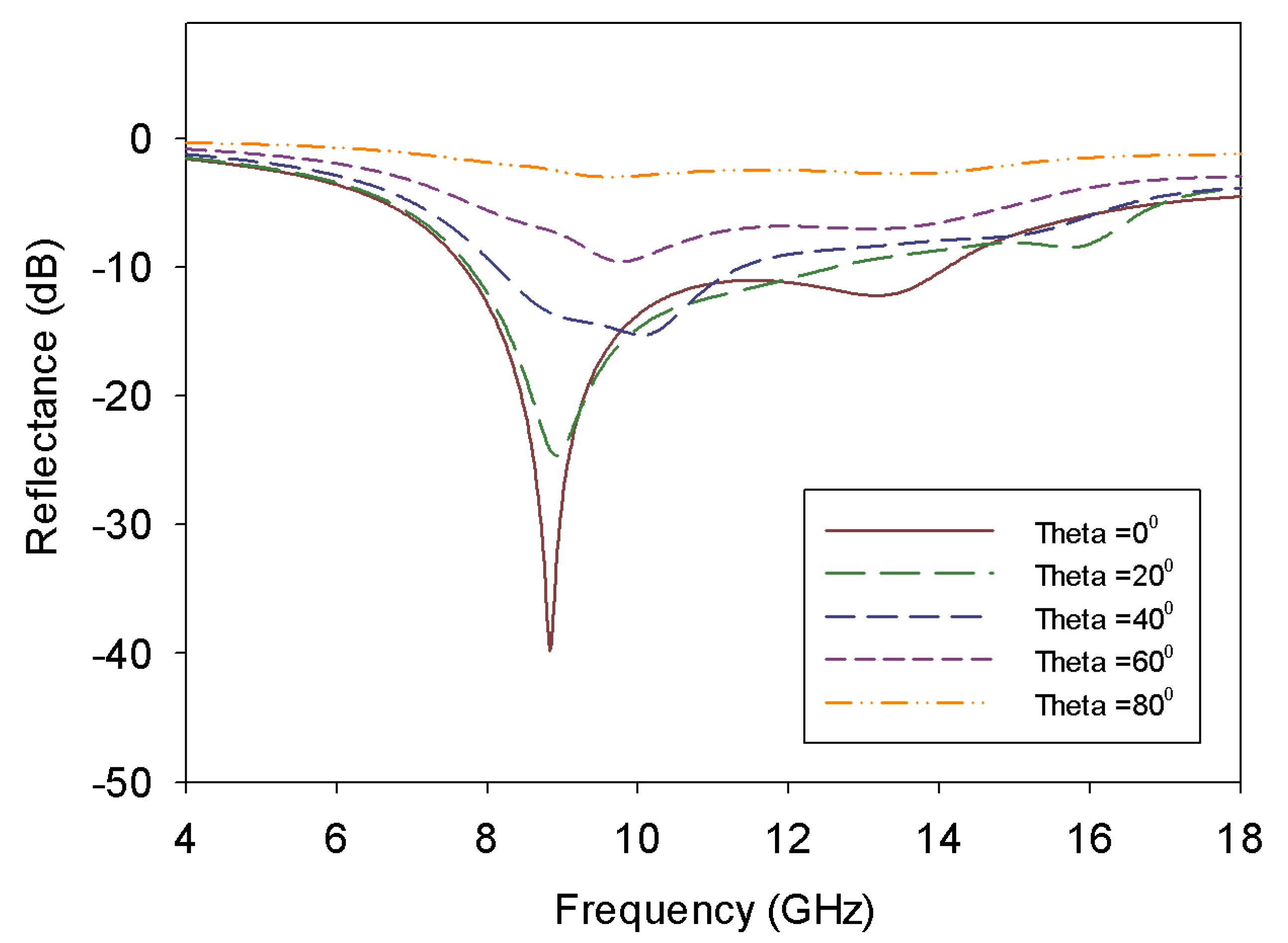
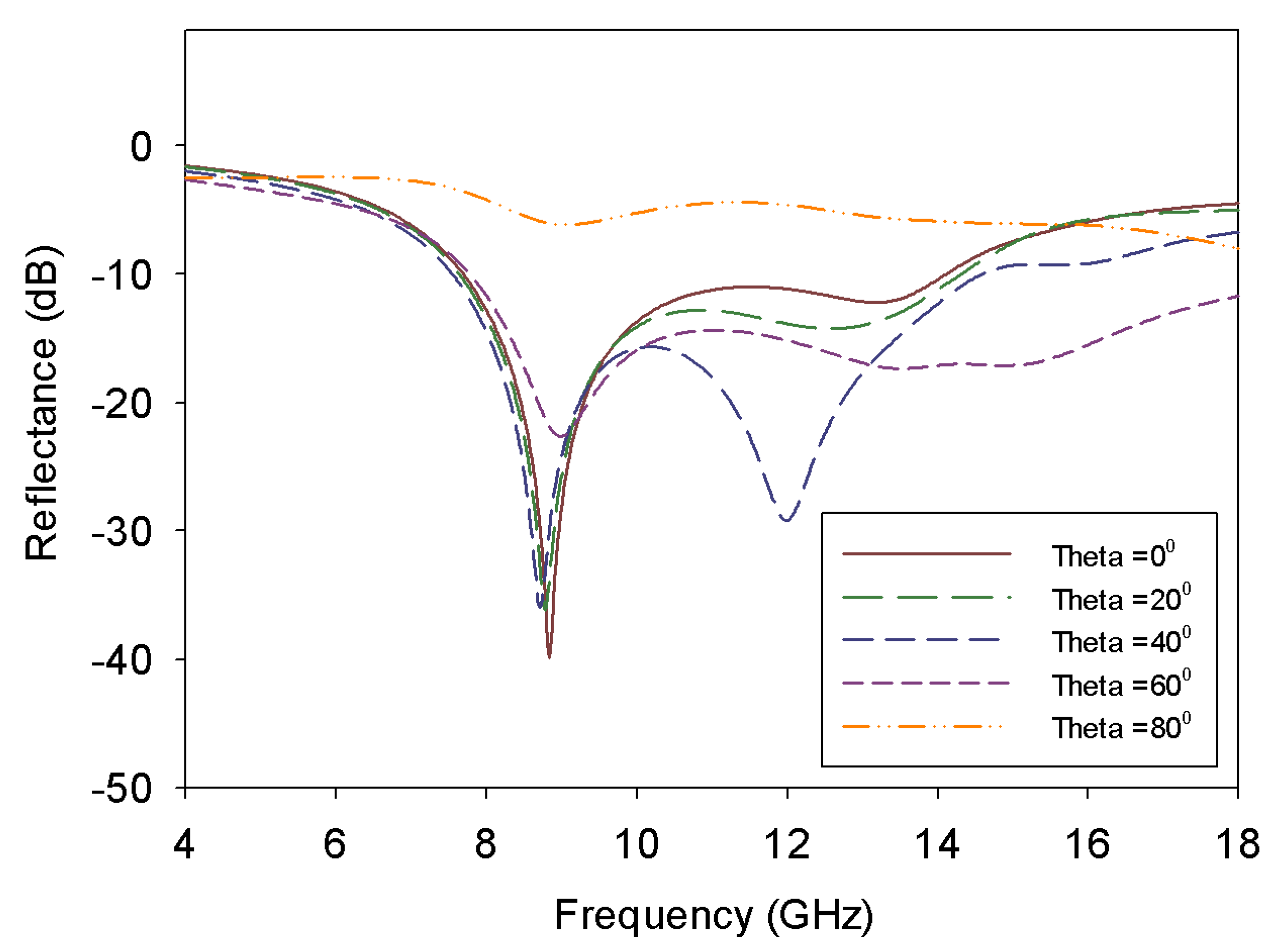
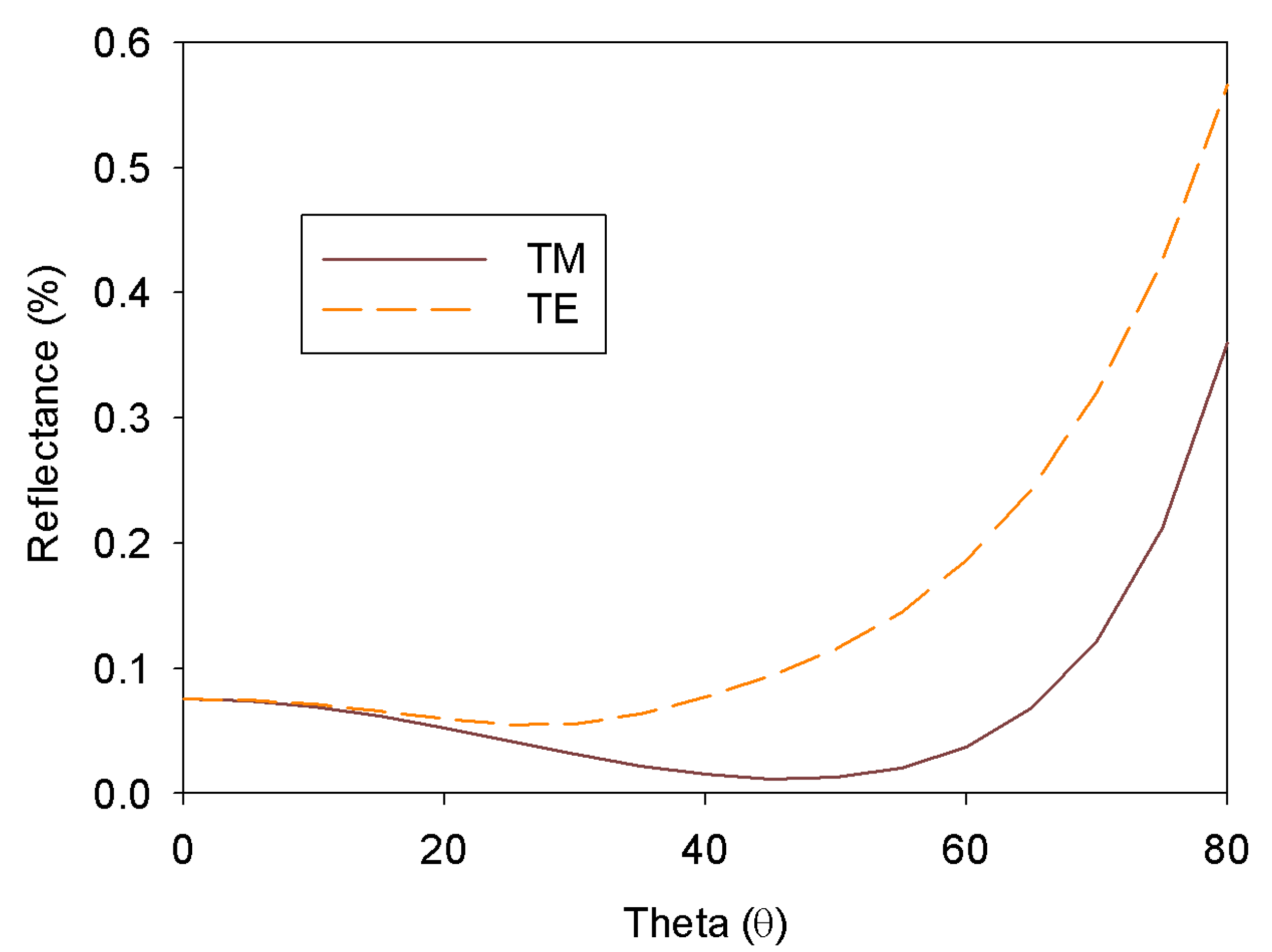
4.2. Angular Variation
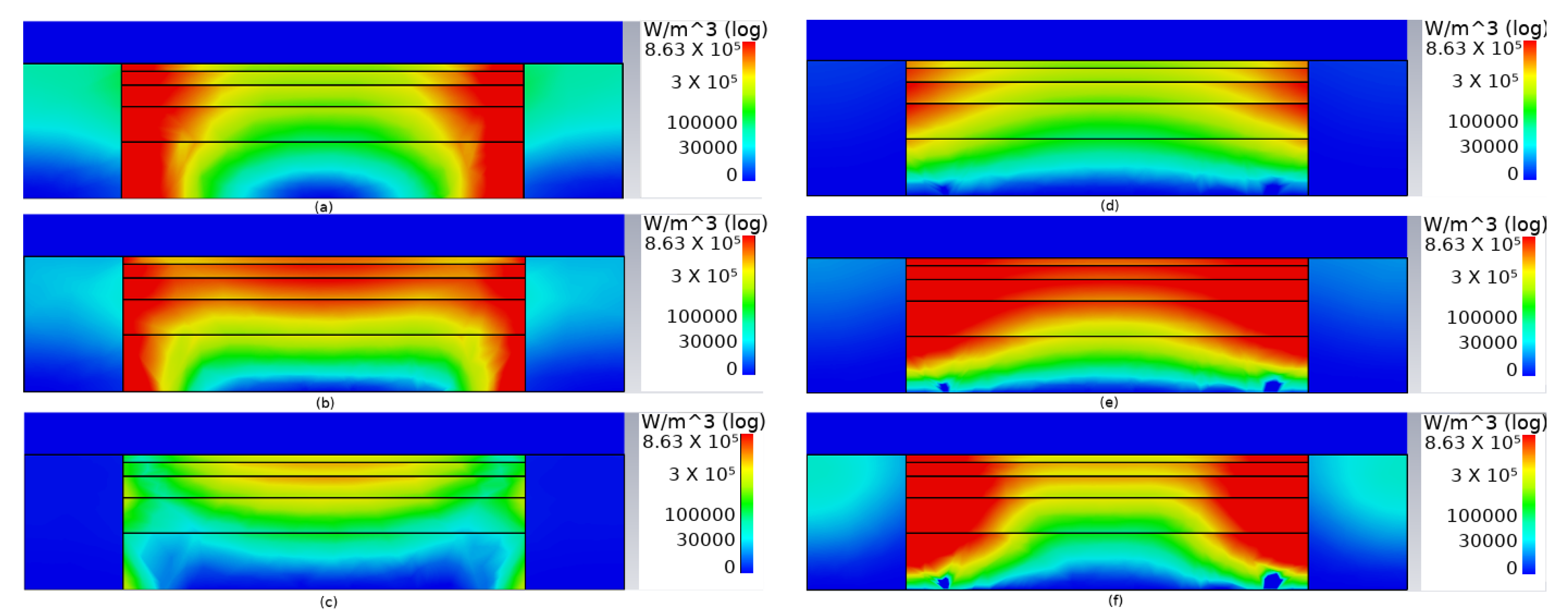
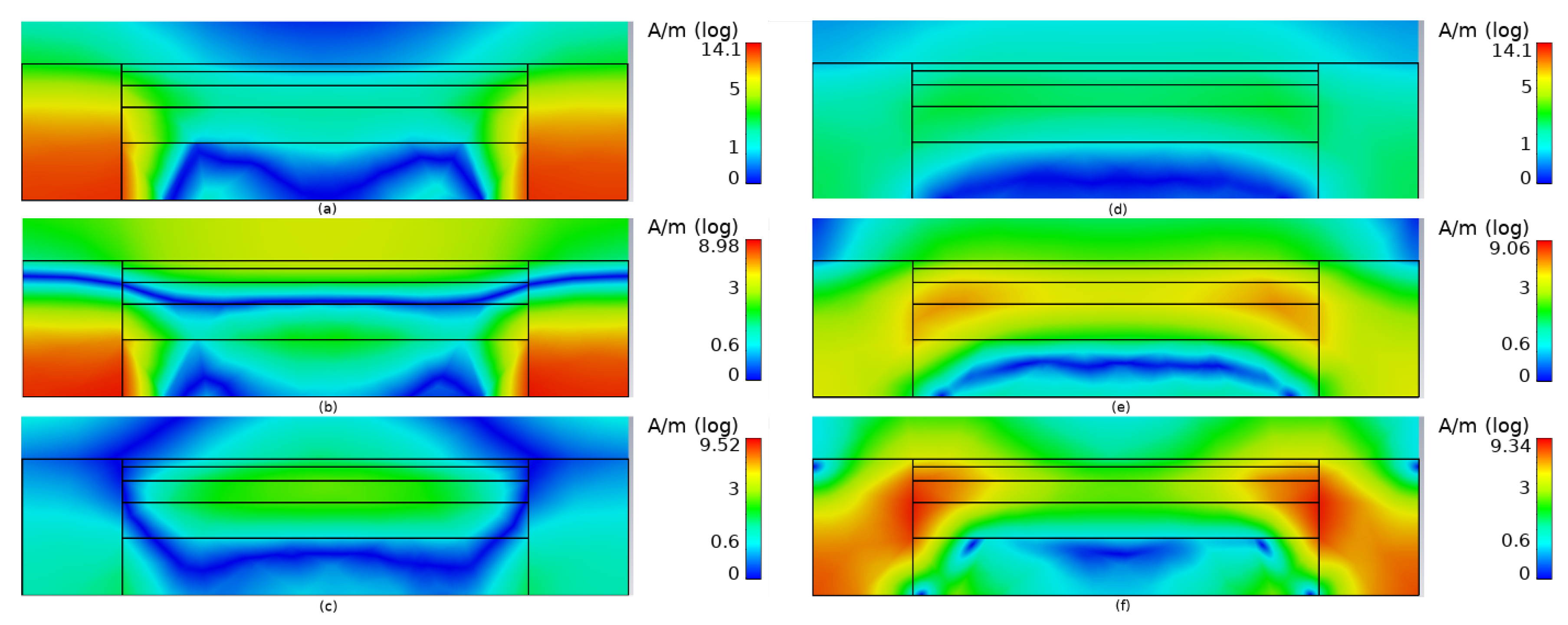
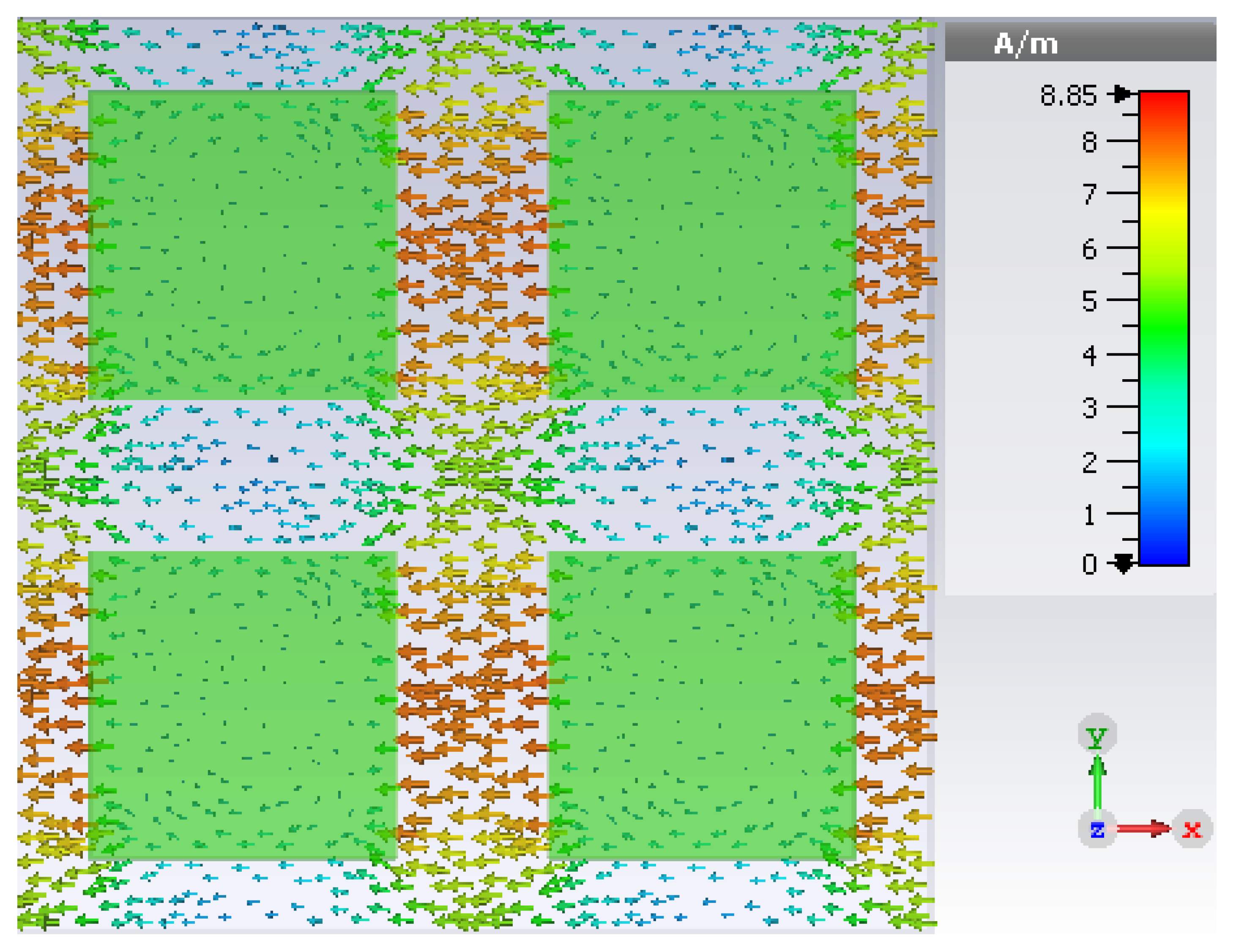
4.3. Field Distribution and Power Loss
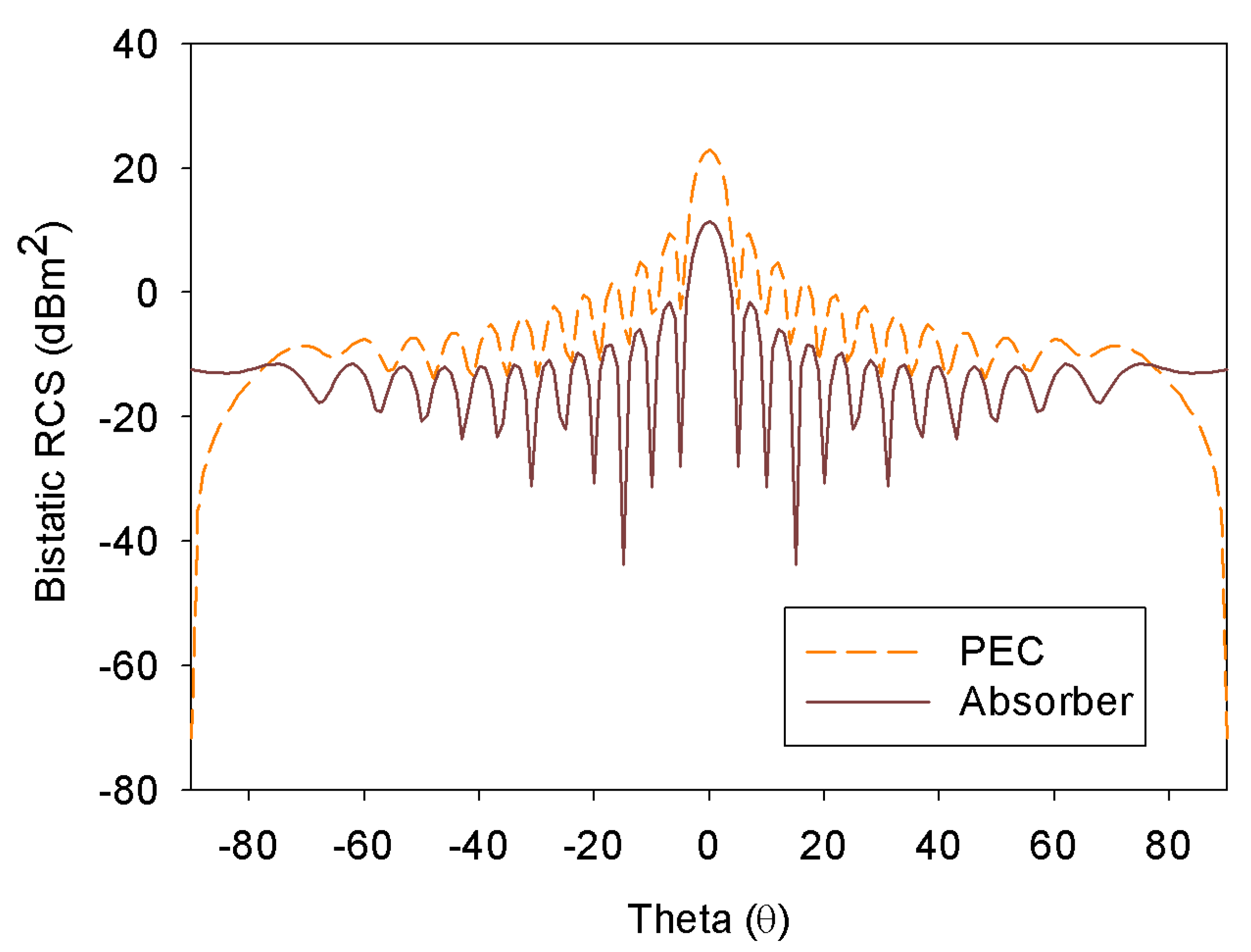
4.4. Bistatic RCS Analysis
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Emerson, W. Electromagnetic Wave Absorbers and Anechoic Chambers Through the Years. IEEE Trans. Antennas Propag. 1973, 21, 484–490. [Google Scholar] [CrossRef]
- Plyushch, A.; Macutkevic, J.; Svirskas, S.; Banys, J.; Plausinaitiene, V.; Bychanok, D.; Maksimenko, S.A.; Selskis, A.; Sokal, A.; Lapko, K.N.; et al. Silicon carbide/phosphate ceramics composite for electromagnetic shielding applications: Whiskers vs particles. Appl. Phys. Lett. 2019, 114, 183105. [Google Scholar] [CrossRef]
- Deng, R.; Li, M.; Muneer, B.; Zhu, Q.; Shi, Z.; Song, L.; Zhang, T. Theoretical analysis and design of ultrathin broadband optically transparent microwave metamaterial absorbers. Materials 2018, 11, 107. [Google Scholar] [CrossRef] [PubMed]
- Beeharry, T.; Yahiaoui, R.; Selemani, K.; Ouslimani, H. A dual layer broadband radar absorber to minimize electromagnetic interference in radomes. Sci. Rep. 2018, 8, 382. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Huang, J.; Hong, S.; Wu, Z.; Liu, Z. A new assessment method for the comprehensive stealth performance of penetration aircrafts. Aerosp. Sci. Technol. 2011, 15, 511–518. [Google Scholar] [CrossRef]
- Theofano, M.K.; Alexandros, I.D.; Stylianos, D.A.; Nikolaos, V.K.; Christos, S.A. A family of ultra-thin, polarization-insensitive, multi-band, highly absorbing metamaterial structures. Prog. Electromagn. Res. 2013, 136, 579–594. [Google Scholar]
- Zabri, S.; Cahill, R.; Schuchinsky, A. Compact FSS absorber design using resistively loaded quadruple hexagonal loops for bandwidth enhancement. Electron. Lett 2015, 51, 162–164. [Google Scholar] [CrossRef]
- Minyeong, Y.; Hyung, K.K.; Sungjoon, L. Angular- and Polarization-Insensitive Metamaterial Absorber Using Subwavelength Unit Cell in Multilayer Technology. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 414–417. [Google Scholar]
- Li, L.; Lv, Z. Ultra-wideband polarization-insensitive and wide-angle thin absorber based on resistive metasurfaces with three resonant modes. J. Appl. Phys 2017, 122, 055104. [Google Scholar] [CrossRef]
- Jiang, W.; Yan, L.; Ma, H.; Fan, Y.; Wang, J.; Feng, M.; Qu, S. Electromagnetic wave absorption and compressive behavior of a three-dimensional metamaterial absorber based on 3D printed honeycomb. Sci. Rep. 2018, 8, 4817. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; Chi, B.; Liu, D.; Gao, S.; Gao, P.; Huang, Y.; Yang, J.; Yin, Z.; Deng, G. Wideband Metamaterial Absorbers Based on Conductive Plastic with Additive Manufacturing Technology. ACS Omega 2018, 3, 11144–11150. [Google Scholar] [CrossRef] [PubMed]
- Fante, R.; Mccormack, M. Reflection Properties of the Salisbury Screen. IEEE Trans. Antennas Propag. 1988, 36, 1443–1454. [Google Scholar] [CrossRef]
- Du Toit, L.J. The Design of Jauman Absorbers. IEEE Antennas Propag. Mag. 1994, 36, 17–25. [Google Scholar] [CrossRef]
- Munk, B.; Munk, P.; Pryor, J. On designing Jaumann and circuit analog absorbers (CA absorbers) for oblique angle of incidence. IEEE Trans. Antennas Propag. 2007, 55, 186–193. [Google Scholar] [CrossRef]
- Zadeh, A.; Karlsson, A. Capacitive circuit method for fast and efficient design of wideband radar absorbers. IEEE Trans. Antennas Propag. 2009, 57, 2307–2314. [Google Scholar] [CrossRef]
- Tan, L.J.; Zhu, W.; Zhou, K. Recent Progress on Polymer Materials for Additive Manufacturing. Adv. Funct. Mater. 2020, 30, 2003062. [Google Scholar] [CrossRef]
- Meisak, D.; Gurnevich, E.; Plyushch, A.; Bychanok, D.; Georgiev, V.; Kotsilkova, R.; Kuzhir, P. Robust design of compact microwave absorbers and waveguide matched loads based on DC-conductive 3D-printable filament. J. Phys. D Appl. Phys. 2020, 53, 305301. [Google Scholar] [CrossRef]
- Noor, A.; Hu, Z. Wideband multilayer Sierpinski carpet array radar absorber. Electron. Lett. 2016, 52, 1617–1618. [Google Scholar] [CrossRef]
- Matthew, P.; John, A.; Karwan, R.; Charles, L.B.; Joseph, E.; Tobias, M.; Joshua, R.; Edward, J.W. A 3D-printed broadband millimeter wave absorber. Rev. Sci. Instrum. 2019, 90, 024701. [Google Scholar]
- Ren, J.; Yin, J. 3D-printed low-cost dielectric-resonator-based ultra-broadband microwave absorber using carbon-loaded Acrylonitrile Butadiene styrene polymer. Materials 2018, 10, 1249. [Google Scholar] [CrossRef]
- Heeger, A. Semiconducting and metallic polymers: The fourth generation of polymeric materials. Curr. Appl. Phys. 2001, 1, 247–267. [Google Scholar] [CrossRef]
- Le, T.H.; Kim, Y.; Yoon, H. Electrical and Electrochemical Properties of Conducting Polymers. Polymers 2017, 9, 150. [Google Scholar] [CrossRef] [PubMed]
- Kumar, V.; Yokozeki, T.; Goto, T.; Takahashi, T.; Dhakate, S.; Singh, B. Irreversible tunability of through-thickness electrical conductivity of polyaniline-based CFRP by de-doping. Compos. Sci. Technol. 2017, 152, 20–26. [Google Scholar] [CrossRef]
- Patil, A.; Heeger, A.; Wudl, F. Optical properties of conducting polymers. Compos. Sci. Technol. 1988, 88, 183–200. [Google Scholar] [CrossRef]
- Anno, H.; Yamaguchi, K.; Nakabayashi, T.; Kurokawa, H.; Akagi, F.; Hojo, M.; Toshima, N. Thermoelectric properties of conducting polyaniline/BaTiO3 nanoparticle composite films. IOP Conf. Ser. Mater. Sci. Eng. 2011, 18, 142003. [Google Scholar] [CrossRef]
- Evans, D. A bird’s eye view of the synthesis and practical application of conducting polymers. Polym. Int. 2017, 67, 351–355. [Google Scholar] [CrossRef]
- Tantawy, H.R.; Aston, D.E.; Smith, J.R.; Young, J.L. Comparison of Electromagnetic Shielding with Polyaniline Nanopowders Produced in Solvent-Limited Conditions. ACS Appl. Mater. Interfaces 2013, 5, 4648–4658. [Google Scholar] [CrossRef] [PubMed]
- Hamouda, Z.; Wojkiewicz, J.; Pud, A.; Kone, L.; Bergheul, S.; Lasri, T. Flexible UWB organic antenna for wearable technologies application. IET Microw. Antennas Propag. 2018, 12, 160–166. [Google Scholar] [CrossRef]
- Simone, P.; Mirabel, R. Performance Prediction of Microwave Absorbers Based on POMA/Carbon Black Composites in the Frequency Range of 8.2 to 20 GHz. J. Aerosp. Technol. Manag. 2018, 10. [Google Scholar] [CrossRef]
- Qiu, M.; Zhang, Y.; Wen, B. Facile synthesis of polyaniline nanostructures with effective electromagnetic interference shielding performance. J. Mater. Sci. Mater. Electron. 2018, 29, 10437–10444. [Google Scholar] [CrossRef]
- Deepak, V.; Dutta, V. Novel microstructure in spin coated polyaniline thin films. J. Phys. Condens. Matter 2007, 19, 186212. [Google Scholar]
- Karyakin, A.A.; Vuki, M.; Lukachova, L.V.; Karyakina, E.E.; Orlov, A.V.; Karpachova, G.P.; Wang, J. Processible polyaniline as an advanced potentiometric pH transducer. Application to biosensors. Anal. Chem. 1999, 71, 2534–2540. [Google Scholar] [CrossRef] [PubMed]
- Gervais, F.; Petit, N.; Popon, C.; Buvat, P. Doping dependence of infrared conductivity of camphor-sulphonic- acid-doped polyaniline. Eur. Phys. J. B 2003, 31, 47–52. [Google Scholar] [CrossRef]
- Lamouri, S.; Bendahgane, S.; Oudia, A. The Preparation and Analytical Study of Conducting Polyaniline Thin Films. J. Pet. Environ. Biotechnol. 2014, 5. [Google Scholar] [CrossRef]
- Yazid, M.; Ghani, S.; Osman, A.; Din, S.; Jin, T. The effect on poly (ethylene oxide)/poly (vinyl chloride)/ polyaniline (PAni) films by ethylene dimethacrylate as surface modifier: Electrical conductivity and characterization. J. Phys. Conf. Ser. 2017, 908, 012007. [Google Scholar] [CrossRef]
- Lakshmi, G.; Anju, D.; Azher, M.S.; Zulfequar, M.; Avasthi, D. RF-plasma polymerization and characterization of polyaniline. Eur. Polym. J. 2009, 45, 2873–2877. [Google Scholar] [CrossRef]
- Kumar, A.; Kumar, V.; Awasthi, K. Polyaniline-Carbon Nanotube Composites: Preparation Methods, Properties, and Applications. Polym. Plast. Technol. Eng. 2018, 57, 70–97. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, Q.; Wang, J.; Huang, X.; Bai, H. A self-assembly route to porous polyaniline/reduced graphene oxide composite materials with molecular-level uniformity for high-performance supercapacitors. Energy Environ. Sci. 2018, 11, 1280–1286. [Google Scholar] [CrossRef]
- Kumar, N.A.; Choi, H.J.; Shin, Y.R.; Chang, D.W.; Dai, L.; Baek, J.B. Polyaniline-Grafted Reduced Graphene Oxide for Efficient Electrochemical Supercapacitors. ACS Nano 2012, 6, 1715–1723. [Google Scholar] [CrossRef]
- Chen, X.; Meng, F.; Zhou, Z.; Tian, X.; Shan, L.; Zhu, S.; Xu, X.; Jiang, M.; Wang, L.; Hui, D.; et al. One-step synthesis of graphene/polyaniline hybrids by in situ intercalation polymerization and their electromagnetic properties. Nanoscale 2014, 6, 8140–8148. [Google Scholar] [CrossRef]
- Paton, K.R.; Varrla, E.; Backes, C.; Smith, R.J.; Khan, U.; O’Neill, A.; Boland, C.; Lotya, M.; Istrate, O.M.; King, P.; et al. Scalable production of large quantities of defect-free few-layer graphene by shear exfoliation in liquids. Nat. Mater. 2014, 13, 624. [Google Scholar] [CrossRef]
- Huang, X.; Leng, T.; Zhang, X.; Chen, J.C.; Chang, K.H.; Geim, A.K.; Novoselov, K.S.; Hu, Z. Binder-free highly conductive graphene laminate for low cost printed radio frequency applications. Appl. Phys. Lett. 2015, 106, 203105. [Google Scholar] [CrossRef]
- Lee, H.J.; Jeong, N.; Choi, H.H. Microwave Properties of Coplanar Waveguide-Based PEDOT:PSS Conducting Polymer Line in Ethanol Gas Atmosphere. Materials 2020, 13, 1759. [Google Scholar] [CrossRef]
- Jang, C.; Park, J.K.; Yun, G.H.; Choi, H.H.; Lee, H.J.; Yook, J.G. Radio-Frequency/Microwave Gas Sensors Using Conducting Polymer. Materials 2020, 13, 2859. [Google Scholar] [CrossRef] [PubMed]
- Jayalakshmi, C.G.; Inamdar, A.; Anand, A.; Kandasubramanian, B. Polymer matrix composites as broadband radar absorbing structures for stealth aircrafts. J. Appl. Polym. Sci. 2019, 136, 47241. [Google Scholar] [CrossRef]
- Thomas, P.; Pushkaran, N.; Aanandan, C. Preparation and microwave characterization of novel polyaniline- graphene composite for antenna applications. In Proceedings of the 2017 Progress in Electromagnetics Research Symposium—Fall, Singapore, 19–22 November 2017. [Google Scholar]
- Liang, Y.; Li, C.T.; Guan, Y.; Hu, Y. Gait recognition based on the golden ratio. EURASIP J. Image Video Process. 2016, 2016, 22. [Google Scholar] [CrossRef]
- Unal, E.; Dincer, F.; Tetik, E.; Karaaslan, M.; Bakir, M.; Sabah, C. Tunable perfect metamaterial absorber design using the golden ratio and energy harvesting and sensor applications. J. Mater. Sci. Mater. Electron. 2015, 26, 9735–9740. [Google Scholar] [CrossRef]
- Press, W.; Teukolsky, S.; Vetterling, W.; Flannery, B. Numerical Recipes 3rd Edition: The Art of Scientific Computing; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Soeini, R.A.; Niroomand, A.; Parizi, A.K. Using fibonacci numbers to forecast the stock market. Int. J. Manag. Sci. Eng. Manag. 2012, 7, 268–279. [Google Scholar] [CrossRef]
- Kumar, A.; Singh Pharwaha, A.P. On the Design of Wideband Sierpinski Carpet Fractal Antenna for Radio Navigation and Fixed Satellite Services. In Proceedings of the 2019 6th International Conference on Signal Processing and Integrated Networks (SPIN), Noida, India, 7–8 March 2019; pp. 736–738. [Google Scholar] [CrossRef]
- Xiong, H.; Hong, J.; Luo, C.; Zhong, L. An ultrathin and broadband metamaterial absorber using multi-layer structures. J. Appl. Phys. 2013, 114, 064109. [Google Scholar] [CrossRef]
- Sun, J.; Liu, L.; Dong, G.; Zhou, J. An extremely broad band metamaterial absorber based on destructive interference. Opt. Express 2011, 19, 21155–21162. [Google Scholar] [CrossRef]
- Ohman, G. The pseudo-Brewster angle. IEEE Trans. Antennas Propag. 1977, 25, 903–904. [Google Scholar] [CrossRef]
- Makio, A.; Yasuo, G. Brewster and Pseudo-Brewster Angle Technique for Determination of Optical Constants. Jpn. J. Appl. Phys. 1992, 31, 120. [Google Scholar]





| Reference | Lower Frequency | Upper Frequency | Thickness (Lambda) |
|---|---|---|---|
| [9] | −7.0 GHz | −37.4 GHz | −3.80 mm () |
| [10] | −3.5 GHz | −24.0 GHz | −15.51 mm () |
| [11] | −16.3 GHz | −54.3 GHz | −2.70 mm () |
| [52] | −8.4 GHz | −21.0 GHz | −3.65 mm () |
| [53] | −10.0 GHz | −70.0 GHz | −4.50 mm () |
| Proposed | −7.8 GHz | −14.0 GHz | −4.16 mm () |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thomas, P.; Abdulhakim, L.V.; Pushkaran, N.K.; Karuvandi, A.C. Wideband Radar Absorbing Structure Using Polyaniline-Graphene Nanocomposite. C 2020, 6, 72. https://doi.org/10.3390/c6040072
Thomas P, Abdulhakim LV, Pushkaran NK, Karuvandi AC. Wideband Radar Absorbing Structure Using Polyaniline-Graphene Nanocomposite. C. 2020; 6(4):72. https://doi.org/10.3390/c6040072
Chicago/Turabian StyleThomas, Paulbert, Libimol V. Abdulhakim, Neeraj K. Pushkaran, and Aanandan C. Karuvandi. 2020. "Wideband Radar Absorbing Structure Using Polyaniline-Graphene Nanocomposite" C 6, no. 4: 72. https://doi.org/10.3390/c6040072
APA StyleThomas, P., Abdulhakim, L. V., Pushkaran, N. K., & Karuvandi, A. C. (2020). Wideband Radar Absorbing Structure Using Polyaniline-Graphene Nanocomposite. C, 6(4), 72. https://doi.org/10.3390/c6040072




