Suppression of the Spatial Hydrodynamic Instability in Scale-Resolving Simulations of Turbulent Flows Inside Lined Ducts
Abstract
1. Introduction
2. Flows under Consideration and Some Numerical Details
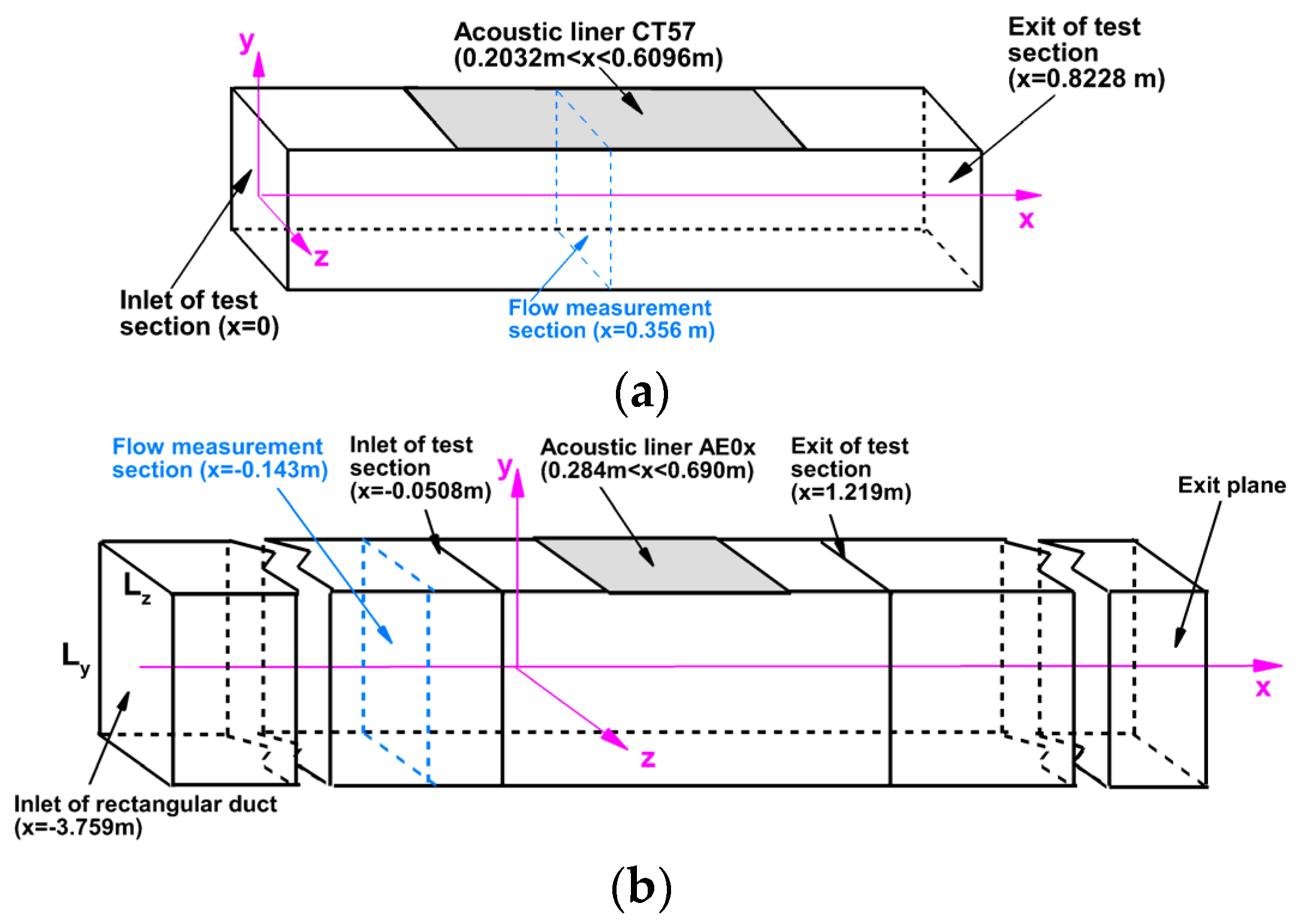
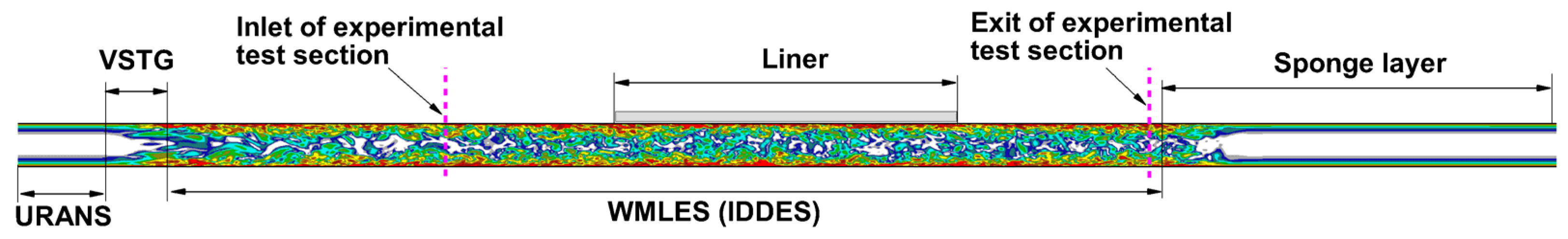
2.1. Ducts Geometries and Flow Regimes
2.2. Computational Problem Statement
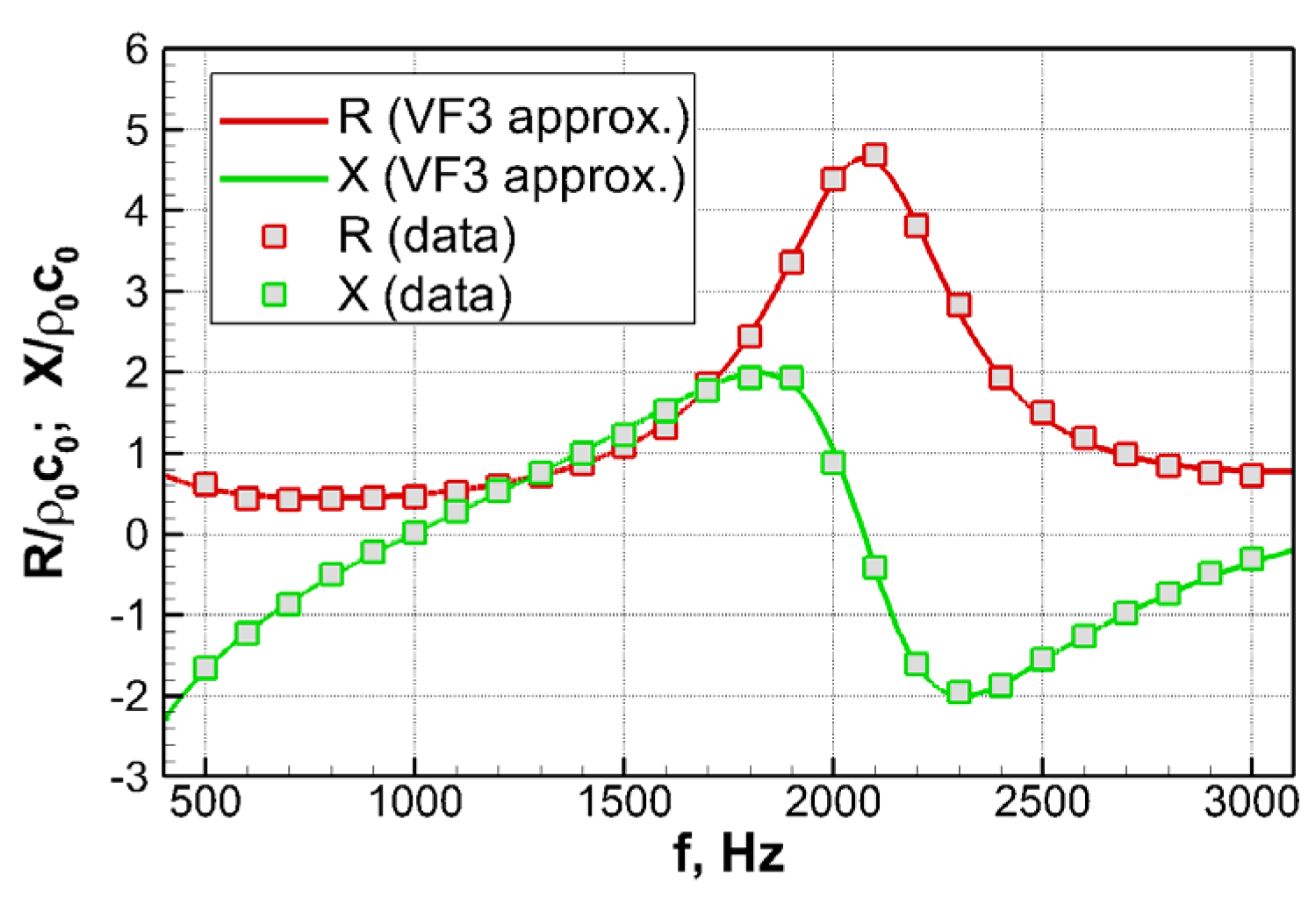
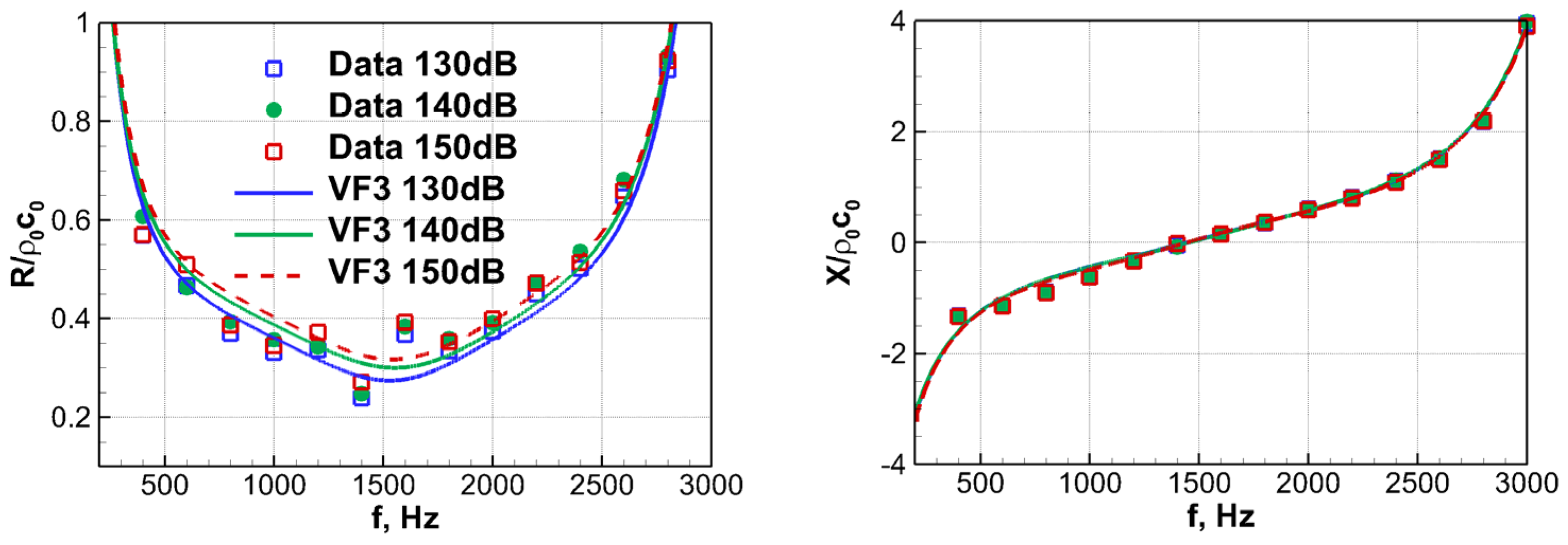
2.3. Impedance Approximations, Numerics, and Grids
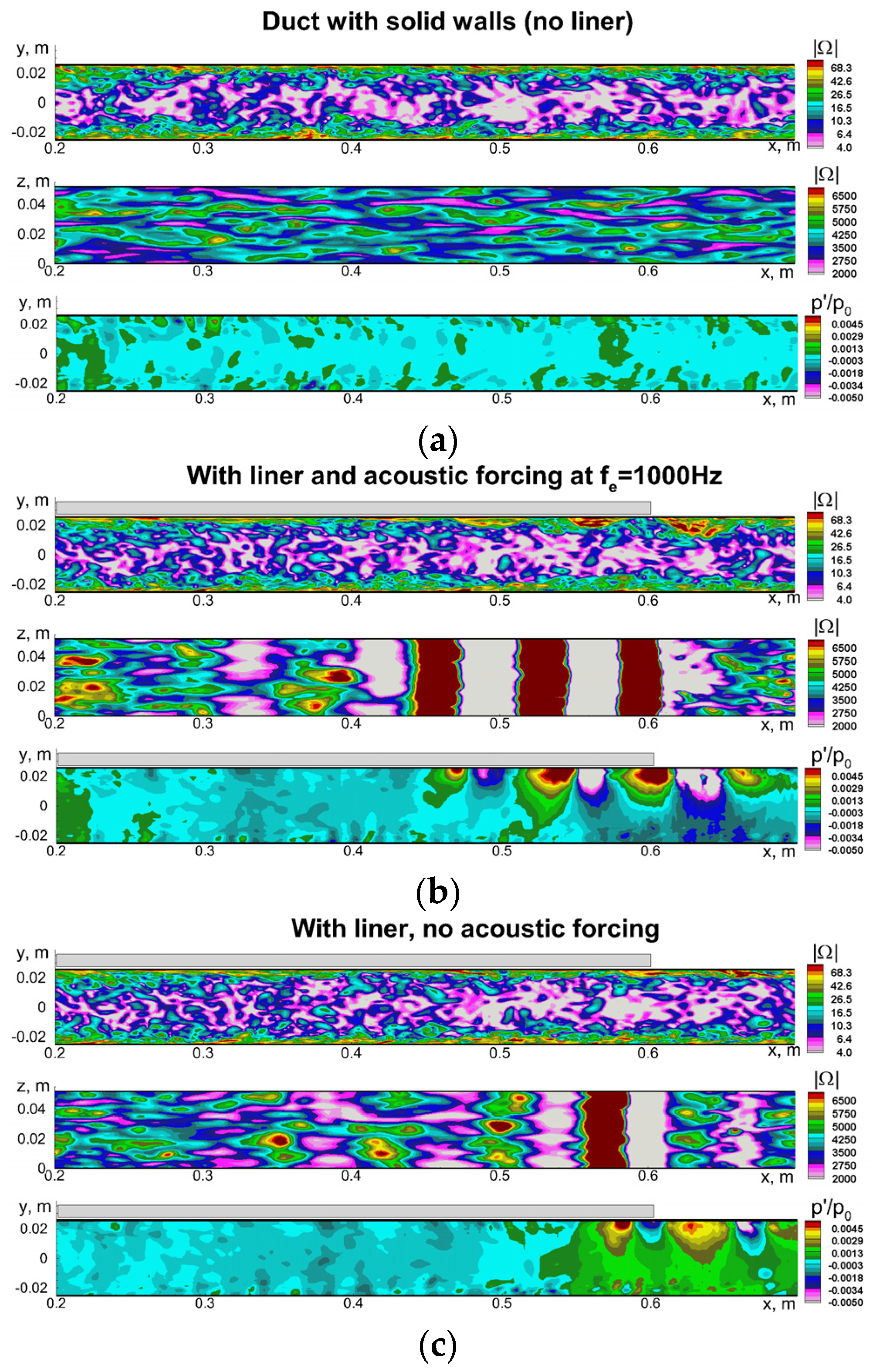
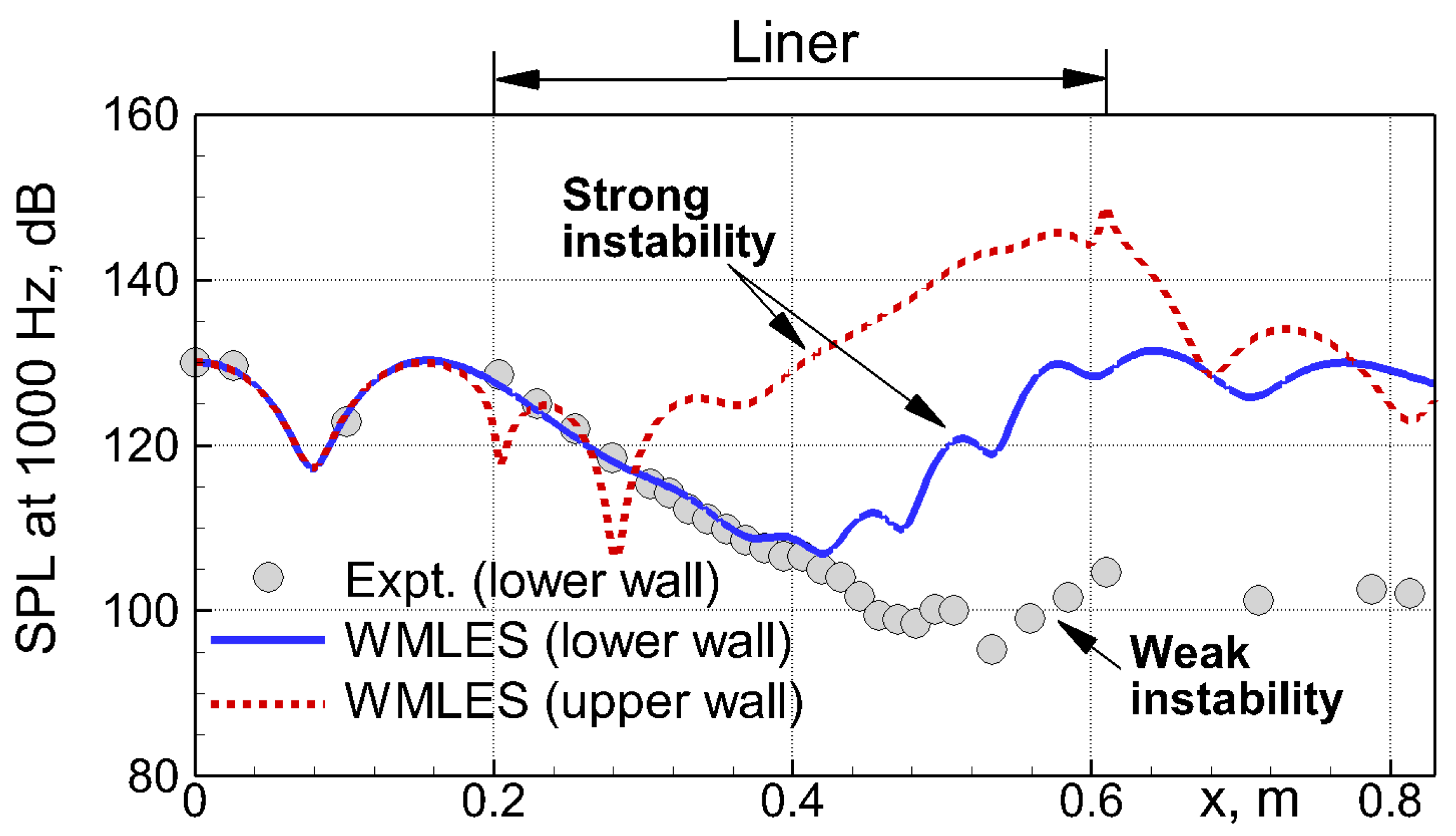
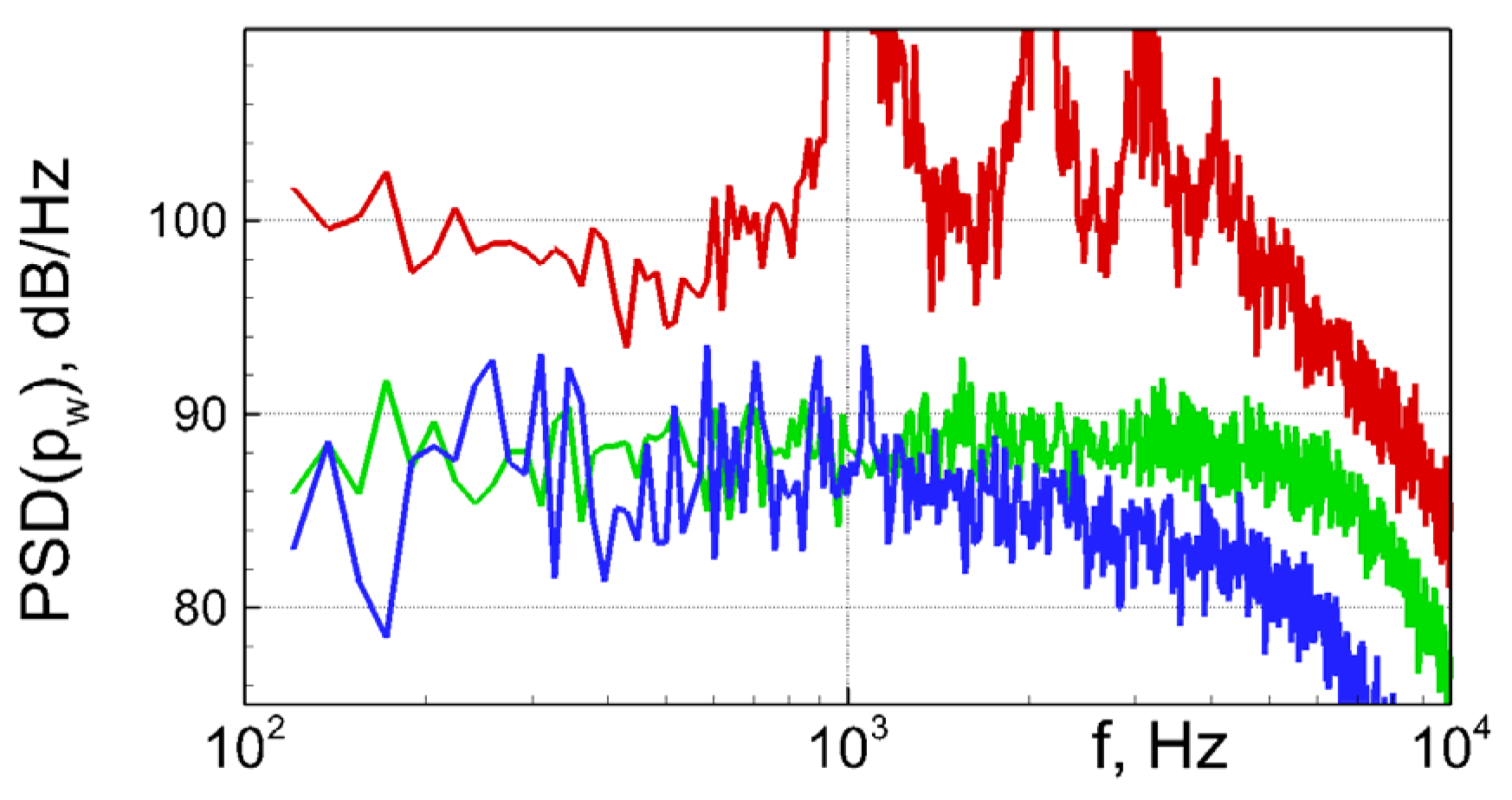
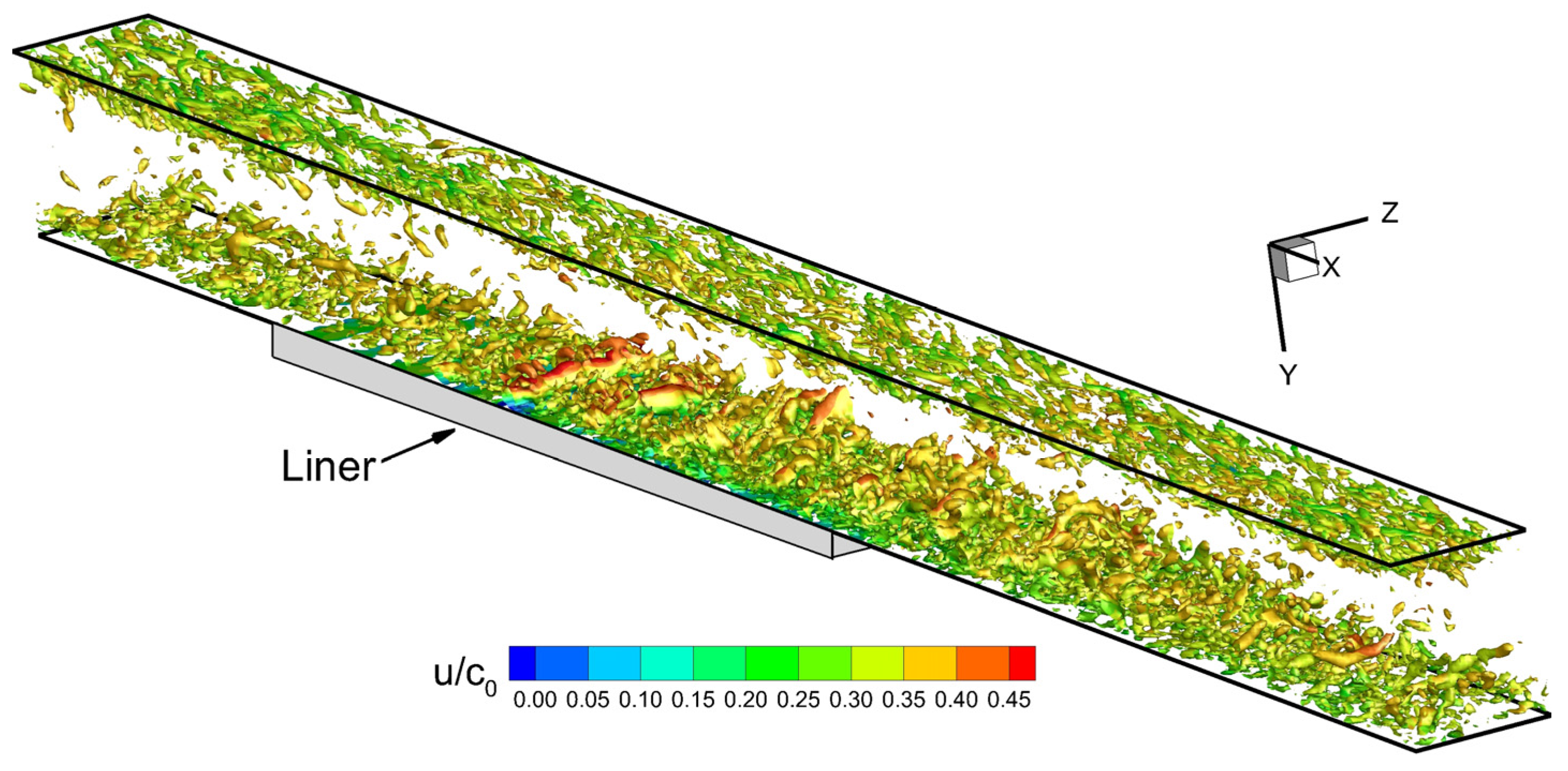
3. Design of Novel Stabilization Body Force Based on Simulations of NASA GIT Flow over a Ceramic Tubular Liner
3.1. General Consideration and Shortcomings of PGTS Method
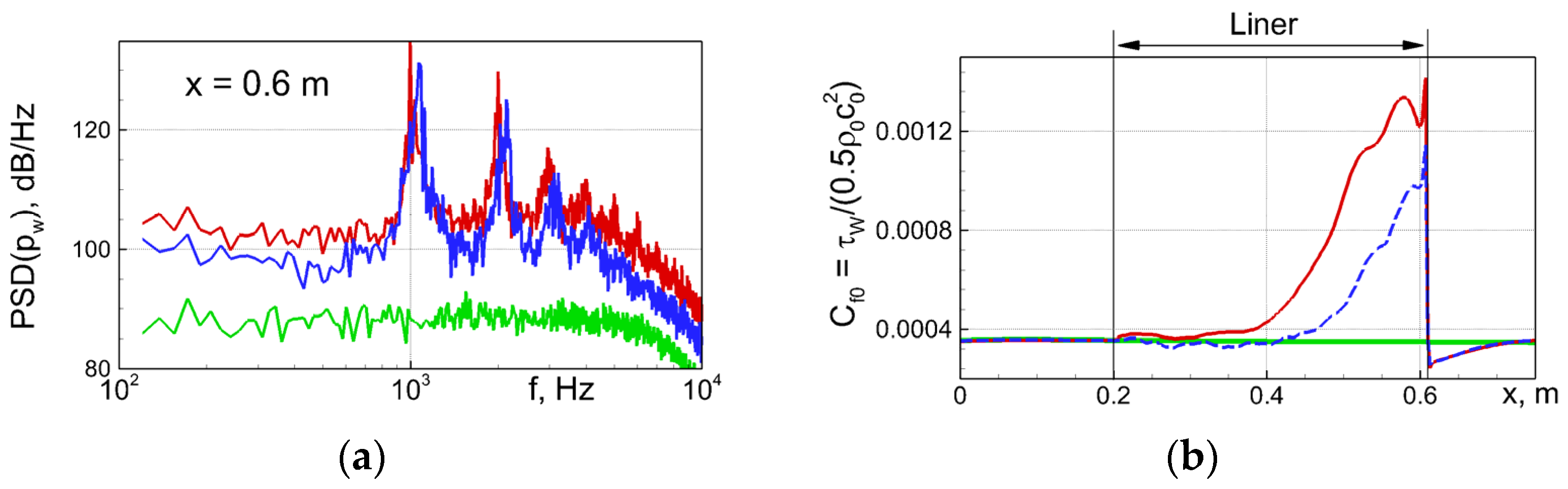
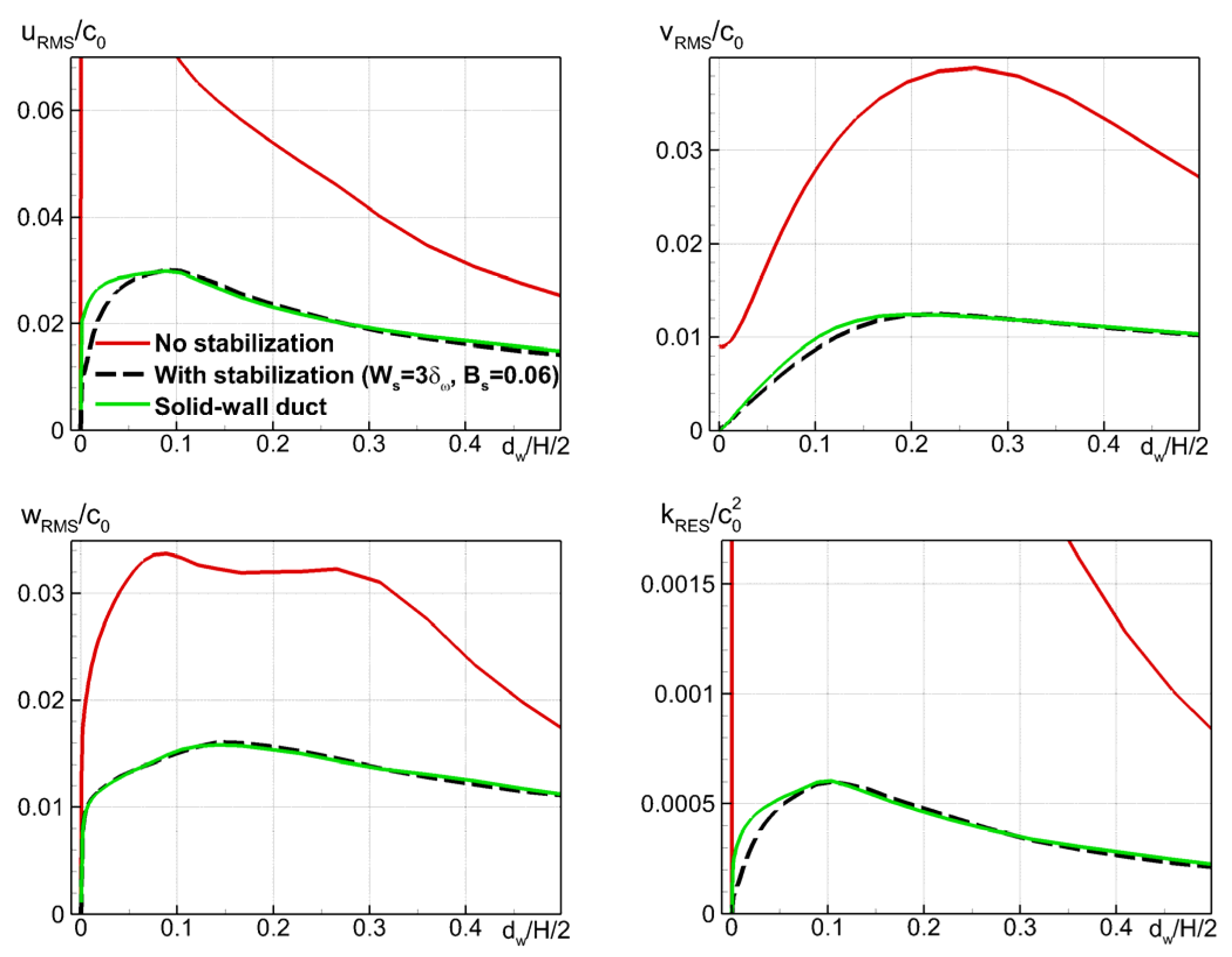
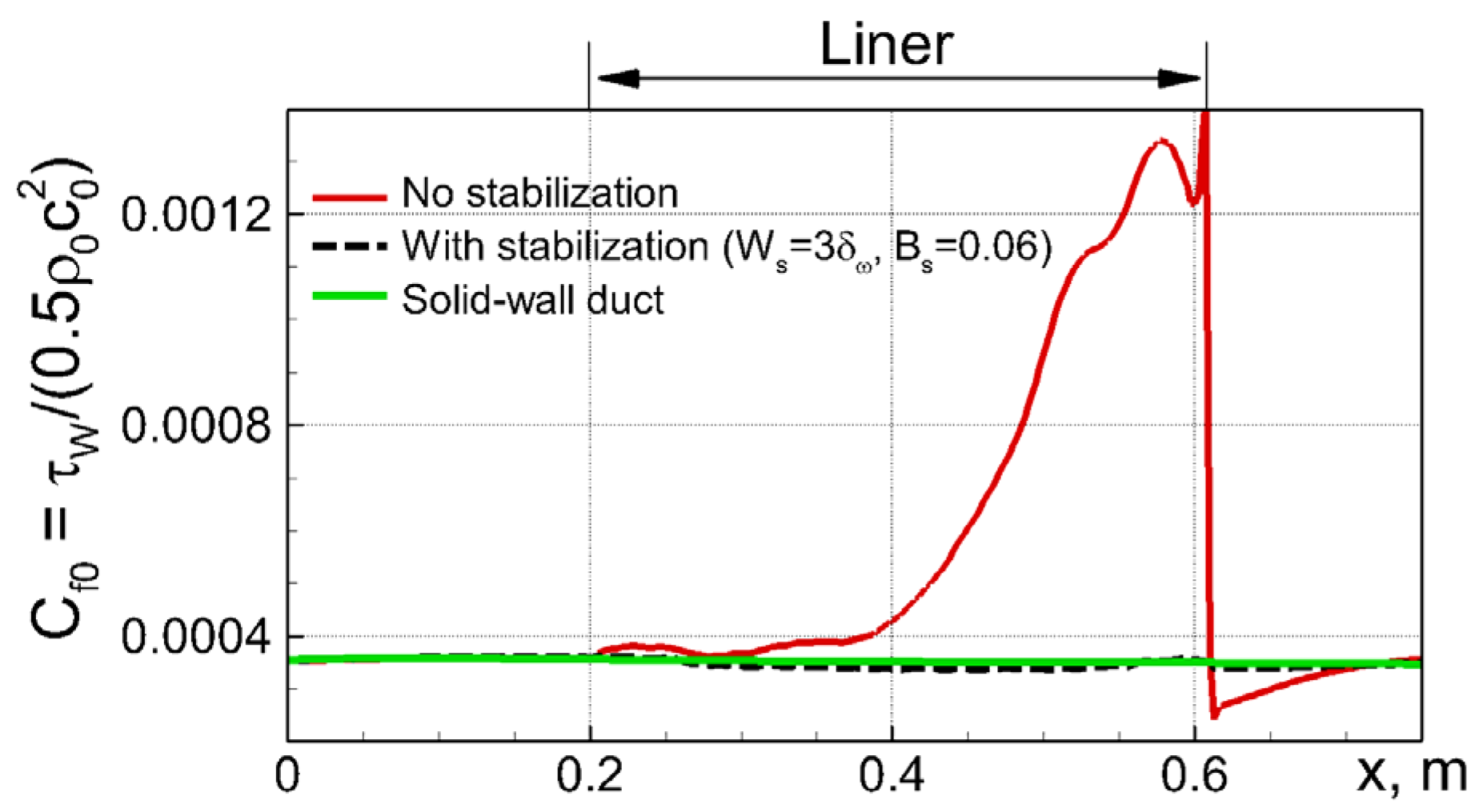
3.2. Design of New Body Force
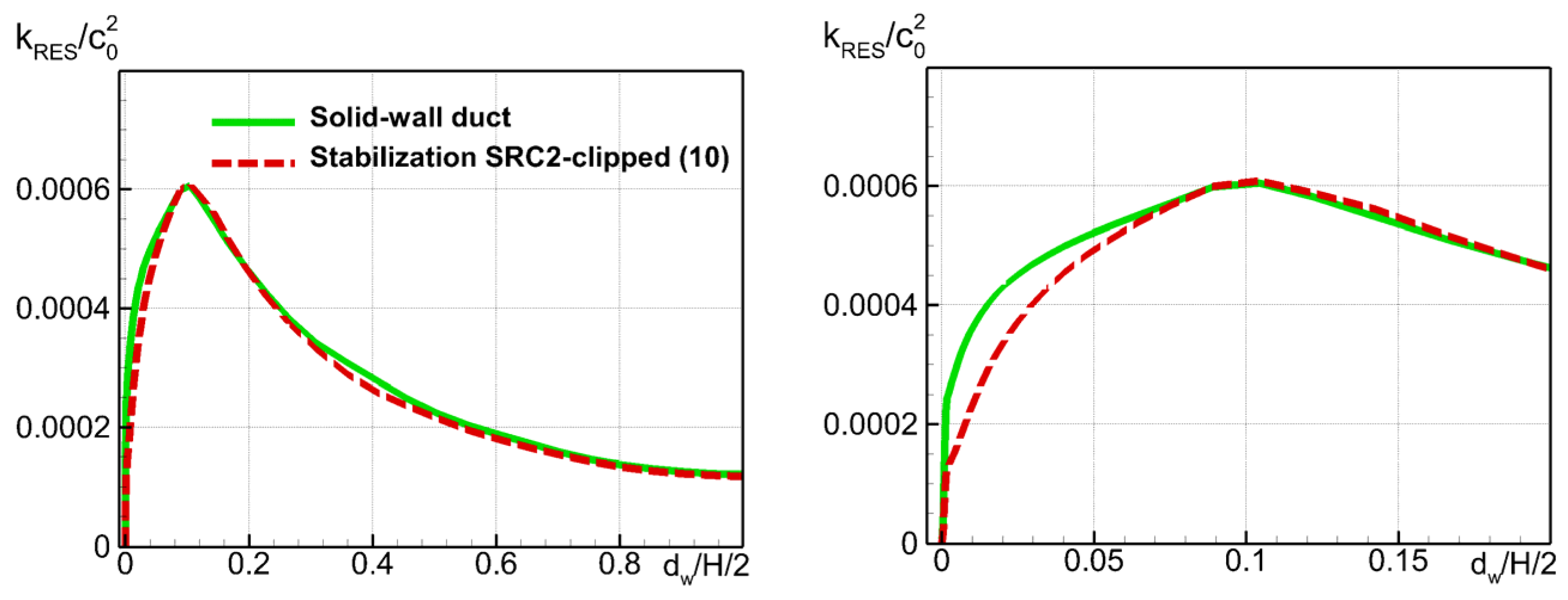
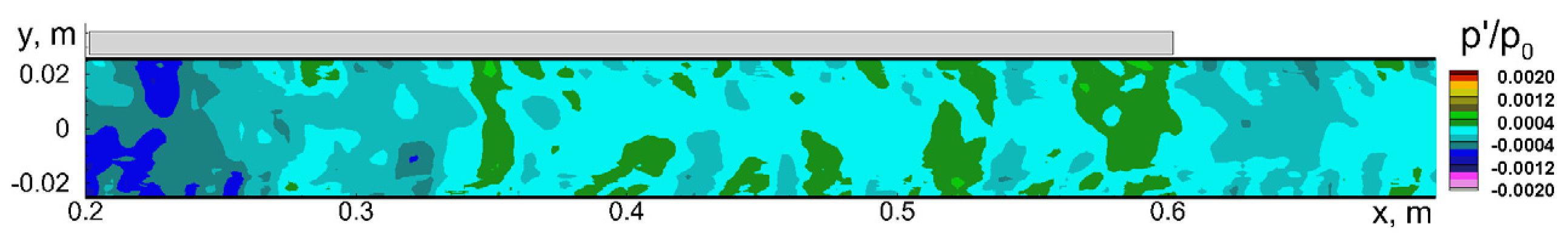
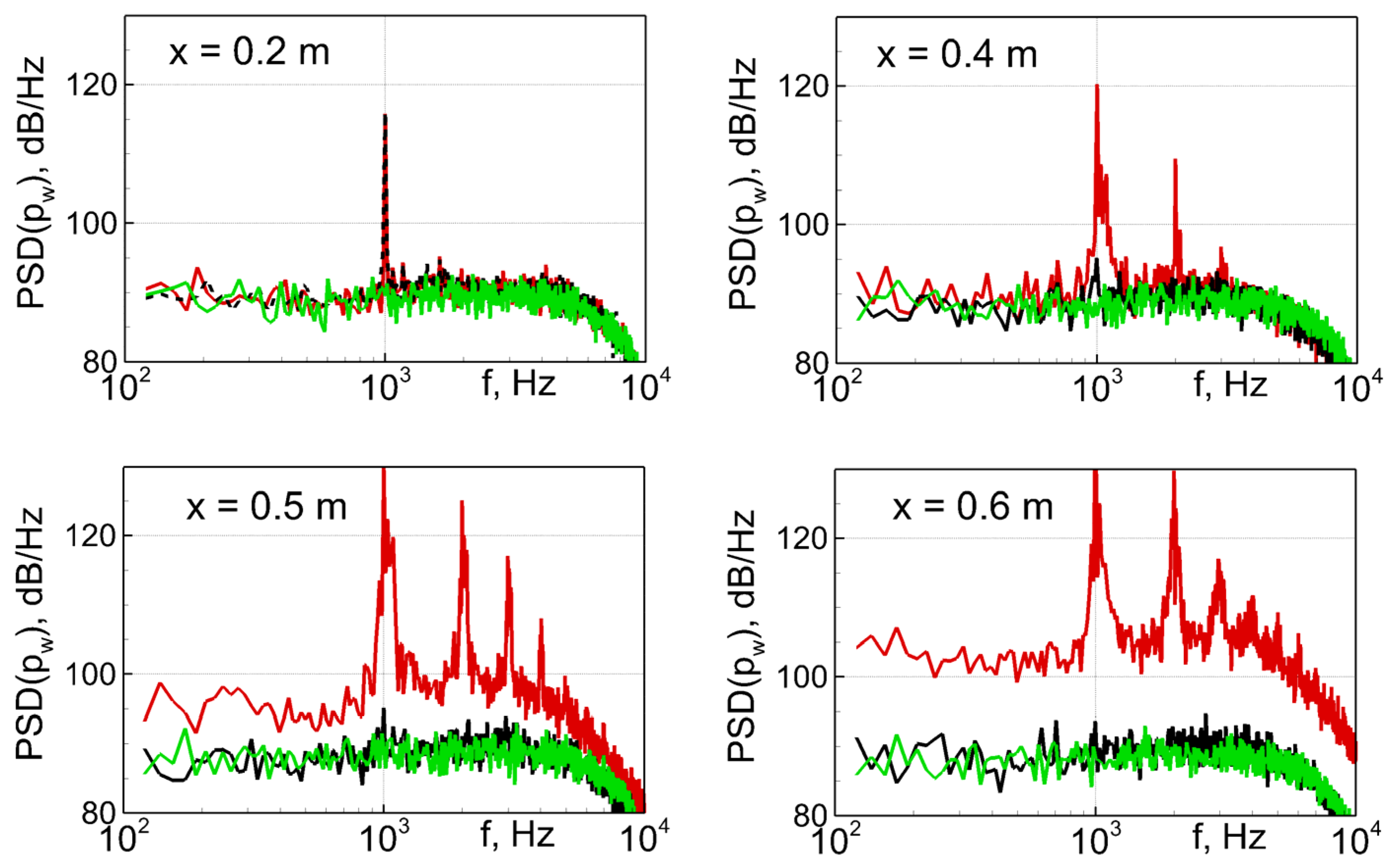
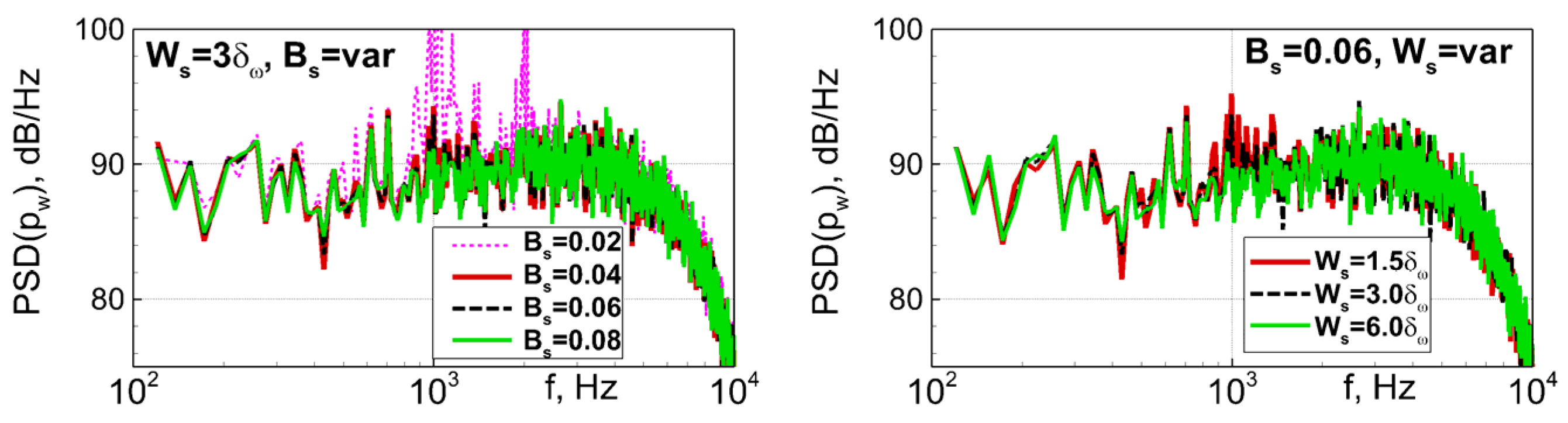
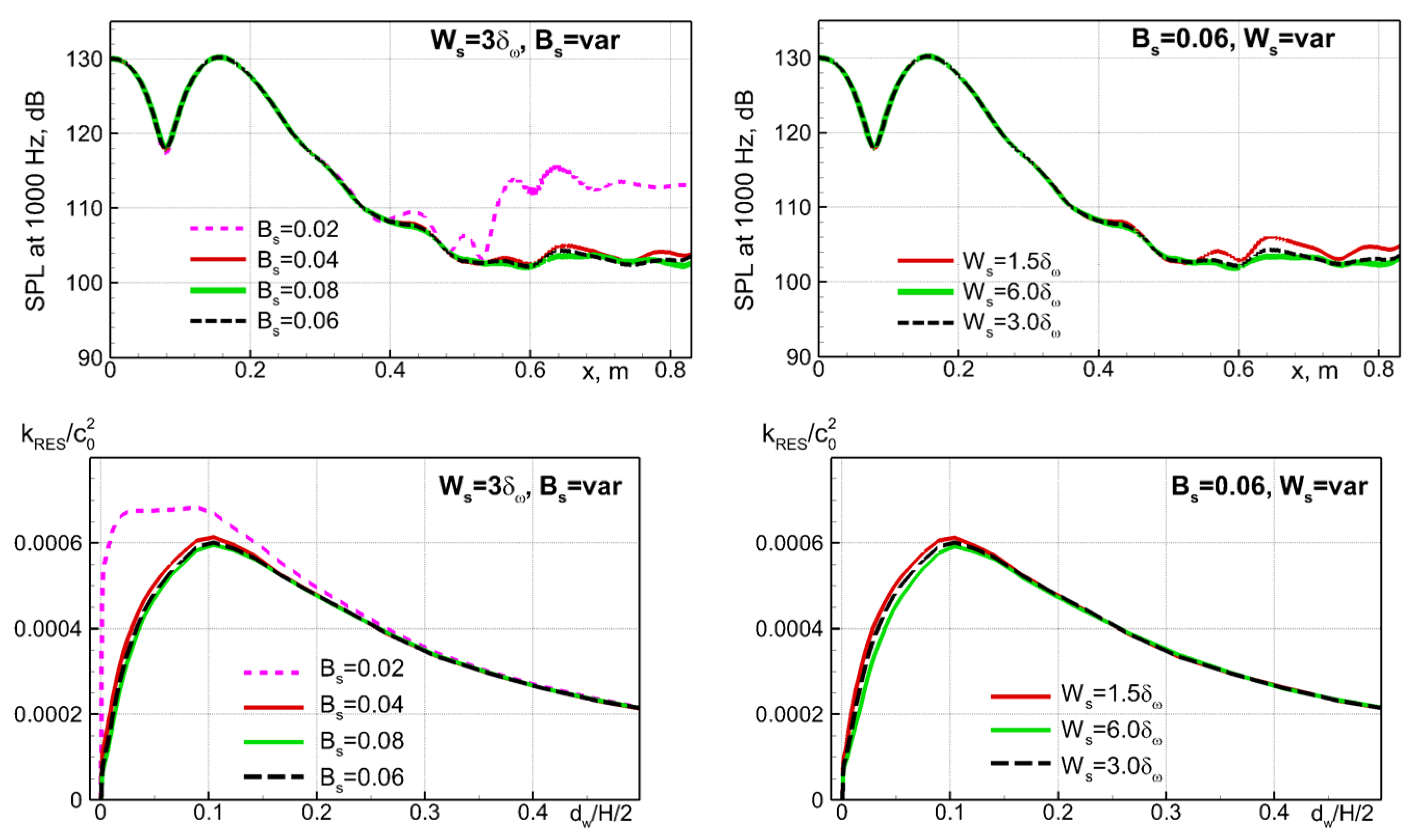
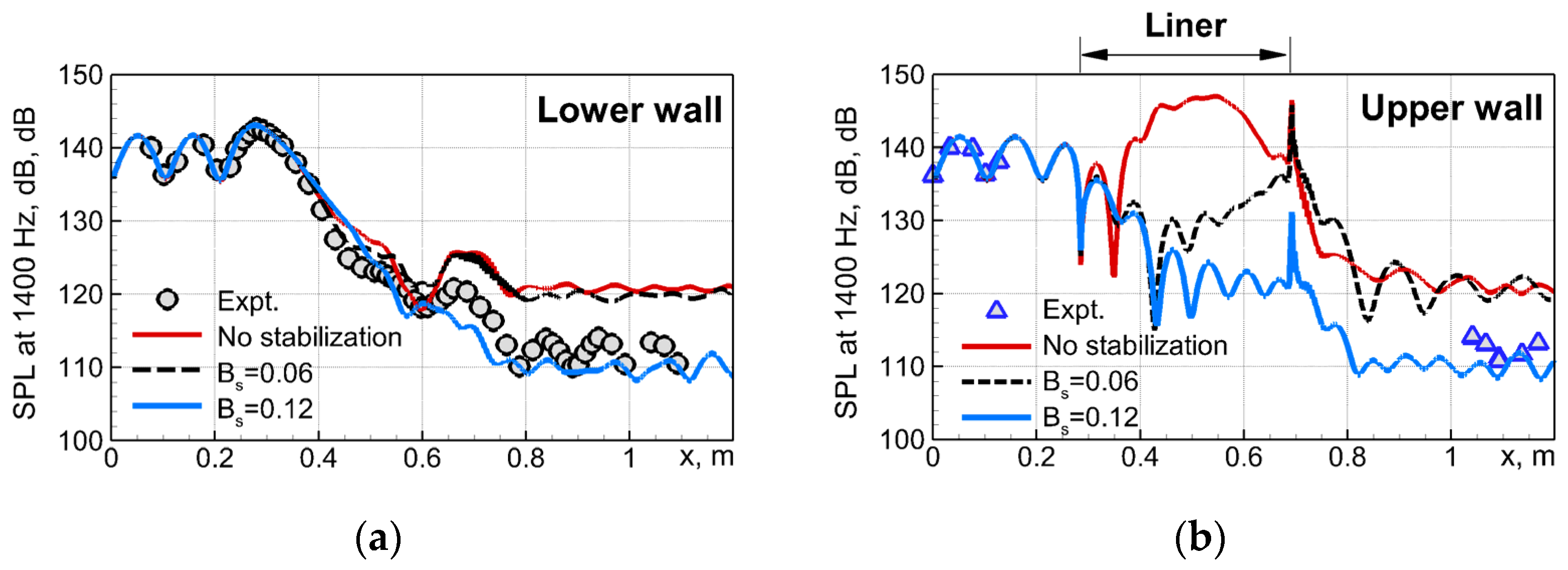
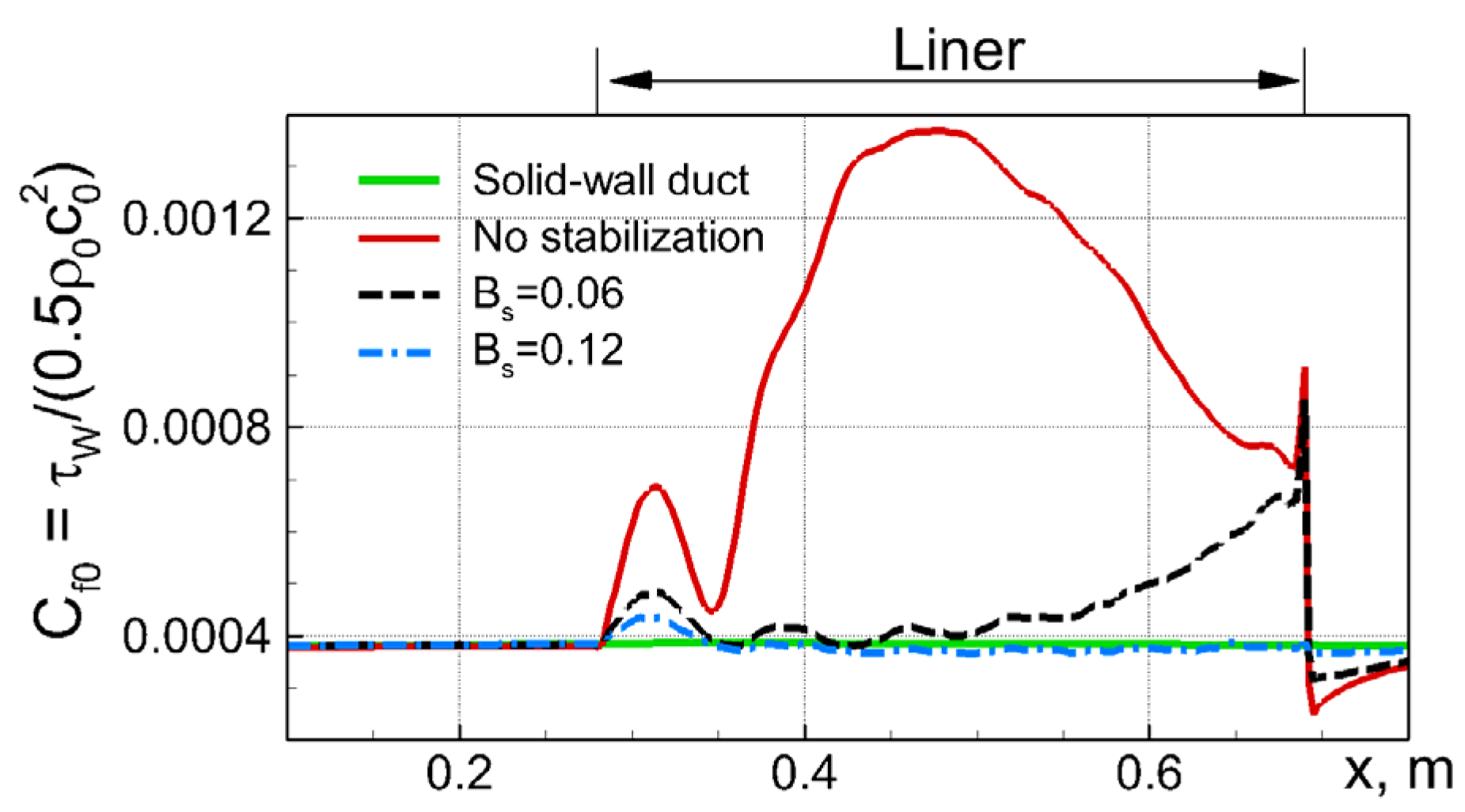
3.3. Calibration of the Body Force Parameters and Its Effect on Propagation of Sound Waves at Non-Resonant Frequencies
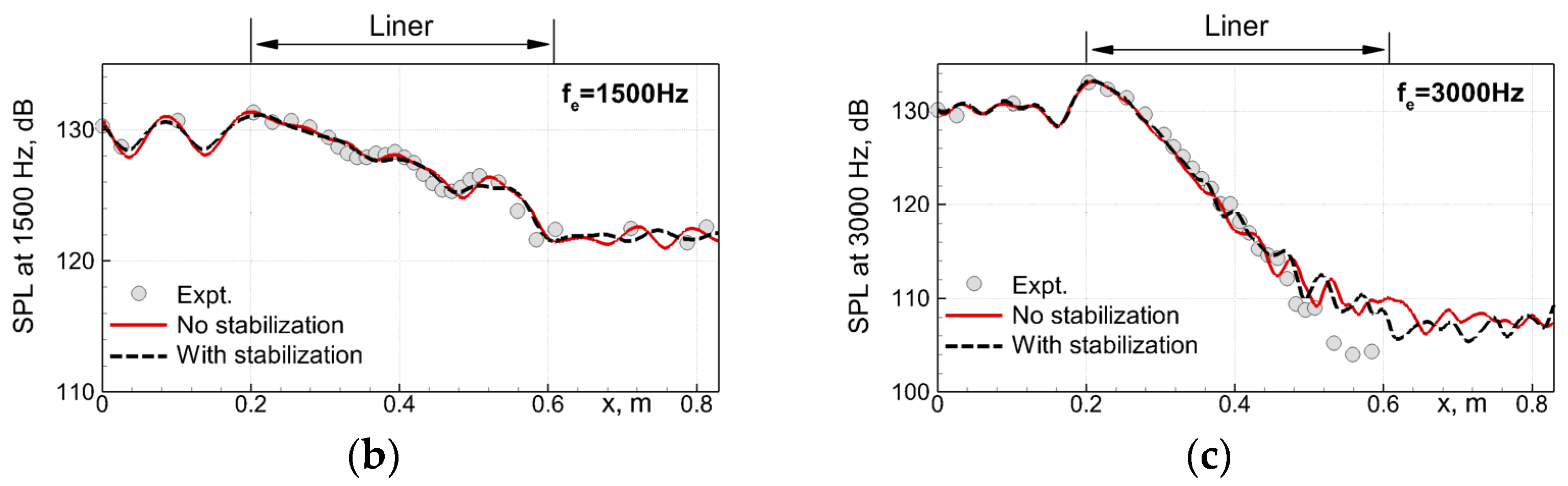
4. Application to an Alternative (SDoF) Liner
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Eversman, W. Theoretical Models for Duct Acoustic Propagation and Radiation. In Aeroacoustics of Flight Vehicles: Theory and Practice, Noise Control; NASA RP-1258; NASA Langley Research Center: Hampton, VA, USA, 1991; Volume 2, pp. 101–163. [Google Scholar]
- Motsinger, R.E.; Kraft, R.E. Design and Performance of Duct Acoustic Treatment. In Aeroacoustics of Flight Vehicles: Theory and Practice, Noise Control; NASA RP-1258; NASA Langley Research Center: Hampton, VA, USA, 1991; Volume 2, pp. 165–206. [Google Scholar]
- Rienstra, S.W.; Hirschberg, A. An Introduction to Acoustics. Ph.D. Thesis, Eindhoven University of Technology, Eindhoven, The Netherlands, 2019. [Google Scholar]
- Myers, M.K. On the Acoustic Boundary Condition in the Presence of Flow. J. Sound Vib. 1980, 71, 429–434. [Google Scholar] [CrossRef]
- Rienstra, S.W. Impedance Models in Time Domain, Including the Extended Helmholtz Resonator Model. In Proceedings of the 12th AIAA/CEAS Aeroacoustics Conference, Cambridge, MA, USA, 8–10 May 2006; AIAA Paper: Cambridge, MA, USA, 2006; p. 2686. [Google Scholar] [CrossRef]
- Tam, C.K.W.; Auriault, L. Time-Domain Impedance Boundary Conditions for Computational Aeroacoustics. AIAA J. 1996, 34, 917–923. [Google Scholar] [CrossRef]
- Reymen, Y.; Baelmans, M.; Desmet, W. Efficient Implementation of Tam and Auriault’s Time-Domain Impedance Boundary Condition. AIAA J. 2008, 46, 2368–2376. [Google Scholar] [CrossRef]
- Li, X.Y.; Li, X.D.; Tam, C.K.W. Improved Multipole Broadband Time-Domain Impedance Boundary Condition. AIAA J. 2012, 50, 980–984. [Google Scholar] [CrossRef]
- Ozyoruk, Y.; Long, L.N.; Jones, M.G. Time-Domain Numerical Simulation of a Flow-Impedance Tube. J. Comp. Phys. 1998, 146, 29–57. [Google Scholar] [CrossRef]
- Fung, K.-Y.; Ju, H. Broadband Time-Domain Impedance Models. AIAA J. 2001, 39, 1449–1454. [Google Scholar] [CrossRef]
- Dragna, D.; Pineau, P.; Blanc-Benon, P. A Generalized Recursive Convolution Method for Time-Domain Propagation in Porous Media. J. Acoust. Soc. Amer. 2015, 138, 1030–1042. [Google Scholar] [CrossRef]
- Troian, R.; Dragna, D.; Bailly, C.; Galland, M.-A. Broadband Liner Impedance Eduction for Multimodal Acoustic Propagation in the Presence of a Mean Flow. J. Sound Vib. 2017, 392, 200–216. [Google Scholar] [CrossRef]
- Shur, M.; Strelets, M.; Travin, A.; Suzuki, T.; Spalart, P. Unsteady Simulations of Sound Propagation in Turbulent Flow Inside a Lined Duct. AIAA J. 2021, 59, 3054–3070. [Google Scholar] [CrossRef]
- Jones, M.G.; Watson, W.R.; Parrott, T.L. Benchmark Data for Evaluation of Aeroacoustic Propagation Codes with Grazing Flow. In Proceedings of the 11th AIAA/CEAS Aeroacoustics Conference, Monterey, CA, USA, 23–25 May 2005; AIAA Paper: Monterey, CA, USA, 2005; p. 2853. [Google Scholar] [CrossRef]
- Shur, M.L.; Spalart, P.R.; Strelets, M.K.; Travin, A.K. A Hybrid RANS-LES Approach with Delayed-DES and Wall-Modelled LES Capabilities. Int. J. Heat Fluid Flow 2008, 29, 1638–1649. [Google Scholar] [CrossRef]
- Rienstra, S.W.; Vilenski, G.G. Spatial Instability of Boundary Layer Along Impedance Wall. In Proceedings of the 14th AIAA/CEAS Aeroacoustics Conference, Vancouver, BC, Canada, 5–7 May 2008; AIAA Paper: Vancouver, BC Canada, 2008; p. 2932. [Google Scholar] [CrossRef]
- Deng, Y.; Alomar, A.; Dragna, D.; Galland, M.-A. Characterization and Suppression of the Hydrodynamic Instability in the Time Domain for Acoustic Propagation in a Lined Flow Duct. J. Sound Vib. 2021, 500, 115999. [Google Scholar] [CrossRef]
- Dai, X.; Auregan, Y. A Cavity-by-Cavity Description of the Aeroacoustic Instability Over a Liner with a Grazing Flow. J. Fluid Mech. 2018, 852, 126–145. [Google Scholar] [CrossRef]
- Marx, D.; Auregan, Y.; Bailliet, H.; Valiere, J.-C. PIV and LDV Evidence of Hydrodynamic Instability over a Liner in a Duct Flow. J. Sound Vib. 2010, 329, 3798–3812. [Google Scholar] [CrossRef]
- Marx, D. Numerical Computation of a Lined Duct Instability Using the Linearized Euler Equations. AIAA J. 2015, 53, 2379–2388. [Google Scholar] [CrossRef]
- Rienstra, S.W.; Darau, M. Boundary-Layer Thickness Effects of the Hydrodynamic Instability along an Impedance Wall. J. Fluid Mech. 2011, 671, 559–573. [Google Scholar] [CrossRef]
- Dai, X.; Auregan, Y. Hydrodynamic Instability and Sound Amplification Over a Perforated Plate Backed by a Cavity. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019; AIAA Paper: Delft, The Netherlands, 2019; p. 2703. [Google Scholar] [CrossRef]
- Marx, D.; Sebastian, R.; Fortune, V. Spatial Numerical Simulation of a Turbulent Plane Channel Flow with an Impedance Wall. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019; AIAA Paper: Delft, The Netherlands, 2019; p. 2543. [Google Scholar] [CrossRef]
- Shur, M.; Strelets, M.; Travin, A.; Suzuki, T.; Spalart, P.R. Unsteady Simulation of Sound Propagation in Turbulent Flow Inside a Lined Duct Using a Broadband Time-Domain Impedance Model. In Proceedings of the AIAA Aviation 2020 Forum Virtual Event, Virtual, 15–19 June 2020; AIAA Paper: Delft, The Netherlands, 2020; p. 2535. [Google Scholar] [CrossRef]
- Marx, D.; Auregan, Y. Effect of Turbulent Eddy Viscosity on the Unstable Surface Mode above an Acoustic Liner. J. Sound Vib. 2013, 332, 3803–3820. [Google Scholar] [CrossRef]
- Sebastien, R.; Marx, D.; Fortune, V. Numerical Simulation of a Turbulent Channel Flow with an Acoustic Liner. J. Sound Vib. 2019, 456, 306–330. [Google Scholar] [CrossRef]
- Richter, C.; Thiele, F.H.; Li, X.; Zhuang, M. Comparison of Time-Domain Impedance Boundary Conditions for Lined Duct Flows. AIAA J. 2007, 45, 1333–1345. [Google Scholar] [CrossRef]
- Burak, M.O.; Billson, M.; Eriksson, L.-E.; Baralon, S. Validation of a Time- and Frequency-Domain Grazing Flow Acoustic Liner Model. AIAA J. 2009, 47, 1841–1848. [Google Scholar] [CrossRef]
- Deng, Y.; Dragna, D.; Galland, M.-A.; Alomar, A. Comparison of Three Numerical Methods for Acoustic Propagation in a Lined Duct with Flow. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019; AIAA Paper: Delft, The Netherlands, 2019; p. 2658. [Google Scholar] [CrossRef]
- Bogey, C.; Bailly, C.; Juve, D. Computation of Flow Noise Using Source Terms in Linearized Euler’s Equations. AIAA J. 2002, 40, 235–243. [Google Scholar] [CrossRef]
- Shur, M.; Strelets, M.; Travin, A.; Suzuki, T. Further Evaluation of Prediction Capability of the Broadband Time-Domain Impedance Model for Sound Propagation in Turbulent Grazing Flow. In Proceedings of the AIAA Aviation 2021 Forum Virtual Event, Virtual, 2–6 August 2021; AIAA Paper: Delft, The Netherlands, 2021; p. 2171. [Google Scholar] [CrossRef]
- Howerton, B.M.; Jones, M.G. A Conventional Liner Acoustic/Drag Interaction Benchmark Database. In Proceedings of the 23rd AIAA/CEAS Aeroacoustics Conference, Denver, CO, USA, 5–9 June 2017; AIAA Paper: Denver, CO, USA, 2017; p. 4190. [Google Scholar] [CrossRef]
- Zheng, S.; Zhuang, M. Verification and Validation of Time-Domain Impedance Boundary Condition in Lined Ducts. AIAA J. 2005, 43, 306–313. [Google Scholar] [CrossRef]
- Parrott, T.L.; Watson, W.R.; Jones, M.G. Experimental Validation of a Two-Dimensional Shear-Flow Model for Determining Acoustic Impedance; NASA TP: Washington, DC, USA, 1987; p. 2679. [Google Scholar]
- Shur, M.; Strelets, M.; Travin, A.; Probst, A.; Probst, S.; Schwamborn, D.; Deck, S.; Skillen, A.; Holgate, J.; Revell, A. Improved Embedded Approaches. In Go4Hybrid: Grey Area Mitigation for Hybrid RANS-LES Methods, Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Springer: New York, NY, USA, 2017; Volume 134, pp. 51–87. [Google Scholar] [CrossRef]
- Shur, M.L.; Spalart, P.R.; Strelets, M.K. Noise prediction for increasingly complex jets. Part 1: Methods and tests. Int. J. Aeroacoustics 2005, 4, 213–245. [Google Scholar] [CrossRef]
- Gustavsen, B.; Semlyen, A. Rational Approximation of Frequency Domain Responses by Vector Fitting. IEEE Trans. Power Deliv. 1999, 14, 1052–1061. [Google Scholar] [CrossRef]
- Gustavsen, B. Improving the Pole Relocating Properties of Vector Fitting. IEEE Trans. Power Deliv. 2006, 21, 1587–1592. [Google Scholar] [CrossRef]
- Deschrijver, D.; Mrozowski, M.; Dhaene, T.; De Zutter, D. Macromodeling of Multiport Systems Using a Fast Implementation of the Vector Fitting Method. IEEE Microw. Wirel. Compon. Lett. 2008, 18, 383–385. [Google Scholar] [CrossRef]
- Zhang, Q.; Bodony, D.J. Numerical Investigation of a Honeycomb Liner Grazed by Laminar and Turbulent Boundary Layers. J. Fluid Mech. 2016, 792, 936–980. [Google Scholar] [CrossRef]
- Leon, O.; Mery, F.; Piot, E.; Conte, C. Near-wall Aerodynamic Response of an Acoustic Liner to Harmonic Excitation with Grazing Flow. Exp. Fluid 2019, 60, 144. [Google Scholar] [CrossRef]
- Qui, X.; Xin, B.; Wu, L.; Meng, Y.; Jing, X. Investigation of Straightforward Impedance Eduction Method on Single-Degree-of-Freedom Acoustic Liners. Chin. J. Aeronaut. 2018, 31, 2221–2233. [Google Scholar] [CrossRef]
- Shur, M.; Strelets, M.; Travin, A. High-order Implicit Multi-block Navier-Stokes Code: Ten-year Experience of Application to RANS/DES/LES/DNS of Turbulent Flows. In Proceedings of the 7th Symposium on Overset Composite Grids & Solution Technology, Huntington Beach, CA, USA; 2004. Available online: https://cfd.spbstu.ru//agarbaruk/doc/NTS_code.pdf (accessed on 11 April 2023).
- Roe, P.L. Approximate Riemann Solvers, Parameter Vectors and Difference Schemes. J. Comput. Phys. 1981, 43, 357–372. [Google Scholar] [CrossRef]
- Howerton, B.M.; Jones, M.G. Acoustic Liner Drag: Measurements on Novel Facesheet Perforate Geometries. In Proceedings of the 22nd AIAA/CEAS Aeroacoustics Conference, Lyon, France, 30 May–1 June 2016; AIAA Paper: Lyon, France, 2016; p. 2979. [Google Scholar] [CrossRef]
- Spalart, P.R.; Garbaruk, A.V.; Howerton, B.M. CFD Analysis of an Installation Used to Measure the Skin-Friction Penalty of Acoustic Treatments. In Proceedings of the 23rd AIAA/CEAS Aeroacoustics Conference, Denver, CO, USA, 5–9 June 2017; AIAA Paper: Denver, CO, USA, 2017; p. 3691. [Google Scholar] [CrossRef]


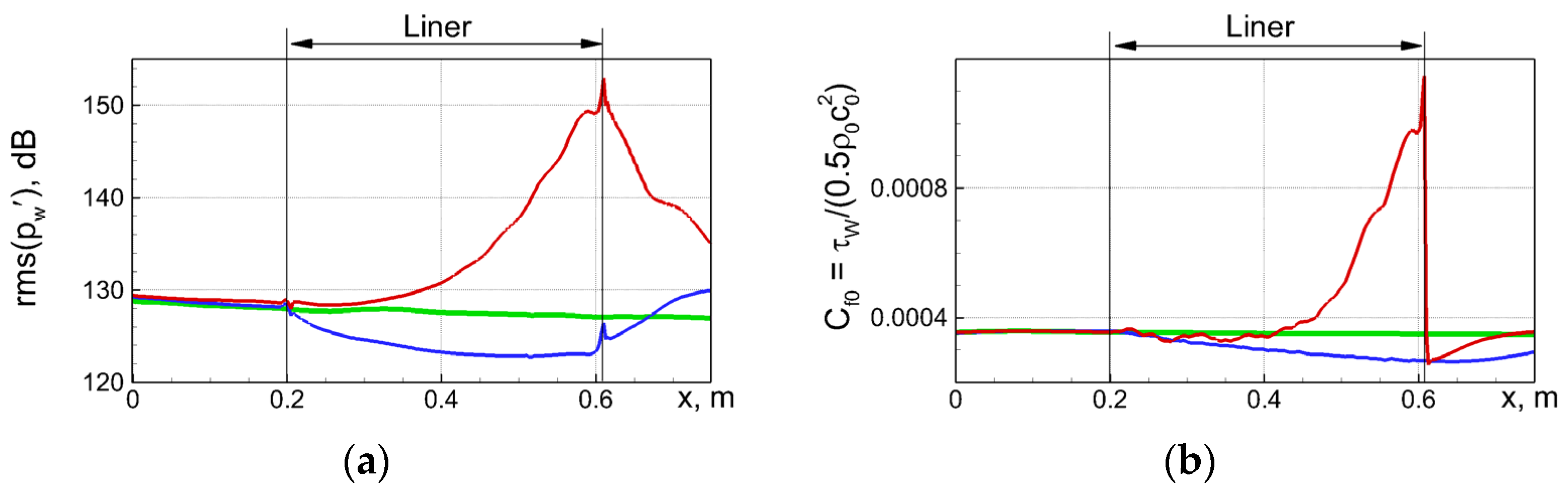
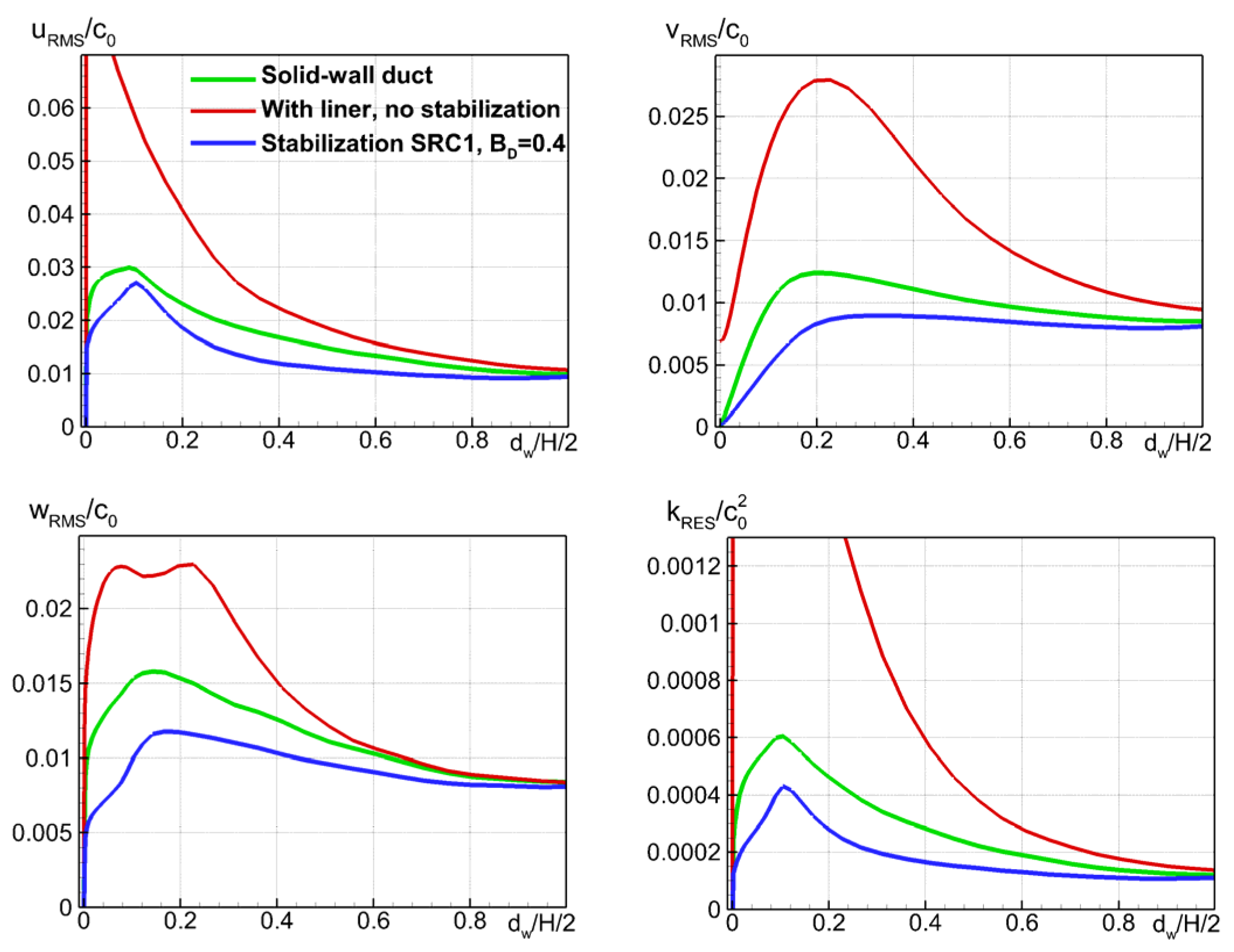
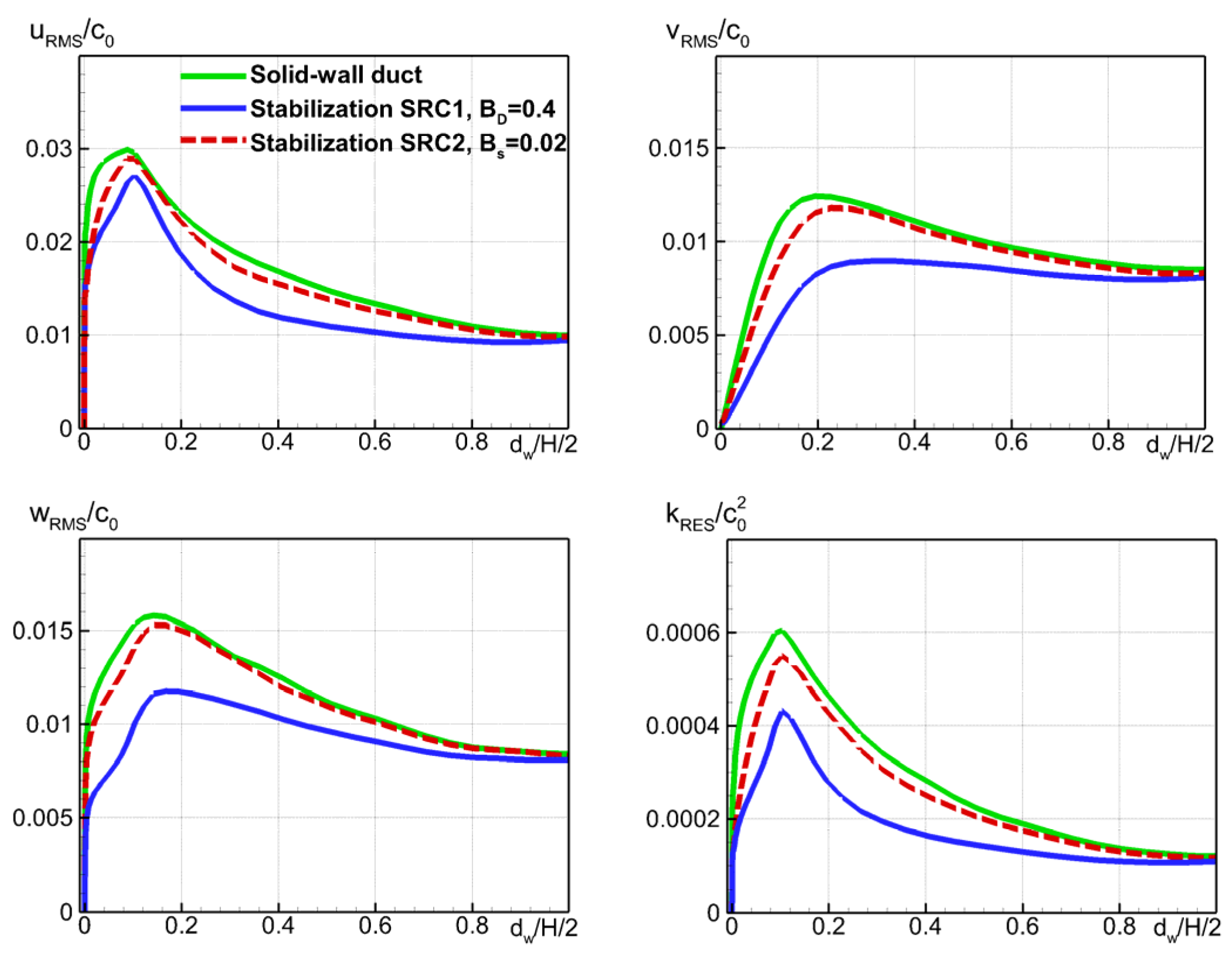
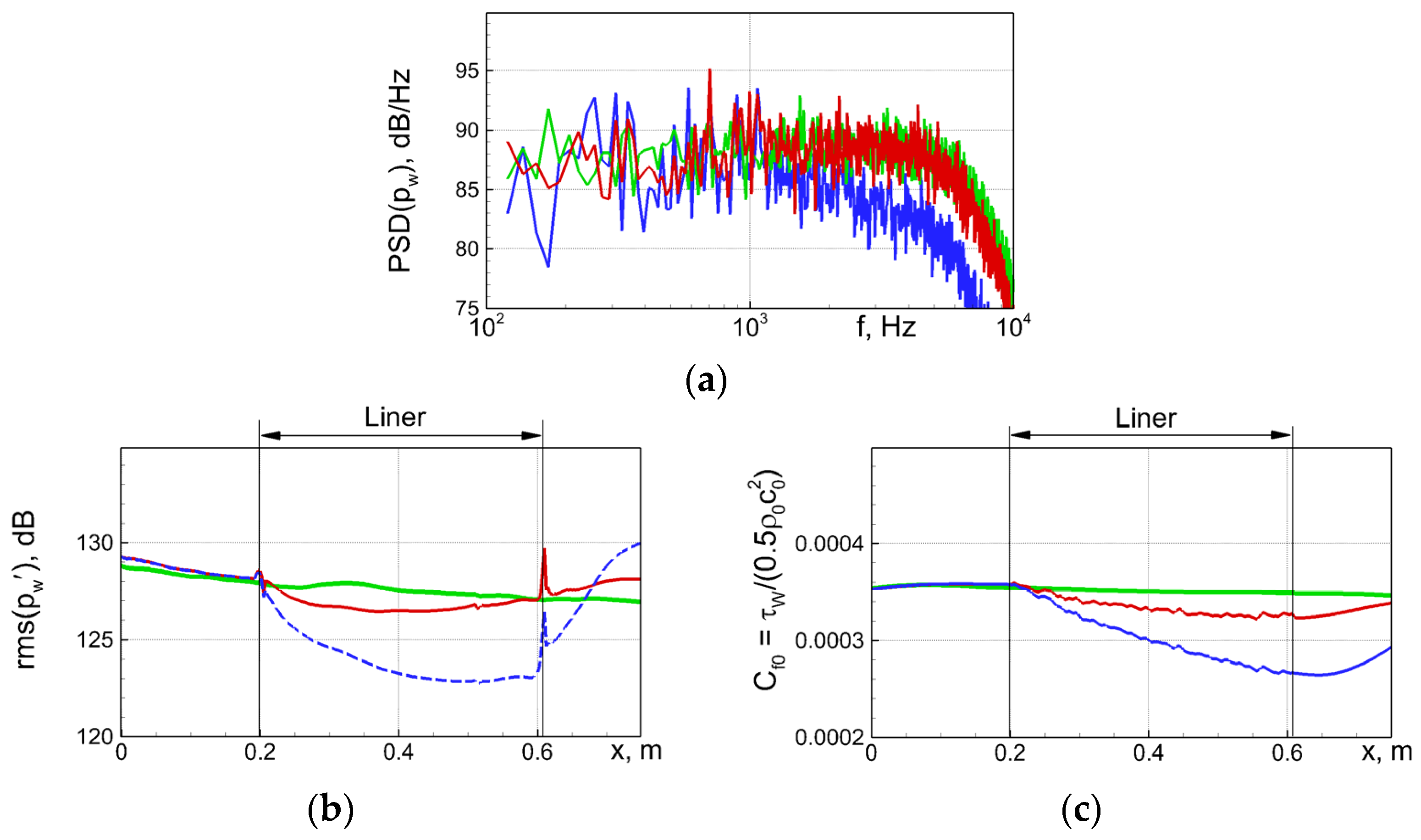





| f, Hz | k1 | k2 | ||||
|---|---|---|---|---|---|---|
| Unchanged Equations | with SRC1 | with SRC2 | Unchanged Equations | with SRC1 | with SRC2 | |
| 500 | 0.235 + 0.020i | 0.242 + 0.021i | 0.238 + 0.023i | −0.642 − 0.114i | −0.593 − 0.096i | −0.621 − 0.100i |
| 1000 | 0.432 + 0.281i | 0.431 + 0.323i | 0.427 + 0.295i | −0.406 − 0.486i | −0.432 − 0.478i | −0.415 − 0.489i |
| 1500 | 0.449 + 0.058i | 0.443 + 0.062i | 0.447 + 0.058i | −0.863 − 0.110i | −0.877 − 0.103i | −0.868 − 0.111i |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shur, M.; Strelets, M.; Travin, A. Suppression of the Spatial Hydrodynamic Instability in Scale-Resolving Simulations of Turbulent Flows Inside Lined Ducts. Fluids 2023, 8, 134. https://doi.org/10.3390/fluids8040134
Shur M, Strelets M, Travin A. Suppression of the Spatial Hydrodynamic Instability in Scale-Resolving Simulations of Turbulent Flows Inside Lined Ducts. Fluids. 2023; 8(4):134. https://doi.org/10.3390/fluids8040134
Chicago/Turabian StyleShur, Mikhail, Mikhail Strelets, and Andrey Travin. 2023. "Suppression of the Spatial Hydrodynamic Instability in Scale-Resolving Simulations of Turbulent Flows Inside Lined Ducts" Fluids 8, no. 4: 134. https://doi.org/10.3390/fluids8040134
APA StyleShur, M., Strelets, M., & Travin, A. (2023). Suppression of the Spatial Hydrodynamic Instability in Scale-Resolving Simulations of Turbulent Flows Inside Lined Ducts. Fluids, 8(4), 134. https://doi.org/10.3390/fluids8040134