Influence of Morphological Parameters on the Flow Development within Human Airways
Abstract
1. Introduction
2. Methodology
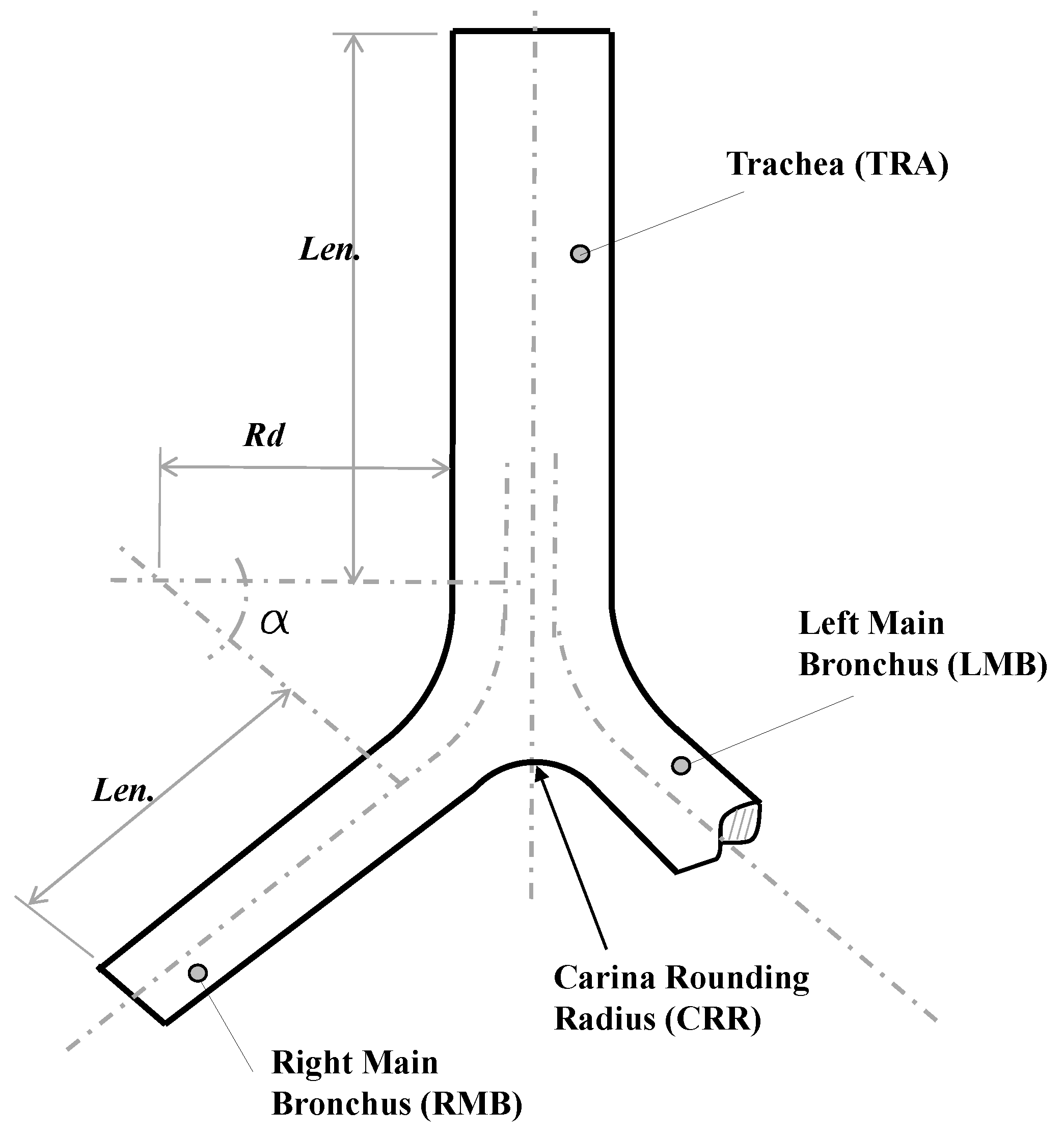
2.1. Bifurcation Angle (BA). Morphological Definition
2.2. Carina Rounding Radius (CRR). Morphological Definition
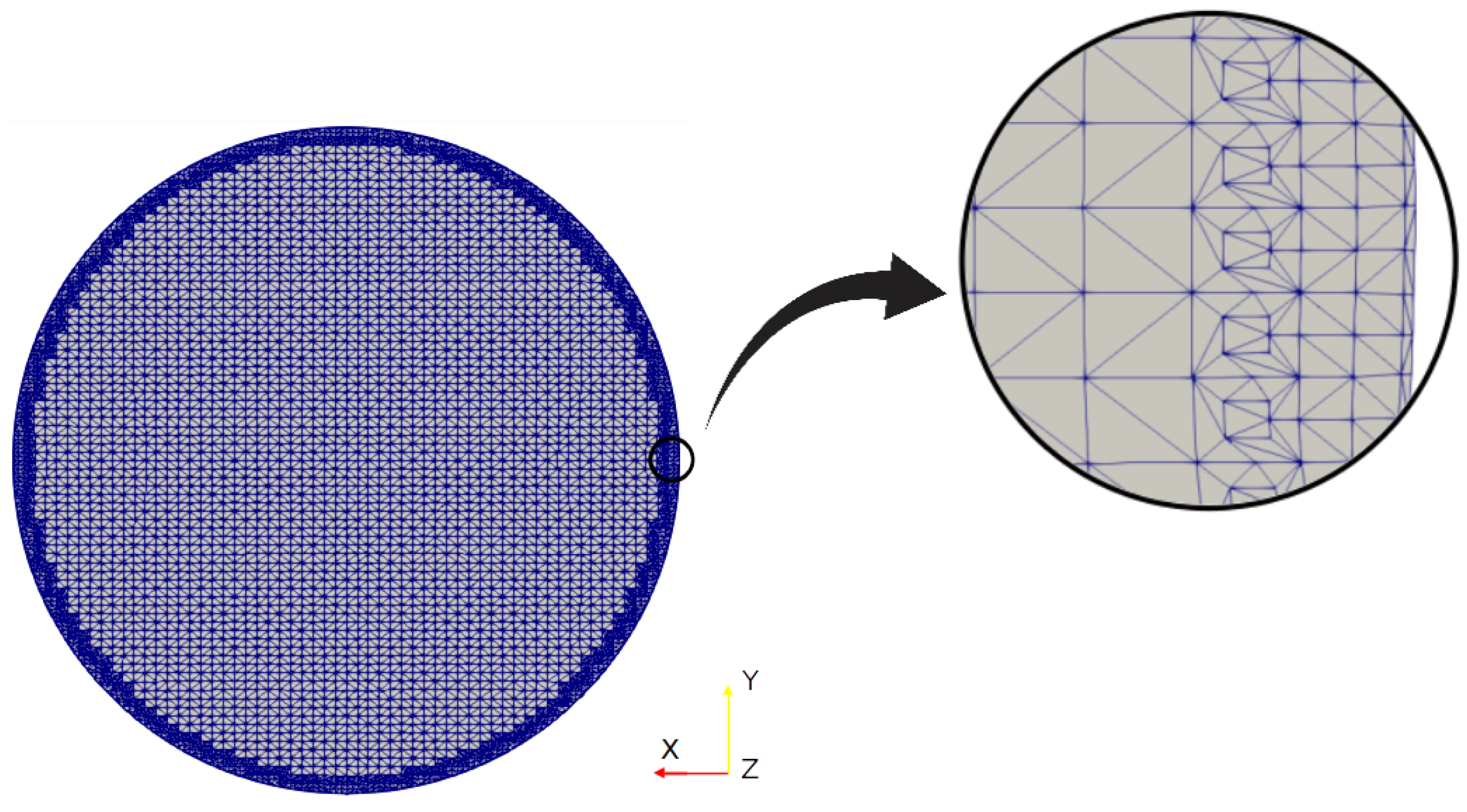
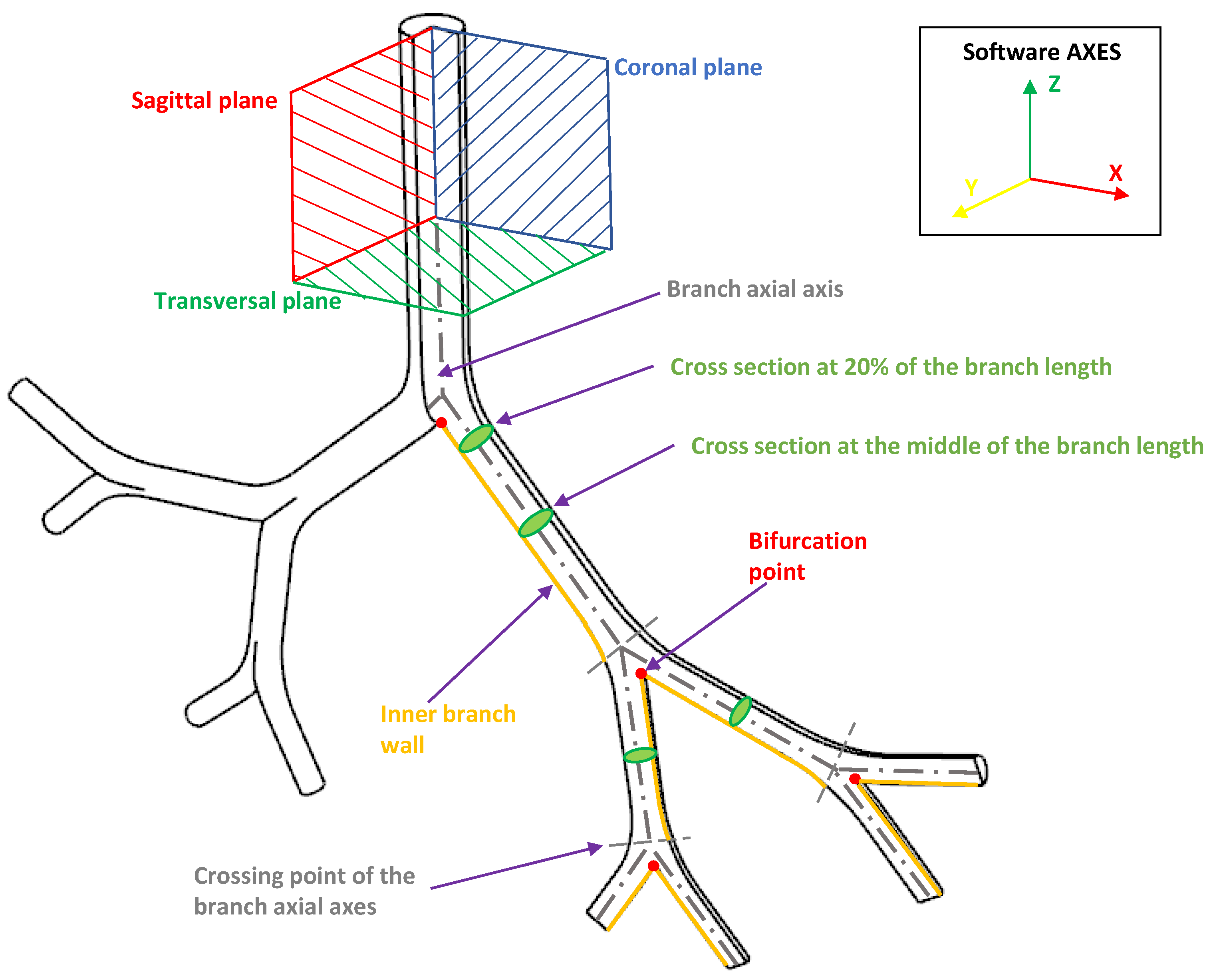
2.3. Governing Equations and Computational Model
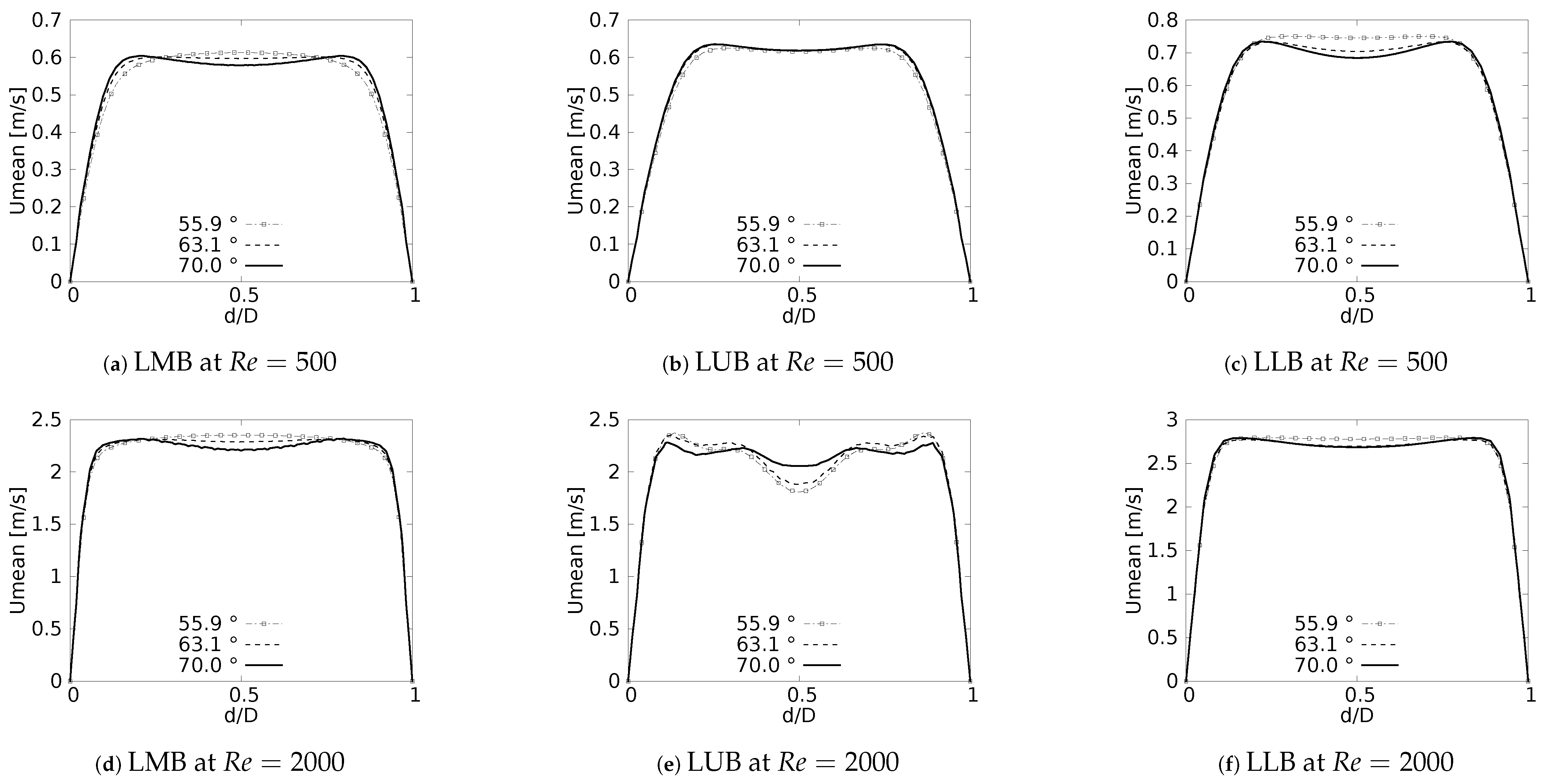
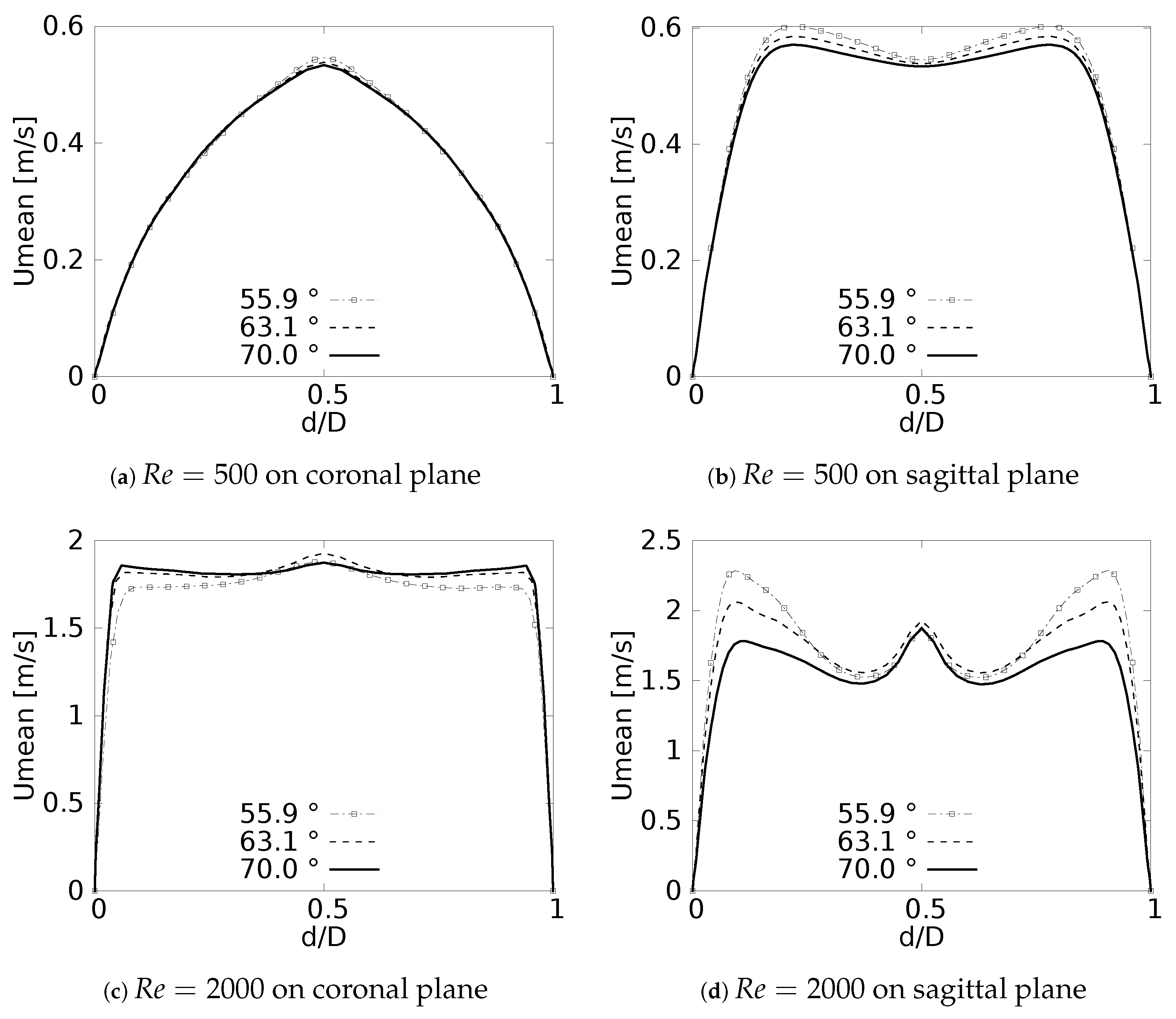
2.4. Validation of the Numerical Model
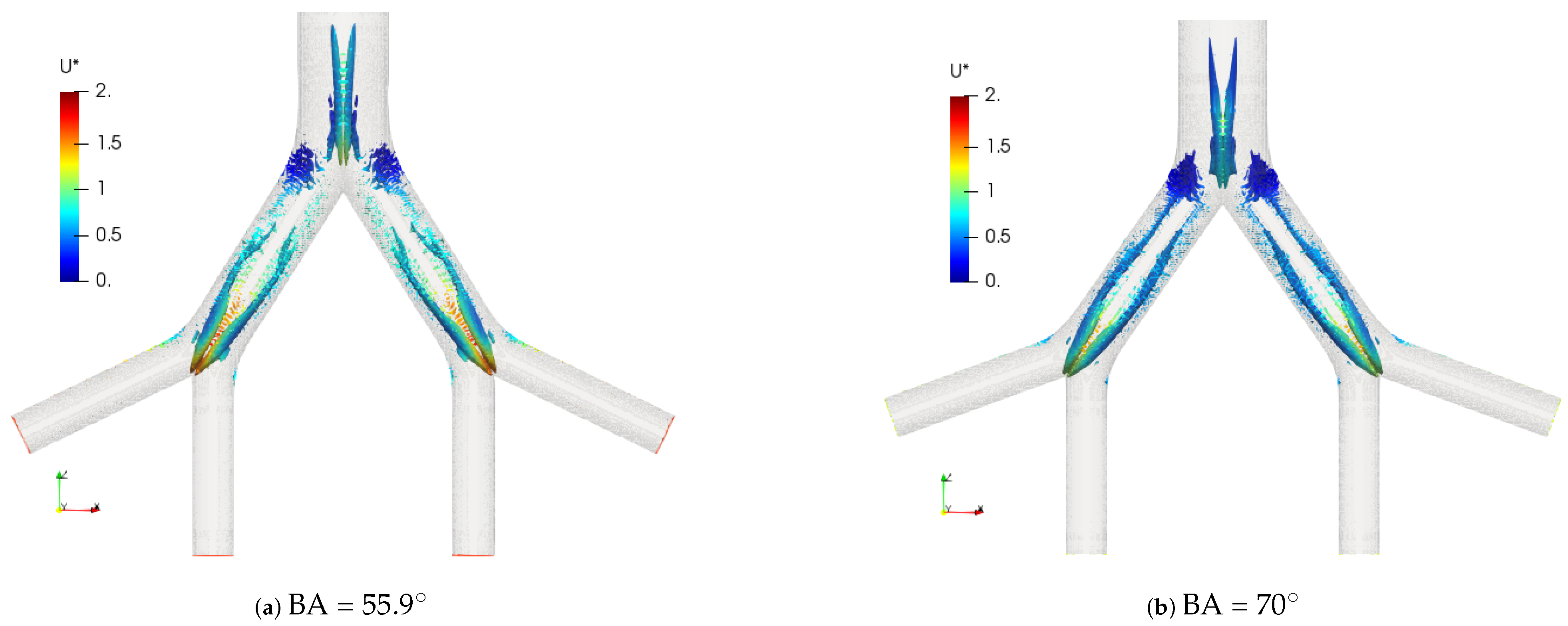
3. Results I. Effect of Variations of the Bifurcation Angle (BA)
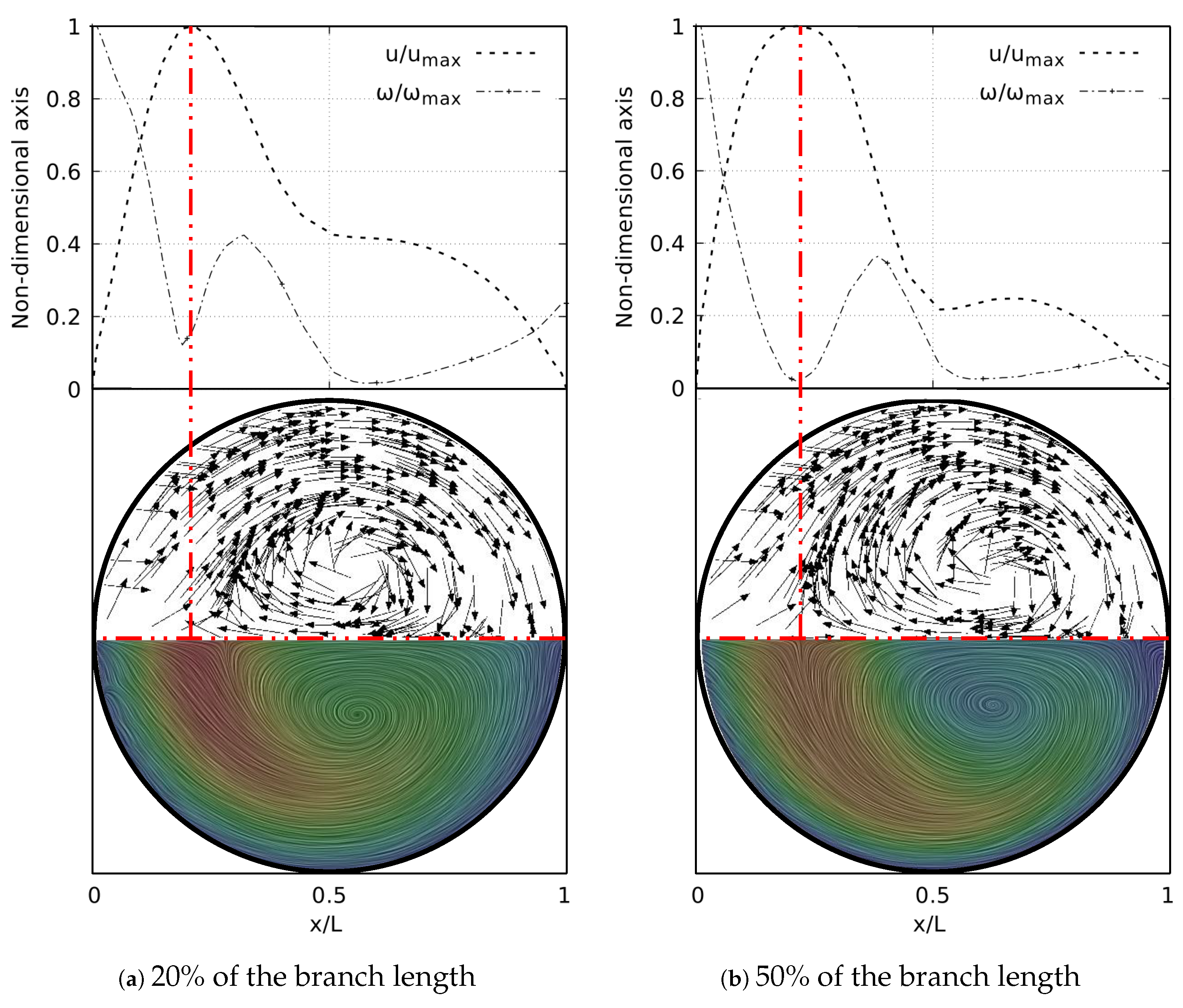
3.1. Velocity Profiles
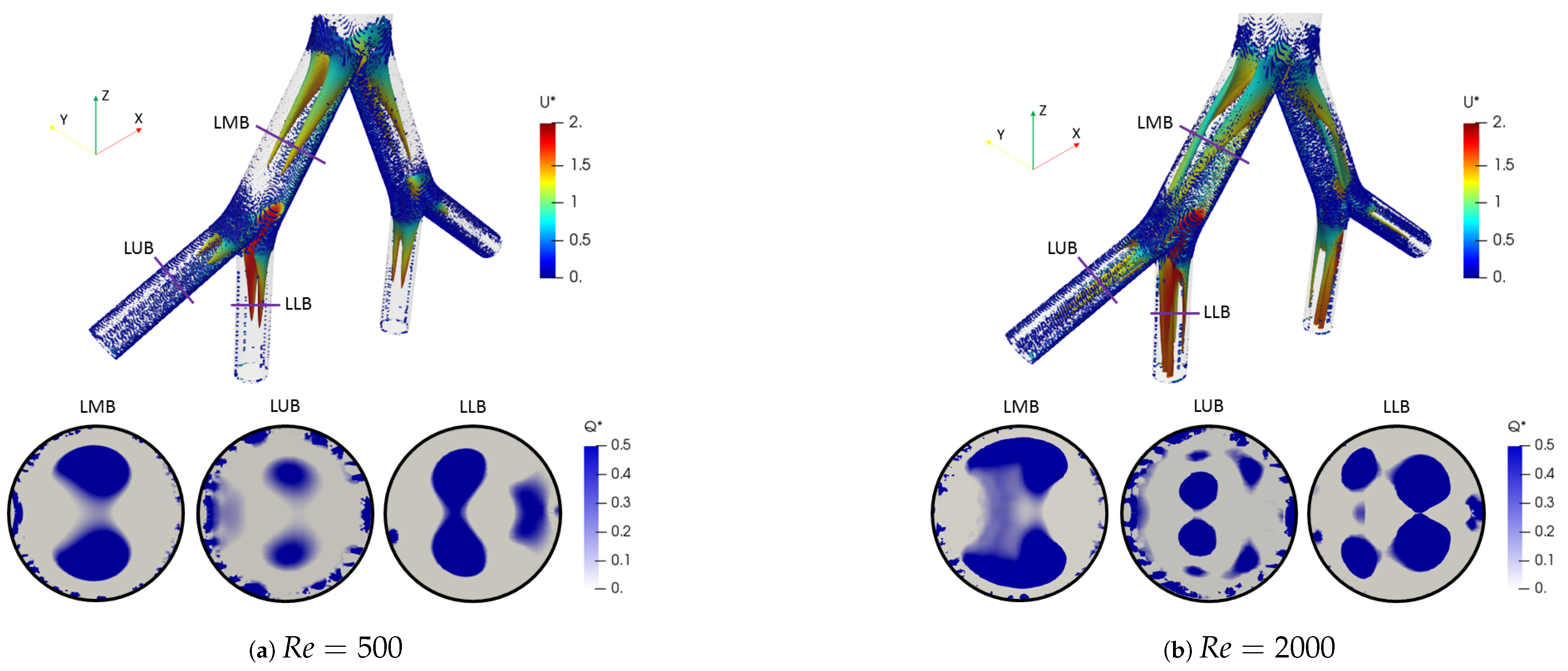
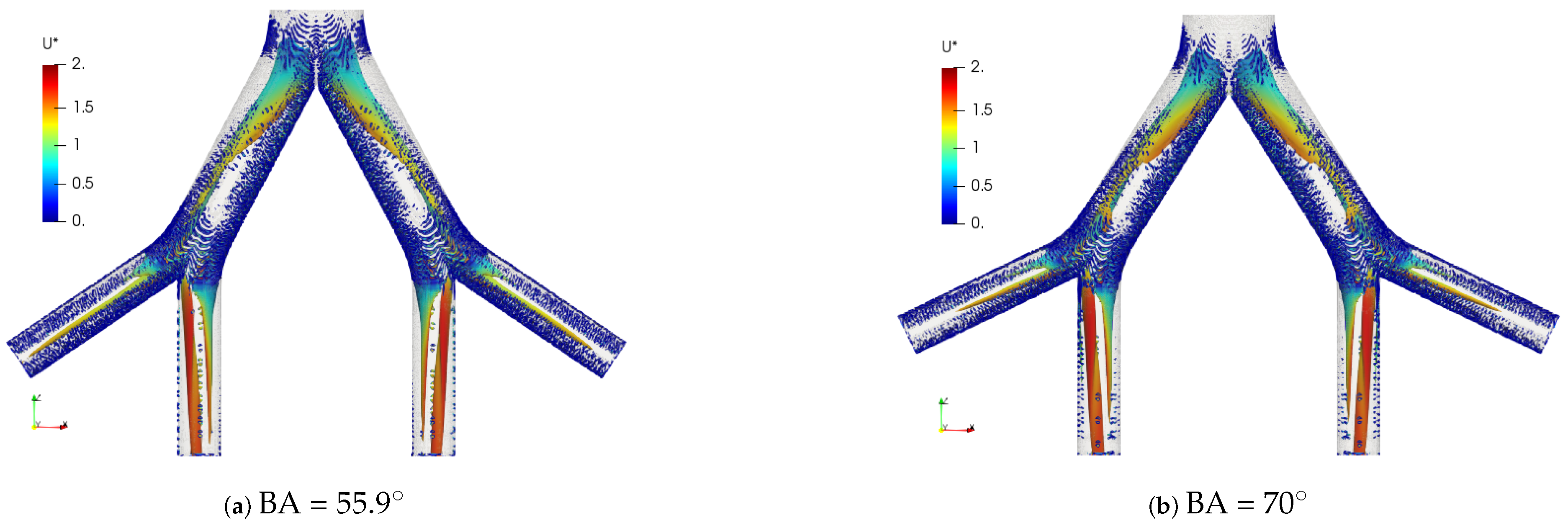
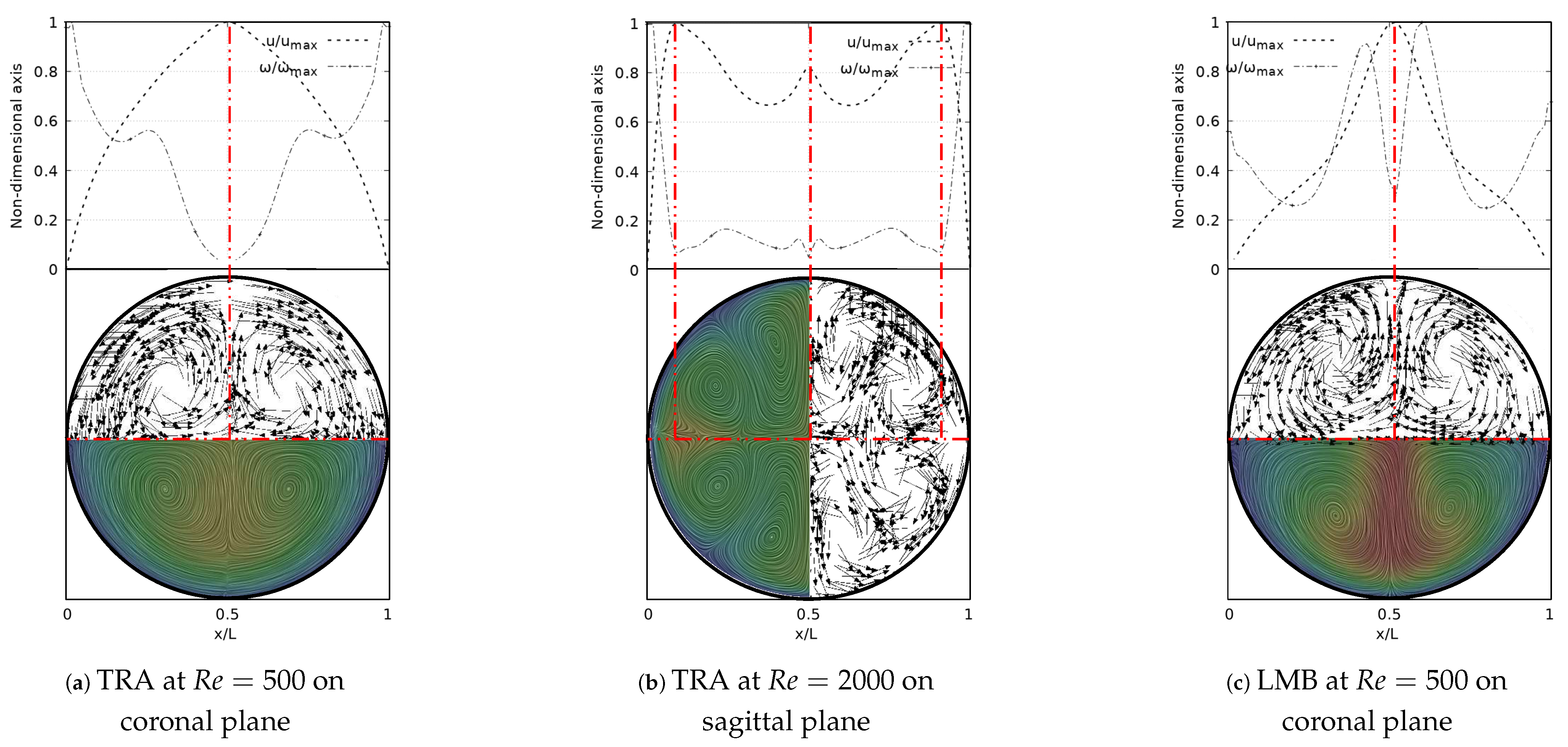
3.2. Flow Patterns
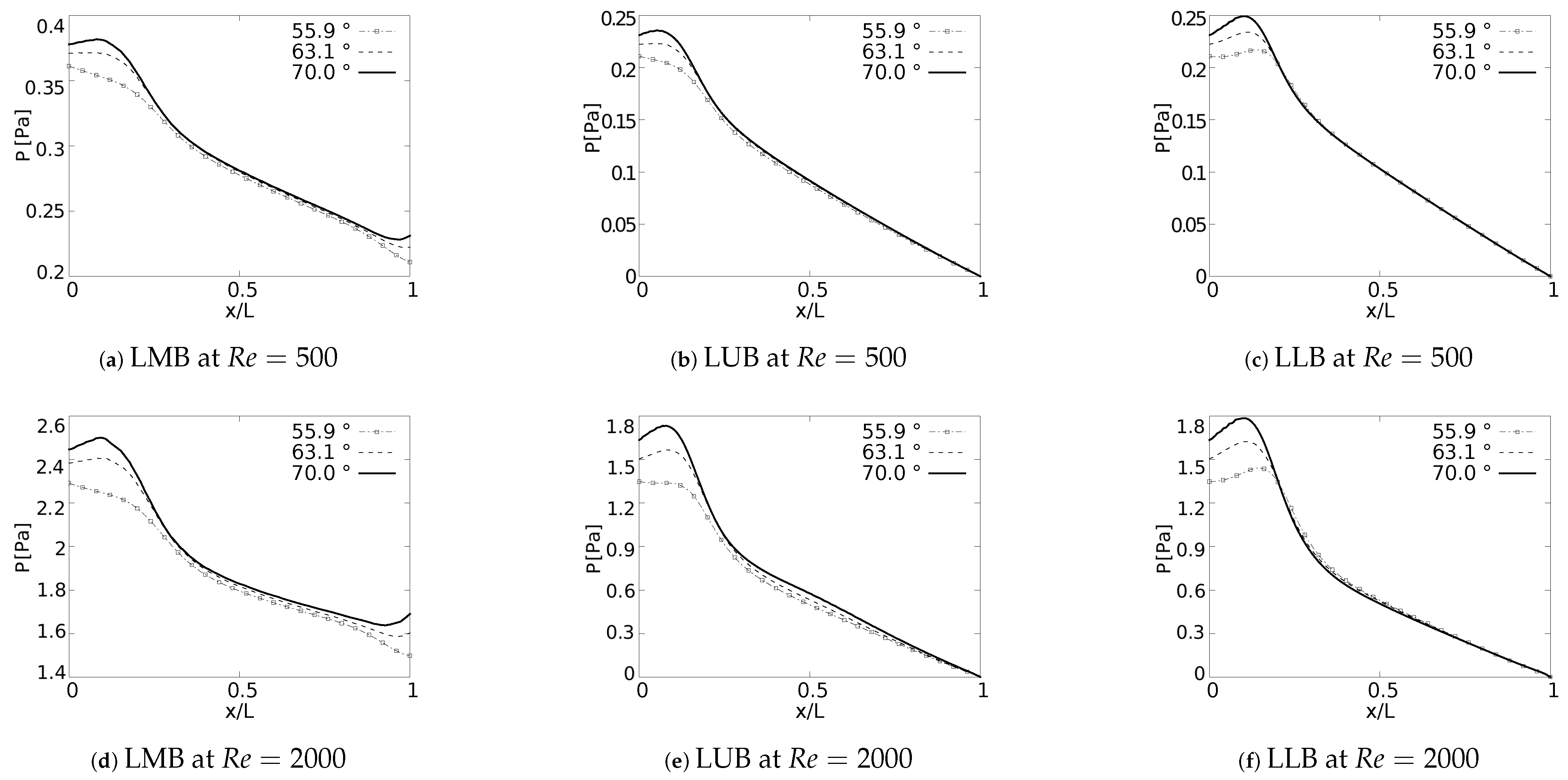
3.3. Pressure Behaviour
3.4. Wall Shear Stresses
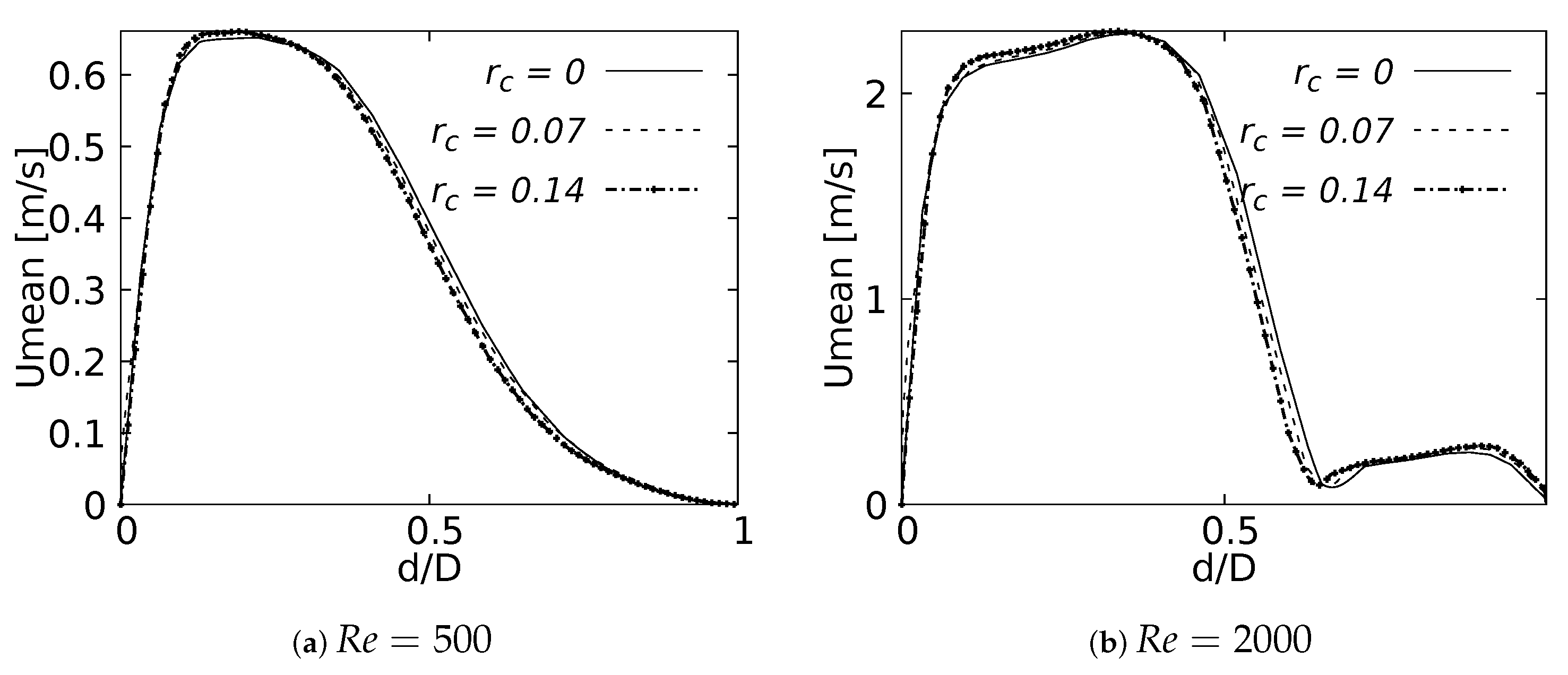
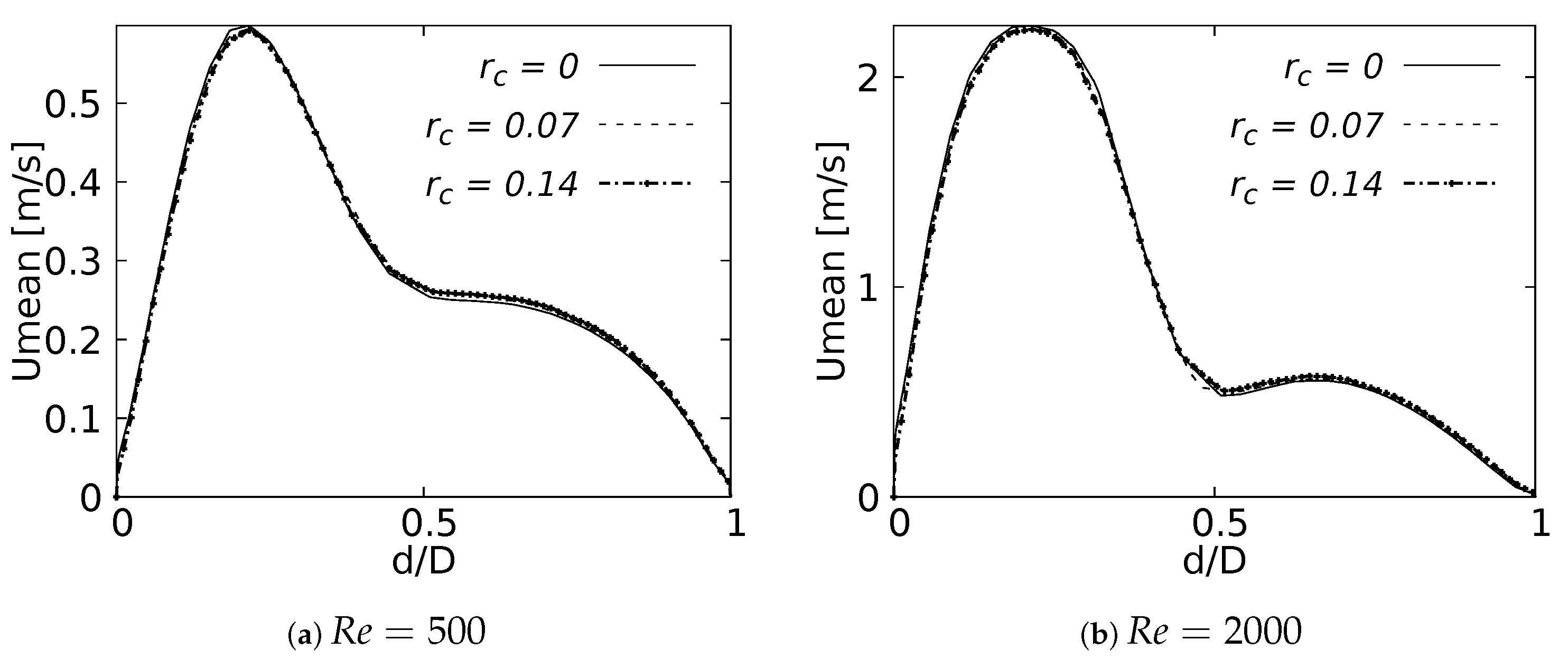
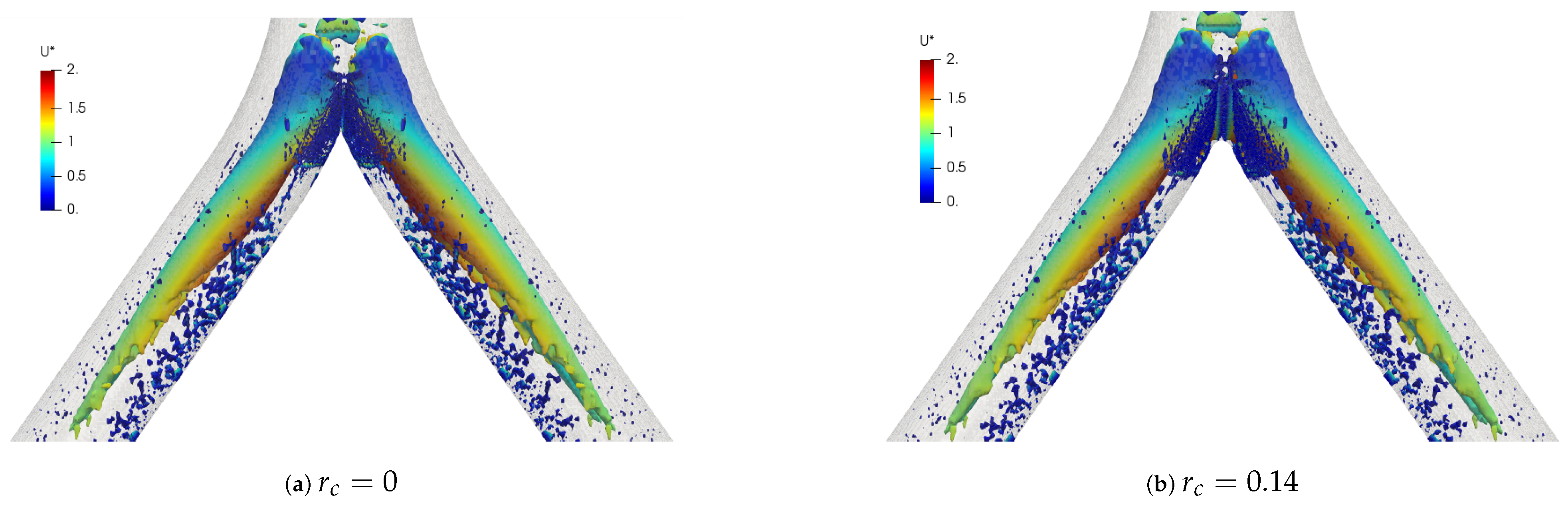
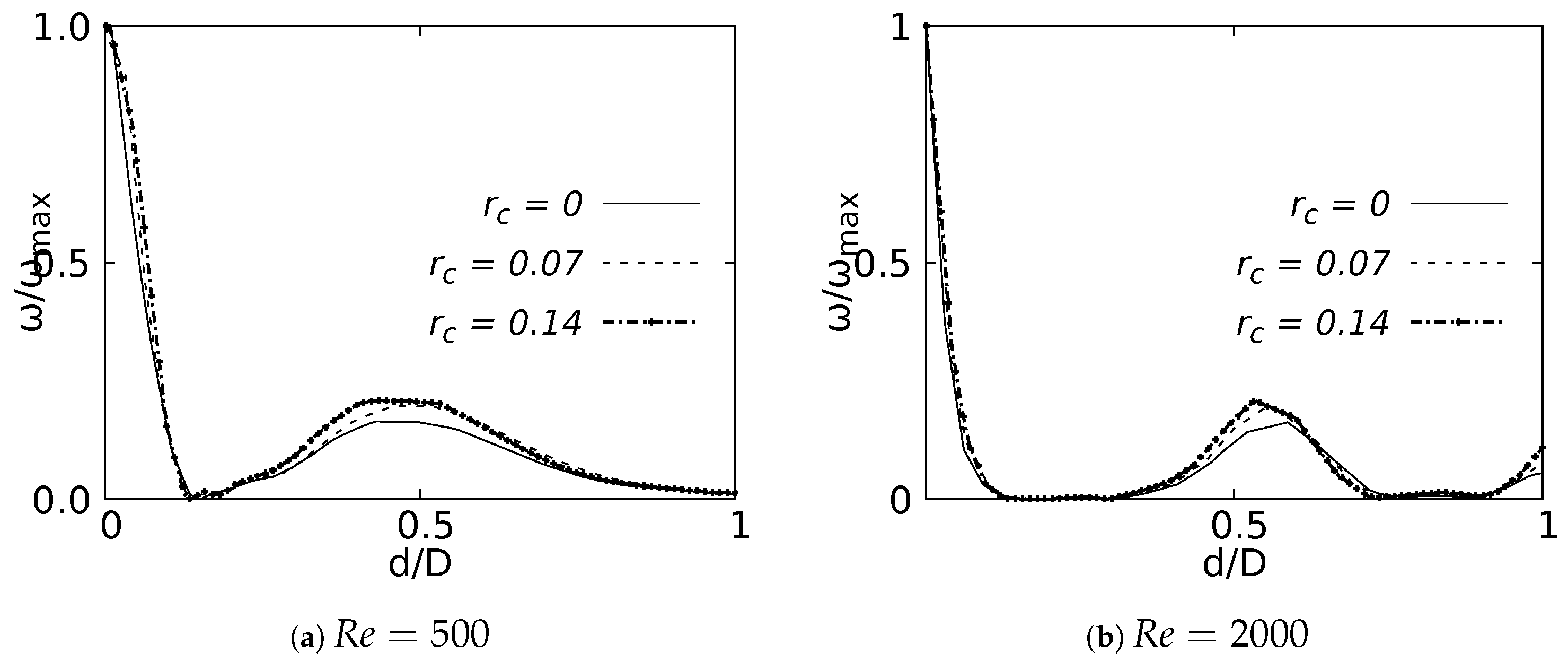
4. Results II. Effect of Variations of the Carina Rounding Radius (CRR)
4.1. Velocity Profiles
4.2. Flow Patterns
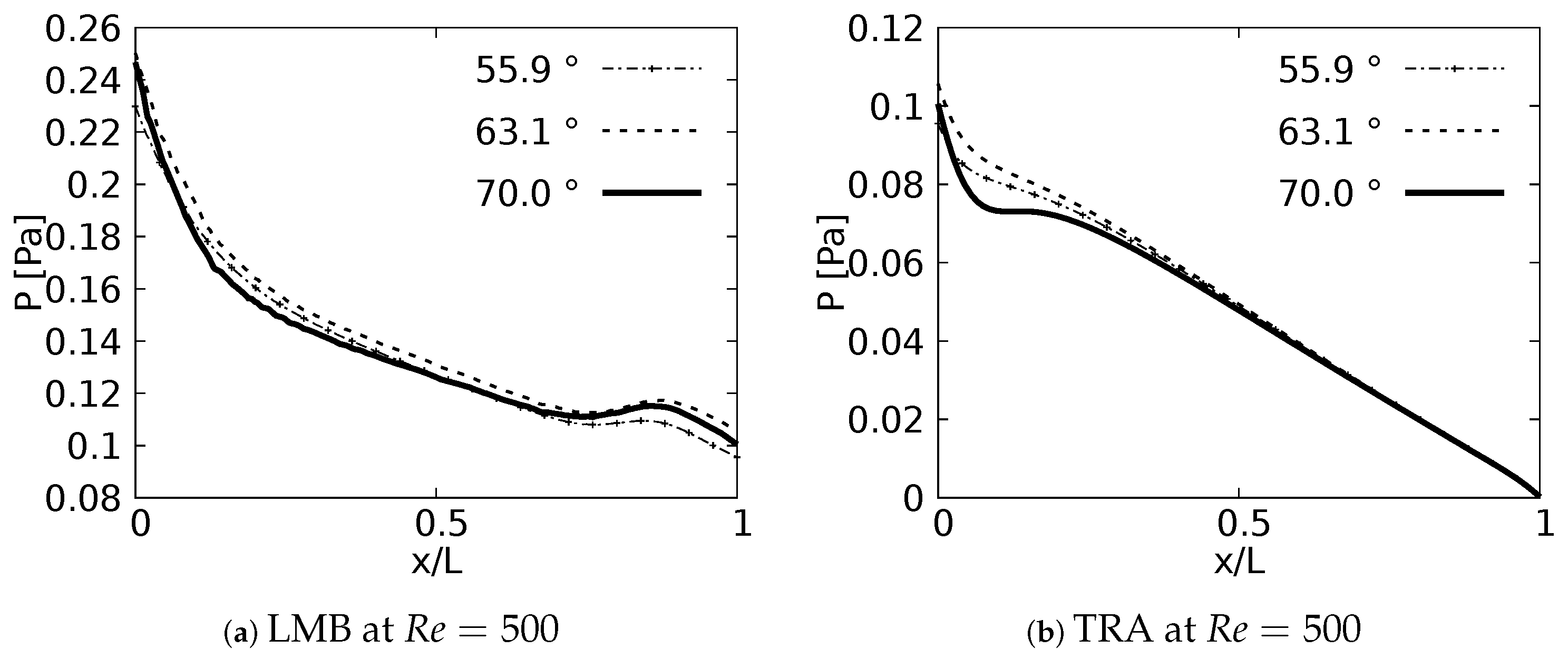
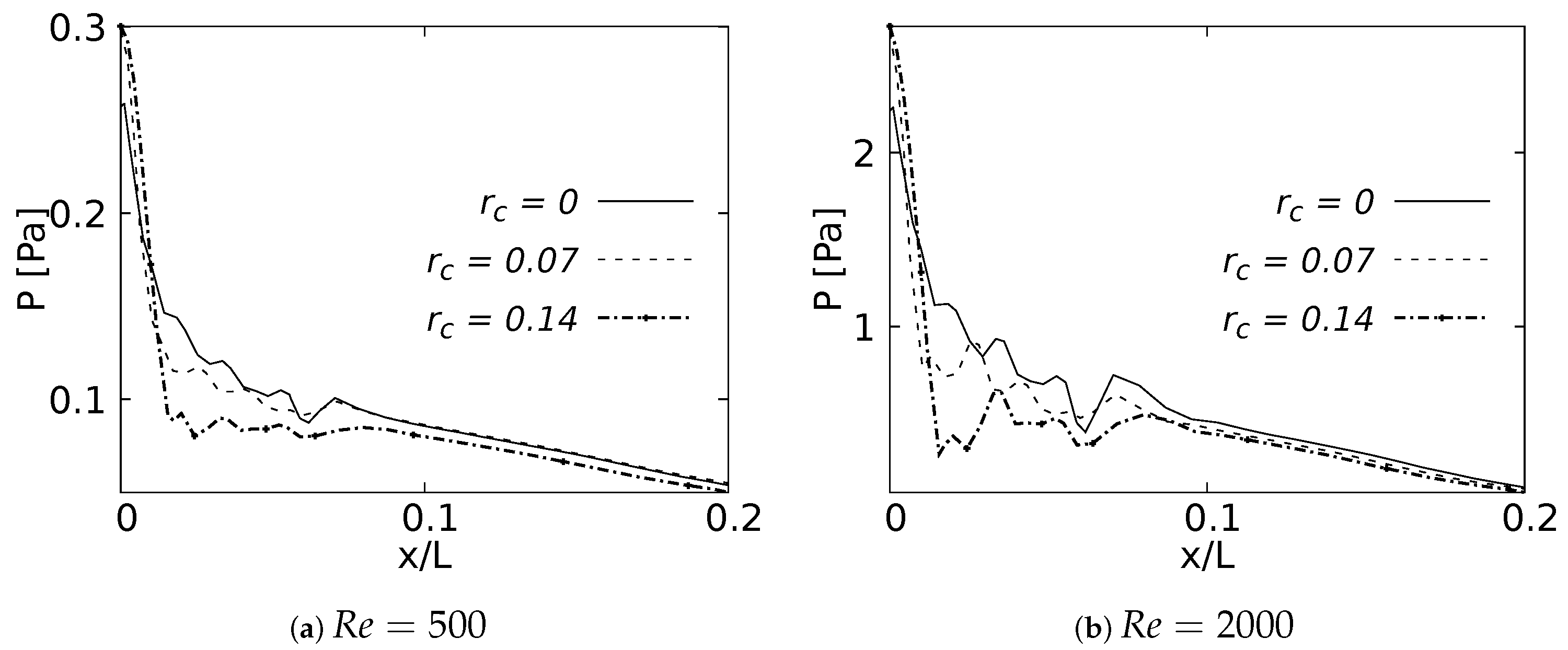
4.3. Pressure Behavior
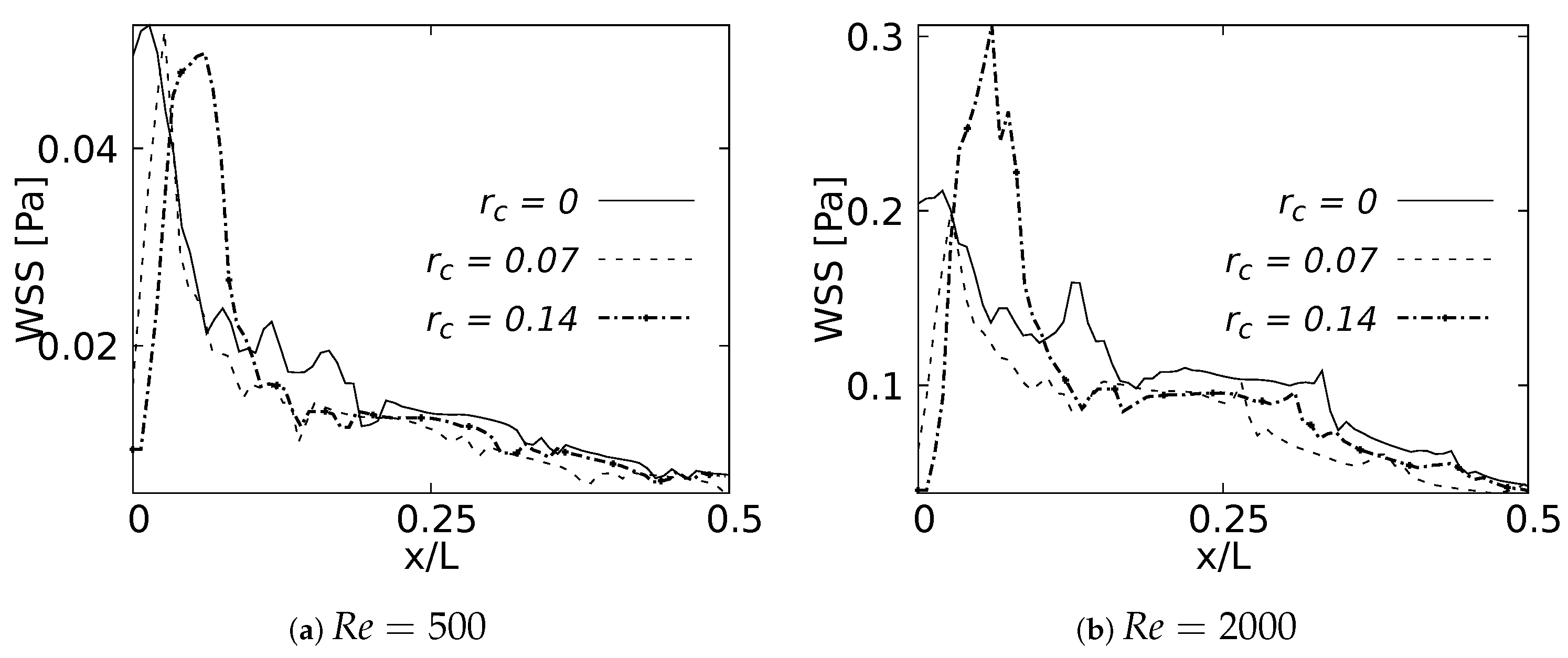
4.4. Wall Shear Stresses
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| D | Branch diameter [mm] |
| R | Branch radius [mm] |
| L | Branch length [mm] |
| Dimensionless rounding radius | |
| y | Grid length in the normal direction of the wall [m] |
| U | Inlet velocity [m/s] |
| Maximun velocity [m/s] | |
| Average velocity [m/s] | |
| Friction velocity [m/s] | |
| p | Fluid pressure [Pa] |
| Reynolds number | |
| fluid density [kg/m] | |
| kinematic viscosity [m] | |
| Dynamic viscosity [Pa.s] |
Abbreviations
| CFD | Computational Fluids Dynamics |
| WSS | Wall Shear Stress |
| BA | Bifurcation angle |
| CRR | Carina rounding radius |
| TRA | Trachea |
| RMB | Right Main Bronchus |
| LMB | Left Main Bronchus |
| RUB | Right Upper Lobar Bronchus |
| LUB | Left Upper Lobar Bronchus |
| RIB | Right Intermediate Bronchus |
| LLB | Left Lower Lobar Bronchus |
References
- West, J.B. Respiratory Physiology: The Essentials, 9th ed.; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 2012. [Google Scholar]
- Minnich, D.J.; Mathisen, D.J. Anatomy of the trachea, carina, and bronchi. Thorac. Surg. Clin. 2007, 17, 571–585. [Google Scholar] [CrossRef] [PubMed]
- Singhal, S.; Henderson, R.; Horsfield, K.; Harding, K.; Cumming, G. Morphometry of the human pulmonary arterial tree. Circ. Res. 1973, 33, 190–197. [Google Scholar] [CrossRef] [PubMed]
- Haskin, P.H.; Goodman, L. Normal tracheal bifurcation angle: A reassessment. Am. J. Roentgenol. 1982, 139, 879–882. [Google Scholar] [CrossRef] [PubMed]
- Alavi, S.M.; Keats, T.E.; O’Brien, W.M. The angle of tracheal bifurcation: Its normal mensuration. Am. J. Roentgenol. 1970, 108, 546–549. [Google Scholar] [CrossRef] [PubMed]
- Khade, B.; Waheed, A.R.; Yadav, N.; Diwan, C. Study of sub carinal angle of human trachea by computerized tomography. Int. J. Anat. Res. 2016, 4, 2828–2832. [Google Scholar] [CrossRef]
- Christou, S.; Chatziathanasiou, T.; Angeli, S.; Koullapis, P.; Stylianou, F.; Sznitman, J.; Kassinos, S.C. Anatomical variability in the upper tracheobronchial tree: Sex-based differences and implications for personalized inhalation therapies. J. Appl. Physiol. 2021, 130, 678–707. [Google Scholar] [CrossRef]
- Sahni, D.; Batra, Y.K.; Rajeev, S. Anatomical dimensions of trachea, main bronchi, subcarinal and bronchial angles in fetuses measured ex vivo. Pediatr. Anesth. 2006, 18, 1029–1034. [Google Scholar]
- Chen, J.; Putman, C.E.; Hedlund, L.W.; Dahmash, N.; Roberts, L. Widening of the subcarinal angle by pericardial effusion. Am. J. Roentgenol. 1982, 139, 883–887. [Google Scholar] [CrossRef]
- Lin, C.; Lee, J.-H.; Hsieh, C.-M. The correlation between subcarinal angle and left atrial volume. Acta Cardiol. Sin. 2012, 28, 332–336. [Google Scholar]
- Murray, J.; Brown, A.; Anagnostou, E.; Senior, R. Widening of the tracheal bifurcation on chest radiographs: Value as a sign of left atrial enlargement. AJR. Am. J. Roentgenol. 1995, 164, 1089–1092. [Google Scholar] [CrossRef]
- Horsfield, K.; Dart, G.; Olson, D.E.; Filley, G.F.; Cumming, G. Models of the human bronchial tree. J. Appl. Physiol. 1971, 31, 207–217. [Google Scholar] [CrossRef]
- Martonen, T.; Yang, Y.; Xue, Z. Effects of carinal ridge shapes on lung airstreams. Aerosol Sci. Technol. 1994, 21, 119–136. [Google Scholar] [CrossRef]
- Xu, X.; Wu, J.; Weng, W.; Fu, M. Investigation of inhalation and exhalation flow pattern in a realistic human upper airway model by PIV experiments and CFD simulations. Biomech. Model. Mechanobiol. 2020, 19, 1679–1695. [Google Scholar] [CrossRef]
- Faizal, W.M.; Ghazali, N.N.N.; Khor, C.Y.; Badruddin, I.A.; Zainon, M.Z.; Yazid, A.A.; Razi, R.M. Computational fluid dynamics modelling of human upper airway: A review. Comput. Methods Programs Biomed. 2020, 196, 105627. [Google Scholar] [CrossRef]
- Tran, T.M.; Huh, S.; Kim, S.; Cui, X.; Choi, S. Numerical investigation of the effect of tracheostomy on flow and particle transport characteristics in human airways. Phys. Fluids 2022, 34, 121901. [Google Scholar] [CrossRef]
- Choi, S.; Yoon, S.; Jeon, J.; Zou, C.; Choi, J.; Tawhai, M.H.; Lin, C.L. 1D network simulations for evaluating regional flow and pressure distributions in healthy and asthmatic human lungs. J. Appl. Physiol. 2019, 127, 122–133. [Google Scholar] [CrossRef]
- Zhang, W.; Xiang, Y.; Lu, C.; Ou, C.; Deng, Q. Numerical modeling of particle deposition in the conducting airways of asthmatic children. Med. Eng. Phys. 2020, 76, 40–46. [Google Scholar] [CrossRef] [PubMed]
- Hariprasad, D.S.; Sul, B.; Liu, C.; Kiger, K.T.; Altes, T.; Ruppert, K.; Wallqvist, A. Obstructions in the lower airways lead to altered airflow patterns in the central airway. Respir. Physiol. Neurobiol. 2020, 272, 103311. [Google Scholar] [CrossRef] [PubMed]
- Kadota, K.; Matsumoto, K.; Uchiyama, H.; Tobita, S.; Maeda, M.; Maki, D.; Tozuka, Y. In silico evaluation of particle transport and deposition in the airways of individual patients with chronic obstructive pulmonary disease. Eur. J. Pharm. Biopharm. 2022, 174, 10–19. [Google Scholar] [CrossRef] [PubMed]
- Zobaer, T.; Sutradhar, A. Modeling the effect of tumor compression on airflow dynamics in trachea using contact simulation and CFD analysis. Comput. Biol. Med. 2021, 135, 104574. [Google Scholar] [CrossRef] [PubMed]
- Morita, K.; Takeishi, N.; Wada, S.; Hatakeyama, T. Computational fluid dynamics assessment of congenital tracheal stenosis. Pediatr. Surg. Int. 2022, 38, 1769–1776. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Tong, Z.; Chan, H.; Yang, R. CFD modelling of air and particle flows in different airway models. J. Aerosol Sci. 2019, 134, 14–28. [Google Scholar] [CrossRef]
- Piemjaiswang, R.; Shiratori, S.; Chaiwatanarat, T.; Piumsomboon, P.; Chalermsinsuwan, B. Computational fluid dynamics simulation of full breathing cycle for aerosol deposition in trachea: Effect of breathing frequency. J. Taiwan Inst. Chem. Eng. 2019, 97, 66–79. [Google Scholar] [CrossRef]
- Hassani, K.; Khorramymehr, S. In silico investigation of sneezing in a full real human upper airway using computational fluid dynamics method. Comput. Methods Programs Biomed. 2019, 177, 203–209. [Google Scholar]
- Ren, S.; Li, W.; Wang, L.; Shi, Y.; Cai, M.; Hao, L.; Luo, Z. Numerical analysis of airway mucus clearance effectiveness using assisted coughing techniques. Sci. Rep. 2020, 10, 1–10. [Google Scholar] [CrossRef]
- Mason, E.C.; Wu, Z.; McGhee, S.; Markley, J.; Koenigs, M.; Onwuka, A.; Zhao, K. Computational fluid dynamic modeling reveals nonlinear airway stress during trachea development. J. Pediatr. 2021, 238, 324–328. [Google Scholar] [CrossRef]
- Weibel, E.R.; Cournand, A.F.; Richards, D.W. Morphometry of the Human Lung, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1963. [Google Scholar]
- Hofmann, W. Modelling inhaled particle deposition in the human lung: A review. J. Aerosol Sci. 2011, 42, 693–724. [Google Scholar] [CrossRef]
- Islam, M.S.; Paul, G.; Ong, H.X.; Young, P.M.; Gu, Y.T.; Saha, S.C. A review of respiratory anatomical development, air flow characterization and particle deposition. Int. J. Environ. Res. Public Health 2020, 17, 380. [Google Scholar] [CrossRef]
- Lai, T.C.; Morsi, Y.S.; Das, S.; Owida, A. Numerical analysis of particle deposition in asymmetrical human upper airways under different inhalation cycles. J. Mech. Med. Biol. 2013, 13, 1350068. [Google Scholar] [CrossRef]
- Tsega, E.G.; Katiyar, V.K.; Gupta, P. Numerical Simulation of Transport and Deposition of Dust Particles in Human Tracheobronchial Airways. Int. J. Biomed. Sci. Eng. 2019, 7, 8. [Google Scholar] [CrossRef]
- Balashazy, I.; Heistracher, T.; Hofmann, W. Air flow and particle deposition patterns in bronchial airway bifurcations: The effect of different CFD models and bifurcation geometries. J. Aerosol Med. 1996, 9, 287–301. [Google Scholar] [CrossRef]
- Horsfield, K.; Cumming, G. Angles of branching and diameters of branches in the human bronchial tree. Bull. Math. Biophys. 1967, 29, 245–259. [Google Scholar] [CrossRef] [PubMed]
- Lee, D.; Park, S.S.; Ban-Weiss, G.A.; Fanucchi, M.V.; Plopper, C.G.; Wexler, A.S. Bifurcation model for characterization of pulmonary architecture. Anat. Rec. 2008, 291, 379–389. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; So, R.; Zhang, C. Modeling the bifurcating flow in a human lung airway. J. Biomech. 2002, 35, 465–473. [Google Scholar] [CrossRef]
- Comer, J.K.; Kleinstreuer, C.; Kim, C.S. Flow structures and particle deposition patterns in double-bifurcation airway models. Part 2. Aerosol transport and deposition. J. Fluid Mech. 2001, 435, 55–80. [Google Scholar] [CrossRef]
- Fresconi, F.E.; Prasad, A.K. Secondary velocity fields in the conducting airways of the human lung. J. Biomech. Eng. 2007, 129, 722–732. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; So, R.; Yang, J. The effect of inlet velocity profile on the bifurcation COPD airway flow. Comput. Biol. Med. 2006, 36, 181–194. [Google Scholar] [CrossRef]
- Yu, G.; Zhang, Z.; Lessmann, R. Computer simulation of the flow field and particle deposition by diffusion in a 3-D human airway bifurcation. Aerosol Sci. Technol. 1996, 25, 338–352. [Google Scholar] [CrossRef]
- Zhang, Z.; Kleinstreuer, C.; Kim, C.S. Effects of curved inlet tubes on air flow and particle deposition in bifurcating lung models. J. Biomech. 2001, 34, 659–669. [Google Scholar] [CrossRef]
- Zhao, Y.; Lieber, B.B. Steady inspiratory flow in a model symmetric bifurcation. Trans. ASME-K-J. Biomech. Eng. 1994, 116, 488–496. [Google Scholar] [CrossRef]
- Zhao, Y.; Lieber, B.B. Steady expiratory flow in a model symmetric bifurcation. J. Biomech. Eng. 1994, 116, 318–323. [Google Scholar] [CrossRef]
- Adler, K.; Brücker, C. Dynamic flow in a realistic model of the upper human lung airways. Exp. Fluids 2007, 43, 411. [Google Scholar] [CrossRef]
- Bauer, K.; Brücker, C. The influence of airway tree geometry and ventilation frequency on airflow distribution. J. Biomech. Eng. 2015, 137, 081001. [Google Scholar] [CrossRef] [PubMed]
- Wall, W.A.; Rabczuk, T. Fluid–structure interaction in lower airways of CT-based lung geometries. Int. J. Numer. Methods Fluids 2008, 57, 653–675. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Y.; Sun, X.; Yu, S.; Gao, F. Numerical analysis of respiratory flow patterns within human upper airway. Acta Mech. Sin. 2009, 25, 737–746. [Google Scholar] [CrossRef]
- Koombua, K.; Pidaparti, R.M.; Longest, P.W.; Ward, K.R. Computational analysis of fluid characteristics in rigid and flexible human respiratory airway models. Eng. Appl. Comput. Fluid Mech. 2008, 2, 185–194. [Google Scholar] [CrossRef]
- Duque-Daza, C.A.; Ramirez-Pastran, J.; Lain, S. Influence of particle mass fraction over the turbulent behaviour of an incompressible particle-laden flow. Fluids 2020, 6, 374. [Google Scholar] [CrossRef]
- Wang, W.; Dai, Z.; Li, J.; Zhou, L. A hybrid Laplace transform finite analytic method for solving transport problems with large Peclet and Courant numbers. Comput. Geosci. 2009, 49, 182–189. [Google Scholar] [CrossRef]
- Liu, W.; Sun, H.; Lai, D.; Xue, Y.; Kabanshi, A.; Hu, S. Performance of fast fluid dynamics with a semi-Lagrangian scheme and an implicit upwind scheme in simulating indoor/outdoor airflow. Build. Environ. 2022, 207, 108477. [Google Scholar] [CrossRef]
- Calay, R.; Kurujareon, J.; Holdø, A.E. Numerical simulation of respiratory flow patterns within human lung. Respir. Physiol. Neurobiol. 2002, 130, 201–221. [Google Scholar] [CrossRef]
- Schroter, R.; Sudlow, M. Flow patterns in models of the human bronchial airways. Respir. Physiol. 1969, 7, 341–355. [Google Scholar] [CrossRef] [PubMed]
- Van Ertbruggen, C.; Hirsch, C.; Paiva, M. Anatomically based three-dimensional model of airways to simulate flow and particle transport using computational fluid dynamics. J. Appl. Physiol. 2005, 98, 970–980. [Google Scholar] [CrossRef] [PubMed]
- Corieri, P. Experimental and Numerical Investigation of Flows in Bifurcations within Lung Airways. Ph.D. Thesis, von Karman Institute for Fluid Dynamics, Université Libre de Bruxelles and Rheinisch-Westfälische Technische Hochschule Aachen, Brussels, Belgium, 1994. [Google Scholar]
- Han, F.; Liu, Y.; Lan, Q.; Li, W.; Wang, Z. CFD investigation on secondary flow characteristics in double-curved subsea pipelines with different spatial structures. J. Mar. Sci. Eng. 2022, 10, 1264. [Google Scholar] [CrossRef]
- Dean, W.R. Note on the motion of fluid in a curved pipe. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1927, 4, 208–223. [Google Scholar] [CrossRef]
- Pedley, T.; Schroter, R.; Sudlow, M. Energy losses and pressure drop in models of human airways. Respir. Physiol. 1970, 9, 371–386. [Google Scholar] [CrossRef] [PubMed]
- Pedley, T.; Schroter, R.; Sudlow, M. Flow and pressure drop in systems of repeatedly branching tubes. J. Fluid Mech. 1971, 46, 365–383. [Google Scholar] [CrossRef]
- Wang, X.-Q.; Mujumdar, A.S.; Yap, C. Effect of bifurcation angle in tree-shaped microchannel networks. J. Appl. Phys. 2007, 102, 073530. [Google Scholar] [CrossRef]
- Green, A.S. Modelling of peak-flow wall shear stress in major airways of the lung. J. Biomech. 2004, 37, 661–667. [Google Scholar] [CrossRef]
- Nucci, G.; Suki, B.; Lutchen, K. Modeling airflow-related shear stress during heterogeneous constriction and mechanical ventilation. J. Appl. Physiol. 2003, 95, 348–356. [Google Scholar] [CrossRef]
- Xia, G.; Tawhai, M.H.; Hoffman, E.A.; Lin, C.-L. Airway wall stiffening increases peak wall shear stress: A fluid-structure interaction study in rigid and compliant airways. Ann. Biomed. Eng. 2010, 38, 1836–1853. [Google Scholar] [CrossRef]


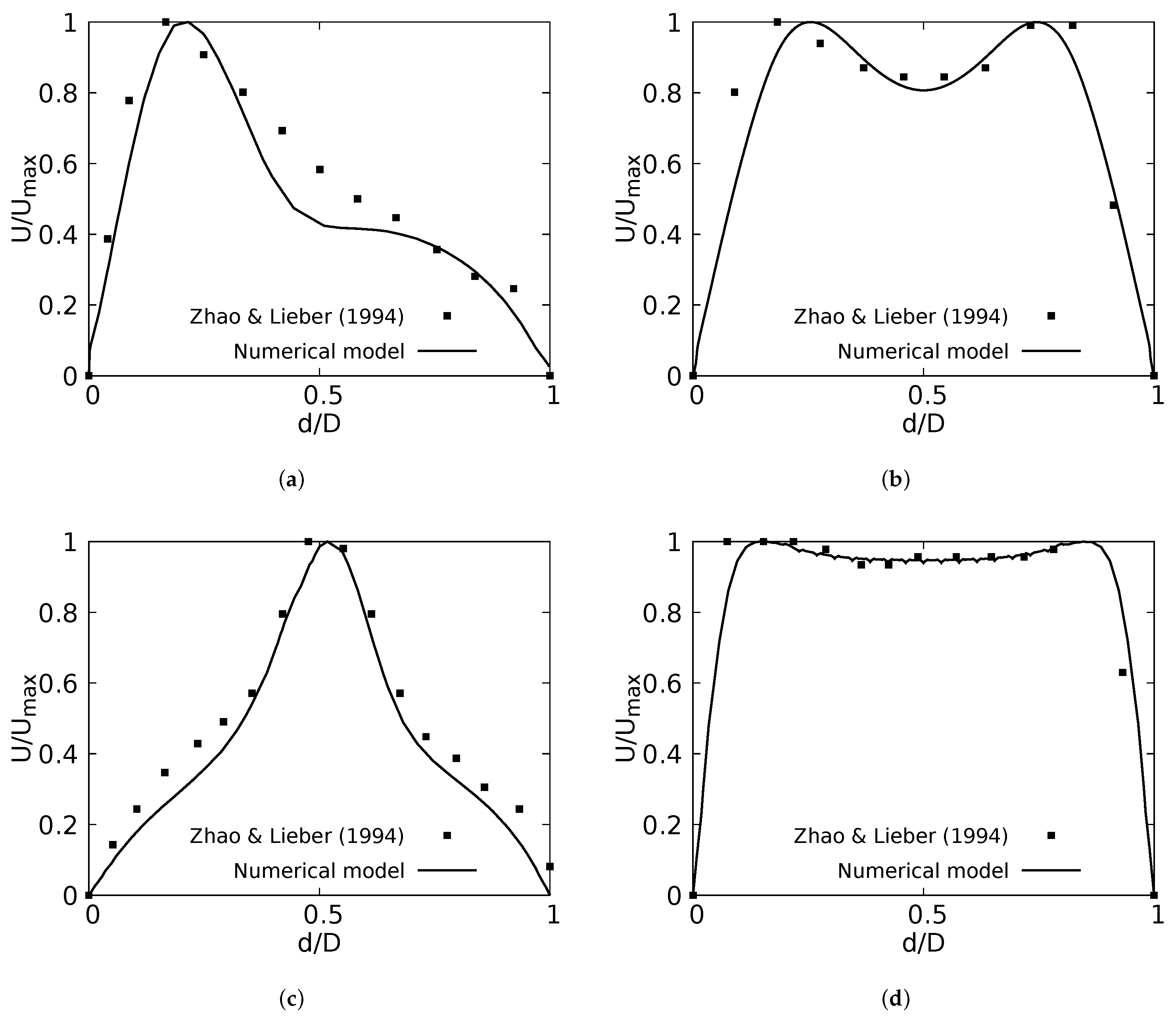








| AIRWAY | D (mm) | L (mm) |
|---|---|---|
| TRA | 18 | 120 |
| LMB/RMB | 12 | 47.6 |
| LUB/RUB/LLB/RIB | 8.3 | 41.5 |
| AGE [YEARS] | ANGLE [] |
|---|---|
| 21–30 | 64.2 |
| 31–40 | 58.9 |
| 41–50 | 61.9 |
| 51–60 | 63.1 |
| 61–+ | 55.9 |
| All ages | 60.8 |
| Branch | D (mm) | Len. (mm) | Rd (mm) | |
|---|---|---|---|---|
| TRA | 16 | 80 | 35 | 81.5 |
| LMB/RMB | 14 | 70 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Espinosa-Moreno, A.S.; Duque-Daza, C.A.; Garzón-Alvarado, D.A. Influence of Morphological Parameters on the Flow Development within Human Airways. Fluids 2023, 8, 78. https://doi.org/10.3390/fluids8030078
Espinosa-Moreno AS, Duque-Daza CA, Garzón-Alvarado DA. Influence of Morphological Parameters on the Flow Development within Human Airways. Fluids. 2023; 8(3):78. https://doi.org/10.3390/fluids8030078
Chicago/Turabian StyleEspinosa-Moreno, Andres Santiago, Carlos Alberto Duque-Daza, and Diego Alexander Garzón-Alvarado. 2023. "Influence of Morphological Parameters on the Flow Development within Human Airways" Fluids 8, no. 3: 78. https://doi.org/10.3390/fluids8030078
APA StyleEspinosa-Moreno, A. S., Duque-Daza, C. A., & Garzón-Alvarado, D. A. (2023). Influence of Morphological Parameters on the Flow Development within Human Airways. Fluids, 8(3), 78. https://doi.org/10.3390/fluids8030078








