Flow Boiling Heat Transfer Performance and Boiling Phenomena on Various Straight Fin Configurations
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Facility
2.2. Test Samples
2.3. Measurement and Data Acquisition
3. Results and Discussion
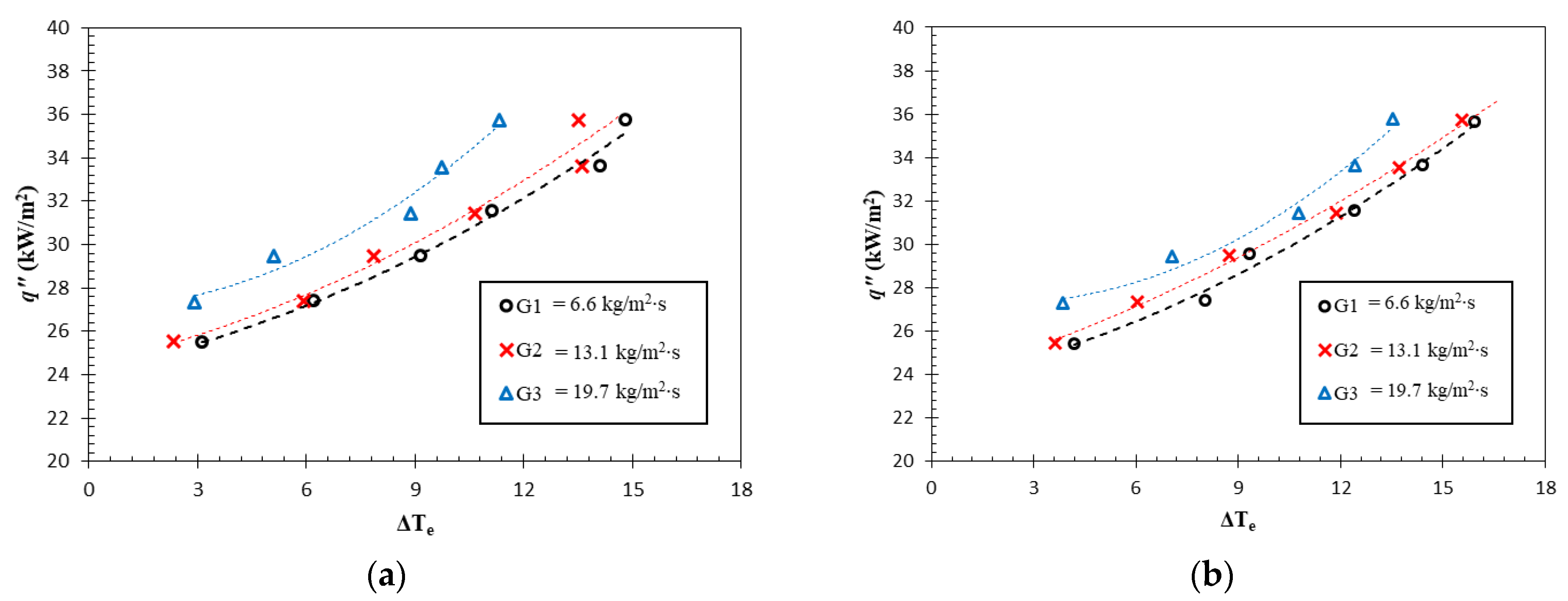
3.1. Boiling Curve and Cooling Performance
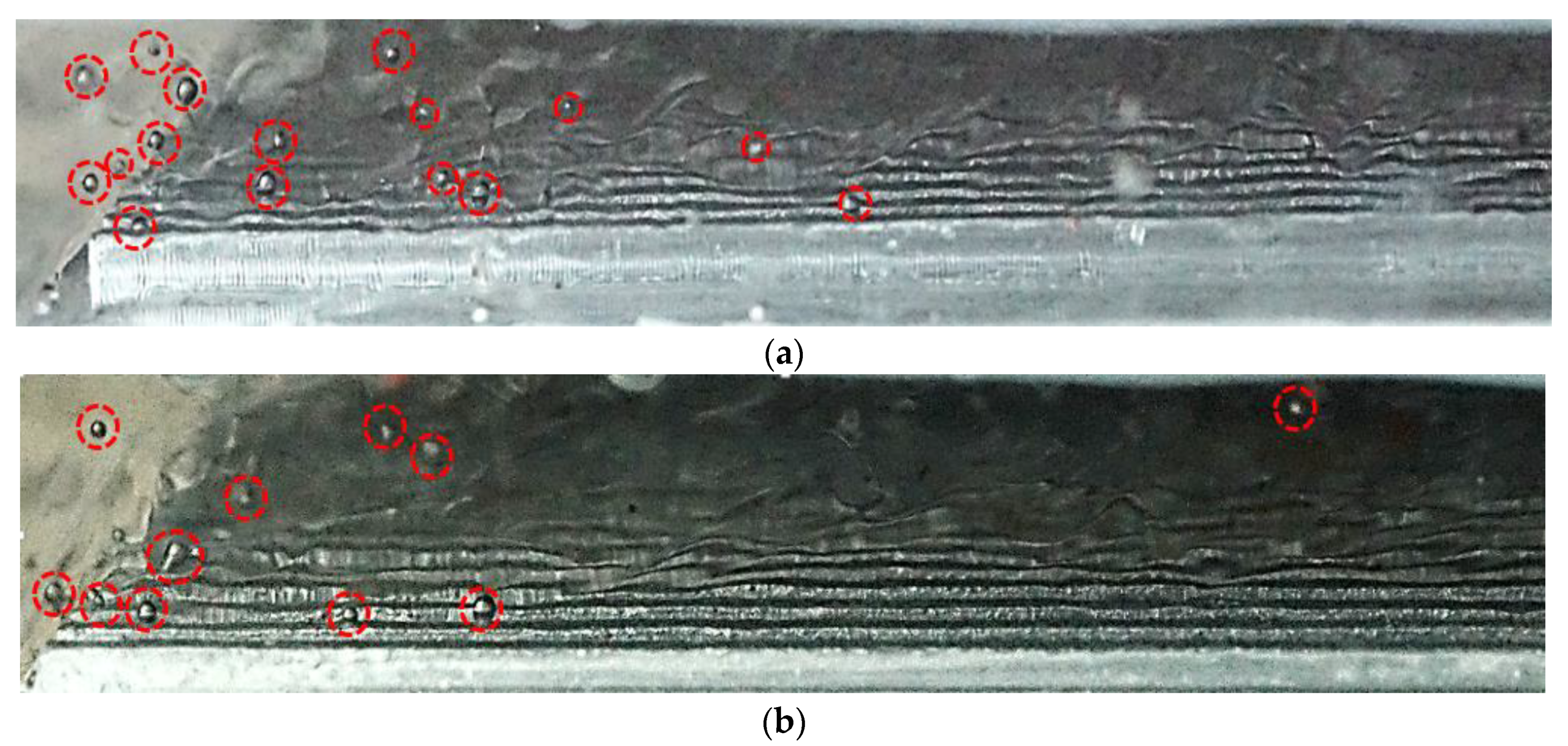
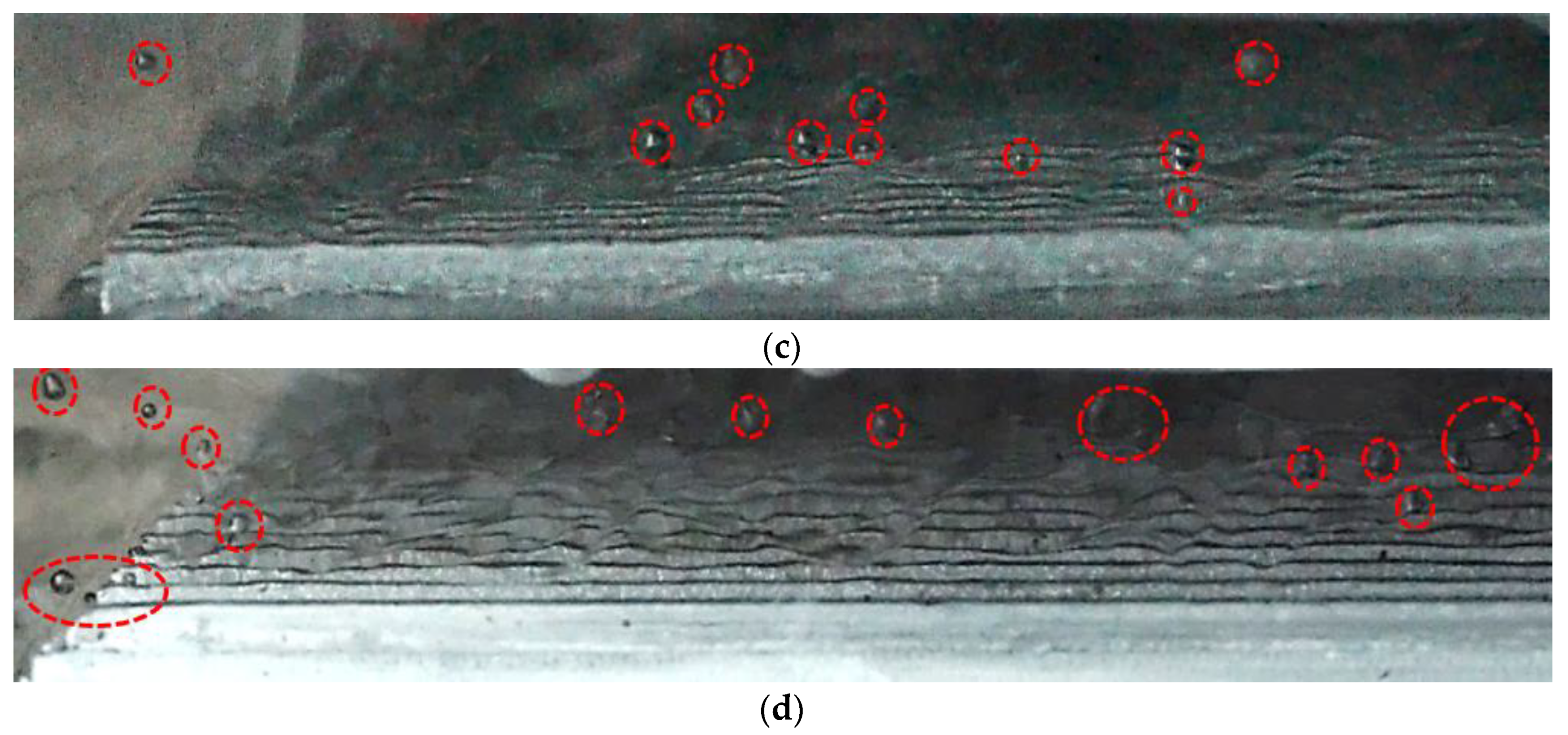
3.2. Boiling Phenomenon and Bubble Dynamics
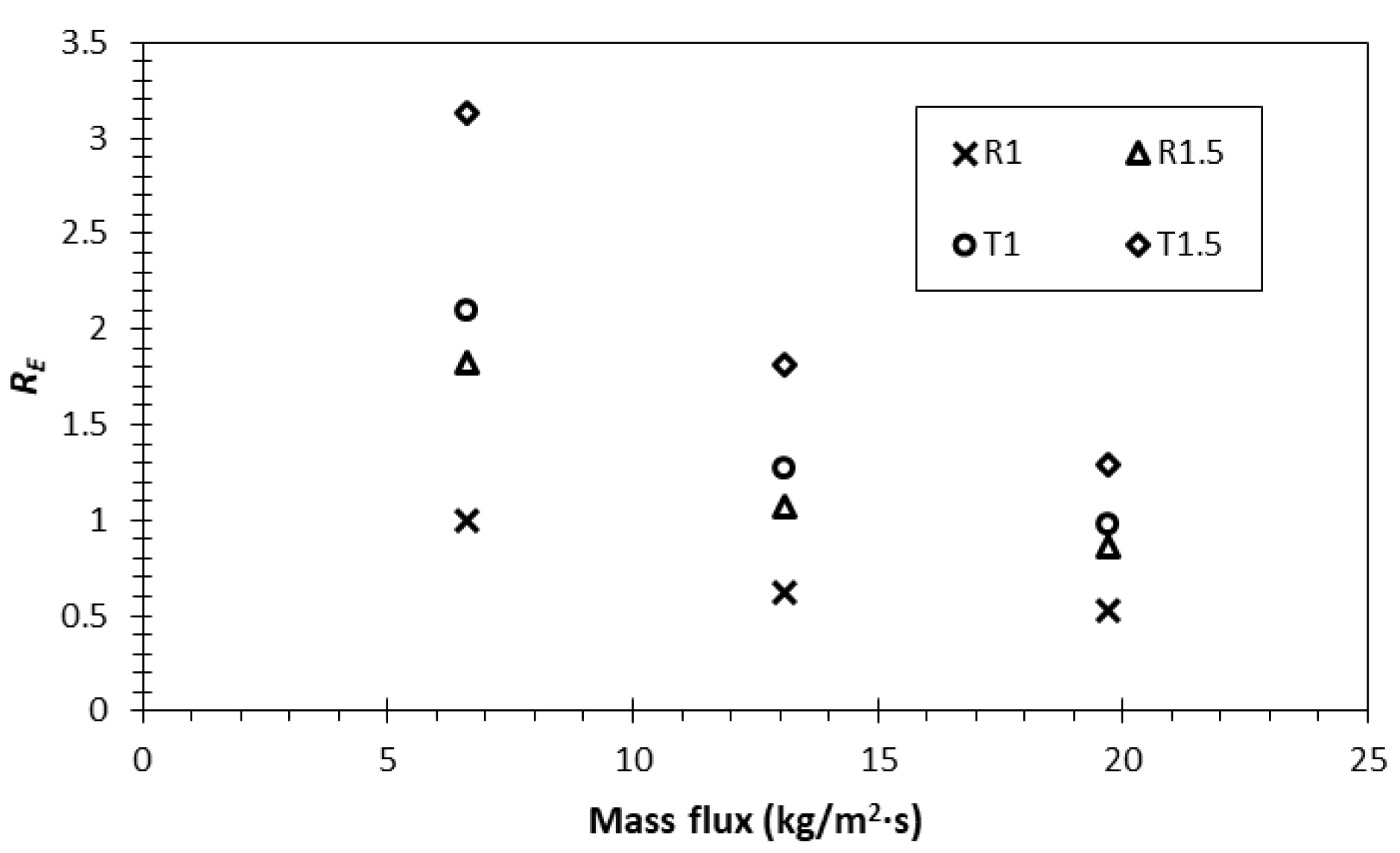
3.3. Enhancement Efficiency Analysis
4. Conclusions
- The inclining trend of heat transfer coefficient with the rise in heat flux was indicated by the sharper boiling curves, whereby the lower surface temperature could be achieved at the same supplied heat flux. This was the result of the more bubbles being generated, increasing the contribution of the evaporative and quenching heat flux.
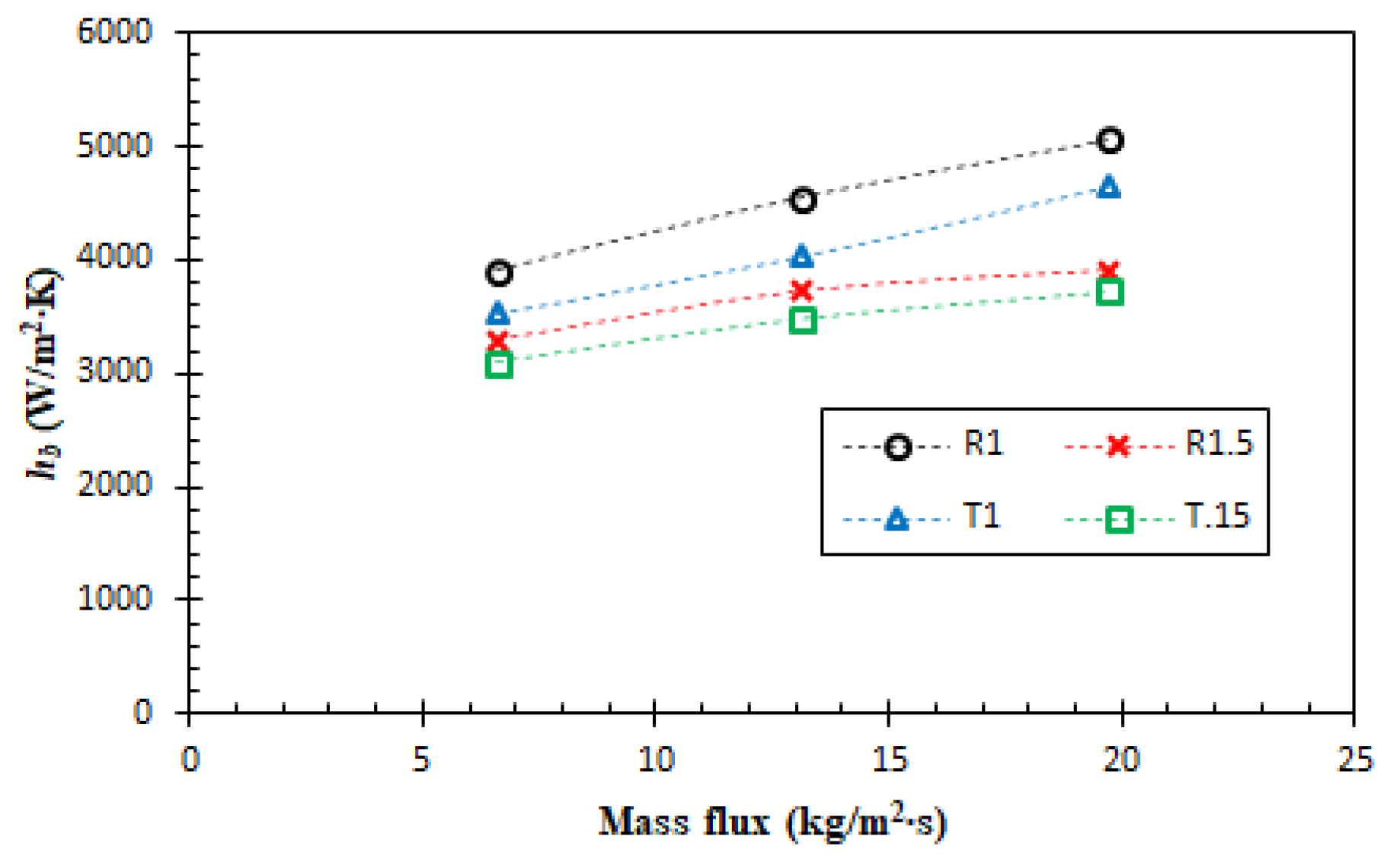
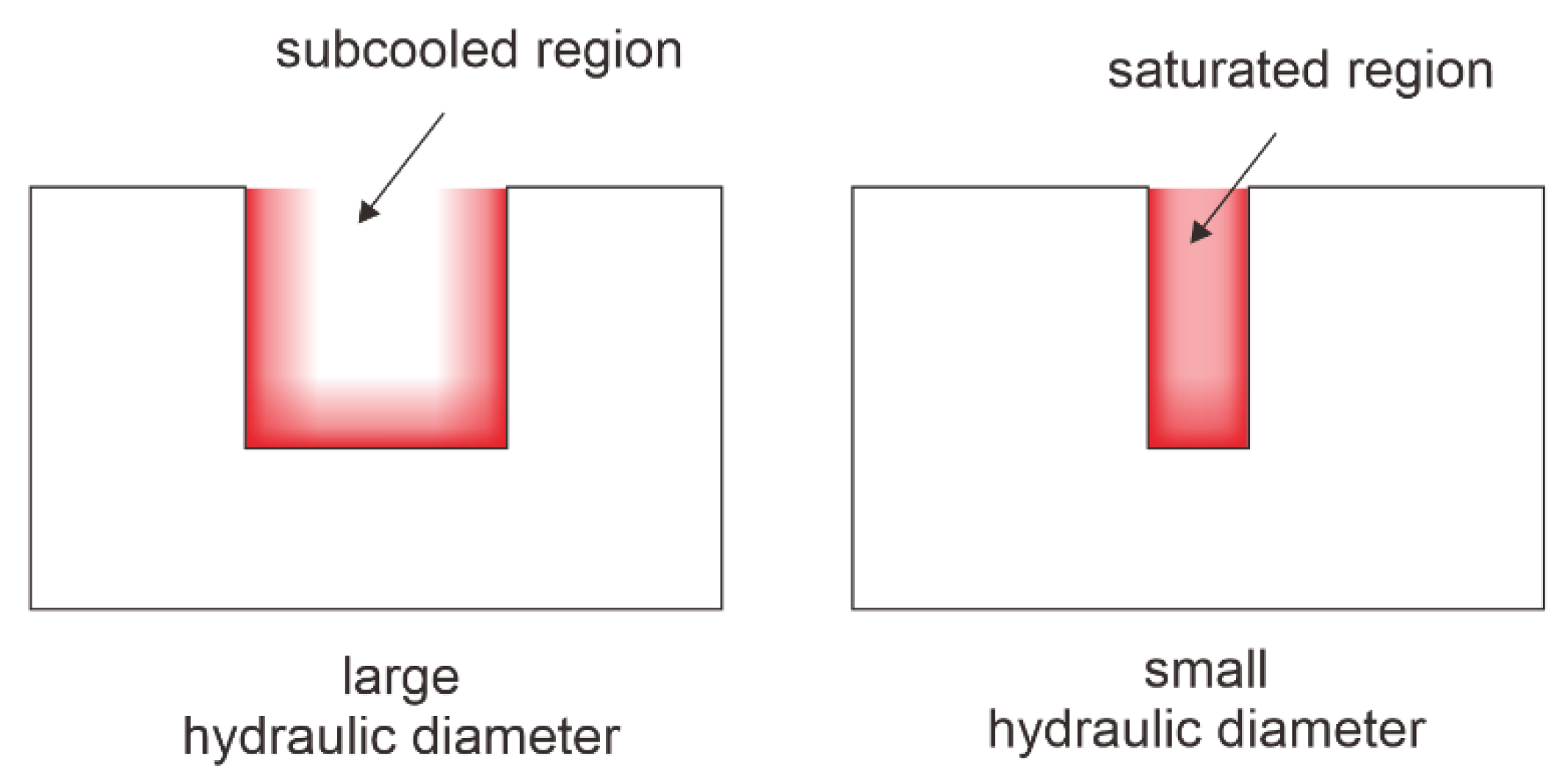
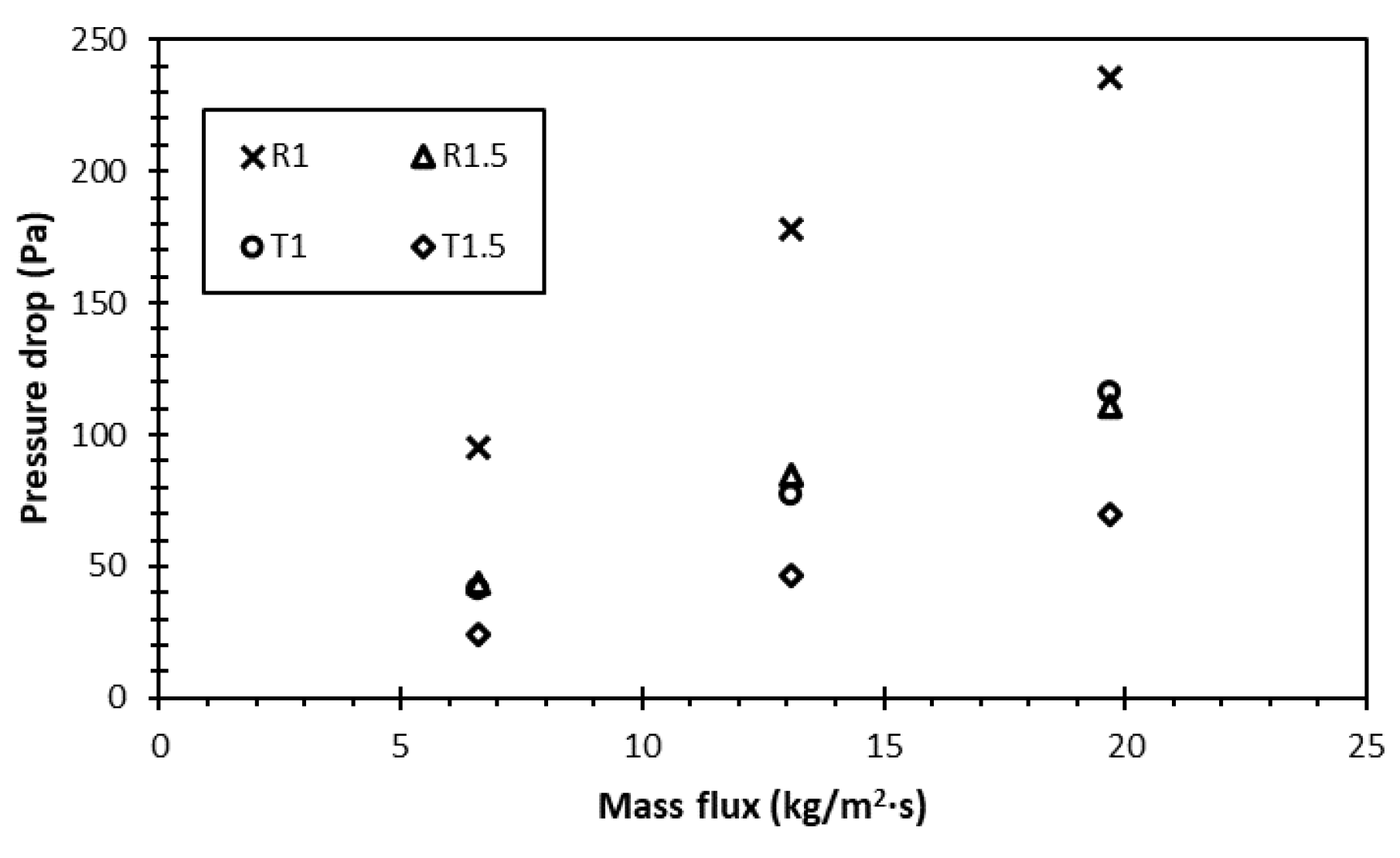
- The cooling performance improved as the fin gap reduced up to 22.5% and 17.1% for rectangular and trapezoidal fins, respectively. The hydraulic diameter had an important impact on the heat transfer coefficient, whereby the lower diameter contributed to the more distributed saturated region. As the hydraulic diameter decreased, there would be more covered area to fulfil the requirement of the ONB, resulting in better cooling performance by the bubble nucleation process.
- In general, the rectangular fin had a higher heat transfer coefficient than the trapezoidal fin. The effect of the total extended area was undermined by the effect of the hydraulic diameter. In order, the best test cases in terms of cooling performance were R1, T1, R1.5 and T1.5, with the highest observed at 5066.84 W/m2∙K.
- Bubble sliding was observed during the boiling process. As the heat transfer coefficient increased, the bubbles were observed to exist in larger numbers but with a shorter period of detachment. It was found that there was no significant effect of mass flux on the bubble sliding distance.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| a | Sample length (mm) |
| Abase | Area of fin base (mm2) |
| Aext | Area of fin extended surface (mm2) |
| Atot | Total area of fin surface (mm2) |
| b | Sample width (mm) |
| CHF | Critical heat flux |
| Dh | Hydraulic diameter (mm) |
| G | Mass flux (kg/m2·s) |
| h | Fin height (mm) |
| hb | Boiling heat transfer coefficient (W/m2·K) |
| P | Wetted perimeter (m) |
| q″ | Heat flux (W/m2) |
| RE | Enhancement ratio (dimensionless) |
| s | Fin width (mm) |
| Ts | Surface temperature (K) |
| Tsat | Saturation temperature (K) |
| Δp | Pressure drop (Pa) |
| ΔTe | Ts − Tsat, Excess temperature (K) |
| σ | Uncertainty |
References
- El-Genk, M.S. Nucleate boiling enhancements on porous graphite and microporous and macro—Finned copper surfaces nucleate boiling enhancements on porous graphite and microporous and macro—Finned copper surfaces. Heat Transfer. Eng. 2012, 33, 175–204. [Google Scholar] [CrossRef]
- Liang, G.; Mudawar, I. Review of pool boiling enhancement by surface modification. Int. J. Heat Mass Transf. 2021, 128, 892–933. [Google Scholar] [CrossRef]
- Giustini, G. Modelling of boiling flows for nuclear thermal hydraulics applications—A brief review. Invention 2020, 5, 47. [Google Scholar] [CrossRef]
- Kurul, N.; Podowski, M.Z. Multidimensional effects in forced convection subcooled boiling. In International Heat Transfer Conference Digital Library; Begel House Inc.: Danbury, CT, USA, 1990. [Google Scholar]
- Anderson, T.M.; Mudawar, I. Microelectronic cooling by enhanced pool boiling of a dielectric fluorocarbon liquid. J. Heat Transfer. 1989, 111, 752–759. [Google Scholar] [CrossRef]
- He, Z.; Yan, Y.; Zhang, Z. Thermal management and temperature uniformity enhancement of electronic devices by micro heat sinks: A review. Energy 2020, 216, 119223. [Google Scholar] [CrossRef]
- Sur, A.; Lu, Y.; Pascente, C.; Ruchhoeft, P.; Liu, D. Pool boiling heat transfer enhancement with electrowetting. Int. J. Heat Mass Transf. 2018, 120, 202–217. [Google Scholar] [CrossRef]
- Deng, D.; Zeng, L.; Sun, W. A review on flow boiling enhancement and fabrication of enhanced microchannels of microchannel heat sinks. Int. J. Heat Mass Transf. 2021, 175, 121332. [Google Scholar] [CrossRef]
- Milanova, D.; Kumar, R. Heat transfer behavior of silica nanoparticles in pool boiling. J. Heat Transf. 2018, 130, 042401. [Google Scholar] [CrossRef]
- Ho, J.Y.; Leong, K.C.; Yang, C.; Pranoto, I. An experimental study of carbon nanotube coatings for pool boiling heat transfer enhancement. In Proceedings of the International Heat Transfer Conference 15, Kyoto, Japan, 10–15 August 2014. [Google Scholar]
- Wei, J.J.; Honda, H. Effects of fin geometry on boiling heat transfer from silicon chips with micro-pin-fins immersed in FC-72. Int. J. Heat Mass Transf. 2003, 46, 4059–4070. [Google Scholar] [CrossRef]
- Shojaeian, M.; Kosar, A. Pool boiling and flow boiling on micro- and nanostructured surfaces. Exp. Therm. Fluid Sci. 2015, 63, 45–73. [Google Scholar] [CrossRef]
- Bian, H.; Kurwitz, C.; Sun, Z.; Cheng, K.; Chen, K. Enhanced nucleate boiling on 3D-printed micro-porous structured surface. Appl. Therm. Eng. 2018, 141, 422–434. [Google Scholar] [CrossRef]
- Pranoto, I.; Leong, K.C. An experimental study of flow boiling heat transfer from porous foam structures in a channel. Appl. Therm. Eng. 2014, 70, 100–114. [Google Scholar] [CrossRef]
- Harirchian, T.; Garimella, S.V. Microchannel size effects on local flow boiling heat transfer to a dielectric fluid. Int. J. Heat Mass Transfer 2008, 51, 3724–3735. [Google Scholar] [CrossRef]
- Deng, D.; Tang, Y.; Shao, H.; Zeng, J.; Zhou, W.; Liang, D. Effects of structural parameters on flow boiling performance of reentrant porous microchannels. J. Micromech. Microeng. 2014, 24, 065025. [Google Scholar] [CrossRef]
- Cheng, P.; Wu, H.Y.; Hong, F.J. Phase-change heat transfer in microsystems. J. Heat Transfer. 2006, 129, 101–108. [Google Scholar] [CrossRef]
- Ma, A.; Wei, J.; Yuan, M.; Fang, J. Enhanced flow boiling heat transfer of FC-72 on micro-pin-finned surfaces. Int. J. Heat Mass Transfer. 2009, 52, 2925–2931. [Google Scholar] [CrossRef]
- Law, M.; Lee, P. A comparative study of experimental flow boiling heat transfer and pressure characteristics in straight- and oblique-finned microchannels. Int. J. Heat Mass Transfer. 2015, 85, 797–810. [Google Scholar] [CrossRef]
- Prajapati, Y.K.; Pathak, M.; Khan, M.K. A comparative study of flow boiling heat transfer in three different configurations of microchannels. Int. J. Heat Mass Transf. 2015, 85, 711–722. [Google Scholar] [CrossRef]
- Sun, Y.; Zhang, L.; Xu, H.; Zhong, X. Flow boiling enhancement of FC-72 from microporous surfaces in minichannels. Exp. Therm. Fluid Sci. 2011, 35, 1418–1426. [Google Scholar] [CrossRef]
- Zhou, J.; Qiang, L.; Xuemei, C. Micro pin fins with topologically optimized configurations enhance flow boiling heat transfer in manifold microchannel heat sinks. Int. J. Heat Mass Transfer. 2023, 206, 123956. [Google Scholar] [CrossRef]
- Tian, Z.; Kun, L.; Yuan, Z.; Zhikang, H.; Shuming, X.; Wenzhong, G. Numerical simulation of microchannel flow boiling and critical heat flux under rolling motion. Int. J. Refrigeration. 2023, 145, 118–128. [Google Scholar] [CrossRef]
- Qi, H.; Fang, D.; Meng, X.; Wu, J. Liquid density of HFE-7000 and HFE-7100 from T = (283 to 363) K at pressures up to 100 MPa. J. Chem. Thermodyn. 2014, 77, 131–136. [Google Scholar] [CrossRef]
- An, B.; Duan, Y.; Yang, F.; Yang, Z. pvT property of HFE 7100 in the gaseous phase. J. Chem. Eng. Data. 2015, 60, 3289−3295. [Google Scholar] [CrossRef]
- Rausch, M.H.; Kretschmer, L.; Will, S.; Leipertz, A.; Froba, A.P. Density, surface tension, and kinematic viscosity of hydrofluoroethers HFE-7000, HFE-7100, HFE-7200, HFE-7300, and HFE-7500. J. Chem. Eng. Data. 2015, 60, 3759–3765. [Google Scholar] [CrossRef]
- Taylor, J.R. An Introduction to Error Analysis, 2nd ed.; University of Science Book: Sausalito, CA, USA, 1997. [Google Scholar]
- Pranoto, I.; Rahman, M.A.; Waluyo, J. The Role of Pin Fin Array Configurations and Bubble Characteristics on the Pool Boiling Heat Transfer Enhancement. Fluids 2022, 7, 232. [Google Scholar] [CrossRef]
- Pranoto, I.; Rahman, M.A.; Mahardika, P.A.P. Pool boiling heat transfer performance and bubble dynamics from pin fin-modified surfaces with geometrical shape variation. Energies 2022, 15, 1847–1858. [Google Scholar] [CrossRef]
- Vajc, V.; Moze, M.; Hadzic, A.; Sulc, R.; Golobic, I. Saturated and subcooled pool boiling heat transfer in mixtures of water and glycerin. Exp. Heat Transf. 2023, 36, 283–311. [Google Scholar] [CrossRef]




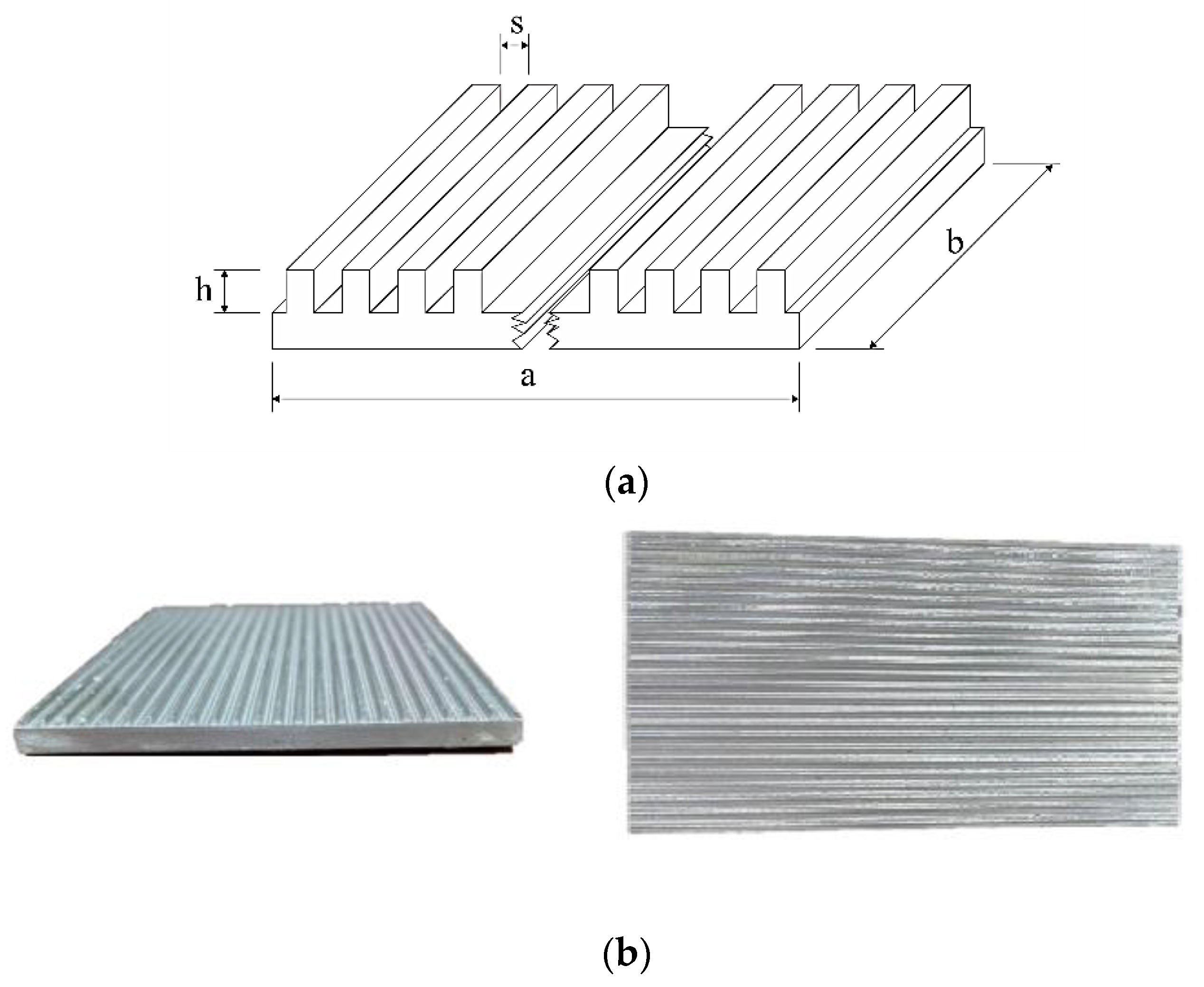

| Test Case | Profile | s (mm) | h (mm) | Aext | Abase | Atot | Atot/Abase |
|---|---|---|---|---|---|---|---|
| R1 | Rectangular | 1 | 0.95 | 3602.4 | 4779 | 8381.4 | 1.75 |
| R1.5 | Rectangular | 1.5 | 0.925 | 3507.6 | 4779 | 8286.6 | 1.73 |
| T1 | Trapezoidal | 1 | 0.95 | 2777.0 | 4779 | 7556.0 | 1.58 |
| T1.5 | Trapezoidal | 1.5 | 0.925 | 2685.45 | 4779 | 7464.45 | 1.56 |
| Properties | Values |
|---|---|
| Boiling point (K) | 334.15 |
| Specific heat (J/kg∙K) | 1170 |
| Latent heat vaporisation (kJ/kg) | 112 |
| Thermal conductivity (W/m∙K) | 0.068 |
| Liquid density (kg/m3) | 1418.64 |
| Vapour density (kg/m3) | 0.98 |
| Kinematic viscosity (m2/s) | 3.008 × 10−7 |
| Test Case | (hb, W/m2·K) | Hydraulic Diameter (Dh, mm) |
|---|---|---|
| R1 | 4505.64 | 1.31 |
| R1.5 | 3635.95 | 1.66 |
| T1 | 4070.53 | 1.60 |
| T1.5 | 3433.79 | 1.90 |
| Time (s) | G = 13.1 kg/m2∙s | G = 19.7 kg/m2∙s |
|---|---|---|
| 0.4 |  |  |
| 0.8 |  |  |
| 1.2 |  |  |
| 1.6 |  |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pranoto, I.; Rahman, M.A.; Wicaksana, C.D.; Wibisono, A.E.; Fauzun; Widyatama, A. Flow Boiling Heat Transfer Performance and Boiling Phenomena on Various Straight Fin Configurations. Fluids 2023, 8, 102. https://doi.org/10.3390/fluids8030102
Pranoto I, Rahman MA, Wicaksana CD, Wibisono AE, Fauzun, Widyatama A. Flow Boiling Heat Transfer Performance and Boiling Phenomena on Various Straight Fin Configurations. Fluids. 2023; 8(3):102. https://doi.org/10.3390/fluids8030102
Chicago/Turabian StylePranoto, Indro, Muhammad Aulia Rahman, Cahya Dhika Wicaksana, Alan Eksi Wibisono, Fauzun, and Arif Widyatama. 2023. "Flow Boiling Heat Transfer Performance and Boiling Phenomena on Various Straight Fin Configurations" Fluids 8, no. 3: 102. https://doi.org/10.3390/fluids8030102
APA StylePranoto, I., Rahman, M. A., Wicaksana, C. D., Wibisono, A. E., Fauzun, & Widyatama, A. (2023). Flow Boiling Heat Transfer Performance and Boiling Phenomena on Various Straight Fin Configurations. Fluids, 8(3), 102. https://doi.org/10.3390/fluids8030102









