Kinetic Features of Cd and Zn Cathodic Formations in the Membrane Electrolysis Process
Abstract
1. Introduction
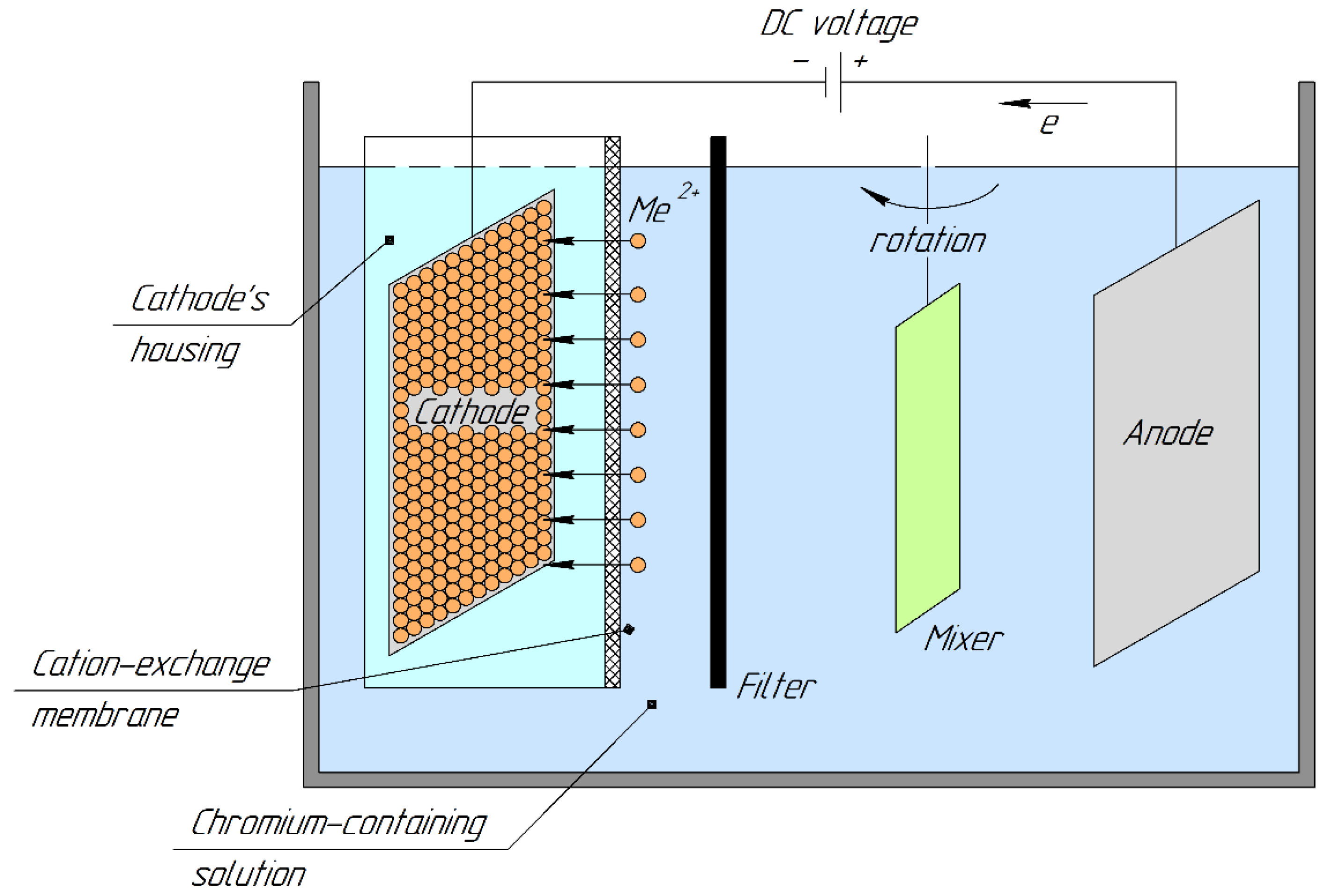
2. Materials and Methods
2.1. Experimental Studies
2.2. Mathematical Modeling
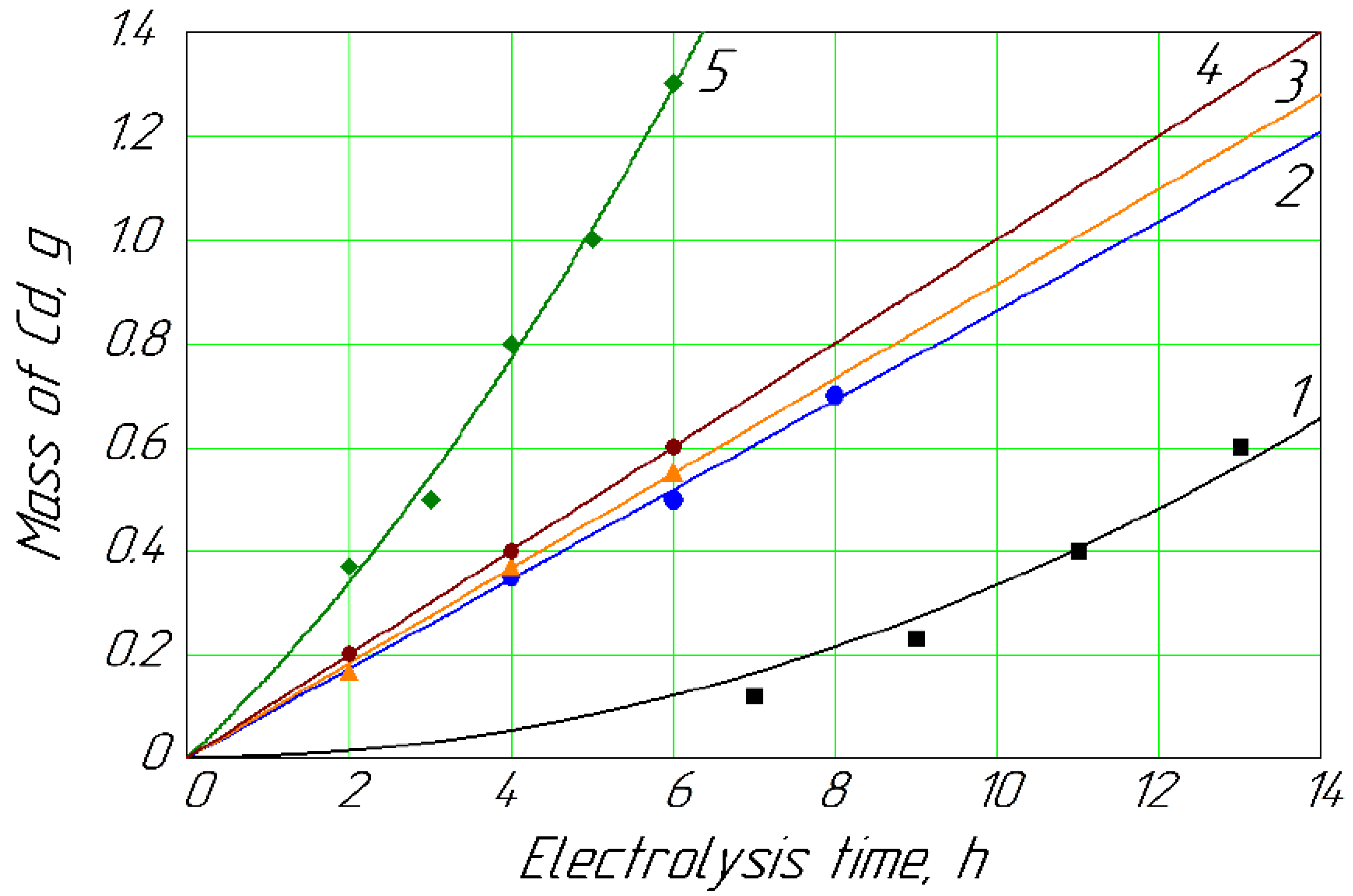
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Briffa, J.; Sinagra, E.; Blundell, R. Heavy metal pollution in the environment and their toxicological effects on humans. Heliyon 2020, 6, e04691. [Google Scholar] [CrossRef] [PubMed]
- Irannajad, M.; Haghighi, H.K.; Soleimanipour, M. Adsorption of Zn2+, Cd2+ and Cu2+ on zeolites coated by manganese and iron oxides. Physicochem. Probl. Miner. Proce 2016, 52, 2016–2894. [Google Scholar] [CrossRef]
- Shi, Y.; Jiang, K.-X.; Zhang, T.-A.; Zhu, X.-F. Simultaneous and clean separation of titanium, iron, and alumina from coal fly ash in one spot: Electrolysis-hydrolysis method. Sep. Purif. Technol. 2022, 294, 121247. [Google Scholar] [CrossRef]
- Kruglikov, S.S. Application of electromembrane processes in chromium electroplating technology. Pet. Chem. 2016, 56, 969–976. [Google Scholar] [CrossRef]
- Fischer, R.; Seidel, H.; Rahner, D.; Morgenstern, P.; Löser, C. Elimination of Heavy Metals from Leachates by Membrane Electrolysis. Eng. Life Sci. 2004, 4, 438–444. [Google Scholar] [CrossRef]
- Younesi, S.; Alimadadi, H.; Alamdari, E.K.; Marashi, S. Kinetic mechanisms of cementation of cadmium ions by zinc powder from sulphate solutions. Hydrometallurgy 2006, 84, 155–164. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, Z.; Qiu, H.; Yang, W.; Zhao, Z.; Zhao, J.; Cui, G. Pursuit of reversible Zn electrochemistry: A time-honored challenge towards low-cost and green energy storage. NPG Asia Mater. 2020, 12, 7. [Google Scholar] [CrossRef]
- Kubáň, P.; Boček, P. The effects of electrolysis on operational solutions in electromembrane extraction: The role of acceptor solution. J. Chromatogr. A 2015, 1398, 11–19. [Google Scholar] [CrossRef]
- Fu, Y.; Li, Y.; Zhang, X.; Liu, Y.; Qiao, J.; Zhang, J.; Wilkinson, D.P. Novel hierarchical SnO2 microsphere catalyst coated on gas diffusion electrode for enhancing energy efficiency of CO2 reduction to formate fuel. Appl. Energy 2016, 175, 536–544. [Google Scholar] [CrossRef]
- Danaee, I.; Jafarian, M.; Forouzandeh, F.; Gobal, F.; Mahjani, M.G. Electrocatalytic oxidation of methanol on Ni and NiCu alloy modified glassy carbon electrode. Int. J. Hydrogen Energy 2008, 33, 4367–4376. [Google Scholar] [CrossRef]
- Plevová, M.; Hnát, J.; Žitka, J.; Pavlovec, L.; Otmar, M.; Bouzek, K. Optimization of the membrane electrode assembly for an alkaline water electrolyser based on the catalyst-coated membrane. J. Power Sources 2022, 539, 231476. [Google Scholar] [CrossRef]
- Chen, G.; An, Y.; Liu, S.; Sun, F.; Qi, H.; Wu, H.; He, Y.; Liu, P.; Shi, R.; Zhang, J.; et al. Highly accessible and dense surface single metal FeN4 active sites for promoting the oxygen reduction reaction. Energy Environ. Sci. 2022, 15, 2619–2628. [Google Scholar] [CrossRef]
- Halim, E.M.; Demir-Cakan, R.; Perrot, H.; El Rhazi, M.; Sel, O. Interfacial charge storage mechanisms of composite electrodes based on poly(ortho-phenylenediamine)/carbon nanotubes via advanced electrogravimetry. J. Chem. Phys. 2022, 156, 124703. [Google Scholar] [CrossRef]
- Luin, U.; Valant, M. Electrolysis energy efficiency of highly concentrated FeCl2 solutions for power-to-solid energy storage technology. J. Solid State Electrochem. 2022, 26, 929–938. [Google Scholar] [CrossRef]
- Serdiuk, V.O.; Sklavbinskyi, V.I.; Bolshanina, S.B.; Ivchenko, V.D.; Qasim, M.N.; Zaytseva, K.O. Membrane Processes during the Regeneration of Galvanic Solution. J. Eng. Sci. 2018, 5, F1–F6. [Google Scholar] [CrossRef] [PubMed]
- Stanislaw, L.N.; Gerhardt, M.R.; Weber, A.Z. Modeling Electrolyte Composition Effects on Anion-Exchange-Membrane Water Electrolyzer Performance. ECS Trans. 2019, 92, 767–779. [Google Scholar] [CrossRef]
- Ahmed, M.E.I.; Huang, K.-L.; Holsen, T.M. Nafion-117 Behavior during Cation Separation from Spent Chromium Plating Solutions. Ind. Eng. Chem. Res. 2009, 48, 6805–6810. [Google Scholar] [CrossRef]
- Tamburini, A.; La Barbera, G.; Cipollina, A.; Micale, G.; Ciofalo, M. CFD prediction of scalar transport in thin channels for reverse electrodialysis. Desalination Water Treat. 2015, 55, 3424–3445. [Google Scholar] [CrossRef]
- Liu, Y.; Vijayakumar, P.; Liu, Q.; Sakthivel, T.; Chen, F.; Dai, Z. Shining Light on Anion-Mixed Nanocatalysts for Efficient Water Electrolysis: Fundamentals, Progress, and Perspectives. Nano-Micro Lett. 2022, 14, 219–242. [Google Scholar] [CrossRef]
- Veerman, J.; Post, J.W.; Saakes, M.; Metz, S.J.; Harmsen, G.J. Reducing power losses caused by ionic shortcut currents in reverse electrodialysis stacks by a validated model. J. Membr. Sci. 2008, 310, 418–430. [Google Scholar] [CrossRef]
- Liang, Y.; Chapman, M.; Weihs, G.F.; Wiley, D. CFD modelling of electro-osmotic permeate flux enhancement on the feed side of a membrane module. J. Membr. Sci. 2014, 470, 378–388. [Google Scholar] [CrossRef]
- Vakal, V.; Pavlenko, I.; Vakal, S.; Hurets, L.; Ochowiak, M. Mathematical Modeling of Nutrient Release from Capsulated Fertilizers. Period. Polytech. Chem. Eng. 2020, 64, 562–568. [Google Scholar] [CrossRef]



| Concentration a, kg/mol | Reynolds Number Re | Mass Rate Parameters | |
|---|---|---|---|
| b, g/h | c, 10−3 g/h2 | ||
| 0.013 | – | 0.001 | 3.342 |
| 0.018 | – | 0.083 | 0.516 |
| 0.022 | – | 0.084 | 0.790 |
| 0.026 | 392 | 0.085 | – |
| 0.026 | 2975 | 0.140 | – |
| 0.027 | – | 0.098 | 0.263 |
| 0.089 | – | 0.147 | 11.447 |
| 0.089 | 392 | 0.207 | – |
| 0.089 | 2975 | 0.327 | – |
| Concentration a, kg/mol | Reynolds Number Re | Mass Rate Parameters | |
|---|---|---|---|
| b, g/h | c, 10−3 g/h2 | ||
| 0.013 | – | 0.008 | 1.129 |
| 0.018 | – | 0.049 | 6.907 |
| 0.022 | – | 0.061 | 8.637 |
| 0.026 | 392 | 0.072 | – |
| 0.026 | 2975 | 0.075 | – |
| 0.027 | – | 0.073 | 10.332 |
| 0.089 | – | 0.101 | 18.929 |
| 0.089 | 392 | 0.113 | – |
| 0.089 | 2975 | 0.128 | – |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Serdiuk, V.; Pavlenko, I.; Bolshanina, S.; Sklabinskyi, V.; Włodarczak, S.; Krupińska, A.; Matuszak, M.; Bielecki, Z.; Ochowiak, M. Kinetic Features of Cd and Zn Cathodic Formations in the Membrane Electrolysis Process. Fluids 2023, 8, 74. https://doi.org/10.3390/fluids8020074
Serdiuk V, Pavlenko I, Bolshanina S, Sklabinskyi V, Włodarczak S, Krupińska A, Matuszak M, Bielecki Z, Ochowiak M. Kinetic Features of Cd and Zn Cathodic Formations in the Membrane Electrolysis Process. Fluids. 2023; 8(2):74. https://doi.org/10.3390/fluids8020074
Chicago/Turabian StyleSerdiuk, Vasyl, Ivan Pavlenko, Svitlana Bolshanina, Vsevolod Sklabinskyi, Sylwia Włodarczak, Andżelika Krupińska, Magdalena Matuszak, Zdzisław Bielecki, and Marek Ochowiak. 2023. "Kinetic Features of Cd and Zn Cathodic Formations in the Membrane Electrolysis Process" Fluids 8, no. 2: 74. https://doi.org/10.3390/fluids8020074
APA StyleSerdiuk, V., Pavlenko, I., Bolshanina, S., Sklabinskyi, V., Włodarczak, S., Krupińska, A., Matuszak, M., Bielecki, Z., & Ochowiak, M. (2023). Kinetic Features of Cd and Zn Cathodic Formations in the Membrane Electrolysis Process. Fluids, 8(2), 74. https://doi.org/10.3390/fluids8020074












