The Effects of Buoyancy on Laminar Heat Transfer Rates to Supercritical CO2 in Vertical Upward Flows
Abstract
:1. Introduction
- In contrast to turbulent flows, buoyancy effects in vertical, upward laminar flows can result in an augmentation in heat transfer rates to sCO2 near its TPC. Therefore, the primary variables responsible for this augmentation need to be identified/recognized.
- There is a lack of correlation with the laminar regime allowing us to exploit these buoyancy-induced sCO2 heat transfer enhancement characteristics by enabling us to identify the location of the TPC pinch point within a heat exchanger, as well as the variables controlling these buoyancy effects. For instance, correlations for the Nusselt number (Nu) have been proposed by Dang and Hihara [13], as well as Viswanathan and Krishnamoorthy [14], in terms of the ratios of thermophysical properties evaluated at wall and bulk fluid temperatures. However, neither of these correlations account for the effects of buoyancy. While the effects of buoyancy in mini channels are generally small, this effect needs to be assessed rigorously for a range of pipe diameters and heat fluxes.
- While the buoyancy criterion for the turbulent regime have been well discussed in the literature [9], their validity in the laminar regime needs to be assessed to enable us to select an appropriate correlation for use in our analysis.
2. Materials and Methods
3. Results and Discussion
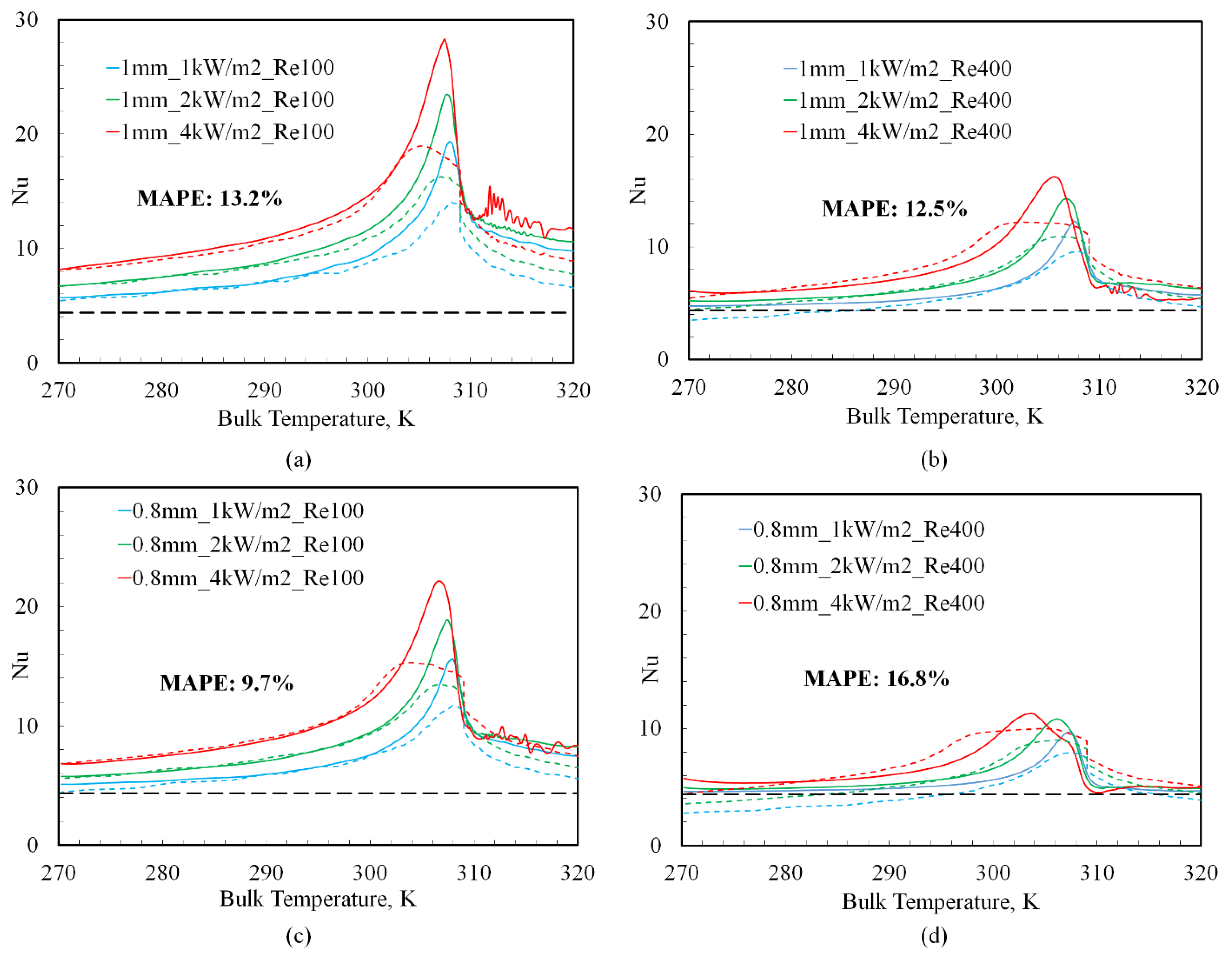
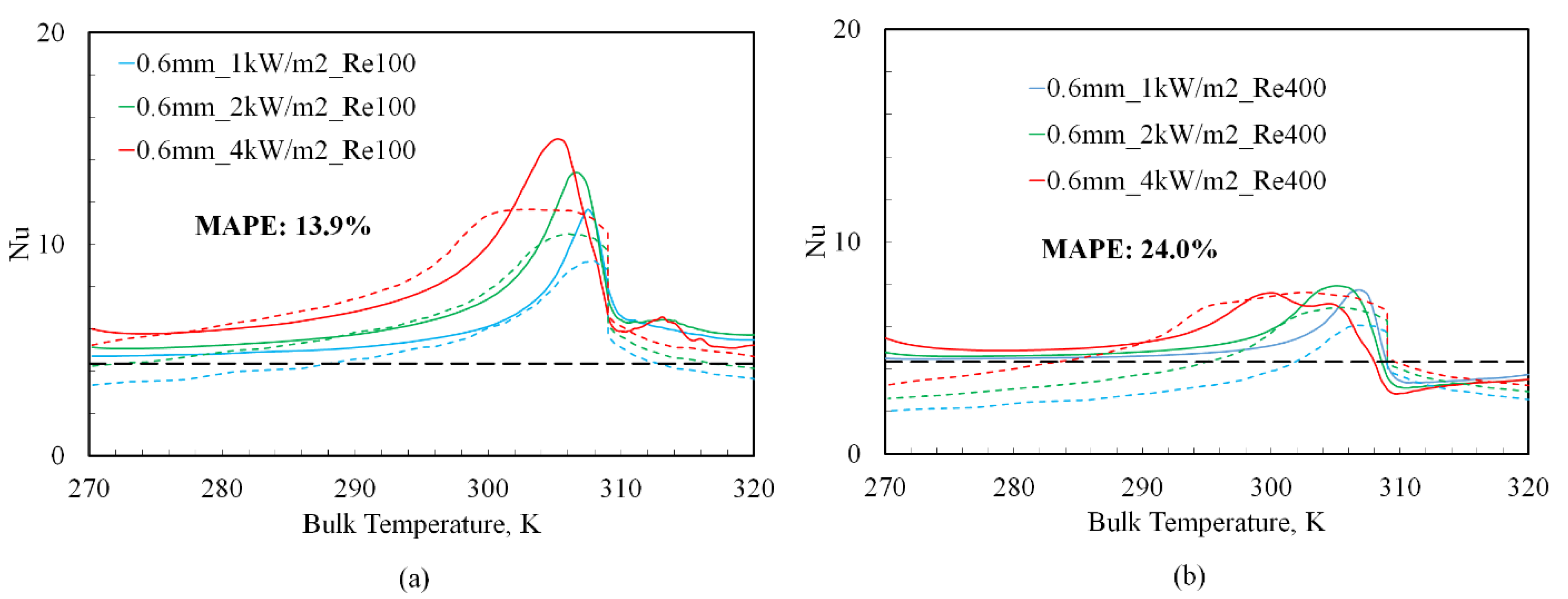
3.1. Effect of Variations in Heat Flux, Inlet Re, and Diameter on Nu
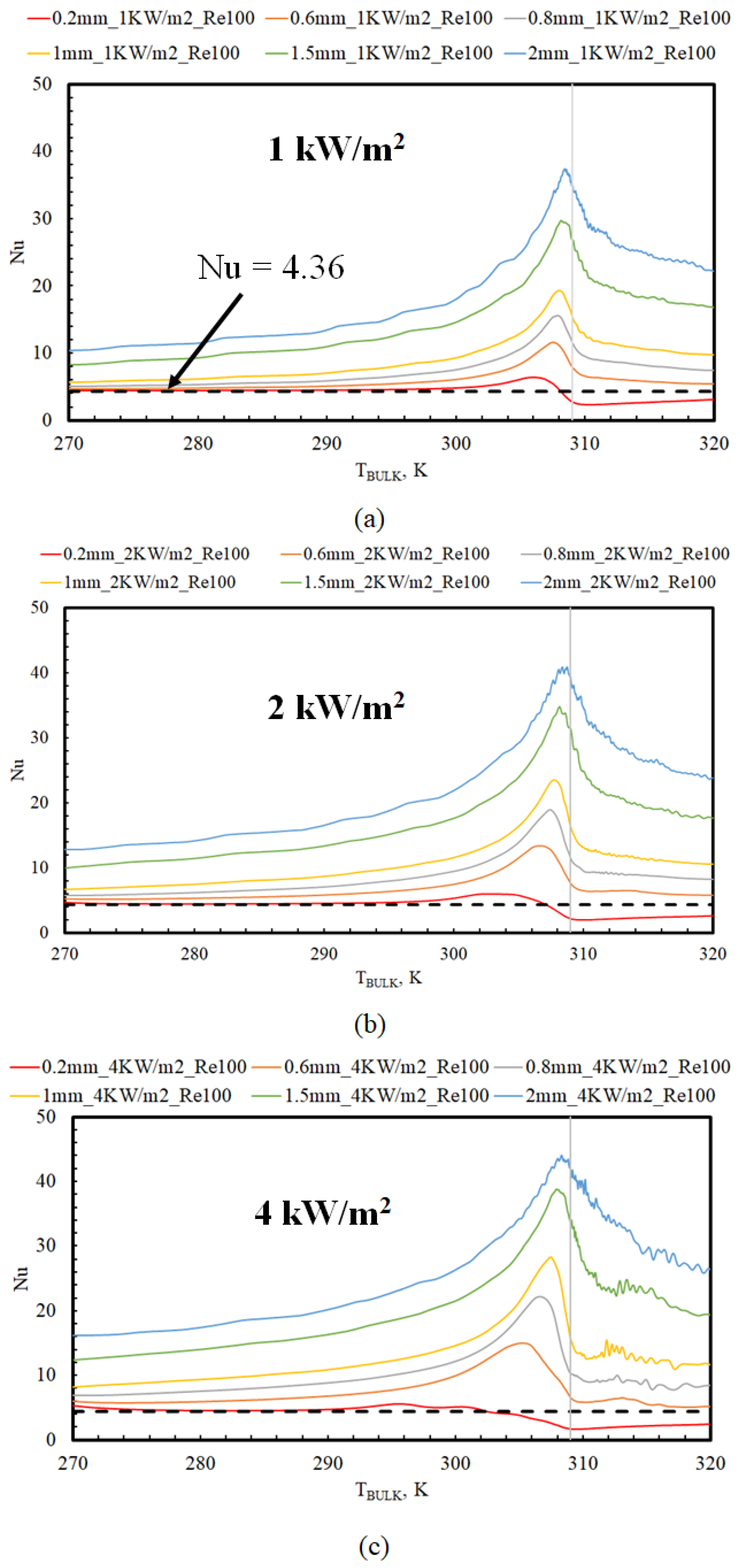
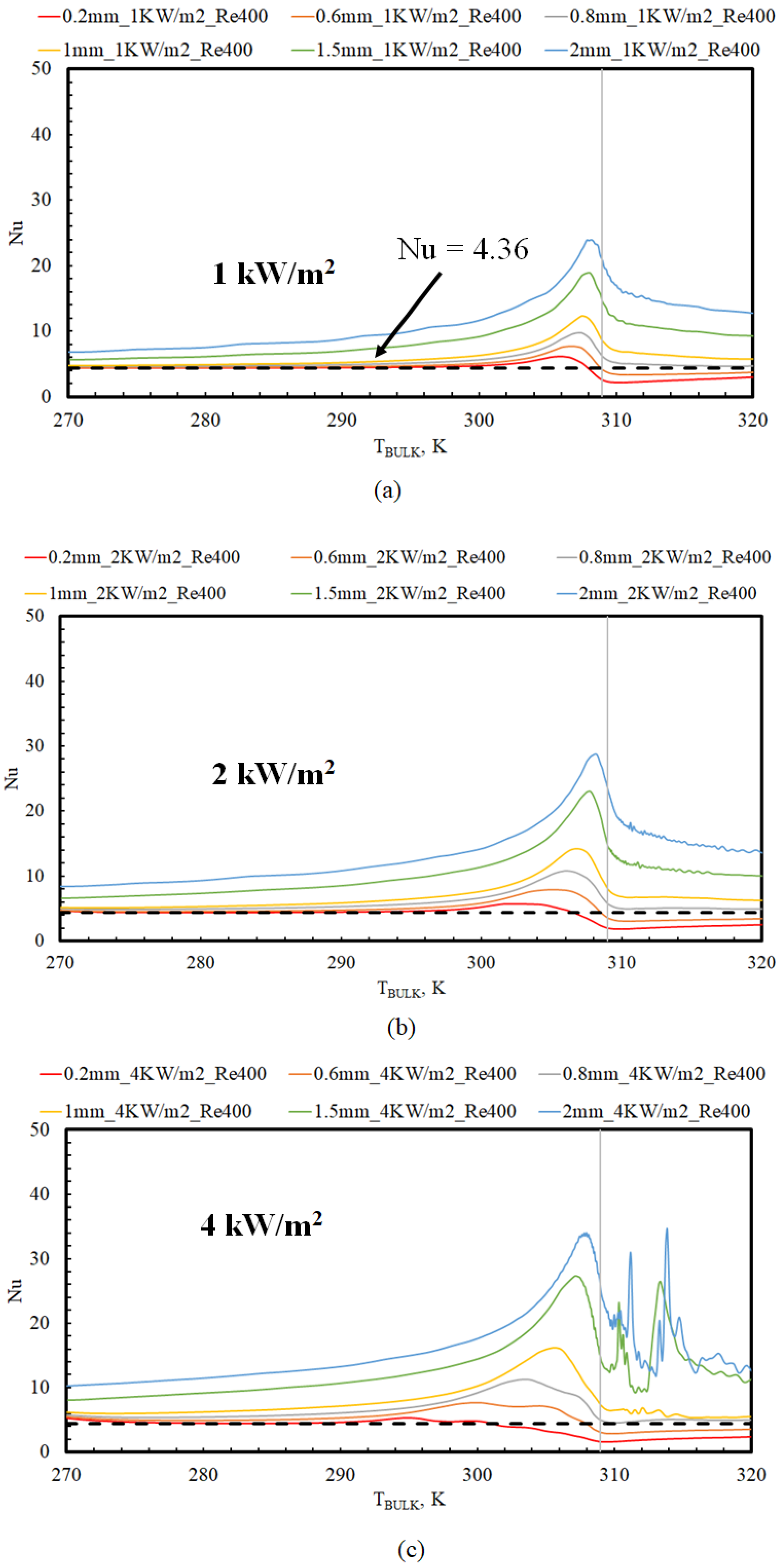
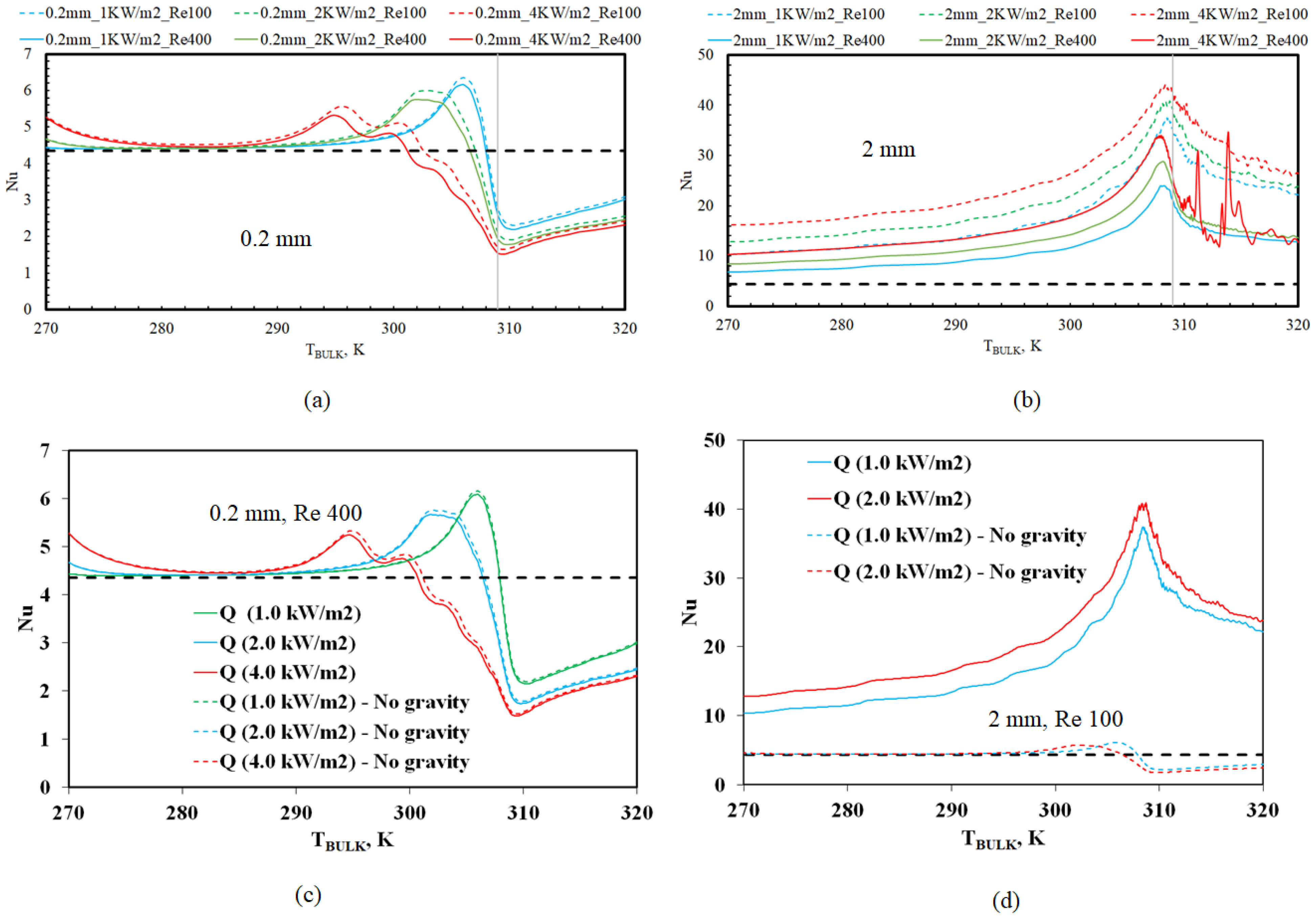
- For TBULK < 285 K: Thermophysical property variations are gradual in this region (cf. Figure 1a). Therefore, the local Nu should ideally approach the value of 4.36 corresponding to fully developed, constant property, laminar flow conditions (indicated by the horizontal dotted lines in Figure 2 and Figure 3). Further, this should be independent of inlet Re, tube diameters, or the imposed fluxes. However, Nu was close to 4.36 in this region across all fluxes only for the tube diameter of 0.2 mm and for diameters less than 1.5 mm when the inlet Re was 400. This indicates that the buoyancy to convection effects are larger with a lower inlet Re and larger tube diameters, as expected from Equation (9). At larger tube diameters and lower inlet Re (>0.2 mm and >1.5 mm for Re 100), the local Nu in this bulk temperature region was greater than 4.36, indicating that buoyancy is augmenting the heat transfer rates. In addition, the Nu is also a function of tube diameter (D) and the imposed heat flux (Q), as seen in Figure 2 and Figure 3. This is attributed to larger differences in wall and bulk fluid properties at larger heat fluxes and the strong dependence of Gr on the tube diameter (cf. Equation (10)).
- For 285 K < TBULK < 310 K: There are wide variations in the thermophysical properties in this region (cf. Figure 1a). Therefore, Nu increases sharply (beyond 4.36). The extent of these Nu deviations (from the constant property flow value of 4.36), before and after TPC, were again found to be functions of the imposed heat fluxes (Q), tube diameter (D), and inlet Re. Nu reaches a peak value when TBULK is close to TPC across all scenarios.
- For TBULK > 310 K: Thermophysical property variations are again gradual in this region (cf. Figure 1a). However, Nu asymptotically approaches different values. In most cases, Nu attains a value much higher than the constant property fully developed flow value of 4.36, depending on the imposed heat flux and tube diameters. This points to buoyancy effects upstream affecting the flow behavior downstream. However, in some cases (tube diameters of 0.2 mm and for diameters less than 0.8 mm when the inlet Re was 400), Nu drops below 4.36 in this region and slowly recovers, as observed in previous studies where the influence of gravity was ignored [14].
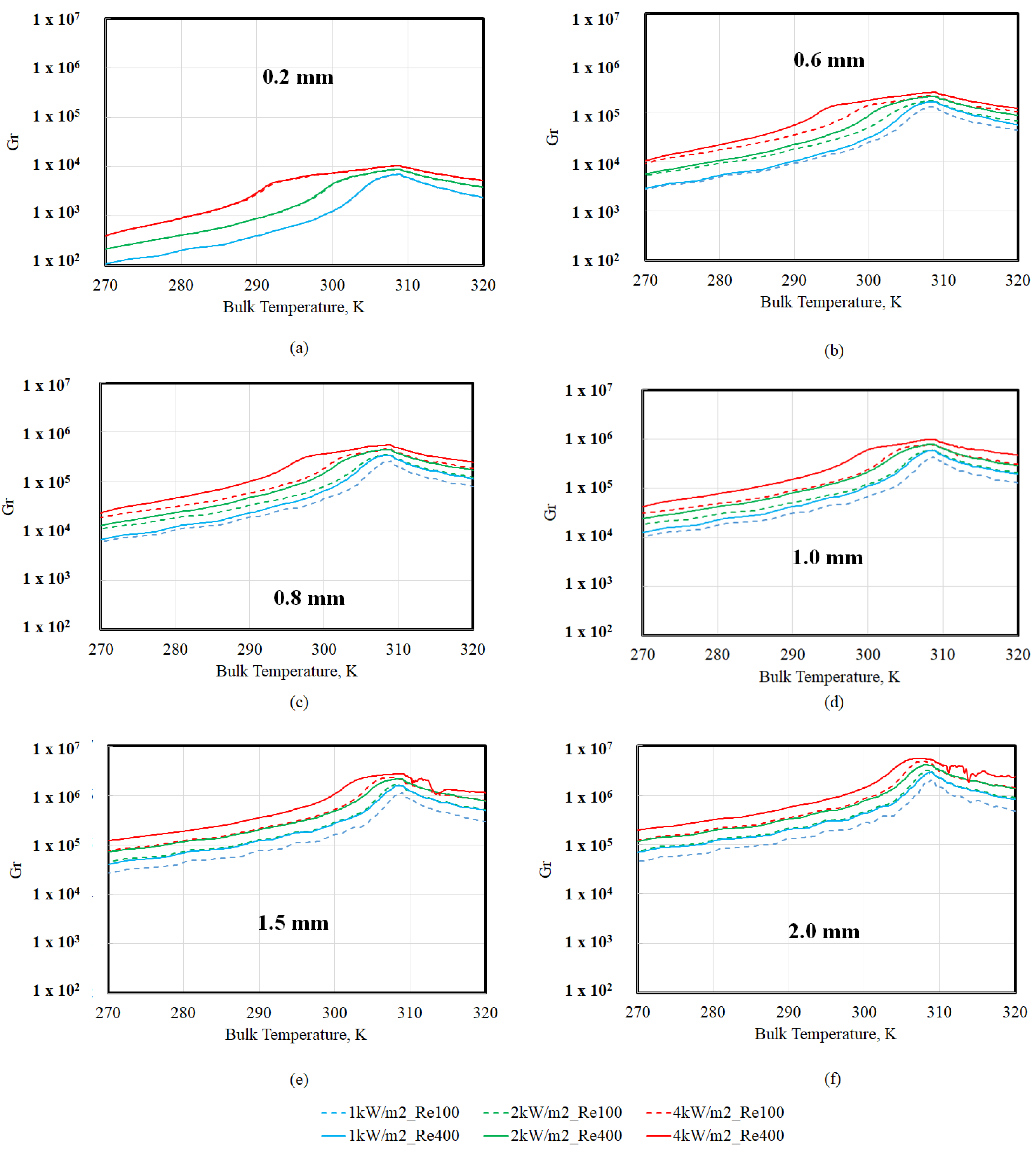
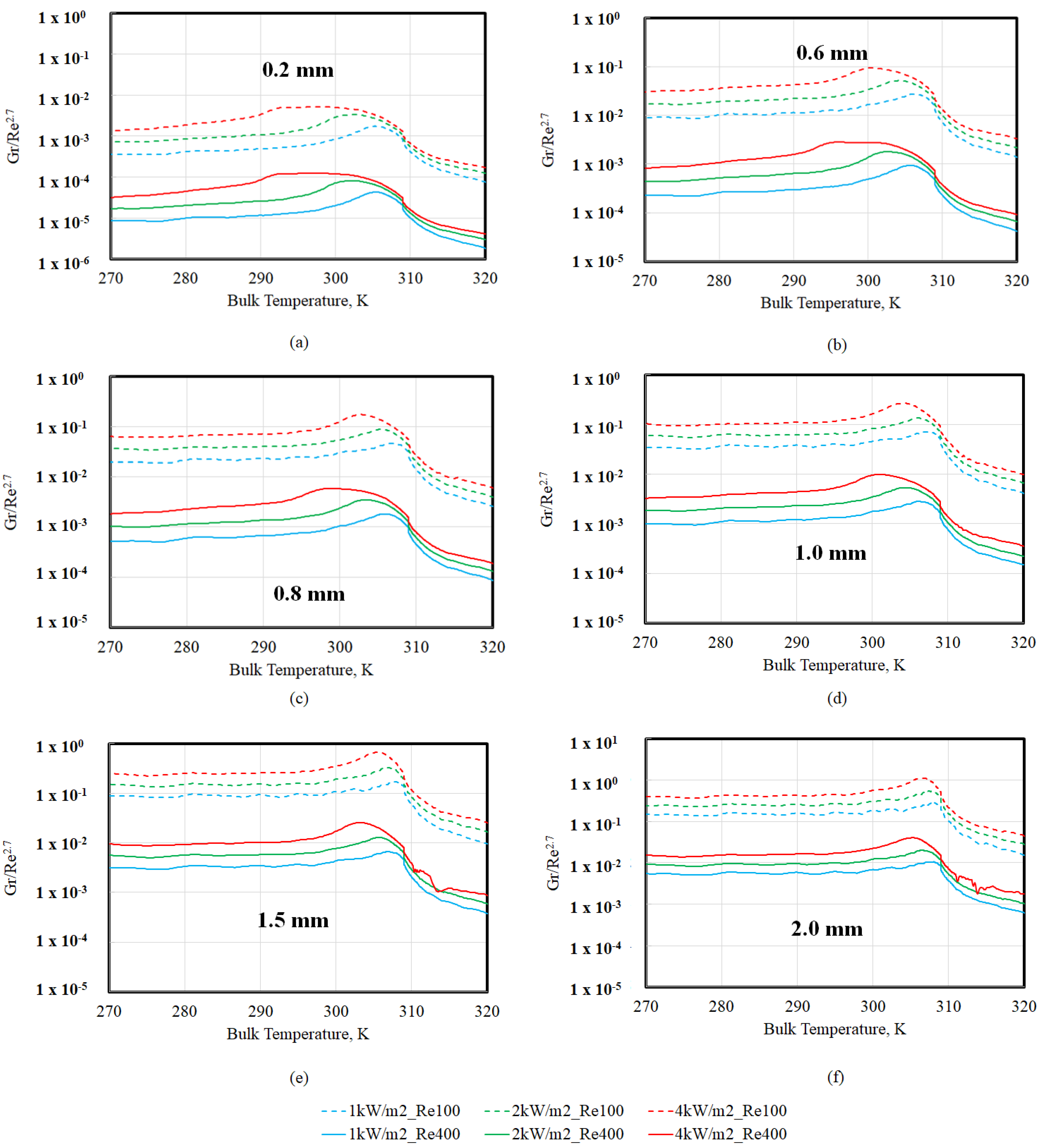
3.2. Quantifying Grashof Numbers and Arriving at Buoyancy Criterion
3.3. A New Correlation for Laminar Mixed Convection sCO2 Heat Transfer
4. Conclusions
- When 265 K < TBULK < 285 K, the thermophysical property variations are gradual, and the local Nu should ideally approach the value of 4.36. However, this was observed to be true only for tube diameter of 0.2 mm. At tube diameters greater than 0.2 mm, the local Nu in this bulk temperature region was greater than 4.36 and was also a function of tube diameter (D), the imposed heat flux (Q), and inlet Re. Further, the local Nu was inversely proportional to the Re and directly proportional to tube diameter and heat flux. This indicates the role played by buoyancy and Gr in this region. This is because, in addition to the direct dependence of Gr on the third-power of the tube diameter, an increase in heat flux increases the temperature difference between the wall and the bulk fluid and therefore, the density differences evaluated at these two temperatures. The fact that Nu was dependent on the inlet Re is in contrast with the results of our previous study (carried out without considering the effect of gravity) [14], which showed that Nu attains identical values, as long as “Q * D” was held constant and was independent of inlet Re.
- When 285 K < TBULK < 310 K, the thermophysical properties vary significantly, and Nu increases sharply (beyond 4.36). The extent of these Nu deviations (from 4.36), before and after TPC, were again found to be functions of the imposed heat fluxes, the tube diameter, and the inlet Re. Again, the local Nu was inversely proportional to the Re and directly proportional to the tube diameters and heat fluxes in this region.
- When TBULK > 325 K, the thermophysical property variations are gradual and asymptotically approach different values, depending on the imposed heat flux and tube diameters, pointing to buoyancy effects upstream affecting the flow behavior downstream.
- The Grashof (Gr) numbers vary sharply (by nearly two orders of magnitude) from 270 K to TPC, followed by a gradual reduction beyond TPC. Further, they were sensitive to the imposed fluxes and inlet Re, pointing to the important role played by TWALL in the observed behavior. While Gr increases with tube diameter (through its obvious third-power dependency), its magnitude across all scenarios was in the range of 102–107, which is well within the 109 laminar to turbulence transition limit for constant property fluids undergoing natural convection.
- The results indicate that buoyancy augmented laminar heat transfer rates may be expected when Gr/Re2.7 > 10−4 (Gr = Grashof number).
- A modified Nusselt number correlation in terms of (Gr/Re) is proposed and is determined to fit the observed variations within a mean absolute percentage error <15%, in most regions. While the correlation is functionally similar, with constants close to other correlations proposed for laminar, mixed convection flow involving subcritical water in a vertically heated tube, the equation identifies the major variables associated with buoyancy-induced heat transfer augmentation in the laminar regime and provides a starting point for formulating more complex correlations that incorporate additional variables for improved accuracies.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| d | diameter, mm |
| G | inlet mass flow, kg/s |
| Gr | Grashof number |
| Hin | enthalpy at inlet, J/kg |
| Nu | Nusselt number |
| Pr | Prandtl number |
| q | heat flux, W/m2 |
| Re | Reynolds number |
| T | temperature, K |
| v | velocity, m/s |
| Greek Symbols: | |
| ρ | density, kg/m3 |
| Subscripts: | |
| in | inlet |
| PC | pseudocritical |
| t | turbulent |
| w | wall |
References
- Huang, D.; Wu, Z.; Sunden, B.; Li, W. A brief review on convection heat transfer of fluids at supercritical pressures in tubes and the recent progress. Appl. Energy 2016, 162, 494–505. [Google Scholar] [CrossRef]
- Wright, S.A.; Radel, R.F.; Vernon, M.E.; Rochau, G.E.; Pickard, P.S. Operation and Analysis of a Supercritical CO2 Brayton Cycle; Sandia Report, No. SAND2010-017l; Sandia National Laboratories: Livermore, CA, USA, 2010. [Google Scholar]
- Ma, Y.; Liu, Z.; Tian, H. A review of transcritical carbon dioxide heat pump and refrigeration cycles. Energy 2013, 55, 156–172. [Google Scholar] [CrossRef]
- Kandlikar, S.G. Fundamental issues related to flow boiling in minichannels and microchannels. Exp. Therm. Fluid Sci. 2002, 26, 389–407. [Google Scholar] [CrossRef]
- Lopes, N.C.; Chao, Y.; Dasarla, V.; Sullivan, N.P.; Ricklick, M.A.; Boetcher, S.K. Comprehensive Review of Heat Transfer Correlations of Supercritical CO2 in Straight Tubes Near the Critical Point: A Historical Perspective. J. Heat Transf. 2022, 144, 120801. [Google Scholar] [CrossRef]
- Ehsan, M.M.; Guan, Z.; Klimenko, A.Y. A comprehensive review on heat transfer and pressure drop characteristics and correlations with supercritical CO2 under heating and cooling applications. Renew. Sustain. Energy Rev. 2018, 92, 658–675. [Google Scholar] [CrossRef]
- Cheng, L.; Gherhardt, R.; Thome, J.R. Analysis of supercritical CO2 cooling in macro-and micro-channels. Int. J. Refrig. 2008, 31, 1301–1316. [Google Scholar] [CrossRef]
- Yoo, J.Y. The turbulent flows of supercritical fluids with heat transfer. Annu. Rev. Fluid Mech. 2013, 45, 495–525. [Google Scholar] [CrossRef]
- Huang, D.; Li, W. A brief review on the buoyancy criteria for supercritical fluids. Appl. Therm. Eng. 2018, 131, 977–987. [Google Scholar] [CrossRef]
- Cao, X.L.; Rao, Z.H.; Liao, S.M. Laminar convective heat transfer of supercritical CO2 in horizontal miniature circular and triangular tubes. Appl. Therm. Eng. 2011, 31, 2374–2384. [Google Scholar] [CrossRef]
- Liao, S.M.; Zhao, T.S. A numerical investigation of laminar convection of supercritical carbon dioxide in vertical mini/micro tubes. Prog. Comput. Fluid Dyn. Int. J. 2002, 2, 144–152. [Google Scholar] [CrossRef]
- Zhang, X.R.; Yamaguchi, H. Forced convection heat transfer of supercritical CO2 in a horizontal circular tube. J. Supercrit. Fluids 2007, 41, 412–420. [Google Scholar] [CrossRef]
- Dang, C.; Hihara, E. Numerical study on in-tube laminar heat transfer of supercritical fluids. Appl. Therm. Eng. 2010, 30, 1567–1573. [Google Scholar] [CrossRef]
- Viswanathan, K.; Krishnamoorthy, G. The effects of wall heat fluxes and tube diameters on laminar heat transfer rates to supercritical CO2. Int. Commun. Heat Mass 2021, 123, 105197. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS Fluent Theory Guide; Release 19.1; ANSYS, Inc.: Canonsburg, PA, USA, 2018. [Google Scholar]
- Lemmon, E.W.; Huber, M.L.; McLinden, M.O. NIST Reference Fluid Thermodynamic and Transport Properties—REFPROP; U.S. Department of Commerce: Gaithersburg, ML, USA, 2010.
- Jackson, D.; Hall, W.B. Influence of buoyancy on heat transfer to fluids flowing in vertical tubes under turbulent conditions. Turbul. Forced Convect. Channels Bundles 1979, 2, 613–640. [Google Scholar]
- Abdul-Sater, H.; Krishnamoorthy, G. An assessment of radiation modeling strategies in simulations of laminar to transitional, oxy-methane, diffusion flames. Appl. Therm. Eng. 2013, 61, 507–518. [Google Scholar] [CrossRef]
- Zaim, E.H.; Nassab, S.A.G. Numerical investigation of laminar forced convection of water upwards in a narrow annulus at supercritical pressure. Energy 2010, 35, 4172–4177. [Google Scholar] [CrossRef]
- Kang, G.U.; Chung, B.J. The experimental study on transition criteria of natural convection inside a vertical pipe. Int. Commun. Heat Mass Transf. 2010, 37, 1057–1063. [Google Scholar] [CrossRef]
- Jackson, J.D.; Cotton, M.A.; Axcell, B.P. Studies of mixed convection in vertical tubes. Int. J. Heat Fluid Flow 1989, 10, 2–15. [Google Scholar] [CrossRef]
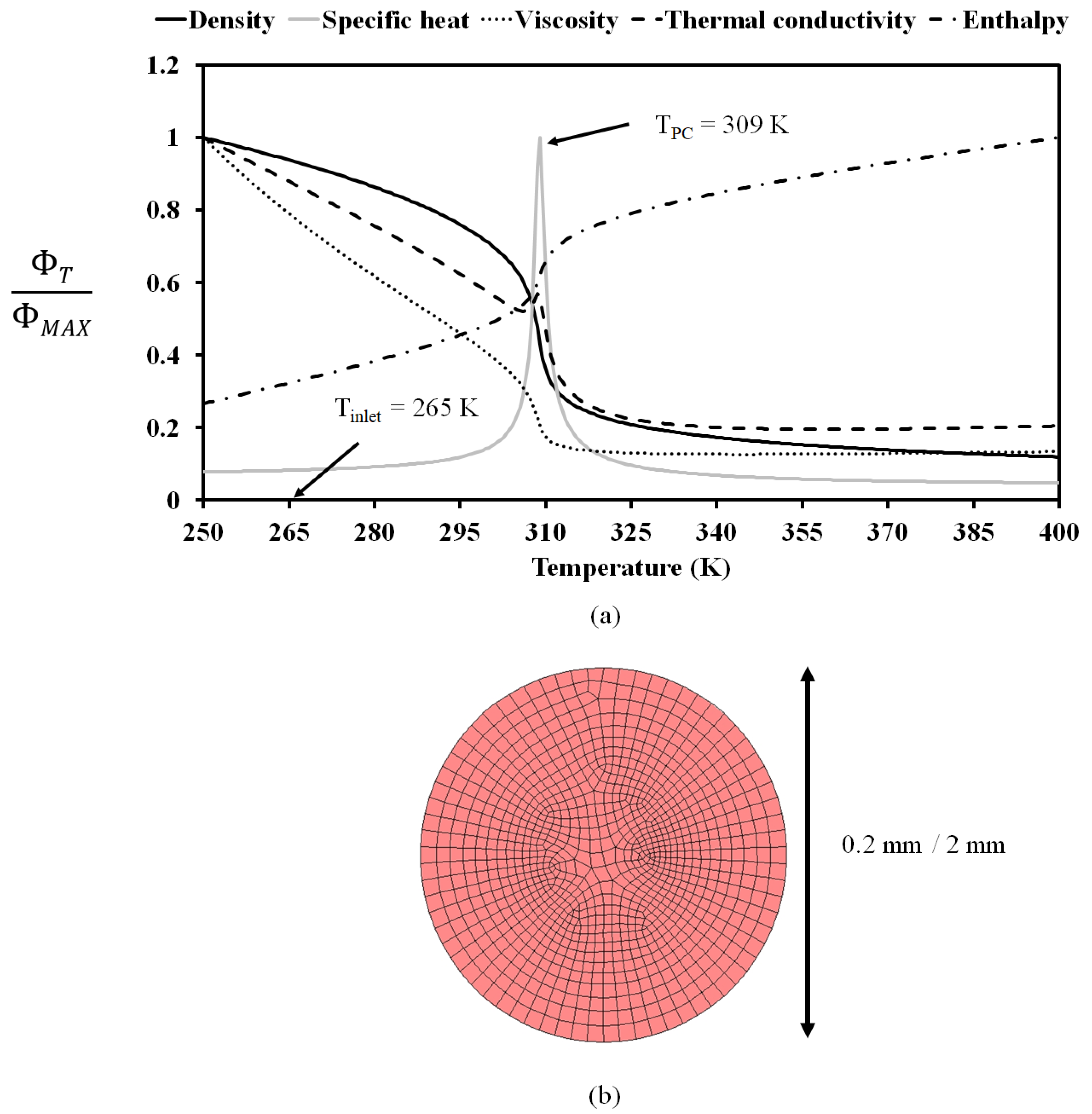


| Reference | Geometry and Orientation | Temperature, Pressure Range | Summary |
|---|---|---|---|
| Cao, Rao, Liao [10] | Numerical simulations (3D) of circular and triangular tubes (0.5 mm hydraulic diameter and length of 1000 mm), with gravity effects included | A total of 80 bars, wall temperature 25 °C, fluid inlet temperature 120 °C [cooling], inlet Reynolds number 1866 | Circumferential Nu variations were significant near the entrance region, but became more uniform farther away from the inlet (x/D = 800) and close to that of the constant property fluid flow (Nu = 3.66) due to thermal equilibrium between the fluid and wall temperatures. The bulk Nusselt (NuBULK) numbers increased close to TBULK/TPC > 0.97 for both horizontal and vertical orientations. However, a correlation capturing these effects was not proposed. |
| Liao and Zhao [11] | Numerical simulations in 2D axisymmetric co-ordinates, circular tubes 0.5 to 2.16 mm in diameter and 1000 mm in length, heating and cooling flows, with and without gravity | A total of 80 bars, Cooling flow: inlet temperature 120 °C, wall temperature 25 °C ; Heating flow: Inlet temperature 25 °C, wall temperature 90 °C | Buoyancy was found to play an important role in laminar convective heat transfer, implying the important influence of wall temperature. When TBULK was close to TPC, the Nu numbers exhibited rather large variations/deviations from those exhibited by constant-property fluids. However, a correlation for predicting the heat transfer behavior was not suggested. |
| Zhang and Yamaguchi [12] | Numerical simulations (2D-axisymmetric) of a 6 mm diameter, heated section 3.6 m long, horizontal orientation, gravity effects included | Inlet temperature 32 °C (>TPC); 80 bars; Heating condition (100–800 W/m2); Inlet Reynolds number (210) | A three-fold increase in Nu compared to that of constant property fluids (where Nu = 4.364) was observed at fully developed flow conditions. Heat transfer enhancement was found to increase with increases in the Reynolds number (Re) and heat flux (Q) for ranges of 210 ≤ Re ≤ 1800 and 100 W/m2 ≤ q ≤ 800 W/m2. Mechanisms responsible for the heat transfer enhancement were postulated, but no correlation was proposed. The buoyancy effects were ignored based on low values of Gr/Re2. |
| Diameter (mm) | Heat Flux (kW/m2) | Inlet Reynolds Number |
|---|---|---|
| 0.2 | 1, 2, 4 | 100, 400 |
| 0.6 | 1, 2, 4 | 100, 400 |
| 0.8 | 1, 2, 4 | 100, 400 |
| 1 | 1, 2, 4 | 100, 400 |
| 1.5 | 1, 2, 4 | 100, 400 |
| 2 | 1, 2, 4 | 100, 400 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viswanathan, K.; Krishnamoorthy, G. The Effects of Buoyancy on Laminar Heat Transfer Rates to Supercritical CO2 in Vertical Upward Flows. Fluids 2023, 8, 30. https://doi.org/10.3390/fluids8010030
Viswanathan K, Krishnamoorthy G. The Effects of Buoyancy on Laminar Heat Transfer Rates to Supercritical CO2 in Vertical Upward Flows. Fluids. 2023; 8(1):30. https://doi.org/10.3390/fluids8010030
Chicago/Turabian StyleViswanathan, Krishnamoorthy, and Gautham Krishnamoorthy. 2023. "The Effects of Buoyancy on Laminar Heat Transfer Rates to Supercritical CO2 in Vertical Upward Flows" Fluids 8, no. 1: 30. https://doi.org/10.3390/fluids8010030
APA StyleViswanathan, K., & Krishnamoorthy, G. (2023). The Effects of Buoyancy on Laminar Heat Transfer Rates to Supercritical CO2 in Vertical Upward Flows. Fluids, 8(1), 30. https://doi.org/10.3390/fluids8010030







