Coexistence of Inverse and Direct Energy Cascades in Faraday Waves
Abstract
:1. Introduction
2. Materials and Methods
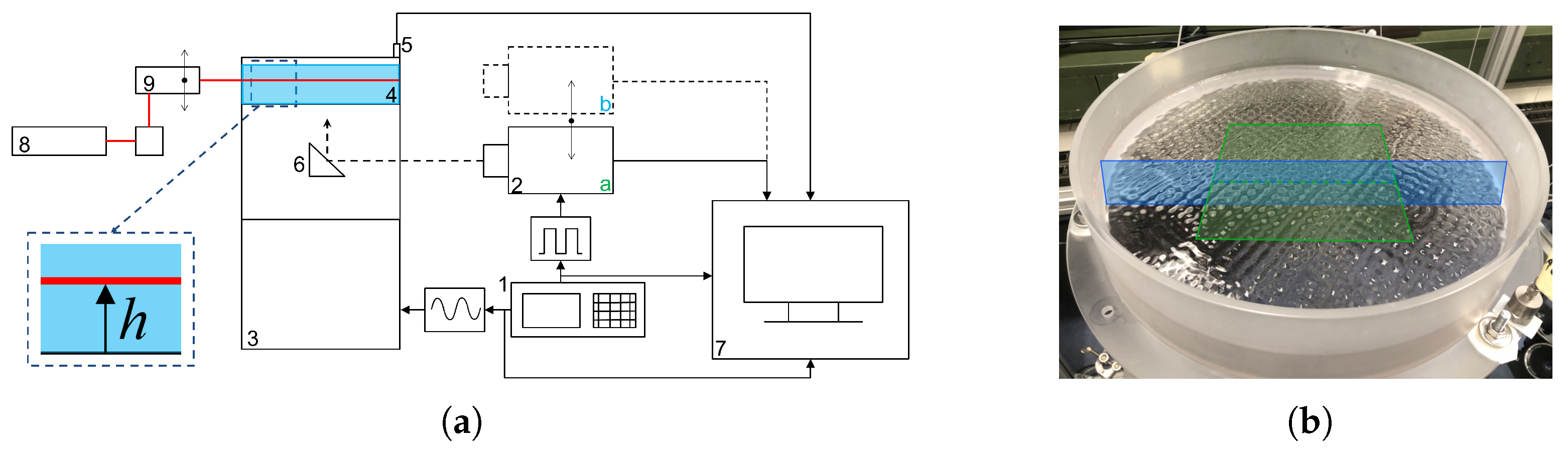
2.1. Experimental Setup
2.2. PIV Processing
2.3. Spectral Analysis of Velocity Fields
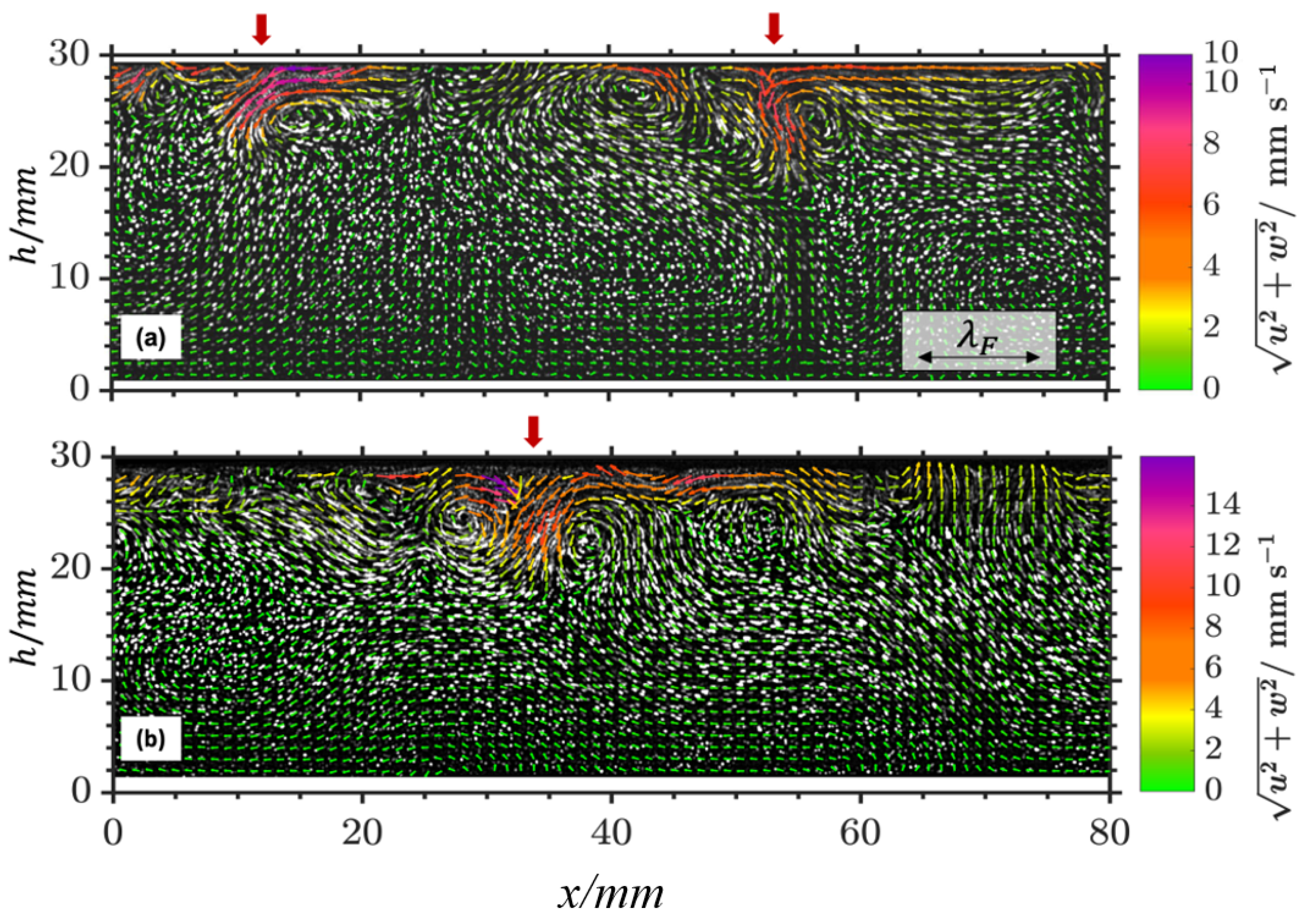
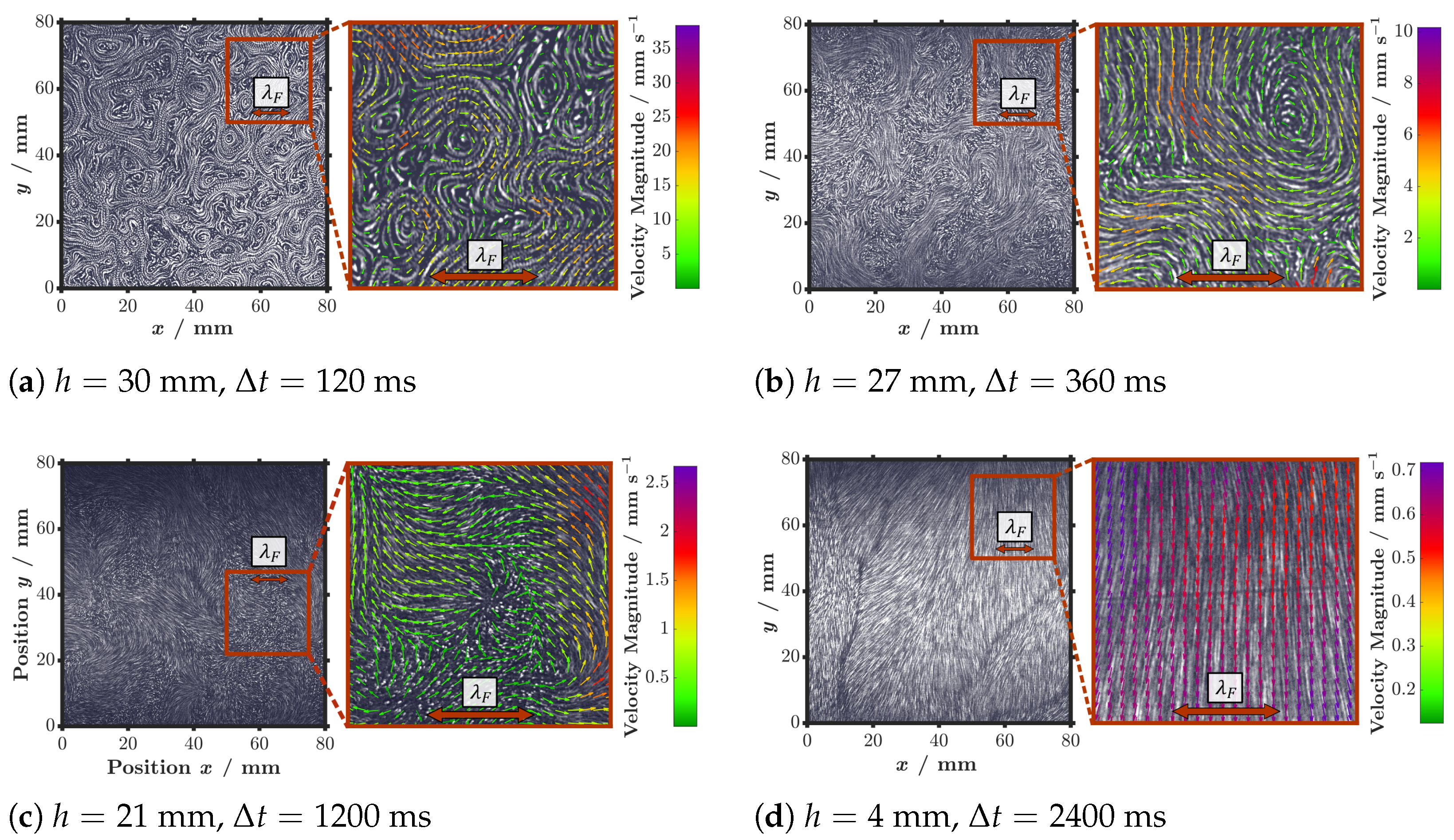
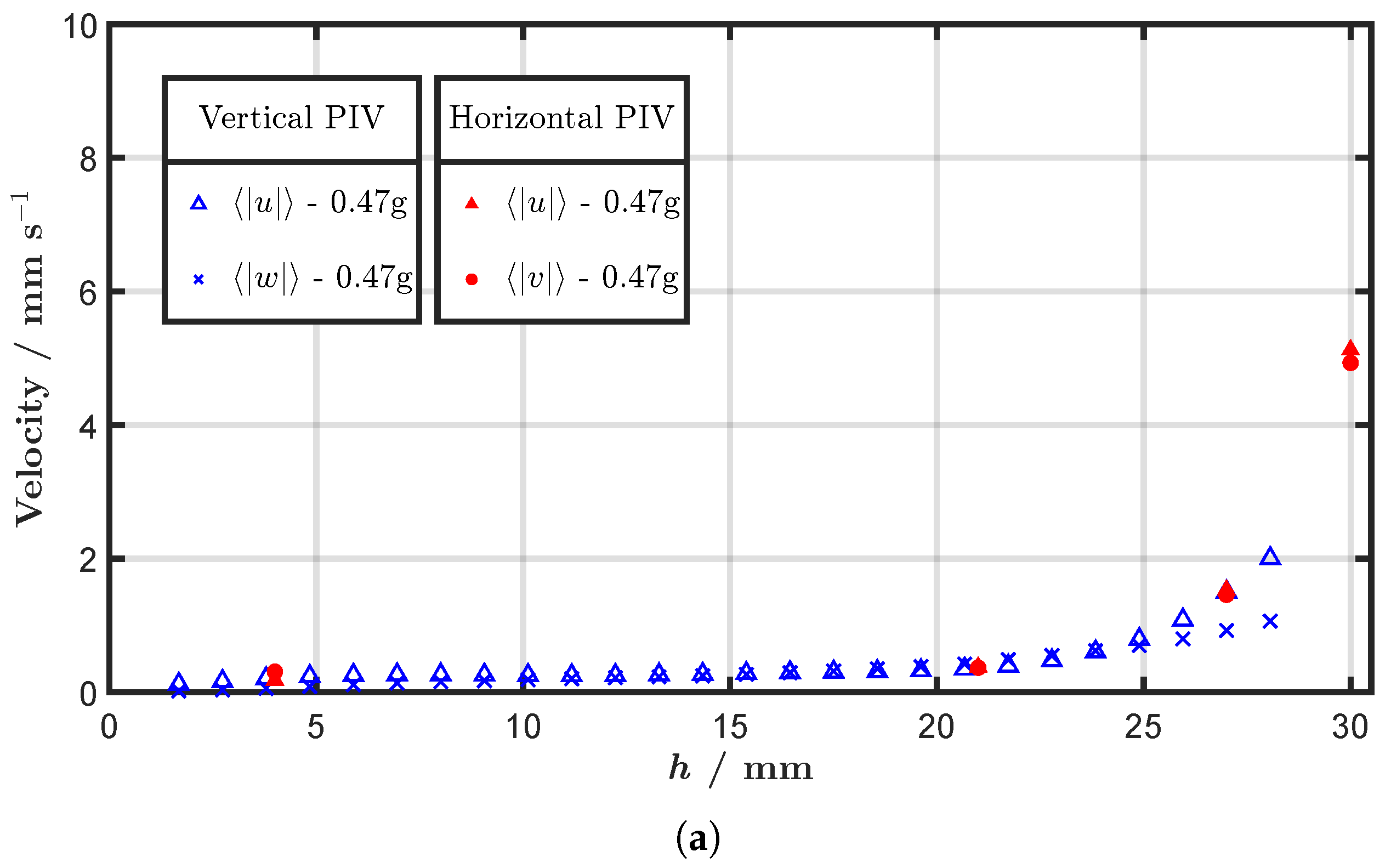
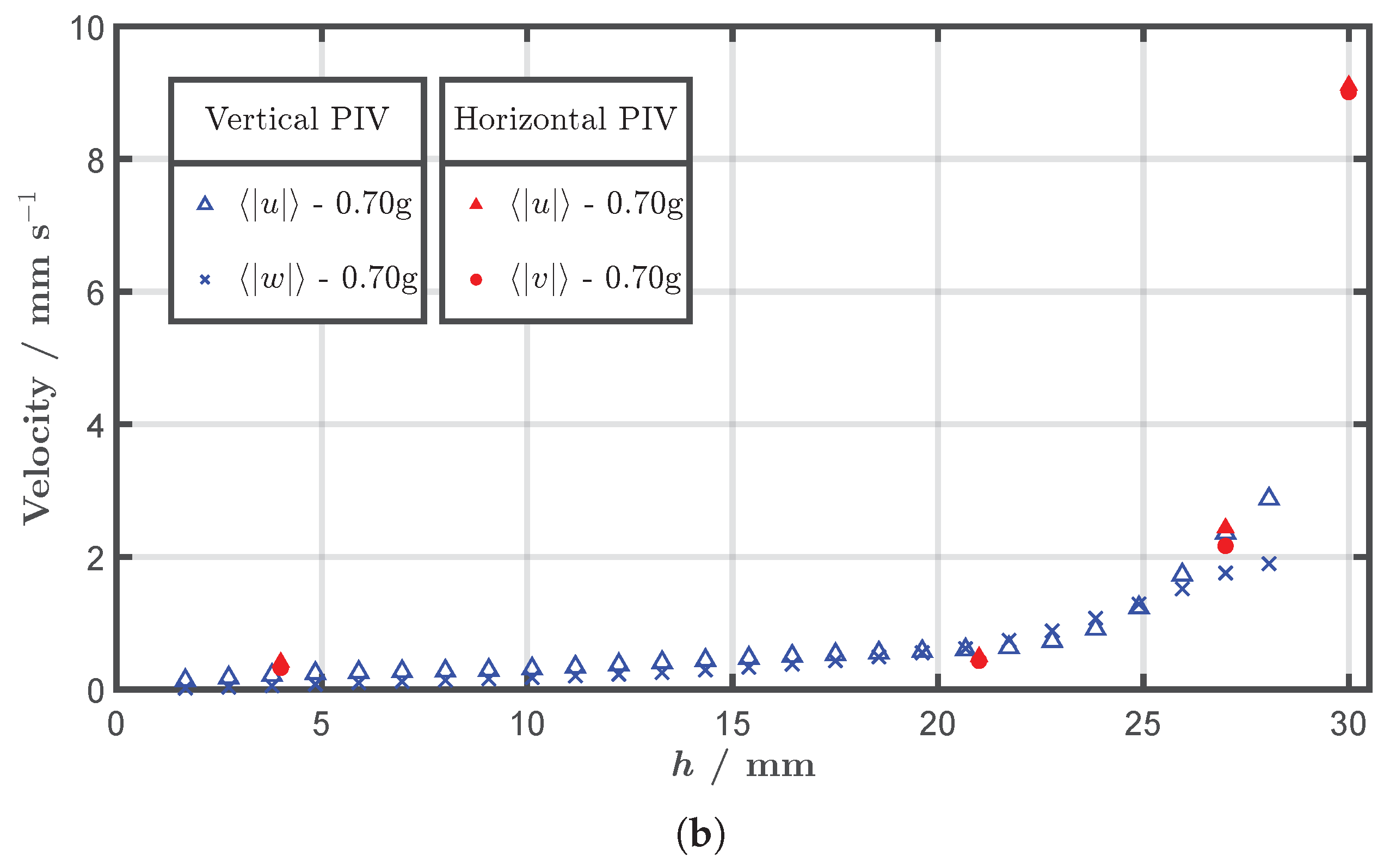
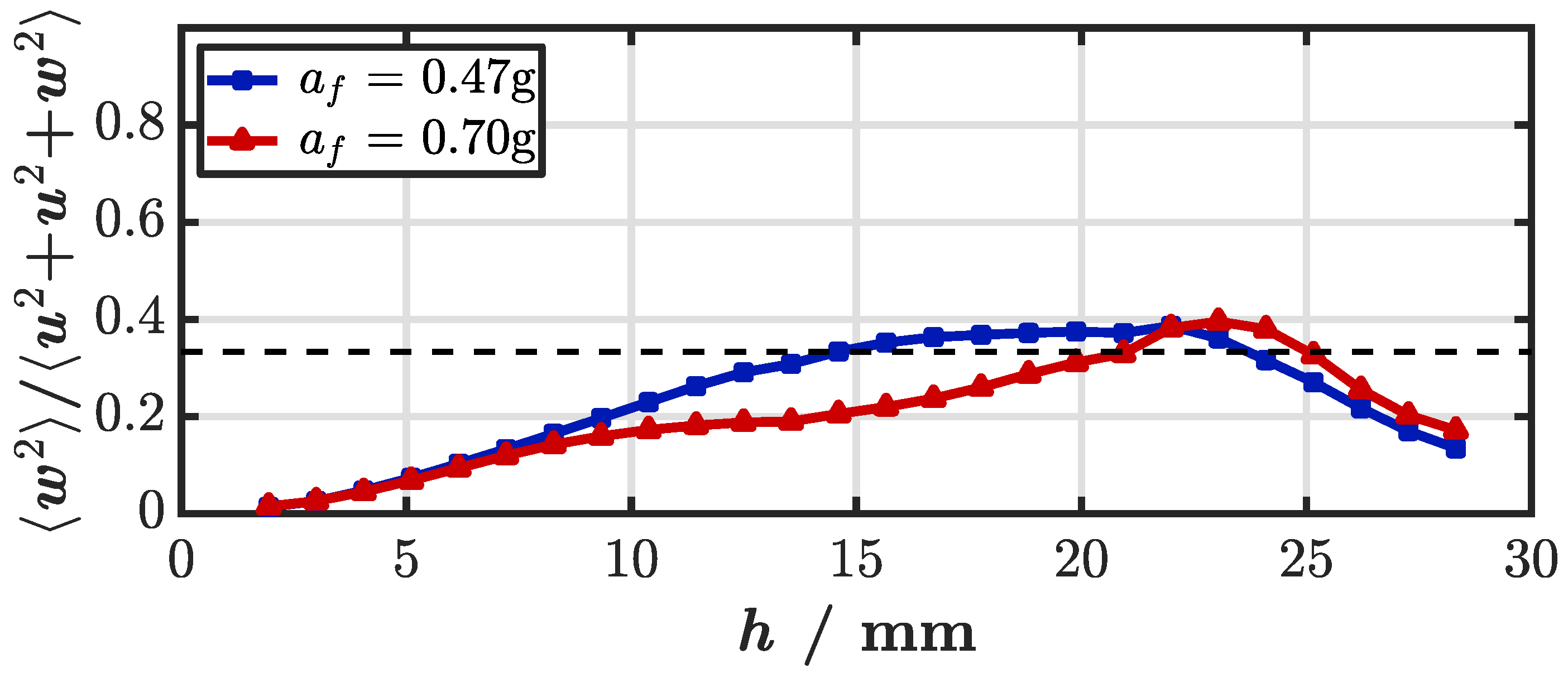
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| 2D3C | Two-dimensional three-component |
| PIV | Particle image velocimetry |
| EMD | Electromagnetically driven |
| PTV | Particle Tracking Velocimetry |
Appendix A. PIV Processing Parameters
| h | , | Conv. | N | ||||
|---|---|---|---|---|---|---|---|
| mm | m s−2 | px|mm | mm | ms | ms | px/mm | |
| 30 | 0.70 g | 14 1.13 | 169 × 127 | 40 | 7680 | 12.42 | 6 |
| 30 | 0.47 g | 14 1.13 | 169 × 127 | 40 | 7680 | 12.42 | 4 |
| 27 | 0.70 g | 14 1.21 | 175 × 99 | 80 | 12,800 | 11.53 | 4 |
| 27 | 0.47 g | 14 1.21 | 175 × 99 | 120 | 12,800 | 11.53 | 4 |
| 21 | 0.70 g | 14 1.20 | 173 × 98 | 160 | 12,800 | 11.65 | 4 |
| 21 | 0.47 g | 14 1.20 | 173 × 98 | 240 | 12,800 | 11.65 | 4 |
| 4 | 0.70 g | 14 1.18 | 170 × 96 | 480 | 12,800 | 11.90 | 4 |
| 4 | 0.47 g | 14 1.18 | 170 × 96 | 480 | 12,800 | 11.90 | 4 |
| Vertical | , | Conv. | N | ||||
|---|---|---|---|---|---|---|---|
| Section | m s−2 | px|mm | mm | ms | ms | px/mm | |
| Upper | 0.70 g | 16 1.06 | 102 | 40 | 15,360 | 15.16 | 4 |
| Middle | 0.70 g | 16 1.06 | 102 | 160 | 15,360 | 15.16 | 4 |
| Bottom | 0.70 g | 16 1.06 | 102 | 320 | 15,360 | 15.16 | 4 |
| Upper | 0.47 g | 16 1.06 | 102 | 40 | 15,360 | 15.16 | 4 |
| Middle | 0.47 g | 16 1.06 | 102 | 200 | 15,360 | 15.16 | 4 |
| Bottom | 0.47 g | 16 1.06 | 102 | 400 | 15,360 | 15.16 | 4 |
Appendix B. PIV Algorithms
References
- Biferale, L.; Buzzicotti, M.; Linkmann, M. From two-dimensional to three-dimensional turbulence through two-dimensional three-component flows. Phys. Fluids 2017, 29, 111101. [Google Scholar] [CrossRef] [Green Version]
- Kokot, G.; Das, S.; Winkler, R.G.; Gompper, G.; Aranson, I.S.; Snezhko, A. Active turbulence in a gas of self-assembled spinners. Proc. Natl. Acad. Sci. USA 2017, 114, 12870–12875. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kelley, D.H.; Ouellette, N.T. Onset of three-dimensionality in electromagnetically driven thin-layer flows. Phys. Fluids 2011, 23, 045103. [Google Scholar] [CrossRef] [Green Version]
- Von Kameke, A.; Huhn, F.; Fernández-García, G.; Munuzuri, A.; Pérez-Muñuzuri, V. Double cascade turbulence and Richardson dispersion in a horizontal fluid flow induced by Faraday waves. Phys. Rev. Lett. 2011, 107, 074502. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Ramsden, S.; Shats, M. Three-dimensional fluid motion in Faraday waves: Creation of vorticity and generation of two-dimensional turbulence. Phys. Rev. X 2014, 4, 021021. [Google Scholar] [CrossRef] [Green Version]
- Xia, H.; Francois, N. Two-dimensional turbulence in three-dimensional flows. Phys. Fluids 2017, 29, 111107. [Google Scholar] [CrossRef] [Green Version]
- Faraday, M. XVII. On a peculiar class of acoustical figures; and on certain forms assumed by groups of particles upon vibrating elastic surfaces. Philos. Trans. R. Soc. Lond. 1831, 121, 299–340. [Google Scholar]
- Von Kameke, A.; Huhn, F.; Munuzuri, A.; Pérez-Muñuzuri, V. Measurement of large spiral and target waves in chemical reaction-diffusion-advection systems: Turbulent diffusion enhances pattern formation. Phys. Rev. Lett. 2013, 110, 088302. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Inverse energy cascade and emergence of large coherent vortices in turbulence driven by Faraday waves. Phys. Rev. Lett. 2013, 110, 194501. [Google Scholar]
- Colombi, R.; Schlüter, M.; von Kameke, A. Three dimensional flows beneath a thin layer of 2D turbulence induced by Faraday waves. Exp. Fluids 2021, 62, 865. [Google Scholar]
- Filatov, S.V.; Parfenyev, V.M.; Vergeles, S.S.; Brazhnikov, M.Y.; Levchenko, A.A.; Lebedev, V.V. Nonlinear generation of vorticity by surface waves. Phys. Rev. Lett. 2016, 116, 054501. [Google Scholar] [CrossRef] [PubMed]
- Levchenko, A.A.; Mezhov-Deglin, L.P.; Pel’menev, A.A. Faraday waves and vortices on the surface of superfluid He II. JETP Lett. 2017, 106, 252–257. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Fontana, P.W.; Shats, M. Wave-based liquid-interface metamaterials. Nat. Commun. 2017, 8, 6261. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Byrne, D.; Xia, H.; Shats, M. Robust inverse energy cascade and turbulence structure in three-dimensional layers of fluid. Phys. Fluids 2011, 23, 095109. [Google Scholar] [CrossRef] [Green Version]
- Ouellette, N.T.; O’Malley, P.J.J.; Gollub, J.P. Transport of finite-sized particles in chaotic flow. Phys. Rev. Lett. 2008, 101, 174504. [Google Scholar] [CrossRef] [Green Version]
- Singh, S.P.; Mittal, S. Energy spectra of flow past a circular cylinder. Int. J. Comput. Fluid Dyn. 2004, 18, 671–679. [Google Scholar] [CrossRef]
- Feldmann, D.; Umair, M.; Avila, M.; von Kameke, A. How does filtering change the perspective on the scale-energetics of the near-wall cycle? arXiv 2020, arXiv:2008.03535. [Google Scholar]
- Kelley, D.H.; Ouellette, N.T. Spatiotemporal persistence of spectral fluxes in two-dimensional weak turbulence. Phys. Fluids 2011, 23, 115101. [Google Scholar] [CrossRef] [Green Version]
- Alexakis, A.; Chibbaro, S. Local energy flux of turbulent flows. Phys. Rev. Fluids 2020, 5, 094604. [Google Scholar] [CrossRef]
- Natrajan, V.K.; Christensen, K.T. The role of coherent structures in subgrid-scale energy transfer within the log layer of wall turbulence. Phys. Fluids 2006, 18, 065104. [Google Scholar] [CrossRef] [Green Version]
- Vreman, B.; Geurts, B.; Kuerten, H. Realizability conditions for the turbulent stress tensor in large-eddy simulation. J. Fluid Mech. 1994, 278, 351–362. [Google Scholar] [CrossRef] [Green Version]
- Liao, Y.; Ouellette, N.T. Spatial structure of spectral transport in two-dimensional flow. J. Fluid Mech. 2013, 725, 281–298. [Google Scholar] [CrossRef]
- Schlichting, H. Berechnung ebener periodischer Grenzschichtstromungen. Z. Phys. 1932, 33, 327–335. [Google Scholar]
- Périnet, N.; Gutiérrez, P.; Urra, H.; Mujica, N.; Gordillo, L. Streaming patterns in Faraday waves. J. Fluid Mech. 2017, 819, 285–310. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.; Ecke, R.E.; Eyink, G.L.; Rivera, M.; Wan, M.; Xiao, Z. Physical mechanism of the two-dimensional inverse energy cascade. Phys. Rev. Lett. 2006, 96, 084502. [Google Scholar] [CrossRef] [PubMed]
- Kraichnan, R.H. Inertial ranges in two-dimensional turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef] [Green Version]
- Xia, H.; Francois, N.; Punzmann, H.; Shats, M. Tunable diffusion in wave-driven two-dimensional turbulence. J. Fluid Mech. 2019, 865, 811–830. [Google Scholar] [CrossRef] [Green Version]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Rectification of chaotic fluid motion in two-dimensional turbulence. Phys. Rev. Fluids 2018, 3, 124602. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Wave-particle interaction in the Faraday waves. Eur. Phys. J. E 2015, 38, 106. [Google Scholar] [CrossRef]
- Martín, E.; Vega, J.M. The effect of surface contamination on the drift instability of standing Faraday waves. J. Fluid Mech. 2006, 546, 203–225. [Google Scholar] [CrossRef] [Green Version]
- Strickland, S.L.; Shearer, M.; Daniels, K.E. Spatiotemporal measurement of surfactant distribution on gravity–capillary waves. J. Fluid Mech. 2015, 777, 523–543. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Colombi, R.; Rohde, N.; Schlüter, M.; von Kameke, A. Coexistence of Inverse and Direct Energy Cascades in Faraday Waves. Fluids 2022, 7, 148. https://doi.org/10.3390/fluids7050148
Colombi R, Rohde N, Schlüter M, von Kameke A. Coexistence of Inverse and Direct Energy Cascades in Faraday Waves. Fluids. 2022; 7(5):148. https://doi.org/10.3390/fluids7050148
Chicago/Turabian StyleColombi, Raffaele, Niclas Rohde, Michael Schlüter, and Alexandra von Kameke. 2022. "Coexistence of Inverse and Direct Energy Cascades in Faraday Waves" Fluids 7, no. 5: 148. https://doi.org/10.3390/fluids7050148
APA StyleColombi, R., Rohde, N., Schlüter, M., & von Kameke, A. (2022). Coexistence of Inverse and Direct Energy Cascades in Faraday Waves. Fluids, 7(5), 148. https://doi.org/10.3390/fluids7050148







