Analysis of Tip Clearance Effect on the Transportation Characteristics of a Multiphase Rotodynamic Pump Based on the Non-Uniform Bubble Model

Abstract

The multiphase rotodynamic pump is widely used in petroleum and gas exploitation, and blade tip clearance may cause flow instability and performance deterioration. In the present work, the influence of tip clearance on the transportation characteristic in a multiphase rotodynamic pump is investigated based on the non-uniform bubble model, in which the bubbles’ coalescence and break-up are considered. The influence mechanism of tip clearance on the energy performance is revealed. The results show that the leakage flow rate increases linearly with the increase in tip clearance, but variation in pump energy performance shows the opposite trend. In addition, a larger tip clearance results in a sharply decreased pressure increment in the impeller, while in the guide vane, the increment is raised slightly. For the 0 mm tip clearance condition, a positive vortex (relative to the impeller rotation direction) is observed in the impeller passage. However, the opposite leakage vortex is also found in the region near the tip clearance when the tip clearance is considered, and the vortex strength increases for a larger tip clearance.

1. Introduction

Energy is related to human development and social progress, and petroleum and gas resource exploitation has received increasing attention in the recent decades. However, due to the limitation of exploitation technology, petroleum and gas resource extraction accounts for only 15% of the global production [1]; therefore, it is urgent to develop this technology. In recent years, the multiphase pump technique has received much attention [2,3]. Compared to the traditional production platform of delivering petroleum and gas resources in a single-phase pump and compressor, only one pipeline is needed if the multiphase pump’s technology is used [4,5]. It is reported that the multiphase pump method can reduce the operation and maintenance costs of the transport systems by 40% and increase recovery by 10% [6].

A variety of investigations have been carried out to obtain the detailed flow mechanisms of the multiphase pump by numerical and experimental methods. For the experiment, the gas liquid flow pattern can be visualized by high-speed photography technology, and the visualization results show four types of flow pattern in the flow passage: isolated bubbles flow, bubbly flow, gas pocket flow, and segregated gas flow [7]. Furthermore, the experimental results demonstrate that the bubble size is influenced by the inlet gas volume fraction and rotation speed [8]. Moreover, an increase in bubble size will result in a decline in energy performance [9].

Based on the experiment measurement results, the numerical method is widely used to understand the detailed flow characteristics [10,11], which has a unique advantage in internal flow field investigations. It is reported that multiphase pump energy performance is directly related to the interphase forces between liquid and gas phases [12,13]. Drag force plays a dominating role among the interphase forces, which has an important influence on simulation accuracy [14,15]. Notably, the interphase forces are affected by the bubble size and distribution. Therefore, the bubble number equation is used to predict the variation in bubble diameter caused by the bubbles’ break-up and coalescence [16]. Furthermore, the gas cavity equation is developed by considering the bubbles’ coalescence near the impeller suction side [17]. In addition, a modified drag coefficient model is proposed under turbulent flow conditions, which takes the effect of turbulent flow on the bubble path into consideration [18].

According to the discussion above, the bubble size and distribution have a close relationship with the flow pattern. For a single-phase vane pump, one of the main reasons for flow instability is tip leakage flow [19,20]. The tip leakage is caused by the pressure difference between the pressure side (PS) and suction side (SS) [21,22,23]; it can also induce pressure fluctuation [24], unbalanced radical force [25], intense noise [26], and the cavitation phenomenon [27]. Results show that the pressure rise of an axial flow pump decreases by 41.39% when the tip clearance size increases from 0 to 0.8 mm under the single-phase condition [28]. Moreover, with increasing tip clearance size, the pump head and efficiency of the mixed flow pump decrease accordingly with a nearly linear rule [29].

In recent years, considerable works [30,31] have been conducted to investigate the mechanism of tip leakage flow in hydraulic machinery under the single-phase condition. Moreover, based on previous works, some effective methods have been approved to suppress tip leakage flow, such as the T shape tip [32] and C groove [33] in simple hydrofoils. In addition, the T shape tip [34] is successfully used in a mixed flow pump. According to the report, this method can improve efficiency by 1.86%, and the leakage flow rate decreases by up to 15.95%. To our knowledge, a control method that can be effectively used in a multiphase pump has not been reported, and one of the prime reasons is that the mechanism of tip leakage flow and its effect on the main flow are not fully understood.

In this work, the influence of tip clearance on the energy performance and the transportation characteristics in a multiphase rotodynamic pump are systematically investigated as follows. Firstly, flow simulations of the pump are conducted using the non-uniform bubble model and modified drag force model. Then, the distributions of vorticity, gas volume fraction, turbulence kinetic energy, and pressure increment are presented under different tip clearance sizes. Finally, the energy performances are given to reveal the relationship between energy performance and flow field. The present work provides a foundation for the suppression of tip leakage flow.

2. Physical Model and Numerical Methods

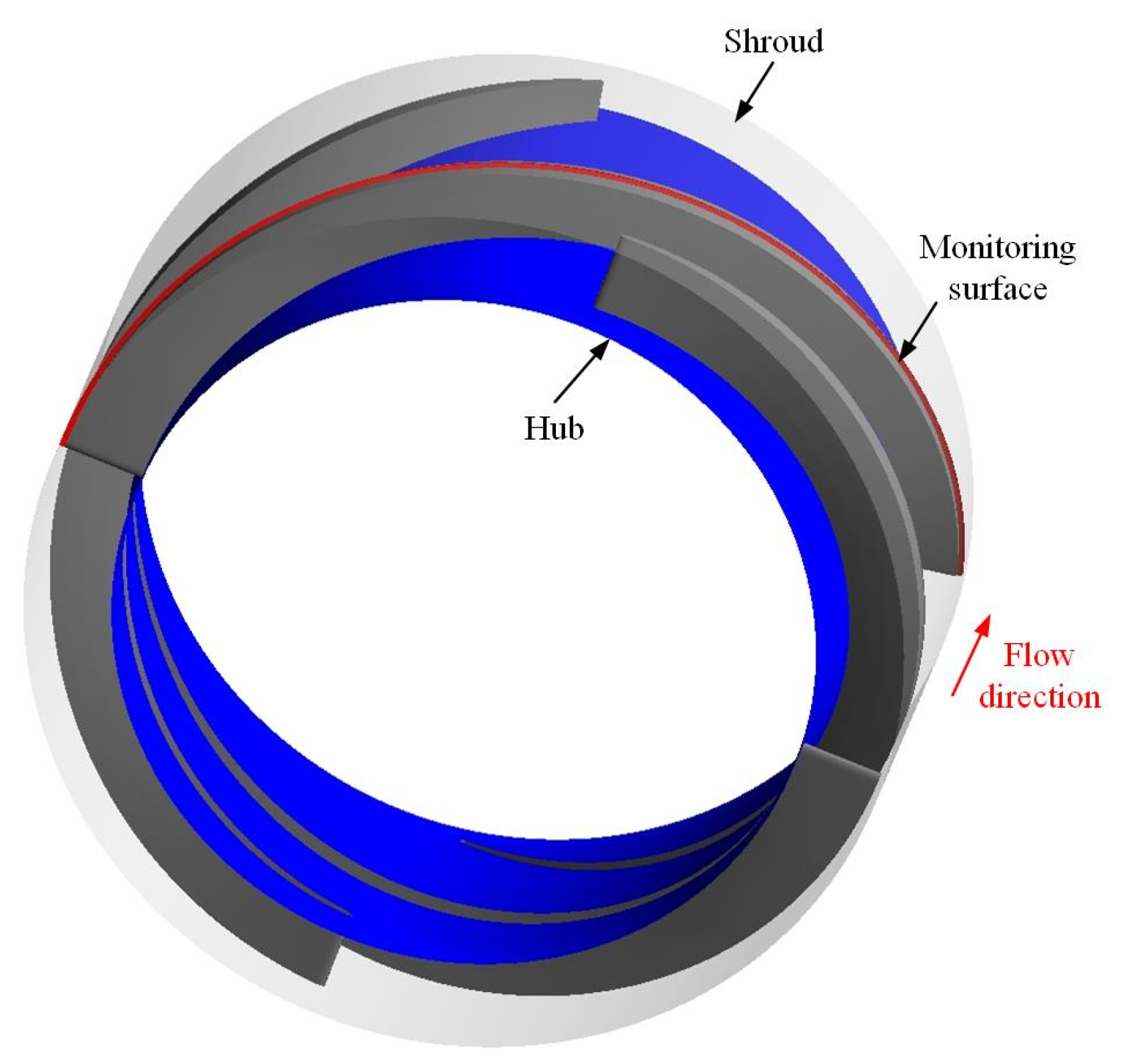
2.1. Geometrical Model of the Pump

A single-stage multiphase pump with 4 impeller blades and 11 guide vane blades was employed in this work. The primary parameters of the pump are given in

Table 1. Parameters of the multiphase pump.

Multiphase Pump	Items	Values	Units
Impeller	Number of impeller blade	4	-
	Shroud diameter of impeller inlet	150	mm
	Shroud diameter of impeller outlet	150	mm
	Hub diameter of impeller inlet	120	mm
	Hub diameter of impeller outlet	134	mm
Guide vane	Number of guide vane blade	11	-
	Shroud diameter of guide vane outlet	150	mm
	Hub diameter of guide vane outlet	120	mm
Design operating point	Rotating speed n	2950	r/min
	Specific speed ns	166	-
	Design head H	15	m
	Design flow rate Qd	50	m3/h
	Power P	7.35	KW

, and the tested pump is shown in Figure 1. The tip clearance size δ of the tested pump is 0.4 mm.

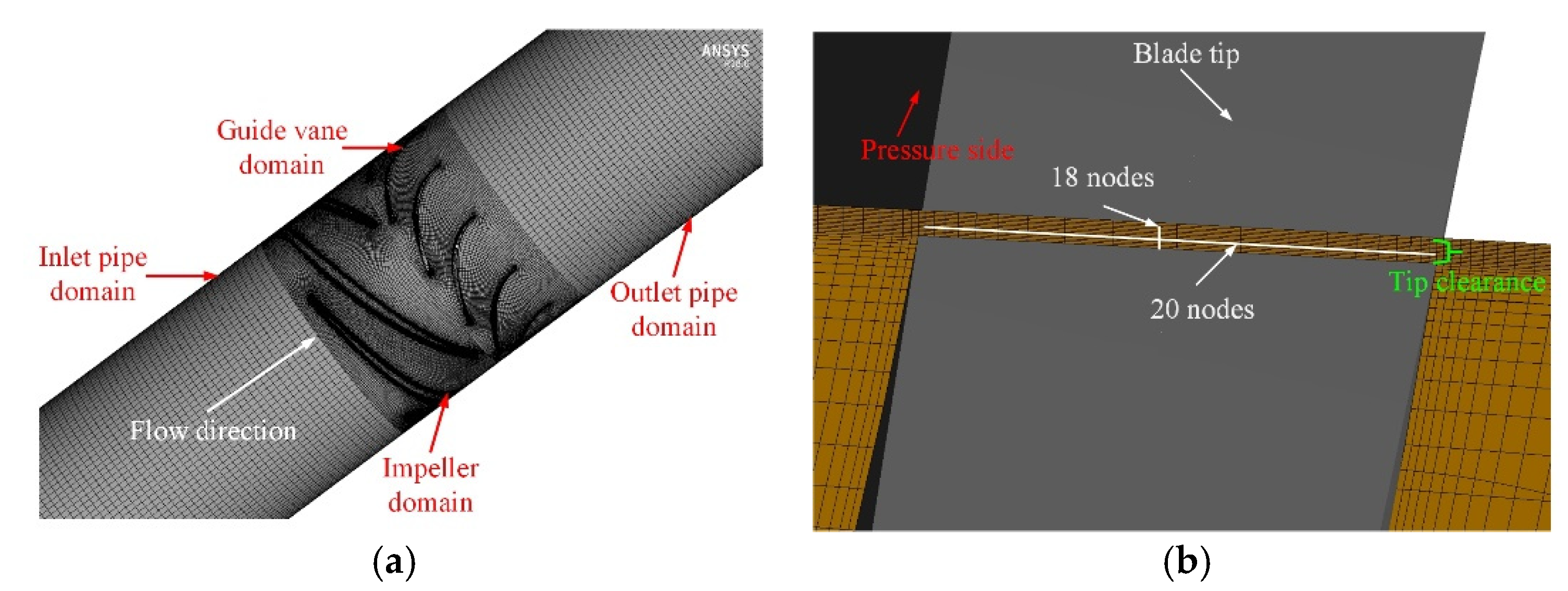
2.2. Mesh Arrangement

In this work, a hexahedral mesh is used to discretize the computational domain (as shown in Figure 2a). To obtain the detailed flow characteristics around the blade surface and tip clearance region, mesh refinement is adopted. As shown in Figure 2b, there are 20 nodes from the pressure side (PS) to the suction side (SS), and 18 nodes are arranged from the blade tip to the shroud. According to the independence test of mesh number, as shown in

Table 2. Independence test of the mesh number.

Item	Mesh1	Mesh2	Mesh3	Mesh4
Pipe	54,516	54,516	54,516	54,516
Impeller	660,384	1,226,640	2,364,667	3,237,444
Guide vane	386,848	619,080	1,164,240	1,424,192
Total	1,101,748	1,900,236	3,583,423	4,716,152
Δp/Δp1	1.0000	0.9831	0.9783	0.9775

, when the number of whole passages increases to 3,583,423, there is limited influence on the pressure increment (Δp). Therefore, Mesh 3 with 3,583,423 elements is used in the present work, and detailed information is given in our previous work [17].

2.3. Non-Uniform Bubble Model (NUBM)

Based on the visualization results, the bubble diameter, D_b, changes during the impeller rotation in the multiphase pump [8]. Therefore, a non-uniform bubble model is developed to simulate the D_b variation. In this model, the variation is presented by the bubble number density, ξ, which is defined as follows:

$$\xi =\frac{{\alpha }_{\mathrm{g}}}{\pi /6D_{\mathrm{b}}{}^3},$$

(1)

where, α_g is the gas phase volume fraction.

As ξ is an unknown variable, the conservation equation of ξ is employed, which is expressed as follows:

$$\frac{\partial \xi }{\partial t}+\nabla \cdot (\xi U_{\mathrm{g}})={\psi }_{\mathrm{br}}-{\psi }_{\mathrm{co}}+{\psi }_{\mathrm{ph}},$$

(2)

where t denotes time. U_g is the gas phase relative velocity vector; and ψ_br, ψ_co and ψ_ph are the source terms relevant to break-up, coalescence, and phase change, respectively (the last term being neglected here). The expressions of ψ_br and ψ_co are shown as follows:

$${\psi }_{\mathrm{br}}=C_{\mathrm{br}}(1-{\alpha }_{\mathrm{g}}){\left(\frac{\epsilon }{D_{\mathrm{b}}}\right)}^{1/3}\xi \exp \left(-\frac{W{e}_{\mathrm{c}}}{We}\right),$$

(3)

$$We=\frac{2\rho {\epsilon }^{2/3}{D}_{\mathrm{b}}{}^{5/3}}{\sigma },$$

(4)

where C_br is the break-up coefficient, which is set as 1; ε is turbulent dissipation; ρ_l is the water density. σ is the surface tension coefficient; and We and We_c are the Weber number and the critical Weber number, respectively. The value of We_c has been studied extensively, and it is taken as 1.5 [35].

$${\psi }_{\mathrm{co}}=C_{\mathrm{co}}(1-{\alpha }_{\mathrm{g}}){\eta }_{\mathrm{co}}{\epsilon }^{1/3}{D}_{\mathrm{b}}{}^{7/3}{\xi }^2,$$

(5)

where C_co is the coalescence coefficient, which is taken as 3.0, and η_co is the efficiency of coalescence behavior, defined as follows:

$${\eta }_{\mathrm{co}}=\exp \left(-\sqrt{\frac{W_{\mathrm{e}}}{4}}-K_{\mathrm{co}}\frac{D_{\mathrm{b}}{}^3}{\epsilon }\right).$$

(6)

2.4. Drag Force Model

According to previous investigations [12,13,14], the interphase force mainly includes drag force, F_D,k; lift force, F_L,k; added mass force, F_A,k; and turbulent dispersion force, F_T,k, in the multiphase pump. However, F_D,k plays a leading role in those forces [14], and it is expressed as follows:

$$F_{D,\mathrm{l}}=-F_{D,}{}_{\mathrm{g}}=\frac{3}{4}{C}_D\frac{{\rho }_{\mathrm{l}}}{D_{\mathrm{b}}}{\alpha }_{\mathrm{g}}\left|U_{\mathrm{g}}-U_{\mathrm{l}}\right|\left(U_{\mathrm{g}}-U_{\mathrm{l}}\right),$$

(7)

where U_l and U_g are the velocity vectors of the liquid phase and gas phase, respectively, and C_D is the drag coefficient. The Schiller Naumann model (Equation (8)) has been widely used to calculate C_D.

$$C_{D\_\mathrm{SN}}=\max \left(\frac{24\left(1+0.15{\mathrm{Re}}^{0.687}\right)}{\mathrm{Re}},0.44\right).$$

(8)

The Schiller Naumann model is built based on the investigation of a single spherical particle. Therefore, C_D is modified by considering the interaction of bubbles [36] in the present work, and the modified C_D (C_{D_mod}) is expressed as follows:

$$C_{D\_\mathrm{mod}}=\max \left(\frac{24\left(1+0.1{\mathrm{Re}}_{\mathrm{b}}{}^{0.75}\right)}{{\mathrm{Re}}_{\mathrm{b}}},\frac{2}{3}{D}_{\mathrm{b}}\sqrt{\frac{\left({\rho }_{\mathrm{l}}-{\rho }_{\mathrm{g}}\right)}{\sigma }}{\left(1-{\alpha }_{\mathrm{g}}\right)}^{-0.5}\right),$$

(9)

where Re_b is the modified Reynolds number, which is shown as

$${\mathrm{Re}}_{\mathrm{b}}=\frac{{\rho }_{\mathrm{l}}{D}_{\mathrm{b}}\left|U_{\mathrm{l}}-U_{\mathrm{g}}\right|}{{\mu }_{\mathrm{l}}}\left(1-{\alpha }_{\mathrm{g}}\right),$$

(10)

where μL is the liquid phase dynamic viscosity. Re_b is usually calculated based on the specific D_b. In the present work, Re_b is modified using the non-uniform D_b, as described in Section 2.3.

2.5. Calculation Settings

According to previous studies, the Shear Stress Transport (SST) k-ω turbulence model is used for the turbulent flow of the liquid phase, and the governing equations of the gas phase are solved by the Dispersed Phase Zero Equation [3,13,17]. The total mass flow rate and volume fraction for gas and liquid phases are set at the computational domain inlet, while at the outlet, the average pressure is defined. The no-slip wall condition is applied on the wall. As the tip clearance is considered in this simulation, a counter-rotating wall condition is applied on the impeller shroud. The non-uniform bubble model and the modified Schiller Naumann model are encoded in CFX using the CFX expression language [37].

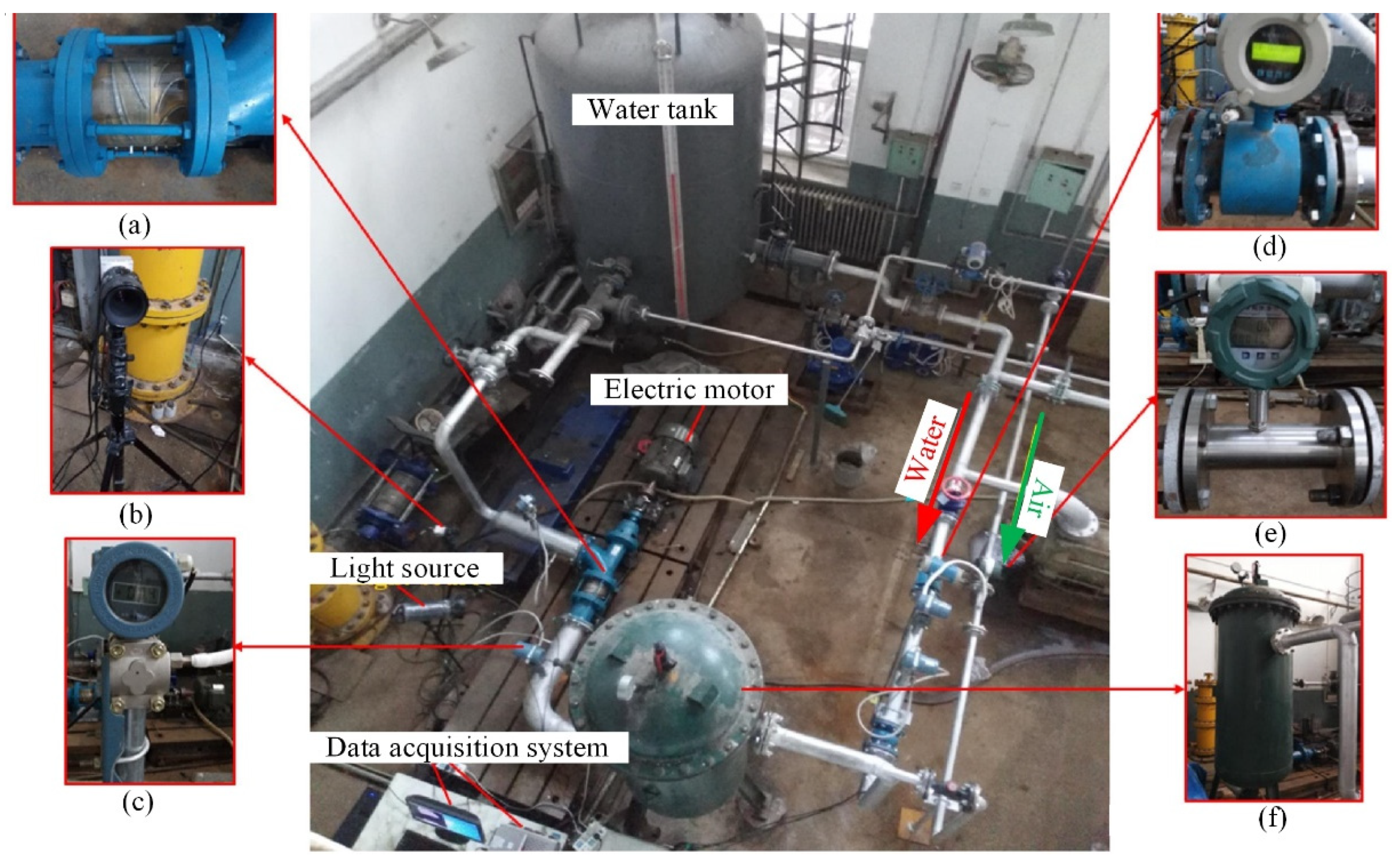
3. Experimental Setup

Experimental measurement of the energy performance when handling the mixture of water and air is carried out in a test bench for hydraulic machinery, as shown in Figure 3. The gas and liquid phases are provided by the air compressor and the water tank, respectively. The two fluids are mixed in the buffer tank and then transported to the water tank by the tested pump. The water tank is opened, so the gas phase eventually overflows from the water tank due to the density difference.

The water and air flow rates are measured by an LDBE-80 electromagnetic flowmeter (Jinhu Simeite Instrument Co., LTD, Huaian, China) with an accuracy of 0.5% and an LWQ-50 turbine flowmeter (Jiangsu Fenghui Instrument Co., LTD, Huaian, China) with an accuracy of 1.5%, respectively. A 1151 pressure transducer (Beijing Chengchuang Tiansheng Automation Technology Co., Ltd., Beijing, China) is used to measure the tested pump inlet and outlet pressure, and the accuracy is 0.1%. Considering the possibility of negative pressure at the pump entrance, the range of the inlet pressure transducer is −0.1–1.6 MPa. In addition, a CYT-302 torque speed sensor (Beijing Haibohua Technology Co., LTD, Beijing, China)is employed to test the pump shaft rotational speed and torque, and the accuracy is 0.2%. Thus, the uncertainties of the head and efficiency are 0.79% and 0.81%, respectively.

4. Results and Discussion

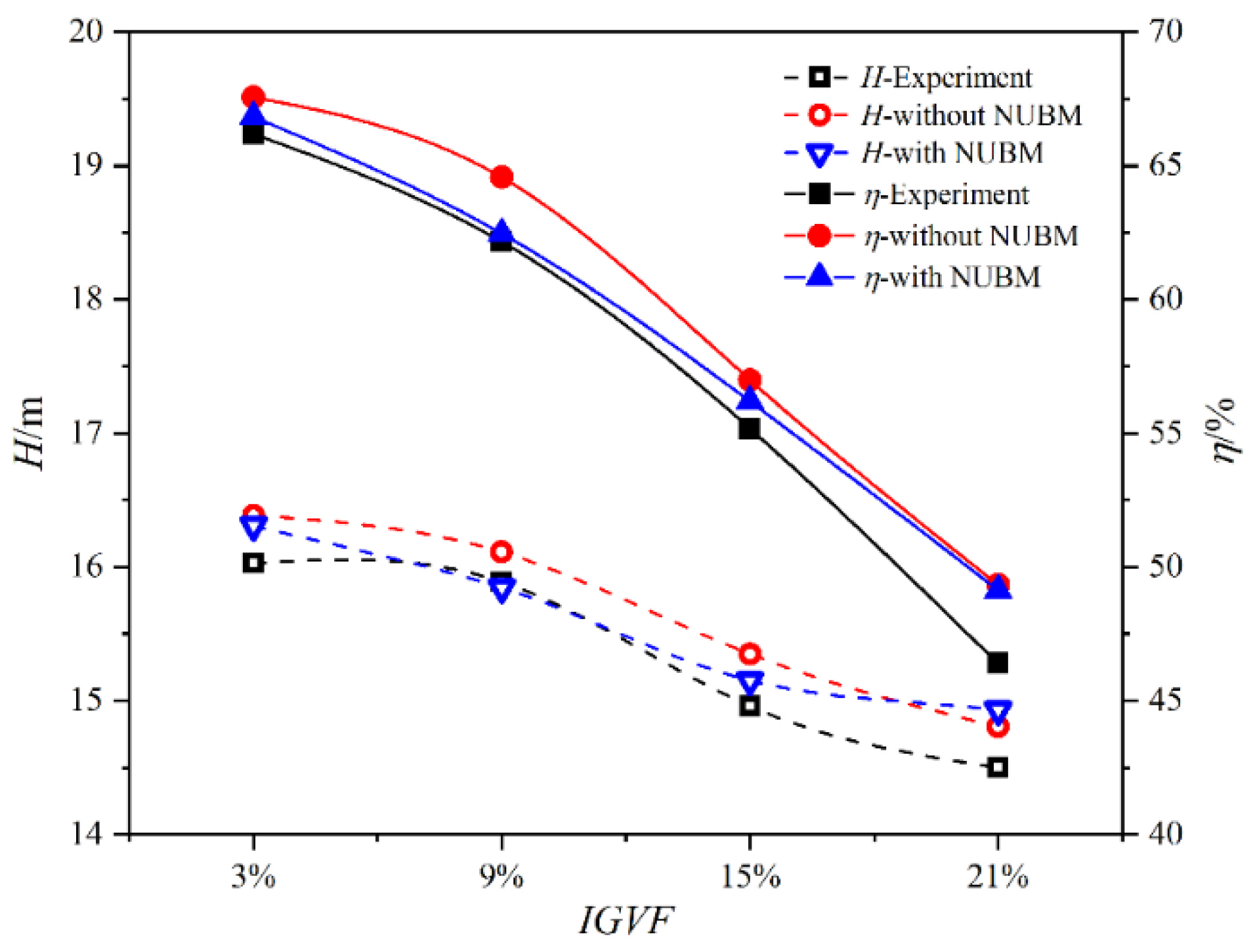
4.1. Validation of the Simulation

As shown in Figure 4, an experimental measurement with an inlet gas volume fraction (IGVF) ranging from 3% to 21% is chosen to verify the simulation result with and without the NUBM. In comparison with the NUBM case, the result with NUBM is closer to the experimental result, especially under low IGVF conditions (IGVF ≤ 15%). Therefore, the NUBM method is qualified for the following calculations. Notably, the IGVF = 9% is chosen for the following investigations.

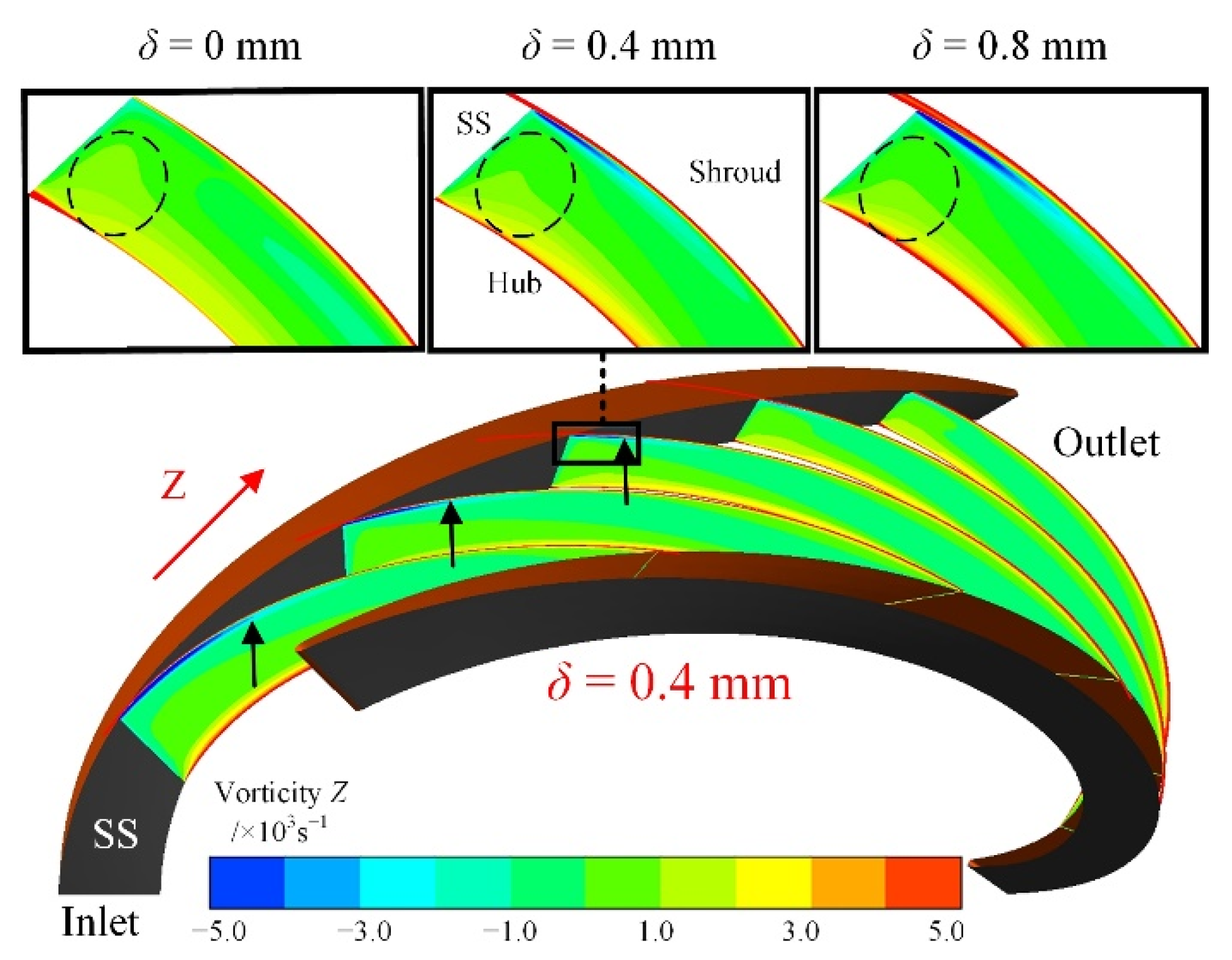
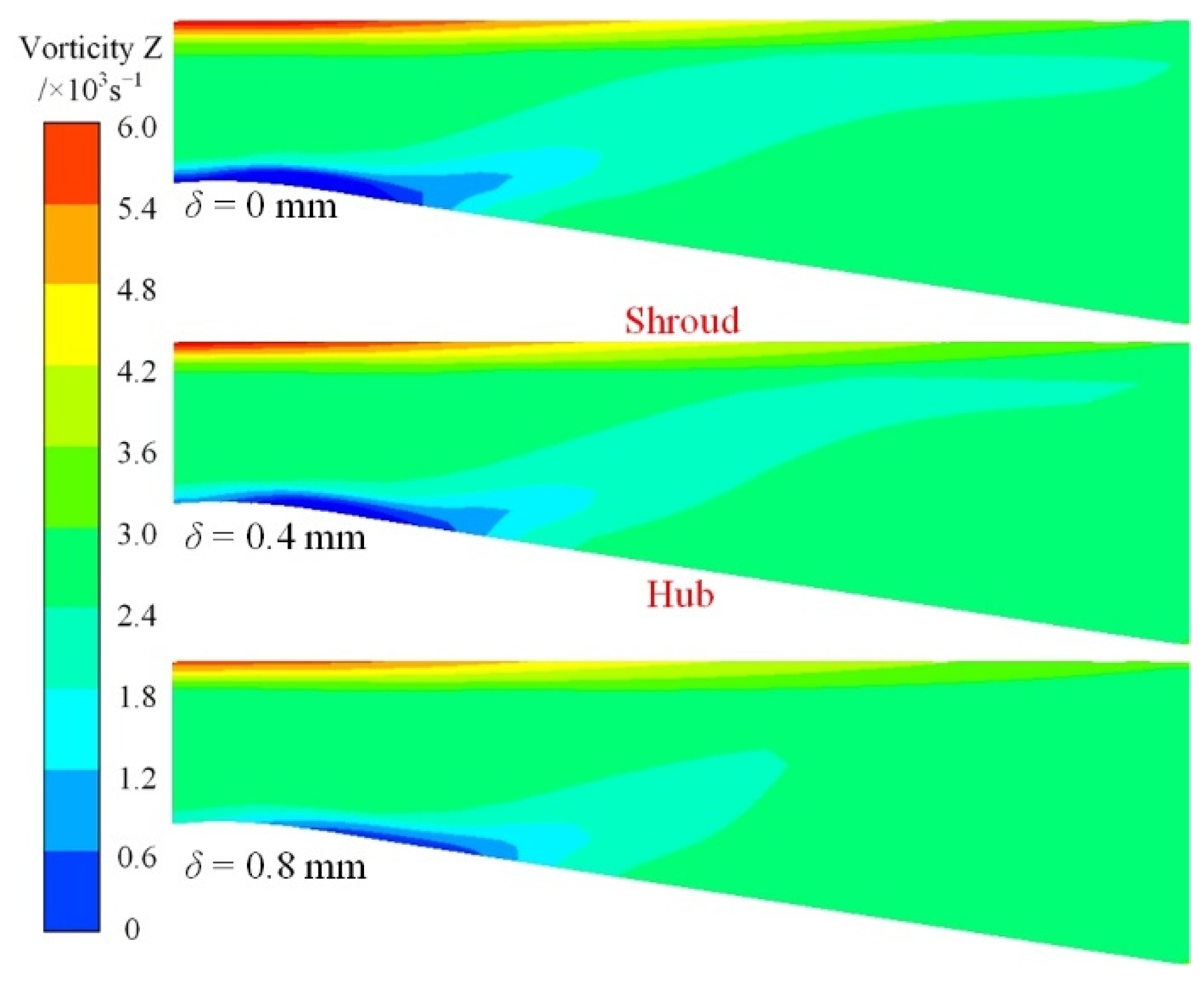
4.2. Leakage Flow Rate and Leakage Vortex

To reveal the influence of tip clearance on multiphase pump transportation by the numerical method, five tip clearance sizes ranging from 0.2 mm to 1.0 mm are employed in the investigation, which is approximately 1.74–8.70% of the mean blade height.

The mass flow rate of leakage flow, Q_L, is monitored to quantitatively analyze the effect of δ on the energy performance of the pump. Figure 5 shows the schematic diagram of the monitoring surface (marked by the red surface) in the region of tip clearance along the blade chord.

Figure 6 shows the variations in H, η, and Q_L/Q with the increase in δ under the design flow rate. It reveals that the Q_L sharply increases as the δ increases, and the variation is linear in the range 0 mm to 1 mm. The variations in H and η show the opposite trend due to the higher disturbance of the larger leakage flow rate. The fitting linear relationships for Q_L, H, and η with δ are expressed as Q_L = 2.00547 × δ + 0.03558, H = −8.12295 × δ + 18.42373, and η = −12.61436 × δ + 63.91689, respectively.

Leakage flow will lead to a tip leakage vortex, and the characteristics of the vortex can influence the distribution of the gas phase and further induce variation of the impeller pressurization ability. The Z component of vorticity (vorticity Z) on the circumferential sections is shown in Figure 7. In addition, the magnification (marked by the black rectangle) for different tip clearance sizes is also presented at the top of Figure 7. Comparing the vorticity distributions for different tip clearance sizes, a positive vortex (relative to the impeller rotation direction) can be observed in the impeller passage under the condition of δ = 0 mm. However, for a δ of 0.4 mm or 0.8 mm, the opposite leakage vortex is also found in the region near the tip clearance of the SS, and it will strengthen with the increase in δ. Moreover, an increase in δ leads to a reduction in the passage vortex (marked by the black dashed circle) strength, which is due to the interference of leakage vortex. The leakage vortex strength can also be seen to gradually weaken along the streamwise direction, as presented by the black arrow, which is related to the decreased pressure difference between the PS and SS.

Figure 8 displays the vorticity Z on the meridional plane in the guide vane. Due to the extended structure of the guide vane, the separation vortex occurs near the hub and develops to the shroud along the streamwise direction. In Figure 6 and Figure 7, the tip leakage flow rate and leakage vortex intensity increase sharply at larger δ, together with higher flow loss. Therefore, the fluid kinetic energy and inertia force decrease in the guide vane inlet, and the vortex intensity weakens as the δ increases accordingly. In addition, the effect of wall friction leads to the vortex structure near the shroud, while the vortex rotation direction is different from the separation vortex near the shroud. Similarly, an increase in δ causes a weakened vortex strength, which is also related to the increased flow loss in the impeller.

Figure 9 shows the variation in average turbulence kinetic energy (TKE) from the impeller inlet to the guide vane outlet for different tip clearance sizes. When the tip clearance is neglected, due to the effect of impeller work, the TKE gradually rises along the streamline in the impeller. For the condition of δ = 0.4 mm, the TKE increases sharply relative to δ = 0 mm, which is related to the disturbance of tip leakage flow. In addition, the variation in TKE shows the opposite trend, which can be explained by the decreased strength of the tip leakage vortex along the flow direction, as shown in Figure 7. As δ increases to 0.8 mm, the magnitude and descent rate of TKE further increase. When the fluid media flow into the vanless region, the flow is mainly influenced by the effect of rotor-stator interaction for δ = 0 mm, and it shows an increasing trend. The tip leakage flow is another factor in the flow stability for this region when the tip clearance is considered. In addition, Figure 9 shows that the variation in TKE in the vanless region will continue to decrease in this region, which indicates that the effect of tip leakage flow plays a leading role in the flow stability in this region for δ = 0.4 or 0.8 mm. In the guide vane, as a whole, the TKE gradually increases along the flow direction regardless of whether it is with or without tip clearance. However, the magnitude of TKE decreases with increasing δ, which can be explained by the weakened flow separation, as shown in Figure 8.

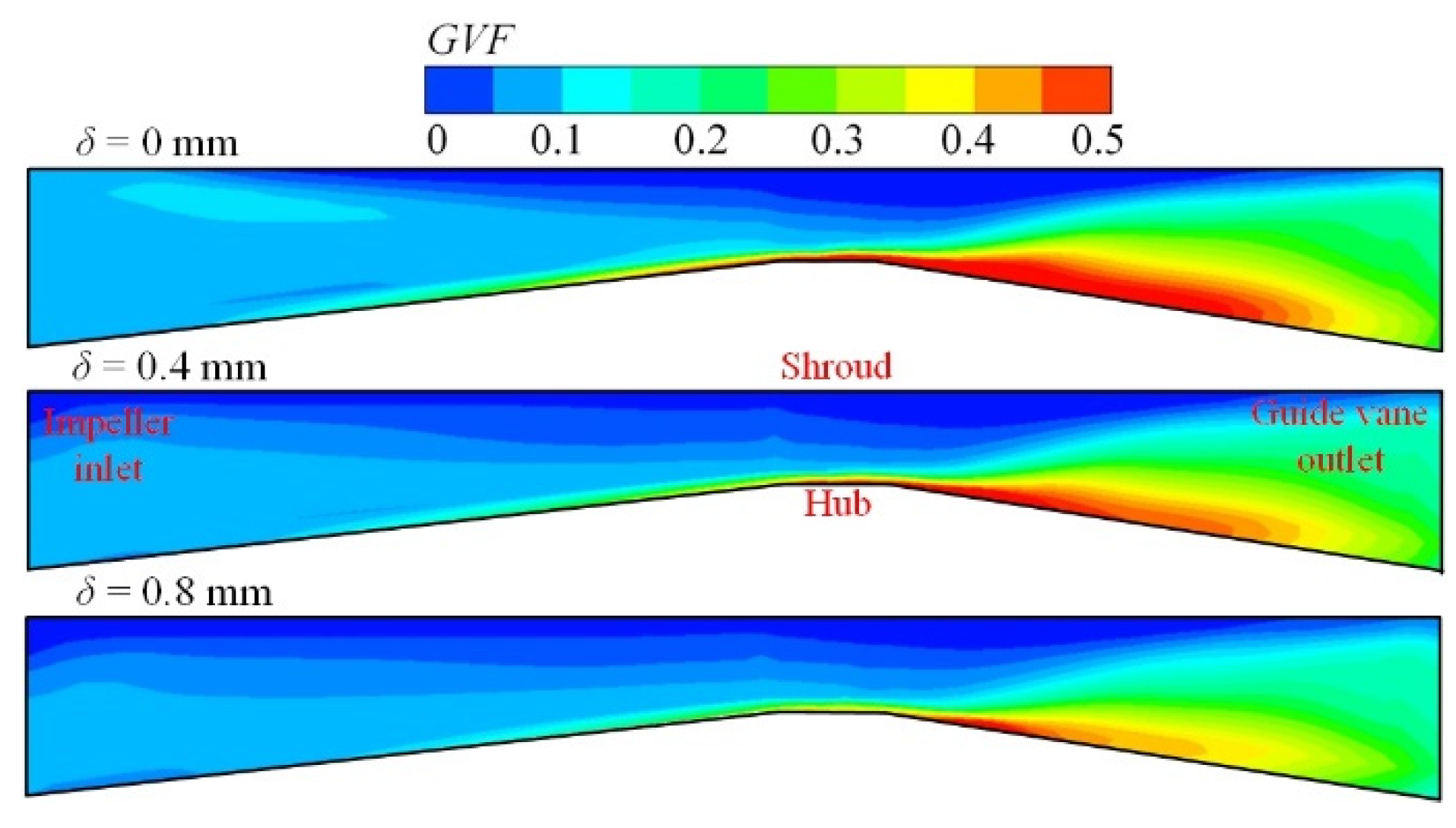
4.3. Gas Phase Distribution

Figure 10 displays the distribution of the gas volume fraction (GVF) on the meridional plane in the whole passage under various tip clearance sizes condition. In the impeller region, the gas phase mainly occurs near the hub caused by the density difference of gas and liquid phases. At larger δ, the blocking effect of the tip leakage vortex strengthens (Figure 7), and the liquid velocity near the hub increases accordingly. Therefore, the gas accumulation will be weakened with increasing δ. In the guide vane region, the effect of centrifugal force on the fluid media weakens along the streamwise direction, so the gas phase develops to the shroud. In addition, by comparing the distribution of the GVF for different tip clearance sizes, it can be found that the gas phase accumulation decreases with increasing δ, which is related to the weaker separation vortex, as shown in Figure 8.

The distribution of the GVF on the blade surface is directly related to the impeller’s ability, and the distributions of the GVF on the PS and SS for different tip clearance sizes are shown in Figure 11. Compared with the distribution on the PS, more severe gas accumulation occurs on the SS, which is related to the pressure difference between the two sides and the phase density difference. At δ = 0 mm, severe accumulation can be observed near the blade leading edge of the SS. As δ increases, the high magnitude region of GVF gradually disappears, while at the leading edge of the PS, the distribution shows the opposite trend. In the rear part of the PS, the gas phase distribution tends to be the same under different conditions. However, the gas accumulation near the hub weakens with the increase in δ in the SS rear part. This phenomenon can be explained by the strengthened blocking effect of the leakage vortex for larger δ, as shown in Figure 7, which leads to the increased liquid kinetic energy near the hub, together with the strengthened ability of the liquid to carry the gas phase.

Figure 12 shows the variation in the average D_b from the impeller inlet to the guide vane outlet for different tip clearance sizes. The variation shows the same trend for different conditions. In the impeller region, overall, the D_b is nearly unchanged, while it shows a trend of sharp increase followed by a gradual decrease in the guide vane region. Figure 10 reveals that as more severe gas phase accumulation occurs in the guide vane, the rate of collision between the bubbles will increase, so the D_b in the impeller region is significantly larger than that of impeller. In addition, the D_b tends to decrease with increasing δ, which is also related to the decreased gas accumulation (Figure 10).

Figure 13 shows the variation in pressure on the impeller blade PS and SS of the 0.5 span along the blade chord for different tip clearance sizes. According to Figure 10, with increasing δ, more severe phase accumulation occurs near the leading edge of the PS, so the pressure increment in this region decreases accordingly. However, the higher ability of the PS results in more severe flow separation in the SS leading edge together with lower pressure in this region as the δ decreases. In the rear part of the blade, the pressure tends to be uniform for different conditions in the PS, while in the SS, it decreases with increasing δ. This can also be explained by the difference in GVF distribution among those conditions, as shown in Figure 11.

4.4. Energy Performance

Figure 14 shows the influence of δ on the pump energy performance. The energy performance shows a similar curve trend under the different conditions. An increase in δ results in a dramatic pump head and efficiency decrease, despite its relatively small size. Moreover, the influence of δ becomes more evident with the increasing flow rate. Under the 1.2 times design flow rate condition, the efficiency and head decrease by as much as 32.45% and 40.36%, respectively. In addition, the pump efficiency drops sharply when the flow rate is higher than the best efficiency point (BEF) for larger δ conditions, which indicates that a larger δ leads to the deterioration of the pump operation stability.

Figure 15 shows the pressure increments (Δp) in the impeller and the guide vane. As shown in Figure 10, a larger δ causes severe gas accumulation on the PS, which greatly restricts the impeller pressurization ability (Figure 13). Moreover, the strength of the leakage vortex and the leakage flow rate increase dramatically with increasing δ, which will lead to greater volume loss (Figure 6) and flow loss (Figure 12). Therefore, the most obvious difference in the pressure increment occurs in the impeller, and the reduction in the pressure increment reaches 34.69% as the δ increases from 0 mm to 0.8 mm. However, the pressure increment increases slightly (2.26%) in the guide vane with increasing δ, which is caused by the weaker flow separation for larger δ, as shown in Figure 8.

5. Conclusions

Based on the non-uniform bubble model, the effect of tip clearance size (δ) on the vortex structure, the distribution of the gas phase, and the energy performance in a multiphase pump are systematically investigated, and the main conclusions are as follows:

(1)

A larger δ causes a rise in the leakage flow rate and further strengthens the leakage vortex. However, due to the higher flow loss in the impeller, the separation vortex is reduced in the guide vane passage with increasing δ;

(2)

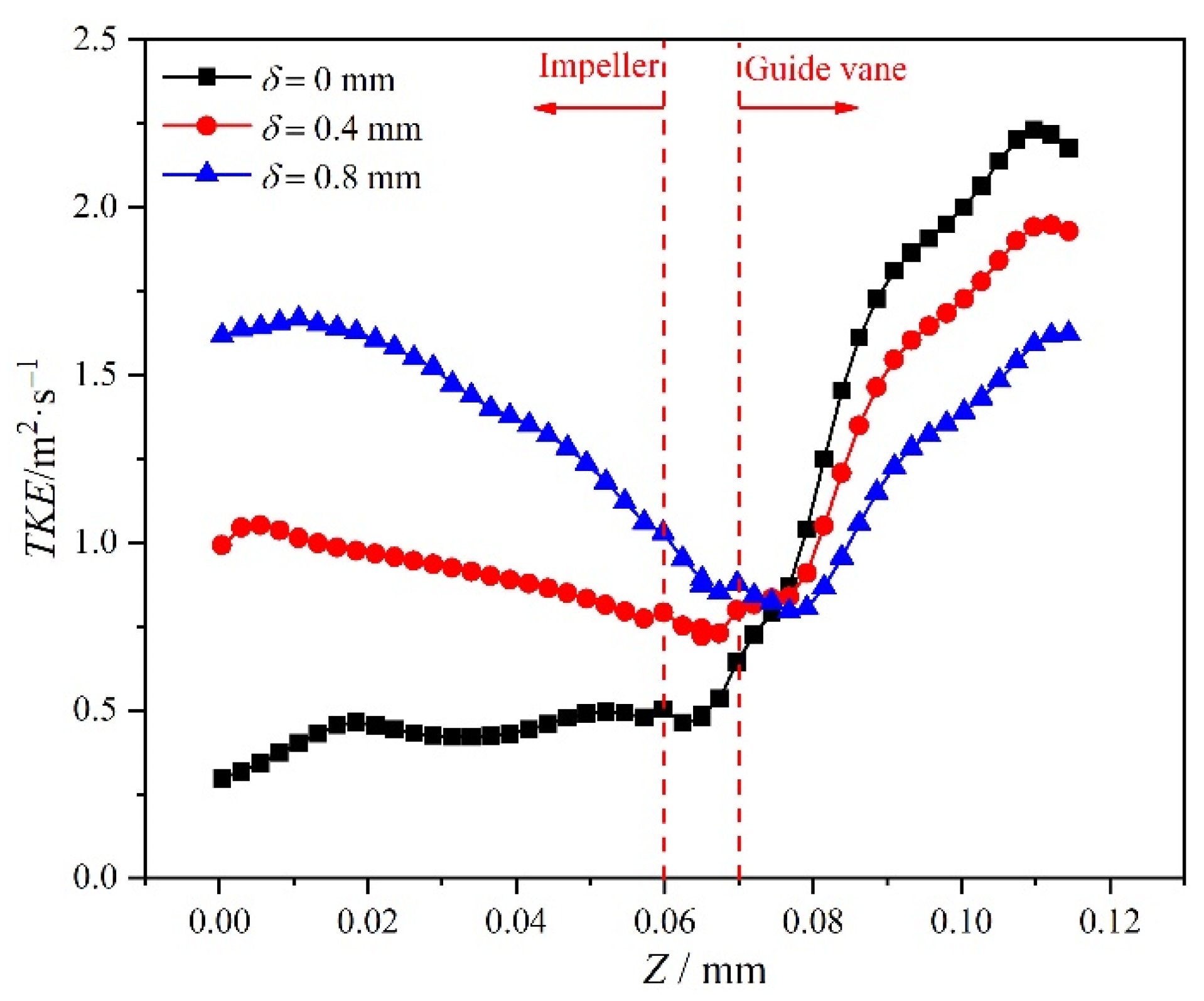
As δ increases, the disturbance of the tip leakage vortex makes the average TKE increase obviously in the impeller region. The variation in TKE shows the opposite trends with or without tip clearance, and the vortex strength weakens along the streamwise direction, which leads to a decreasing trend of TKE when the tip clearance is considered;

(3)

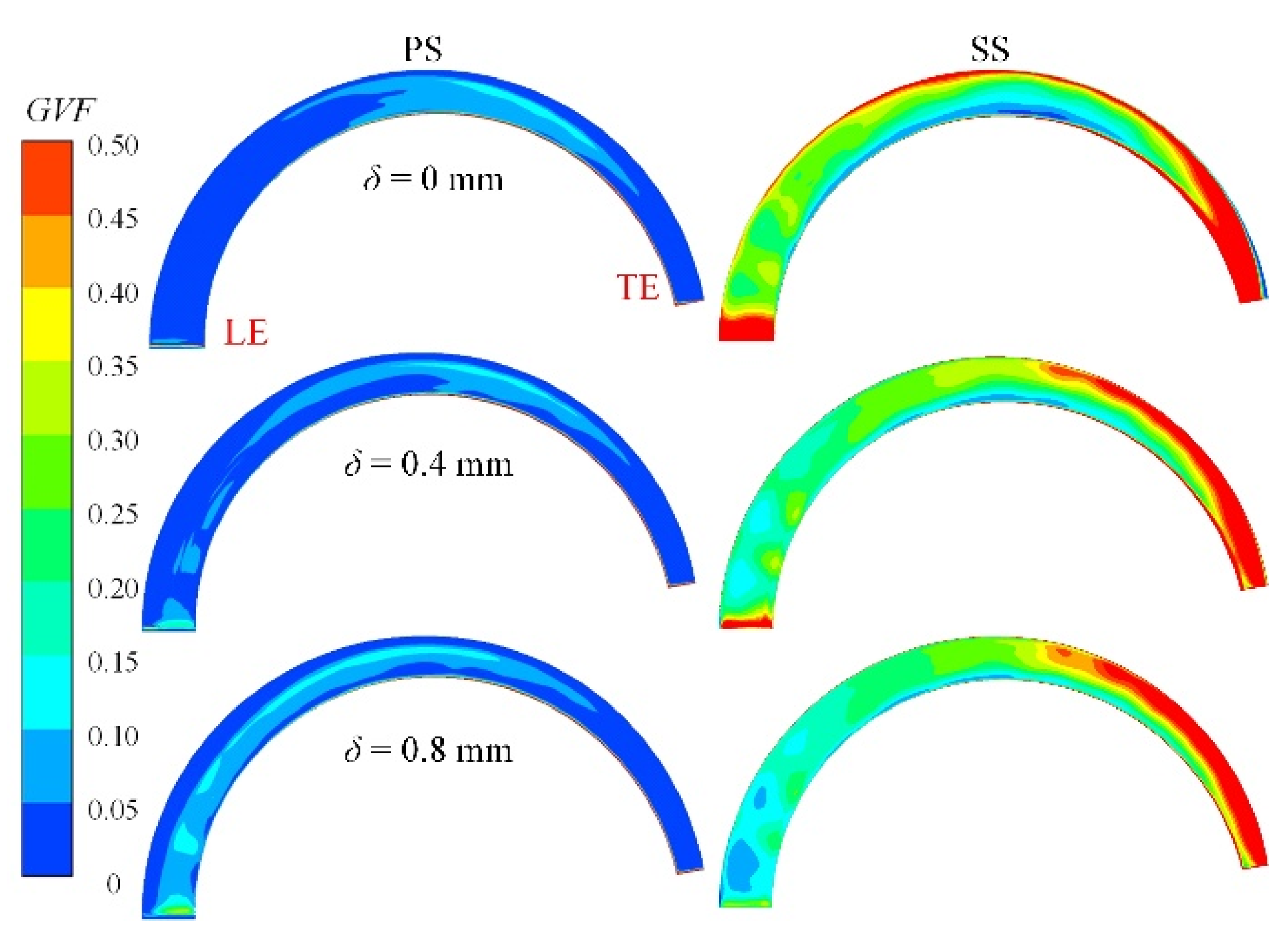
In the impeller inlet, a larger δ leads to more severe accumulation on the PS and decreased gas content on the SS. The blocking effect of the tip leakage vortex makes the liquid kinetic energy increase near the impeller hub; thus, gas accumulation weakens in this region;

(4)

Due to the combined effect of more severe gas accumulation and higher flow loss caused by the leakage flow, the pressure increment drops sharply in the impeller region as the δ increases. In the guide vane, however, the pressure increment is raised slightly with a larger δ because of the weaker flow separation in this condition.

In future work, we will assess the spatial-temporal evolution of tip leakage. Furthermore, based on the in-depth investigation of leakage flow, a suppressing the tip leakage flow will be proposed.