Physics of Dynamic Contact Line: Hydrodynamics Theory versus Molecular Kinetic Theory
Abstract
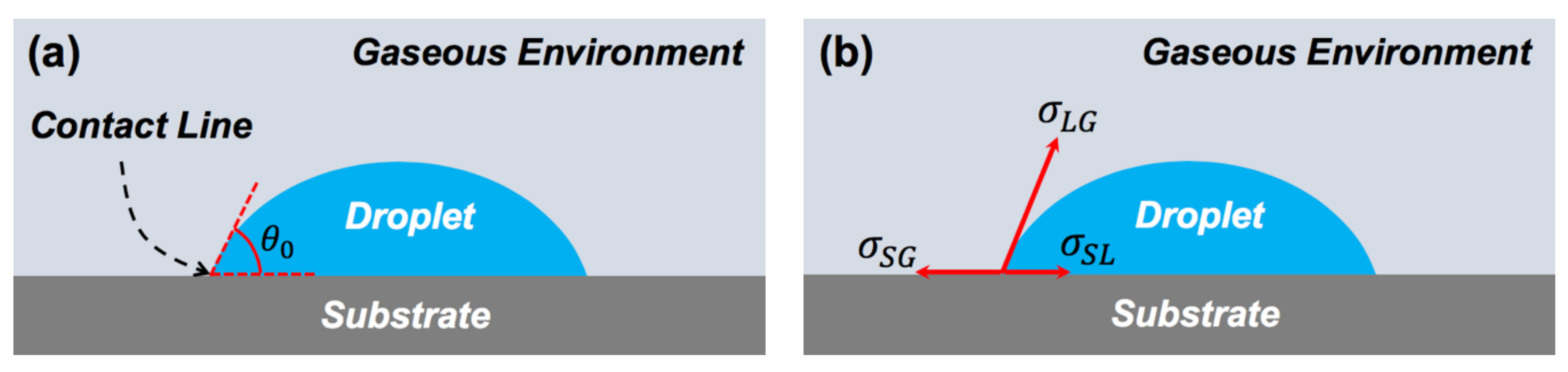
1. Introduction
2. Applications of the Dynamic Contact Line
2.1. Coating and Printing
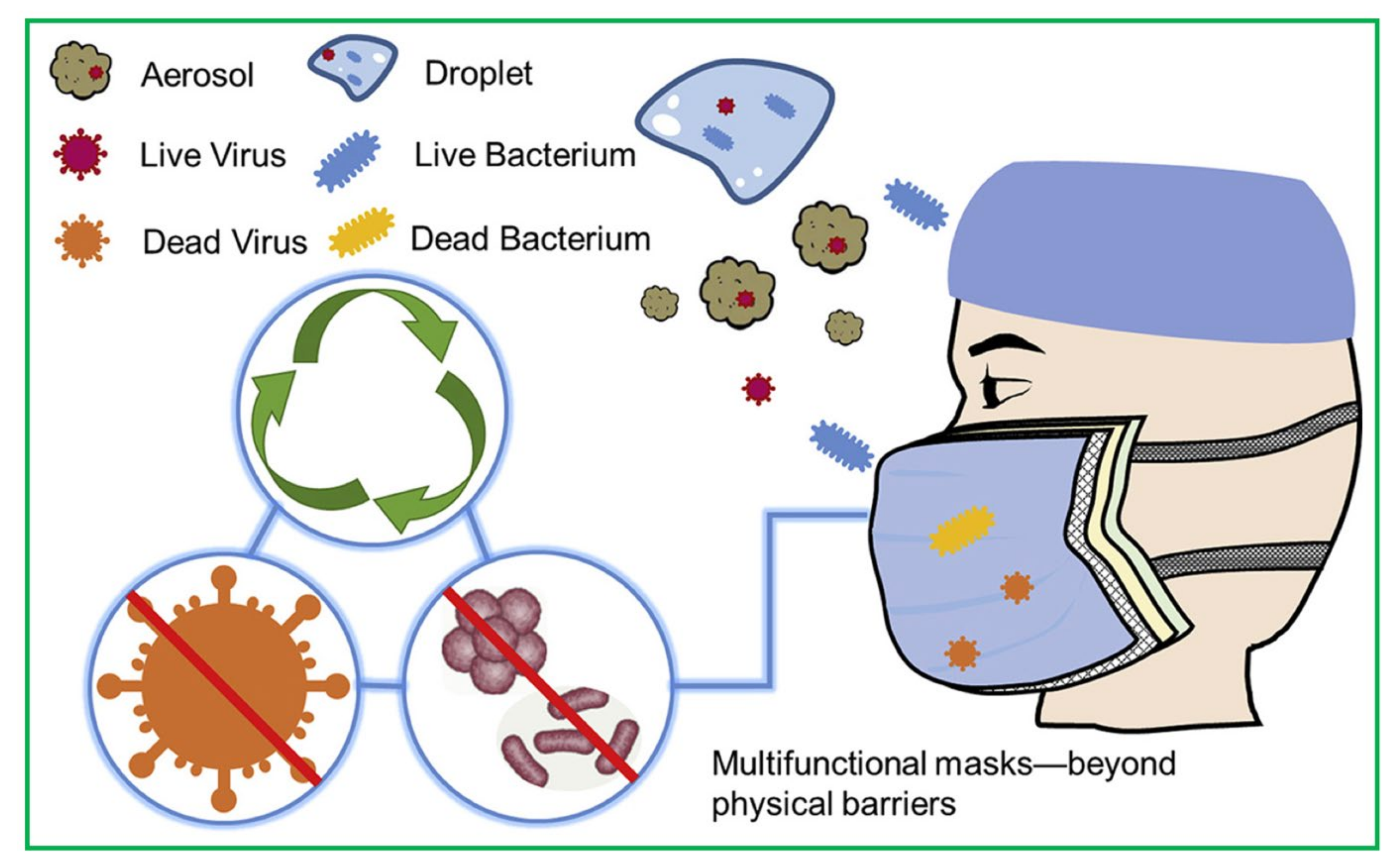
2.2. Healthcare
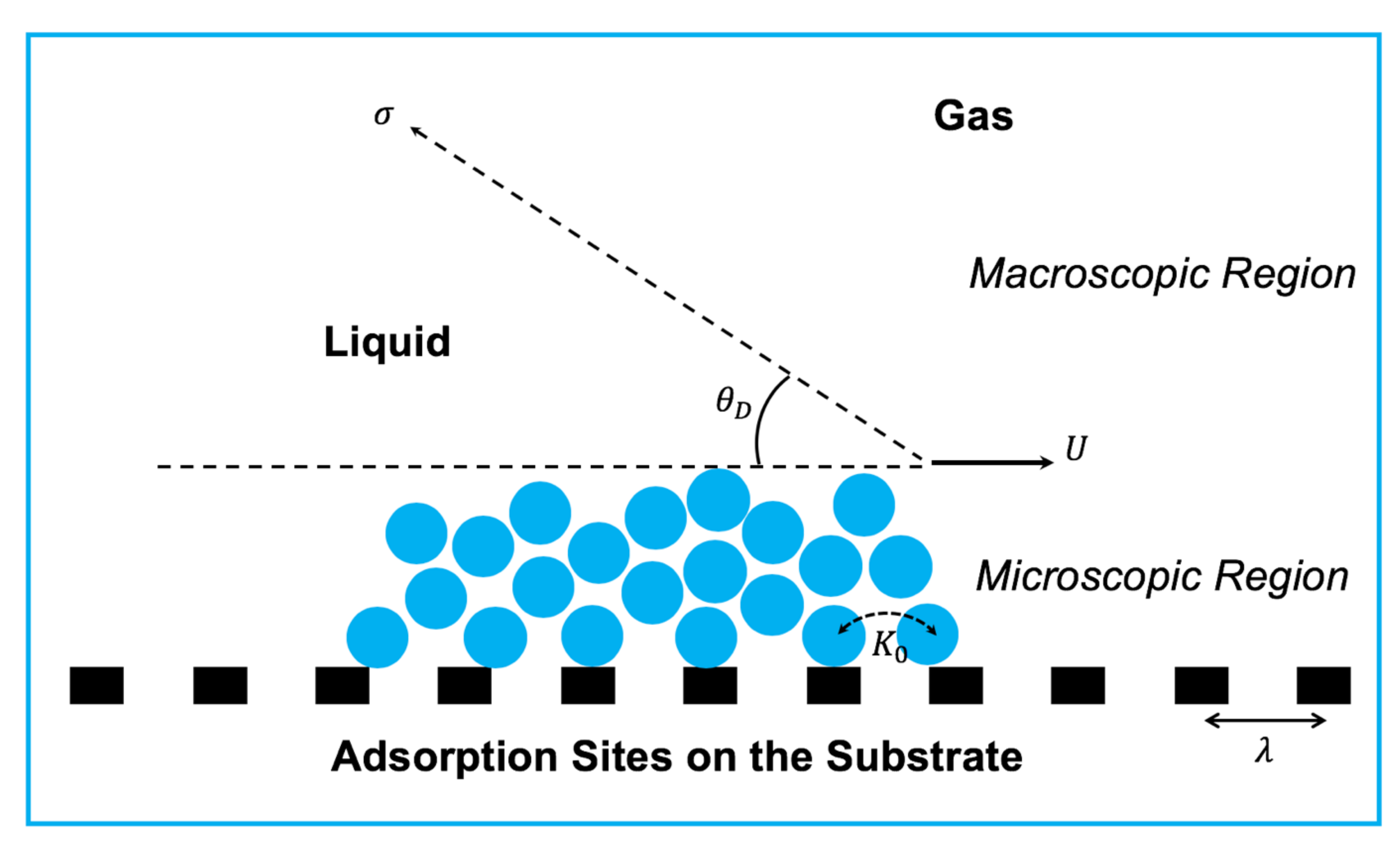
3. Molecular Kinetic Theory
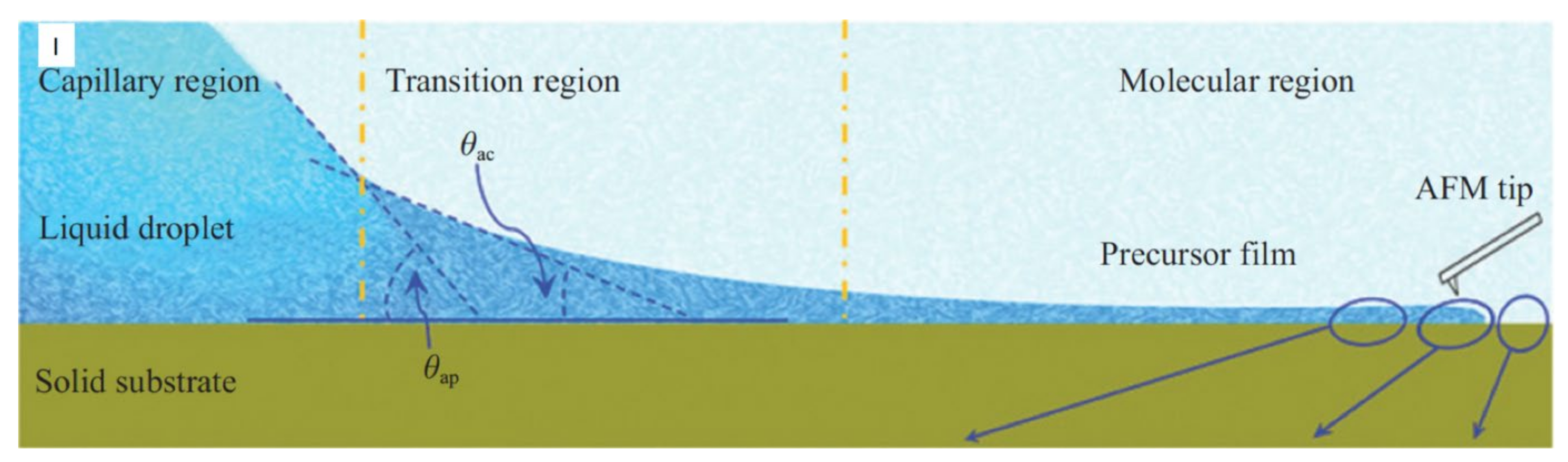
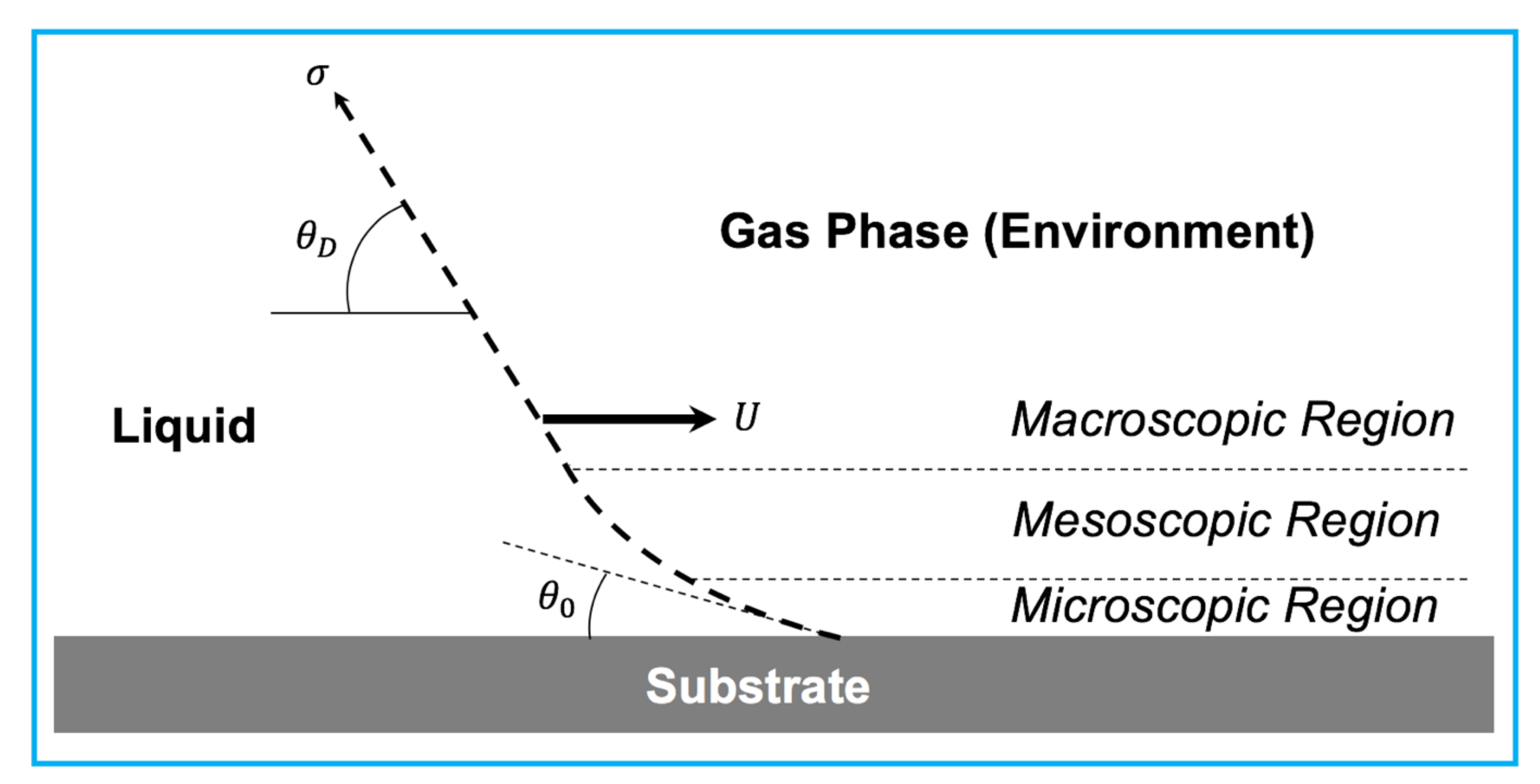
4. Hydrodynamics Theory
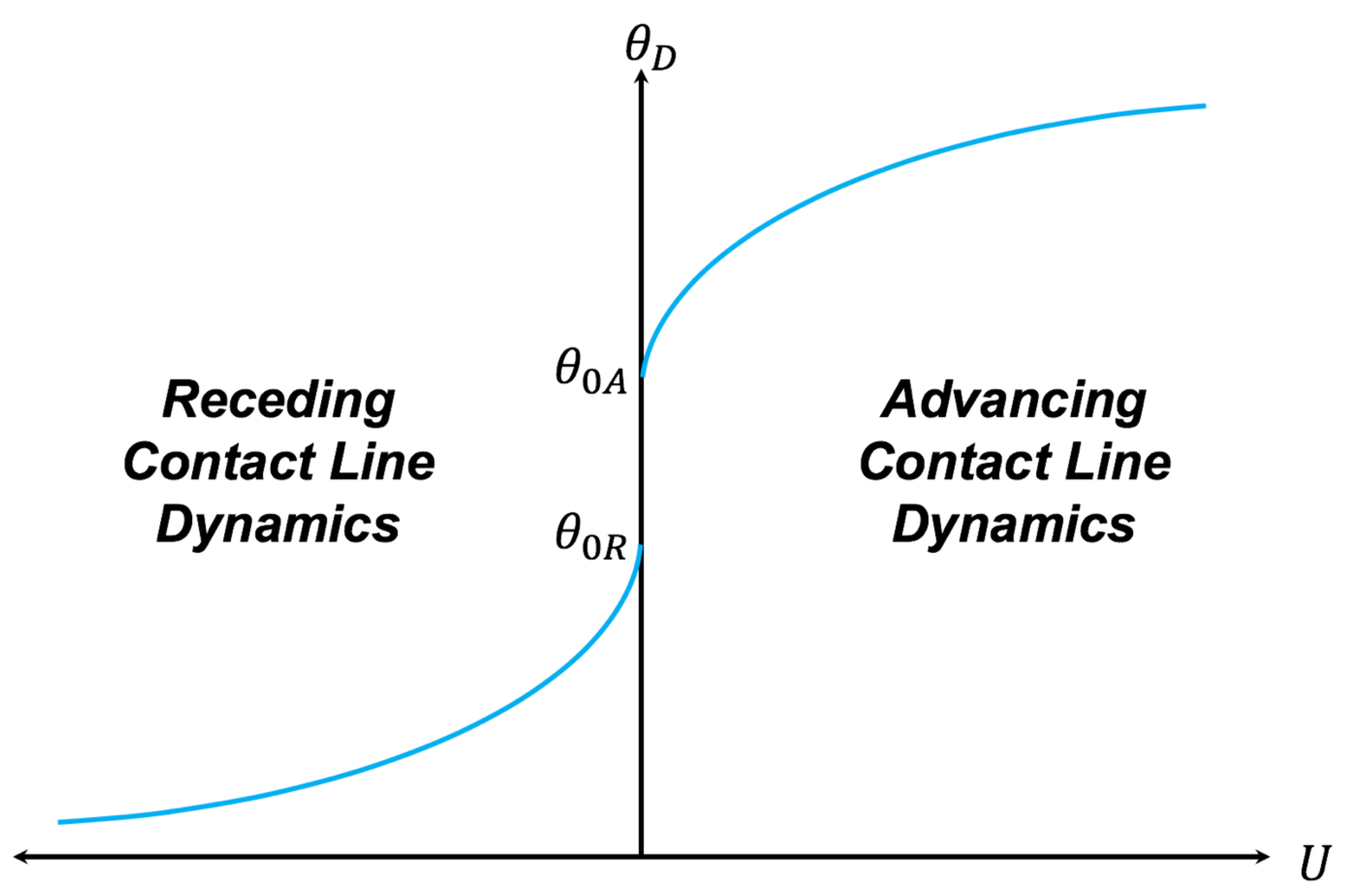
5. Molecular Kinetic Theory: Advantages and Limitations
6. Hydrodynamics Theory: Advantages and Limitations
7. Future Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ren, W.; Weinan, E. Contact Line Dynamics on Heterogeneous Surfaces. Phys. Fluids 2011, 23, 072103. [Google Scholar] [CrossRef]
- Eggers, J.; Stone, H.A. Characteristic Lengths at Moving Contact Lines for a Perfectly Wetting Fluid: The Influence of Speed on The Dynamic Contact Angle. J. Fluid Mech. 2004, 505, 309–321. [Google Scholar] [CrossRef]
- Diaz, M.E.; Cerro, R.L. A General Solution of Dewetting Flow with a Moving Contact Line. Phys. Fluids 2021, 33, 103601. [Google Scholar] [CrossRef]
- Dodds, S.; Carvalho, M.D.S.; Kumar, S. Stretching and Slipping of Liquid Bridges Near Plates and Cavities. Phys. Fluids 2009, 21, 092103. [Google Scholar] [CrossRef]
- Dodds, S.; Carvalho, M.; Kumar, S. Stretching Liquid Bridges with Moving Contact Lines: The Role of Inertia. Phys. Fluids 2011, 23, 092101. [Google Scholar] [CrossRef]
- Dodds, S.; Carvalho, M.S.; Kumar, S. The Dynamics of Three-Dimensional Liquid Bridges with Pinned and Moving Contact Lines. J. Fluid Mech. 2012, 707, 521–540. [Google Scholar] [CrossRef]
- Ghosh, A.; Bandyopadhyay, D.; Sharma, A. Micro-Patterning of Coatings on A Fiber Surface Exploiting the Contact Instabilities of Thin Viscoelastic Films. Phys. Fluids 2018, 30, 114101. [Google Scholar] [CrossRef]
- Harikrishnan, A.R.; Dhar, P.; Agnihotri, P.K.; Gedupudi, S.; Das, S.K. Correlating Contact Line Capillarity and Dynamic Contact Angle Hysteresis in Surfactant-Nanoparticle Based Complex Fluids. Phys. Fluids 2018, 30, 042006. [Google Scholar] [CrossRef]
- Kumar, S. Liquid Transfer in Printing Processes: Liquid Bridges with Moving Contact Lines. Annu. Rev. Fluid Mech. 2015, 47, 67–94. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Vandre, E.; Carvalho, M.S.; Kumar, S. Dynamic Wetting Failure and Hydrodynamic Assist in Curtain Coating. J. Fluid Mech. 2016, 808, 290–315. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Vandre, E.; Carvalho, M.S.; Kumar, S. Dynamic wetting failure in surfactant solutions. J. Fluid Mech. 2016, 789, 285–309. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Carvalho, M.S.; Kumar, S. Mechanisms of Dynamic Wetting Failure in the Presence of Soluble Surfactants. J. Fluid Mech. 2017, 825, 677–703. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Carvalho, M.S.; Kumar, S. Dynamic Wetting Failure in Curtain Coating: Comparison of Model Predictions and Experimental Observations. Chem. Eng. Sci. 2018, 195, 74–82. [Google Scholar] [CrossRef]
- Liu, M.; Chen, X.-P. Numerical Study on the Stick-Slip Motion of Contact Line Moving on Heterogeneous Surfaces. Phys. Fluids 2017, 29, 082102. [Google Scholar] [CrossRef]
- Ding, H.; Spelt, P.D.M. Inertial Effects in Droplet Spreading: A Comparison Between Diffuse-Interface and Level-Set Simulations. J. Fluid Mech. 2007, 576, 287–296. [Google Scholar] [CrossRef]
- Biance, A.-L.; Clanet, C.; Quéré, D. First Steps in the Spreading of a Liquid Droplet. Phys. Rev. E 2004, 69, 016301. [Google Scholar] [CrossRef]
- Foister, R.T. The Kinetics of Displacement Wetting in Liquid/Liquid/Solid Systems. J. Colloid Interface Sci. 1990, 136, 266–282. [Google Scholar] [CrossRef]
- Spelt, P.D. A level-Set Approach for Simulations of Flows with Multiple Moving Contact Lines with Hysteresis. J. Comput. Phys. 2005, 207, 389–404. [Google Scholar] [CrossRef]
- Wang, H. From Contact Line Structures to Wetting Dynamics. Langmuir 2019, 35, 10233–10245. [Google Scholar] [CrossRef]
- Popescu, M.N.; Oshanin, G.; Dietrich, S.; Cazabat, A.-M. Precursor Films in Wetting Phenomena. J. Phys. Condens. Matter 2012, 24, 243102. [Google Scholar] [CrossRef]
- Pham, T.; Kumar, S. Imbibition and Evaporation of Droplets of Colloidal Suspensions on Permeable Substrates. Phys. Rev. Fluids 2019, 4, 034004. [Google Scholar] [CrossRef]
- Charitatos, V.; Kumar, S. A Thin-Film Model for Droplet Spreading on Soft Solid Substrates. Soft Matter 2020, 16, 8284–8298. [Google Scholar] [CrossRef]
- Raske, N.; Hewson, R.W.; Kapur, N.; De Boer, G. A Predictive Model for Discrete Cell Gravure Roll Coating. Phys. Fluids 2017, 29, 062101. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Griffith, W.B.; Pujari, S.; Francis, L.F.; Carvalho, M.S. Effect of Viscoelasticity on Stability of Liquid Curtain. J. Non-Newton. Fluid Mech. 2018, 257, 83–94. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Griffith, W.B.; Pujari, S.; Francis, L.F.; Carvalho, M.S. Effect of Rheological Properties of Shear Thinning Liquids on Curtain Stability. J. Non-Newton. Fluid Mech. 2018, 263, 69–76. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Pujari, S.; Francis, L.F.; Carvalho, M.S. Delaying Breakup and Avoiding Air Entrainment in Curtain Coating Using a Two-Layer Liquid Structure. Chem. Eng. Sci. 2019, 213, 115376. [Google Scholar] [CrossRef]
- Karim, A.M. Experimental Dynamics of Newtonian and Non-Newtonian Droplets Impacting Liquid Surface with Different Rheology. Phys. Fluids 2020, 32, 043102. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Pujari, S.; Francis, L.F.; Carvalho, M.S. Contact Line Dynamics in Curtain Coating of Non-Newtonian Liquids. Phys. Fluids 2021, 33, 103103. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Pujari, S. Liquid Film Stability and Contact Line Dynamics of Emulsion Liquid Films in Curtain Coating Process. J. Coat. Technol. Res. 2021, 18, 1531–1541. [Google Scholar] [CrossRef]
- Mohammad Karim, A.; Pujari, S.; Suszynski, W.J.; Francis, L.F.; Carvalho, M.S.; Yadav, V. Methods for Curtain Coating Substrates. U.S. Patent No: 11,369,988 B2, 28 June 2022. [Google Scholar]
- Mohammad Karim, A.; Pujari, S.; Suszynski, W.J.; Francis, L.F.; Carvalho, M.S.; Yadav, V. Methods for Curtain Coating Substrates. Application Publication No: WO 2019/190623 A1, 3 October 2019. [Google Scholar]
- Omori, T.; Kajishima, T. Apparent and Microscopic Dynamic Contact Angles in Confined Flows. Phys. Fluids 2017, 29, 112107. [Google Scholar] [CrossRef]
- Patil, N.D.; Shaikh, J.; Sharma, A.; Bhardwaj, R. Droplet Impact Dynamics Over a Range of Capillary Numbers and Surface Wettability: Assessment of Moving Contact Line Models and Energy Budget Analysis. Phys. Fluids 2022, 34, 052119. [Google Scholar] [CrossRef]
- Peschka, D. Variational Approach to Dynamic Contact Angles for Thin Films. Phys. Fluids 2018, 30, 082115. [Google Scholar] [CrossRef]
- Park, J.; Kumar, S. Droplet Sliding on an Inclined Substrate with a Topographical Defect. Langmuir 2017, 33, 7352–7363. [Google Scholar] [CrossRef]
- Vandre, E.; Carvalho, M.S.; Kumar, S. Delaying the Onset of Dynamic Wetting Failure through Meniscus Confinement. J. Fluid Mech. 2012, 707, 496–520. [Google Scholar] [CrossRef]
- Vandre, E.; Carvalho, M.S.; Kumar, S. On the Mechanism of Wetting Failure during Fluid Displacement along a Moving Substrate. Phys. Fluids 2013, 25, 102103. [Google Scholar] [CrossRef]
- Vandre, E.; Carvalho, M.S.; Kumar, S. Characteristics of Air Entrainment during Dynamic Wetting Failure along a Planar Substrate. J. Fluid Mech. 2014, 747, 119–140. [Google Scholar] [CrossRef]
- Xia, Y.; Qin, J. Dynamics of Moving Contact Line on a Transversely Patterned Inclined Surface. Phys. Fluids 2020, 32, 042101. [Google Scholar] [CrossRef]
- Xu, X.; Wang, X. Theoretical Analysis for Dynamic Contact Angle Hysteresis on Chemically Patterned Surfaces. Phys. Fluids 2020, 32, 112102. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, P.; Borg, M.K.; Reese, J.; Wen, D. A Critical Assessment of the Line Tension Determined by the Modified Young’s Equation. Phys. Fluids 2018, 30, 082003. [Google Scholar] [CrossRef]
- Zheng, W.; Wen, B.; Sun, C.; Bai, B. Effects of Surface Wettability on Contact Line Motion in Liquid–Liquid Displacement. Phys. Fluids 2021, 33, 082101. [Google Scholar] [CrossRef]
- Bergeron, V.; Bonn, D.; Martin, J.Y.; Vovelle, L. Controlling Droplet Deposition with Polymer Additives. Nature 2000, 405, 772–775. [Google Scholar] [CrossRef]
- Sansom, M.S.; Biggin, P.C. Water at the Nanoscale. Nature 2001, 414, 157–159. [Google Scholar] [CrossRef]
- Xu, J.; Yan, X.; Liu, G.; Xie, J. The Critical Nanofluid Concentration as the Crossover between Changed and Unchanged Solar-Driven Droplet Evaporation Rates. Nano Energy 2019, 57, 791–803. [Google Scholar] [CrossRef]
- Wang, H.; Garimella, S.V.; Murthy, J.Y. Characteristics of an Evaporating Thin Film in a Microchannel. Int. J. Heat Mass Transf. 2007, 50, 3933–3942. [Google Scholar] [CrossRef]
- Wang, X.D.; Lee, D.J.; Peng, A.X.F.; Lai, J.Y. Spreading Dynamics and Dynamic Contact Angle of Non-Newtonian Fluids. Langmuir 2007, 23, 8042–8047. [Google Scholar] [CrossRef]
- Wang, X.D.; Zhang, Y.; Lee, D.J.; Peng, X.F. Spreading of Completely Wetting or Partially Wetting Power-Law Fluid on Solid Surface. Langmuir 2007, 23, 9258–9262. [Google Scholar] [CrossRef]
- Raghupathi, P.A.; Kandlikar, S.G. Contact Line Region Heat Transfer Mechanisms for an Evaporating Interface. Int. J. Heat Mass Transf. 2016, 95, 296–306. [Google Scholar] [CrossRef]
- Lu, G.; Wang, X.D.; Duan, Y.Y. A Critical Review of Dynamic Wetting by Complex Fluids: From Newtonian Fluids to Non-Newtonian Fluids and Nanofluids. Adv. Colloid Interface Sci. 2016, 236, 43–62. [Google Scholar] [CrossRef]
- Sui, Y.; Ding, H.; Spelt, P.D. Numerical Simulations of Flows with Moving Contact Lines. Annu. Rev. Fluid Mech. 2014, 46, 97–119. [Google Scholar] [CrossRef]
- Abe, Y.; Zhang, B.; Gordillo, L.; Mohammad Karim, A.; Francis, L.F.; Cheng, X. Dynamic Self-Assembly of Charged Colloidal Strings and Walls in Simple Fluid Flows. Soft Matter 2017, 13, 1681–1692. [Google Scholar] [CrossRef]
- Karim, A.M.; Suszynski, W.J.; Francis, L.F.; Carvalho, M.S. Effect of Viscosity on Liquid Curtain Stability. AIChE J. 2017, 64, 1448–1457. [Google Scholar] [CrossRef]
- Dwivedi, R.K.; Jain, V.; Muralidhar, K. Dynamic Contact Angle Model for Resolving Low-Viscosity Droplet Oscillations During Spreading Over a Surface with Varying Wettability. Phys. Rev. Fluids 2022, 7, 034002. [Google Scholar] [CrossRef]
- Kim, J.-H.; Kavehpour, H.P.; Rothstein, J.P. Dynamic Contact Angle Measurements on Superhydrophobic Surfaces. Phys. Fluids 2015, 27, 032107. [Google Scholar] [CrossRef]
- Fernández-Toledano, J.C.; Blake, T.D.; De Coninck, J. Taking a Closer Look: A Molecular-Dynamics Investigation of Microscopic and Apparent Dynamic Contact Angles. J. Colloid Interface Sci. 2021, 587, 311–323. [Google Scholar] [CrossRef]
- Qian, T.; Wang, X.-P.; Sheng, P. Molecular Scale Contact Line Hydrodynamics of Immiscible Flows. Phys. Rev. E 2003, 68, 016306. [Google Scholar] [CrossRef]
- Wang, X.-P.; Qian, T.; Sheng, P. Moving Contact Line on Chemically Patterned Surfaces. J. Fluid Mech. 2008, 605, 59–78. [Google Scholar] [CrossRef]
- Chen, X.; Wang, X.; Xu, X. Analysis of the Cahn–Hilliard Equation with a Relaxation Boundary Condition Modeling the Contact Angle Dynamics. Arch. Ration. Mech. Anal. 2014, 213, 1–24. [Google Scholar] [CrossRef]
- Savva, N.; Kalliadasis, S. Dynamics of Moving Contact Lines: A Comparison between Slip and Precursor Film Models. Eur. Lett. 2011, 94, 64004. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Ramachandran, R. Geometric Interpretation of Surface Tension Equilibrium in Superhydrophobic Systems. Entropy 2015, 17, 4684–4700. [Google Scholar] [CrossRef]
- Nikolayev, V.S. Evaporation Effect on the Contact Angle and Contact Line Dynamics. In The Surface Wettability Effect on Phase Change; Marengo, M., De Coninck, J., Eds.; Springer Nature: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Young, T. III. An essay on the cohesion of fluids. Philos. Trans. R. Soc. Lond. 1805, 95, 65–87. [Google Scholar] [CrossRef]
- Shikhmurzaev, Y. The Moving Contact Line on a Smooth Solid Surface. Int. J. Multiph. Flow 1993, 19, 589–610. [Google Scholar] [CrossRef]
- Shikhmurzaev, Y.D. Mathematical Modeling of Wetting Hydrodynamics. Fluid Dyn. Res. 1994, 13, 45–64. [Google Scholar] [CrossRef]
- Shikhmurzaev, Y.D. Moving Contact Lines in Liquid/Liquid/Solid Systems. J. Fluid Mech. 1997, 334, 211–249. [Google Scholar] [CrossRef]
- De Ruijter, M.J.; De Coninck, J.; Blake, T.D.; Clarke, A.A.; Rankin, A. Contact Angle Relaxation during the Spreading of Partially Wetting Drops. Langmuir 1997, 13, 7293–7298. [Google Scholar] [CrossRef]
- Blake, T.D. The Physics of Moving Wetting Lines. J. Colloid Interface Sci. 2006, 299, 1–13. [Google Scholar] [CrossRef]
- Cox, R.G. The Dynamics of the Spreading of Liquids on a Solid Surface. Part 1. Viscous Flow. J. Fluid Mech. 1986, 168, 169–194. [Google Scholar] [CrossRef]
- Huh, C.; Scriven, J.L.E. Hydrodynamic Model of Steady Movement of a Solid/Liquid/Fluid Contact Line. J. Colloid Interface Sci. 1971, 35, 85–101. [Google Scholar] [CrossRef]
- Voinov, O.V. Hydrodynamics of Wetting. Izv. Akad. Nauk. SSSR Mekhanika Zhidkosti I Gaza 1976, 5, 714–721. [Google Scholar] [CrossRef]
- De Gennes, P.G. Deposition of Langmuir-Blodgett Layers. Colloid Polym. Sci. 1986, 264, 463–465. [Google Scholar] [CrossRef]
- Butt, H.; Liu, J.; Koynov, K.; Straub, B.; Hinduja, C.; Roismann, I.; Berger, R.; Li, X.; Vollmer, D.; Steffen, W.; et al. Contact Angle Hysteresis. Curr. Opin. Colloid Interface Sci. 2022, 59, 101574. [Google Scholar] [CrossRef]
- Bartell, F.E.; Wooley, A.D. Solid-Liquid-Air Contact Angles and Their Dependence upon the Surface Condition of the Solid. J. Am. Chem. Soc. 1933, 55, 3518–3527. [Google Scholar] [CrossRef]
- Schäffer, E.; Wong, P.-Z. Contact Line Dynamics Near the Pinning Threshold: A Capillary Rise and Fall Experiment. Phys. Rev. E 2000, 61, 5257–5277. [Google Scholar] [CrossRef]
- Johnson, R.E.; Dettre, R.H. Wettability and Contact Angles. In Surface and Colloid Science; Matijevic, E., Ed.; Wiley-Interscience: New York, NY, USA, 1969; pp. 85–153. [Google Scholar]
- Wang, J.-H.; Claesson, P.M.; Parker, J.L.; Yasuda, H. Dynamic Contact Angles and Contact Angle Hysteresis of Plasma Polymers. Langmuir 1994, 10, 3887–3897. [Google Scholar] [CrossRef]
- Vega, M.J.; Gouttiere, C.; Seveno, D.; Blake, T.D.; Voue, M.; de Coninck, J. Experimental Investigation of the Link Be-Tween Static and Dynamic Wetting by Forced Wetting of Nylon Filament. Langmuir 2007, 23, 10628–10634. [Google Scholar] [CrossRef]
- Karim, A.M.; Kavehpour, H.P. Effect of Viscous Force on Dynamic Contact Angle Measurement Using Wilhelmy Plate Method. Colloids Surfaces A Physicochem. Eng. Asp. 2018, 548, 54–60. [Google Scholar] [CrossRef]
- Bartell, F.E.; Shepard, J.W. The Effect of Surface Roughness on Apparent Contact Angles and on Contact Angle Hysteresis. I. The System Paraffin−Water−Air. J. Phys. Chem. 1953, 57, 211–215. [Google Scholar] [CrossRef]
- Eick, J.; Good, R.; Neumann, A. Thermodynamics of Contact Angles. II. Rough Solid Surfaces. J. Colloid Interface Sci. 1975, 53, 235–248. [Google Scholar] [CrossRef]
- Oliver, J.F.; Mason, S.G. Liquid Spreading on Rough Metal Surfaces. J. Mater. Sci. 1980, 15, 431–437. [Google Scholar] [CrossRef]
- Good, R.J.A. Thermodynamic Derivation of Wenzel’s Modification of Young’s Equation for Contact Angles, Together with a Theory of Hysteresis. J. Am. Chem. Soc. 1952, 74, 5041–5042. [Google Scholar] [CrossRef]
- Neumann, A.; Good, R. Thermodynamics of Contact Angles. I. Heterogeneous Solid Surfaces. J. Colloid Interface Sci. 1972, 38, 341–358. [Google Scholar] [CrossRef]
- Schwartz, L.W.; Garoff, S. Contact Angle Hysteresis on Heterogeneous surfaces. Langmuir 1985, 1, 219–230. [Google Scholar] [CrossRef]
- Marmur, A. Contact Angle Hysteresis on Heterogeneous Smooth Surfaces. J. Colloid Interface Sci. 1994, 168, 40–46. [Google Scholar] [CrossRef]
- Decker, E.L.; Garoff, S. Contact Line Structure and Dynamics on Surfaces with Contact Angle Hysteresis. Langmuir 1997, 13, 6321–6332. [Google Scholar] [CrossRef]
- Brandon, S.; Marmur, A. Simulation of Contact Angle Hysteresis on Chemically Heterogeneous Surfaces. J. Colloid Interface Sci. 1996, 183, 351–355. [Google Scholar] [CrossRef] [PubMed]
- Yue, P.; Zhou, C.; Feng, J.J. Sharp-Interface Limit of the Cahn–Hilliard Model for Moving Contact Lines. J. Fluid Mech. 2010, 645, 279–294. [Google Scholar] [CrossRef]
- Yue, P.; Zhou, C.; Feng, J.J.; Ollivier-Gooch, C.F.; Hu, H.H. Phase-Field Simulations of Interfacial Dynamics in Viscoelastic Fluids Using Finite Elements with Adaptive Meshing. J. Comput. Phys. 2006, 219, 47–67. [Google Scholar] [CrossRef]
- Shanahan, M.E.R. Simple Theory of “Stick-Slip” Wetting Hysteresis. Langmuir 1995, 11, 1041–1043. [Google Scholar] [CrossRef]
- Kalinin, Y.V.; Berejnov, V.; Thorne, R.E. Contact Line Pinning by Microfabricated Patterns: Effects of Microscale topography. Langmuir 2009, 25, 5391–5397. [Google Scholar] [CrossRef]
- Dussan, V.E.B. On the Spreading of Liquids on Solid Surfaces: Static and Dynamic Contact Lines. Annu. Rev. Fluid Mech. 1979, 11, 371–400. [Google Scholar] [CrossRef]
- Van Der Waals, J.D. The Thermodynamic Theory of Capillarity under the Hypothesis of a Continuous Variation of Density. J. Stat. Phys. 1979, 20, 200–244. [Google Scholar] [CrossRef]
- Blake, T.D.; Berg, J.C. Wettability: Dynamic Contact Angles and Wetting Kinetics; Marcel Dekker: New York, NY, USA, 1993; Volume 49, pp. 251–309. [Google Scholar]
- Blake, T.; Shikhmurzaev, Y. Dynamic Wetting by Liquids of Different Viscosity. J. Colloid Interface Sci. 2002, 253, 196–202. [Google Scholar] [CrossRef]
- Blake, T.D.; Bracke, M.; Shikhmurzaev, Y.D. Experimental Evidence of Non-Local Hydrodynamic Influence on the Dynamic Contact Angle. Phys. Fluids 1999, 11, 1995. [Google Scholar] [CrossRef]
- Cahn, J.W. Critical Point Wetting. J. Chem. Phys. 1977, 66, 3667–3672. [Google Scholar] [CrossRef]
- Jacqmin, D. Contact-Line Dynamics of a Diffuse Fluid Interface. J. Fluid Mech. 2000, 402, 57–88. [Google Scholar] [CrossRef]
- Cahn, J.W.; Hilliard, J.E. Free Energy of a Non-Uniform System. Part I. Interfacial Free Energy. J. Chem. Phys. 1958, 28, 258–267. [Google Scholar] [CrossRef]
- Blake, T.D.; Haynes, J.M. Kinetics of Liquid/Liquid Displacement. J. Colloid Interf. Sci. 1969, 30, 421–423. [Google Scholar] [CrossRef]
- Prevost, A.; Rolley, E.; Guthmann, C. Thermally Activated Motion of the Contact Line of a Liquid 4 He Meniscus on a Cesium Substrate. Phys. Rev. Lett. 1999, 83, 348–351. [Google Scholar] [CrossRef]
- Huh, C.; Mason, S.G. The Steady Movement of a Liquid Meniscus in a Capillary Tube. J. Fluid Mech. 1977, 81, 401–419. [Google Scholar] [CrossRef]
- Dussan, V.E.B. The Moving Contact Line: The Slip Boundary Condition. J. Fluid Mech. 1976, 77, 665–684. [Google Scholar] [CrossRef]
- Ngan, C.G.; Dussan, V.E.B. On the Dynamics of Liquid Spreading on Solid Surfaces. J. Fluid Mech. 1989, 209, 191–226. [Google Scholar] [CrossRef]
- Hoffman, R.L. A Study of the Advancing Interface. I. Interface Shape in Liquid—Gas Systems. J. Colloid Interface Sci. 1975, 50, 228–241. [Google Scholar] [CrossRef]
- Tanner, L.H. The Spreading of Silicone Oil Drops on Horizontal Surfaces. J. Phys. D Appl. Phys. 1979, 12, 1473–1484. [Google Scholar] [CrossRef]
- Hocking, L.M. A Moving Fluid Interface. Part 2. The Removal of the Force Singularity by A Slip Flow. J. Fluid Mech. 1977, 79, 209–229. [Google Scholar] [CrossRef]
- Petrov, P.G.; Petrov, J.G. A Combined Molecular-Hydrodynamic Approach to Wetting Kinetics. Langmuir 1992, 8, 1762–1767. [Google Scholar] [CrossRef]
- Brochard-Wyart, F.; de Gennes, P. Dynamics of Partial Wetting. Adv. Colloid Interface Sci. 1992, 39, 1–11. [Google Scholar] [CrossRef]
- De Ruijter, M.J.; De Coninck, J.; Oshanin, G. Droplet Spreading: Partial Wetting Regime Revisited. Langmuir 1999, 15, 2209–2216. [Google Scholar] [CrossRef]
- Blake, T.D.; Ruschak, K.J. Liquid Film Coating; Schweizer, P.M., Kistler, S.F., Eds.; Chapman & Hall: London, UK, 1997; Volume 63. [Google Scholar]
- Petrov, J.G.; Ralston, J.; Schneemilch, A.M.; Hayes, R.A. Dynamics of Partial Wetting and Dewetting in Well-Defined Systems. J. Phys. Chem. B 2003, 107, 1634–1645. [Google Scholar] [CrossRef]
- Yuan, Q.; Zhao, Y.-P. Precursor Film in Dynamic Wetting, Electrowetting, and Electro-Elasto-Capillarity. Phys. Rev. Lett. 2010, 104, 246101. [Google Scholar] [CrossRef]
- Hardy, W.B., III. The Spreading of Fluids on Glass. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1919, 38, 49–55. [Google Scholar] [CrossRef]
- Espín, L.; Kumar, S. Sagging of Evaporating Droplets of Colloidal Suspensions on Inclined Substrates. Langmuir 2014, 30, 11966–11974. [Google Scholar] [CrossRef]
- Karapetsas, G.; Sahu, K.C.; Matar, O.K. Evaporation of Sessile Droplets Laden with Particles and Insoluble Surfactants. Langmuir 2016, 32, 6871–6881. [Google Scholar] [CrossRef]
- Maki, K.L.; Kumar, S. Fast Evaporation of Spreading Droplets of Colloidal Suspensions. Langmuir 2011, 27, 11347–11363. [Google Scholar] [CrossRef]
- Wray, A.W.; Papageorgiou, D.T.; Craster, R.V.; Sefiane, K.; Matar, O.K. Electrostatic Suppression of the Coffee Stain Effect. Langmuir 2014, 30, 5849–5858. [Google Scholar] [CrossRef]
- Charitatos, V.; Kumar, S. Droplet Evaporation on Soft Solid Substrates. Soft Matter 2021, 17, 9339–9352. [Google Scholar] [CrossRef]
- Derjaguin, B.; Churaev, N. Structural Component of Disjoining Pressure. J. Colloid Interface Sci. 1974, 49, 249–255. [Google Scholar] [CrossRef]
- Karim, A.M. A Review of Physics of Moving Contact Line Dynamics Models and Its Applications in Interfacial Science. J. Appl. Phys. 2022, 132, 080701. [Google Scholar] [CrossRef]
- Afkhami, S. Challenges of Numerical Simulation of Dynamic Wetting Phenomena: A Review. Curr. Opin. Colloid Interface Sci. 2022, 57, 101523. [Google Scholar] [CrossRef]
- Zhao, Y. Moving Contact Line Problem: Advances and Perspectives. Theor. Appl. Mech. Lett. 2014, 4, 034002. [Google Scholar] [CrossRef]
- Soltman, D.; Smith, B.; Kang, H.; Morris, S.J.S.; Subramanian, V. Methodology for Inkjet Printing of Partially Wetting Films. Langmuir 2010, 26, 15686–15693. [Google Scholar] [CrossRef]
- Tarasevich, Y.Y.; Pravoslavnova, D.M. Segregation in Desiccated Sessile Drops of Biological Fluids. Eur. Phys. J. E 2007, 22, 311–314. [Google Scholar] [CrossRef]
- Karim, A.M. Experimental Dynamics of Newtonian Non-Elastic and Viscoelastic Droplets Impacting Immiscible Liquid Surface. AIP Adv. 2019, 9, 125141. [Google Scholar] [CrossRef]
- Backholm, M.; Molpeceres, D.; Vuckovac, M.; Nurmi, H.; Hokkanen, M.J.; Jokinen, V.; Timonen, J.V.I.; Ras, R.H.A. Water droplet friction and rolling dynamics on superhydrophobic surfaces. Commun. Mater. 2020, 1, 64. [Google Scholar] [CrossRef]
- De Coninck, J. An Introduction to Wettability and Wetting Phenomena. In The Surface Wettability Effect on Phase Change; Springer: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Mugele, F.; Baret, J.-C. Electrowetting: From Basics to Applications. J. Phys. Condens. Matter 2005, 17, R705–R774. [Google Scholar] [CrossRef]
- Jarosz, J.; Molliex, N.; Chenon, G.; Berge, B. Adaptive Eyeglasses for Presbyopia Correction: An Original Variable-Focus Technology. Opt. Express 2019, 27, 10533–10552. [Google Scholar] [CrossRef] [PubMed]
- Modak, C.D.; Kumar, A.; Tripathy, A.; Sen, P. Drop Impact Printing. Nat. Commun. 2020, 11, 4327. [Google Scholar] [CrossRef] [PubMed]
- Biswas, S.; Gawande, S.; Bromberg, V.; Sun, Y. Effects of Particle Size and Substrate Surface Properties on Deposition Dynamics of Inkjet-Printed Colloidal Drops for Printable Photovoltaics Fabrication. J. Sol. Energy Eng. 2010, 132, 021010. [Google Scholar] [CrossRef]
- Joshi, A.S.; Sun, Y. Numerical Simulation of Colloidal Drop Deposition Dynamics on Patterned Substrates for Printable Electronics Fabrication. J. Disp. Technol. 2010, 6, 579–585. [Google Scholar] [CrossRef]
- Chhasatia, V.H.; Joshi, A.S.; Sun, Y. Effect of Relative Humidity on Contact Angle and Particle Deposition Morphology of An Evaporating Colloidal Drop. Appl. Phys. Lett. 2010, 97, 231909. [Google Scholar] [CrossRef]
- Bigioni, T.P.; Lin, X.-M.; Nguyen, T.; Corwin, E.I.; Witten, T.A.; Jaeger, H.M. Kinetically Driven Self Assembly of Highly Ordered Nanoparticle Monolayers. Nat. Mater. 2006, 5, 265–270. [Google Scholar] [CrossRef]
- Sobac, B.; Brutin, D. Structural and Evaporative Evolutions in Desiccating Sessile Drops of Blood. Phys. Rev. E 2011, 84, 011603. [Google Scholar] [CrossRef]
- Gosselin, E.; Eynde, J.J.V.; Petit, A.; Conti, J.; De Coninck, J. Designing a High Performance, Stable Spectroscopic Biosensor for the Binding of Large and Small Molecules. J. Colloid Interface Sci. 2017, 508, 443–454. [Google Scholar] [CrossRef]
- Yao, M.; Tijing, L.D.; Naidu, G.; Kim, S.-H.; Matsuyama, H.; Fane, A.G.; Shon, H.K. A Review of Membrane Wettability for the Treatment of Saline Water Deploying Membrane Distillation. Desalination 2020, 479, 114312. [Google Scholar] [CrossRef]
- Melayil, K.R.; Mitra, S.K. Wetting, Adhesion, and Droplet Impact on Face Masks. Langmuir 2021, 37, 2810–2815. [Google Scholar] [CrossRef]
- Sharma, S.; Pinto, R.; Saha, A.; Chaudhuri, S.; Basu, S. Video: On Secondary Atomization and Blockage of Surrogate Cough Droplets in Single and Multi-Layer Face Masks. Sci. Adv. 2020, 7, eabf0452. [Google Scholar] [CrossRef]
- Liao, M.; Liu, H.; Wang, X.; Hu, X.; Huang, Y.; Liu, X.; Brenan, K.; Mecha, J.; Nirmalan, M.; Lu, J.R. A Technical Review of Face Mask Wearing in Preventing Respiratory COVID-19 Transmission. Curr. Opin. Colloid Interface Sci. 2021, 52, 101417. [Google Scholar] [CrossRef]
- Montessori, A.; Lauricella, M.; Tiribocchi, A. Computational Droplets: Where We Stand and How Far We Can Go. Eur. Lett. 2022, 138, 67001. [Google Scholar] [CrossRef]
- Powell, R.E.; Roseveare, W.E.; Eyring, H. The Theory of Rate Processes. Ind. Eng. Chem. 1941, 33, 430. [Google Scholar] [CrossRef]
- Niavarani, A.; Priezjev, N. The Effective Slip Length and Vortex Formation in Laminar Flow over a Rough Surface. Phys. Fluids 2009, 21, 052105. [Google Scholar] [CrossRef]
- Niavarani, A.; Priezjev, N. Modeling the Combined Effect of Surface Roughness and Shear Rate on Slip Flow of Simple Fluids. Phys. Rev. E 2010, 81, 011606. [Google Scholar] [CrossRef]
- Xiaodong, W.; Xiaofeng, P.; Yuanyuan, D.; Buxuan, W. Dynamics of Spreading of Liquid on Solid Surface. Chin. J. Chem. Eng. 2007, 15, 730–737. [Google Scholar] [CrossRef]
- Cazabat, A.-M. How Does a Droplet Spread? Contemp. Phys. 1987, 28, 347–364. [Google Scholar] [CrossRef]
- Roques-Carmes, T.; Mathieu, V.; Gigante, A. Experimental Contribution to the Understanding of the Dynamics of Spreading of Newtonian Fluids: Effect of Volume, Viscosity and Surfactant. J. Colloid Interface Sci. 2010, 344, 180–197. [Google Scholar] [CrossRef]
- Eddi, A.; Winkels, K.G.; Snoeijer, J.H. Short time dynamics of viscous drop spreading. Phys. Fluids 2013, 25, 013102. [Google Scholar] [CrossRef]
- Legendre, D.; Maglio, M. Numerical Simulation of Spreading Drops. Colloids Surf. A Physicochem. Eng. Asp. 2013, 432, 29–37. [Google Scholar] [CrossRef]
- Zeid, W.B.; Brutin, D. Beyond Tanner’s Law: Role of Contact Line Evaporation on the Spreading of Viscous Droplet. Interfacial Phenom. Heat Transf. 2015, 3, 221–229. [Google Scholar] [CrossRef]
- Blake, T.D.; Dobson, R.A.; Ruschak, K.J. Wetting at High Capillary Numbers. J. Colloid Interface Sci. 2004, 279, 198–205. [Google Scholar] [CrossRef]
- Sedev, R. The Molecular-Kinetic Approach to Wetting Dynamics: Achievements and Limitations. Adv. Colloid Interface Sci. 2015, 222, 661–669. [Google Scholar] [CrossRef]
- Mohammad Karim, A. Parametric Study of Liquid Contact Line Dynamics: Adhesion vs. Hydrodynamics. Ph.D. Thesis, University of California, Los Angeles, CA, USA, 2015. [Google Scholar]
- Karim, A.M.; Kavehpour, H.P. Dynamics of Spreading on Ultra-Hydrophobic Surfaces. J. Coat. Technol. Res. 2015, 12, 959–964. [Google Scholar] [CrossRef]
- Karim, A.M.; Rothstein, J.P.; Kavehpour, H.P. Experimental Study of Dynamic Contact Angles on Rough Hydrophobic Surfaces. J. Colloid Interface Sci. 2018, 513, 658–665. [Google Scholar] [CrossRef]
- Karim, A.M.; Fujii, K.; Kavehpour, H.P. Contact Line Dynamics of Gravity Driven Spreading of Liquids. Fluid Dyn. Res. 2021, 53, 035503. [Google Scholar] [CrossRef]
- Seppecher, P. Moving Contact Lines in the Cahn-Hilliard Theory. Int. J. Eng. Sci. 1996, 34, 977–992. [Google Scholar] [CrossRef]
- Bonn, D.; Eggers, J.; Indekeu, J.; Meunier, J.; Rolley, E. Wetting and Spreading. Rev. Mod. Phys. 2009, 81, 739–805. [Google Scholar] [CrossRef]
- Zheng, L.; Wang, Y.-X.; Plawsky, J.L.; Wayner, P.C. Accuracy of Measurements of Curvature and Apparent Contact Angle in a Constrained Vapor Bubble Heat Exchanger. Int. J. Heat Mass Transf. 2002, 45, 2021–2030. [Google Scholar] [CrossRef]
- Karim, A.M.; Davis, S.H.; Kavehpour, H.P. Forced versus Spontaneous Spreading of Liquids. Langmuir 2016, 32, 10153–10158. [Google Scholar] [CrossRef]
- Karim, A.M.; Kavehpour, H.P. Spreading of Emulsions on a Solid Substrate. J. Coat. Technol. Res. 2013, 11, 103–108. [Google Scholar] [CrossRef]
- Carré, A.; Eustache, F. Spreading Kinetics of Shear-Thinning Fluids in Wetting and Dewetting Modes. Langmuir 2000, 16, 2936–2941. [Google Scholar] [CrossRef]
- Betelu, S.; Fontelos, M. Capillarity Driven Spreading of Power-Law Fluids. Appl. Math. Lett. 2003, 16, 1315–1320. [Google Scholar] [CrossRef]
- Betelú, S.; Fontelos, M. Capillarity Driven Spreading of Circular Drops of Shear-Thinning Fluid. Math. Comput. Model. 2004, 40, 729–734. [Google Scholar] [CrossRef]
- Starov, V.M.; Tyatyushkin, A.N.; Velarde, M.G.; Zhdanov, S.A. Spreading of Non-Newtonian Liquids over Solid Substrates. J. Colloid Interface Sci. 2003, 257, 284–290. [Google Scholar] [CrossRef]
- Liang, Z.-P.; Wang, X.-D.; Duan, Y.-Y.; Min, Q. Energy-Based Model for Capillary Spreading of Power-Law Liquids on a Horizontal Plane. Colloids Surf. A Physicochem. Eng. Asp. 2012, 403, 155–163. [Google Scholar] [CrossRef]
- Liang, Z.-P.; Wang, X.-D.; Lee, D.-J.; Peng, X.-F.; Su, A. Spreading Dynamics of Power-Law Fluid Droplets. J. Phys. Condens. Matter 2009, 21, 464117. [Google Scholar] [CrossRef]
- Liu, K.; Tian, Y.; Jiang, L. Bio-Inspired Superoleophobic and Smart Materials: Design, Fabrication, and Application. Prog. Mater. Sci. 2013, 58, 503–564. [Google Scholar] [CrossRef]
- Blake, T.; De Coninck, J. The Influence of Solid–Liquid Interactions on Dynamic Wetting. Adv. Colloid Interface Sci. 2002, 96, 21–36. [Google Scholar] [CrossRef]
- Ferrari, M.; Ravera, F. Surfactants and Wetting at Superhydrophobic Surfaces: Water Solutions and Non Aqueous Liquids. Adv. Colloid Interface Sci. 2010, 161, 22–28. [Google Scholar] [CrossRef]
- Yan, Y.; Gao, N.; Barthlott, W. Mimicking Natural Superhydrophobic Surfaces and Grasping the Wetting Process: A Review on Recent Progress in Preparing Superhydrophobic Surfaces. Adv. Colloid Interface Sci. 2011, 169, 80–105. [Google Scholar] [CrossRef]
- Bhushan, B.; Jung, Y.C. Natural and Biomimetic Artificial Surfaces for Superhydrophobicity, Self-Cleaning, Low Adhesion, and Drag Reduction. Prog. Mater. Sci. 2011, 56, 1–108. [Google Scholar] [CrossRef]
- Oberli, L.; Caruso, D.; Hall, C.; Fabretto, M.; Murphy, P.J.; Evans, D. Condensation and Freezing of Droplets on Superhydrophobic Surfaces. Adv. Colloid Interface Sci. 2014, 210, 47–57. [Google Scholar] [CrossRef]
- Webb, H.K.; Crawford, R.J.; Ivanova, E.P. Wettability of Natural Superhydrophobic Surfaces. Adv. Colloid Interface Sci. 2014, 210, 58–64. [Google Scholar] [CrossRef]
- Ma, M.; Hill, R.M. Superhydrophobic Surfaces. Curr. Opin. Colloid Interface Sci. 2006, 11, 193–202. [Google Scholar] [CrossRef]
- Koch, K.; Bhushan, B.; Barthlott, W. Multifunctional Surface Structures of Plants: An Inspiration for Biomimetics. Prog. Mater. Sci. 2009, 54, 137–178. [Google Scholar] [CrossRef]
- Savva, N.; Kalliadasis, S. Two-Dimensional Droplet Spreading Over Topographical Substrates. Phys. Fluids 2009, 21, 092102. [Google Scholar] [CrossRef]
- Savva, N.; Pavliotis, G.A.; Kalliadasis, S. Contact Lines Over Random Topographical Substrates. Part 2. Dynamics. J. Fluid Mech. 2011, 672, 384–410. [Google Scholar] [CrossRef]
- Pham, T.; Kumar, S. Drying of Droplets of Colloidal Suspensions on Rough Substrates. Langmuir 2017, 33, 10061–10076. [Google Scholar] [CrossRef]
- Charitatos, V.; Pham, T.; Kumar, S. Droplet Evaporation on Inclined Substrates. Phys. Rev. Fluids 2021, 6, 084001. [Google Scholar] [CrossRef]
- Espín, L.; Kumar, S. Droplet Spreading and Absorption on Rough, Permeable Substrates. J. Fluid Mech. 2015, 784, 465–486. [Google Scholar] [CrossRef]
- Ren, W.; Hu, D.; Weinan, E. Continuum Models for the Contact Line Problem. Phys. Fluids 2010, 22, 102103. [Google Scholar] [CrossRef]
- Lukyanov, A.V. Non-Locality of the Contact Line in Dynamic Wetting Phenomena. J. Colloid Interface Sci. 2021, 608, 2131–2141. [Google Scholar] [CrossRef]
- Li, X.; Pozrikidis, C. Shear Flow Over a Liquid Drop Adhering to a Solid Surface. J. Fluid Mech. 1996, 307, 167–190. [Google Scholar] [CrossRef]
- Moriarty, J.A.; Schwartz., L.W. Unsteady Spreading of Thin Liquid Films with Small Surface Tension. Phys. Fluids A Fluid Dyn. 1991, 3, 733–742. [Google Scholar] [CrossRef]
- Myers, T. Thin Films with High Surface Tension. SIAM Rev. 1998, 40, 441–462. [Google Scholar] [CrossRef]
- Wang, X.; Min, Q.; Zhang, Z.; Duan, Y. Effect of Moving Contact Line’s Curvature on Dynamic Wetting of non-Newtonian Fluids. Langmuir 2018, 34, 15612–15620. [Google Scholar] [CrossRef] [PubMed]
- Thompson, P.A.; Robbins, M.O. Simulations of Contact-Line Motion: Slip and the Dynamic Contact Angle. Phys. Rev. Lett. 1989, 63, 766–769. [Google Scholar] [CrossRef] [PubMed]
- Thompson, P.A.; Brinckerhoff, W.; Robbins, M.O. Microscopic Studies of Static and Dynamic Contact Angles. J. Adhes. Sci. Technol. 1993, 7, 535–554. [Google Scholar] [CrossRef]
- Romero, O.J.; Suszynski, W.J.; Scriven, L.E.; Carvalho, M.S. Low-Flow Limit in Slot Coating of Dilute Solutions of High Molecular Weight Polymer. J. Non-Newton. Fluid Mech. 2004, 118, 137–156. [Google Scholar] [CrossRef]
- Wei, Y.; Ramé, E.; Walker, L.; Garoff, S. Dynamic wetting with viscous Newtonian and non-Newtonian Fluids. J. Phys. Condens. Matter 2009, 21, 464126. [Google Scholar] [CrossRef] [PubMed]
- Cohu, O.; Benkreira, H. Entrainment of Air by a Solid Surface Plunging into a Non-Newtonian Liquid. AIChE J. 1998, 44, 2360–2368. [Google Scholar] [CrossRef]
- Charitatos, V.; Suszynski, W.J.; Carvalho, M.S.; Kumar, S. Dynamic Wetting Failure in Shear-Thinning and Shear-Thickening Liquids. J. Fluid Mech. 2020, 892, A1. [Google Scholar] [CrossRef]
- Liang, Z.-P.; Wang, X.-D.; Duan, Y.; Min, Q.; Wang, C.; Lee, D.-J. Dynamic Wetting of Non-Newtonian Fluids: Multicomponent Molecular-Kinetic Approach. Langmuir 2010, 26, 14594–14599. [Google Scholar] [CrossRef]
- Carré, A.; Woehl, P. Hydrodynamic Behavior at the Triple Line of Spreading Liquids and the Divergence Problem. Langmuir 2002, 18, 3600–3603. [Google Scholar] [CrossRef]
- Carré, A.; Woehl, P. Spreading of Silicone Oils on Glass in Two Geometries. Langmuir 2005, 22, 134–139. [Google Scholar] [CrossRef]

| Model | Equation | Condition(s) | Reference |
|---|---|---|---|
| Hoffman | Experimental study; forced motion of silicone oil on a glass capillary; applicable for nonvolatile liquids with zero static contact angle; negligible inertia and gravity; flow with low capillary number | [106] | |
| Tanner | ; | Experimental study and scaling analysis of spontaneous motion of a viscous liquid droplet on a horizontal substrate; steady-state flow; proportionality constant depends on physical characteristics of liquids and flow; based on lubrication assumption; is the time-varying droplet radius of the droplet and is the time-varying dynamic contact angle; suitable for small dynamic contact angles | [107] |
| Hoffman–Voinov–Tanner | Dynamics of contact line motion at low capillary numbers on a substrate with zero static contact angle; microscopic region of the flow is excluded; also suitable for flow with finite (non-zero) static contact angle; derived by numerical, experimental, and theoretical studies; also applicable for physics of viscous evaporative droplet spreading on a substrate | [71,106,107,147,148,149,150,151,152] | |
| Generalized Hoffman–Voinov–Tanner | Generalized hydrodynamics theory by Hoffman study; considers non-zero static contact angle; proportionality constant depends on physical characteristics of liquid | [71,106,107] | |
| de Gennes | Experimental/theoretical work on liquid movement on a smooth substrate; non-zero finite static contact angle; similar to generalized “Hoffman-Voinov-Tanner” model; slip length is the length of microscopic part of the dynamic contact line in which slip condition is valid; lubrication assumption is applied in the analysis; describes the physics of dynamic contact line for flow at low capillary numbers in the mesoscopic region | [72] | |
| Cox | ; ; ; denotes the shear viscosity ratio; positive sign is for advancing dynamic contact line; and negative sign is for receding dynamic contact line | Theoretical analysis; applies slip condition to resolve stress singularity at the dynamic contact line; slip length is the length of microscopic part of the dynamic contact line in which slip condition is valid | [69,108] |
| Combined hydrodynamics–molecular kinetic | : positive sign stands for advancing dynamic contact line, and negative sign presents receding dynamic contact line : is Young’s static contact angle for stationary condition; positive sign is for receding liquid motion, and negative sign denotes advancing flow ; is the time-varying droplet radius, and denotes transient dynamic contact angle in the case of droplet spreading on a substrate | Modified hydrodynamics theory proposed by Voinov (1976) with contact line velocity-dependent static contact angle in which static contact angle is defined by molecular kinetic theory proposed by Blake et al. (1969) and Blake et al. (1993); three parameters need to be adjusted to describe the physics of dynamic contact line: λ, κ0, and Ls the combined model is only valid for slow dynamic contact line; not an appropriate model for describing the physics of dynamic contact line for high-speed coatings; a promising model for physics of dynamic contact line on real materials with variable hydrophobicity and diverse contact angle hysteresis | [68,109,110,111,112,113,153] |
| Brochard-Wyart and de Gennes | Similar to combined hydrodynamics–molecular kinetic theory; receding dynamic contact line is an irreversible process with energy dissipation caused by out-of-balance surface tension and contact line speed; the equation is obtained considering energy loss due to viscous flow near the contact line and friction force; the equation is valid for flow at low capillary numbers | [110,111] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammad Karim, A.; Suszynski, W.J. Physics of Dynamic Contact Line: Hydrodynamics Theory versus Molecular Kinetic Theory. Fluids 2022, 7, 318. https://doi.org/10.3390/fluids7100318
Mohammad Karim A, Suszynski WJ. Physics of Dynamic Contact Line: Hydrodynamics Theory versus Molecular Kinetic Theory. Fluids. 2022; 7(10):318. https://doi.org/10.3390/fluids7100318
Chicago/Turabian StyleMohammad Karim, Alireza, and Wieslaw J. Suszynski. 2022. "Physics of Dynamic Contact Line: Hydrodynamics Theory versus Molecular Kinetic Theory" Fluids 7, no. 10: 318. https://doi.org/10.3390/fluids7100318
APA StyleMohammad Karim, A., & Suszynski, W. J. (2022). Physics of Dynamic Contact Line: Hydrodynamics Theory versus Molecular Kinetic Theory. Fluids, 7(10), 318. https://doi.org/10.3390/fluids7100318







