A 3D Numerical Study of Interface Effects Influencing Viscous Gravity Currents in a Parabolic Fissure, with Implications for Modeling with 1D Nonlinear Diffusion Equations
Abstract
1. Introduction
2. Governing Equations and Boundary Conditions
2.1. 1D Flow Model
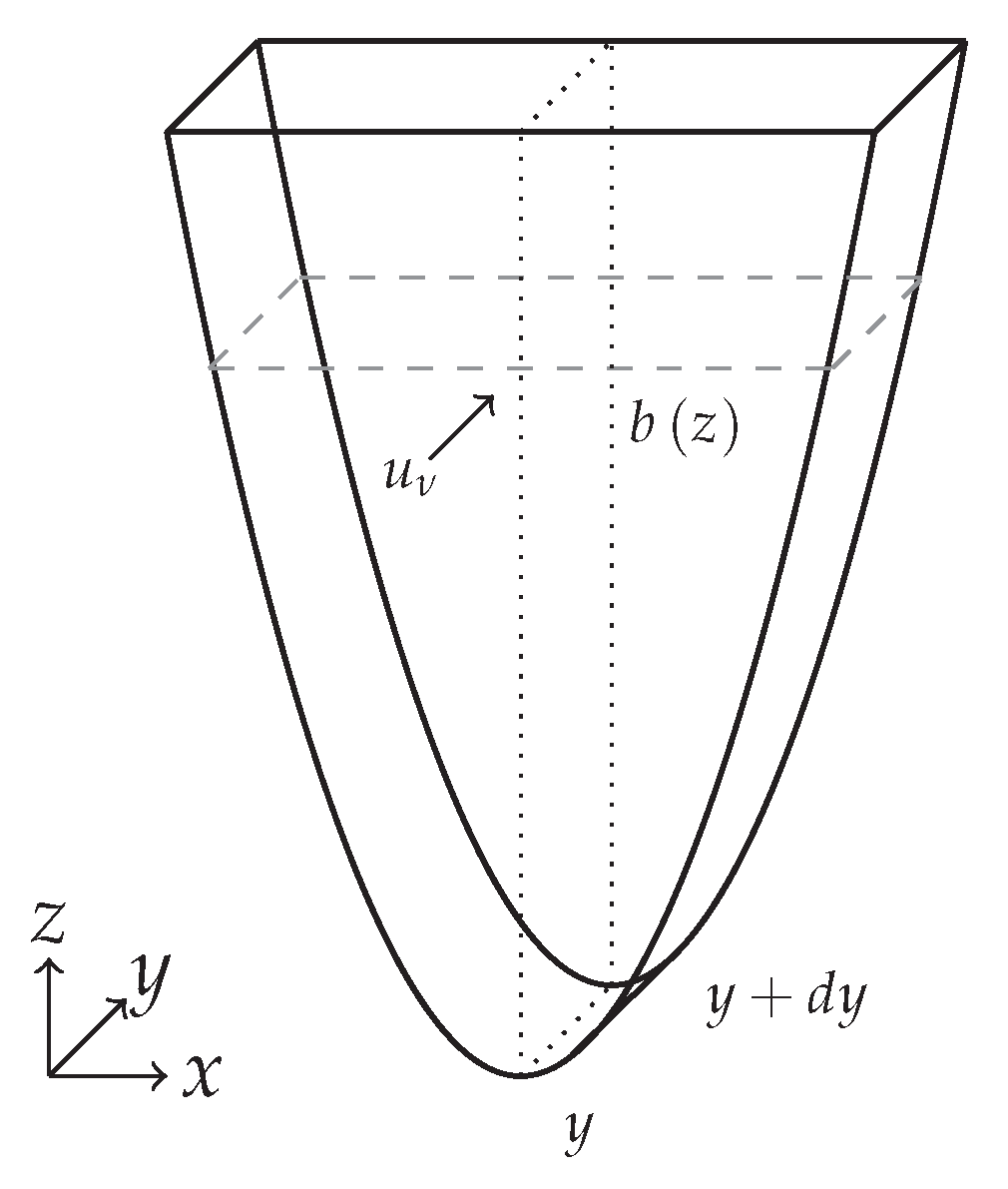
2.2. 3D Flow Model
2.3. Flow Assumptions, Initial Conditions and Parameter Values
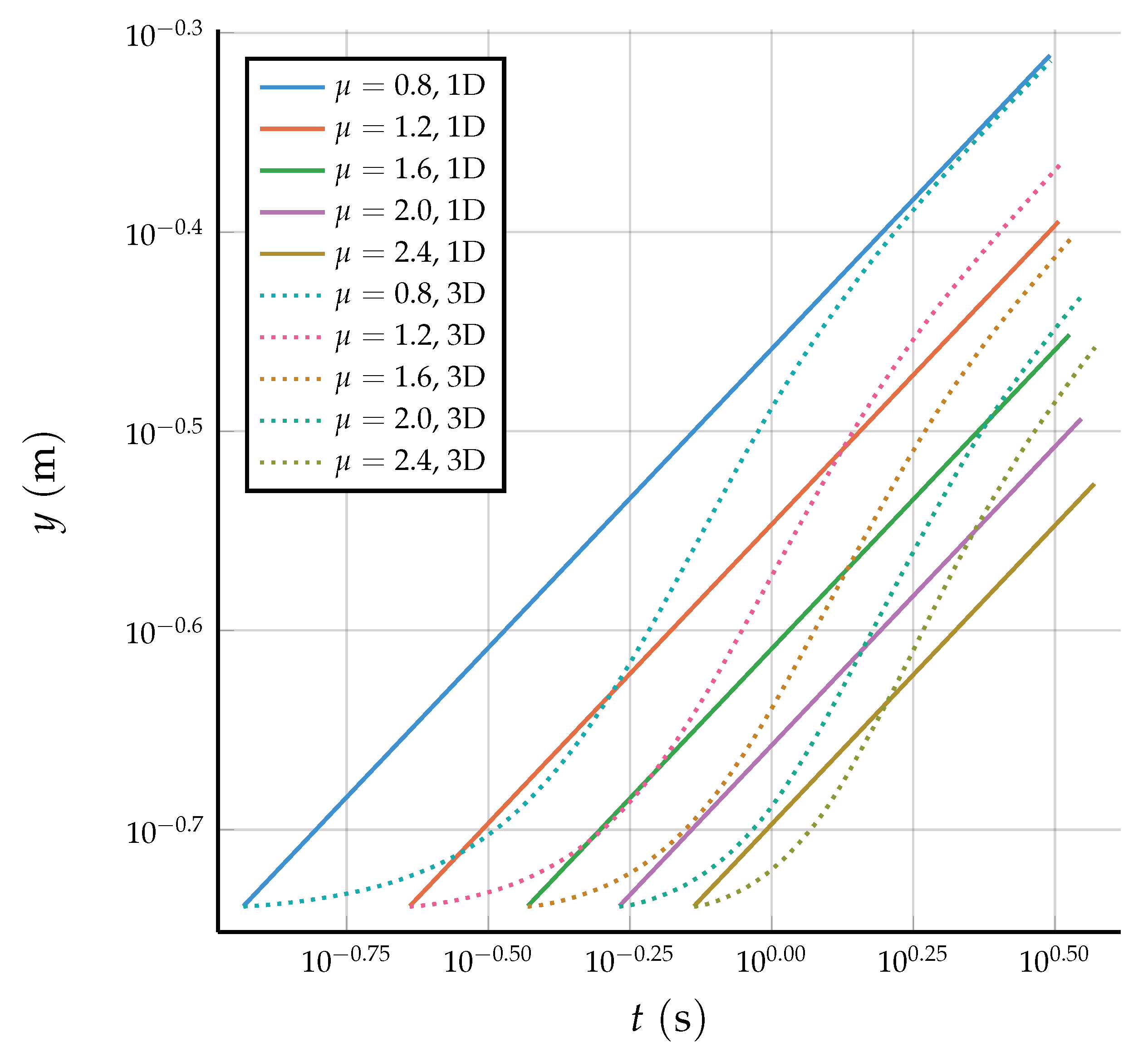
3. Results
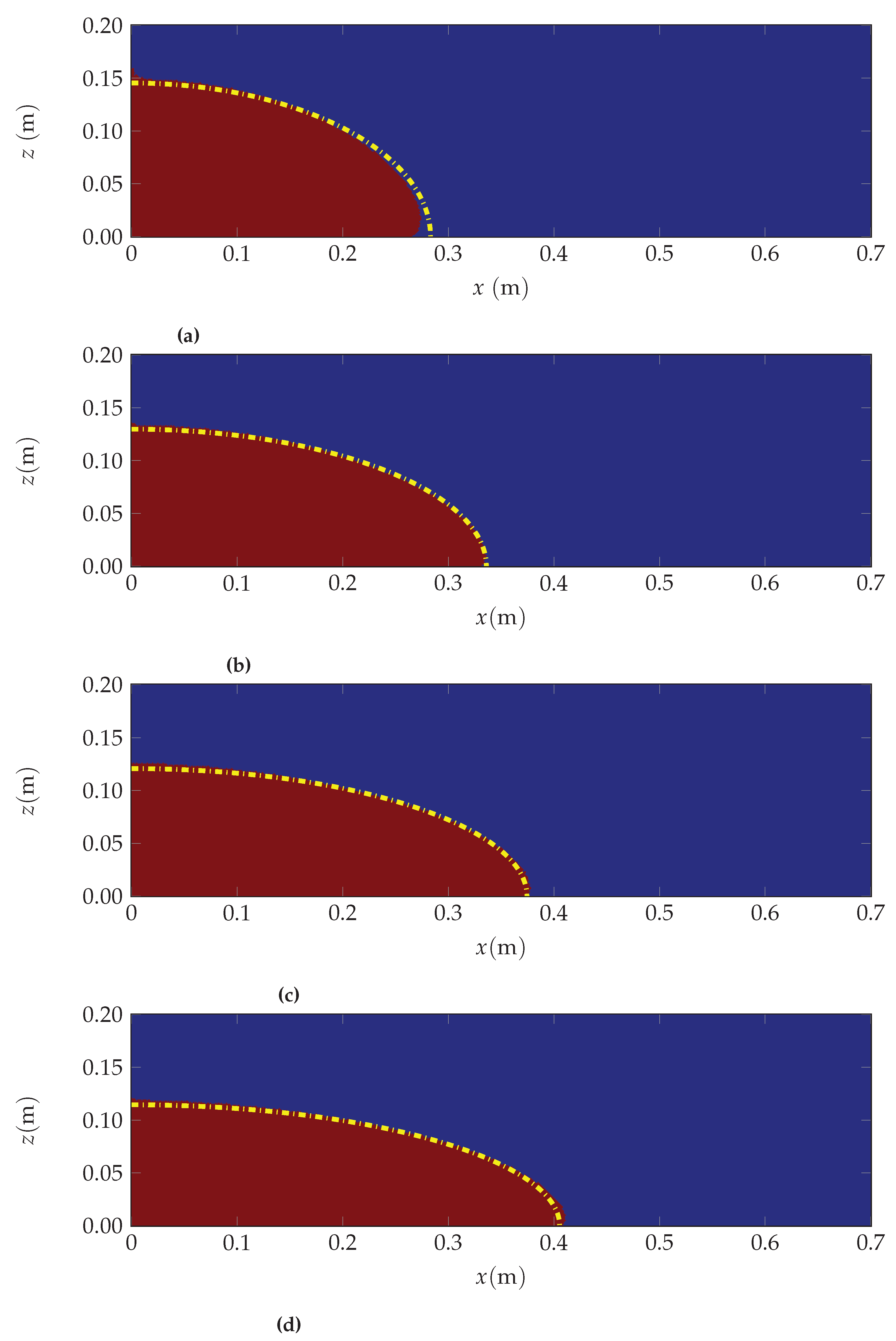
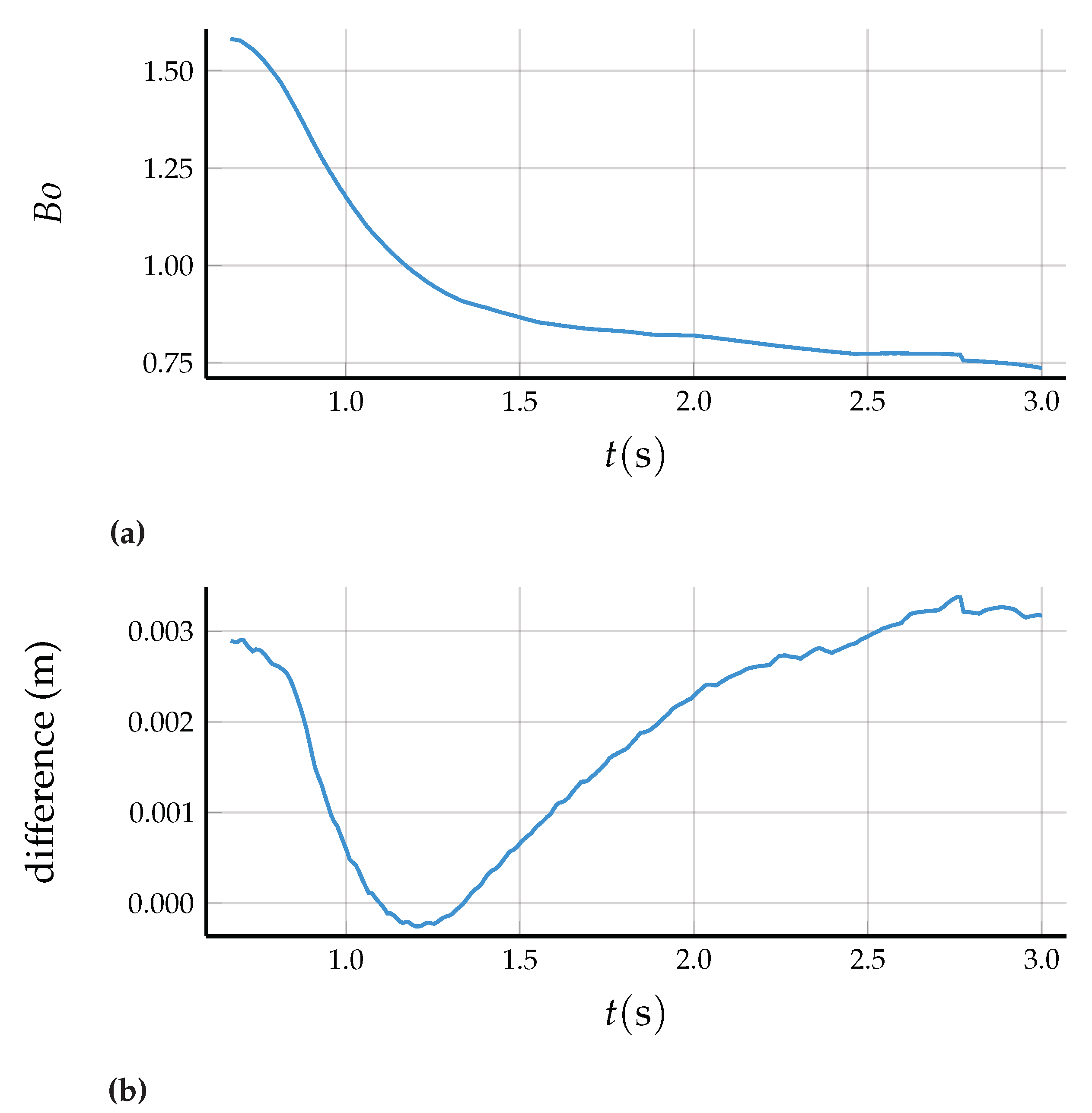
3.1. Profile Comparison and Validation
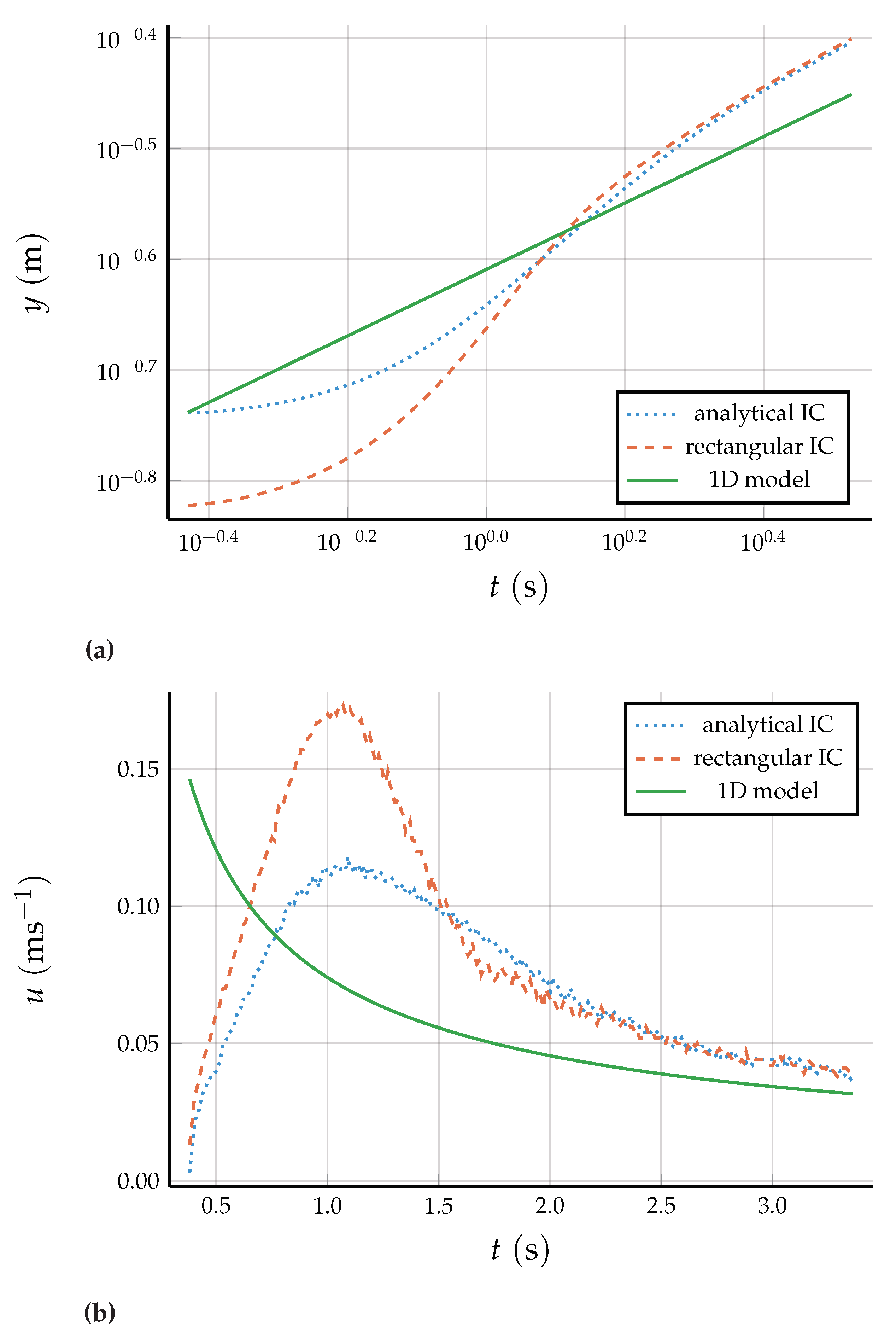
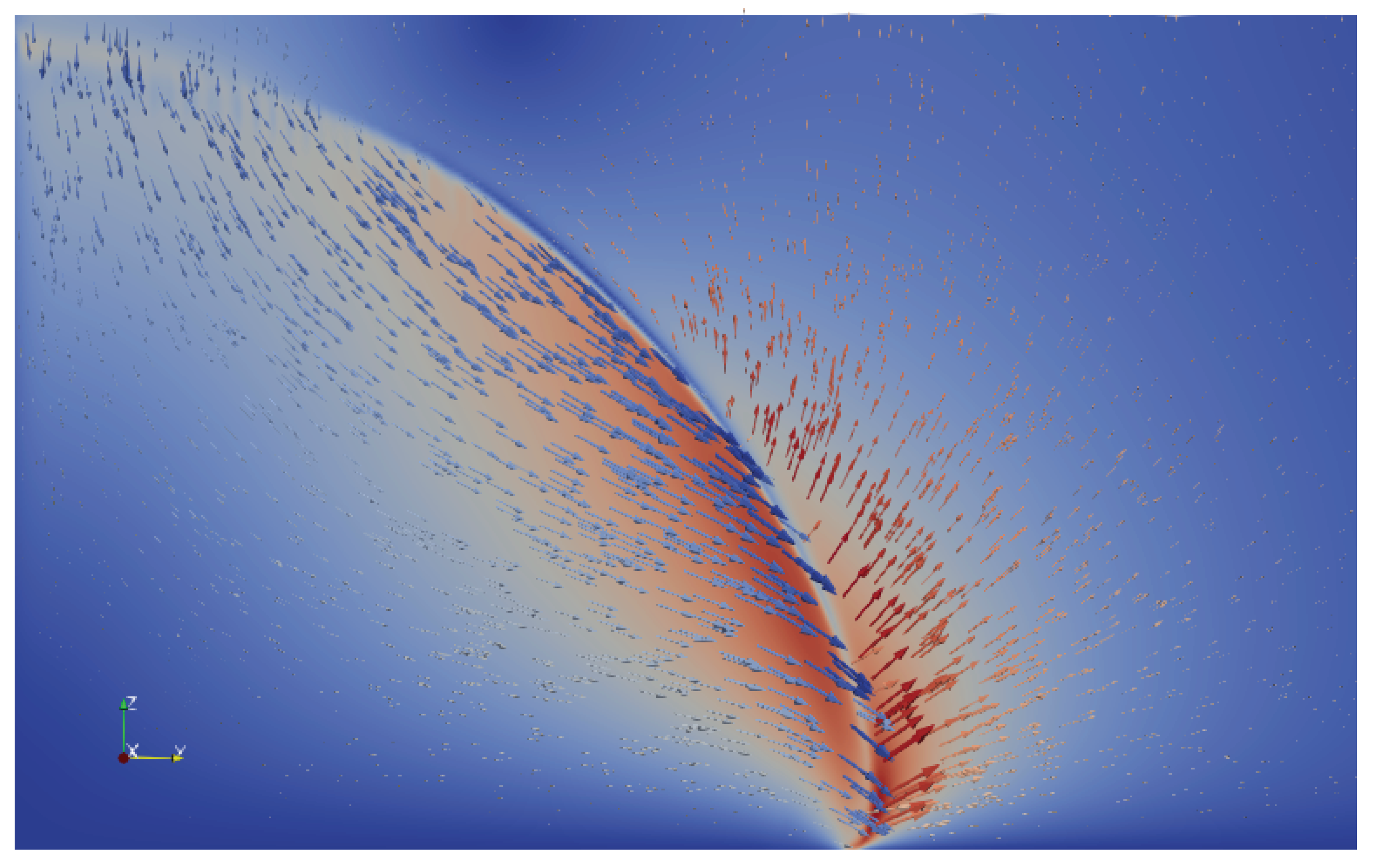
3.2. Effects of Surface Tension, Contact Angle, and Initial Conditions
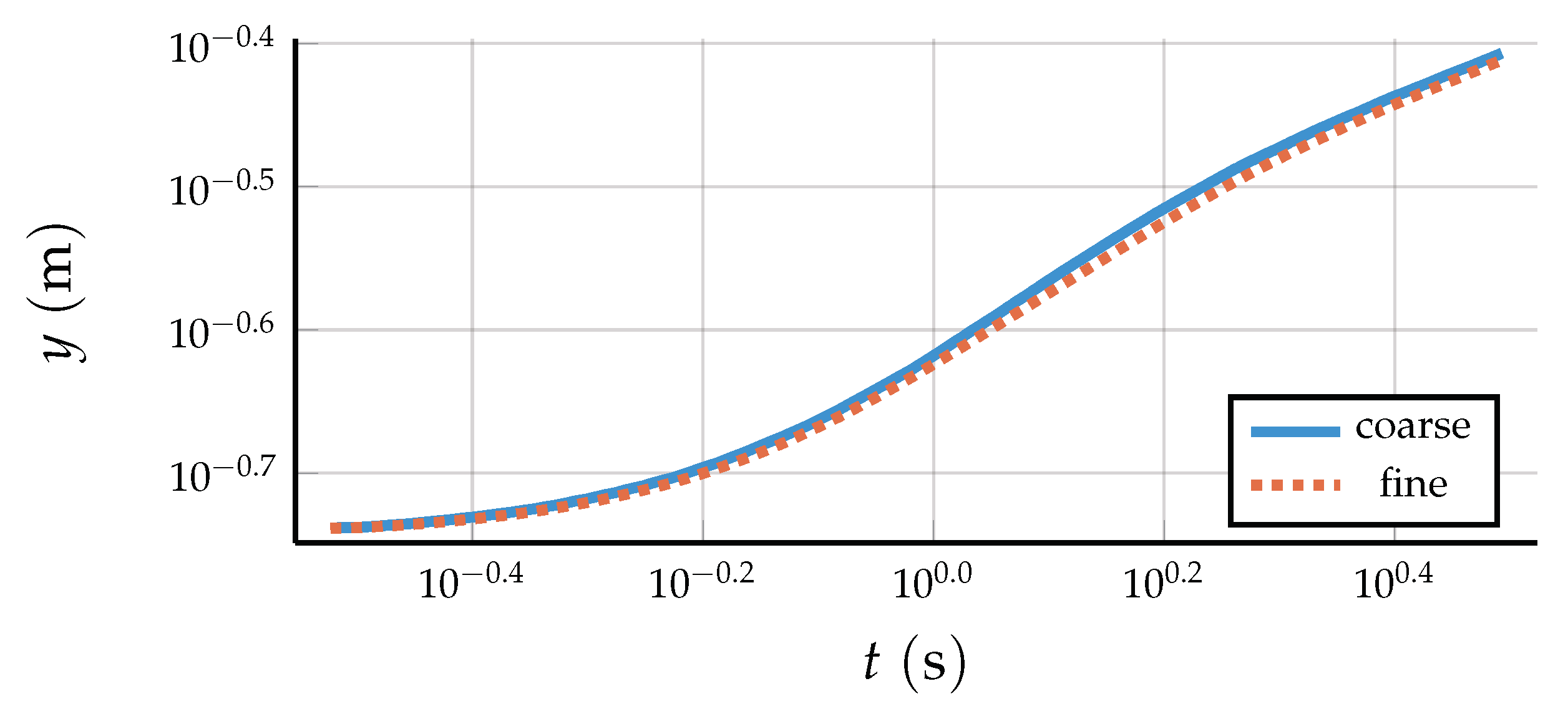
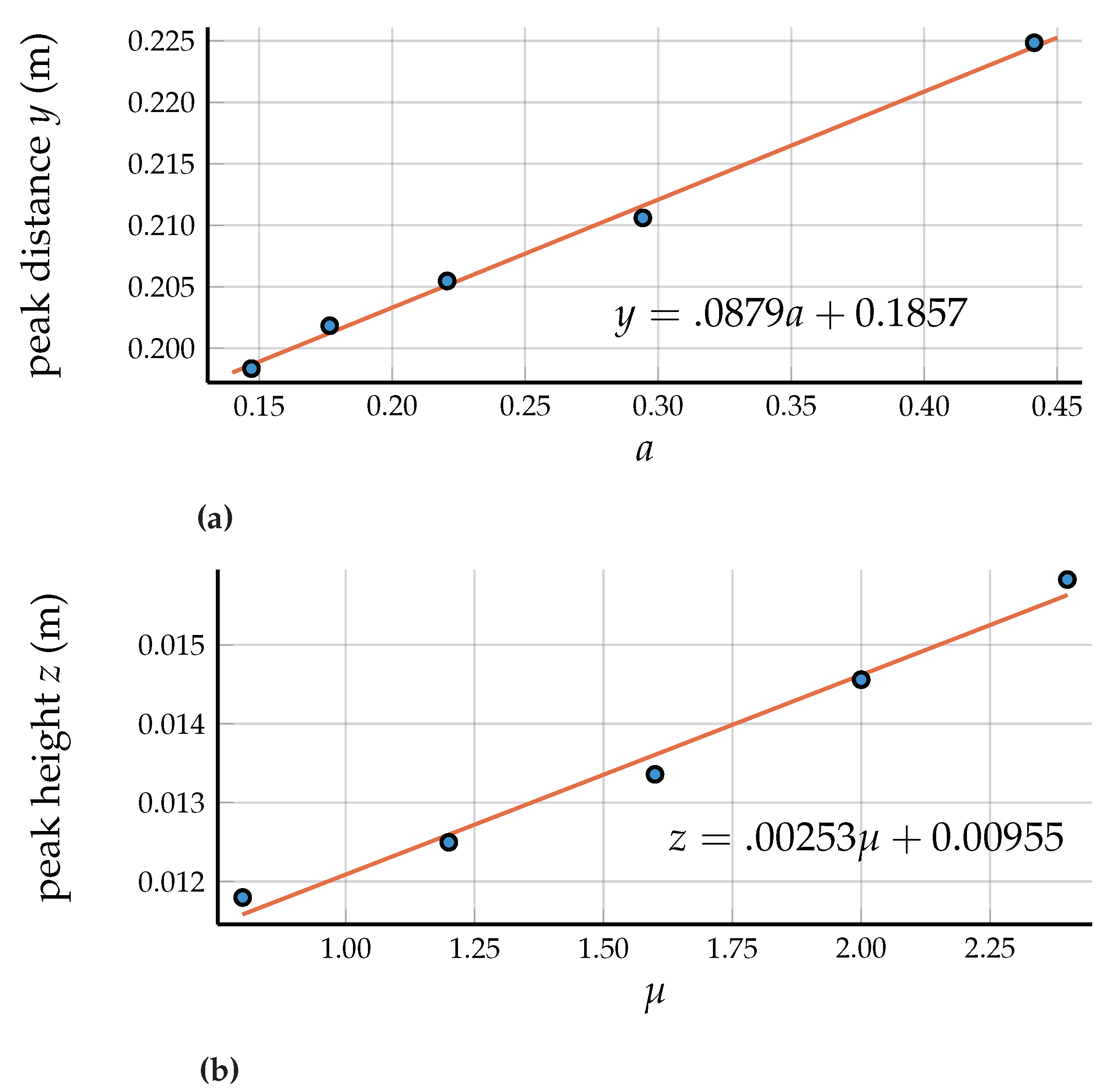
3.3. Scaling Effects
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| PME | Porous medium equation |
| DF | Dupuit-Forcheimer |
| NSE | Navier-Stokes equation |
| VOF | Volume of Fluid |
| Bo | Bond number |
References
- Huppert, H.E.; Woods, A.W. Gravity-driven flows in porous layers. J. Fluid Mech. 1995, 292, 55–69. [Google Scholar] [CrossRef]
- Huppert, H.E. Gravity currents: A personal perspective. J. Fluid Mech. 2006, 554, 299. [Google Scholar] [CrossRef]
- Blanchette, F.; Strauss, M.; Meiburg, E.; Kneller, B.; Glinsky, M.E. High-resolution numerical simulations of resuspending gravity currents: Conditions for self-sustainment. J. Geophys. Res. 2005, 110. [Google Scholar] [CrossRef]
- Simpson, J.E. Gravity Currents in the Laboratory, Atmosphere, and Ocean. Annu. Rev. Fluid Mech. 1982, 14, 213–234. [Google Scholar] [CrossRef]
- Zheng, Z.; Christov, I.; Stone, H. Influence of heterogeneity on second-kind self-similar solutions for viscous gravity currents. J. Fluid Mech. 2014, 747, 218–246. [Google Scholar] [CrossRef]
- Tsay, T.S.; Hoopes, J.A. Numerical simulation of ground water mounding and its verification by Hele–Shaw model. Comput. Geosci. 1998, 24, 979–990. [Google Scholar] [CrossRef]
- Hrebtov, M.; Hanjalić, K. Numerical Study of Winter Diurnal Convection Over the City of Krasnoyarsk: Effects of Non-freezing River, Undulating Fog and Steam Devils. Bound. Layer Meteorol. 2017, 163, 469–495. [Google Scholar] [CrossRef]
- Rupp, D.E.; Selker, J.S. Drainage of a horizontal Boussinesq aquifer with a power law hydraulic conductivity profile. Water Resour. Res. 2005, 41. [Google Scholar] [CrossRef]
- Golding, M.J.; Neufeld, J.A.; Hesse, M.A.; Huppert, H.E. Two-phase gravity currents in porous media. J. Fluid Mech. 2011, 678, 248–270. [Google Scholar] [CrossRef]
- Naaim, M.; Gurer, I. Two-phase Numerical Model of Powder Avalanche Theory and Application. Nat. Hazards 1998, 17, 129–145. [Google Scholar] [CrossRef]
- Barenblatt, G.I. Scaling; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Polubarinova-Kochina, P.Y. Theory of Groundwater Movement; Princeton University Press: Princeton, NJ, USA, 1962. [Google Scholar]
- Longo, S.; Di Federico, V. Axisymmetric gravity currents within porous media: First order solution and experimental validation. J. Hydrol. 2014, 519, 238–247. [Google Scholar] [CrossRef]
- McWhorter, D.B.; Sunada, D.K. Ground-Water Hydrology and Hydraulics; Water Resources Publications: Highlands Ranch, CO, USA, 1977. [Google Scholar]
- Vazquez, J.L. The Porous Medium Equation, Mathematical Theory; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Lockington, D.A.; Parlange, J.Y.; Parlange, M.B.; Selker, J. Similarity solution of the Boussinesq equation. Adv. Water Resour. 2000, 23, 725–729. [Google Scholar] [CrossRef][Green Version]
- Telyakovskiy, A.S.; Braga, G.A.; Kurita, S.; Mortensen, J. On a power series solution to the Boussinesq equation. Adv. Water Resour. 2010, 33, 1128–1129. [Google Scholar] [CrossRef]
- Hayek, M. An exact solution for a nonlinear diffusion equation in a radially symmetric inhomogeneous medium. Comput. Math. Appl. 2014, 68, 1751–1757. [Google Scholar] [CrossRef]
- Barenblatt, G.I. On Some Unsteady-State Movements of Liquid and Gas in Porous Medium. Prikl. Mat. Mekh. 1952, 16, 67–78. (In Russian) [Google Scholar]
- Barenblatt, G.I. On some problems of unsteady filtration. Izv. AN SSSR 1954, 6, 97–110. (In Russian) [Google Scholar]
- Olsen, J.S.; Telyakovskiy, A.S. Polynomial approximate solutions of a generalized Boussinesq equation. Water Resour. Res. 2013, 49, 3049–3053. [Google Scholar] [CrossRef]
- Ciriello, V.; Longo, S.; Chiapponi, L.; Di Federico, V. Porous gravity currents: A survey to determine the joint influence of fluid rheology and variations of medium properties. Adv. Water Resour. 2016, 92, 105–115. [Google Scholar] [CrossRef]
- Helmig, R. Multiphase Flow and Transport Processes in the Subsurface: A Contribution to the Modeling of Hydrosystems; Springer: Berlin/Heidelberg, Germany, 1997. [Google Scholar]
- Zheng, Z.; Soh, B.; Huppert, H.E.; Stone, H.A. Fluid drainage from the edge of a porous reservoir. J. Fluid Mech. 2013, 718, 558–568. [Google Scholar] [CrossRef]
- Longo, S.; Ciriello, V.; Chiapponi, L.; Di Federico, V. Combined effect of rheology and confining boundaries on spreading of gravity currents in porous media. Adv. Water Resour. 2015, 79, 140–152. [Google Scholar] [CrossRef]
- Frolkovič, P. Application of level set method for groundwater flow with moving boundary. Adv. Water Resour. 2012, 47, 56–66. [Google Scholar] [CrossRef]
- Chesnokov, A.; Liapidevskii, V. Viscosity-stratified flow in a Hele–Shaw cell. Int. J. Non Linear Mech. 2017, 89, 168–176. [Google Scholar] [CrossRef][Green Version]
- Bernal, F.; Kindelan, M. RBF meshless modeling of non-Newtonian Hele–Shaw flow. Eng. Anal. Bound. Elem. 2007, 31, 863–874. [Google Scholar] [CrossRef]
- Furtak-Cole, E.; Telyakovskiy, A.S.; Cooper, C.A. A series solution for horizontal infiltration in an initially dry aquifer. Adv. Water Resour. 2018, 116, 145–152. [Google Scholar] [CrossRef]
- Shampine, L.F. Some Singular Concentration Dependent Diffusion Problems. J. Appl. Math. Mech. 1973, 53, 421–422. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Darwish, M.; Moukalled, F. Convective Schemes for Capturing Interfaces of Free-Surface Flows on Unstructured Grids. Numer. Heat Transf. B Fund 2006, 49, 19–42. [Google Scholar] [CrossRef]
- Deshpande, S.S.; Anumolu, L.; Trujillo, M.F. Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Discov. 2012, 5, 014016. [Google Scholar] [CrossRef]
- Yin, X.; Zarikos, I.; Karadimitriou, N.; Raoof, A.; Hassanizadeh, S. Direct simulations of two-phase flow experiments of different geometry complexities using Volume-of-Fluid (VOF) method. Chem. Eng. Sci. 2019, 195, 820–827. [Google Scholar] [CrossRef]
- Issakhov, A.; Imanberdiyeva, M. Numerical simulation of the movement of water surface of dam break flow by VOF methods for various obstacles. Int. J. Heat Mass Transf. 2019, 136, 1030–1051. [Google Scholar] [CrossRef]
- Duguay, J.; Lacey, R.; Gaucher, J. A case study of a pool and weir fishway modeled with OpenFOAM and FLOW-3D. Ecol. Eng. 2017, 103, 31–42. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620. [Google Scholar] [CrossRef]
- Hesse, M.; Tchelepi, H.; Cantwel, B.J.; Orr, F.M., Jr. Gravity currents in horizontal porous layers: Transition from early to late self-similarity. J. Fluid Mech. 2007, 577, 363–383. [Google Scholar] [CrossRef]


| 1D Parameter | Value | 3D Parameter | Value |
|---|---|---|---|
| - | - | ||
| 1261 kg·m−3 | 1261 kg·m−3 | ||
| 0.88–2.4 Pa·s | 0.8–2.4 Pa·s | ||
| g | 9.8 m·s−2 | g | 9.8 m·s−2 |
| 2000 | - | - | |
| - | - | 1 kg·m−3 | |
| - | - | 1.48 × 10−5 Pa·s | |
| - | - | 0.0634 N·m−1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Furtak-Cole, E.; Telyakovskiy, A.S. A 3D Numerical Study of Interface Effects Influencing Viscous Gravity Currents in a Parabolic Fissure, with Implications for Modeling with 1D Nonlinear Diffusion Equations. Fluids 2019, 4, 97. https://doi.org/10.3390/fluids4020097
Furtak-Cole E, Telyakovskiy AS. A 3D Numerical Study of Interface Effects Influencing Viscous Gravity Currents in a Parabolic Fissure, with Implications for Modeling with 1D Nonlinear Diffusion Equations. Fluids. 2019; 4(2):97. https://doi.org/10.3390/fluids4020097
Chicago/Turabian StyleFurtak-Cole, Eden, and Aleksey S. Telyakovskiy. 2019. "A 3D Numerical Study of Interface Effects Influencing Viscous Gravity Currents in a Parabolic Fissure, with Implications for Modeling with 1D Nonlinear Diffusion Equations" Fluids 4, no. 2: 97. https://doi.org/10.3390/fluids4020097
APA StyleFurtak-Cole, E., & Telyakovskiy, A. S. (2019). A 3D Numerical Study of Interface Effects Influencing Viscous Gravity Currents in a Parabolic Fissure, with Implications for Modeling with 1D Nonlinear Diffusion Equations. Fluids, 4(2), 97. https://doi.org/10.3390/fluids4020097





