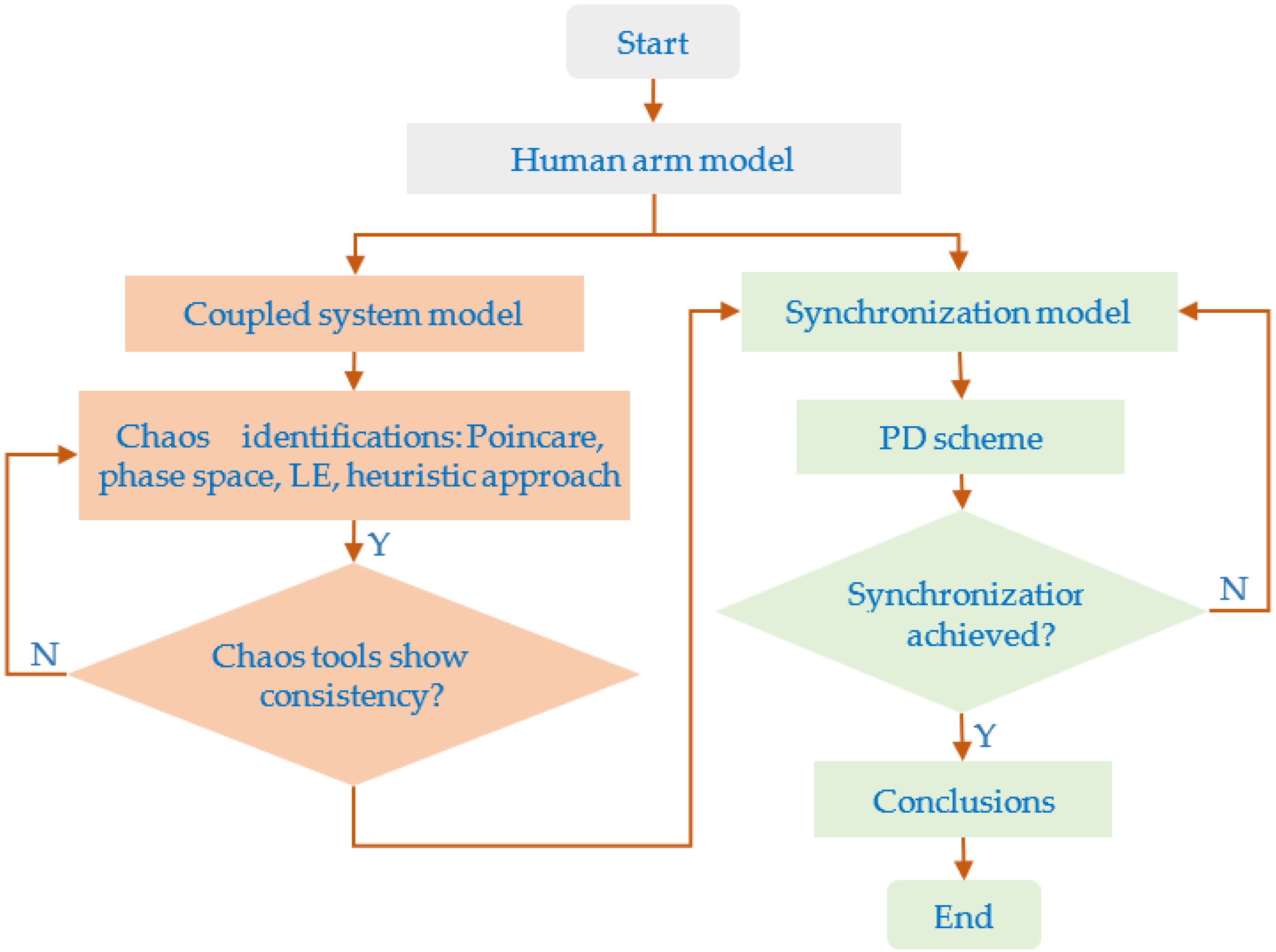
Coupled and Synchronization Models of Rhythmic Arm Movement in Planar Plane
Abstract
1. Introduction
2. System Model
2.1. Mathematical Modeling to Observe Chaotic Behavior of Repetitive Arm Movement
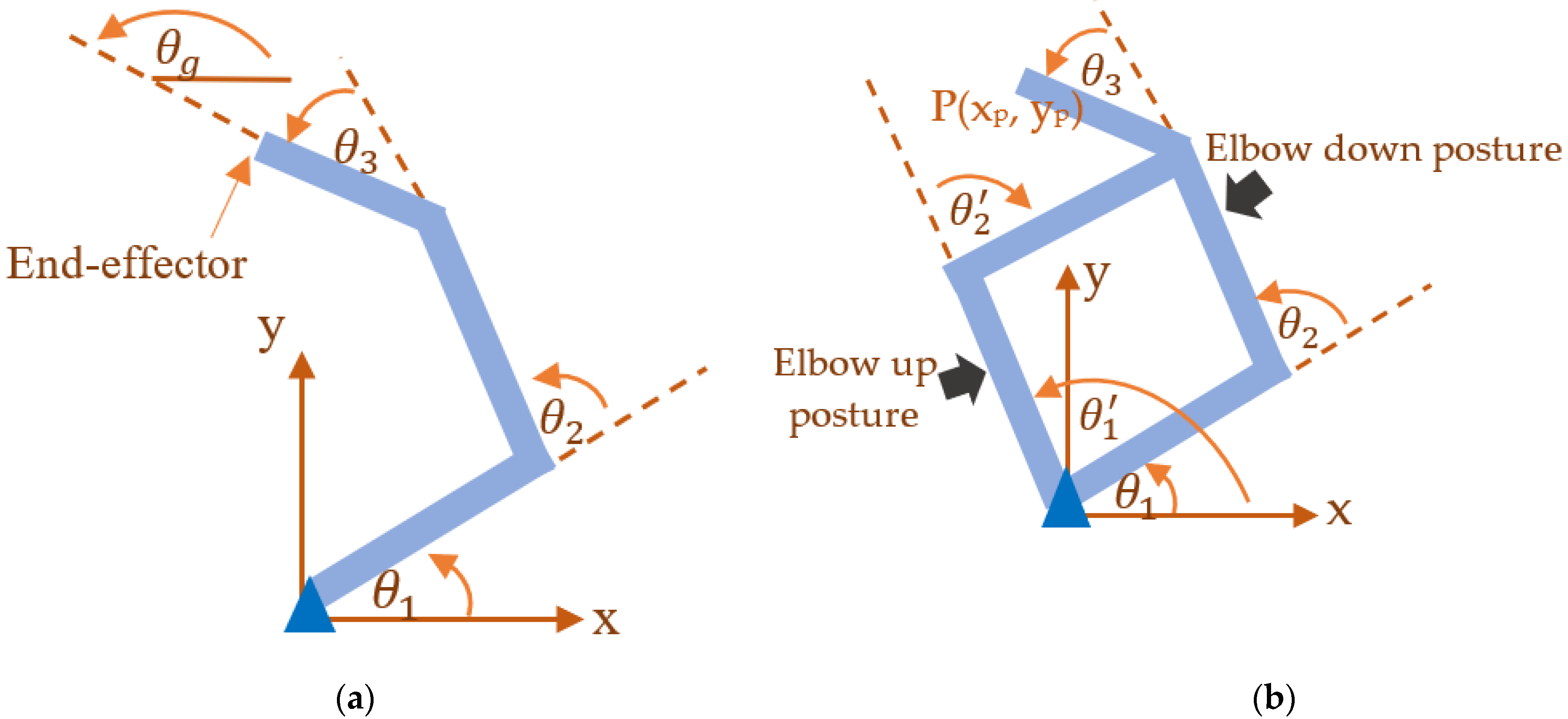
2.2. 2nd ODE of Kinematics Planar Arm Model
2.3. Inverse Kinematics (IK) Solution
2.4. Second Joint Angle Velocity
2.5. Domain of the Orientation Angle
2.6. General Solutions of ODE
3. Coupled Systems
3.1. Scheme 1
3.2. Scheme 2
4. Synchronization of Planar Human Arm System with PD-Scheme
Modeling the θg Trajectories as the DVP Oscillator
5. Results and Discussions
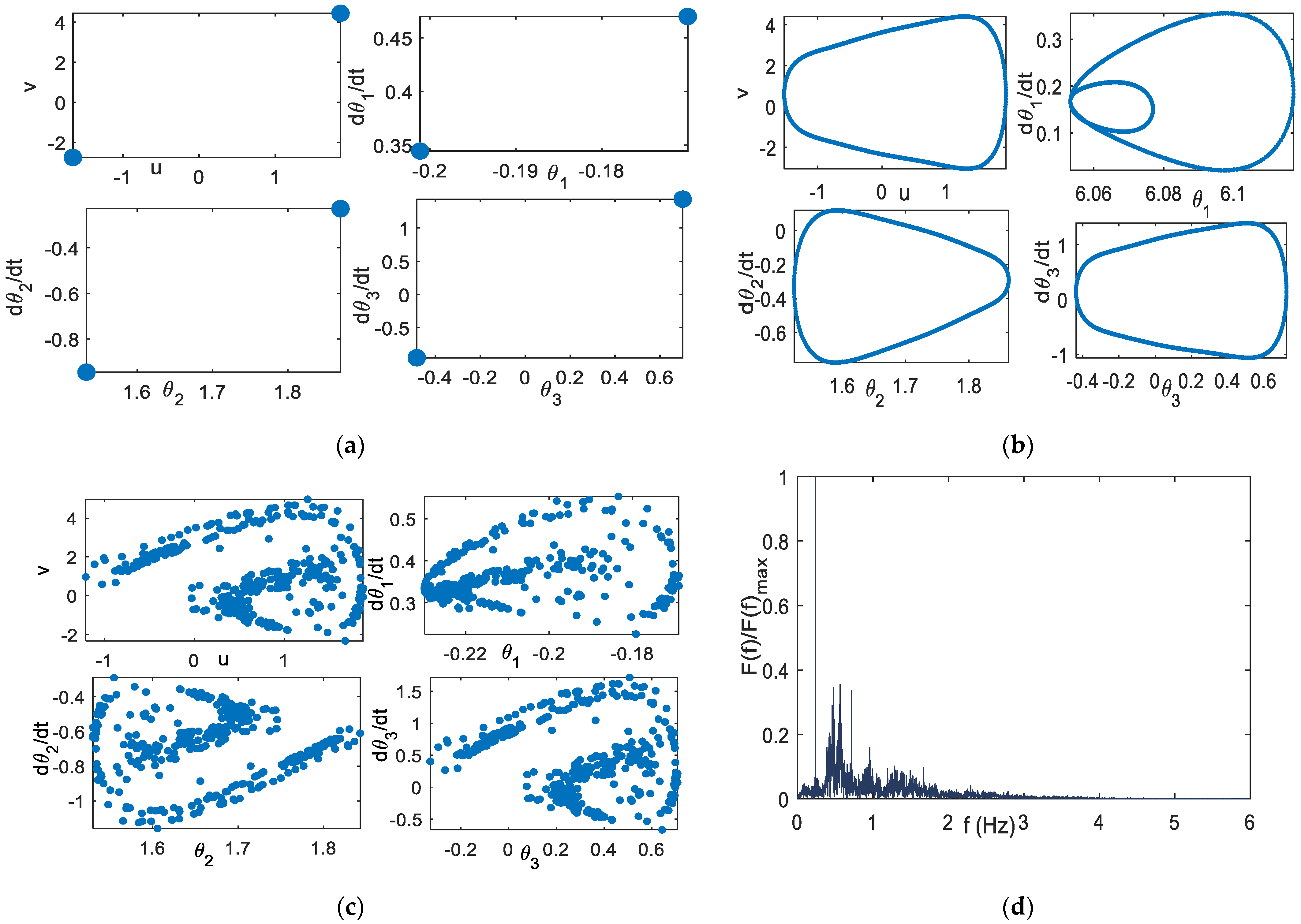
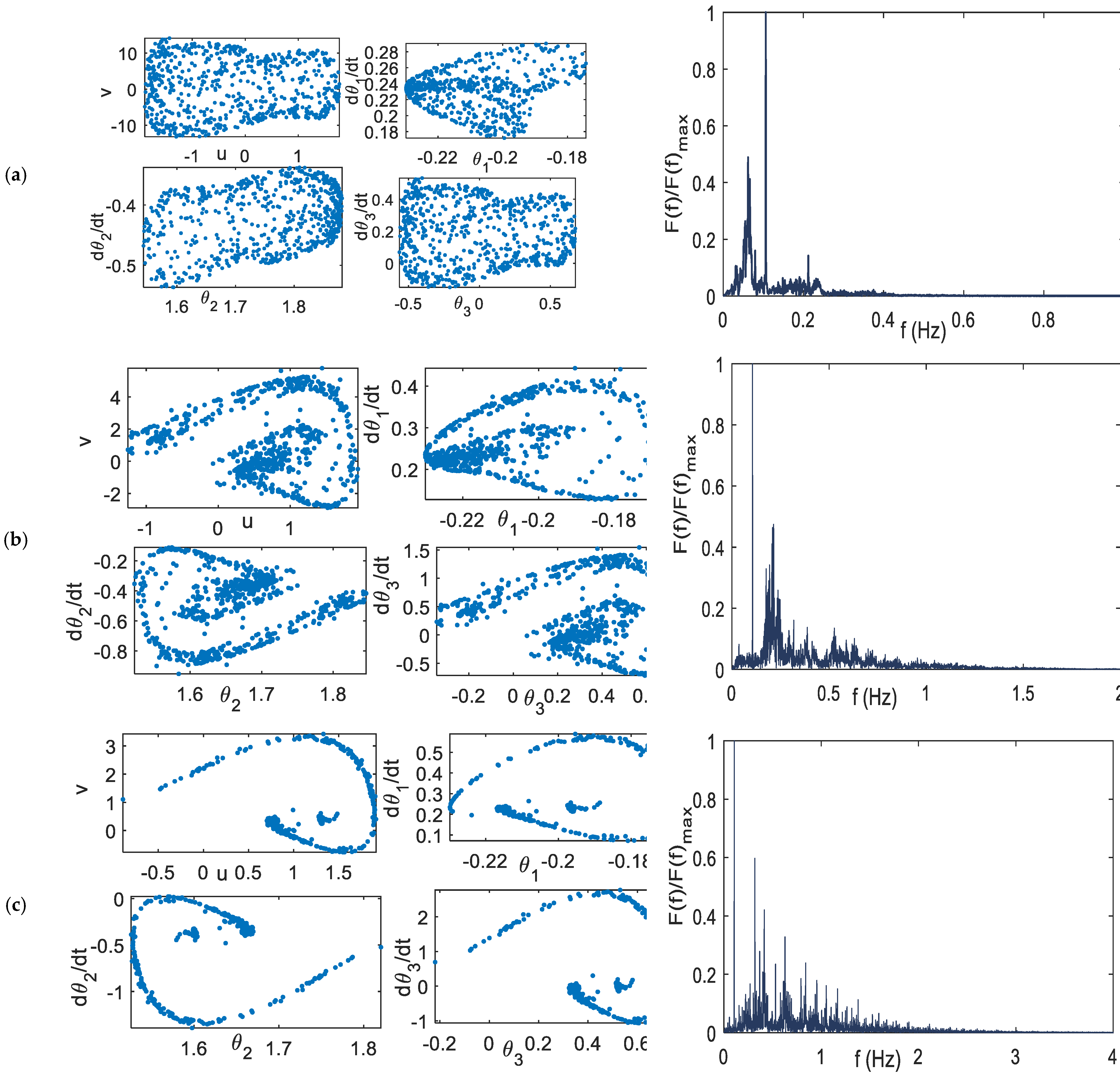
5.1. Scheme-1 of Coupled System Model
5.2. Scheme-2 of Coupled System Model
k Range Which Exhibits the Chaotic Behavior
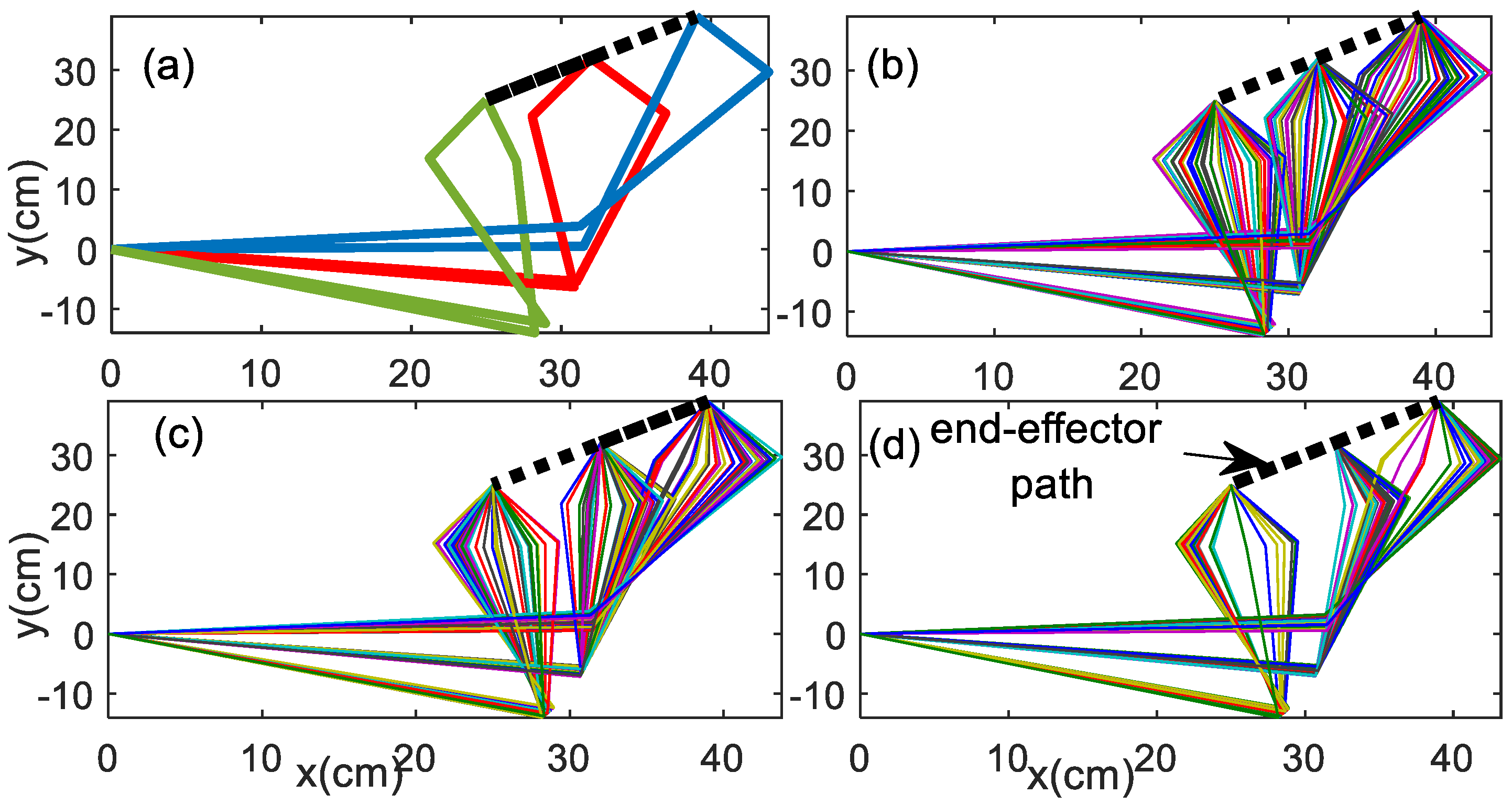
5.3. Sensitivity to Initial Conditions
5.4. Phase Space
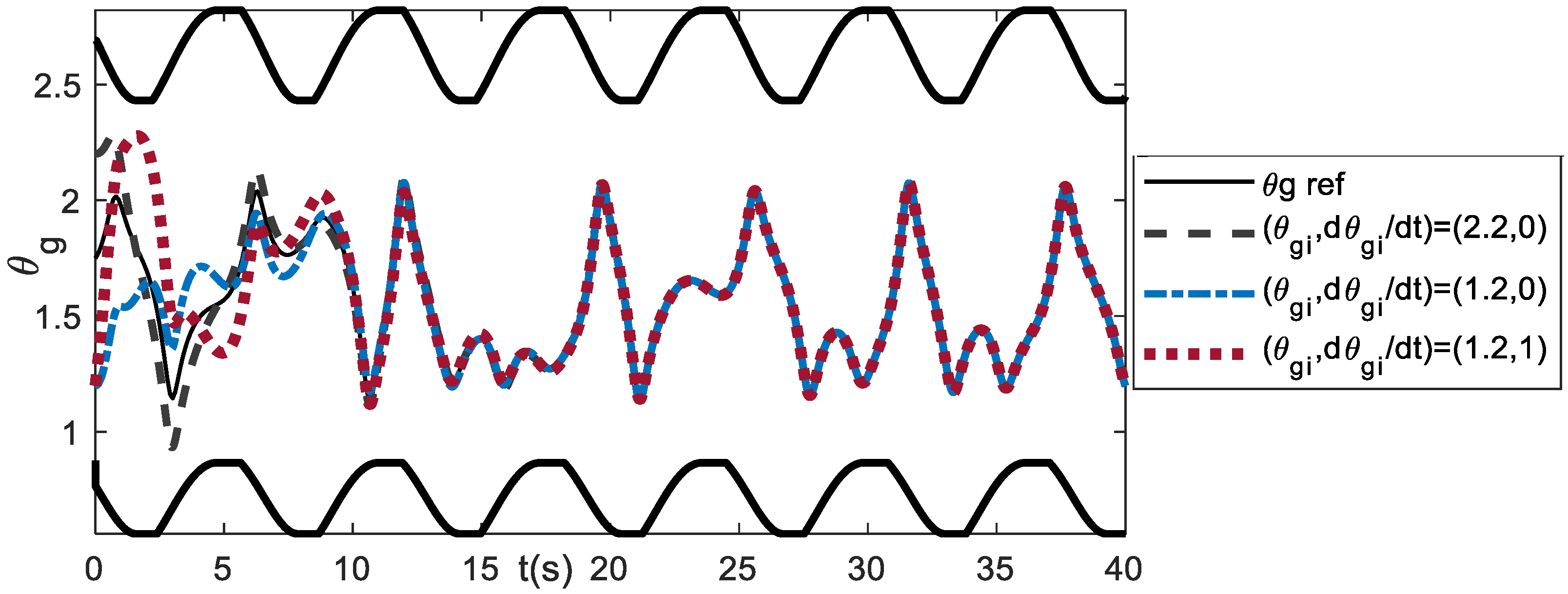
5.5. System Response of Synchronization Model
5.6. Discussions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Constant number | |
| Ap | Amplitude of |
| Integer value | |
| Constant number | |
| Integer value | |
| Cosine of second joint angle | |
| d | Coupling parameter |
| Domain or the operational area of the ith joint angle | |
| ei | Error |
| e1 | Position error |
| e2 | Velocity error |
| Fi | Real parameter of extended DVP oscillator |
| Parametric function | |
| Parametric function | |
| h | Scale factor in synchronization approach |
| J | Jacobian |
| k | Coupling parameter |
| Kp | Proportional gain |
| kp | Vertical shift of |
| Kv | Derivative gain |
| li | ith length of the open kinematic chain |
| M | Controller output |
| Real number | |
| r | Radius |
| Sine of second joint angle | |
| t | Time |
| T~ | Period time |
| U(t) | External force of single well DVP oscillator |
| State variable of coupled system | |
| Displacement of extended DVP oscillator | |
| Initial position of extended DVP oscillator | |
| Initial velocity of extended DVP oscillator | |
| Drive trajectories of synchronization approach | |
| (x, y) | Actual end-effector position |
| (xc, yc) | Curve center |
| (xe, ye) | Target end-effector position |
| α | Real parameter of extended DVP oscillator |
| Constant parameter of single well DVP oscillator | |
| γ | Real parameter of extended DVP oscillator |
| Positive real number | |
| Orientation angle | |
| Joint angle of ith link | |
| Minimum joint angles of ith link | |
| Maximum joint angles of ith link | |
| Initial orientation angle | |
| Constant value of PD synchronization | |
| First derivative of | |
| Initial orientation angle velocity | |
| Second derivative of | |
| μ | Real parameter of extended DVP oscillator |
| Constant parameter of single well DVP oscillator | |
| φp | Phase shift of |
| Angle of curve | |
| State variables of inverse kinematics | |
| Ω | Frequency of curve |
| Real parameter of extended DVP oscillator | |
| Constant parameter of single well DVP oscillator | |
| Real parameter of extended DVP oscillator | |
| Boundary of orientation angle |
Appendix A
Appendix B
References
- Glass, L. Synchronization and rhythmic processes in physiology. Nature 2001, 410, 277–284. [Google Scholar] [CrossRef] [PubMed]
- Bernstein, N.A. The Coordination and Regulation of Movements; Pergamon Press: Oxford, UK, 1967. [Google Scholar]
- Sporns, O.; Edelman, G.M. Solving Bernstein’s problem: A proposal for the development of coordinated movement by selection. Child Dev. 1993, 64, 960–981. [Google Scholar] [CrossRef] [PubMed]
- Bongaardt, R.; Meijer, O.G. Bernstein’s theory of movement behavior: Historical development and contemporary relevance. J. Mot. Behav. 2000, 32, 57–71. [Google Scholar] [CrossRef] [PubMed]
- Loeb, G.E. Optimal isn’t good enough. Biol. Cybern. 2012, 106, 757–765. [Google Scholar] [CrossRef]
- Davids, K.; Glazier, P.; Araujo, D.; Bartlett, R. Movement systems as dynamical systems: The functional role of variability and its implications for sports medicine. Sports Med. 2003, 33, 245–260. [Google Scholar] [CrossRef]
- Caballero, C.; Barbado, D.; Moreno, F.J. Non-linear tools and methodological concerns measuring human movement variability: An overview. Eur. J. Hum. Mov. 2014, 32, 61–81. [Google Scholar]
- Stergiou, N.; Decker, L.M. Human movement variability, nonlinear dynamics, and pathology: Is there a connection? Phys. Ther. 2011, 30, 869–888. [Google Scholar] [CrossRef]
- Miyoshi, T.; Murata, A. Chaotic characteristic in human hand movement. In Proceedings of the IEEE International Workshop on Robot and Human Interactive Communication, Osaka, Japan, 27–29 September 2000. [Google Scholar]
- Mitra, S.; Riley, M.A.; Turvey, M.T. Chaos in human rhythmic movement. J. Mot. Behav. 1997, 29, 195–198. [Google Scholar] [CrossRef]
- Lipsitz, L.A.; Goldberger, A.L. Loss of ‘complexity’ and aging, potential applications of fractals and chaos theory to senescence. J. Am. Med. Assoc. 1992, 267, 1806–1809. [Google Scholar] [CrossRef]
- Stergiou, N.; Harbourne, R.; Cavanaugh, J. Optimal movement variability: A new theoretical perspective for neurologic physical therapy. J. Neurol. Phys. Ther. 2006, 30, 120–129. [Google Scholar] [CrossRef]
- Harbourne, R.T.; Stergiou, N. Perspective on movement variability and the use of nonlinear tools: Principles to guide physical therapy practice. Phys. Ther. 2009, 89, 267–282. [Google Scholar] [CrossRef] [PubMed]
- Cusumano, J.P.; Dingwell, J.B. Movement variability near goal equivalent manifolds: Fluctuations, control, and model-based analysis. Hum. Mov. Sci. 2013, 32, 899–923. [Google Scholar] [CrossRef]
- Otero-Siliceo, E.; Arriada-Mendicoa, N. Is it healthy to be chaotic? Med. Hypotheses 2003, 60, 233–236. [Google Scholar] [CrossRef]
- Deffeyes, J.E. Nonlinear Dynamics of Infant Sitting Postural Control. Ph.D. Thesis, University of Nebraska, Lincoln, NE, USA, 2009. [Google Scholar]
- Micklem, C.N.; Locke, J.C. Cut the noise or couple up: Coordinating circadian and synthetic clocks. Iscience 2021, 24, 103051. [Google Scholar] [CrossRef] [PubMed]
- Brooks, J.; Crone, J.C.; Spangler, D.P. A physiological and dynamical systems model of stress. Int. J. Psychophysiol. 2021, 166, 83–91. [Google Scholar] [CrossRef]
- Daugherty, D.; Roque-Urrea, T.; Urrea-Roque, J.; Troyer, J.; Wirkus, S.; Porter, M.A. Mathematical models of bipolar disorder. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 2897–2908. [Google Scholar] [CrossRef][Green Version]
- Low, L.A.; Reinhall, P.G.; Storti, D.W.; Goldman, E.B. Coupled van der Pol oscillators as a simplified model for generation of neural patterns for jellyfish locomotion. Struct. Control Health Monit. 2006, 13, 417–429. [Google Scholar] [CrossRef]
- Scafetta, N.; Marchi, D.; West, B.J. Understanding the complexity of human gait dynamics. Chaos 2009, 19, 026108. [Google Scholar] [CrossRef] [PubMed]
- Collins, J.J.; Stewart, I.N. Coupled nonlinear oscillators and the symmetries of animal gaits. J. Nonlinear Sci. 1993, 3, 349–392. [Google Scholar] [CrossRef]
- Beek, P.J.; Peper, C.E.; Daffertshofer, A. Modeling rhythmic interlimb coordination: Beyond the Haken-Kelso-Bunz model. Brain Cogn. 2002, 48, 149–165. [Google Scholar] [CrossRef]
- Jing, Z.; Yang, Z.; Jiang, T. Complex dynamics in Duffing-Van der Pol equation. Chaos Solitons Fractals 2006, 27, 722–747. [Google Scholar] [CrossRef]
- Kakmeni, F.M.M.; Bowong, S.; Tchawoua, C.; Kaptouom, E. Chaos control and synchronization of a Φ6-Van der Pol oscillator. Phys. Lett. A 2004, 322, 305–323. [Google Scholar] [CrossRef]
- Yang, C.C. Robust synchronization and anti-synchronization of identical Φ6 oscillators via adaptive sliding mode control. J. Sound Vib. 2012, 331, 501–509. [Google Scholar] [CrossRef]
- Pecora, L.M.; Carroll, T.L. Synchronization in chaotic systems. Phys. Rev. Lett. 1990, 64, 821. [Google Scholar] [CrossRef]
- Idowu, B.A.; Vincent, U.E.; Njah, A.N. Synchronization of chaos in nonidentical parametrically excited systems. Chaos Solitons Fractals 2009, 39, 2322–2331. [Google Scholar] [CrossRef]
- Pecora, L.M.; Carroll, T.L. Synchronization of chaotic systems. Chaos 2015, 25, 97611. [Google Scholar] [CrossRef]
- Lee, B.; Bang, H. A mouse with two optical sensors that eliminates coordinate disturbance during skilled strokes. Hum.-Comput. Interact. 2015, 30, 122–155. [Google Scholar] [CrossRef]
- Ghosal, A. Resolution of redundancy in robots and in a human arm. Mech. Mach. Theory 2018, 125, 126–136. [Google Scholar] [CrossRef]
- Dasgupta, B.; Mruthyunjaya, T.S. Force redundancy in parallel manipulators: Theoretical and practical issues. Mech. Mach. Theory 1998, 33, 727–742. [Google Scholar] [CrossRef]
- Machmudah, A.; Parman, S.; Abbasi, A.; Solihin, M.I.; Abd Manan, T.S.; Beddu, S.; Ahmad, A.; Rasdi, N.W. Cyclic path planning of hyper-redundant manipulator using whale optimization algorithm. Int. J. Adv. Comput. Sci. Appl. 2021, 12, 677–686. [Google Scholar] [CrossRef]
- Liu, J.; Dietz, T.; Carpenter, S.R.; Taylor, W.W.; Alberti, M.; Deadman, P.; Redman, C.; Pell, A.; Folke, C.; Ouyang, Z.; et al. Coupled human and natural systems: The evolution and applications of an integrated framework. Ambio 2021, 50, 1778–1783. [Google Scholar] [CrossRef]
- Sarkar, P.; Debnath, N.; Reang, D. Coupled human-environment system amid COVID-19 crisis: A conceptual model to understand the nexus. Sci. Total Environ. 2021, 753, 141757. [Google Scholar] [CrossRef] [PubMed]
- Grecu, V.; Dumitru, N.; Grecu, L. Analysis of human arm joints and extension of the study to robot manipulator. In Proceedings of the International MultiConference of Engineers and Computer Scientists, Hong Kong, China, 18–20 March 2009. [Google Scholar]
- Wiebe, R.; Virgin, L.N. A heuristic method for identifying chaos from frequency content. Chaos 2012, 22, 013136. [Google Scholar] [CrossRef] [PubMed]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Phys. D Nonlinear Phenom. 1985, 16, 285–317. [Google Scholar] [CrossRef]
- Asllani, M.; Expert, P.; Carletti, T. A minimally invasive neurostimulation method for controlling abnormal synchronisation in the neuronal activity. PLoS Comput. Biol. 2018, 14, e1006296. [Google Scholar] [CrossRef] [PubMed]
- Hammond, C.; Bergman, H.; Brown, P. Pathological synchronization in Parkinson’s disease: Networks, models and treatments. Trends Neurosci. 2007, 30, 357–364. [Google Scholar] [CrossRef]
- Orekhova, E.V.; Stroganova, T.A.; Nygren, G.; Tsetlin, M.M.; Posikera, I.N.; Gillberg, C.; Elam, M. Excess of high frequency electroencephalogram oscillations in boys with autism. Biol. Psychiatry 2007, 62, 1022–1029. [Google Scholar] [CrossRef]
- Chen, C.C.; Litvak, V.; Gilbertson, T.; Kuhn, A.; Lu, C.S.; Lee, S.T.; Tsai, C.H.; Tisch, S.; Limousin, P.; Hariz, M.; et al. Excessive synchronization of basal ganglia neurons at 20 Hz slows movement in Parkinson’s disease. Exp. Neurol. 2007, 205, 214–221. [Google Scholar] [CrossRef]
- Popovych, O.V.; Tass, P.A. Control of abnormal synchronization in neurological disorders. Front. Neurol. 2014, 5, 268. [Google Scholar] [CrossRef]











| l1 (Upper Arm) | l2 (Forearm) | l3 (Hand) | θi Limits |
|---|---|---|---|
| 31.5 cm | 28.7 cm | 10.5 cm | = [ −140°, 90°]; = [0°, 145°]; = [ −70°, 90°] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Machmudah, A.; Dutykh, D.; Parman, S. Coupled and Synchronization Models of Rhythmic Arm Movement in Planar Plane. Bioengineering 2022, 9, 385. https://doi.org/10.3390/bioengineering9080385
Machmudah A, Dutykh D, Parman S. Coupled and Synchronization Models of Rhythmic Arm Movement in Planar Plane. Bioengineering. 2022; 9(8):385. https://doi.org/10.3390/bioengineering9080385
Chicago/Turabian StyleMachmudah, Affiani, Denys Dutykh, and Setyamartana Parman. 2022. "Coupled and Synchronization Models of Rhythmic Arm Movement in Planar Plane" Bioengineering 9, no. 8: 385. https://doi.org/10.3390/bioengineering9080385
APA StyleMachmudah, A., Dutykh, D., & Parman, S. (2022). Coupled and Synchronization Models of Rhythmic Arm Movement in Planar Plane. Bioengineering, 9(8), 385. https://doi.org/10.3390/bioengineering9080385







