In-Silico Modeling to Compare Radiofrequency-Induced Thermal Lesions Created on Myocardium and Thigh Muscle
Abstract
:1. Introduction
2. Methods
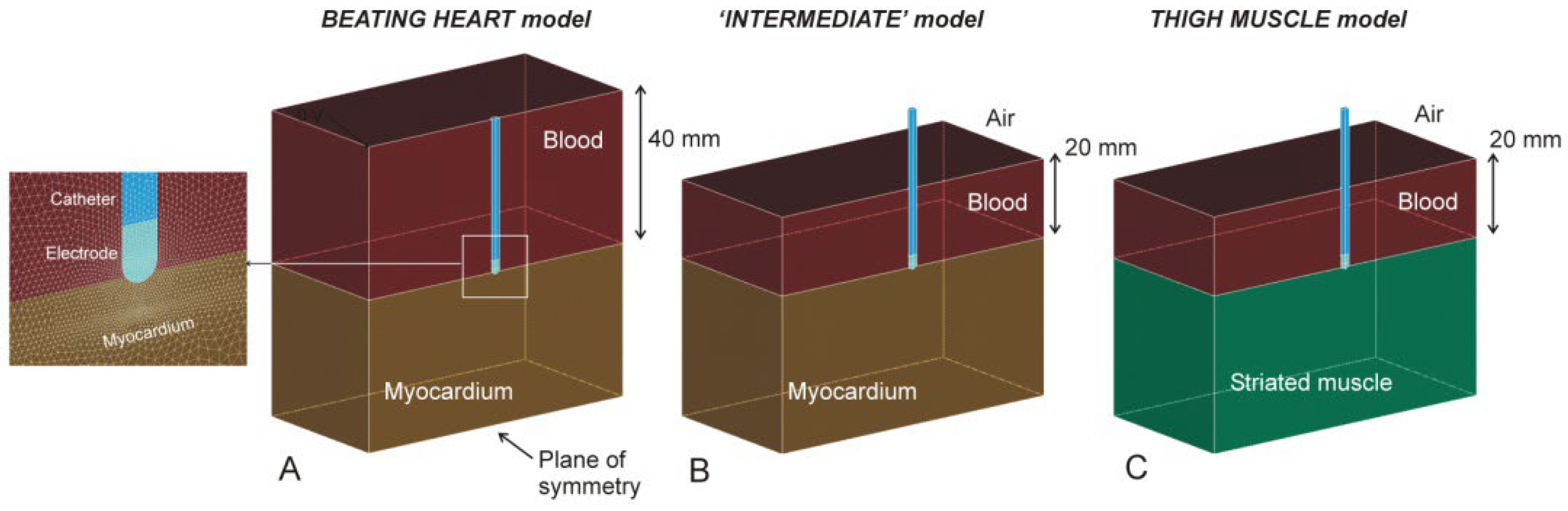
2.1. Model Geometry
2.2. Power-Duration Settings
2.3. Tissue Properties
2.4. Governing Equations
2.5. Boundary Conditions
2.6. Analyzed Outcomes
3. Results
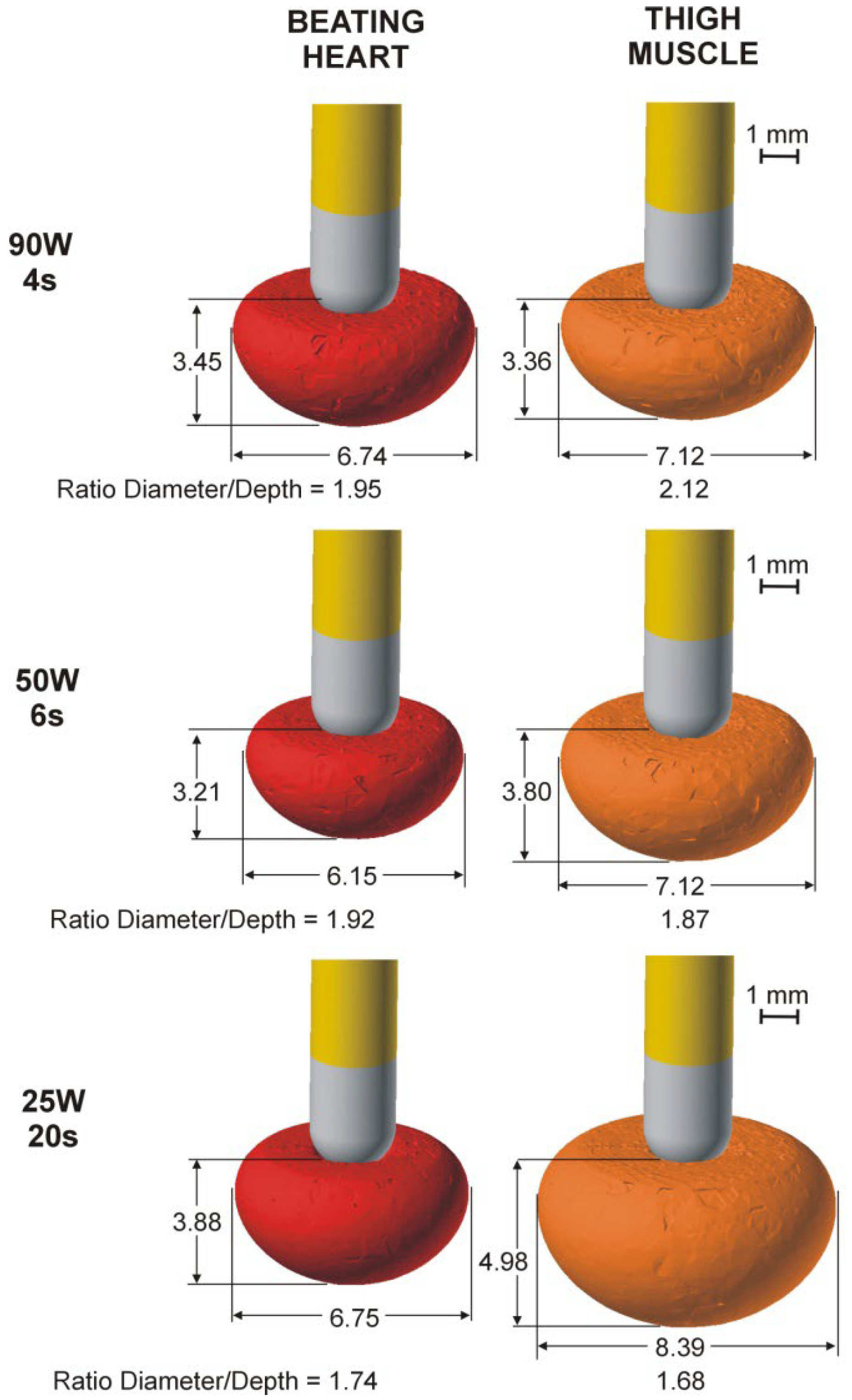
3.1. Comparison of Lesion Sizes
3.2. Electrical Performance
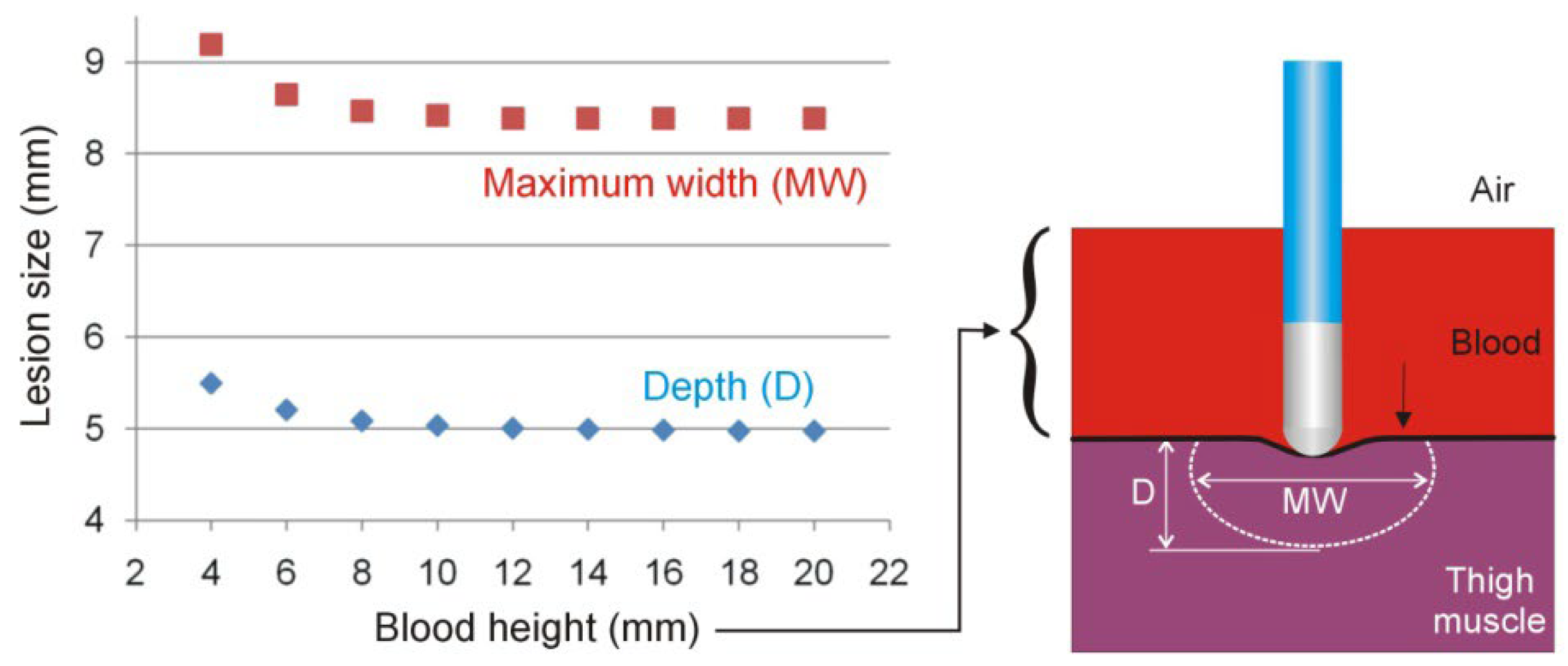
3.3. Effect of Blood Quantity on the Thigh Muscle
4. Discussion
4.1. Main Findings
- (1)
- The beating heart and ‘intermediate’ models predict nearly identical lesions, suggesting that the blood pool artificially created on the thigh to emulate the cardiac cavity has little effect on lesion size. In other words, proximity to the air–blood interface (just 2 cm from the tissue surface) does not appear to affect the distribution of RF current. Computer results suggest that a minimum height of 10 mm for the blood pool must be included in the thigh model.
- (2)
- The different electrical conductivities of striated muscle (in the thigh model) and myocardium (beating heart model) might be responsible for the slight difference in lesion size between models.
- (3)
- The difference in lesion sizes between the thigh and beating heart models seems to depend on the energy setting, with hardly any differences at 90 W/4 s, but with differences of 1 mm in depth and 1.5 m in width at 25 W/20 s.
- (4)
- The fact that more energy is delivered to the tissue in the thigh model, with differences that increase with RF pulse duration, is possibly the cause of the differences in the different lesion sizes of the models.
4.2. Practical Implications
4.3. Limitations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nakagawa, H.; Yamanashi, W.S.; Pitha, J.V.; Arruda, M.; Wang, X.; Ohtomo, K.; Beckman, K.J.; McClelland, J.H.; Lazzara, R.; Jackman, W.M. Comparison of In Vivo Tissue Temperature Profile and Lesion Geometry for Radiofrequency Ablation With a Saline-Irrigated Electrode Versus Temperature Control in a Canine Thigh Muscle Preparation. Circulation 1995, 91, 2264–2273. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leshem, E.; Tschabrunn, C.M.; Contreras-Valdes, F.M.; Zilberman, I.; Anter, E. Evaluation of ablation catheter technology: Comparison between thigh preparation model and an in vivo beating heart. Heart Rhythm 2017, 14, 1234–1240. [Google Scholar] [CrossRef] [PubMed]
- Leshem, E.; Zilberman, I.; Barkagan, M.; Shapira-Daniels, A.; Sroubek, J.; Govari, A.; Buxton, A.E.; Anter, E. Temperature-Controlled Radiofrequency Ablation Using Irrigated Catheters: Maximizing Ventricular Lesion Dimensions While Reducing Steam-Pop Formation. JACC Clin. Electrophysiol. 2020, 6, 83–93. [Google Scholar] [CrossRef]
- Nakagawa, H.; Ikeda, A.; Sharma, T.; Govari, A.; Ashton, J.; Maffre, J.; Lifshitz, A.; Fuimaono, K.; Yokoyama, K.; Wittkampf, F.H.M.; et al. Comparison of In Vivo Tissue Temperature Profile and Lesion Geometry for Radiofrequency Ablation With High Power-Short Duration and Moderate Power-Moderate Duration: Effects of Thermal Latency and Contact Force on Lesion Formation. Circ. Arrhythmia Electrophysiol. 2021, 14, e009899. [Google Scholar] [CrossRef]
- Pérez, J.J.; González-Suárez, A.; Maher, T.; Nakagawa, H.; D’Avila, A.; Berjano, E. Relationship between luminal esophageal temperature and volume of esophageal injury during RF ablation: In silico study comparing low power-moderate duration vs. high power-short duration. J. Cardiovasc. Electrophysiol. 2022, 33, 220–230. [Google Scholar] [CrossRef] [PubMed]
- Irastorza, R.M.; Gonzalez-Suarez, A.; Pérez, J.J.; Berjano, E. Differences in applied electrical power between full thorax models and limited-domain models for RF cardiac ablation. Int. J. Hyperth. 2020, 37, 677–687. [Google Scholar] [CrossRef]
- Pérez, J.J.; Nadal, E.; Berjano, E.; González-Suárez, A. Computer modeling of radiofrequency cardiac ablation including heartbeat-induced electrode displacement. Comput. Biol. Med. 2022, 144, 105346. [Google Scholar] [CrossRef]
- Hasgall, P.A.; Di Gennaro, F.; Baumgartner, C.; Neufeld, E.; Lloyd, B.; Gosselin, M.C.; Payne, D.; Klingenböck, A.; Kuster, N. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues. Version 4.1. 22 February 2022. Available online: https://itis.swiss/virtual-population/tissue-properties/database/ (accessed on 19 July 2012).
- Pérez, J.J.; Ewertowska, E.; Berjano, E. Computer Modeling for Radiofrequency Bipolar Ablation Inside Ducts and Vessels: Relation Between Pullback Speed and Impedance Progress. Lasers Surg. Med. 2020, 52, 897–906. [Google Scholar] [CrossRef]
- Tsai, J.Z.; Will, J.A.; Hubbard-Van Stelle, S.; Cao, H.; Tungjitkusolmun, S.; Choy, Y.B.; Haemmerich, D.; Vorperian, V.R.; Webster, J.G. In-vivo measurement of swine myocardial resistivity. IEEE Trans. Biomed. Eng. 2002, 49, 5472–5483. [Google Scholar] [CrossRef]
- Epstein, B.R.; Foster, K.R. Anisotropy in the dielectric properties of skeletal muscle. Med Biol. Eng. Comput. 1983, 21, 51–55. [Google Scholar] [CrossRef]
- Yue, K.; Cheng, L.; Yang, L.; Jin, B.; Zhang, X. Thermal Conductivity Measurement of Anisotropic Biological Tissue In Vitro. Int. J. Thermophys. 2017, 38, 92. [Google Scholar] [CrossRef]
- Hsu, E.W.; Muzikant, A.L.; Matulevicius, S.A.; Penland, R.C.; Henriquez, C.S. Magnetic resonance myocardial fiber-orientation mapping with direct histological correlation. Am. J. Physiol. 1998, 274, H1627–H1634. [Google Scholar] [CrossRef] [PubMed]
- González-Suárez, A.; Pérez, J.J.; Irastorza, R.M.; D’Avila, A.; Berjano, E. Computer modeling of radiofrequency cardiac ablation: 30 years of bioengineering research. Comput. Methods Programs Biomed. 2022, 214, 106546. [Google Scholar] [CrossRef] [PubMed]
- Abraham, J.P.; Sparrow, E.M. A thermal-ablation bioheat model including liquid-to-vapor phase change, pressure- and necrosis-dependent perfusion, and moisture-dependent properties. Int. J. Heat Mass Transf. 2007, 50, 2537–2544. [Google Scholar] [CrossRef]
- Mitchell, H.H.; Hamilton, T.S.; Steggerda, F.R.; Bean, H.W. The chemical composition of the adult human body and its bearing on the biochemistry of growth. J. Biol. Chem. 1945, 158, 625–637. [Google Scholar] [CrossRef]
- González-Suárez, A.; Pérez, J.J.; Berjano, E. Should fluid dynamics be included in computer models of RF cardiac ablation by irrigated-tip electrodes? Biomed. Eng. Online 2018, 17, 43. [Google Scholar] [CrossRef] [Green Version]
- Irastorza, R.M.; D’Avila, A.; Berjano, E. Thermal latency adds to lesion depth after application of high-power short-duration radiofrequency energy: Results of a computer-modeling study. J. Cardiovasc. Electrophysiol. 2018, 29, 322–327. [Google Scholar] [CrossRef]
- Pearce, J.A. Comparative analysis of mathematical models of cell death and thermal damage processes. Int. J. Hyperth. 2013, 29, 262–280. [Google Scholar] [CrossRef]
- Haines, D.E. Letter regarding article, “Direct measurement of the lethal isotherm for radiofrequency ablation of myocardial tissue”. Circ. Arrhythmia Electrophysiol. 2011, 4, e67. [Google Scholar] [CrossRef] [Green Version]
- Thomsen, S.; Pearce, J.A.; Randeri, R. Determination of isotherms of thermal damage. Lasers and Electro-Optics Society Annual Meeting. In Proceedings of the LEOS ’94 Conference Proceedings, Boston, MA, USA, 6 August 2002; pp. 295–296. [Google Scholar]
- Chik, W.W.; Barry, M.A.; Pouliopoulos, J.; Byth, K.; Midekin, C.; Bhaskaran, A.; Sivagangabalan, G.; Thomas, S.P.; Ross, D.L.; McEwan, A.; et al. Electrogram-gated radiofrequency ablations with duty cycle power delivery negate effects of ablation catheter motion. Circ. Arrhythmia Electrophysiol. 2014, 7, 920–928. [Google Scholar] [CrossRef] [Green Version]
- Bourier, F.; Popa, M.; Kottmaier, M.; Maurer, S.; Bahlke, F.; Telishevska, M.; Lengauer, S.; Koch-Büttner, K.; Kornmayer, M.; Risse, E.; et al. RF electrode–tissue coverage significantly influences steam pop incidence and lesion size. J. Cardiovasc. Electrophysiol. 2021, 32, 1594–1599. [Google Scholar] [CrossRef]
- Pérez, J.J.; González-Suárez, A.; Berjano, E. Numerical analysis of thermal impact of intramyocardial capillary blood flow during radiofrequency cardiac ablation. Int. J. Hyperth. 2018, 34, 243–249. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gu, K.; Yan, S.; Wu, X. Effect of anisotropy in myocardial electrical conductivity on lesion characteristics during radiofrequency cardiac ablation: A numerical study. Int. J. Hyperth. 2022, 39, 120–133. [Google Scholar] [CrossRef] [PubMed]
- Halbfass, P.; Wielandts, J.Y.; Knecht, S.; Le Polain de Waroux, J.B.; Tavernier, R.; De Wilde, V.; Sonne, K.; Nentwich, K.; Ene, E.; Berkovitz, A.; et al. Safety of very high-power short-duration radiofrequency ablation for pulmonary vein isolation: A two-centre report with emphasis on silent oesophageal injury. EP Europace 2022, 24, 400–405. [Google Scholar] [CrossRef] [PubMed]
- Stabile, G.; Schillaci, V.; Strisciuglio, T.; Arestia, A.; Agresta, A.; Shopova, G.; De Simone, A.; Solimene, F. In vivo biophysical characterization of very high power, short duration, temperature-controlled lesions. Pacing Clin. Electrophysiol. 2021, 44, 1717–1723. [Google Scholar] [CrossRef]
- Richard Tilz, R.; Sano, M.; Vogler, J.; Fink, T.; Saraei, R.; Sciacca, V.; Kirstein, B.; Phan, H.L.; Hatahet, S.; Delgado Lopez, L.; et al. Very high-power short-duration temperature-controlled ablation versus conventional power-controlled ablation for pulmonary vein isolation: The fast and furious—AF study. IJC Heart Vasc. 2021, 35, 100847. [Google Scholar] [CrossRef]
- Reddy, V.Y.; Grimaldi, M.; De Potter, T.; Vijgen, J.M.; Bulava, A.; Duytschaever, M.F.; Martinek, M.; Natale, A.; Knecht, S.; Neuzil, P.; et al. Pulmonary Vein Isolation With Very High Power, Short Duration, Temperature-Controlled Lesions: The QDOT-FAST Trial. JACC Clin. Electrophysiol. 2019, 5, 778–786. [Google Scholar] [CrossRef]
- Mathur, A.B.; Collinsworth, A.M.; Reichert, W.M.; Kraus, W.E.; Truskey, G.A. Endothelial, cardiac muscle and skeletal muscle exhibit different viscous and elastic properties as determined by atomic force microscopy. J. Biomech. 2001, 34, 1545–1553. [Google Scholar] [CrossRef]
- Gabriel, C.; Peyman, A.; Grant, E.H. Electrical conductivity of tissue at frequencies below 1 MHz. Phys. Med. Biol. 2009, 54, 4863–4878. [Google Scholar] [CrossRef]
| Element/Material | σ (S/m) | k (W/m·K) | ρ (kg/m3) | c (J/kg·K) | Reference |
|---|---|---|---|---|---|
| Electrode/Pt-Ir | 4.6 × 106 | 71 | 21,500 | 132 | [9] |
| Catheter/Polyurethane | 10−5 | 23 | 1440 | 1050 | [9] |
| Cardiac Chamber/Blood | 0.748 | -- | -- | -- | [8] |
| Striated muscle | 0.446 | 0.49 | 1090 | 3421 | [8] |
| Myocardium | 0.281 | 0.56 | 1081 | 3686 | [8] |
| Maximum Depth D (mm) | Maximum Width MW (mm) | Ratio MW/D | Volume (mm3) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Energy Setting | Catheter Orientation | tRF | t90s | tRF | t90s | tRF | t90s | tRF | t90s |
| 90 W 4 s | 0° | 2.73 | 3.54 | 6.62 | 7.14 | 2.42 | 2.02 | 70.92 | 107.46 |
| 45° | 2.81 | 3.54 | 6.60 | 7.20 | 2.35 | 2.03 | 65.58 | 101.18 | |
| 90° | 2.78 | 3.45 | 6.14 | 6.74 | 2.21 | 1.95 | 59.89 | 90.86 | |
| 50 W 6 s | 0° | 2.59 | 3.24 | 6.09 | 6.51 | 2.35 | 2.01 | 57.99 | 82.50 |
| 45° | 2.67 | 3.26 | 6.07 | 6.55 | 2.27 | 2.01 | 53.34 | 76.92 | |
| 90° | 2.66 | 3.21 | 5.65 | 6.15 | 2.12 | 1.92 | 48.82 | 69.92 | |
| 25 W 20 s | 0° | 3.48 | 4.04 | 6.60 | 6.99 | 1.90 | 1.73 | 93.37 | 121.42 |
| 45° | 3.46 | 3.97 | 6.77 | 7.17 | 1.96 | 1.81 | 87.83 | 113.50 | |
| 90° | 3.41 | 3.88 | 6.34 | 6.75 | 1.86 | 1.74 | 78.64 | 101.62 | |
| Maximum Depth D (mm) | Maximum Width MW (mm) | Ratio MW/D | Volume (mm3) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Energy Setting | Catheter Orientation | tRF | t90s | tRF | t90s | tRF | t90s | tRF | t90s |
| 90 W 4 s | 0° | 2.54 | 3.47 | 6.72 | 7.24 | 2.65 | 2.09 | 73.90 | 112.61 |
| 45° | 2.60 | 3.48 | 7.06 | 7.64 | 2.72 | 2.20 | 71.00 | 112.40 | |
| 90° | 2.53 | 3.36 | 6.53 | 7.12 | 2.58 | 2.12 | 62.20 | 98.69 | |
| 50 W 6 s | 0° | 3.13 | 3.94 | 6.97 | 7.50 | 2.23 | 1.90 | 88.78 | 132.15 |
| 45° | 3.15 | 3.92 | 6.93 | 7.51 | 2.20 | 1.92 | 82.74 | 123.58 | |
| 90° | 3.11 | 3.80 | 6.53 | 7.12 | 2.10 | 1.87 | 75.73 | 111.10 | |
| 25 W 20 s | 0° | 4.25 | 5.11 | 8.03 | 8.62 | 1.89 | 1.69 | 164.11 | 225.75 |
| 45° | 4.25 | 5.06 | 8.11 | 8.73 | 1.91 | 1.73 | 158.06 | 218.32 | |
| 90° | 4.21 | 4.98 | 7.76 | 8.39 | 1.84 | 1.68 | 147.31 | 203.08 | |
| PT (%) | ET (%) | ||||
|---|---|---|---|---|---|
| Energy Setting | Catheter Orientation | BH | TM | BH | TM |
| 90 W 4 s | 0° | 17.60 | 25.62 | 21.31 | 26.32 |
| 45° | 16.91 | 24.67 | 19.75 | 22.61 | |
| 90° | 16.20 | 23.88 | 19.66 | 21.23 | |
| 50 W 7.2 s | 0° | 17.41 | 25.25 | 20.69 | 30.23 |
| 45° | 16.77 | 24.34 | 19.80 | 28.78 | |
| 90° | 16.06 | 23.52 | 19.05 | 27.29 | |
| 30 W 12 s | 0° | 17.29 | 24.98 | 20.27 | 30.75 |
| 45° | 16.68 | 24.12 | 19.40 | 29.67 | |
| 90° | 15.96 | 23.29 | 18.57 | 28.81 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pérez, J.J.; Berjano, E.; González-Suárez, A. In-Silico Modeling to Compare Radiofrequency-Induced Thermal Lesions Created on Myocardium and Thigh Muscle. Bioengineering 2022, 9, 329. https://doi.org/10.3390/bioengineering9070329
Pérez JJ, Berjano E, González-Suárez A. In-Silico Modeling to Compare Radiofrequency-Induced Thermal Lesions Created on Myocardium and Thigh Muscle. Bioengineering. 2022; 9(7):329. https://doi.org/10.3390/bioengineering9070329
Chicago/Turabian StylePérez, Juan J., Enrique Berjano, and Ana González-Suárez. 2022. "In-Silico Modeling to Compare Radiofrequency-Induced Thermal Lesions Created on Myocardium and Thigh Muscle" Bioengineering 9, no. 7: 329. https://doi.org/10.3390/bioengineering9070329
APA StylePérez, J. J., Berjano, E., & González-Suárez, A. (2022). In-Silico Modeling to Compare Radiofrequency-Induced Thermal Lesions Created on Myocardium and Thigh Muscle. Bioengineering, 9(7), 329. https://doi.org/10.3390/bioengineering9070329








