Computational Modeling of the Liver Arterial Blood Flow for Microsphere Therapy: Effect of Boundary Conditions
Abstract
1. Introduction
2. Materials and Methods
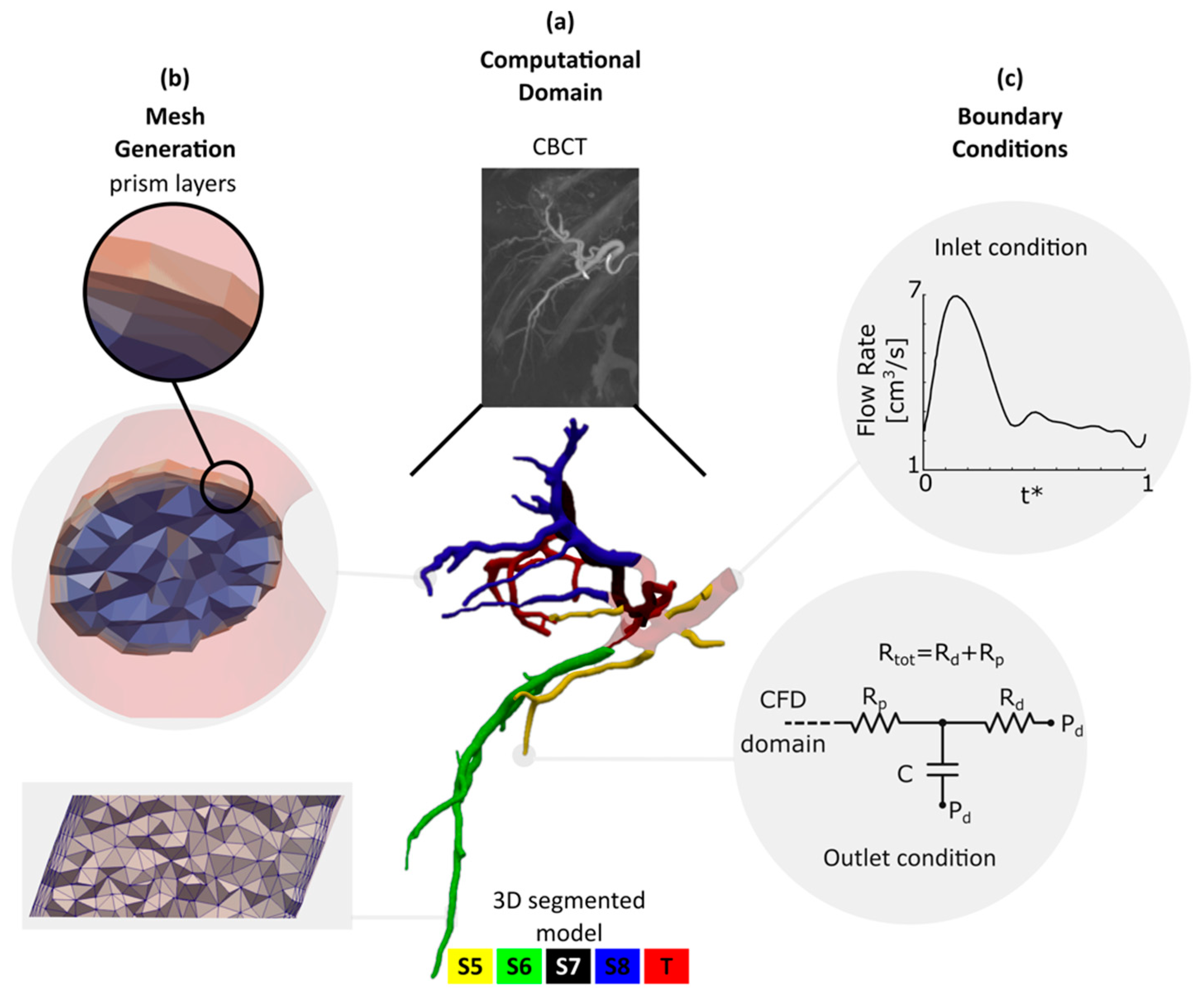
2.1. Computational Domain Extracted from CBCT
2.2. Meshing
2.3. Governing Equations
2.4. Boundary Conditions
2.5. Solver
2.6. Postprocessing of CFD Results
3. Results
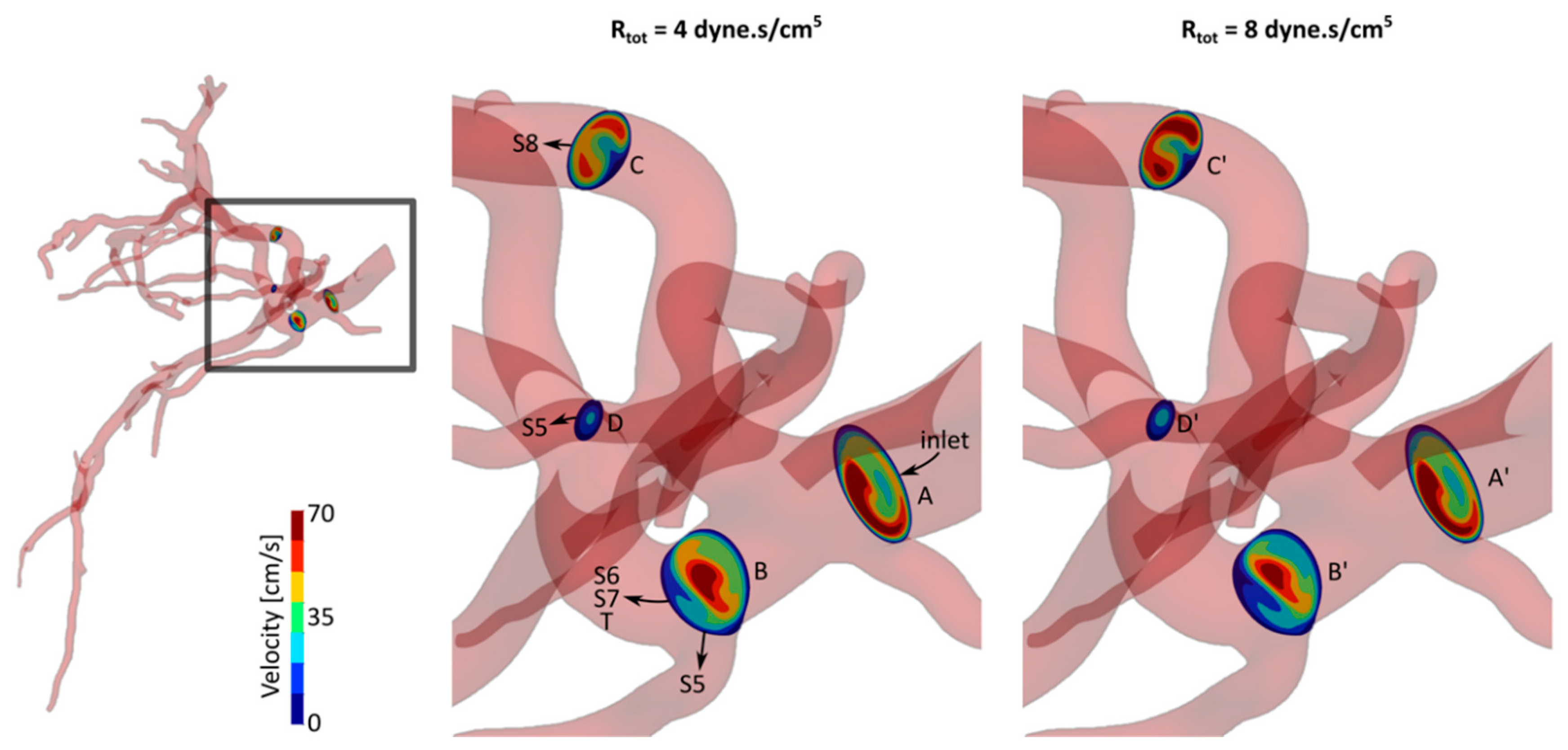
3.1. Hepatic Arterial Tree Hemodynamics
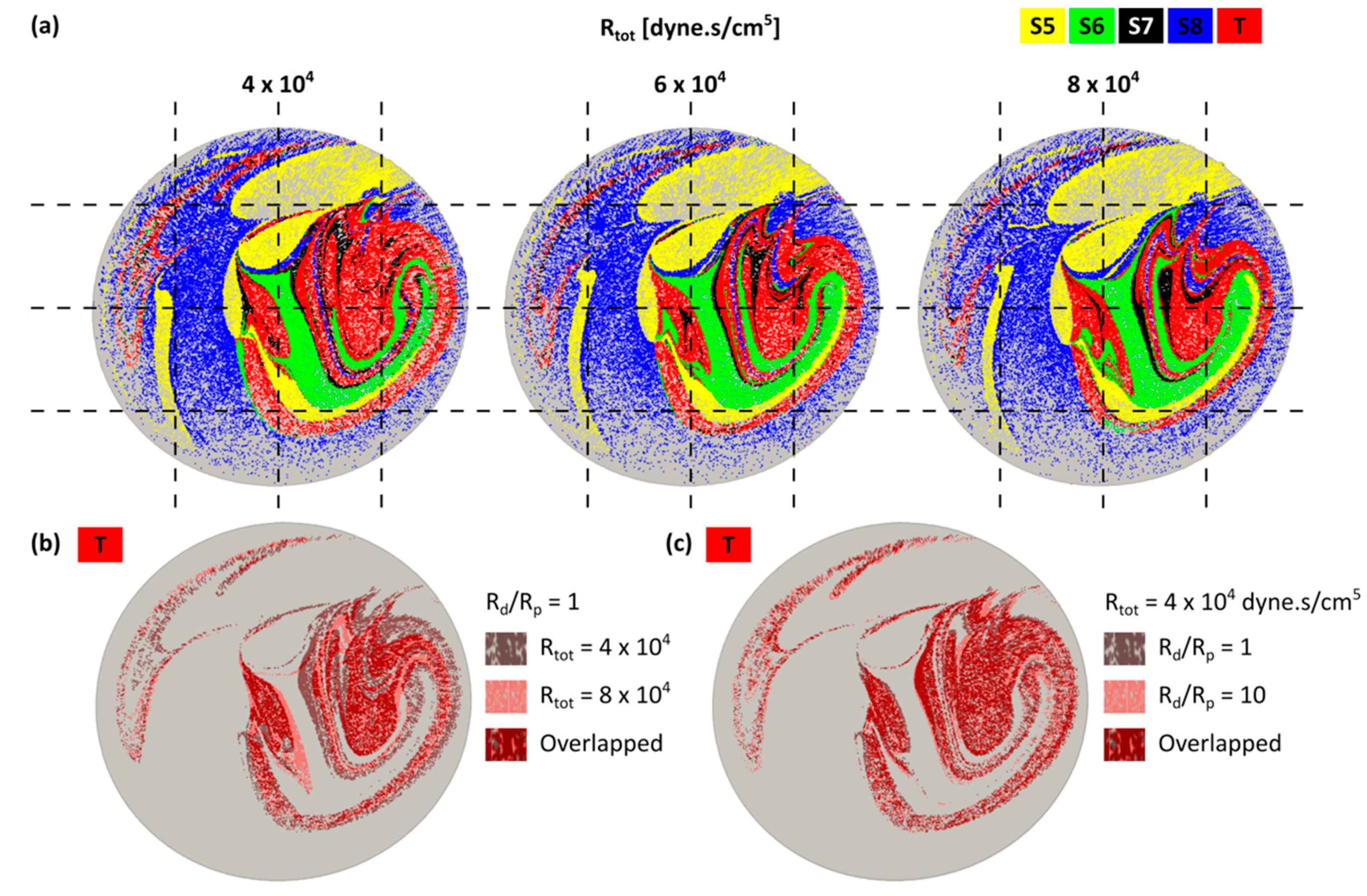
3.2. Particle Release Maps
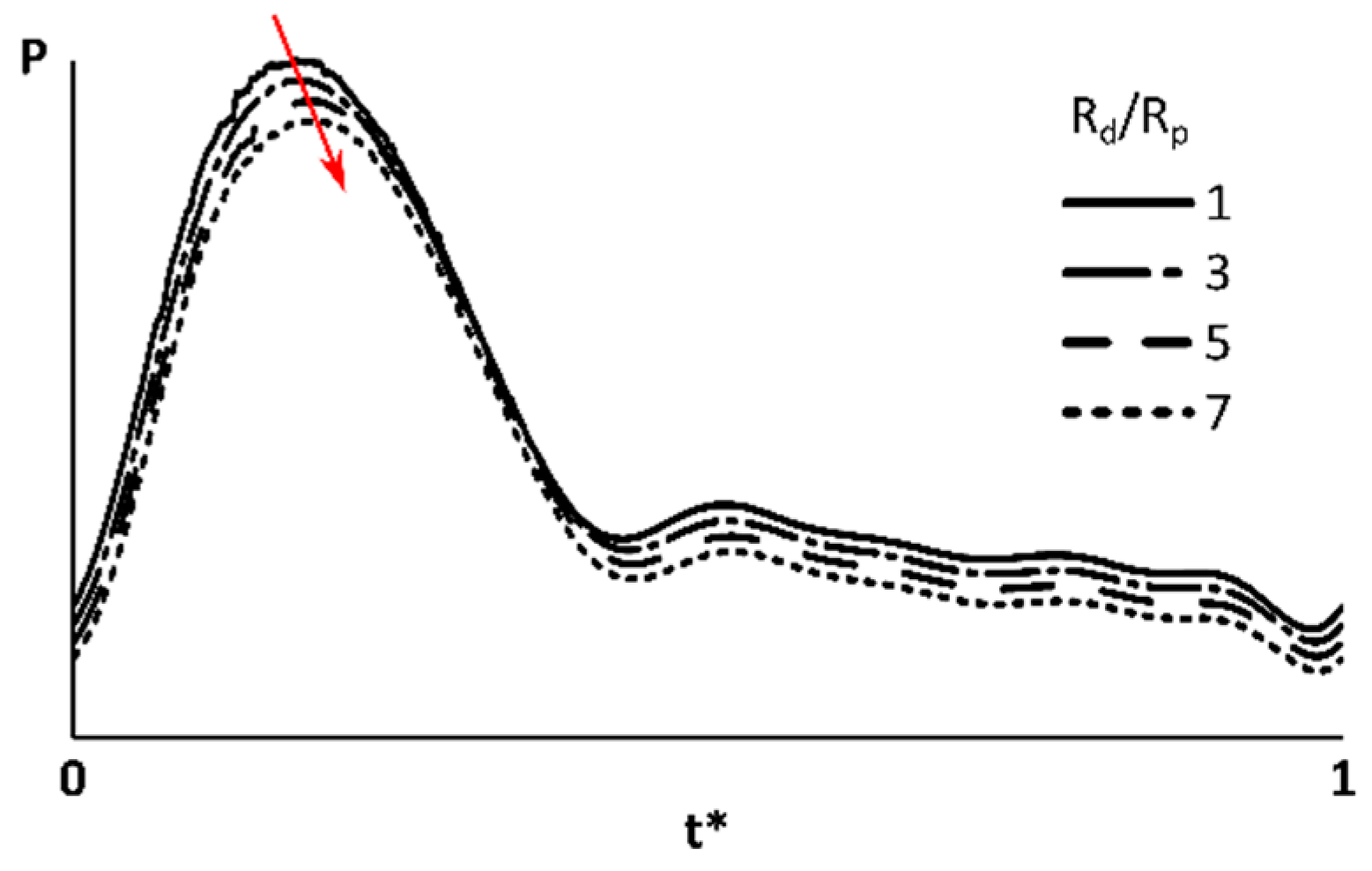
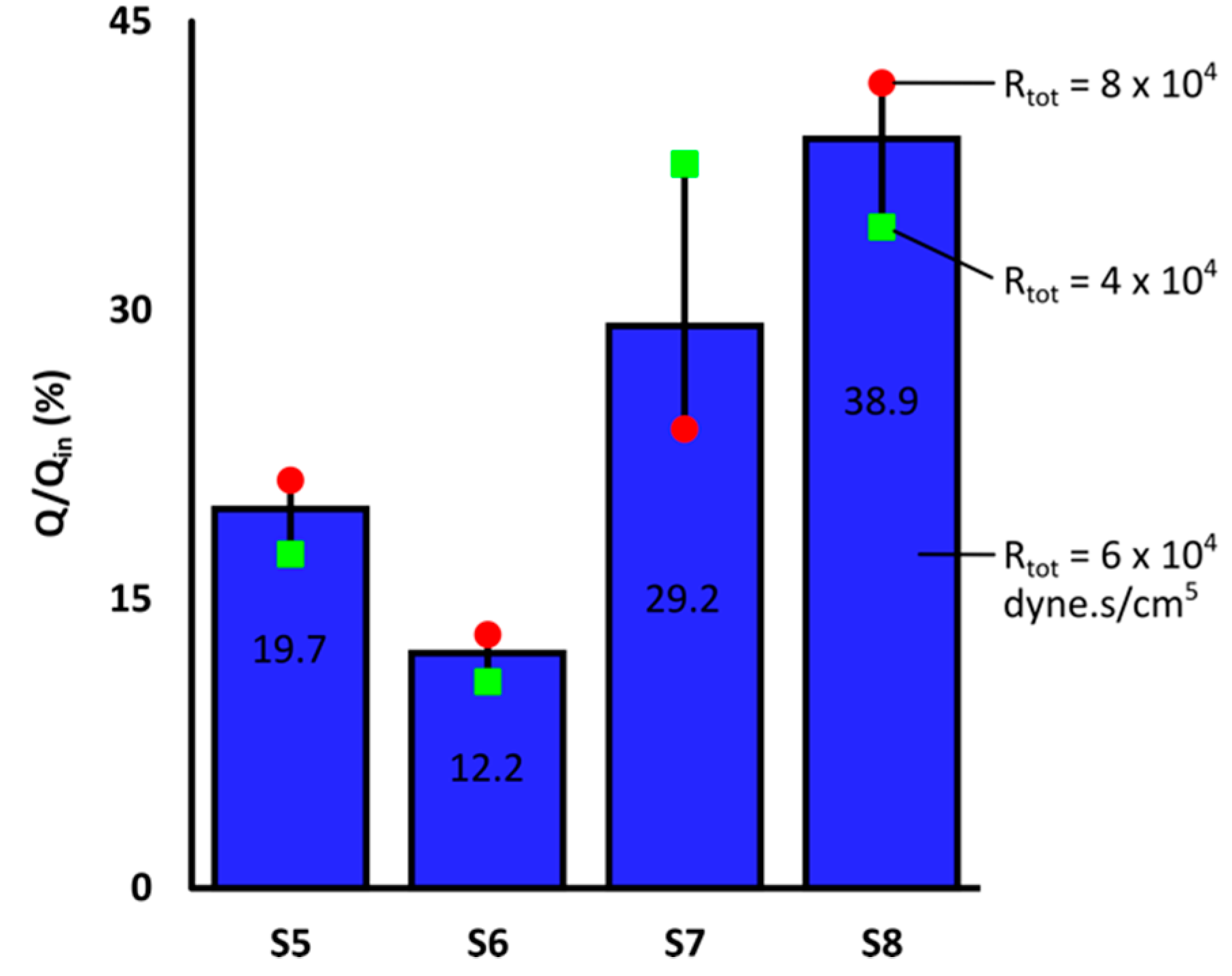
3.3. Effect of Rtot and Rd/Rp Ratio on Outlet Pressure and Flow Rate
3.4. Blood Flow Distribution and 90Y Delivery in Liver
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A

References
- Llovet, J.M.; Zucman-Rossi, J.; Pikarsky, E.; Sangro, B.; Schwartz, M.; Sherman, M.; Gores, G. Hepatocellular carcinoma. Nat. Rev. Dis. Primers 2016, 2, 16018. [Google Scholar] [CrossRef] [PubMed]
- Kennedy, A.S.; Kleinstreuer, C.; Basciano, C.A.; Dezarn, W.A. Computer Modeling of Yttrium-90-Microsphere Transport in the Hepatic Arterial Tree to Improve Clinical Outcomes. Int. J. Radiat. Oncol. Biol. Phys. 2010, 76, 631–637. [Google Scholar] [CrossRef] [PubMed]
- Basciano, C.A.; Kleinstreuer, C.; Kennedy, A.S. Computational Fluid Dynamics Modeling of 90Y Microspheres in Human Hepatic Tumors. J. Nucl. Med. Radiat. Ther. 2011, 2. [Google Scholar] [CrossRef]
- Aramburu, J.; Antón, R.; Rivas, A.; Ramos, J.C.; Larraona, G.S.; Sangro, B.; Bilbao, J.I. Numerical zero-dimensional hepatic artery hemodynamics model for balloon-occluded transarterial chemoembolization. Int. J. Numer Methods Biomed. Eng. 2018, 34, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Aramburu, J.; Anton, R.; Rivas, A.; Ramos, J.C.; Sangro, B.; Bilbao, J.I. Liver Radioembolization: An Analysis of Parameters that Influence the Catheter-Based Particle-Delivery via CFD. Curr. Med. Chem. 2018, 25, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Ma, R.; Hunter, P.; Cousins, W.; Ho, H.; Bartlett, A.; Safaei, S. Modelling the Hepatic Arterial Flow in Living Liver Donor after Left Hepatectomy and Postoperative Boundary Condition Exploration. Int. J. Numer Methods Biomed. Eng. 2019, 36. [Google Scholar] [CrossRef]
- Audebert, C. Mathematical Liver Modeling: Hemodynamics and Function in Hepatectomy; Université Pierre et Marie Curie-Paris VI: Paris, France, 2017. [Google Scholar]
- Sugihara, F.; Murata, S.; Ueda, T.; Yasui, D.; Yamaguchi, H.; Miki, I.; Kawamoto, C.; Uchida, E.; Kumita, S. Haemodynamic changes in hepatocellular carcinoma and liver parenchyma under balloon occlusion of the hepatic artery. Eur. Radiol. 2017, 27, 2474–2481. [Google Scholar] [CrossRef] [PubMed]
- Rose, S.C.; Kikolski, S.G.; Chomas, J.E. Downstream hepatic arterial blood pressure changes caused by deployment of the surefire antireflux expandable tip. Cardiovasc. Interv. Radiol. 2013, 36, 1262–1269. [Google Scholar] [CrossRef]
- Leen, E.; Goldberg, J.A.; Robertson, J.; Sutherland, G.R.; Hemingway, D.M.; Cooke, T.G.; McARDLE, C.S. Detection of hepatic metastases using duplex/color Doppler sonography. Ann. Surg. 1991, 214, 599. [Google Scholar] [CrossRef] [PubMed]
- Vollmar, B.; Menger, M.D. The Hepatic Microcirculation: Mechanistic Contributions and Therapeutic Targets in Liver Injury and Repair. Physiol. Rev. 2009, 89, 1269–1339. [Google Scholar] [CrossRef]
- Diebel, L.N.; Wilson, R.F.; Dulchavsky, S.A.; Saxe, J. Effect of increased intra-abdominal pressure on hepatic arterial, portal venous, and hepatic microcirculatory blood flow. J. Trauma 1992, 33, 279–282. [Google Scholar] [CrossRef]
- Tse, J.R.; Liang, T.; Jeffrey, R.B.; Kamaya, A. Does measurement of the hepatic artery velocity improve the sonographic diagnosis of cholangitis? Abdom. Radiol. 2019, 44, 4004–4010. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.P.; Cohalan, C.; Kopek, N.; Enger, S.A. A guide to 90Y radioembolization and its dosimetry. Phys. Med. 2019, 68, 132–145. [Google Scholar] [CrossRef] [PubMed]
- Roncali, E.; Taebi, A.; Foster, C.; Vu, C.T. Personalized Dosimetry for Liver Cancer Y-90 Radioembolization Using Computational Fluid Dynamics and Monte Carlo Simulation. Ann. Biomed. Eng. 2020. [Google Scholar] [CrossRef] [PubMed]
- Taebi, A.; Roudsari, B.; Vu, C.; Cherry, S.; Roncali, E. Hepatic arterial tree segmentation: Towards patient-specific dosimetry for liver cancer radioembolization. J. Nucl. Med. 2019, 60, 122. [Google Scholar]
- Couinaud, C. Le foie. Études Anatomiques et Chirurgicales; Masson: Paris, France, 1957. [Google Scholar]
- Kline, T.L.; Zamir, M.; Ritman, E.L. Accuracy of Microvascular Measurements Obtained From Micro-CT Images. Ann. Biomed. Eng. 2010, 38, 2851–2864. [Google Scholar] [CrossRef]
- Kline, T.L.; Zamir, M.; Ritman, E.L. Relating Function to Branching Geometry: A Micro-CT Study of the Hepatic Artery, Portal Vein, and Biliary Tree. Cells Tissues Organs 2011, 194, 431–442. [Google Scholar] [CrossRef]
- Si, H. TetGen, a delaunay-based quality tetrahedral mesh generator. ACM Trans. Math. Softw. 2015. [Google Scholar] [CrossRef]
- Celik, I.B.; Ghia, U.; Roache, P.J.; Freitas, C.J.; Coleman, H.; Raad, P.E. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 078001. [Google Scholar] [CrossRef]
- Taebi, A.; Vu, C.; Roncali, E. Multi-scale computational fluid dynamics modeling for personalized liver cancer radioembolization dosimetry. J. Biomech. Eng. 2020. [Google Scholar] [CrossRef]
- Ku, D.N. Blood flow in arteries. Annu. Rev. Fluid Mech. 1997, 29, 399–434. [Google Scholar] [CrossRef]
- Aramburu, J.; Antón, R.; Rivas, A.; Ramos, J.C.; Sangro, B.; Bilbao, J.I. Liver cancer arterial perfusion modelling and CFD boundary conditions methodology: A case study of the haemodynamics of a patient-specific hepatic artery in literature-based healthy and tumour-bearing liver scenarios. Int. J. Numer Methods Biomed. Eng. 2016, 32, e02764. [Google Scholar] [CrossRef]
- Basciano, C.A.; Kleinstreuer, C.; Kennedy, A.S.; Dezarn, W.A.; Childress, E. Computer modeling of controlled microsphere release and targeting in a representative hepatic artery system. Ann. Biomed. Eng. 2010, 38, 1862–1879. [Google Scholar] [CrossRef]
- Grinberg, L.; Karniadakis, G.E. Outflow Boundary Conditions for Arterial Networks with Multiple Outlets. Ann. Biomed. Eng. 2008, 36, 1496–1514. [Google Scholar] [CrossRef]
- Vignon-Clementel, I.E.; Figueroa, C.A.; Jansen, K.E.; Taylor, C.A. Outflow boundary conditions for 3D simulations of non-periodic blood flow and pressure fields in deformable arteries. Comput. Methods Biomech. Biomed. Eng. 2010, 13, 625–640. [Google Scholar] [CrossRef]
- Wang, T.; Liang, F.; Zhou, Z.; Qi, X. Global sensitivity analysis of hepatic venous pressure gradient (HVPG) measurement with a stochastic computational model of the hepatic circulation. Comput. Biol. Med. 2018, 97, 124–136. [Google Scholar] [CrossRef] [PubMed]
- Esmaily Moghadam, M.; Vignon-Clementel, I.E.; Figliola, R.; Marsden, A.L. A modular numerical method for implicit 0D/3D coupling in cardiovascular finite element simulations. J. Comput. Phys. 2013, 244, 63–79. [Google Scholar] [CrossRef]
- Martini, F.; Nath, J.L.; Bartholomew, E.F. Fundamentals of Anatomy & Physiology; Pearson: Madrid, Spain, 2015. [Google Scholar]
- Olufsen, M.S. Structured tree outflow condition for blood flow in larger systemic arteries. Am. J. Physiol. Heart Circ. Physiol. 1999, 276, H257–H268. [Google Scholar] [CrossRef] [PubMed]
- Lan, H.; Updegrove, A.; Wilson, N.M.; Maher, G.D.; Shadden, S.C.; Marsden, A.L. A Re-Engineered Software Interface and Workflow for the Open-Source SimVascular Cardiovascular Modeling Package. J. Biomech. Eng. 2018, 140, 024501. [Google Scholar] [CrossRef] [PubMed]
- Arzani, A.; Dyverfeldt, P.; Ebbers, T.; Shadden, S.C. In Vivo Validation of Numerical Prediction for Turbulence Intensity in an Aortic Coarctation. Ann. Biomed. Eng. 2012, 40, 860–870. [Google Scholar] [CrossRef]
- Kung, E.O.; Les, A.S.; Figueroa, C.A.; Medina, F.; Arcaute, K.; Wicker, R.B.; McConnell, M.V.; Taylor, C.A. In Vitro Validation of Finite Element Analysis of Blood Flow in Deformable Models. Ann. Biomed. Eng. 2011, 39, 1947–1960. [Google Scholar] [CrossRef] [PubMed]
- Kung, E.O.; Les, A.S.; Medina, F.; Wicker, R.B.; McConnell, M.V.; Taylor, C.A. In Vitro Validation of Finite-Element Model of AAA Hemodynamics Incorporating Realistic Outlet Boundary Conditions. J. Biomech. Eng. 2011, 133, 041003. [Google Scholar] [CrossRef] [PubMed]
- Brooks, A.N.; Hughes, T.J.R. Streamline upwind/Petrov-Galerkin formulations for convection dominated flows with particular emphasis on the incompressible Navier-Stokes equations. Comput. Methods Appl. Mech. Eng. 1982, 32, 199–259. [Google Scholar] [CrossRef]
- Jansen, K.E.; Whiting, C.H.; Hulbert, G.M. A generalized-α method for integrating the filtered Navier–Stokes equations with a stabilized finite element method. Comput. Methods Appl. Mech. Eng. 2000, 190, 305–319. [Google Scholar] [CrossRef]
- Yilmaz, F.; Gundogdu, M.Y. A critical review on blood flow in large arteries; relevance to blood rheology, viscosity models, and physiologic conditions. Korea-Aust. Rheol. J. 2008, 20, 197–211. [Google Scholar]
- Simoncini, C.; Jurczuk, K.; Reska, D.; Esneault, S.; Nunes, J.C.; Bellanger, J.J.; Saint-Jalmes, H.; Rolland, Y.; Eliat, P.A.; Bézy-Wendling, J.; et al. Towards a patient-specific hepatic arterial modeling for microspheres distribution optimization in SIRT protocol. Med. Biol. Eng. Comput. 2018, 56, 515–529. [Google Scholar] [CrossRef]
- Crookston, N.R.; Fung, G.S.K.; Frey, E.C. Development of a Customizable Hepatic Arterial Tree and Particle Transport Model for Use in Treatment Planning. IEEE Trans. Radiat. Plasma Med. Sci. 2018, 3, 31–37. [Google Scholar] [CrossRef]
- Chen, J.; Lu, X.-Y.; Wang, W. Non-Newtonian effects of blood flow on hemodynamics in distal vascular graft anastomoses. J. Biomech. 2006, 39, 1983–1995. [Google Scholar] [CrossRef]

| Rd/Rp | ||||||
|---|---|---|---|---|---|---|
| Rtot [×104 dyne·s/cm5] | 4.0 | 1 | 3 | ̶ | ̶ | 10 |
| 4.7 | 1 | 3 | ̶ | ̶ | 10 | |
| 5.3 | 1 | 3 | ̶ | ̶ | 10 | |
| 6.0 | 1 | 3 | ̶ | ̶ | 10 | |
| 6.8 | 1 | 3 | 5 | 7 | 10 | |
| 7.3 | 1 | 3 | ̶ | ̶ | 10 | |
| 8.0 | 1 | 3 | ̶ | ̶ | 10 | |
| t13 [×10−3] | t15 [×10−3] | t17 [×10−3] | |
|---|---|---|---|
| S5 | 1.5 ± 1.64 | 7.5 ± 1.64 | 12.5 ± 2.26 |
| S6 | 0.3 ± 1.70 | 8.7 ± 0.95 | 12.3 ± 2.21 |
| S7 | 1.4 ± 1.54 | 9.7 ± 1.69 | 14.5 ± 1.59 |
| S8 | 0.9 ± 1.44 | 8.8 ± 0.83 | 13.1 ± 1.95 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taebi, A.; Pillai, R.M.; S. Roudsari, B.; Vu, C.T.; Roncali, E. Computational Modeling of the Liver Arterial Blood Flow for Microsphere Therapy: Effect of Boundary Conditions. Bioengineering 2020, 7, 64. https://doi.org/10.3390/bioengineering7030064
Taebi A, Pillai RM, S. Roudsari B, Vu CT, Roncali E. Computational Modeling of the Liver Arterial Blood Flow for Microsphere Therapy: Effect of Boundary Conditions. Bioengineering. 2020; 7(3):64. https://doi.org/10.3390/bioengineering7030064
Chicago/Turabian StyleTaebi, Amirtahà, Rex M. Pillai, Bahman S. Roudsari, Catherine T. Vu, and Emilie Roncali. 2020. "Computational Modeling of the Liver Arterial Blood Flow for Microsphere Therapy: Effect of Boundary Conditions" Bioengineering 7, no. 3: 64. https://doi.org/10.3390/bioengineering7030064
APA StyleTaebi, A., Pillai, R. M., S. Roudsari, B., Vu, C. T., & Roncali, E. (2020). Computational Modeling of the Liver Arterial Blood Flow for Microsphere Therapy: Effect of Boundary Conditions. Bioengineering, 7(3), 64. https://doi.org/10.3390/bioengineering7030064






