Profile of a Multivariate Observation under Destructive Sampling—A Monte Carlo Approach to a Case of Spina Bifida
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Details
2.2. Statistical Methods
- Given X5, simulate the joint distribution of (X1, X2, X3, X4). This requires knowledge of the conditional mean and conditional dispersion matrix.
- We need μi s, which can be estimated using the individual data on Xi s.
- We need σi s, which can be estimated using the individual data on Xi s.
- The correlation coefficient ρ glues the means, variances, and joint distribution. There was no way we can estimate the correlation coefficient using the marginal data we have. We performed simulations by assuming the value of ρ = 0.0 (0.1) 0.9.
- We conducted Monte Carlo simulations. For each choice of ρ and fluid, Steps 1 through 4 were repeated one thousand times. The average of (X1, X2, X3, X4) s was the desired profile. The 95% band surrounding the mean was built using the following inequality:
3. Results
3.1. Conditional Profile
3.2. Unconditional Profile
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Iskandar, B.J.; Finnell, R.H. Spina Bifida. N. Engl. J. Med. 2022, 387, 444–450. [Google Scholar] [CrossRef] [PubMed]
- Avagliano, L.; Massa, V.; George, T.M.; Qureshy, S.; Bulfamante, G.; Finnell, R.H. Overview on Neural Tube Defects: From Development to Physical Characteristics. Birth Defects Res. 2018, 111, 1455–1467. [Google Scholar] [CrossRef] [PubMed]
- Hassan, A.-E.S.; Du, Y.; Lee, S.Y.; Wang, A.; Farmer, D.L. Spina Bifida: A Review of the Genetics, Pathophysiology and Emerging Cellular Therapies. J. Dev. Biol. 2022, 10, 22. [Google Scholar] [CrossRef] [PubMed]
- About Spina Bifida. Available online: https://www.nichd.nih.gov/health/topics/spinabifida/conditioninfo (accessed on 23 January 2024).
- How Do Healthcare Providers Diagnose Spina Bifida? Available online: https://www.nichd.nih.gov/health/topics/spinabifida/conditioninfo/diagnose (accessed on 23 January 2024).
- Song, R.B.; Glass, E.N.; Kent, M. Spina Bifida, Meningomyelocele, and Meningocele. Vet. Clin. N. Am. Small Anim. Pract. 2016, 46, 327–345. [Google Scholar] [CrossRef] [PubMed]
- Muñiz, L.M.; Del Magno, S.; Gandini, G.; Pisoni, L.; Menchetti, M.; Foglia, A.; Ródenas, S. Surgical Outcomes of Six Bulldogs with Spinal Lumbosacral Meningomyelocele or Meningocele. Vet. Surg. 2019, 49, 200–206. [Google Scholar] [CrossRef] [PubMed]
- Piatt, J.H. Treatment of Myelomeningocele: A Review of Outcomes and Continuing Neurosurgical Considerations among Adults. J. Neurosurg. 2010, 6, 515–525. [Google Scholar] [CrossRef] [PubMed]
- Copp, A.J.; Adzick, N.S.; Chitty, L.S.; Fletcher, J.Μ.; Holmbeck, G.N.; Shaw, G.M. Spina Bifida. Nat. Rev. Dis. Primers 2015, 1, 15007. [Google Scholar] [CrossRef]
- Bibbins-Domingo, K.; Grossman, D.C.; Curry, S.J.; Davidson, K.W.; Epling, J.W.; García, F.; Kemper, A.R.; Krist, A.H.; Kurth, A.; Landefeld, C.S.; et al. Folic Acid Supplementation for the Prevention of Neural Tube Defects. JAMA 2017, 317, 183. [Google Scholar] [CrossRef]
- Spina Bifida Data and Statistics|CDC. Centers for Disease Control and Prevention. Available online: https://www.cdc.gov/ncbddd/spinabifida/data.html (accessed on 23 January 2024).
- Spina Bifida—Diagnosis and Treatment—Mayo Clinic. Available online: https://www.mayoclinic.org/diseases-conditions/spina-bifida/diagnosis-treatment/drc-20377865 (accessed on 23 January 2024).
- Adzick, N.S.; Thom, E.; Spong, C.Y.; Brock, J.W.; Burrows, P.K.; Johnson, M.P.; Howell, L.J.; Farrell, J.A.; Dabrowiak, M.E.; Sutton, L.N.; et al. A Randomized Trial of Prenatal versus Postnatal Repair of Myelomeningocele. N. Engl. J. Med. 2011, 364, 993–1004. [Google Scholar] [CrossRef]
- Moldenhauer, J.S.; Soni, S.; Rintoul, N.E.; Spinner, S.S.; Khalek, N.; Martinez-Poyer, J.; Flake, A.W.; Hedrick, H.L.; Peranteau, W.H.; Rendon, N.; et al. Fetal Myelomeningocele Repair: The Post-MOMS Experience at the Children’s Hospital of Philadelphia. Fetal Diagn. Ther. 2014, 37, 235–240. [Google Scholar] [CrossRef]
- Cortés, M.S.; Chmait, R.H.; Lapa, D.A.; Belfort, M.A.; Carreras, E.; Miller, J.L.; Brawura-Biskupski-Samaha, R.; González, G.S.; Gielchinsky, Y.; Yamamoto, M.; et al. Experience of 300 Cases of Prenatal Fetoscopic Open Spina Bifida Repair: Report of the International Fetoscopic Neural Tube Defect Repair Consortium. Am. J. Obstet. Gynecol. 2021, 225, 678.e1–678.e11. [Google Scholar] [CrossRef]
- Tatu, R.; Oria, M.; Pulliam, S.; Signey, L.; Rao, M.B.; Peiró, J.L.; Lin, C. Using Poly(L-lactic Acid) and Poly(Ɛ-caprolactone) Blends to Fabricate Self-expanding, Watertight and Biodegradable Surgical Patches for Potential Fetoscopic Myelomeningocele Repair. J. Biomed. Mater. Res. Part B Appl. Biomater. 2018, 107, 295–305. [Google Scholar] [CrossRef]
- Oria, M.; Tatu, R.; Lin, C.; Peiró, J.L. In Vivo Evaluation of Novel PLA/PCL Polymeric Patch in Rats for Potential Spina Bifida Coverage. J. Surg. Res. 2019, 242, 62–69. [Google Scholar] [CrossRef]
- Tatu, R.; Oria, M.; Rao, M.B.; Peiró, J.L.; Lin, C. Biodegradation of Poly(l-Lactic Acid) and Poly(ε-Caprolactone) Patches by Human Amniotic Fluid in an in-Vitro Simulated Fetal Environment. Sci. Rep. 2022, 12, 3950. [Google Scholar] [CrossRef]
- Bonate, P.L. A Brief Introduction to Monte Carlo Simulation. Clin. Pharmacokinet. 2001, 40, 15–22. [Google Scholar] [CrossRef]
- Martins, M.T.; Lourenço, F.R. Measurement Uncertainty for <905> Uniformity of Dosage Units Tests Using Monte Carlo and Bootstrapping Methods—Uncertainties Arising from Sampling and Analytical Steps. J. Pharm. Biomed. Anal. 2024, 238, 115857. [Google Scholar] [CrossRef]
- Lecina, D.; Gilabert, J.F.; Guallar, V. Adaptive Simulations, towards Interactive Protein-Ligand Modeling. Sci. Rep. 2017, 7, 8466. [Google Scholar] [CrossRef]
- Bailer, A.J.; Ruberg, S.J. Randomization tests for assessing the equality of area under curves for studies using destructive sampling. J. Appl. Toxicol. 1996, 16, 391–395. [Google Scholar] [CrossRef]
- Holder, D.J.; Hsuan, F.; Dixit, R.; Soper, K. A method for estimating and testing area under the curve in serial sacrifice, batch, and complete data designs. J. Biopharm. Stat. 1999, 9, 451–464. [Google Scholar] [CrossRef] [PubMed]
- Wolfsegger, M.J.; Jaki, T. Estimation of AUC from 0 to infinity in serial sacrifice designs. J. Pharmacokinet. Pharmacodyn. 2005, 32, 757–766. [Google Scholar] [CrossRef] [PubMed]
- Rabbee, N. Biomarker Analysis in Clinical Trials with R, 1st ed.; CRC Press: Boca Raton, FL, USA; Taylor & Francis Group: Abingdon, UK, 2020. [Google Scholar] [CrossRef]
- Rubinstein, R.Y.; Kroese, D.P. Simulation and the Monte Carlo Method, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar] [CrossRef]
- Dykstra, R.L. Product Inequalities Involving the Multivariate Normal Distribution. J. Am. Stat. Assoc. 1980, 75, 646–650. [Google Scholar] [CrossRef]
- Tong, Y.L. Some Probability Inequalities of Multivariate Normal and Multivariate t. J. Am. Stat. Assoc. 1970, 65, 1243–1247. [Google Scholar] [CrossRef]
- Tong, Y.L. Probability Inequalities in Multivariate Distributions. J. Am. Stat. Assoc. 1982, 77, 690. [Google Scholar] [CrossRef]
- Ripley, B.D. Ohio Library and Information Network. In Stochastic Simulation; Wiley: New York, NY, USA, 1987; p. 28. [Google Scholar]


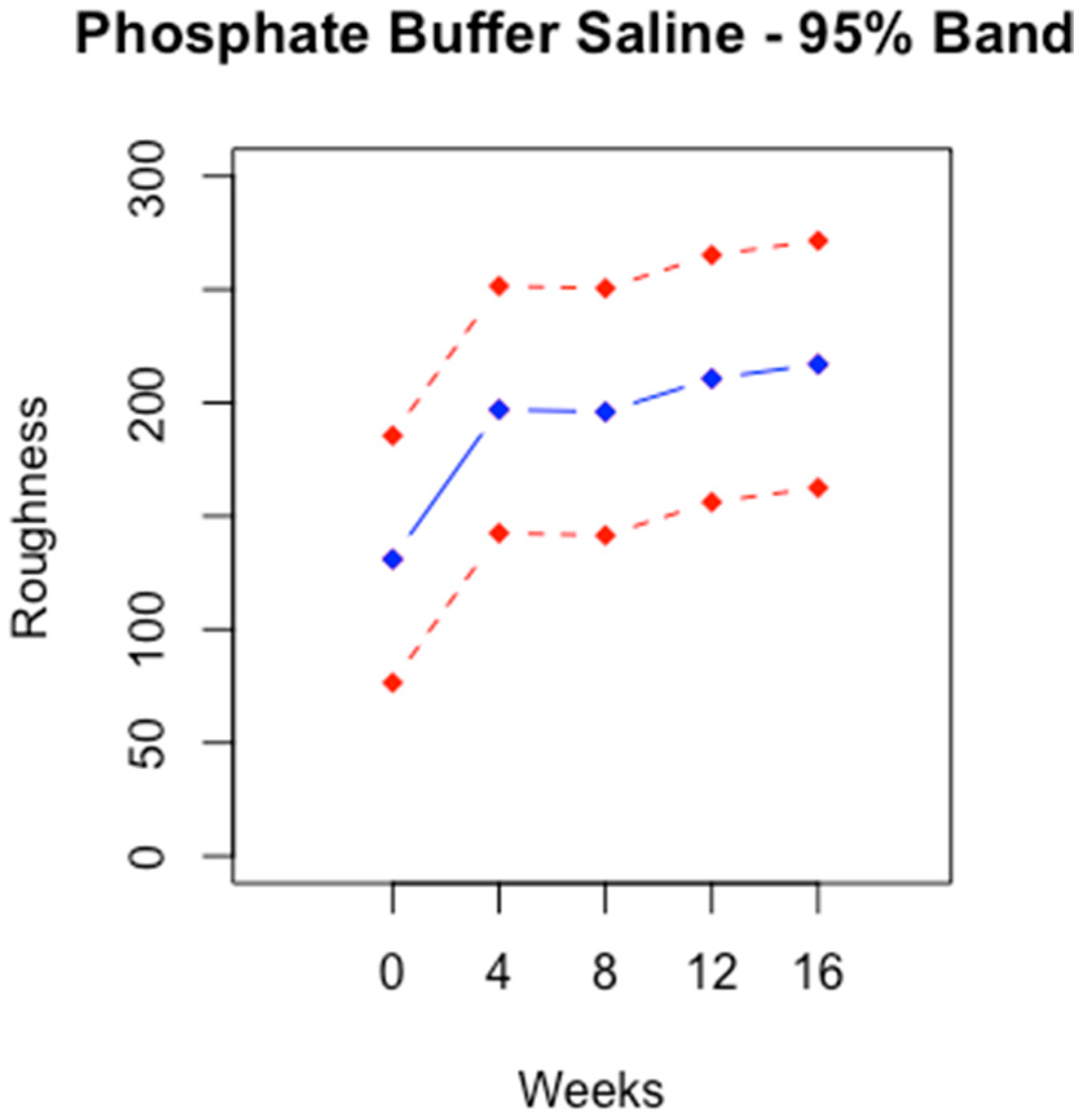
| Roughness | |||
|---|---|---|---|
| Week | Baseline | AF | PBS |
| 0 | 139 | ||
| 0 | 122 | ||
| 0 | 132 | ||
| 4 | 223 | 177 | |
| 4 | 267 | 202 | |
| 4 | 217 | 212 | |
| 8 | 245 | 185 | |
| 8 | 269 | 198 | |
| 8 | 257 | 205 | |
| 12 | 265 | 167 | |
| 12 | 283 | 217 | |
| 12 | 285 | 248 | |
| 16 | 306 | 224 | |
| 16 | 247 | 198 | |
| 16 | 320 | 229 | |
| Week | Baseline | AF | PBS | |||
|---|---|---|---|---|---|---|
| Mean | SD | Mean | SD | Mean | SD | |
| 0 | 131 | 8.54 | ||||
| 4 | 235.67 | 27.3 | 197 | 18.03 | ||
| 8 | 257 | 12 | 196 | 10.15 | ||
| 12 | 277.67 | 11.02 | 210.67 | 40.87 | ||
| 16 | 291 | 38.74 | 217 | 16.64 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, T.; Tatu, R.; Wima, K.; Oria, M.; Peiro, J.L.; Lin, C.-Y.; Rao, M.B. Profile of a Multivariate Observation under Destructive Sampling—A Monte Carlo Approach to a Case of Spina Bifida. Bioengineering 2024, 11, 249. https://doi.org/10.3390/bioengineering11030249
Guan T, Tatu R, Wima K, Oria M, Peiro JL, Lin C-Y, Rao MB. Profile of a Multivariate Observation under Destructive Sampling—A Monte Carlo Approach to a Case of Spina Bifida. Bioengineering. 2024; 11(3):249. https://doi.org/10.3390/bioengineering11030249
Chicago/Turabian StyleGuan, Tianyuan, Rigwed Tatu, Koffi Wima, Marc Oria, Jose L. Peiro, Chia-Ying Lin, and Marepalli. B. Rao. 2024. "Profile of a Multivariate Observation under Destructive Sampling—A Monte Carlo Approach to a Case of Spina Bifida" Bioengineering 11, no. 3: 249. https://doi.org/10.3390/bioengineering11030249
APA StyleGuan, T., Tatu, R., Wima, K., Oria, M., Peiro, J. L., Lin, C.-Y., & Rao, M. B. (2024). Profile of a Multivariate Observation under Destructive Sampling—A Monte Carlo Approach to a Case of Spina Bifida. Bioengineering, 11(3), 249. https://doi.org/10.3390/bioengineering11030249







