Novel Multivariable Evolutionary Algorithm-Based Method for Modal Reconstruction of the Corneal Surface from Sparse and Incomplete Point Clouds
Abstract
1. Introduction
2. Materials and Methods
2.1. Data Source
2.2. Data Acquisition
2.3. NS-MVGA-Based Modal Reconstruction
2.3.1. Discretization
2.3.2. Fitting of the Modal Surface Function
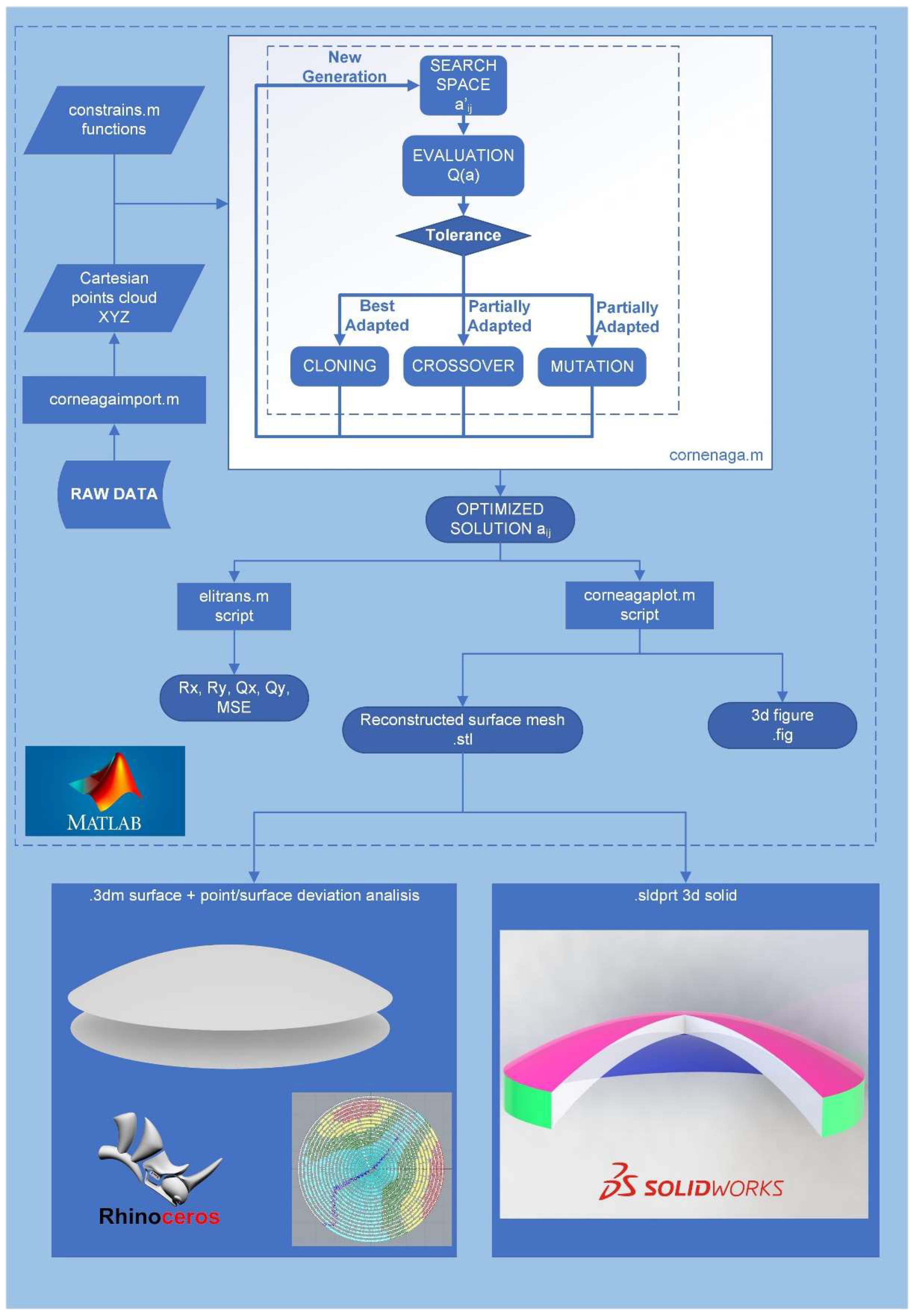
2.3.3. Reconstruction of the Corneal Surface Using NS-MVGA Algorithm CORNEAGA
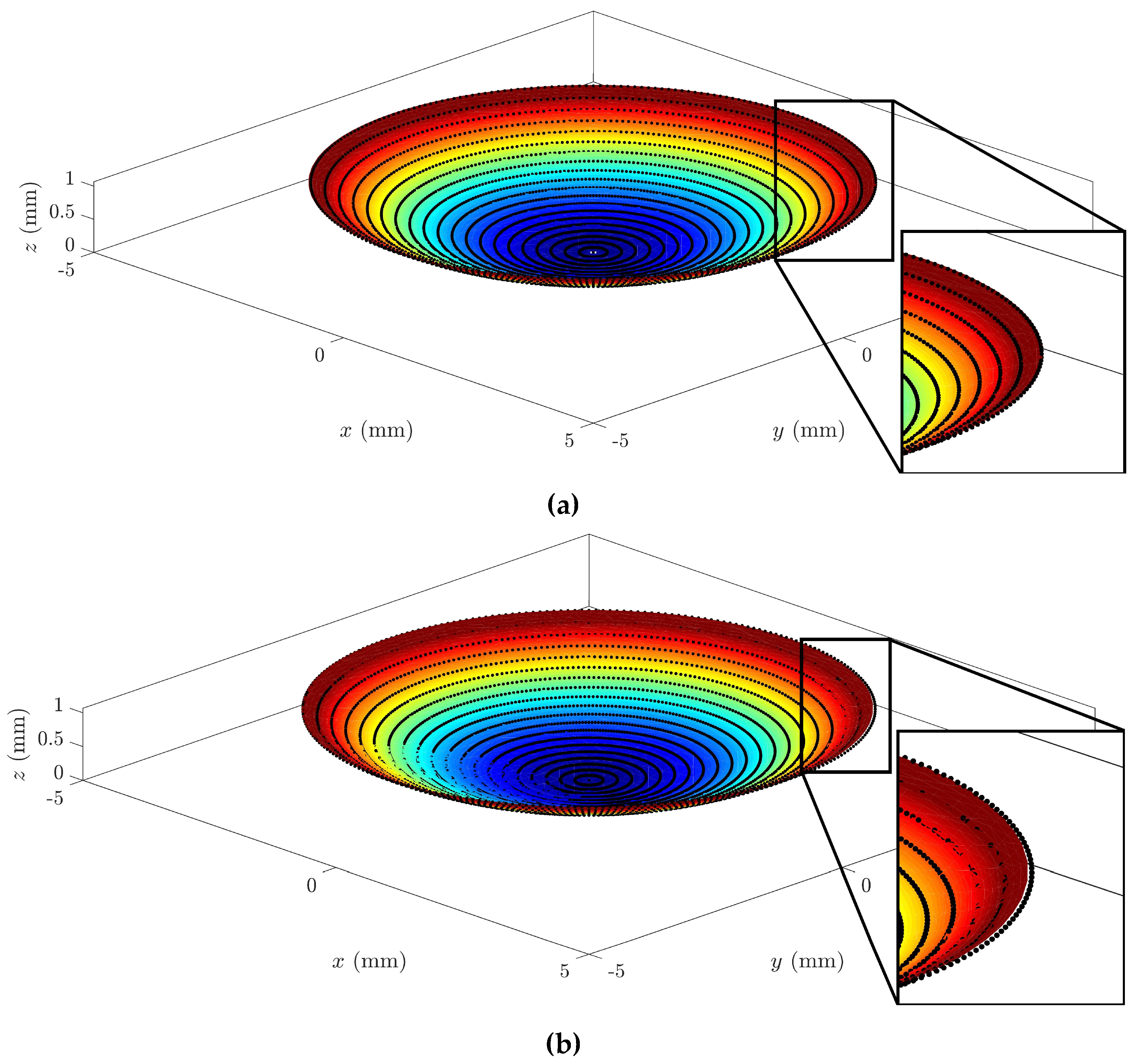
2.3.4. Obtaining the Reconstructed Surface, Morpho-Geometric Parameters, and Graphical Representation
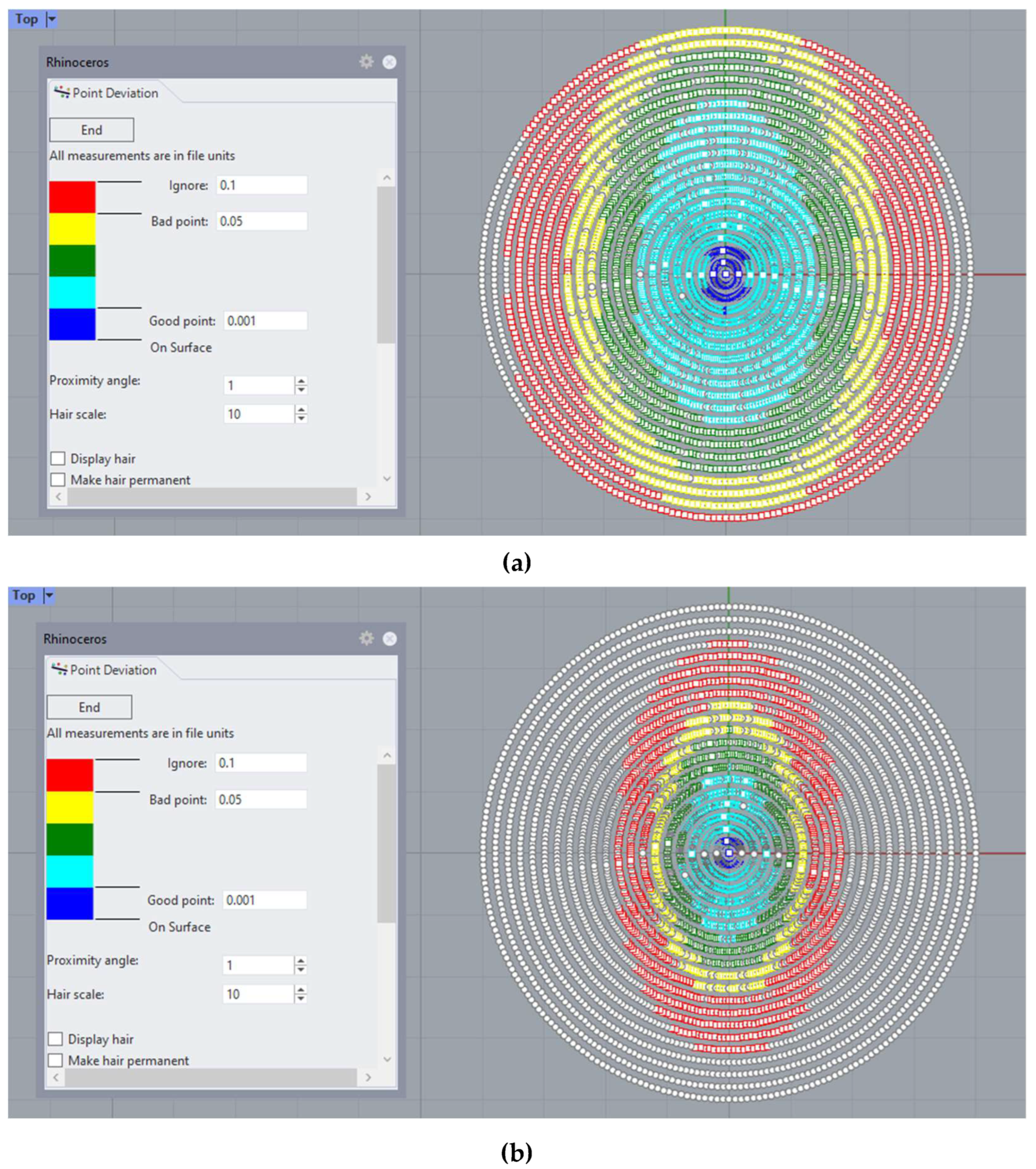
2.3.5. Method Validation and Error Calculation
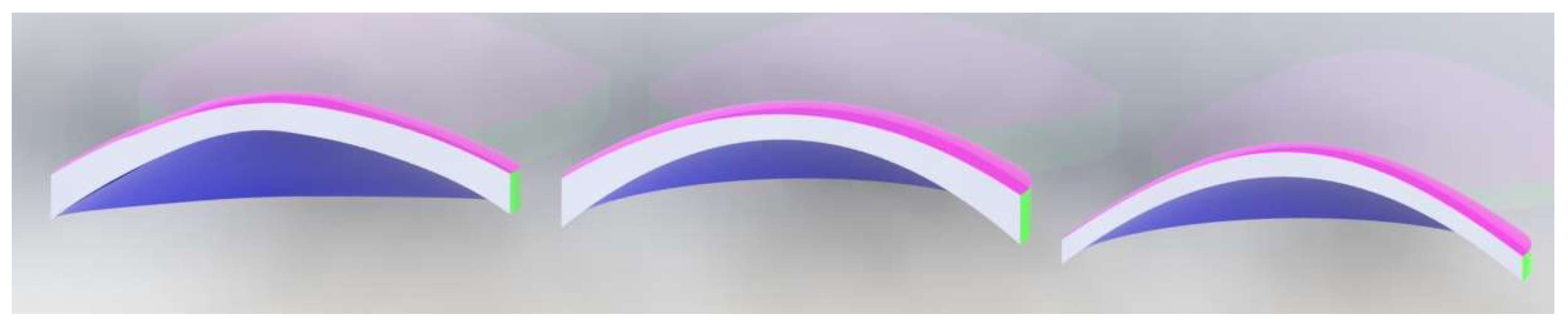
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ribeiro, F.J.; Castanheira-Dinis, A.; Dias, J.M. Personalized Pseudophakic Model for Refractive Assessment. PLoS ONE 2012, 7, e46780. [Google Scholar] [CrossRef]
- Toprak, I.; Cavas, F.; Velázquez, J.S.; Alió del Barrio, J.L.; Alió, J.L. Three-Dimensional Morphogeometric and Volumetric Characterization of Cornea in Pediatric Patients With Early Keratoconus. Am. J. Ophthalmol. 2021, 222, 102–111. [Google Scholar] [CrossRef] [PubMed]
- Zhou, D.; Abass, A.; Eliasy, A.; Studer, H.P.; Movchan, A.; Movchan, N.; Elsheikh, A. Microstructure-based numerical simulation of the mechanical behaviour of ocular tissue. J. R. Soc. Interface 2019, 16, 20180685. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Abad, A.; Piñero, D.P.; Ruiz-Fortes, P.; Artola, A. Evaluation of the diagnostic ability of vector parameters characterizing the corneal astigmatism and regularity in clinical and subclinical keratoconus. Contact Lens Anterior Eye 2017, 40, 88–96. [Google Scholar] [CrossRef] [PubMed]
- Nicula, C.A.; Bulboacă, A.E.; Nicula, D.; Nicula, A.P.; Horvath, K.U.; Bolboacă, S.D. Performances of Corneal Topography and Tomography in the Diagnosis of Subclinical and Clinical Keratoconus. Front. Med. 2022, 9, 904604. [Google Scholar] [CrossRef]
- Shiga, S.; Kojima, T.; Nishida, T.; Nakamura, T.; Ichikawa, K. Evaluation of CorvisST biomechanical parameters and anterior segment optical coherence tomography for diagnosing forme fruste keratoconus. Acta Ophthalmol. 2021, 99, 644–651. [Google Scholar] [CrossRef]
- Ares, M.; Royo, S. Comparison of cubic B-spline and Zernike-fitting techniques in complex wavefront reconstruction. Appl. Opt. 2006, 45, 6954–6964. [Google Scholar] [CrossRef] [PubMed]
- Hall, R.C.; Mohamed, F.K.; Htoon, H.M.; Tan, D.T.; Mehta, J.S. Laser in situ keratomileusis flap measurements: Comparison between observers and between spectral-domain and time-domain anterior segment optical coherence tomography. J. Cataract Refract. Surg. 2011, 37, 544–551. [Google Scholar] [CrossRef]
- Kosaki, R.; Maeda, N.; Bessho, K.; Hori, Y.; Nishida, K.; Suzaki, A.; Hirohara, Y.; Mihashi, T.; Fujikado, T.; Tano, Y. Magnitude and orientation of Zernike terms in patients with keratoconus. Investig. Ophthalmol. Vis. Sci. 2007, 48, 3062–3068. [Google Scholar] [CrossRef]
- Trevino, J.P.; Gómez-Correa, J.E.; Iskander, D.R.; Chávez-Cerda, S. Zernike vs. Bessel circular functions in visual optics. Ophthalmic Physiol. Opt. 2013, 33, 394–402. [Google Scholar] [CrossRef]
- Williams, D.; Zheng, Y.; Bao, F.; Elsheikh, A. Automatic segmentation of anterior segment optical coherence tomography images. J. Biomed. Opt. 2013, 18, 056003. [Google Scholar] [CrossRef] [PubMed]
- Cavas-Martínez, F.; De la Cruz Sánchez, E.; Nieto Martínez, J.; Fernández Cañavate, F.J.; Fernández-Pacheco, D.G. Corneal topography in keratoconus: State of the art. Eye Vis. 2016, 3, 5. [Google Scholar] [CrossRef]
- Ramos-López, D.; Martínez-Finkelshtein, A.; Castro-Luna, G.M.; Piñero, D.; Alió, J.L. Placido-based indices of corneal irregularity. Optom. Vis. Sci. 2011, 88, 1220–1231. [Google Scholar] [CrossRef]
- Martínez-Finkelshtein, A.; López, D.R.; Castro, G.M.; Alió, J.L. Adaptive cornea modeling from keratometric data. Investig. Ophthalmol. Vis. Sci. 2011, 52, 4963–4970. [Google Scholar] [CrossRef] [PubMed]
- Landi, G.; Spinelli, G.V.; Zama, F.; Martino, D.C.; Conte, P.; Lo Meo, P.; Bortolotti, V. An automatic L1-based regularization method for the analysis of FFC dispersion profiles with quadrupolar peaks. Appl. Math. Comput. 2023, 444, 127809. [Google Scholar] [CrossRef]
- Butler, A.; Kwon, R.H. Efficient differentiable quadratic programming layers: An ADMM approach. Comput. Optim. Appl. 2023, 84, 449–476. [Google Scholar] [CrossRef]
- Carvalho, L.A. Accuracy of Zernike polynomials in characterizing optical aberrations and the corneal surface of the eye. Investig. Ophthalmol. Vis. Sci. 2005, 46, 1915–1926. [Google Scholar] [CrossRef]
- Smolek, M.K.; Klyce, S.D. Zernike Polynomial Fitting Fails to Represent All Visually Significant Corneal Aberrations. Investig. Ophthalmol. Vis. Sci. 2003, 44, 4676–4681. [Google Scholar] [CrossRef]
- Vicent, L.E.; Wolf, B. Unitary transformation between Cartesian- and polar-pixellated screens. J. Opt. Soc. Am. A 2008, 25, 1875–1884. [Google Scholar] [CrossRef]
- Da, D.C.; Cui, X.Y.; Long, K.; Li, G.Y. Concurrent topological design of composite structures and the underlying multi-phase materials. Comput. Struct. 2017, 179, 1–14. [Google Scholar] [CrossRef]
- Gunpinar, E.; Gunpinar, S. A shape sampling technique via particle tracing for CAD models. Graph. Models 2018, 96, 11–29. [Google Scholar] [CrossRef]
- Zou, X.; Cheng, P.; An, B.; Song, J. Sectorization and Configuration Transition in Airspace Design. Math. Probl. Eng. 2016, 2016, 6048326. [Google Scholar] [CrossRef]
- Alió, J.L.; Piñero, D.P.; Alesón, A.; Teus, M.A.; Barraquer, R.I.; Murta, J.; Maldonado, M.J.; Castro De Luna, G.; Gutiérrez, R.; Villa, C.; et al. Keratoconus-integrated characterization considering anterior corneal aberrations, internal astigmatism, and corneal biomechanics. J. Cataract Refract. Surg. 2011, 37, 552–568. [Google Scholar] [CrossRef] [PubMed]
- Piñero, D.P.; Nieto, J.C.; Lopez-Miguel, A. Characterization of corneal structure in keratoconus. J. Cataract Refract. Surg. 2012, 38, 2167–2183. [Google Scholar] [CrossRef] [PubMed]
- Rabinowitz, Y.S. Keratoconus. Surv. Ophthalmol. 1998, 42, 297–319. [Google Scholar] [CrossRef]
- Cavas-Martínez, F.; Bataille, L.; Fernández-Pacheco, D.G.; Cañavate, F.J.F.; Alió, J.L. A new approach to keratoconus detection based on corneal morphogeometric analysis. PLoS ONE 2017, 12, e0184569. [Google Scholar] [CrossRef]
- Navarro, R.; González, L.; Hernández, J.L. Optics of the average normal cornea from general and canonical representations of its surface topography. J. Opt. Soc. Am. A 2006, 23, 219–232. [Google Scholar] [CrossRef]
- Ding, S.; Ye, Y.; Tu, J.; Subic, A. Region-based geometric modelling of human airways and arterial vessels. Comput. Med. Imaging Graph. 2010, 34, 114–121. [Google Scholar] [CrossRef]
- Duan, C.Y.; Lü, H.B.; Hu, J.Z. In vivo study on three-dimensional structure of lumbar facet joints based on computer-assisted medical image processing method. Yiyong Shengwu Lixue 2012, 27, 159–165. [Google Scholar]
- Martinez-Finkelshtein, A.; Delgado, A.M.; Castro, G.M.; Zarzo, A.; Alio, J.L. Comparative analysis of some modal reconstruction methods of the shape of the cornea from corneal elevation data. Investig. Ophthalmol. Vis. Sci. 2009, 50, 5639–5645. [Google Scholar] [CrossRef]
- Alonso-Caneiro, D.; Iskander, D.R.; Collins, M.J. Estimating corneal surface topography in videokeratoscopy in the presence of strong signal interference. IEEE Trans. Biomed. Eng. 2008, 55, 2381–2387. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Sáez-Gutiérrez, F.L.; Velázquez, J.S.; Mira, J.; Gómez, C.; Alió, J.; Cavas, F. Geometric Reconstruction of a Biological Structure by Explicit Modal Methods. A Case-Study in Human Corneal Surface. In Advances in Design Engineering III, Proceedings of the Lecture Notes in Mechanical Engineering, Málaga, Spain, 29–30 June, 1 July 2022; Springer Science and Business Media Deutschland GmbH: Berlin, Germany, 2023; pp. 531–538. [Google Scholar]
- Bao, F.; Chen, H.; Yu, Y.; Yu, J.; Zhou, S.; Wang, J.; Wang, Q.; Elsheikh, A. Evaluation of the Shape Symmetry of Bilateral Normal Corneas in a Chinese Population. PLoS ONE 2013, 8, e73412. [Google Scholar] [CrossRef] [PubMed]
- Sáez-Gutiérrez, F.L.; Velázquez, J.S.; del Barrio, J.L.A.; Alió, J.L.; Cavas, F. Analysis of the Use of Genetic Algorithms in the Design of Models and Graphical Techniques for Early Detection, Diagnosis, and Characterization of Clinical Pathologies. In Advances in Design Engineering II, Proceedings of the Lecture Notes in Mechanical Engineering, Valencia, Spain, 24–25 June 2021; Springer Science and Business Media Deutschland GmbH: Berlin, Germany, 2022; pp. 201–207. [Google Scholar]
- Bevilacqua, V.; Simeone, S.; Brunetti, A.; Loconsole, C.; Trotta, G.F.; Tramacere, S.; Argentieri, A.; Ragni, F.; Criscenti, G.; Fornaro, A.; et al. A computer aided ophthalmic diagnosis system based on tomographic features. In Intelligent Computing Methodologies, Proceedings of the Lecture Notes in Computer Science (Including Subseries Lecture Notes in Artificial Intelligence and Lecture Notes in Bioinformatics), 13th International Conference, ICIC 2017, Liverpool, UK, 7–10 August 2017; Springer Verlag: Berlin, Germany, 2017; pp. 598–609. [Google Scholar]
- Williams, D.; Zheng, Y.; Davey, P.G.; Bao, F.; Shen, M.; Elsheikh, A. Reconstruction of 3D surface maps from anterior segment optical coherence tomography images using graph theory and genetic algorithms. Biomed. Signal Process. Control 2016, 25, 91–98. [Google Scholar] [CrossRef]
- Edelsbrunner, H.; Mücke, E.P. Three-Dimensional Alpha Shapes. ACM Trans. Graph. 1994, 13, 43–72. [Google Scholar] [CrossRef]
- Shewchuk, J.R. Triangle: Engineering a 2D quality mesh generator and delaunay triangulator. In Applied Computational Geometry. Towards Geometric Engineering, Proceedings of the Lecture Notes in Computer Science (Including Subseries Lecture Notes in Artificial Intelligence and Lecture Notes in Bioinformatics), Philadelphia, PA, USA, 27–28 May1996; Springer Verlag: Berlin, Germany, 1996; pp. 203–222. [Google Scholar]
- Belin, M.W.; Duncan, J.K. Keratoconus: The ABCD Grading System. Klin. Monatsbl. Augenheilkd. 2016, 233, 701–707. [Google Scholar] [CrossRef]
- Bao, F.; Savini, G.; Shu, B.; Zhu, S.; Gao, R.; Dang, G.; Yu, A.; Wang, Q.; Huang, J. Repeatability, reproducibility, and agreement of two scheimpflug-placido anterior corneal analyzers for posterior corneal surface measurement. J. Refract. Surg. 2017, 33, 524–530. [Google Scholar] [CrossRef]
- Montalbán, R.; Piñero, D.P.; Javaloy, J.; Alió, J.L. Intrasubject repeatability of corneal morphology measurements obtained with a new Scheimpflug photography-based system. J. Cataract Refract. Surg. 2012, 38, 971–977. [Google Scholar] [CrossRef]
- Savini, G.; Schiano-Lomoriello, D.; Hoffer, K.J. Repeatability of automatic measurements by a new anterior segment optical coherence tomographer combined with Placido topography and agreement with 2 Scheimpflug cameras. J. Cataract Refract. Surg. 2018, 44, 471–478. [Google Scholar] [CrossRef]
- Montalbán, R.; Alió, J.L.; Javaloy, J.; Piñero, D.P. Intrasubject repeatability in keratoconus-eye measurements obtained with a new Scheimpflug photography-based system. J. Cataract Refract. Surg. 2013, 39, 211–218. [Google Scholar] [CrossRef]
- Kanellopoulos, A.J.; Asimellis, G. Revisiting keratoconus diagnosis and progression classification based on evaluation of corneal asymmetry indices, derived from scheimpflug imaging in keratoconic and suspect cases. Clin. Ophthalmol. 2013, 7, 1539–1548. [Google Scholar] [CrossRef]
- Holladay, J.T. Corneal topography using the Holladay diagnostic summary. J. Cataract Refract. Surg. 1997, 23, 209–221. [Google Scholar] [CrossRef] [PubMed]
- Cavas-Martínez, F.; Fernández-Pacheco, D.G.; De La Cruz-Sánchez, E.; Nieto Martínez, J.; Fernández Cañavate, F.J.; Vega-Estrada, A.; Plaza-Puche, A.B.; Alió, J.L. Geometrical custom modeling of human cornea in vivo and its use for the diagnosis of corneal ectasia. PLoS ONE 2014, 9, e110249. [Google Scholar] [CrossRef] [PubMed]

| Function Tolerance Exponent | Constrain Tolerance Exponent | MSE (10−3) | Processing Time (s) 1 |
|---|---|---|---|
| −2 | −2 | 5.256 | 32 |
| −10 | −10 | 4.6522 | 65 |
| −30 | −30 | 0.0804 | 299 |
| −50 | −50 | 0.0117 | 299 |
| −100 | −1000 | 0.0804 | 1972 |
| Method | Control (SD) | AK Grade 1 (SD) | AK Grade 2 (SD) | AK Grade 3 + 4 (SD) |
|---|---|---|---|---|
| LSQ | 8.96 × 10−3 (5.25 × 10−4) | 6.83 × 10−3 (1.7 × 10−3) | 6.03 × 10−3 (8.54 × 10−4) | 6.79 × 10−3 (5.06 × 10−4) |
| SQP | 1.08 × 10−2 (5.78 × 10−2) | 5.91 × 10−1 (1.14 x10−1) | 7.48 × 10−1 (3.40 x10−1) | 5.08 × 10−1 (5.44 x10−1) |
| CORNEAGA | 1.24 × 10−3 (2.57 × 10−4) | 2.89 × 10−3 (2.74 × 10−4) | 2.86 × 10−3 (1.89 × 10−4) | 2.88 × 10−3 (1.69 × 10−4) |
| Method | Control | AK Grade 1 | AK Grade 2 | AK Grade 3 + 4 |
|---|---|---|---|---|
| LSQ | 5 | 8 | 19 | 11 |
| SQP | 25 | 18 | 13 | 2 |
| CORNEAGA | 0 | 0 | 0 | 0 |
| METHOD | Control (SD) | AK Grade 1 (SD) | AK Grade 2 (SD) | AK Grade 3 + 4 (SD) |
|---|---|---|---|---|
| LSQ | −0.3114 (0.1911) | −0.6587 (0.3598) | −0.8206 (0.3837) | −1.1602 (0.5122) |
| SQP | −0.8216 (0.1636) | −0.8827 (0.2542) | −0.9062 (0.2041) | −1.1602 (0.2214) |
| CORNEAGA | −0.3348 (0.1637) | −0.6190 (0.2845) | −0.7344 (0.2418) | −0.8202 (0.2241) |
| METHOD | AK Grade 1 (SD) | AK Grade 2 (SD) | AK Grade 3 + 4 (SD) |
|---|---|---|---|
| LSQ | 6.89/5.41 (0.89/1.21) | 6.6/5.31 (0.56/1.07) | 5.43/4.39 (1.64/0.82) |
| SQP | 4.07/8.45 (5.15/15,38) | 2.81/4.04 (2.69/7.16) | 1.39/2.71 (2.27/1.63) |
| CORNEAGA | 7.18/5.78 (0.37/0.54) | 6.85/5.62 (0.43/0.43) | 6.7/5.12 (0.61/0.39) |
| AK criteria [belin2016] 1 | >7.05/>5.7 | >6.35/>5.15 | ~6.15/~4.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sáez-Gutiérrez, F.L.; Velázquez, J.S.; Alió del Barrio, J.L.; Alio, J.L.; Cavas, F. Novel Multivariable Evolutionary Algorithm-Based Method for Modal Reconstruction of the Corneal Surface from Sparse and Incomplete Point Clouds. Bioengineering 2023, 10, 989. https://doi.org/10.3390/bioengineering10080989
Sáez-Gutiérrez FL, Velázquez JS, Alió del Barrio JL, Alio JL, Cavas F. Novel Multivariable Evolutionary Algorithm-Based Method for Modal Reconstruction of the Corneal Surface from Sparse and Incomplete Point Clouds. Bioengineering. 2023; 10(8):989. https://doi.org/10.3390/bioengineering10080989
Chicago/Turabian StyleSáez-Gutiérrez, Francisco L., Jose S. Velázquez, Jorge L. Alió del Barrio, Jorge L. Alio, and Francisco Cavas. 2023. "Novel Multivariable Evolutionary Algorithm-Based Method for Modal Reconstruction of the Corneal Surface from Sparse and Incomplete Point Clouds" Bioengineering 10, no. 8: 989. https://doi.org/10.3390/bioengineering10080989
APA StyleSáez-Gutiérrez, F. L., Velázquez, J. S., Alió del Barrio, J. L., Alio, J. L., & Cavas, F. (2023). Novel Multivariable Evolutionary Algorithm-Based Method for Modal Reconstruction of the Corneal Surface from Sparse and Incomplete Point Clouds. Bioengineering, 10(8), 989. https://doi.org/10.3390/bioengineering10080989








