An Integrated Method of Biomechanics Modeling for Pelvic Bone and Surrounding Soft Tissues
Abstract
:1. Introduction
2. Materials and Methods
2.1. Pelvic Bone Modeling
2.1.1. Thickness of Cortical Bone
2.1.2. Material Properties Associated with Cortical and Cancellous Locations
2.1.3. Meshing
2.2. Modeling of Surrounding Soft Tissues
2.2.1. Modeling of Pelvic Ligaments
2.2.2. Modeling of Pelvic Joints and Cartilage
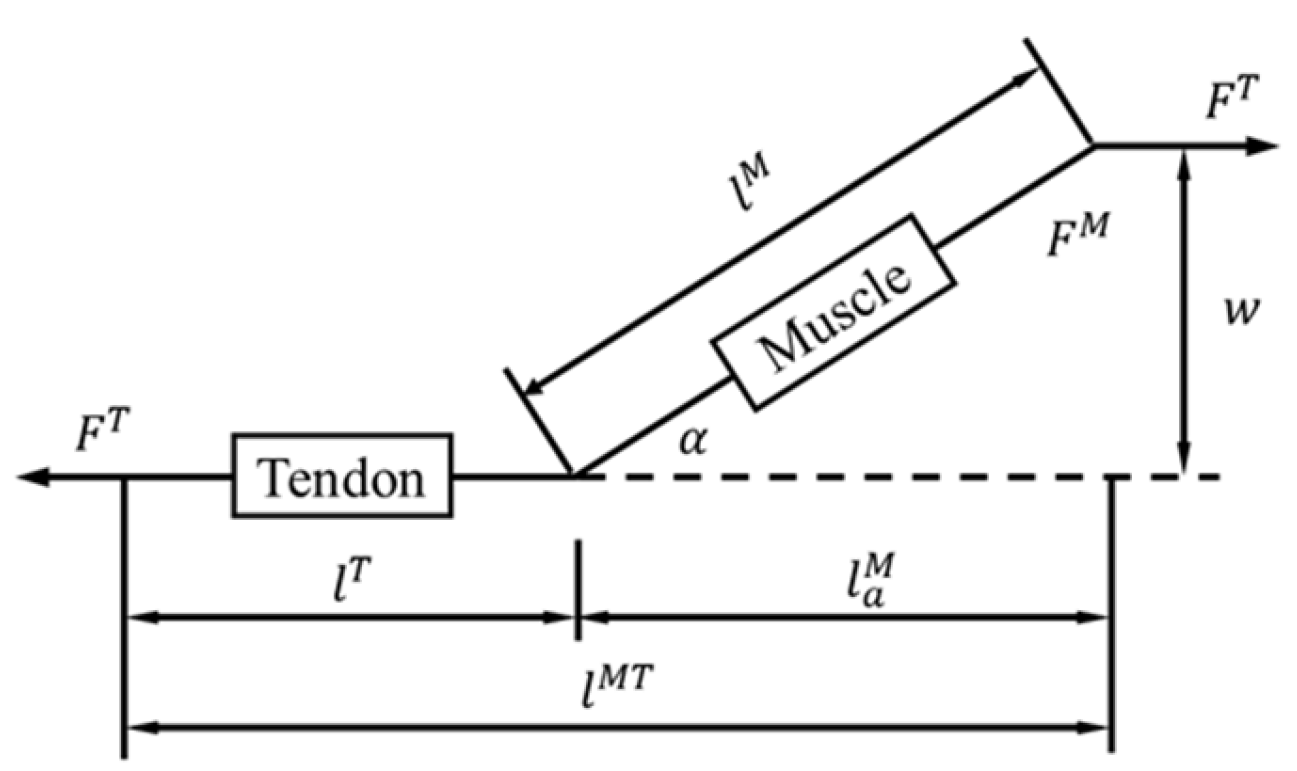
2.2.3. Pelvic Muscle Modeling
2.3. An Integrated Biomechanical Modelling Method for Pelvis with Surrounding Soft Tissues
| Component | Material Properties | |
|---|---|---|
| Bone Structures | Anderson et al. [32], Rho et al. [35], Dalstra et al. [36] | |
| Cortical bone thickness | 1.41 mm | |
| Young’s modulus of cortical bone | 17 GPa | |
| Young’s modulus of cancellous bone | Equations (1) and (2) | |
| Poisson’s ratio | 0.26 | |
| Material | Viscoelastic materials | |
| Mesh | Triangular surface, Tetrahedral meshing | |
| Ligaments | Phillips et al. [27], Eichenseer et al. [30], Zheng et al. [43], Hammer et al. [48,49,50] | |
| Component | Spring Stiffness (N/mm) | No. of springs 10 |
| Anterior sacroiliac ligaments | 230 | |
| Long posterior sacroiliac ligaments | 150 | 6 |
| Short posterior sacroiliac ligaments | 150 | 4 |
| Sacrospinous ligaments | 200 | 3 |
| Sacrotuberous ligaments | 80 | 6 |
| Iliolumbar ligaments | 200 | 5 |
| Inguinal ligaments | 250 | 1 |
| Supraspinous ligaments | 15 | 1 |
| Intertransverse ligaments | 15 | 1 |
| Muscle | Phillips et al. [28], Volinski et al. [58] | |
| Component | Spring Stiffness (N/mm) × Number | |
| Gluteus maximus | 962 × 5 | 4822 |
| Gluteus medius | - | - |
| Gluteus minimus | - | - |
| pectineus | 158 × 2 | 196 |
| Adductor magnus | 250 × 1 | 330 |
| Adductor longus | 67 × 2 | 95 |
| Piriformis | 168 × 1 | 60 |
| Gemellus superior | 198 × 3 | 667 |
| Gemellus inferior | - | - |
| Cartilage | Li et al. [38] | |
| Materials | Mooney–Rivlin hyperplastic material C10 = 0.1, C01 = 0.45, C11 = 1.67, ν = 0.2 |
3. Validation of the Finite Element Model of the Pelvis
3.1. Comparison Experiments of the Finite Element Model with and without Surrounding Sof Tissues
3.2. Comparison between the Finite Element Model Simulation and a Cadavic Experiment
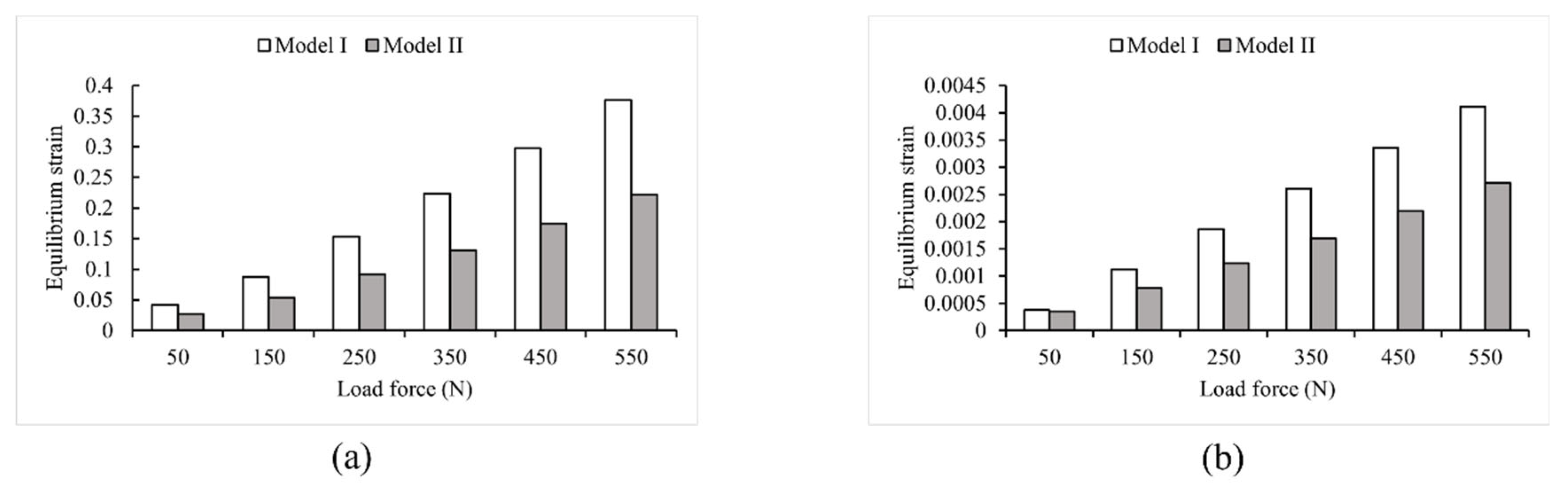
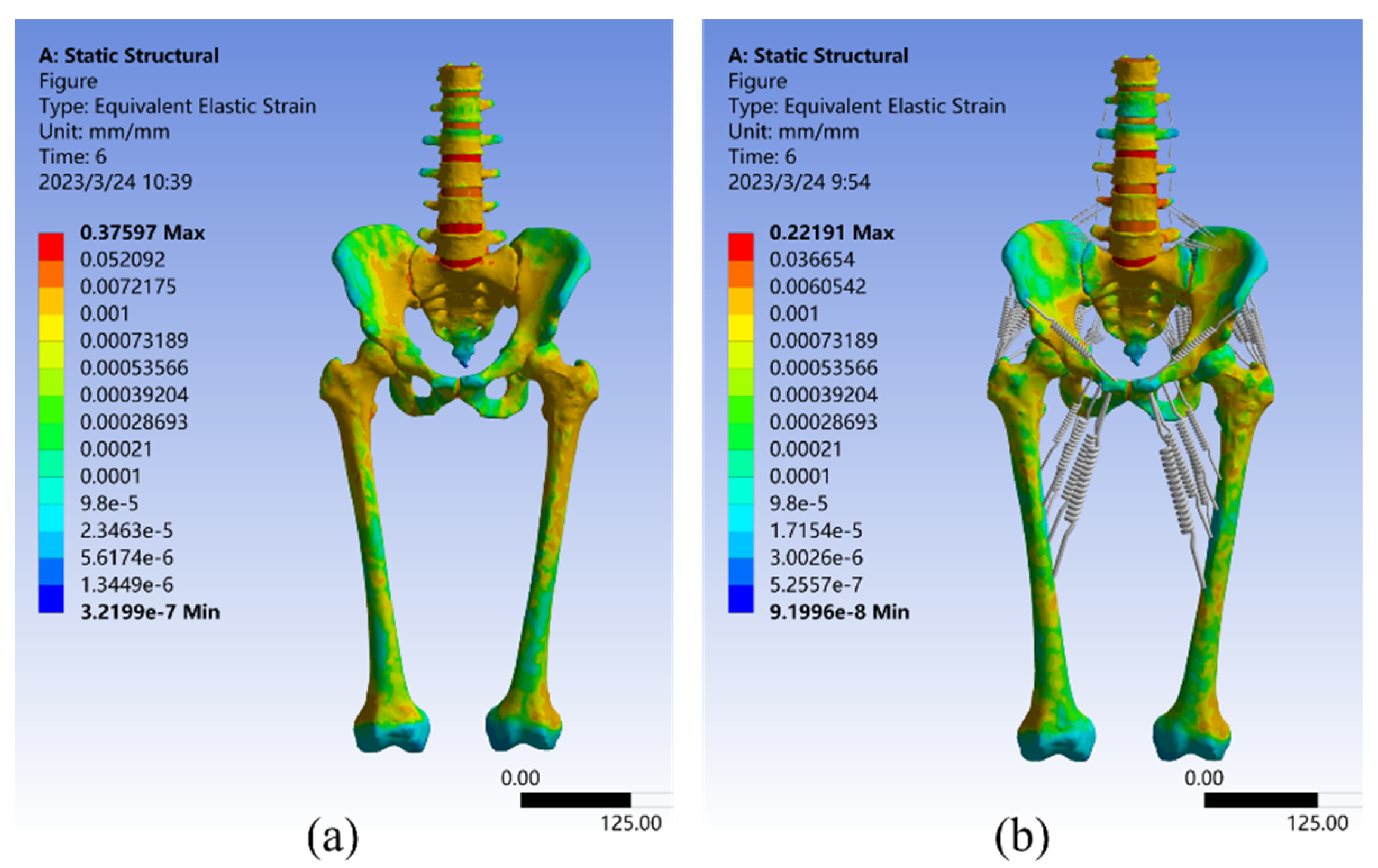
4. Results
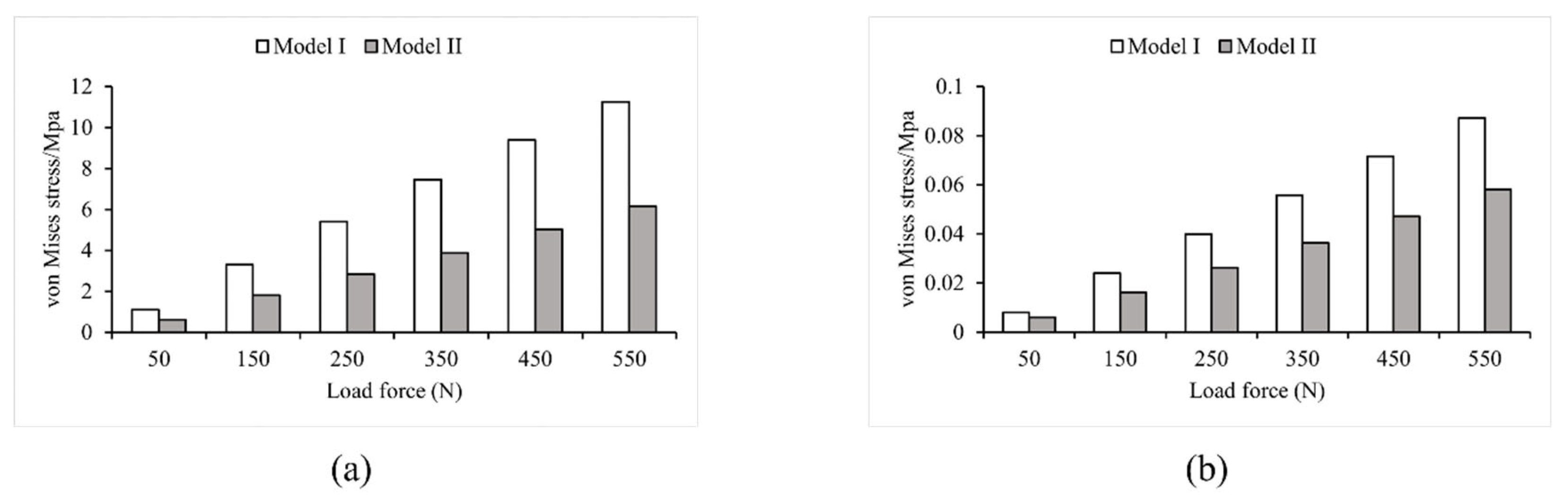
4.1. Model Stress Analysis
4.2. Model Strain Analysis
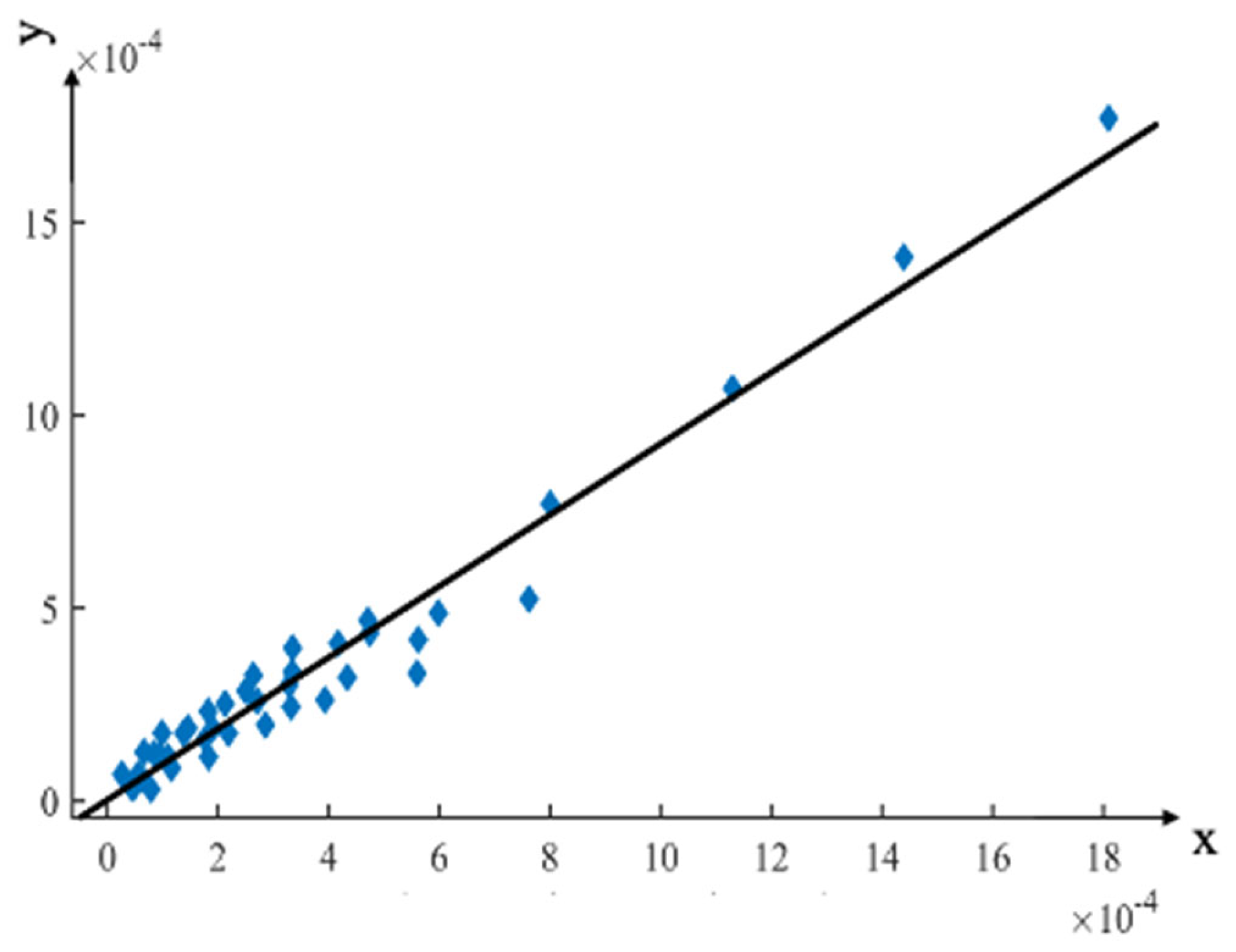
4.3. Linear Regression Analysis
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FE | Finite Element |
References
- Mi, M.; Kanakaris, N.K.; Wu, X.; Giannoudis, P.V. Management and Outcomes of Open Pelvic Fractures: An Update. Injury 2021, 52, 2738–2745. [Google Scholar] [CrossRef] [PubMed]
- Davis, D.D.; Foris, L.A.; Kane, S.M.; Waseem, M. Pelvic Fracture. In StatPearls; StatPearls Publishing: Treasure Island, FL, USA, 2022. [Google Scholar]
- Kannus, P.; Parkkari, J.; Niemi, S.; Sievänen, H. Low-Trauma Pelvic Fractures in Elderly Finns in 1970–2013. Calcif. Tissue Int. 2015, 97, 577–580. [Google Scholar] [CrossRef] [PubMed]
- Melhem, E.; Riouallon, G.; Habboubi, K.; Gabbas, M.; Jouffroy, P. Epidemiology of Pelvic and Acetabular Fractures in France. Orthop. Traumatol. Surg. Res. 2020, 106, 831–839. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Wang, T.; Li, C.; Tang, P.; Xu, Y.; Zhang, L.; Guo, N.; Zhao, Y.; Zhao, L.; Hu, L. Physical symmetry and virtual plane-based reduction reference: A preliminary study for robot-assisted pelvic fracture reduction. J. Mech. Med. Biol. 2016, 16, 1640014. [Google Scholar] [CrossRef]
- Zhang, L.; Zhao, J.; Zhao, Z.; Su, X.; Zhang, L.; Zhao, Y.; Tang, P. Computer-Aided Pelvic Reduction Frame for Anatomical Closed Reduction of Unstable Pelvic Fractures. J. Orthop. Res. 2016, 34, 81–87. [Google Scholar] [CrossRef]
- Peng, Y.; Zhang, W.; Zhang, G.; Wang, X.; Zhang, S.; Ma, X.; Tang, P.; Zhang, L. Using the Starr Frame and Da Vinci Surgery System for Pelvic Fracture and Sacral Nerve Injury. J. Orthop. Surg. Res. 2019, 14, 29. [Google Scholar] [CrossRef] [Green Version]
- Westphal, R.; Winkelbach, S.; Gösling, T.; Hüfner, T.; Faulstich, J.; Martin, P.; Krettek, C.; Wahl, F.M. A Surgical Telemanipulator for Femur Shaft Fracture Reduction. Int. J. Med. Robot. Comput. Assist. Surg. 2006, 2, 238–250. [Google Scholar] [CrossRef]
- Sun, X.; Zhu, Q.; Wang, X.; Liang, B. A Remote Control Robotic Surgical System for Femur Shaft Fracture Reduction. In Proceedings of the 2015 IEEE International Conference on Robotics and Biomimetics (ROBIO), Zhuhai, China, 6–9 December 2015; pp. 1649–1653. [Google Scholar]
- Dagnino, G.; Georgilas, I.; Morad, S.; Gibbons, P.; Tarassoli, P.; Atkins, R.; Dogramadzi, S. Image-Guided Surgical Robotic System for Percutaneous Reduction of Joint Fractures. Ann. Biomed. Eng. 2017, 45, 2648–2662. [Google Scholar] [CrossRef] [Green Version]
- Du, H.; Hu, L.; Li, C.; Wang, T.; Zhao, L.; Li, Y.; Mao, Z.; Liu, D.; Zhang, L.; He, C.; et al. Advancing Computer-Assisted Orthopaedic Surgery Using a Hexapod Device for Closed Diaphyseal Fracture Reduction. Int. J. Med. Robot. Comput. Assist. Surg. 2015, 11, 348–359. [Google Scholar] [CrossRef]
- Collinge, C.; Tornetta, P. Soft Tissue Injuries Associated with Pelvic Fractures. Orthop. Clin. N. Am. 2004, 35, 451–456. [Google Scholar] [CrossRef]
- Li, C.; Wang, T.; Hu, L.; Zhang, L.; Zhao, Y.; Du, H.; Wang, L.; Tang, P. Robot-Musculoskeletal Dynamic Biomechanical Model in Robot-Assisted Diaphyseal Fracture Reduction. Biomed. Mater. Eng. 2015, 26 (Suppl. 1), S365–S374. [Google Scholar] [CrossRef] [Green Version]
- Joung, S.; Shikh, S.S.; Kobayashi, E.; Ohnishi, I.; Sakuma, I. Musculoskeletal Model of Hip Fracture for Safety Assurance of Reduction Path in Robot-Assisted Fracture Reduction. In Proceedings of the 5th Kuala Lumpur International Conference on Biomedical Engineering 2011, Kuala Lumpur, Malaysia, 20–23 June 2011; Osman, N.A.A., Abas, W.A.B.W., Wahab, A.K.A., Ting, H.-N., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 116–120. [Google Scholar]
- Brekelmans, W.A.; Poort, H.W.; Slooff, T.J. A New Method to Analyse the Mechanical Behaviour of Skeletal Parts. Acta Orthop. Scand. 1972, 43, 301–317. [Google Scholar] [CrossRef] [Green Version]
- Tse, K.M.; Lee Robinson, D.; Franklyn, M.; Zhang, J.Y.; Spratley, E.M.; Salzar, R.S.; Fernandez, J.; Ackland, D.C.; Lee, P.V.S. Effect of Sitting Posture on Pelvic Injury Risk under Vertical Loading. J. Mech. Behav. Biomed. Mater. 2020, 108, 103780. [Google Scholar] [CrossRef]
- Routzong, M.R.; Moalli, P.A.; Maiti, S.; De Vita, R.; Abramowitch, S.D. Novel Simulations to Determine the Impact of Superficial Perineal Structures on Vaginal Delivery. Interface Focus 2019, 9, 20190011. [Google Scholar] [CrossRef] [Green Version]
- Jhou, S.-Y.; Shih, K.-S.; Huang, P.-S.; Lin, F.-Y.; Hsu, C.-C. Biomechanical Analysis of Different Surgical Strategies for the Treatment of Rotationally Unstable Pelvic Fracture Using Finite Element Method. J. Mech. Med. Biol. 2019, 19, 1940015. [Google Scholar] [CrossRef]
- Li, L.; Lu, J.; Yang, L.; Zhang, K.; Jin, J.; Sun, G.; Wang, X.; Jiang, Q. Stability Evaluation of Anterior External Fixation in Patient with Unstable Pelvic Ring Fracture: A Finite Element Analysis. Ann. Transl. Med. 2019, 7, 303. [Google Scholar] [CrossRef]
- Liu, D.; Hua, Z.; Yan, X.; Jin, Z. Design and Biomechanical Study of a Novel Adjustable Hemipelvic Prosthesis. Med. Eng. Phys. 2016, 38, 1416–1425. [Google Scholar] [CrossRef]
- Hua, Z.; Yan, X.; Liu, D.; Jin, Z.; Wang, X.; Liu, L. Analysis of the Friction-Induced Squeaking of Ceramic-on-Ceramic Hip Prostheses Using a Pelvic Bone Finite Element Model. Tribol. Lett. 2016, 61, 26. [Google Scholar] [CrossRef]
- Iqbal, T.; Shi, L.; Wang, L.; Liu, Y.; Li, D.; Qin, M.; Jin, Z. Development of Finite Element Model for Customized Prostheses Design for Patient with Pelvic Bone Tumor. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2017, 231, 525–533. [Google Scholar] [CrossRef]
- Dong, E.; Wang, L.; Iqbal, T.; Li, D.; Liu, Y.; He, J.; Zhao, B.; Li, Y. Finite Element Analysis of the Pelvis after Customized Prosthesis Reconstruction. J. Bionic Eng. 2018, 15, 443–451. [Google Scholar] [CrossRef]
- Dong, E.; Iqbal, T.; Fu, J.; Li, D.; Liu, B.; Guo, Z.; Cuadrado, A.; Zhen, Z.; Wang, L.; Fan, H. Preclinical Strength Checking for Artificial Pelvic Prosthesis under Multi-Activities—A Case Study. J. Bionic Eng. 2019, 16, 1092–1102. [Google Scholar] [CrossRef]
- Graham, A.E.; Xie, S.Q.; Aw, K.C.; Mukherjee, S.; Xu, W.L. Bone–Muscle Interaction of the Fractured Femur. J. Orthop. Res. 2008, 26, 1159–1165. [Google Scholar] [CrossRef] [PubMed]
- Buschbaum, J.; Fremd, R.; Pohlemann, T.; Kristen, A. Introduction of a Computer-Based Method for Automated Planning of Reduction Paths under Consideration of Simulated Muscular Forces. Int. J. CARS 2017, 12, 1369–1381. [Google Scholar] [CrossRef] [PubMed]
- Phillips, A.T.M.; Pankaj, P.; Howie, C.R.; Usmani, A.S.; Simpson, A.H.R.W. Finite Element Modelling of the Pelvis: Inclusion of Muscular and Ligamentous Boundary Conditions. Med. Eng. Phys. 2007, 29, 739–748. [Google Scholar] [CrossRef] [PubMed]
- Watson, P.J.; Dostanpor, A.; Fagan, M.J.; Dobson, C.A. The Effect of Boundary Constraints on Finite Element Modelling of the Human Pelvis. Med. Eng. Phys. 2017, 43, 48–57. [Google Scholar] [CrossRef]
- Hammer, N.; Höch, A.; Klima, S.; Le Joncour, J.-B.; Rouquette, C.; Ramezani, M. Effects of Cutting the Sacrospinous and Sacrotuberous Ligaments. Clin. Anat. 2019, 32, 231–237. [Google Scholar] [CrossRef]
- Eichenseer, P.H.; Sybert, D.R.; Cotton, J.R. A Finite Element Analysis of Sacroiliac Joint Ligaments in Response to Different Loading Conditions. Spine 2011, 36, E1446–E1452. [Google Scholar] [CrossRef]
- Anderson, A.E.; Peters, C.L.; Tuttle, B.D.; Weiss, J.A. Subject-Specific Finite Element Model of the Pelvis: Development, Validation and Sensitivity Studies. J. Biomech. Eng. 2005, 127, 364–373. [Google Scholar] [CrossRef] [Green Version]
- Ramezani, M.; Klima, S.; de la Herverie, P.L.C.; Campo, J.; Le Joncour, J.B.; Rouquette, C.; Scholze, M.; Hammer, N. In Silico Pelvis and Sacroiliac Joint Motion: Refining a Model of the Human Osteoligamentous Pelvis for Assessing Physiological Load Deformation Using an Inverted Validation Approach. BioMed Res. Int. 2019, 2019, 3973170. [Google Scholar] [CrossRef] [Green Version]
- Leung, A.S.O.; Gordon, L.M.; Skrinskas, T.; Szwedowski, T.; Whyne, C.M. Effects of Bone Density Alterations on Strain Patterns in the Pelvis: Application of a Finite Element Model. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2009, 223, 965–979. [Google Scholar] [CrossRef]
- Rho, J.Y.; Hobatho, M.C.; Ashman, R.B. Relations of Mechanical Properties to Density and CT Numbers in Human Bone. Med. Eng. Phys. 1995, 17, 347–355. [Google Scholar] [CrossRef]
- Dalstra, M.; Huiskes, R.; Odgaard, A.; van Erning, L. Mechanical and Textural Properties of Pelvic Trabecular Bone. J. Biomech. 1993, 26, 523–535. [Google Scholar] [CrossRef] [Green Version]
- Sichting, F.; Rossol, J.; Soisson, O.; Klima, S.; Milani, T.; Hammer, N. Pelvic Belt Effects on Sacroiliac Joint Ligaments: A Computational Approach to Understand Therapeutic Effects of Pelvic Belts. Pain Physician 2014, 17, 43–51. [Google Scholar] [CrossRef]
- Shim, V.B.; Battley, M.; Anderson, I.A.; Munro, J.T. Validation of an Efficient Method of Assigning Material Properties in Finite Element Analysis of Pelvic Bone. Comput. Methods Biomech. Biomed. Eng. 2015, 18, 1495–1499. [Google Scholar] [CrossRef]
- Li, Z.; Alonso, J.E.; Kim, J.-E.; Davidson, J.S.; Etheridge, B.S.; Eberhardt, A.W. Three-Dimensional Finite Element Models of the Human Pubic Symphysis with Viscohyperelastic Soft Tissues. Ann. Biomed. Eng. 2006, 34, 1452–1462. [Google Scholar] [CrossRef]
- Conza, N.E.; Rixen, D.J.; Plomp, S. Vibration Testing of a Fresh-Frozen Human Pelvis: The Role of the Pelvic Ligaments. J. Biomech. 2007, 40, 1599–1605. [Google Scholar] [CrossRef]
- Qu, A.; Wang, D.; Zeng, X.; Wang, Q. Dynamic response of pelvic complex finite element study and validation under side impact. J. Mech. Med. Biol. 2017, 17, 1740036. [Google Scholar] [CrossRef]
- Hammer, N.; Steinke, H.; Lingslebe, U.; Bechmann, I.; Josten, C.; Slowik, V.; Böhme, J. Ligamentous Influence in Pelvic Load Distribution. Spine J. 2013, 13, 1321–1330. [Google Scholar] [CrossRef]
- Hao, Z.; Wan, C.; Gao, X.; Ji, T. The Effect of Boundary Condition on the Biomechanics of a Human Pelvic Joint under an Axial Compressive Load: A Three-Dimensional Finite Element Model. J. Biomech. Eng. 2011, 133, 101006. [Google Scholar] [CrossRef]
- Zheng, N.; Watson, L.G.; Yong-Hing, K. Biomechanical Modelling of the Human Sacroiliac Joint. Med. Biol. Eng. Comput. 1997, 35, 77–82. [Google Scholar] [CrossRef]
- Ivanov, A.A.; Kiapour, A.; Ebraheim, N.A.; Goel, V. Lumbar Fusion Leads to Increases in Angular Motion and Stress Across Sacroiliac Joint: A Finite Element Study. Spine 2009, 34, E162–E169. [Google Scholar] [CrossRef] [PubMed]
- Butler, D.L.; Guan, Y.; Kay, M.D.; Cummings, J.F.; Feder, S.M.; Levy, M.S. Location-Dependent Variations in the Material Properties of the Anterior Cruciate Ligament. J. Biomech. 1992, 25, 511–518. [Google Scholar] [CrossRef] [PubMed]
- Wismans, J.; Veldpaus, F.; Janssen, J.; Huson, A.; Struben, P. A Three-Dimensional Mathematical Model of the Knee-Joint. J. Biomech. 1980, 13, 677–685. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cosson, M.; Boukerrou, M.; Lacaze, S.; Lambaudie, E.; Fasel, J.; Mesdagh, H.; Lobry, P.; Ego, A. A Study of Pelvic Ligament Strength. Eur. J. Obstet. Gynecol. Reprod. Biol. 2003, 109, 80–87. [Google Scholar] [CrossRef] [PubMed]
- Hammer, N.; Steinke, H.; Slowik, V.; Josten, C.; Stadler, J.; Böhme, J.; Spanel-Borowski, K. The Sacrotuberous and the Sacrospinous Ligament—A Virtual Reconstruction. Ann. Anat-Anat. Anz. 2009, 191, 417–425. [Google Scholar] [CrossRef] [PubMed]
- Steinke, H.; Hammer, N.; Slowik, V.; Stadler, J.; Josten, C.; Böhme, J.; Spanel-Borowski, K. Novel Insights Into the Sacroiliac Joint Ligaments. Spine 2010, 35, 257–263. [Google Scholar] [CrossRef]
- Hammer, N.; Steinke, H.; Böhme, J.; Stadler, J.; Josten, C.; Spanel-Borowski, K. Description of the Iliolumbar Ligament for Computer-Assisted Reconstruction. Ann. Anat-Anat. Anz. 2010, 192, 162–167. [Google Scholar] [CrossRef]
- Hu, P.; Wu, T.; Wang, H.; Qi, X.; Yao, J.; Cheng, X.; Chen, W.; Zhang, Y. Influence of Different Boundary Conditions in Finite Element Analysis on Pelvic Biomechanical Load Transmission. Orthop. Surg. 2017, 9, 115–122. [Google Scholar] [CrossRef] [Green Version]
- Forst, S.L.; Wheeler, M.T.; Fortin, J.D.; Vilensky, J.A. The Sacroiliac Joint: Anatomy, Physiology and Clinical Significance. Pain Physician 2006, 9, 61–67. [Google Scholar]
- Foley, B.S.; Buschbacher, R.M. Sacroiliac Joint Pain: Anatomy, Biomechanics, Diagnosis, and Treatment. Am. J. Phys. Med. Rehabil. 2006, 85, 997–1006. [Google Scholar] [CrossRef] [Green Version]
- Ravera, E.P.; Crespo, M.J.; Catalfamo Formento, P.A. A subject-specific integrative biomechanical framework of the pelvis for gait analysis. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2018, 232, 1083–1097. [Google Scholar] [CrossRef]
- Liu, D.; Hua, Z.; Yan, X.; Jin, Z. Biomechanical Analysis of a Novel Hemipelvic Endoprosthesis during Ascending and Descending Stairs. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2016, 230, 962–975. [Google Scholar] [CrossRef]
- Finlay, J.B.; Repo, R.U. Impact Characteristics of Articular Cartilage. ISA Trans 1978, 17, 29–34. [Google Scholar]
- Repo, R.U.; Finlay, J.B. Survival of Articular Cartilage after Controlled Impact. J. Bone Joint Surg. Am. 1977, 59, 1068–1076. [Google Scholar] [CrossRef]
- Volinski, B.; Kalra, A.; Yang, K. Evaluation of Full Pelvic Ring Stresses Using a Bilateral Static Gait-Phase Finite Element Modeling Method. J. Mech. Behav. Biomed. Mater. 2018, 78, 175–187. [Google Scholar] [CrossRef]
- Anderson, A.E.; Ellis, B.J.; Maas, S.A.; Weiss, J.A. Effects of Idealized Joint Geometry on Finite Element Predictions of Cartilage Contact Stresses in the Hip. J. Biomech. 2010, 43, 1351–1357. [Google Scholar] [CrossRef] [Green Version]
- Zajac, F.E. Muscle and Tendon: Properties, Models, Scaling, and Application to Biomechanics and Motor Control. Crit. Rev. Biomed. Eng. 1989, 17, 359–411. [Google Scholar]
- Delp, S.L. Surgery Simulation: A Computer Graphics System to Analyze and Design Musculoskeletal Reconstructions of the Lower Limb; Stanford University: Stanford, CA, USA, 1990; pp. 17–24. [Google Scholar]
- Friederich, J.A.; Brand, R.A. Muscle Fiber Architecture in the Human Lower Limb. J. Biomech. 1990, 23, 91–95. [Google Scholar] [CrossRef]
- Wickiewicz, T.L.; Roy, R.R.; Powell, P.L.; Edgerton, V.R. Muscle Architecture of the Human Lower Limb. Clin. Orthop. Relat. Res. 1983, 179, 275. [Google Scholar] [CrossRef]
- Dalstra, M.; Huiskes, R. Load Transfer across the Pelvic Bone. J. Biomech. 1995, 28, 715–724. [Google Scholar] [CrossRef] [Green Version]
- Denis, F.; Davis, S.; Comfort, T. Sacral Fractures: An Important Problem. Retrospective Analysis of 236 Cases. Clin. Orthop. Relat. Res. 1988, 227, 67–81. [Google Scholar] [CrossRef] [PubMed]
- Salo, Z.; Beek, M.; Wright, D.; Maloul, A.; Whyne, C.M. Analysis of Pelvic Strain in Different Gait Configurations in a Validated Cohort of Computed Tomography Based Finite Element Models. J. Biomech. 2017, 64, 120–130. [Google Scholar] [CrossRef] [PubMed]

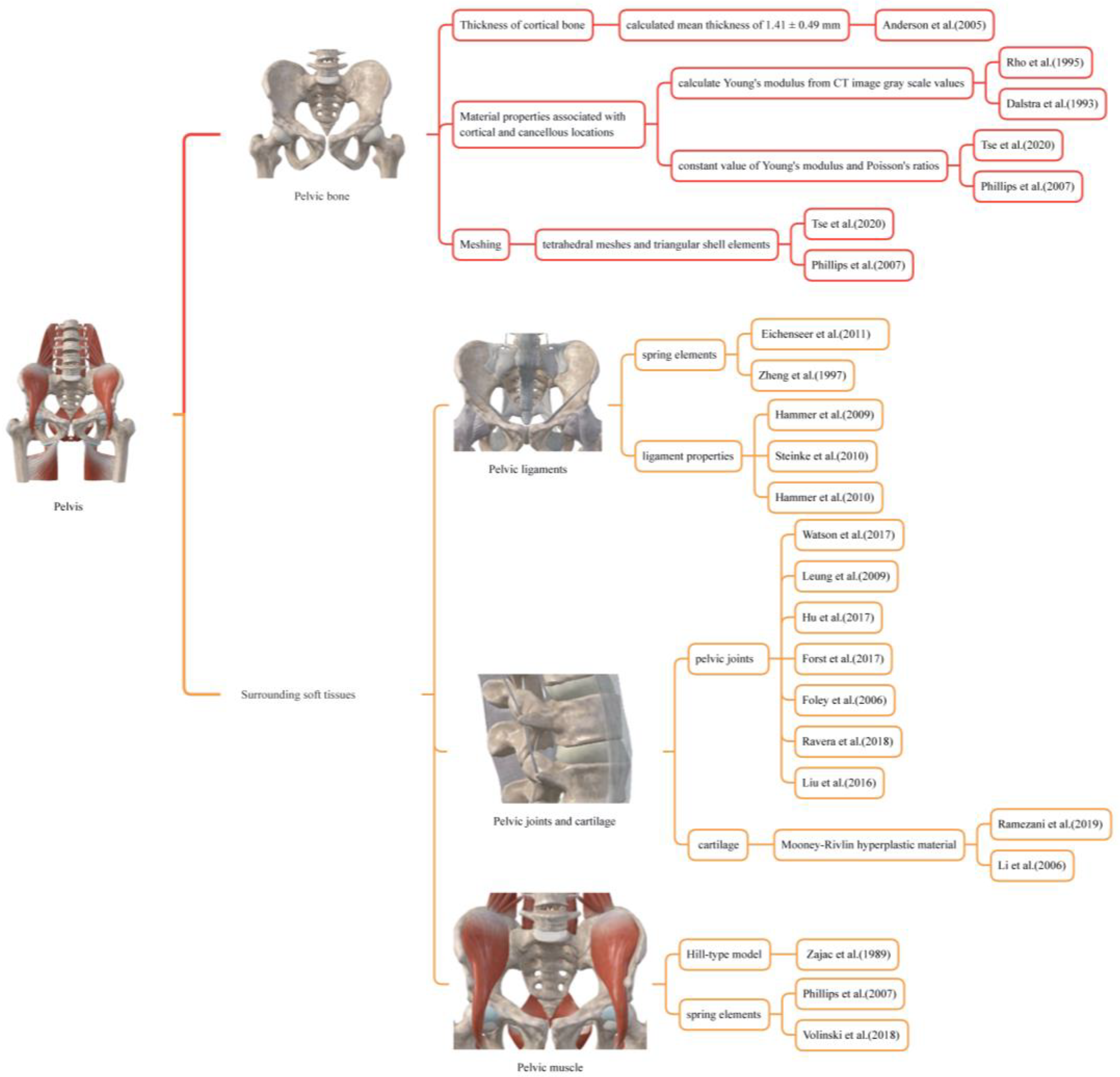
| Components | Key Aspects | Parameter Configuration | References |
|---|---|---|---|
 Pelvic bone | thickness of cortical bone | constant value of 0.45–3 mm | Tse et al. [16], Liu et al. [20], Phillips et al. [27], Watson et al. [28], Hammer et al. [29], Eichenseer et al. [30] |
| calculated mean thickness of 1.41 ± 0.49 mm | Anderson et al. [31] | ||
| 2 mm and 3 mm bone shell | Ramezani et al. [32] | ||
| material properties associated with cortical and cancellous locations | calculate Young’s modulus from CT image gray-scale values | Leung et al. [33], Rho et al. [34], Dalstra et al. [35] | |
| constant value of Young’s modulus and Poisson’s ratios | Tse et al. [16], Liu et al. [20], Phillips et al. [27], Watson et al. [28], Sichting et al. [36] | ||
| assign material properties | Shim et al. [37] | ||
| Meshing | hexahedral meshes | Li et al. [38] | |
| tetrahedral meshes and triangular shell elements | Tse et al. [16] Anderson et al. [31], Phillips et al. [27] | ||
 Pelvic ligaments | influence of ligaments | / | Hammer et al. [29], Conza et al. [39], Qu et al. [40], Hammer et al. [41], Hao et al. [42] |
| introduced spring elements | constant stiffness coefficients | Zheng et al. [43] | |
| nonlinear spring elements | Eichenseer et al. [30], Ivanov et al. [44], Butler et al. [45], Wismans et al. [46] | ||
| ligament properties | / | Cosson et al. [47], Hammer et al. [48,49,50] | |
 Pelvic joints and cartilage | influence of pelvic joints | / | Li et al. [19], Liu et al. [20], Watson et al. [28], Leung et al. [33], Hu et al. [51], Forst et al. [52], Foley et al. [53], Ravera et al. [54], Liu et al. [55] |
| influence of cartilage | material properties of cartilage | Finlay et al. [56], Repo et al. [57] | |
| linear elastic material | Hammer et al. [41], Liu et al. [55], Volinski et al. [58] | ||
| Mooney-Rivlin hyperplastic material | Ramezani et al. [32], Li et al. [38] | ||
| neo-Hookean hyperplastic material | Ramezani et al. [32], Anderson et al. [59] | ||
| viscoelastic material | Ramezani et al. [32] | ||
 Pelvic muscle | / | Hill-type model | Zajac et al. [60] |
| / | incorporating muscle models into FE models | Phillips et al. [27], Delp et al. [61], Friederich et al. [62], Wickiewicz et al. [63] | |
| / | calculating the forces and applying them to the FE model | Watson et al. [28], Ravera et al. [54] |
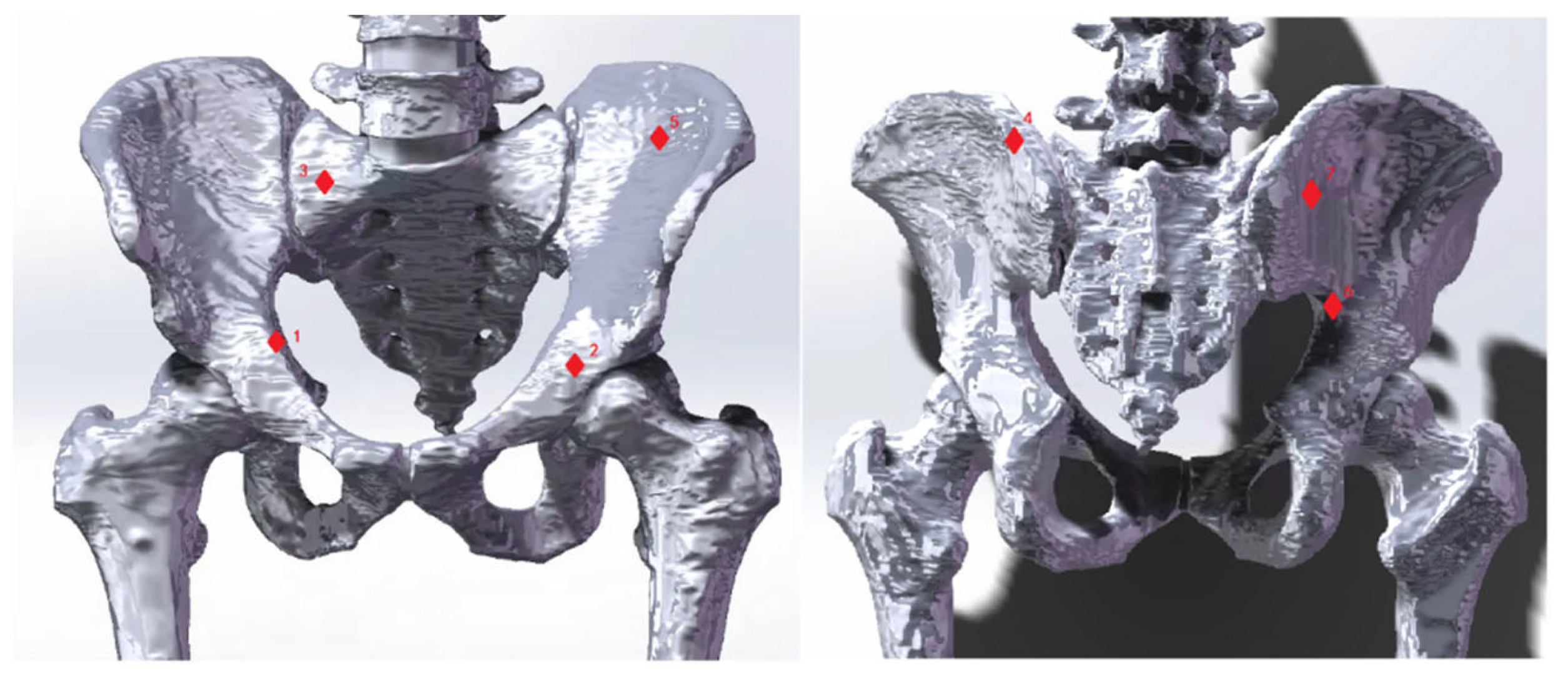
| 50 N (mm/mm) | 150 N (mm/mm) | 250 N (mm/mm) | 350 N (mm/mm) | 450 N (mm/mm) | 550 N (mm/mm) | |
|---|---|---|---|---|---|---|
| 1 | 3.08 × 10−5 | 8.57 × 10−5 | 1.14 × 10−4 | 1.97 × 10−4 | 2.62 × 10−4 | 3.31 × 10−4 |
| 2 | 6.94 × 10−5 | 1.27 × 10−4 | 1.89 × 10−4 | 2.52 × 10−4 | 3.26 × 10−4 | 3.97 × 10−4 |
| 3 | 1.76 × 10−4 | 4.68 × 10−4 | 7.70 × 10−4 | 1.07 × 10−3 | 1.41 × 10−3 | 1.77 × 10−3 |
| 4 | 6.07 × 10−5 | 1.14 × 10−4 | 1.76 × 10−4 | 2.44 × 10−4 | 3.21 × 10−4 | 4.18 × 10−4 |
| 5 | 3.83 × 10−5 | 1.15 × 10−4 | 1.88 × 10−4 | 2.59 × 10−4 | 3.34 × 10−4 | 4.09 × 10−4 |
| 6 | 3.51 × 10−5 | 6.88 × 10−5 | 1.23 × 10−4 | 1.76 × 10−4 | 2.32 × 10−4 | 2.85 × 10−4 |
| 7 | 9.54 × 10−5 | 4.11 × 10−4 | 7.21 × 10−4 | 1.02 × 10−3 | 1.32 × 10−3 | 1.61 × 10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kou, W.; Liang, Y.; Wang, Z.; Liang, Q.; Sun, L.; Kuang, S. An Integrated Method of Biomechanics Modeling for Pelvic Bone and Surrounding Soft Tissues. Bioengineering 2023, 10, 736. https://doi.org/10.3390/bioengineering10060736
Kou W, Liang Y, Wang Z, Liang Q, Sun L, Kuang S. An Integrated Method of Biomechanics Modeling for Pelvic Bone and Surrounding Soft Tissues. Bioengineering. 2023; 10(6):736. https://doi.org/10.3390/bioengineering10060736
Chicago/Turabian StyleKou, Wei, Yefeng Liang, Zhixing Wang, Qingxi Liang, Lining Sun, and Shaolong Kuang. 2023. "An Integrated Method of Biomechanics Modeling for Pelvic Bone and Surrounding Soft Tissues" Bioengineering 10, no. 6: 736. https://doi.org/10.3390/bioengineering10060736
APA StyleKou, W., Liang, Y., Wang, Z., Liang, Q., Sun, L., & Kuang, S. (2023). An Integrated Method of Biomechanics Modeling for Pelvic Bone and Surrounding Soft Tissues. Bioengineering, 10(6), 736. https://doi.org/10.3390/bioengineering10060736






