Using a Bed Sill as a Countermeasure for Clear-Water Scour at a Complex Pier with Inclined Columns Footed on Capped Piles
Abstract
:1. Introduction
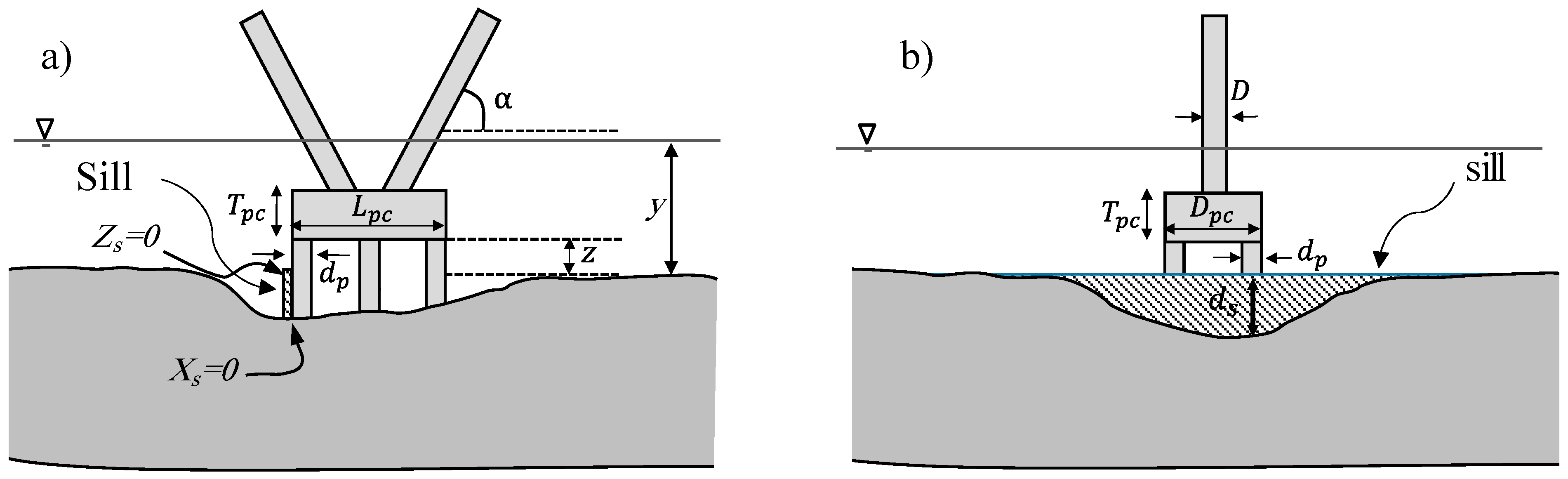
2. Dimensionless Framework for Analysis
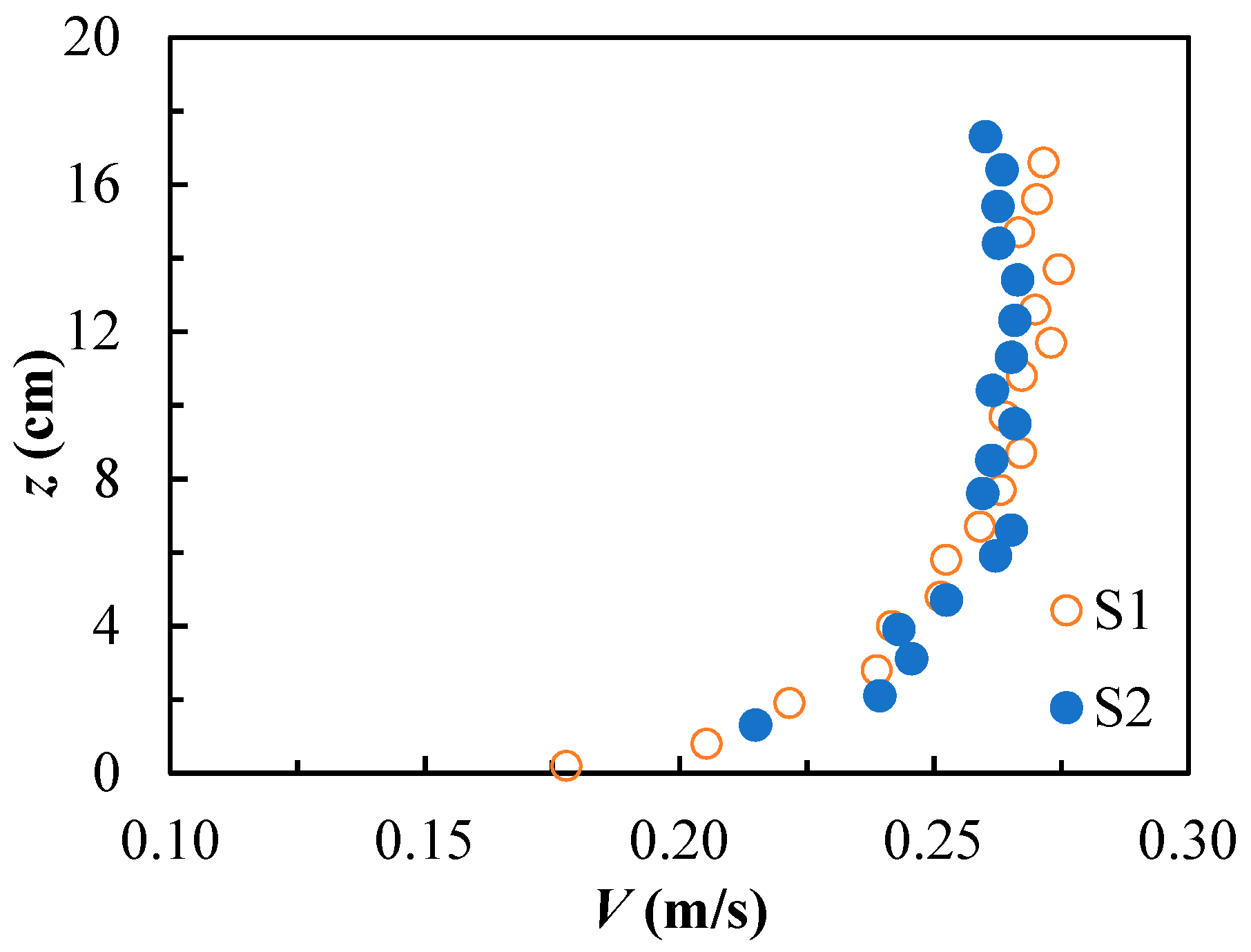
3. Experimental Setup
4. Experimental Procedure
5. Results
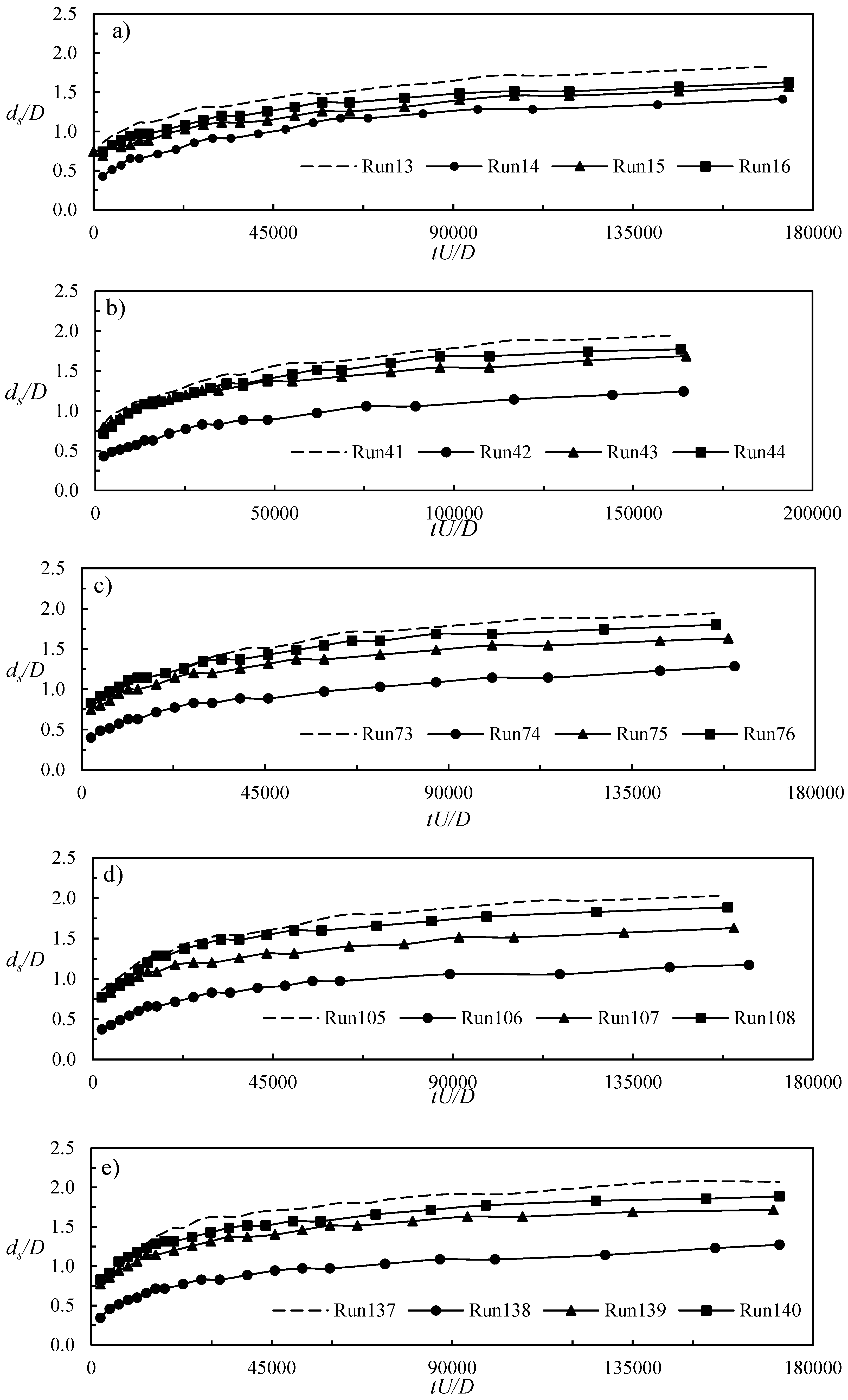
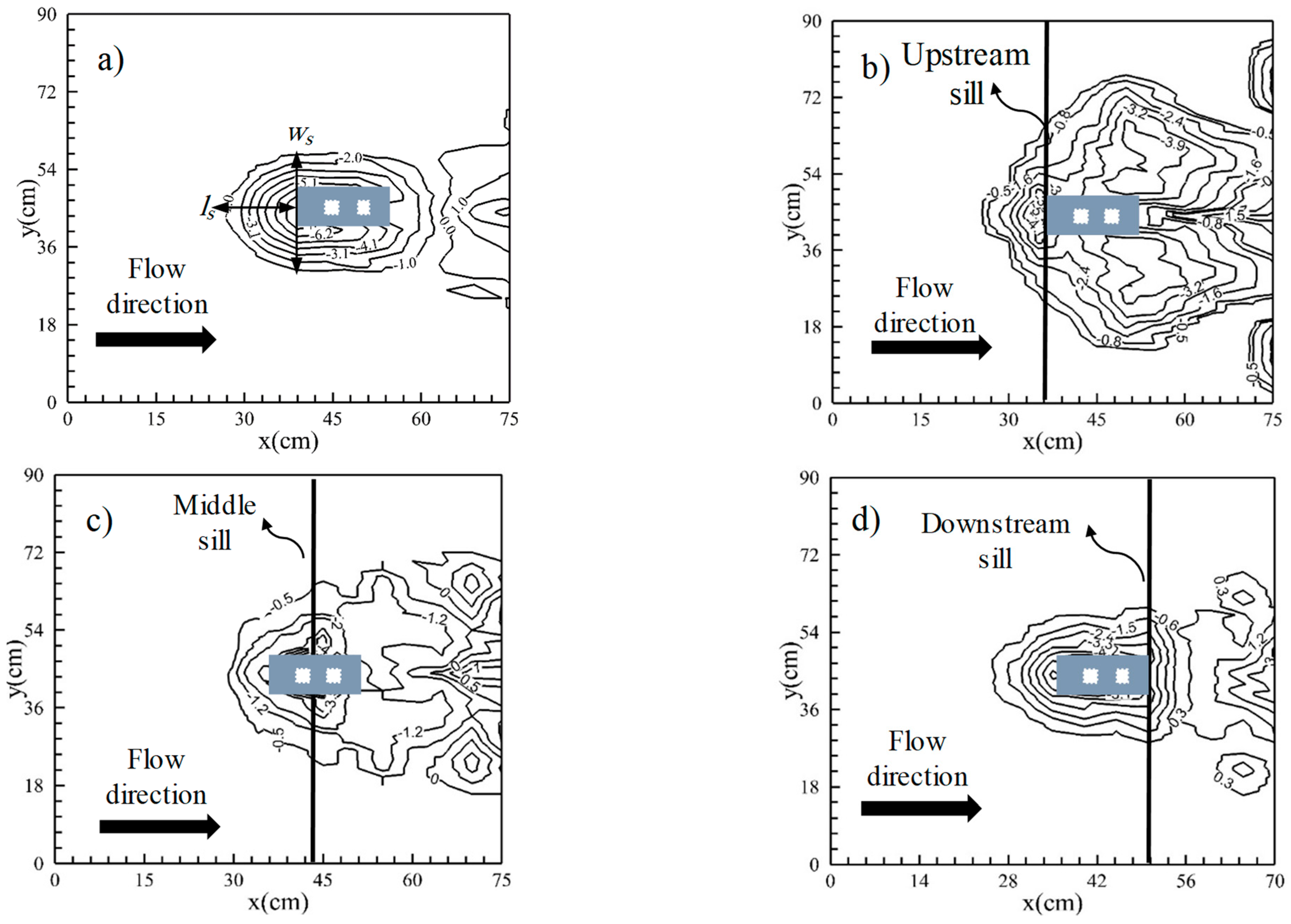
5.1. Phenomenological Description and Temporal Evolution of the Maximum Scour Depth
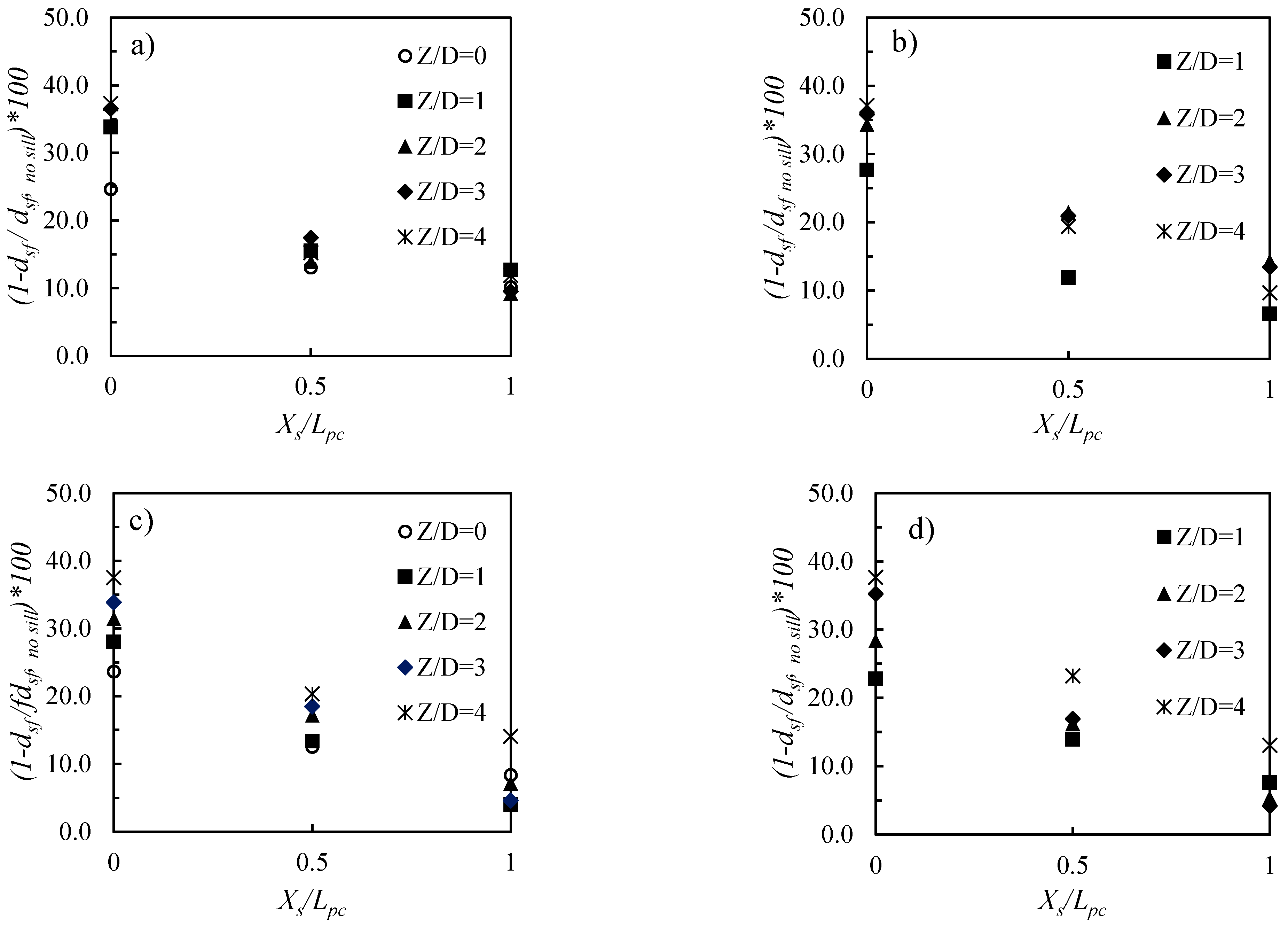
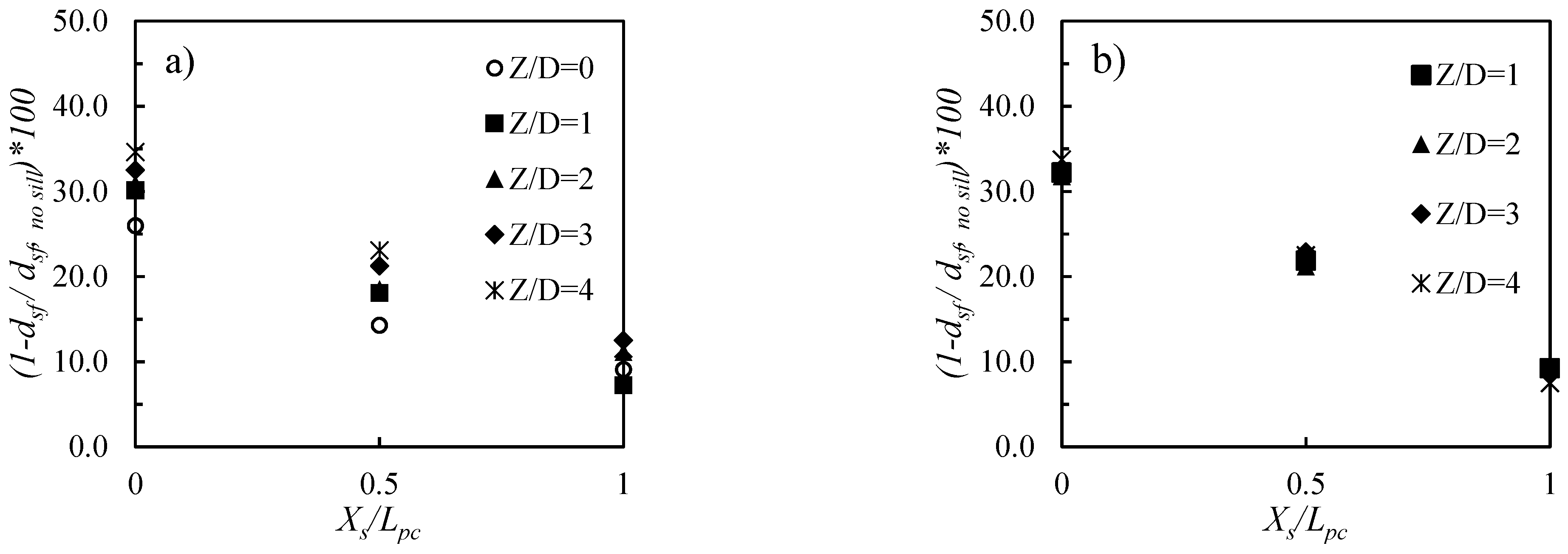
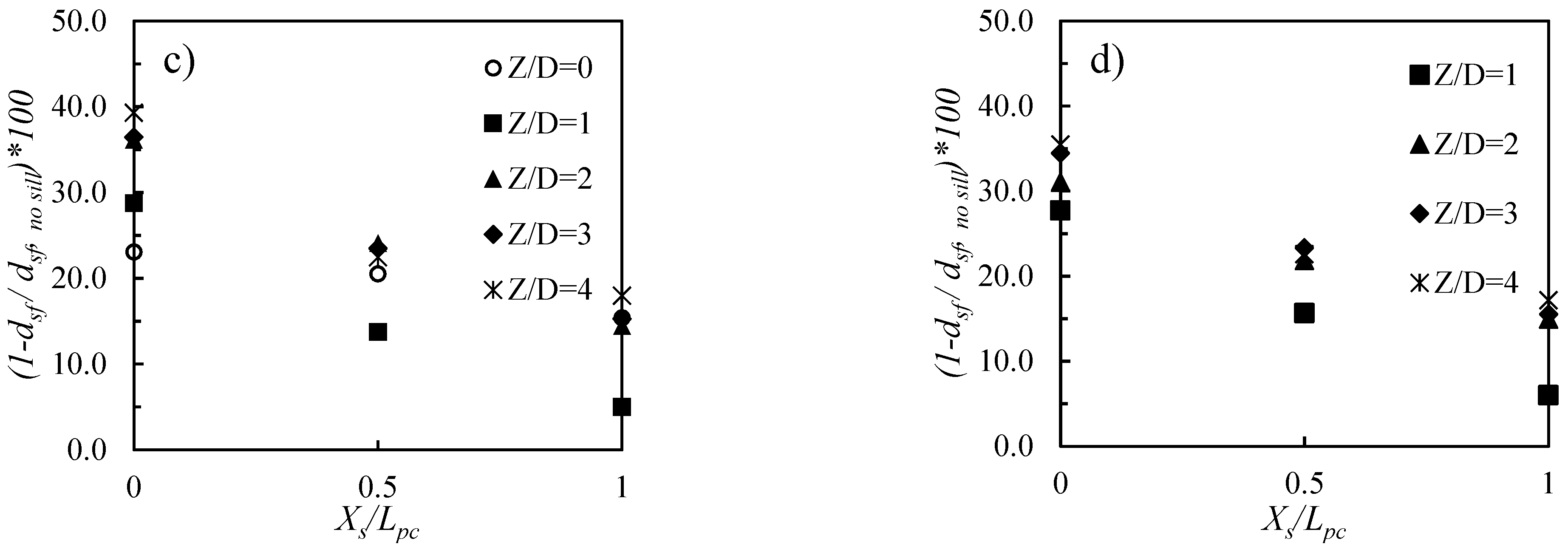
Effect of a Sill Location on the Final Scour Depth
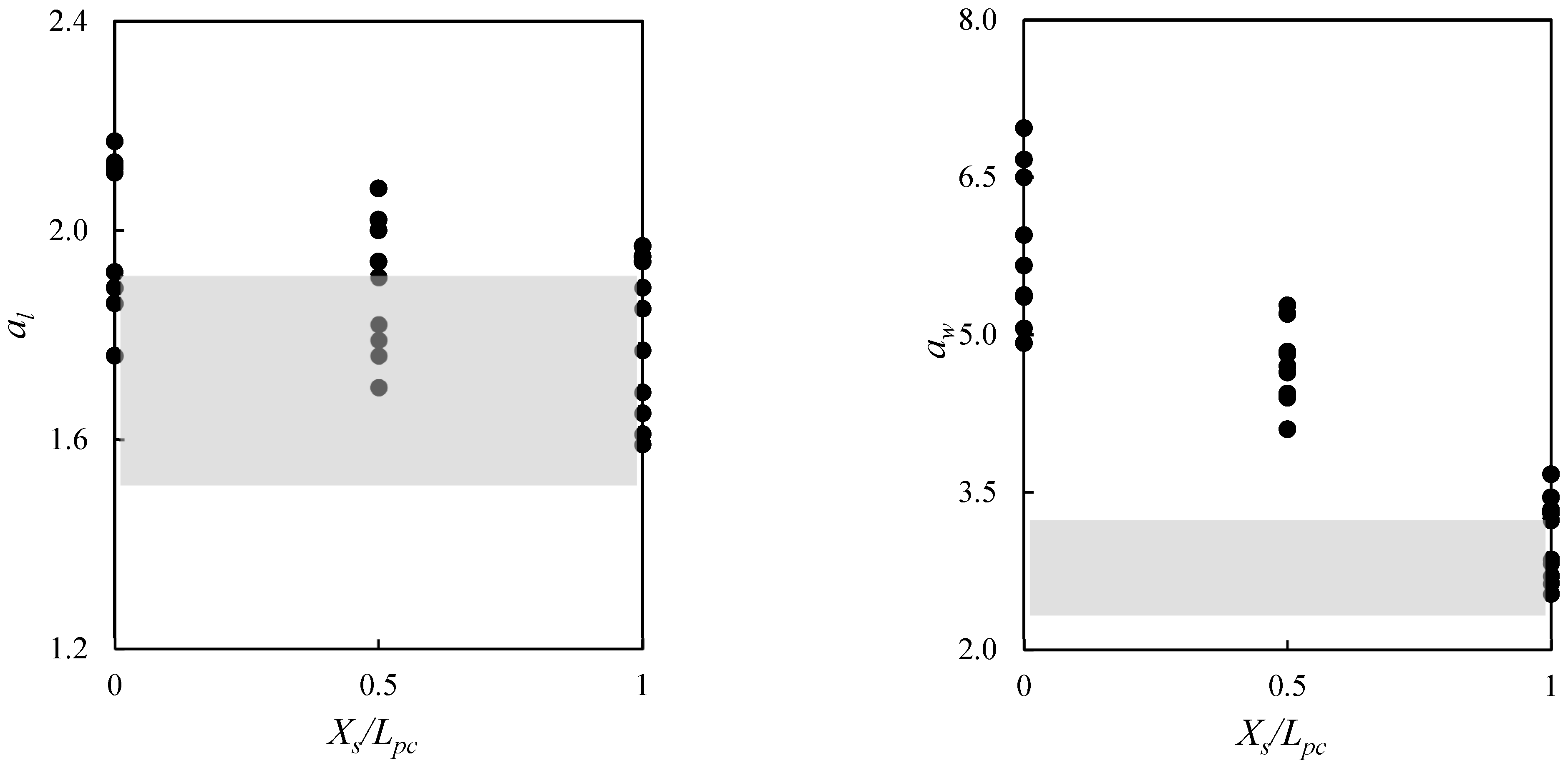
5.2. Dimensions of the Scour Hole
6. Discussion and Prospects for Further Research
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Symbols
| B | flume width |
| D | column width |
| D50, σg and ρs | median size, uniformity coefficient and density of sediment |
| dp | diameter of piles |
| Dpc and Lpc | transverse and stream-wise dimensions of the pile cap |
| ds | the time-dependent maximum scour depth |
| ds5 | the maximum scour depth at tU/D = 1.5 × 105 |
| dsf | the maximum scour depth measured after 25 h |
| g | acceleration due to gravity |
| m | number of piles in the stream-wise direction |
| n | number of piles in the transverse direction |
| t | time |
| Tpc | thickness of the pile cap |
| U | section-averaged flow velocity |
| Xs | stream-wise coordinate of the sill, measured from the upstream edge of the pile cap |
| y | flow depth |
| Z | elevation of the pile cap above the non-scoured bed elevation |
| Zs | elevation of the sill above the non-scoured bed level |
| α | pier inclination |
| ρ and μ | density and viscosity of water |
References
- Melville, B.W.; Coleman, S.E. Bridge Scour; Water Resources Publications LLC: Littleton, CO, USA, 2000. [Google Scholar]
- Ataie-Ashtiani, B.; Beheshti, A.A. Experimental Investigation of Clear-Water Local Scour at Pile Groups. J. Hydraul. Eng. 2006, 132, 1100–1104. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Tang, H.; Liu, Q.; Wang, Y. Local scouring around twin bridge piers in open-channel flow. J. Hydraul. Eng. 2016, 142, 06016008. [Google Scholar] [CrossRef]
- Salim, M.; Jones, J.S. Scour around exposed pile foundation. In Proceedings of the North American Water and Environment Congress & Destructive Water, Anaheim, CA, USA; 1996; pp. 335–346. [Google Scholar]
- Ataie-Ashtiani, B.; Baratian-Ghorghi, Z.; Beheshti, A.A. Experimental investigation of clear-water local scour of compound piers. J. Hydraul. Eng. 2010, 136, 343–351. [Google Scholar] [CrossRef]
- Amini, A.; Melville, B.; Ali, T.M.; Ghazali, A.H. Clear-water local scour around pile groups in shallow-water flow. J. Hydraul. Eng. 2012, 138, 177–185. [Google Scholar] [CrossRef]
- Yang, Y.; Qi, M.; Wang, X.; Li, J. Experimental study of scour around pile groups in steady flows. Ocean Eng. 2020, 195, 106651. [Google Scholar] [CrossRef]
- Ferraro, D.; Tafarojnoruz, A.; Gaudio, R.; Cardoso, A.H. Effects of pile cap thickness on the maximum scour depth at a complex pier. J. Hydraul. Eng. 2013, 132, 482–491. [Google Scholar] [CrossRef]
- Yang, Y.; Melville, B.W.; Sheppard, D.M.; Shamseldin, A.Y. Clear-water local scour at skewed complex bridge piers. J. Hydraul. Eng. 2018, 144, 04018019. [Google Scholar] [CrossRef]
- Yang, Y.; Melville, B.W.; Macky, G.H.; Shamseldin, A.Y. Local Scour at Complex Bridge Piers in Close Proximity under Clear-Water and Live-Bed Flow Regime. Water 2019, 11, 1530. [Google Scholar] [CrossRef] [Green Version]
- Varaki, M.E.; Radice, A.; Hossini, S.S.; Ola, R.F. Local scour at a complex pier with inclined columns footed on capped piles: Effect of the pile arrangement and of the cap thickness and elevation. ISH J. Hydraul. Eng. 2019, 28, 91–100. [Google Scholar] [CrossRef]
- Sheppard, D.M.; Renna, R. Florida Scour Manual; Florida Department of Transportation: Tallahassee, FL, USA, 2010.
- Arneson, L.A.; Zevenbergen, L.W.; Lagasse, P.F.; Clopper, P.E. Evaluating Scour at Bridges (HEC-18); Technical Rep. No. HIF-12-003; Federal Highway Administration (FHWA): Washington, DC, USA, 2012.
- Amini, A.; Mohammad, T.A. Local scour prediction around piers with complex geometry. Mar. Georesources Geotechnol. 2017, 35, 857–864. [Google Scholar] [CrossRef]
- Richardson, E.V.; Davis, S.R. Evaluating Scour at Bridges, 4th ed.; Hydraulic Engineering Circular No. 18, FHWA-NHI-01-001; Federal Highway Administration: Washington, DC, USA, 2001.
- Graziano, F.G.; Jones, J.S.; Parola, A.C. Design of riprap to protect bridge Piers from local scour. J. Highw. Res. Dev. Public Roads 1990, 54, 193–199. [Google Scholar]
- Fotherby, L.M.; Jones, J.S. The influence of exposed footings on pier scour depths. In Proceedings of the National Conference on Hydraulic Engineering, San Francisco, CA, USA; 1993; Volume 1, pp. 922–927. [Google Scholar]
- Melville, B.W.; Ballegooy, S.; Coleman, S.; Barkdoll, B. Riprap size selection at wing-wall abutments. J. Hydraul. Eng. 2007, 133, 1265–1269. [Google Scholar] [CrossRef]
- Zarrati, A.; Nazariha, M.; Mashahir, M.B. Reduction of local scour in the vicinity of bridge pier groups using collars and riprap. J. Hydraul. Eng. 2006, 132, 154–162. [Google Scholar] [CrossRef]
- Cardoso, A.H.; Simarro, G.; Le Doucen, O.; Schleiss, A. Sizing of riprap for spill-through abutments. Proc. Inst. Civ. Eng. Water Manag. 2010, 163, 499–507. [Google Scholar] [CrossRef] [Green Version]
- Grimaldi, C.; Gaudio, R.; Calomino, F.; Cardoso, A.H. Control of scour at bridge piers by a downstream bed sill. J. Hydraul. Eng. 2009, 135, 13–21. [Google Scholar] [CrossRef]
- Grimaldi, C.; Gaudio, R.; Calomino, F.; Cardoso, A.H. Countermeasures against local scouring at bridge piers: Slot and combined system of slot and bed sill. J. Hydraul. Eng. 2009, 135, 425–431. [Google Scholar] [CrossRef]
- Mashahir, M.B.; Zarrati, A.R.; Mokallaf, E. Application of riprap and collar to prevent scouring around rectangular bridge piers. J. Hydraul. Eng. 2010, 136, 183–187. [Google Scholar] [CrossRef]
- Zarrati, A.; Gholami, H.; Mashahir, M.B. Application of collar to control scouring around rectangular bridge piers. J. Hydraul. Res. 2004, 42, 97–103. [Google Scholar] [CrossRef]
- Cardoso, A.H.; Fael, C.M.S. Protecting vertical-wall abutments with riprap mattresses. J. Hydraul. Eng. 2009, 135, 457–465. [Google Scholar] [CrossRef] [Green Version]
- Chiew, Y.M.; Lim, S.Y. Protection of bridge piers using a sacrificial sill. Proc. ICE Water Marit. Eng. 2003, 156, 53–62. [Google Scholar] [CrossRef]
- Gaudio, R.; Tafarojnoruz, A.; Calomino, F. Combined flow altering countermeasures against bridge pier scour. J. Hydraul. Res. 2012, 50, 35–43. [Google Scholar] [CrossRef]
- Tafarojnoruz, A.; Gaudio, R.; Calomino, F. Bridge pier scour mitigation under steady and unsteady flow conditions. Acta Geophys. 2012, 60, 1076–1097. [Google Scholar] [CrossRef]
- Saadati, P.S.; Esmaeili, V.M.; Fazl, O.R. Experimental investigation of effect of sill Location on local scour around inclined bridge piers group. J. Water Soil 2014, 28, 406–419. [Google Scholar]
- Wang, L.; Melville, B.W.; Whittaker, C.N.; Guan, D. Effects of a downstream submerged weir on local scour at bridge piers. J. Hydro-Environ. Res. 2018, 20, 101–109. [Google Scholar] [CrossRef]
- Kirkgöz, M.S.; Ardichoğlu, M. Velocity profiles of developing and developed open channel flow. J. Hydraul. Eng. 1997, 123, 1099–1105. [Google Scholar] [CrossRef]
- Raudkivi, A.J.; Ettema, R. Clear-water scour at cylindrical piers. J. Hydraul. Eng. 1983, 109, 339–350. [Google Scholar] [CrossRef]
- Oliveto, G.; Hager, W.H. Temporal evolution of clear-water pier and abutment scour. J. Hydraul. Eng. 2002, 128, 811–820. [Google Scholar] [CrossRef]
- Lança, R.; Simarro, G.; Fael, C.M.S.; Cardoso, A.H. Effect of viscosity on the equilibrium scour depth at single cylindrical piers. J. Hydraul. Eng. 2016, 142, 06015022. [Google Scholar] [CrossRef]
- Dey, S. Fluvial Hydrodynamics. In Hydrodynamic and Sediment Transport Phenomena; Springer: Berlin, Germany, 2014. [Google Scholar]
- Buffington, J.M.; Montgomery, D.R. A systematic analysis of eight decades of incipient motion studies, with special reference to gravel bedded rivers. Water Resour. Res. 1997, 33, 1993–2029. [Google Scholar] [CrossRef] [Green Version]
- Tafarojnoruz, A.; Gaudio, R.; Calomino, F. Evaluation of flow-altering countermeasures against bridge pier scour. J. Hydraul. Eng. 2012, 138, 297–305. [Google Scholar] [CrossRef]
- Marion, A.; Tregnaghi, M.; Tait, S. Sediment supply and local scouring at bed sills in high-gradient streams. Water Resour. Res. 2006, 42, W06416. [Google Scholar] [CrossRef]
- Ben Meftah, M.; Mossa, M. Scour holes downstream of bed sills in low-gradient channels. J. Hydraul. Res. 2006, 44, 497–509. [Google Scholar] [CrossRef]




| Run Num. | dp (m) | m | Tpc (m) | Z (m) | Xs/Lps | ds5 (m) | dsf (m) | Run Num. | dp (m) | m | Tpc (m) | Z (m) | Xs/Lps | ds5 (m) | dsf (m) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.02 | 2 | 0.03 | 0 | NS | 0.057 | 0.069 | 32 | 0.02 | 3 | 0.05 | 0.035 | 1 | 0.061 | 0.073 |
| 2 | 0.02 | 2 | 0.03 | 0 | 0 | 0.044 | 0.052 | 33 | 0.03 | 2 | 0.03 | 0.035 | NS | 0.068 | 0.083 |
| 3 | 0.02 | 2 | 0.03 | 0 | 0.5 | 0.048 | 0.06 | 34 | 0.03 | 2 | 0.03 | 0.035 | 0 | 0.043 | 0.058 |
| 4 | 0.02 | 2 | 0.03 | 0 | 1 | 0.05 | 0.062 | 35 | 0.03 | 2 | 0.03 | 0.035 | 0.5 | 0.057 | 0.068 |
| 5 | 0.02 | 2 | 0.05 | 0 | NS | 0.06 | 0.072 | 36 | 0.03 | 2 | 0.03 | 0.035 | 1 | 0.064 | 0.077 |
| 6 | 0.02 | 2 | 0.05 | 0 | 0 | 0.043 | 0.054 | 37 | 0.03 | 3 | 0.03 | 0.035 | NS | 0.07 | 0.087 |
| 7 | 0.02 | 2 | 0.05 | 0 | 0.5 | 0.053 | 0.063 | 38 | 0.03 | 3 | 0.03 | 0.035 | 0 | 0.045 | 0.059 |
| 8 | 0.02 | 2 | 0.05 | 0 | 1 | 0.055 | 0.066 | 39 | 0.03 | 3 | 0.03 | 0.035 | 0.5 | 0.059 | 0.068 |
| 9 | 0.03 | 2 | 0.03 | 0 | NS | 0.063 | 0.077 | 40 | 0.03 | 3 | 0.03 | 0.035 | 1 | 0.067 | 0.079 |
| 10 | 0.03 | 2 | 0.03 | 0 | 0 | 0.044 | 0.057 | 41 | 0.03 | 2 | 0.05 | 0.035 | NS | 0.068 | 0.08 |
| 11 | 0.03 | 2 | 0.03 | 0 | 0.5 | 0.055 | 0.066 | 42 | 0.03 | 2 | 0.05 | 0.035 | 0 | 0.043 | 0.057 |
| 12 | 0.03 | 2 | 0.03 | 0 | 1 | 0.061 | 0.07 | 43 | 0.03 | 2 | 0.05 | 0.035 | 0.5 | 0.059 | 0.069 |
| 13 | 0.03 | 2 | 0.05 | 0 | NS | 0.064 | 0.078 | 44 | 0.03 | 2 | 0.05 | 0.035 | 1 | 0.062 | 0.076 |
| 14 | 0.03 | 2 | 0.05 | 0 | 0 | 0.049 | 0.057 | 45 | 0.03 | 3 | 0.05 | 0.035 | NS | 0.07 | 0.083 |
| 15 | 0.03 | 2 | 0.05 | 0 | 0.5 | 0.055 | 0.062 | 46 | 0.03 | 3 | 0.05 | 0.035 | 0 | 0.049 | 0.06 |
| 16 | 0.03 | 2 | 0.05 | 0 | 1 | 0.057 | 0.066 | 47 | 0.03 | 3 | 0.05 | 0.035 | 0.5 | 0.06 | 0.07 |
| 17 | 0.02 | 2 | 0.03 | 0.035 | NS | 0.059 | 0.071 | 48 | 0.03 | 3 | 0.05 | 0.035 | 1 | 0.066 | 0.079 |
| 18 | 0.02 | 2 | 0.03 | 0.035 | 0 | 0.045 | 0.053 | 49 | 0.02 | 2 | 0.03 | 0.07 | NS | 0.054 | 0.065 |
| 19 | 0.02 | 2 | 0.03 | 0.035 | 0.5 | 0.052 | 0.06 | 50 | 0.02 | 2 | 0.03 | 0.07 | 0 | 0.033 | 0.043 |
| 20 | 0.02 | 2 | 0.03 | 0.035 | 1 | 0.055 | 0.062 | 51 | 0.02 | 2 | 0.03 | 0.07 | 0.5 | 0.045 | 0.056 |
| 21 | 0.02 | 3 | 0.03 | 0.035 | NS | 0.063 | 0.076 | 52 | 0.02 | 2 | 0.03 | 0.07 | 1 | 0.051 | 0.059 |
| 22 | 0.02 | 3 | 0.03 | 0.035 | 0 | 0.048 | 0.055 | 53 | 0.02 | 3 | 0.03 | 0.07 | NS | 0.06 | 0.07 |
| 23 | 0.02 | 3 | 0.03 | 0.035 | 0.5 | 0.056 | 0.067 | 54 | 0.02 | 3 | 0.03 | 0.07 | 0 | 0.038 | 0.046 |
| 24 | 0.02 | 3 | 0.03 | 0.035 | 1 | 0.06 | 0.071 | 55 | 0.02 | 3 | 0.03 | 0.07 | 0.5 | 0.048 | 0.055 |
| 25 | 0.02 | 2 | 0.05 | 0.035 | NS | 0.063 | 0.075 | 56 | 0.02 | 3 | 0.03 | 0.07 | 1 | 0.051 | 0.06 |
| 26 | 0.02 | 2 | 0.05 | 0.035 | 0 | 0.043 | 0.054 | 57 | 0.02 | 2 | 0.05 | 0.07 | NS | 0.059 | 0.07 |
| 27 | 0.02 | 2 | 0.05 | 0.035 | 0.5 | 0.054 | 0.065 | 58 | 0.02 | 2 | 0.05 | 0.07 | 0 | 0.039 | 0.048 |
| 28 | 0.02 | 2 | 0.05 | 0.035 | 1 | 0.06 | 0.072 | 59 | 0.02 | 2 | 0.05 | 0.07 | 0.5 | 0.051 | 0.058 |
| 29 | 0.02 | 3 | 0.05 | 0.035 | NS | 0.067 | 0.079 | 60 | 0.02 | 2 | 0.05 | 0.07 | 1 | 0.056 | 0.065 |
| 30 | 0.02 | 3 | 0.05 | 0.035 | 0 | 0.048 | 0.061 | 61 | 0.02 | 3 | 0.05 | 0.07 | NS | 0.065 | 0.074 |
| 31 | 0.02 | 3 | 0.05 | 0.035 | 0.5 | 0.056 | 0.068 | 62 | 0.02 | 3 | 0.05 | 0.07 | 0 | 0.044 | 0.053 |
| 63 | 0.02 | 3 | 0.05 | 0.07 | 0.5 | 0.053 | 0.062 | 96 | 0.02 | 3 | 0.05 | 0.105 | 1 | 0.06 | 0.068 |
| 64 | 0.02 | 3 | 0.05 | 0.07 | 1 | 0.059 | 0.07 | 97 | 0.03 | 2 | 0.03 | 0.105 | NS | 0.067 | 0.08 |
| 65 | 0.03 | 2 | 0.03 | 0.07 | NS | 0.068 | 0.081 | 98 | 0.03 | 2 | 0.03 | 0.105 | 0 | 0.04 | 0.054 |
| 66 | 0.03 | 2 | 0.03 | 0.07 | 0 | 0.042 | 0.056 | 99 | 0.03 | 2 | 0.03 | 0.105 | 0.5 | 0.053 | 0.063 |
| 67 | 0.03 | 2 | 0.03 | 0.07 | 0.5 | 0.056 | 0.066 | 100 | 0.03 | 2 | 0.03 | 0.105 | 1 | 0.061 | 0.07 |
| 68 | 0.03 | 2 | 0.03 | 0.07 | 1 | 0.061 | 0.072 | 101 | 0.03 | 3 | 0.03 | 0.105 | NS | 0.07 | 0.083 |
| 69 | 0.03 | 3 | 0.03 | 0.07 | NS | 0.071 | 0.085 | 102 | 0.03 | 3 | 0.03 | 0.105 | 0 | 0.043 | 0.056 |
| 70 | 0.03 | 3 | 0.03 | 0.07 | 0 | 0.047 | 0.058 | 103 | 0.03 | 3 | 0.03 | 0.105 | 0.5 | 0.057 | 0.064 |
| 71 | 0.03 | 3 | 0.03 | 0.07 | 0.5 | 0.06 | 0.067 | 104 | 0.03 | 3 | 0.03 | 0.105 | 1 | 0.064 | 0.076 |
| 72 | 0.03 | 3 | 0.03 | 0.07 | 1 | 0.065 | 0.077 | 105 | 0.03 | 2 | 0.05 | 0.105 | NS | 0.071 | 0.085 |
| 73 | 0.03 | 2 | 0.05 | 0.07 | NS | 0.069 | 0.083 | 106 | 0.03 | 2 | 0.05 | 0.105 | 0 | 0.042 | 0.054 |
| 74 | 0.03 | 2 | 0.05 | 0.07 | 0 | 0.047 | 0.053 | 107 | 0.03 | 2 | 0.05 | 0.105 | 0.5 | 0.057 | 0.065 |
| 75 | 0.03 | 2 | 0.05 | 0.07 | 0.5 | 0.057 | 0.063 | 108 | 0.03 | 2 | 0.05 | 0.105 | 1 | 0.066 | 0.072 |
| 76 | 0.03 | 2 | 0.05 | 0.07 | 1 | 0.063 | 0.071 | 109 | 0.03 | 3 | 0.05 | 0.105 | NS | 0.074 | 0.09 |
| 77 | 0.03 | 3 | 0.05 | 0.07 | NS | 0.071 | 0.087 | 110 | 0.03 | 3 | 0.05 | 0.105 | 0 | 0.05 | 0.059 |
| 78 | 0.03 | 3 | 0.05 | 0.07 | 0 | 0.052 | 0.058 | 111 | 0.03 | 3 | 0.05 | 0.105 | 0.5 | 0.062 | 0.069 |
| 79 | 0.03 | 3 | 0.05 | 0.07 | 0.5 | 0.06 | 0.69 | 112 | 0.03 | 3 | 0.05 | 0.105 | 1 | 0.068 | 0.075 |
| 80 | 0.03 | 3 | 0.05 | 0.07 | 1 | 0.065 | 0.074 | 113 | 0.02 | 2 | 0.03 | 0.14 | NS | 0.051 | 0.059 |
| 81 | 0.02 | 2 | 0.03 | 0.105 | NS | 0.054 | 0.063 | 114 | 0.02 | 2 | 0.03 | 0.14 | 0 | 0.031 | 0.037 |
| 82 | 0.02 | 2 | 0.03 | 0.105 | 0 | 0.036 | 0.04 | 115 | 0.02 | 2 | 0.03 | 0.14 | 0.5 | 0.044 | 0.05 |
| 83 | 0.02 | 2 | 0.03 | 0.105 | 0.5 | 0.047 | 0.052 | 116 | 0.02 | 2 | 0.03 | 0.14 | 1 | 0.047 | 0.052 |
| 84 | 0.02 | 2 | 0.03 | 0.105 | 1 | 0.052 | 0.057 | 117 | 0.02 | 3 | 0.03 | 0.14 | NS | 0.053 | 0.062 |
| 85 | 0.02 | 3 | 0.03 | 0.105 | NS | 0.058 | 0.067 | 118 | 0.02 | 3 | 0.03 | 0.14 | 0 | 0.032 | 0.039 |
| 86 | 0.02 | 3 | 0.03 | 0.105 | 0 | 0.037 | 0.043 | 119 | 0.02 | 3 | 0.03 | 0.14 | 0.5 | 0.045 | 0.05 |
| 87 | 0.02 | 3 | 0.03 | 0.105 | 0.5 | 0.048 | 0.053 | 120 | 0.02 | 3 | 0.03 | 0.14 | 1 | 0.05 | 0.056 |
| 88 | 0.02 | 3 | 0.03 | 0.105 | 1 | 0.052 | 0.058 | 121 | 0.02 | 2 | 0.05 | 0.14 | NS | 0.057 | 0.064 |
| 89 | 0.02 | 2 | 0.05 | 0.105 | NS | 0.057 | 0.065 | 122 | 0.02 | 2 | 0.05 | 0.14 | 0 | 0.032 | 0.04 |
| 90 | 0.02 | 2 | 0.05 | 0.105 | 0 | 0.03 | 0.043 | 123 | 0.02 | 2 | 0.05 | 0.14 | 0.5 | 0.047 | 0.051 |
| 91 | 0.02 | 2 | 0.05 | 0.105 | 0.5 | 0.047 | 0.053 | 124 | 0.02 | 2 | 0.05 | 0.14 | 1 | 0.051 | 0.055 |
| 92 | 0.02 | 2 | 0.05 | 0.105 | 1 | 0.053 | 0.062 | 125 | 0.02 | 3 | 0.05 | 0.14 | NS | 0.06 | 0.069 |
| 93 | 0.02 | 3 | 0.05 | 0.105 | NS | 0.062 | 0.071 | 126 | 0.02 | 3 | 0.05 | 0.14 | 0 | 0.036 | 0.043 |
| 94 | 0.02 | 3 | 0.05 | 0.105 | 0 | 0.034 | 0.046 | 127 | 0.02 | 3 | 0.05 | 0.14 | 0.5 | 0.049 | 0.053 |
| 95 | 0.02 | 3 | 0.05 | 0.105 | 0.5 | 0.051 | 0.059 | 128 | 0.02 | 3 | 0.05 | 0.14 | 1 | 0.053 | 0.06 |
| 129 | 0.03 | 2 | 0.03 | 0.14 | NS | 0.065 | 0.078 | 137 | 0.03 | 2 | 0.05 | 0.14 | NS | 0.072 | 0.089 |
| 130 | 0.03 | 2 | 0.03 | 0.14 | 0 | 0.041 | 0.051 | 138 | 0.03 | 2 | 0.05 | 0.14 | 0 | 0.044 | 0.054 |
| 131 | 0.03 | 2 | 0.03 | 0.14 | 0.5 | 0.054 | 0.06 | 139 | 0.03 | 2 | 0.05 | 0.14 | 0.5 | 0.06 | 0.069 |
| 132 | 0.03 | 2 | 0.03 | 0.14 | 1 | 0.06 | 0.069 | 140 | 0.03 | 2 | 0.05 | 0.14 | 1 | 0.066 | 0.073 |
| 133 | 0.03 | 3 | 0.03 | 0.14 | NS | 0.068 | 0.08 | 141 | 0.03 | 3 | 0.05 | 0.14 | NS | 0.076 | 0.093 |
| 134 | 0.03 | 3 | 0.03 | 0.14 | 0 | 0.043 | 0.053 | 142 | 0.03 | 3 | 0.05 | 0.14 | 0 | 0.051 | 0.06 |
| 135 | 0.03 | 3 | 0.03 | 0.14 | 0.5 | 0.058 | 0.062 | 143 | 0.03 | 3 | 0.05 | 0.14 | 0.5 | 0.065 | 0.072 |
| 136 | 0.03 | 3 | 0.03 | 0.14 | 1 | 0.062 | 0.074 | 144 | 0.03 | 3 | 0.05 | 0.14 | 1 | 0.07 | 0.077 |
| Relative Scour Hole Width (aw) | Tpc/D | dp/D | m | Relative Scour Hole Length (al) | Tpc/D | dp/D | m | Sill Position (Xs/Lpc) | Z/D |
|---|---|---|---|---|---|---|---|---|---|
| 3.06 | 1.42 | 0.57 | 2 | 1.88 | 1.42 | 0.57 | 2 | No sill | 0 |
| 2.82 | 1.42 | 0.85 | 2 | 1.79 | 1.42 | 0.85 | 2 | No sill | |
| 5.06 | 0.85 | 0.85 | 2 | 1.92 | 1.42 | 0.85 | 2 | 0 | |
| 5.38 | 0.85 | 0.57 | 2 | 2.11 | 0.85 | 0.85 | 2 | 0 | |
| 4.44 | 1.42 | 0.57 | 2 | 1.82 | 0.85 | 0.85 | 2 | 0.5 | |
| 4.84 | 1.42 | 0.85 | 2 | 1.94 | 1.42 | 0.85 | 2 | 0.5 | |
| 3.23 | 0.85 | 0.57 | 2 | 1.77 | 0.85 | 0.57 | 2 | 1 | |
| 2.86 | 0.85 | 0.85 | 2 | 1.89 | 1.42 | 0.85 | 2 | 1 | |
| 2.65 | 0.85 | 0.85 | 2 | 1.63 | 1.42 | 0.85 | 2 | No sill | 1 |
| 3.04 | 1.42 | 0.57 | 3 | 1.9 | 1.42 | 0.57 | 3 | No sill | |
| 5.95 | 0.85 | 0.57 | 2 | 2.13 | 0.85 | 0.57 | 2 | 0 | |
| 4.92 | 1.42 | 0.57 | 3 | 1.92 | 1.42 | 0.85 | 3 | 0 | |
| 4.1 | 1.42 | 0.57 | 3 | 1.76 | 0.85 | 0.85 | 2 | 0.5 | |
| 4.7 | 0.85 | 0.85 | 3 | 1.91 | 0.85 | 0.85 | 3 | 0.5 | |
| 2.53 | 1.42 | 0.85 | 3 | 1.65 | 1.42 | 0.85 | 3 | 1 | |
| 3.30 | 0.85 | 0.57 | 2 | 1.85 | 0.85 | 0.57 | 2 | 1 | |
| 2.53 | 1.42 | 0.85 | 3 | 1.73 | 0.85 | 0.85 | 2 | No sill | 2 |
| 3.15 | 1.42 | 0.57 | 2 | 1.92 | 0.85 | 0.57 | 2 | No sill | |
| 5.36 | 0.85 | 0.85 | 2 | 1.89 | 1.42 | 0.85 | 2 | 0 | |
| 6.97 | 0.85 | 0.57 | 2 | 2.11 | 1.42 | 0.85 | 3 | 0 | |
| 4.44 | 1.42 | 0.85 | 2 | 1.79 | 0.85 | 0.57 | 2 | 0.5 | |
| 4.82 | 1.42 | 0.57 | 2 | 2.02 | 1.42 | 0.57 | 3 | 0.5 | |
| 2.82 | 1.42 | 0.85 | 2 | 1.69 | 0.85 | 0.57 | 2 | 1 | |
| 3.67 | 0.85 | 0.57 | 3 | 1.97 | 1.42 | 0.85 | 2 | 1 | |
| 2.5 | 0.85 | 0.85 | 2 | 1.63 | 0.85 | 0.85 | 2 | No sill | 3 |
| 3.18 | 0.85 | 0.57 | 2 | 1.9 | 0.85 | 0.57 | 2 | No sill | |
| 5.36 | 0.85 | 0.85 | 3 | 1.86 | 1.42 | 0.57 | 2 | 0 | |
| 6.5 | 0.85 | 0.57 | 3 | 2.12 | 1.42 | 0.85 | 3 | 0 | |
| 4.64 | 1.42 | 0.85 | 3 | 1.7 | 1.42 | 0.57 | 2 | 0.5 | |
| 5.28 | 0.85 | 0.57 | 3 | 2 | 1.42 | 0.85 | 2 | 0.5 | |
| 2.63 | 0.85 | 0.85 | 3 | 1.61 | 1.42 | 0.57 | 2 | 1 | |
| 3.45 | 0.85 | 0.57 | 3 | 1.94 | 1.42 | 0.85 | 2 | 1 | |
| 2.5 | 0.85 | 0.85 | 3 | 1.54 | 0.85 | 0.85 | 2 | No sill | 4 |
| 3.05 | 0.85 | 0.57 | 2 | 1.86 | 0.85 | 0.57 | 2 | No sill | |
| 5.66 | 0.85 | 0.85 | 3 | 1.76 | 0.85 | 0.85 | 2 | 0 | |
| 6.67 | 0.85 | 0.57 | 3 | 2.17 | 1.42 | 0.85 | 3 | 0 | |
| 4.4 | 0.85 | 0.57 | 2 | 1.7 | 1.42 | 0.57 | 2 | 0.5 | |
| 5.2 | 0.85 | 0.57 | 3 | 2.08 | 1.42 | 0.85 | 3 | 0.5 | |
| 2.7 | 0.85 | 0.85 | 3 | 1.59 | 0.85 | 0.85 | 2 | 1 | |
| 3.33 | 1.42 | 0.57 | 3 | 1.95 | 1.42 | 0.85 | 3 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Esmaeili Varaki, M.; Tavazo, N.; Radice, A. Using a Bed Sill as a Countermeasure for Clear-Water Scour at a Complex Pier with Inclined Columns Footed on Capped Piles. Hydrology 2022, 9, 65. https://doi.org/10.3390/hydrology9040065
Esmaeili Varaki M, Tavazo N, Radice A. Using a Bed Sill as a Countermeasure for Clear-Water Scour at a Complex Pier with Inclined Columns Footed on Capped Piles. Hydrology. 2022; 9(4):65. https://doi.org/10.3390/hydrology9040065
Chicago/Turabian StyleEsmaeili Varaki, Mahdi, Negar Tavazo, and Alessio Radice. 2022. "Using a Bed Sill as a Countermeasure for Clear-Water Scour at a Complex Pier with Inclined Columns Footed on Capped Piles" Hydrology 9, no. 4: 65. https://doi.org/10.3390/hydrology9040065
APA StyleEsmaeili Varaki, M., Tavazo, N., & Radice, A. (2022). Using a Bed Sill as a Countermeasure for Clear-Water Scour at a Complex Pier with Inclined Columns Footed on Capped Piles. Hydrology, 9(4), 65. https://doi.org/10.3390/hydrology9040065







