Flood Mapping from Dam Break Due to Peak Inflow: A Coupled Rainfall–Runoff and Hydraulic Models Approach
Abstract
1. Introduction
Study Area
2. Materials and Methods
2.1. Data Acquisition
2.1.1. Land Surface Data
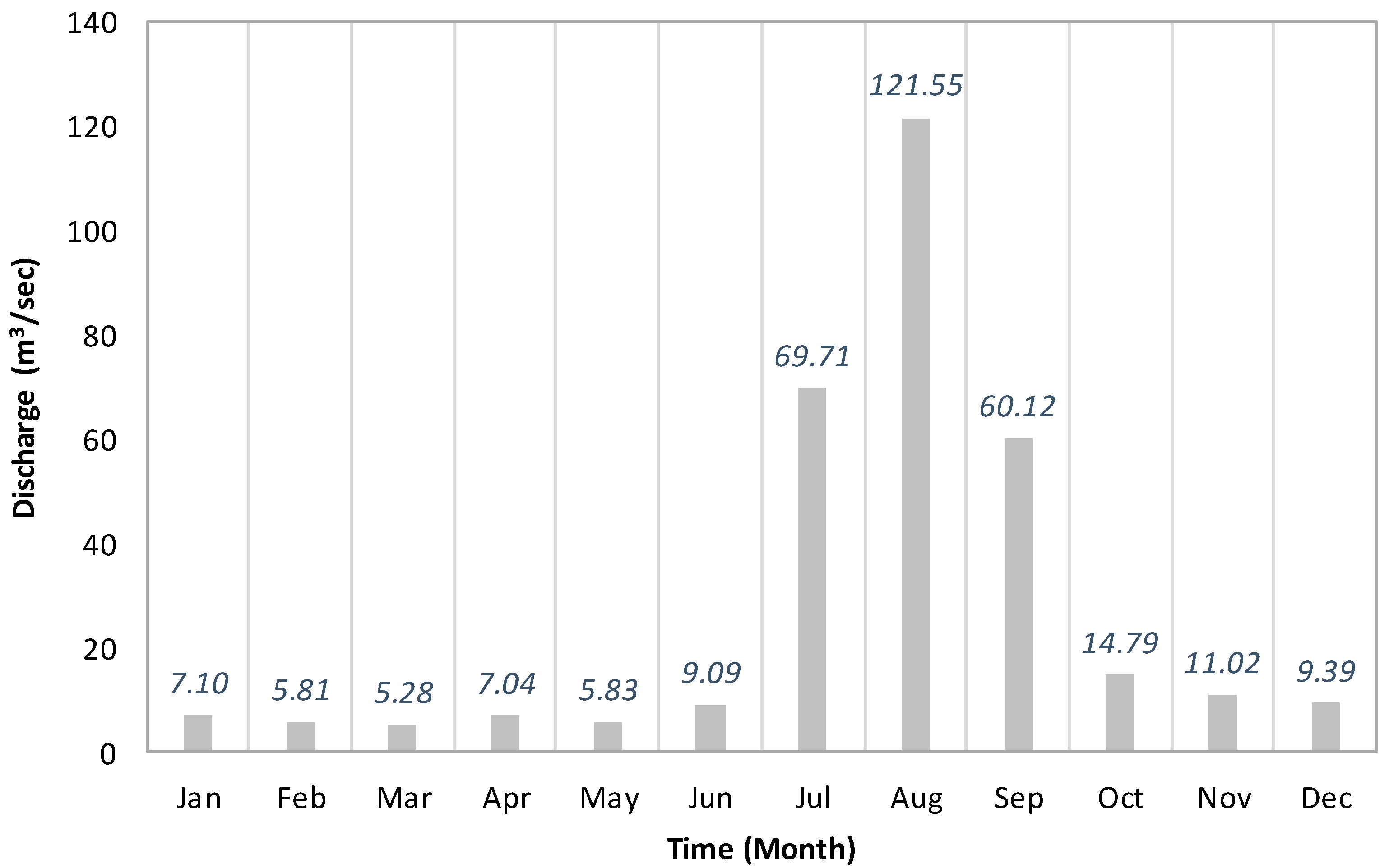
2.1.2. Meteorological and Hydrological Data
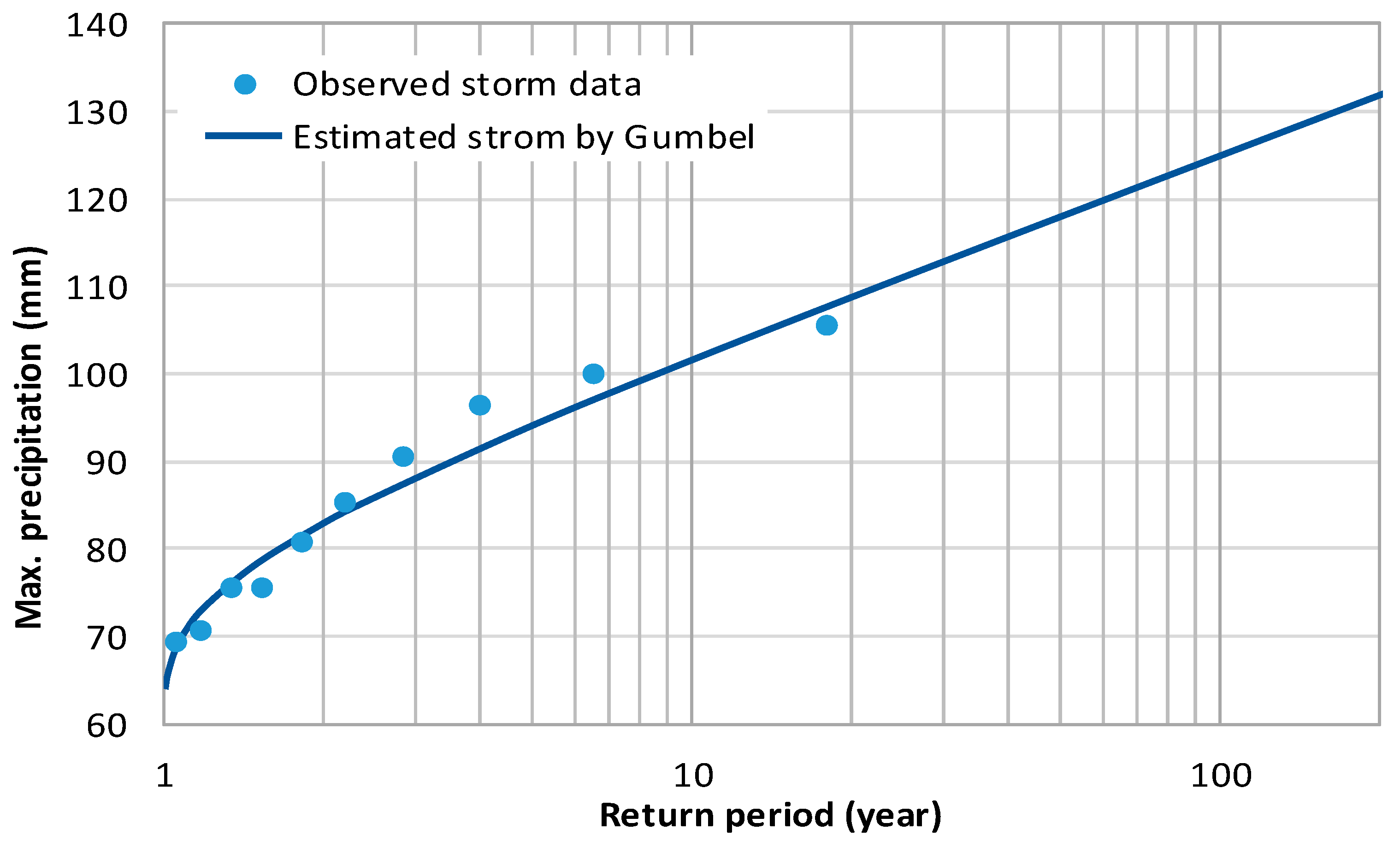
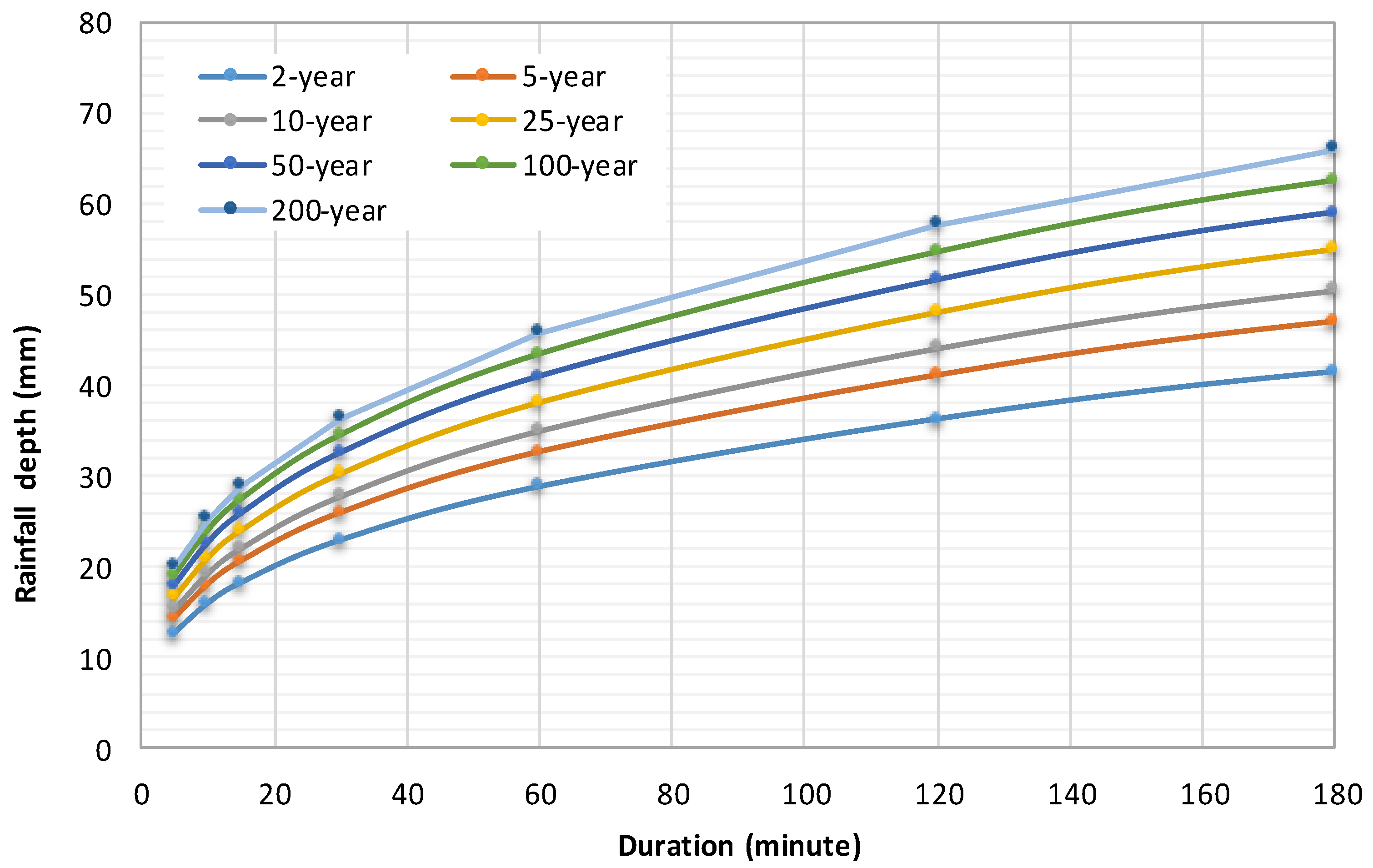
2.2. Estimation of the Designed Storm
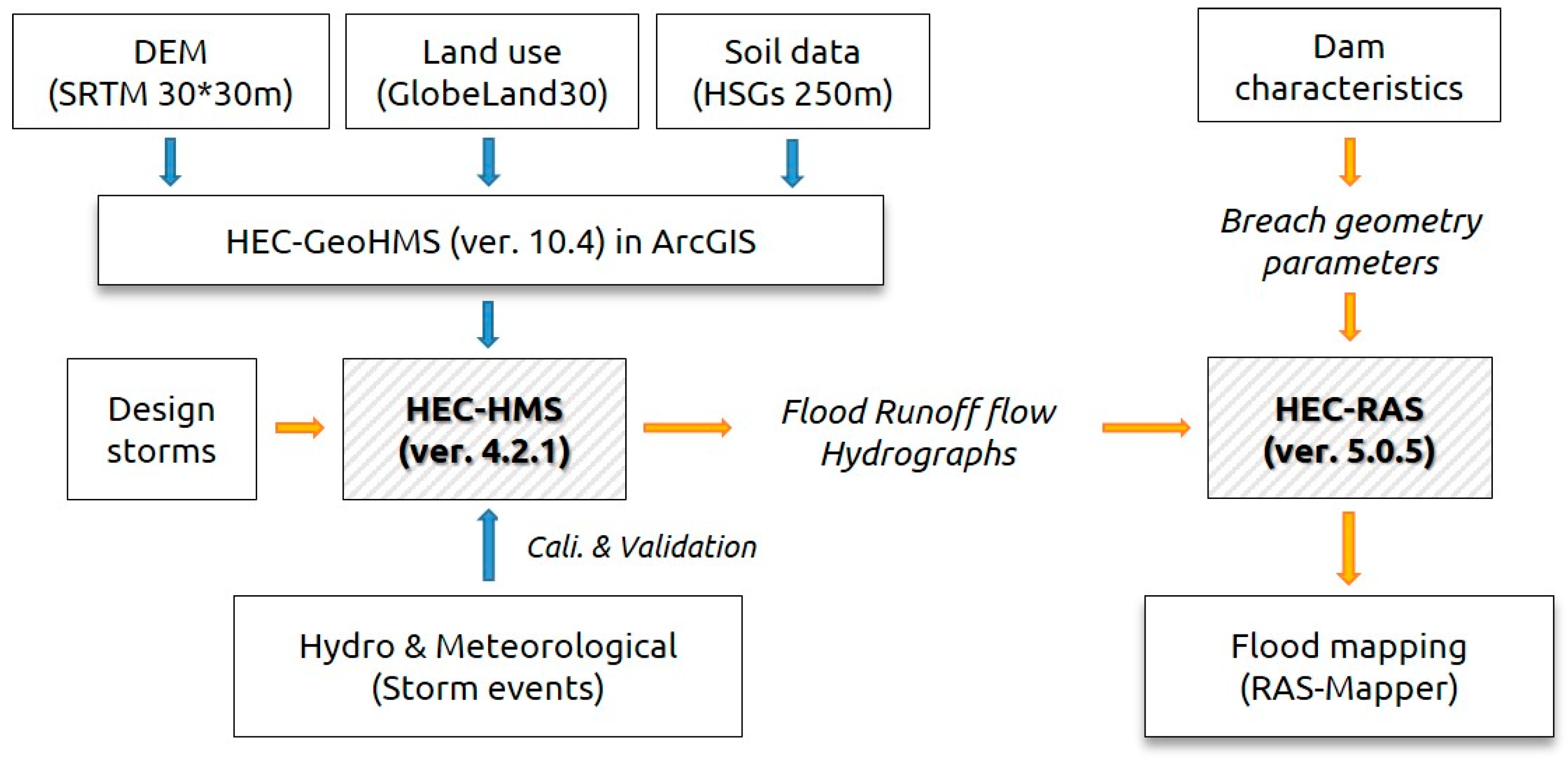
2.3. Hydrologic Modeling (HEC-HMS)
2.4. Hydraulic Modeling (HEC-RAS)
2.5. 2-D Flood Mapping
3. Results
3.1. Designed Storm
3.2. Hydrologic Simulation (Flood Hydrograph)
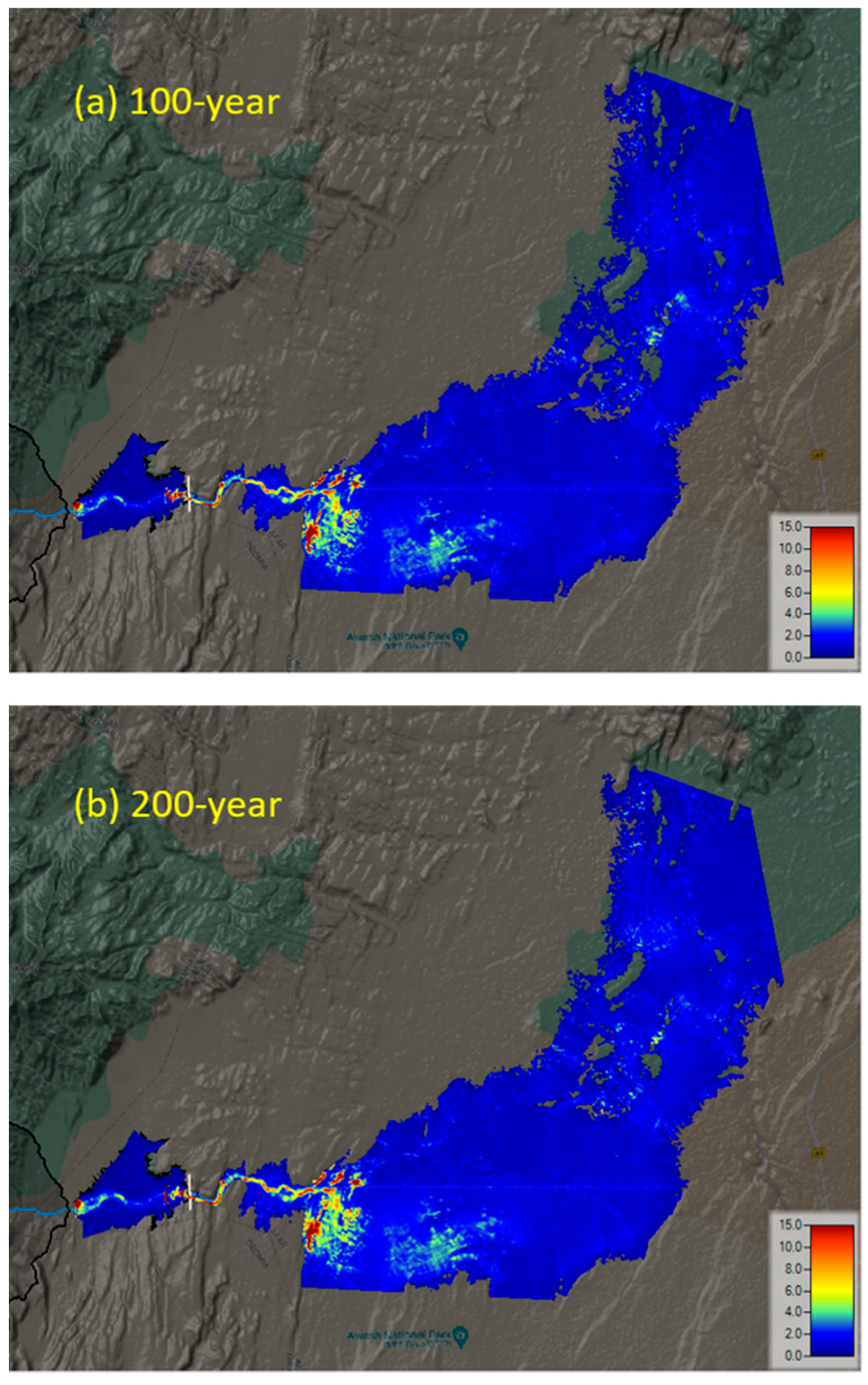
3.3. Hydraulic Simulation (Flood Mapping)
4. Discussion
5. Conclusions
- The use of high-temporal-resolution hydro-meteorological data (i.e., precipitation and streamflow), for flow estimation, and high-spatial-resolution topographic data (i.e., DEM, land use, and soil), for flood inundation mapping, performs well.
- The flood hydrographs produced from event-based runoff estimation by the SCS curve number method displayed suitable results for application to peak storm events.
- The Kesem dam, from the empirical dam break simulations, shows possible failure for the 100- and 200-year return period inflows, whereas the dam remains safe for inflows up to the 50-year return period. This may indicate user-defined breaching, with the HEC-RAS models determining the dam breach time and dimension depending on the breach trigger inputs, such as inflow boundary conditions.
- The 2D flood map provides a satisfactory spatial and temporal resolution result for the inundated area. Furthermore, 2D flood mapping can be used to better understand the flood inundation extent of any type of flood.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cannata, M.; Marzocchi, R. Two-dimensional dam break flooding simulation: A GIS-embedded approach. Nat. Hazards 2012, 61, 1143–1159. [Google Scholar] [CrossRef]
- Science Engineering & Sustainability. 2019. Dam Break Simulation with HEC-RAS: Chepete Proposed Dam. Available online: https://sciengsustainability.blogspot.com/2019/03/dam-break-simulation-hec-ras.html (accessed on 5 May 2019).
- Koskinas, A.; Tegos, A.; Tsira, P.; Dimitriadis, P.; Iliopoulou, T.; Papanicolaou, P.; Koutsoyiannis, D.; Williamson, T. Insights into the Oroville Dam 2017 Spillway Incident. Geosciences 2019, 9, 37. [Google Scholar] [CrossRef]
- Rotta, L.H.; Alcantara, E.; Park, E.; Negri, R.G.; Lin, Y.N.; Bernardo, N.; Mendes, T.S.; Souza Filho, C.R. The 2019 Brumadinho tailings dam collapse: Possible cause and impacts of the worst human and environmental disaster in Brazil. Int. J. Appl. Earth Obs. Geoinf. 2020, 90, 102119. [Google Scholar] [CrossRef]
- Latrubesse, E.M.; Park, E.; Sieh, K.; Dang, T.; Lin, Y.N.; Yun, S.H. Dam failure and a catastrophic flood in the Mekong basin (Bolaven Plateau), southern Laos, 2018. Geomorphology 2020, 362, 107221. [Google Scholar] [CrossRef]
- Dave, P. Hidroituango: Another Landslide Crisis at a Hydroelectric Dam. The Landslide Blog, AGU. 2018. Available online: https://blogs.agu.org/landslideblog/2018/05/21/hidroituango-1/ (accessed on 5 April 2019).
- Aljazeera News. 2018. Search for Survivors after Deadly Kenya Dam Collapse. Available online: https://www.aljazeera.com/news/2018/05/search-survivors-deadly-kenya-dam-collapse-180511054548148.html (accessed on 5 May 2019).
- Zin, W.W.; Kawasaki, A.; Takeuchi, W.; San, Z.M.L.T.; Htun, K.Z.; Aye, T.H.; Win, S. Flood hazard assessment of Bago River Basin, Myanmar. J. Disaster Res. 2018, 13, 14–21. [Google Scholar] [CrossRef]
- Mao, J.; Wang, S.; Ni, J.; Xi, C.; Wang, J. Management System for Dam-Break Hazard Mapping in a Complex Basin Environment. ISPRS Int. J. Geo-Inf. 2017, 6, 162. [Google Scholar] [CrossRef]
- Rodrigues, A.S.; Santos, M.A.; Santos, A.D.; Rocha, F. Dam-break flood emergency management system. Water Resour. Manag. 2002, 16, 489–503. [Google Scholar] [CrossRef]
- Tsakiris, G.D. Flood risk assessment: Concepts, modelling, applications. Nat. Hazards Earth Syst. Sci. 2014, 14, 1361–1369. [Google Scholar] [CrossRef]
- Urzică, A.; Mihu-Pintilie, A.; Stoleriu, C.C.; Cîmpianu, C.I.; Huţanu, E.; Pricop, C.I.; Grozavu, A. Using 2D HEC-RAS Modeling and Embankment Dam Break Scenario for Assessing the Flood Control Capacity of a Multi-Reservoir System (NE Romania). Water 2020, 13, 57. [Google Scholar] [CrossRef]
- Albu, L.-M.; Enea, A.; Iosub, M.; Breabăn, I.-G. Dam Breach Size Comparison for Flood Simulations. A HEC-RAS Based, GIS Approach for Drăcșani Lake, Sitna River, Romania. Water 2020, 12, 1090. [Google Scholar] [CrossRef]
- Cho, Y. Application of NEXRAD Radar-Based Quantitative Precipitation Estimations for Hydrologic Simulation Using ArcPy and HEC Software. Water 2020, 12, 273. [Google Scholar] [CrossRef]
- Tahmasbinejad, H.; Feyzolahpour, M.; Mumipour, M.; Zakerhoseini, F. Rainfall-runoff Simulation and Modeling of Karon River Using HEC-RAS and HEC-HMS Models, Izeh District, Iran. J. Appl. Sci. 2012, 12, 1900–1908. [Google Scholar] [CrossRef]
- Horritt, M.; Bates, P. Evaluation of 1D and 2D numerical models for predicting river flood inundation. J. Hydrol. 2002, 268, 87–99. [Google Scholar] [CrossRef]
- Jayakrishnan, R.; Srinivasan, R.; Arnold, J. Comparison of raingage and WSR-88D Stage III precipitation data over the Texas-Gulf basin. J. Hydrol. 2004, 292, 135–152. [Google Scholar] [CrossRef]
- CEIWR-HEC. HEC-RAS, River Analysis System: Hydraulic Reference Manual; US Army Corps of Engineers Hydrologic Engineering Center: Davis, CA, USA, 2016. [Google Scholar]
- Bhandari, M.; Nyaupane, N.; Mote, S.R.; Kalra, A.; Ahmad, S. 2D Unsteady Flow Routing and Flood Inundation Mapping for Lower Region of Brazos River Watershed. In World Environmental and Water Resources Congress 2017; Digital Scholarship@UNLV: Sacramento, CA, USA, 2017; pp. 292–303. [Google Scholar] [CrossRef]
- Brunner, M.I.; Seibert, J.; Favre, A. Bivariate return periods and their importance for flood peak and volume estimation. Wiley Interdiscip. Rev. Water 2016, 3, 819–833. [Google Scholar] [CrossRef]
- Yang, Z.; Chen, W.-P. Earthquakes along the East African Rift System: A multiscale, system-wide perspective. J. Geophys. Res. Solid Earth 2010, 115. [Google Scholar] [CrossRef]
- Ayele, A.; Ebinger, C.J.; van Alstyne, C.; Keir, D.; Nixon, C.W.; Belachew, M.; Hammond, J.O.S. Seismicity of the central Afar rift and implications for Tendaho dam hazards. Geol. Soc. Lond. Spéc. Publ. 2016, 420, 341–354. [Google Scholar] [CrossRef]
- Yared, M.G.; Nigussie, T.; Yohannis, B.T. Dam Breach Modeling and Flood Inundation Mapping a Case Study on Kesem Dam. ACADEMIA. 2016. Available online: https://www.academia.edu/27150269/Dam_Breach_Modelling_and_Flood_Inundation_Mapping_A_Case_Study_on_Kesem_Dam (accessed on 5 May 2019).
- CEIWR-HEC; HEC-HMS. Hydrological Modeling System: Application Guide; US Army Corps of Engineers Hydrologic Engineering Center: Davis, CA, USA, 2017; pp. 3.1–3.19. [Google Scholar]
- MoWIE, Kesem Kebena Dam and Irrigation Project Final Feasibility study and Dam Design Report. Available online: http://www.fao.org/3/ar867e/ar867e.pdf (accessed on 12 April 2019).
- Ross, C.W.; Prihodko, L.; Anchang, J.; Kumar, S.; Ji, W.J.; Hanan, N.P. HYSOGs250m, global gridded hydrologic soil groups for curve-number-based runoff modeling. Sci. Data 2018, 5, 180091. [Google Scholar] [CrossRef] [PubMed]
- USDA NRCS. Urban Hydrology for Small Watersheds; US Dept. of Agriculture, Soil Conservation Service, Engineering Division: Washington, DC, USA, 1986.
- National Catalog Service for Geographic Information. Globeland30. Available online: http://www.webmap.cn/main.do?method=index (accessed on 3 February 2019).
- Subyani, A.M. Hydrologic behavior and flood probability for selected arid basins in Makkah area, western Saudi Arabia. Arab. J. Geosci. 2011, 4, 817–824. [Google Scholar] [CrossRef]
- CEIWR-HEC. Hydrologic Modeling System HEC-HMS, Technical Reference Manual; U.S. Army Corps of Engineers, Hydrologic Engineering Center (HEC): Davis, CA, USA, 2000. [Google Scholar]
- Maidment, D.R.; Morehouse, S. Arc Hydro: GIS for Water Resources; ESRI, Inc.: Redlands, CA, USA, 2002. [Google Scholar]
- CEIWR-HEC; HEC-GeoHMS. Geospatial Hydrologic Modeling Extension: Hydraulic Reference Manual; US Army Corps of Engineers Hydrologic Engineering Center: Davis, CA, USA, 2013. [Google Scholar]
- Getahun, Y.S.; Gebre, S.L. Flood hazard assessment and mapping of flood inundation area of the Awash River basin in Ethiopia using GIS and HEC-GeoRAS/HEC-RAS model. J. Civ. Environ. Eng. 2015, 5, 1. [Google Scholar] [CrossRef]
- Behailu, S. Stream Flow Simulation for the Upper Awash Basin. Ph.D. Thesis, Faculty of Technology Department of Civil Engineering, Addis Ababa University, Addis Ababa, Ethiopia, 2004. [Google Scholar]
- Kisi, O.; Shiri, J.; Tombul, M. Modeling rainfall-runoff process using soft computing techniques. Comput. Geosci. 2013, 51, 108–117. [Google Scholar] [CrossRef]
- CEIWR-HEC; HEC-GeoRAS. GIS Tools for Support of HEC-RAS Using ArcGIS. [Online] Version 4.3.93; US Army Corps of Engineers, Institute of water Resources: Davis, CA, USA, 2011. [Google Scholar]
- Froehlich, D.C. Embankment Dam Breach Parameters and Their Uncertainties. J. Hydraul. Eng. 2008, 134, 1708–1721. [Google Scholar] [CrossRef]
- Wahl, T. Evaluation of Erodibility-Based Embankment Dam Breach Equations (Hydraulic Laboratory Report HL-2014-02); US Department of the Interior Bureau of Reclamation: Denver, CO, USA, 2014; p. 99.
- Thornton, C.I.; Pierce, M.W.; Abt, S.R. Enhanced Predictions for Peak Outflow from Breached Embankment Dams. J. Hydrol. Eng. 2011, 16, 81–88. [Google Scholar] [CrossRef]
- Yu, D.; Lane, S.N. Urban fluvial flood modelling using a two-dimensional diffusion-wave treatment, part 1: Mesh resolution effects. Hydrol. Process. 2005, 20, 1541–1565. [Google Scholar] [CrossRef]
- Chow, V.T. Open-Channel Hydraulics; McGraw-Hill Civil Engineering Series; McGraw-Hill Book Company Inc.: New York, NY, USA, 1959. [Google Scholar]
- Michaud, J.; Johnson, C.; Iokepa, J.; Marohnic, J. Methods for Estimating the Impact of Hypothetical Dam Break Floods. In Chemistry for the Protection of the Environment; Springer: Boston, MA, USA, 2005; pp. 195–199. [Google Scholar] [CrossRef]
- Onyutha, C.; Tabari, H.; Taye, M.T.; Nyandwaro, G.N.; Willems, P. Analyses of rainfall trends in the Nile River Basin. J. Hydro-Environ. Res. 2016, 13, 36–51. [Google Scholar] [CrossRef]
- Basheer, T.A.; Wayayok, A.; Yusuf, B.; Kamal, M. Dam Breach parameters and their influence on flood hydrographs for Mosul Dam. J. Eng. Sci. Technol. 2017, 12, 2896–2908. [Google Scholar]
- Awal, R.; Nakagawa, H.; Kawaike, K.; Baba, Y.; Zhang, H. Experimental study on piping failure of natural dam. J. Jpn. Soc. Civ. Eng. Ser. B1 Hydraul. Eng. 2011, 67, I_157–I_162. [Google Scholar] [CrossRef]
- Psomiadis, E.; Tomanis, L.; Kavvadias, A.; Soulis, K.; Charizopoulos, N.; Michas, S. Potential Dam Breach Analysis and Flood Wave Risk Assessment Using HEC-RAS and Remote Sensing Data: A Multicriteria Approach. Water 2021, 13, 364. [Google Scholar] [CrossRef]
- Wu, M.; Ge, W.; Li, Z.; Wu, Z.; Zhang, H.; Li, J.; Pan, Y. Improved Set Pair Analysis and Its Application to Environmental Impact Evaluation of Dam Break. Water 2019, 11, 821. [Google Scholar] [CrossRef]





| Land Use | Contents | Area (km2) |
|---|---|---|
| Agriculture | Land used for agriculture, horticulture and gardens | 2242.7 |
| Bare land | Land covered by natural grass, shrubs and with vegetation less than 10% | 1095.9 |
| Forest | Land covered with trees, with vegetation cover over 30% | 148.8 |
| Built-up | Lands modified by human activities | 11.1 |
| Water | Water bodies in the land area | 1.4 |
| Type | Name | Latitude (Decimal Degrees) | Longitude (Decimal Degrees) | Period (Years) |
|---|---|---|---|---|
| Precipitation | Addis Ababa | 9.02 | 38.77 | 2012–2018 |
| Precipitation | Metehara | 8.86 | 39.92 | 2012–2018 |
| Precipitation | Debre Birhan | 9.63 | 39.50 | 2012–2018 |
| Stream flow | Kesem Arerti | 9.04 | 39.56 | 2012–2018 |
| Type | Storm Event Period | NSE | MAE | RMSE | R2 | PBIAS |
|---|---|---|---|---|---|---|
| Calibration | 16–19 August 2012 | 0.81 | 75.45 | 117.63 | 0.90 | 22.36 |
| 22–24 August 2013 | 0.50 | 66.51 | 130.25 | 0.66 | −20.34 | |
| 22–24 August 2016 | −0.30 | 109.79 | 179.80 | 0.21 | −39.74 | |
| Validation | 7–9 August 2014 | 0.61 | 21.19 | 86.51 | 0.64 | −9.01 |
| 18–20 August 2015 | 0.75 | 94.92 | 171.33 | 0.86 | 35.24 |
| Return Period | Inundation Area (km2) | Depth (m) | Duration (h) | ||
|---|---|---|---|---|---|
| Maximum | Mean | Maximum | Mean | ||
| 100 years | 208 | 20.06 | 2.04 | 46 | 10 |
| 200 years | 240 | 31.20 | 2.84 | 93 | 39 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tedla, M.G.; Cho, Y.; Jun, K. Flood Mapping from Dam Break Due to Peak Inflow: A Coupled Rainfall–Runoff and Hydraulic Models Approach. Hydrology 2021, 8, 89. https://doi.org/10.3390/hydrology8020089
Tedla MG, Cho Y, Jun K. Flood Mapping from Dam Break Due to Peak Inflow: A Coupled Rainfall–Runoff and Hydraulic Models Approach. Hydrology. 2021; 8(2):89. https://doi.org/10.3390/hydrology8020089
Chicago/Turabian StyleTedla, Mihretab G., Younghyun Cho, and Kyungsoo Jun. 2021. "Flood Mapping from Dam Break Due to Peak Inflow: A Coupled Rainfall–Runoff and Hydraulic Models Approach" Hydrology 8, no. 2: 89. https://doi.org/10.3390/hydrology8020089
APA StyleTedla, M. G., Cho, Y., & Jun, K. (2021). Flood Mapping from Dam Break Due to Peak Inflow: A Coupled Rainfall–Runoff and Hydraulic Models Approach. Hydrology, 8(2), 89. https://doi.org/10.3390/hydrology8020089








