Assessing the Performance of Different Time of Concentration Equations in Urban Ungauged Watersheds: Case Study of Cartagena de Indias, Colombia
Abstract
1. Introduction
2. Study Area and Data
3. Methodology
3.1. Watershed Delineation, Morphometric Parameters, and Flow Types
3.2. Selected Tc Equations
3.3. Determination of Tc Equation Variables
3.4. Tc Equations Performance Assessment
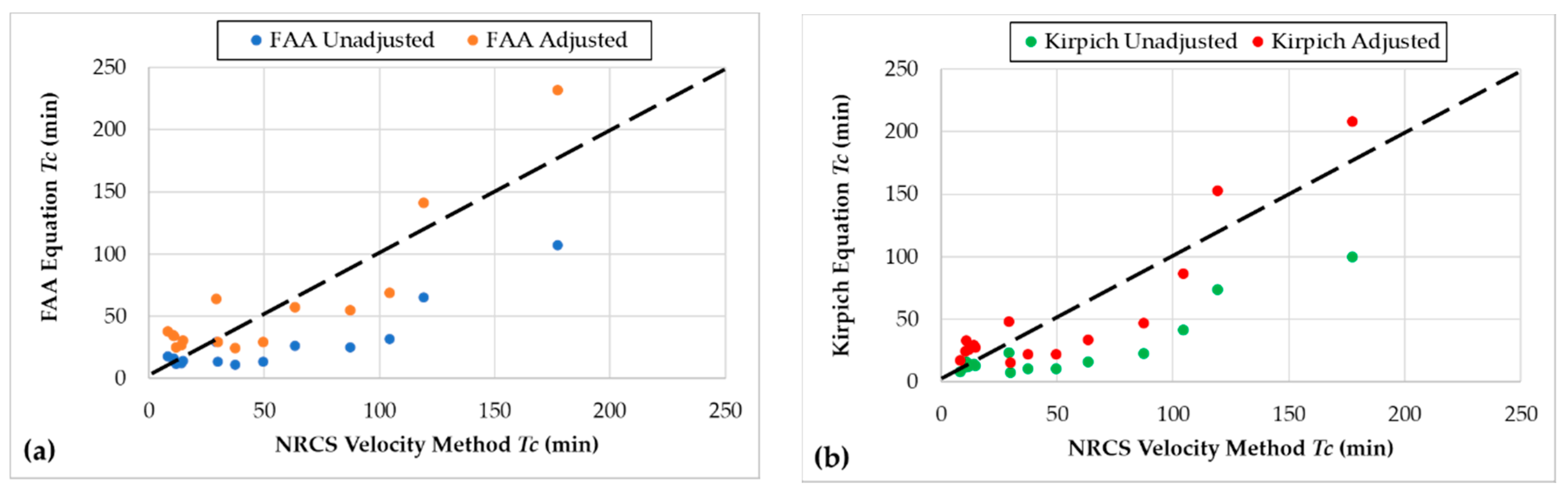
4. Results and Discussion
- (a)
- Equations with two and three variables outperformed Espey–Winslow despite having two additional variables related to land cover (channelization factor and impervious area percentage), which did not bring any advantage, especially in the case of the channelization factor (Table 4 and Tables S7–S10). In fact, Espey–Winslow was the equation with the poorest performance of all (Tables S15 and S16).
- (b)
- The slope and length of each of the different flow types seems to affect the equations’ performance. Their average slope is different, with values of 9.2% and 15.2%, respectively. Additionally, Pie del Cerro Cra 21B has 91% (versus 58% of Pie del Cerro Cra 16A) of its total flow path corresponding to shallow concentrated flow, with a slope of 14.8% (Table 2). In this sense, watershed area and total urbanized area appear to also play a role in the equations’ performance given that the NRCS lag equation and Bransby–Williams performed well in the Bazurto watershed, despite having an average slope of 18.8% (larger than Pie del Cerro Cra 21B), 82% of its total flow path as shallow concentrated flow with a slope of 15.1% (similar to Pie del Cerro Cra 21B), and an area of 66.05 ha (Table 2). These two equations did not perform well in Pie del Cerro Cra 16A and Cra 21B even though the watersheds have similar area sizes and urbanized areas.
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- McCuen, R.H.; Wong, S.L.; Rawls, W.J. Estimating Urban Time of Concentration. J. Hydraul. Eng. 1984, 110, 887–904. [Google Scholar] [CrossRef]
- McCuen, R.H.; Wong, S.L.; Rawls, W.J. Closure to “Estimating Urban Time of Concentration”. J. Hydraul. Eng. 1987, 113, 127–128. [Google Scholar] [CrossRef]
- U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRSC), C.E.D. Urban. Hydrology for Small Watersheds, Technical Release 55 (TR-55); U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRSC), C.E.D.: Washington, DC, USA, 1986.
- Huber, W.C. Discussion of “Estimating Urban. Time of Concentration”. J. Hydraul. Eng. 1987, 113, 122–124. [Google Scholar] [CrossRef]
- McCuen, R.H. Uncertainty Analyses of Watershed Time Parameters. J. Hydrol. Eng. 2009, 14, 490–498. [Google Scholar] [CrossRef]
- Sharifi, S.; Hosseini, S.M. Methodology for Identifying the Best Equations for Estimating the Time of Concentration of Watersheds in a Particular Region. J. Irrig. Drain. Eng. 2011, 137, 712–719. [Google Scholar] [CrossRef]
- Grimaldi, S.; Petroselli, A.; Tauro, F.; Porfiri, M. Temps de concentration: Un paradoxe dans l’hydrologie moderne. Hydrol. Sci. J. 2012, 57, 217–228. [Google Scholar] [CrossRef]
- Gericke, O.J.; Smithers, J.C. Review of methods used to estimate catchment response time for the purpose of peak discharge estimation. Hydrol. Sci. J. 2014, 59, 1935–1971. [Google Scholar] [CrossRef]
- Michailidi, E.M.; Antoniadi, S.; Koukouvinos, A.; Bacchi, B.; Efstratiadis, A. Timing the time of concentration: Shedding light on a paradox. Hydrol. Sci. J. 2018, 63, 721–740. [Google Scholar] [CrossRef]
- Perdikaris, J.; Gharabaghi, B.; Rudra, R. Reference Time of Concentration Estimation for Ungauged Catchments. Earth Sci. Res. 2018, 7, 58. [Google Scholar] [CrossRef]
- U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRCS). Time of Concetration. In National Engineering Handbook (NEH); U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRCS): Washington, DC, USA, 2010; p. 29. [Google Scholar]
- Ontario Ministry of Transportation (OMTO). Drainage Management Manual; Ontario Ministry of Transportation (OMTO): Toronto, ON, Canada, 1997.
- U.S. Department of Transportation Federal Highway Administration (USDOT-FHWA). Hydraulic Engineering Circular No. 22 (HEC-22) . In Urban Drainage Design Manual, 3rd ed.; U.S. Department of Transportation Federal Highway Administration (USDOT-FHWA): Washington, DC, USA, 2009. [Google Scholar]
- Texas Department of Transportation (TxDOT). Hydraulic Design Manual (Revised); Texas Department of Transportation (TxDOT): Austin, TX, USA, 2019.
- U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRCS). Conservation Engineering Division WinTR-20 User Guide; U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRCS): Washington, DC, USA, 2006.
- WinTR-55; (Version 1.00.10); U.S. Department of Agriculture Natural Resources Conservation Service (USDA-NRSC): Washington, DC, USA, 2017.
- HydroCAD Stormwater; (Version 10.1); Modeling System; HydroCAD Software Solutions LLC: Chocorua, NH, USA, 2020.
- Autodesk Storm and Sanitary Analysis; (Version 2019); Autodesk Inc.: San Rafael, CA, USA, 2019.
- Storm Water Management Model (SWMM); (Version 5.1); United States Environmental Agency (USEPA): Washington, DC, USA, 2020.
- Hydrologic Modeling System (HEC-HMS); (Version 4.4.1); U.S. Army Corps of Engineers: Washington, DC, USA, 2018.
- SewerCAD; (Version 10.02.00.55); Bentley Systems: Exton, PA, USA, 2019.
- StormCAD; (Version 10.02.00.55); Bentley Systems: Exton, PA, USA, 2019.
- San Diego State University (SDTU). Online Time of Concentration: Time of Concentration of Small Watersheds. Available online: http://ponce.sdsu.edu/onlinetimeofconcentration.php (accessed on 16 March 2020).
- Manoj, K.C.; Fang, X. Estimating Time of Concentration of Overland Flow on Impervious Surface using Particle Tracking Model. In Proceedings of the World Environmental and Water Resources Congress 2014, Oregon, Portland, 1–5 June 2014; Volume 0300, pp. 30–37. [Google Scholar]
- Yoo, C.; Lee, J.; Park, C.; Jun, C. Method for Estimating Concentration Time and Storage Coefficient of the Clark Model Using Rainfall-Runoff Measurements. J. Hydrol. Eng. 2014, 19, 626–634. [Google Scholar] [CrossRef]
- De Almeida, I.K.; Almeida, A.K.; Steffen, J.L.; Alves Sobrinho, T. Model for Estimating the Time of Concentration in Watersheds. Water Resour. Manag. 2016, 30, 4083–4096. [Google Scholar] [CrossRef]
- Sandoval-Erazo, W.; Toulkeridis, T.; Rodriguez-Espinosa, F.; Mora, M.J.M. Velocity and time of concentration of a basin-A renewed approach applied in the Rio Grande Basin, Ecuador. IOP Conf. Ser. Earth Environ. Sci. 2018, 191. [Google Scholar] [CrossRef]
- Vahabzadeh, G.; Saleh, I.; Safari, A.; Khosravi, K. Determination of the best method of estimating the time of concentration in pasture watersheds (case study: Banadak Sadat and Siazakh Watersheds, Iran). J. Biodivers. Environ. Sci. 2013, 3, 150–159. [Google Scholar]
- Gericke, O.J.; Smithers, J.C. Are estimates of catchment response time inconsistent as used in current flood hydrology practice in South Africa? J. S. Afr. Inst. Civ. Eng. 2016, 58, 2–15. [Google Scholar] [CrossRef]
- De Almeida, I.K.; Almeida, A.K.; Anache, J.A.A.; Steffen, J.L.; Alves Sobrinho, T. Estimation on time of concentration of overland flow in watersheds: A review. Geociencias 2014, 33, 661–671. [Google Scholar]
- Kaufmann de Almeida, I.; Kaufmann Almeida, A.; Garcia Gabas, S.; Alves Sobrinho, T. Performance of methods for estimating the time of concentration in a watershed of a tropical region. Hydrol. Sci. J. 2017, 62, 2406–2414. [Google Scholar] [CrossRef]
- Salimi, E.T.; Nohegar, A.; Malekian, A.; Hoseini, M.; Holisaz, A. Estimating time of concentration in large watersheds. Paddy Water Environ. 2017, 15, 123–132. [Google Scholar] [CrossRef]
- Wong, T.S.W.; Asce, F. Assessment of Time of Concentration Formulas for Overland Flow. J. Irrig. Drain. Eng. 2005, 131, 383–387. [Google Scholar] [CrossRef]
- Fang, X.; Thompson, D.B.; Cleveland, T.G.; Pradhan, P.; Malla, R. Time of Concentration Estimated Using Watershed Parameters Determined by Automated and Manual Methods. J. Irrig. Drain. Eng. 2008, 134, 202–211. [Google Scholar] [CrossRef]
- Wong, T. Evolution of Kinematic Wave Time of Concentration Formulas for Overland Flow. J. Hydrol. Eng. 2009, 14, 739–744. [Google Scholar] [CrossRef]
- Manoj, K.C.; Fang, X.; Yi, Y.J.; Li, M.H.; Cleveland, T.G.; Thompson, D.B. Estimating time of concentration on low-slope planes using diffusion hydrodynamic model. In Proceedings of the World Environmental And Water Resources Congress 2012, Albuquerque, NM, USA, 20–24 May 2012; pp. 360–371. [Google Scholar] [CrossRef]
- Longo, E.; Rooney, J. Development of a General Protocol to Enhance the Hydrological Analysis Techniques for Urban Catchments in Ireland. Geosciences 2018, 8, 252. [Google Scholar] [CrossRef]
- Aristizábal, V.; Hernández, B.; Vélez, J. Manual de Hidrología para Obras Viales Basado en el Uso de Sistemas de Información Geográfica; Universidad Nacional de Colombia: Manizales, Colombia, 2012. [Google Scholar]
- Otálvaro, M. Consideraciones Generales para Realizar el Diseño Hidrológico e Hidráulico de Obras de Drenaje para Vías de Bajos Volúmenes de Tránsito. Caso de Estudio Pista de Prueba Urrao-Antioquia; Universidad de Medellín: Medellín, Colombia, 2016. [Google Scholar]
- Ochoa, T. Hidrología Hidráulica y Socavación en Puentes; ECOE Ediciones Ltda: Bogota, Colombia, 2017. [Google Scholar]
- Instituto Nacional de Vías de Colombia (Invías). Manual de Drenajes para Carreteras (Road Drainage Manual); Ministerio de Transporte de Colombia: Bogotá, Colombia, 2009.
- Ministry of Housing, City, and Territory (MinVivienda), R. of C. Technical Regulations for the Sector of Drinking Water and Basic Sanitation (RAS), Title D, Domestic Wastewater and Stormwater Collection Systems; Ministerio de Vivienda de Colombia: Bogotá, Colombia, 2016.
- Ministry of Housing, City, and Territoty (MinVivienda), R. of C. Resolution 0330 of 8 June 2017, Technical Guidelines for the Sector of Potable Water and Basic Sanitation (RAS); Ministerio de Vivienda de Colombia: Bogotá, Colombia, 2017.
- Vélez, J.J.; Gutiérrez, A. Estimación del Tiempo de Concentración y tiempo de rezago en la Cuenca Experimental Urbana De La Quebrada San Luis, Manizales. Dyna 2011, 78, 58–71. [Google Scholar]
- Torres, J.A.; Ordoñez, J.I.; Duque, R. Análisis de incertidumbre del tiempo de concentración en la modelación de eventos de tormenta en la cuenca alta del Río Magdalena. In Proceedings of the XXV Congreso Latinoamericano de Hidráulica, San José, Costa Rica, 9–12 September 2012. [Google Scholar]
- Montoya, J.; Patiño, J. Estudio Hidrometereológico y Geomorfológico de Diez Quebradas del Valle de Aburrá; Universidad EAFIT: Medellín, Colombia, 2001. [Google Scholar]
- Avila, L.; AÁvila, H.; Sisa, A. A reactive early warning model for urban flash flood management. World Environ. Water Resour. Congr. 2017, 372–382. [Google Scholar] [CrossRef]
- Maidment, D.R. Handbook of Hydrology; MacGraw Hill: New York, NY, USA, 1993. [Google Scholar]
- Welle, P.I.; Woodward, D. Technical Note No. N4, Time of concentration (Revised); U.S. Department of Agriculture National Resources Conservation Service (USDA-NRCS): Chester, PA, USA, 2003.
- Singh, J.; Knapp, H.V.; Arnold, J.G.; Demissie, M. Hydrological modeling of the Iroquois River watershed using HSPF and SWAT. J. Am. Water Resour. Assoc. 2005, 41, 343–360. [Google Scholar] [CrossRef]
- Moriasi, D.N.; Arnold, J.G.; Van Liew, M.W.; Bingner, R.L.; Harmel, R.D.; Veith, T.L. Model Evaluation Guidelines. 2007; Volume 50, pp. 885–900. Available online: https://www.researchgate.net/publication/43261199_Model_Evaluation_Guidelines_for_Systematic_Quantification_of_Accuracy_in_Watershed_Simulations (accessed on 8 May 2020). [CrossRef]
- Moriasi, D.N.; Gitau, M.W.; Pai, N.; Daggupati, P. Hydrologic and Water Quality Models: Performance Measures and Evaluation Criteria. Trans. ASABE 2015, 58, 1763–1785. [Google Scholar] [CrossRef]
- Boskidis, I.; Gikas, G.D.; Sylaios, G.K.; Tsihrintzis, V.A. Hydrologic and Water Quality Modeling of Lower Nestos River Basin. Water Resour. Manag. 2012, 26, 3023–3051. [Google Scholar] [CrossRef]
- Rauf, A.; Ghumman, A. Impact Assessment of Rainfall-Runoff Simulations on the Flow Duration Curve of the Upper Indus River—A Comparison of Data-Driven and Hydrologic Models. Water 2018, 10, 876. [Google Scholar] [CrossRef]
- González-Álvarez, Á.; Coronado-Hernández, O.; Fuertes-Miquel, V.; Ramos, H. Effect of the Non-Stationarity of Rainfall Events on the Design of Hydraulic Structures for Runoff Management and Its Applications to a Case Study at Gordo Creek Watershed in Cartagena de Indias, Colombia. Fluids 2018, 3, 27. [Google Scholar] [CrossRef]
- Instituto de Hidrología Meteorología y Estudios Ambientales (IDEAM) Atlas Climatológico de Colombia 1981–2010. Available online: http://atlas.ideam.gov.co/visorAtlasClimatologico.html (accessed on 8 May 2020).
- Centro de Investigaciones Oceanográficas e Hidrográficas (CIOH). General Circulation of the Atmosphere in Colombia; CIOH: Cartagena de Indias, Colombia, 2010. [Google Scholar]
- Perez, G.; Salazar, I. La Pobreza en Cartagena: Un Análisis por barrios; Banco de La República: Cartagena de Indias, Colombia, 2007; Volume 94. [Google Scholar]
- EPA-Cartagena & UDC. Diseño del sistema inteligente de monitoreo de la calidad ambiental del distrito de Cartagena. Estud. Integr. La Calid. Ambient. Del Sist. Caños Y Lagos 2015, 2. [Google Scholar]
- ArcGIS, (version 10.6.1); Esri Inc.: Redlands, CA, USA, 2019.
- Crippen, R.; Buckley, S.; Agram, P.; Belz, E.; Gurrola, E.; Hensley, S.; Kobrick, M.; Lavalle, M.; Martin, J.; Neumann, M.; et al. NASADEM Global Elevation Model: Methods and Progress. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2016, XLI-B4, 125–128. [Google Scholar] [CrossRef]
- Azizian, A. Uncertainty Analysis of Time of Concentration Equations based on First-Order-Analysis (FOA) Method. Am. J. Eng. Appl. Sci. 2018, 11, 327–341. [Google Scholar] [CrossRef]
- Iowa State University’s Institute for Transportation (InTrans). Iowa Statewide Urban Design and Specifications Program Urban Hydrology and Runoff (2B-3 Time of Concentration). In SUDAS Design Manual; Iowa State University: Ames, IA, USA, 2020. [Google Scholar]
- Miller, W.C. Evolving a shortcut for design of storm sewers. Munic. Util. 1951, 89, 42–59. [Google Scholar]
- Cleveland, T.G.; Thompson, D.B.; Ming-Han Li, X.F. Guidance for Estimation of Time of Concentration in Texas for Low-Slope Conditions (Report # 0-6382-P1); Texas Department of Transportation: Austin, TX, USA, 2012.
- Federal Aviation Agency (FAA). Airport Drainage; Department of Transport Advisory Circular: Washington, DC, USA, 1970.
- Espey, W.H.; Winslow, D.E. The Effects of Urbanisation on Unit Hydrographs for Small Watersheds; Tracor Incorporated: Houston, Austin, TX, USA, 1968; Report No. 68/ 975U and 1006/U. [Google Scholar]
- Kerby, W. Time of concentration for overland flow. Civ. Eng. 1959, 29, 174. [Google Scholar]
- Kirpich, Z. Time of concentration of small agricultural watersheds. Civ. Eng. 1940, 10, 362. [Google Scholar]
- Williams, G.B. Flood discharges and the dimensions of spillways in India. Engineering 1922, 134–321. [Google Scholar]
- Johnstone, D.; Cross, W.P. Elements of Applied Hydrology; Ronald Press: New York, NY, USA, 1949. [Google Scholar]
- Sheridan, J.M. Hydrograph Time Parameters for Flatland Watersheds. Trans. ASAE 1994, 37, 103–113. [Google Scholar] [CrossRef]
- Alcaldía Mayor de Cartagena de Indias (AMCI). Estudios y Diseños del Plan. Maestro de Drenajes Pluviales del Distrito de Cartagena; Consorcio Consultores Cartageneros: Cartagena de Indias, Colombia, 2009. [Google Scholar]
- Roussel, M.C.; Thompson, D.B.; Fang, X.; Cleveland, T.G.; Garcia, C.A. Time-Parameter Estimation for Applicable Texas Watersheds; Texas Department of Transportation: Beaumont, TX, USA, 2005.
- Te Chow, T.; Maidment, D.R.; Mays, L.W. Applied Hydrology, 1st ed.; McGraw-Hill: New York, NY, USA, 1988; ISBN 0-07-010810-2. [Google Scholar]
- ENVI, (version 5.1); Harris Geospatial Solutions Inc.: Boulder, CO, USA, 2019.
- Gupta, H.V.; Sorooshian, S.; Yapo, P.O. Status of Automatic Calibration for Hydrologic Models: Comparison with Multilevel Expert Calibration. J. Hydrol. Eng. 1999, 4, 135–143. [Google Scholar] [CrossRef]
- Legates David, R.; McCabe, G.J., Jr. Evaluating the use of goodness-of-fit measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar] [CrossRef]
- González-Álvarez, Á.; Viloria-Marimón, O.M.; Coronado-Hernández, Ó.E.; Vélez-Pereira, A.M.; Tesfagiorgis, K.; Coronado-Hernández, J.R. Isohyetal maps of daily maximum rainfall for different return periods for the Colombian Caribbean Region. Water 2019, 11, 358. [Google Scholar] [CrossRef]
- Duan, W.; Takara, K.; He, B.; Luo, P.; Nover, D.; Yamashiki, Y. Spatial and temporal trends in estimates of nutrient and suspended sediment loads in the Ishikari River, Japan, 1985 to 2010. Sci. Total Environ. 2013, 461–462, 499–508. [Google Scholar] [CrossRef]
- Duan, W.; He, B.; Nover, D.; Fan, J.; Yang, G.; Chen, W.; Meng, H.; Liu, C. Floods and associated socioeconomic damages in China over the last century. Nat. Hazards 2016, 82, 401–413. [Google Scholar] [CrossRef]
- Martins, L.; Diéz-Herrero, A.; Bodoque, J.; Bateira, C. Time of Concentration equations: The role of morphometric uncertainties in flood risk analysis and management. In Proceedings of the EGUGA, Vienna, Austria, 17–22 April 2016. EPSC2016-15660. [Google Scholar]
- Hoogestraat, G.K. Flood hydrology and dam-breach hydraulic analyses of four reservoirs in the Black Hills, South. Dakota: U.S. In Geological Survey Scientific Investigations Report 2011–5011; U.S. Geological Survey (USGS): Reston, VA, USA, 2011. [Google Scholar]
- Poleto, C.; Rutinéia, T. Sustainable Urban Drainage Systems. In Drainage Systems; IntechOpen: London, UK, 2012; ISBN 978-953-51-0243-4. [Google Scholar]
- Jiang, X.; Yang, L.; Tatano, H. Assessing Spatial Flood Risk from Multiple Flood Sources in a Small River Basin: A Method Based on Multivariate Design Rainfall. Water 2019, 11, 1031. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). Guidance for Flood Risk Analysis and Mapping. In Hydrology: Rainfall-Runoff Analysis; U.S. Department of Homeland Security: Washington, DC, USA, 2019. [Google Scholar]
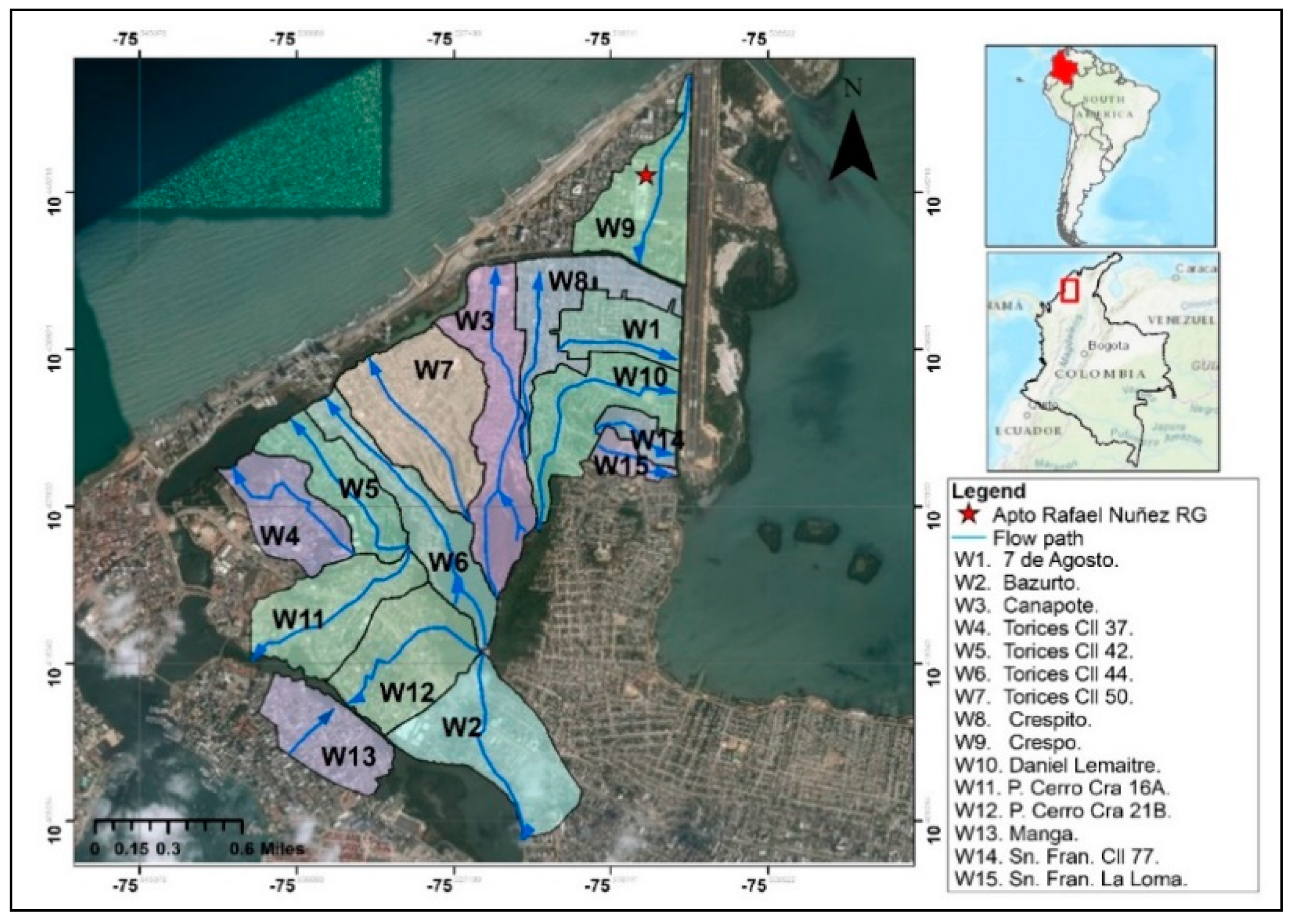


| No. | Watershed Name | Area (ha) | Receiving Water Body |
|---|---|---|---|
| W1 | 7 de Agosto | 29.20 | Juan Angola Bayou (in the vicinity of Canal Paralelo) |
| W2 | Bazurto | 66.05 | Las Quintas Swamp |
| W3 | Canapote | 67.87 | Juan Angola Bayou (in the vicinity of Crespo) |
| W4 | Torices, Calle 37 | 39.52 | Juan Angola Bayou (in the vicinity of El Cabrero Lagoon) |
| W5 | Torices, Calle 42 | 38.12 | Juan Angola Bayou (in the vicinity of El Cabrero Lagoon) |
| W6 | Torices, Calle 44 | 47.76 | Juan Angola Bayou (in the vicinity of El Cabrero Lagoon) |
| W7 | Torices, Calle 50 | 72.80 | Juan Angola Bayou (in the vicinity of Marbella) |
| W8 | Crespito | 44.00 | Juan Angola Bayou (in the vicinity of Crespo) |
| W9 | Crespo | 50.80 | Juan Angola Bayou (in the vicinity of Crespo) |
| W10 | Daniel Lemaitre | 52.56 | Juan Angola Bayou (in the vicinity of Canal Paralelo) |
| W11 | Pie del Cerro, Cra. 16A | 52.85 | Bazurto Bayou |
| W12 | Pie del Cerro, Cra. 21B | 56.58 | Bazurto Bayou |
| W13 | Manga | 38.47 | Bazurto Bayou |
| W14 | San Francisco, Calle 77 (La Amistad) | 11.18 | Juan Angola Bayou (in the vicinity of Canal Paralelo) |
| W15 | San Francisco, sector La Loma | 9.29 | Juan Angola Bayou (in the vicinity of Canal Paralelo) |
| Watershed | Flow Path | Flow Type | Total Length (m) | Watershed Avg. Slope (%) | Shallow Conc. Flow as a % of the Total Flow | ||
|---|---|---|---|---|---|---|---|
| Sheet | Shallow Concentrated | Channel | |||||
| 7 de Agosto | 1 | (100 m; 5.2%) | (748 m; 0.04%) | –– | 848 | 0.2 | 92% |
| Bazurto | 7 | (100 m; 39.4%) | (1264 m; 15.1%) | (170 m; 1.4%) | 1534 | 18.8 | 82% |
| Canapote | 2 | (100 m; 3.3%) | (2361 m; 6.5%) | (10 m; 11.0%) | 2471 | 6.3 | 96% |
| Torices, Calle 37 | 1 | (60 m; 3.4%) | (782 m; 6.0%) | (398 m; 0.6%) | 1240 | 4.3 | 63% |
| Torices, Calle 42 | 2 | (100 m; 12.3%) | (1289 m; 6.9%) | –– | 1389 | 8.4 | 93% |
| Torices, Calle 44 | 1 | (100 m; 10.5%) | (1836 m; 10.0%) | –– | 1935 | 9.9 | 95% |
| Torices, Calle 50 | 2 | (100 m; 3.9%) | (1213 m; 7.3%) | (14 m; 10.1%) | 1327 | 6.4 | 91% |
| Crespito | 2 | –– | (664 m; 2.4%) | –– | 664 | 2.4 | 100% |
| Crespo | 5 | –– | (498 m; 0.01%) | –– | 498 | 0.01 | 100% |
| Daniel Lemaitre | 2 | (100 m; 4.4%) | (1981 m; 6.1%) | –– | 2081 | 4.8 | 95% |
| Pie del Cerro, Cra 16A | 3 | (100 m; 8.1%) | (713 m; 12.7%) | (420 m; 0.1%) | 1233 | 9.2 | 58% |
| Pie del Cerro, Cra 21B | 4 | (100 m; 14.6%) | (1291 m; 14.8%) | (27 m; 0.7%) | 1418 | 15.2 | 91% |
| Manga | 1 | –– | (978 m; 0.05%) | –– | 978 | 0.05 | 100% |
| San Francisco, Calle 77 (La Amistad) | 2 | (100 m; 7.2%) | (647 m; 7.3%) | –– | 747 | 8.3 | 87% |
| San Francisco, sector La Loma | 2 | (100 m; 9.1%) | (579 m; 9.5%) | –– | 679 | 9.4 | 85% |
| Performance Category | Color Bar | Statistical Variables Range of Values | |||
|---|---|---|---|---|---|
| R2 | RSR | PBIAS (%) | NSE | ||
| Very good | 0.85–1.00 | 0.0 ≤ RSR ≤ 0.5 | PBIAS < ±10 | 0.75–1.00 | |
| Good | 0.70–0.84 | 0.5 < RSR ≤ 0.6 | ±10 ≤ PBIAS < ±15 | 0.65–0.74 | |
| Satisfactory | 0.60–0.69 | 0.6 < RSR ≤ 0.7 | ±15 ≤ PBIAS < ±25 | 0.50–0.64 | |
| Acceptable | 0.40–0.59 | Not applicable | Not applicable | 0.40–0.49 | |
| Unsatisfactory | ≤ 0.39 | RSR > 0.7 | PBIAS ≥ ±25 | ≤ 0.39 | |
| Tc Equation | No. of Variables | Flow Type | Variable Description |
|---|---|---|---|
| Miller | 3 | Sheet | Roughness coefficient, flow path length and slope |
| Federal Aviation Administration (FAA) | 3 | Sheet | Runoff coefficient, flow path length and slope |
| Espey–Winslow | 4 | Shallow Concentrated | Flow path length and slope, channelization factor, and impervious area percentage |
| Kerby | 3 | Shallow Concentrated | Roughness coefficient, flow path length and slope |
| Natural Resources Conservation Service (NRCS) Lag Equation | 3 | Shallow Concentrated | Curve number, flow path length and slope |
| Kirpich | 2 | Channel | Flow path length and slope |
| Bransby–Williams | 3 | Channel | Flow path length and slope, and watershed area |
| Johnstone–Cross | 2 | Channel | Flow path length and slope |
| Sheridan | 1 | Channel | Flow path length |
| Plan Maestro de Drenajes Pluviales de Cartagena (PMDPC) | 2 | Channel | Flow path length and slope |
| Time of Concentration Equation | Adjustment Factor | |
|---|---|---|
| Stationary Conditions | Non-Stationary Conditions | |
| FAA | 2.17 | 2.13 |
| NRCS Lag Equation | 2.44 | 2.38 |
| Kirpich | 2.08 | 2.00 |
| Kirpich-Adjusted | –– | 4.17 |
| Johnstone-Cross | 0.72 | 0.69 |
| PMDPC | 0.81 | 0.78 |
| MinVivienda-FAA | 2.27 | 2.22 |
| MinVivienda-NRCS | 2.38 | 2.33 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
González-Álvarez, Á.; Molina-Pérez, J.; Meza-Zúñiga, B.; Viloria-Marimón, O.M.; Tesfagiorgis, K.; Mouthón-Bello, J.A. Assessing the Performance of Different Time of Concentration Equations in Urban Ungauged Watersheds: Case Study of Cartagena de Indias, Colombia. Hydrology 2020, 7, 47. https://doi.org/10.3390/hydrology7030047
González-Álvarez Á, Molina-Pérez J, Meza-Zúñiga B, Viloria-Marimón OM, Tesfagiorgis K, Mouthón-Bello JA. Assessing the Performance of Different Time of Concentration Equations in Urban Ungauged Watersheds: Case Study of Cartagena de Indias, Colombia. Hydrology. 2020; 7(3):47. https://doi.org/10.3390/hydrology7030047
Chicago/Turabian StyleGonzález-Álvarez, Álvaro, José Molina-Pérez, Brandon Meza-Zúñiga, Orlando M. Viloria-Marimón, Kibrewossen Tesfagiorgis, and Javier A. Mouthón-Bello. 2020. "Assessing the Performance of Different Time of Concentration Equations in Urban Ungauged Watersheds: Case Study of Cartagena de Indias, Colombia" Hydrology 7, no. 3: 47. https://doi.org/10.3390/hydrology7030047
APA StyleGonzález-Álvarez, Á., Molina-Pérez, J., Meza-Zúñiga, B., Viloria-Marimón, O. M., Tesfagiorgis, K., & Mouthón-Bello, J. A. (2020). Assessing the Performance of Different Time of Concentration Equations in Urban Ungauged Watersheds: Case Study of Cartagena de Indias, Colombia. Hydrology, 7(3), 47. https://doi.org/10.3390/hydrology7030047





