Analysis of the Distance between the Measured and Assumed Location of a Point Source of Pollution in Groundwater as a Function of the Variance of the Estimation Error
Abstract
:1. Introduction
2. Materials and Methods
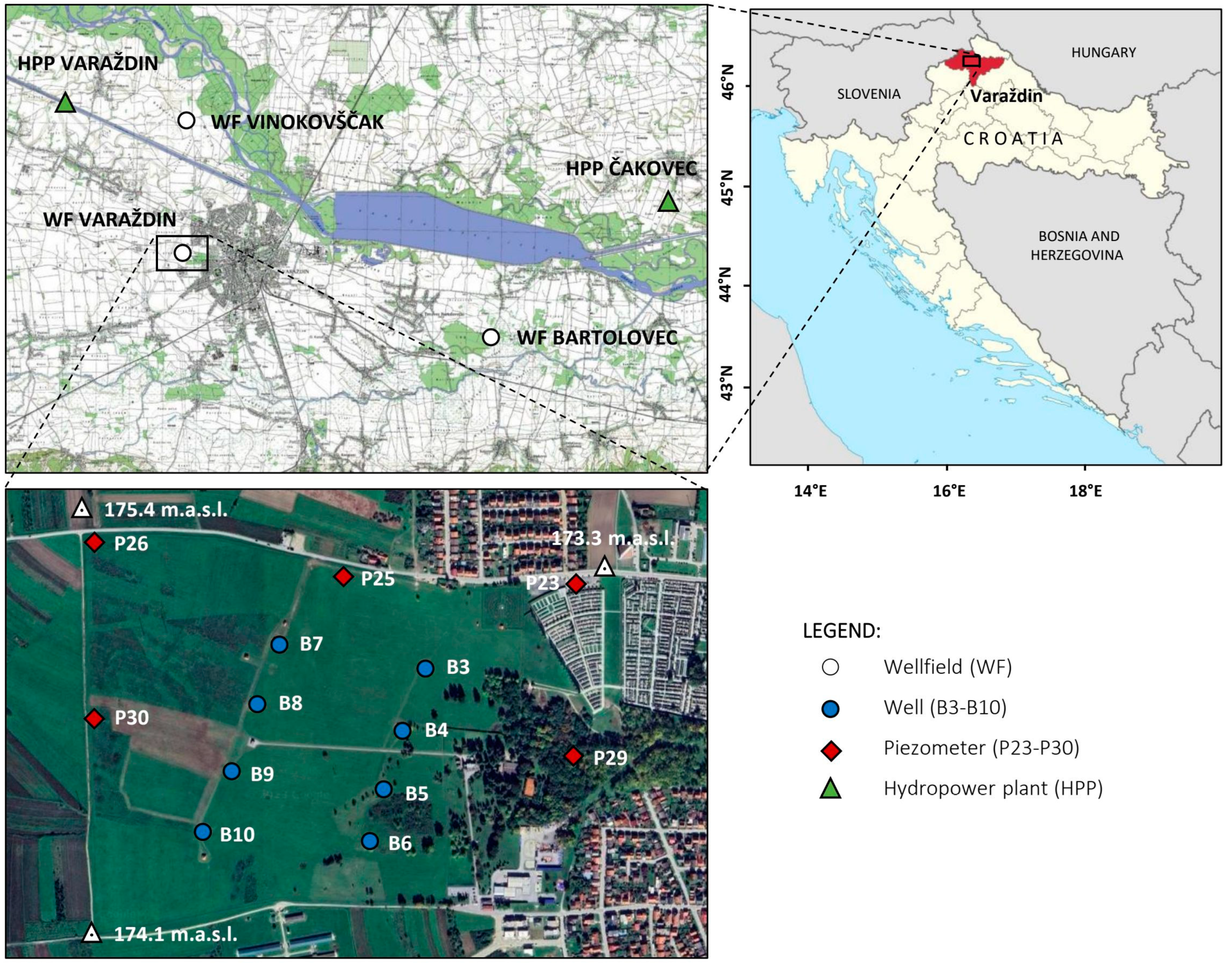
2.1. The Study Area
2.2. Previous Research
2.3. Aim of the Paper
- -
- Based on the value of the variance of the estimation error, choose the interpolation method that gives the best estimate.
- -
- Based on the selected interpolation method, create a model that most closely approximates the actual spatial distribution of groundwater nitrate concentrations.
- -
- Use the created model to assume the location of the pollution source.
- -
- Determine the dependence of the distance between the measured and the assumed location on the variance of the estimation error and prove the hypothesis H0.
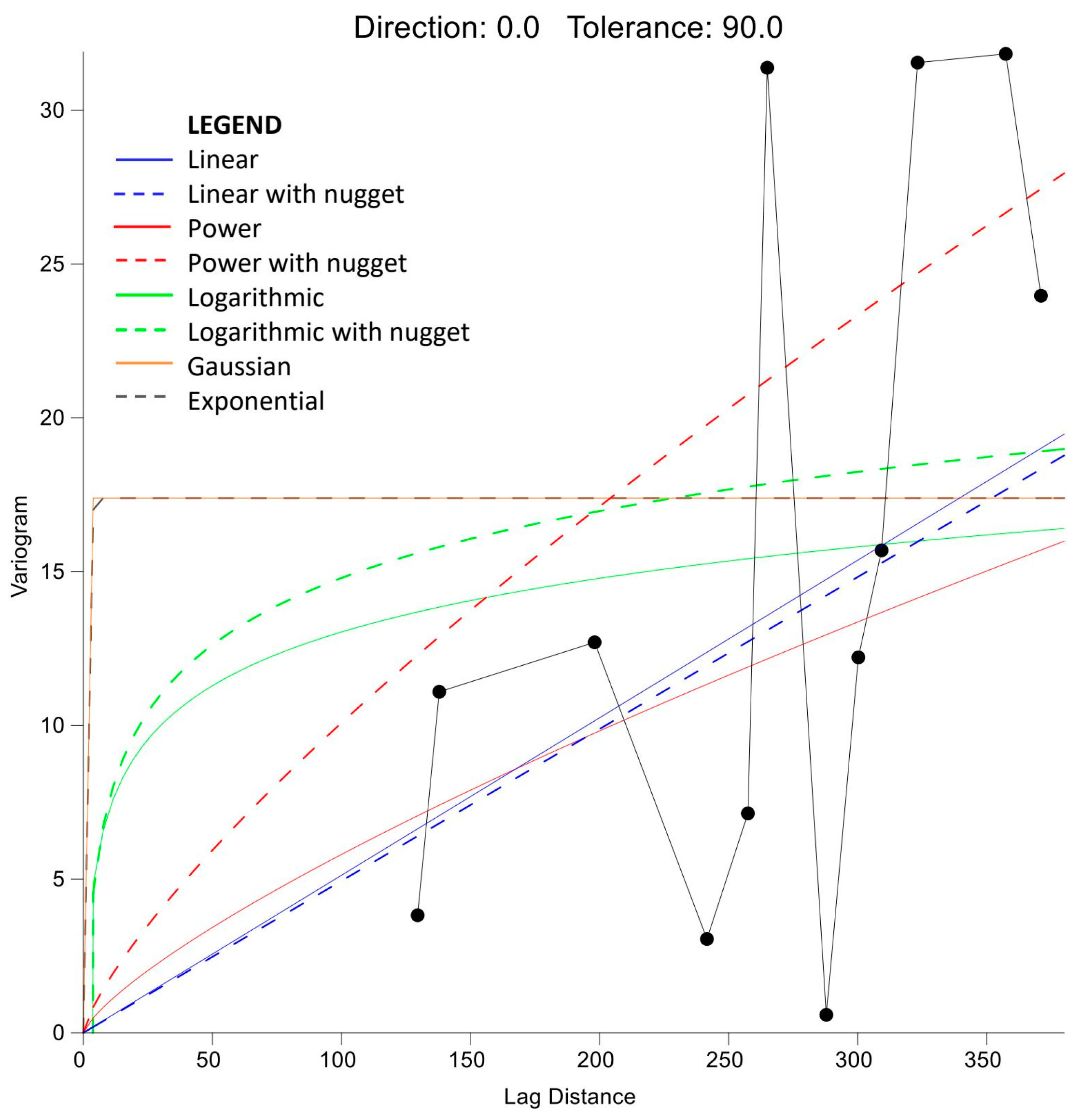
2.4. Nitrate Field Analysis
- —measured i-th value
- —estimated i-th value
- N—total number of measured values
- r—correlation coefficient for the linearized model
- df = N − 2, number of degrees of freedom
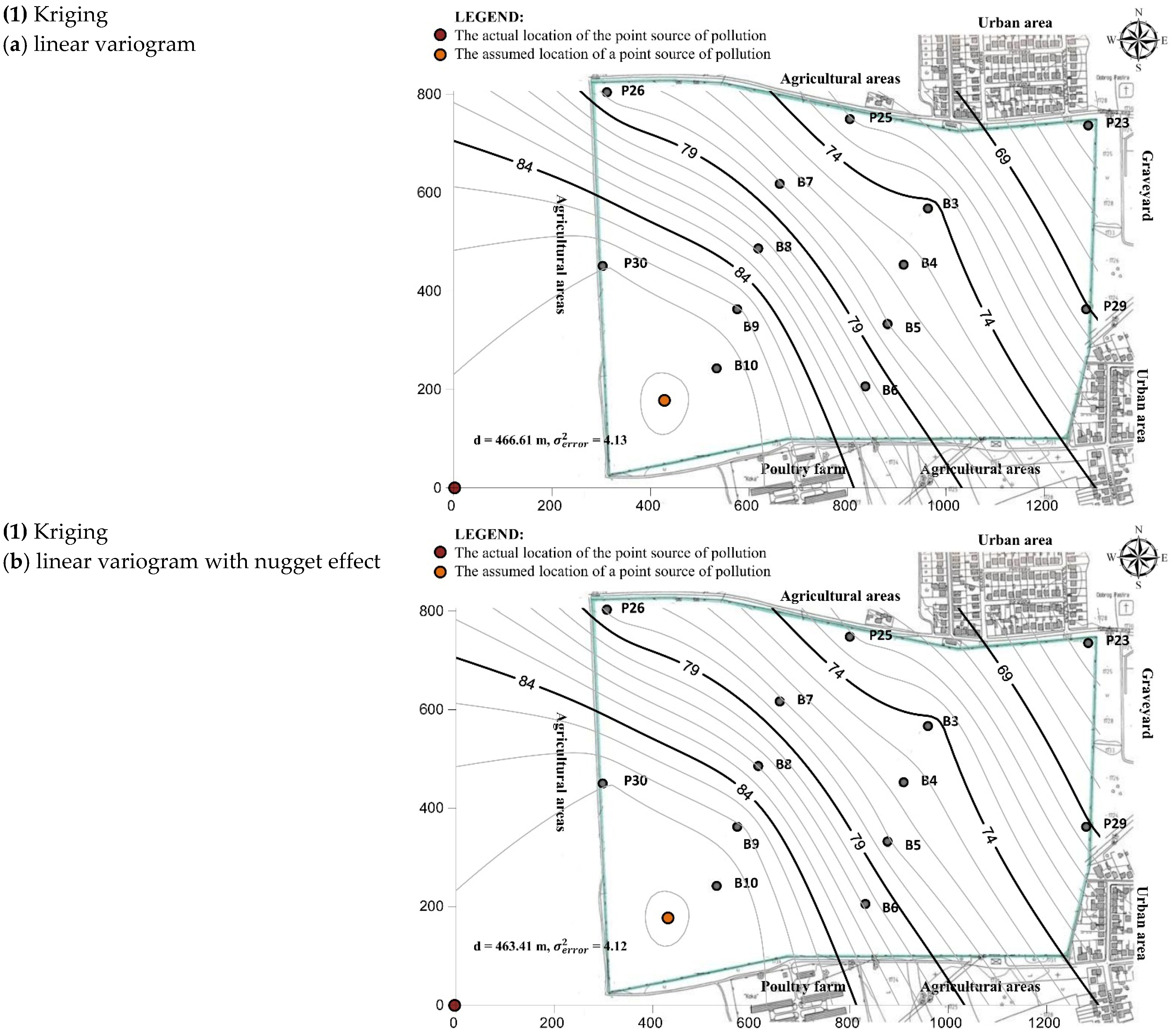
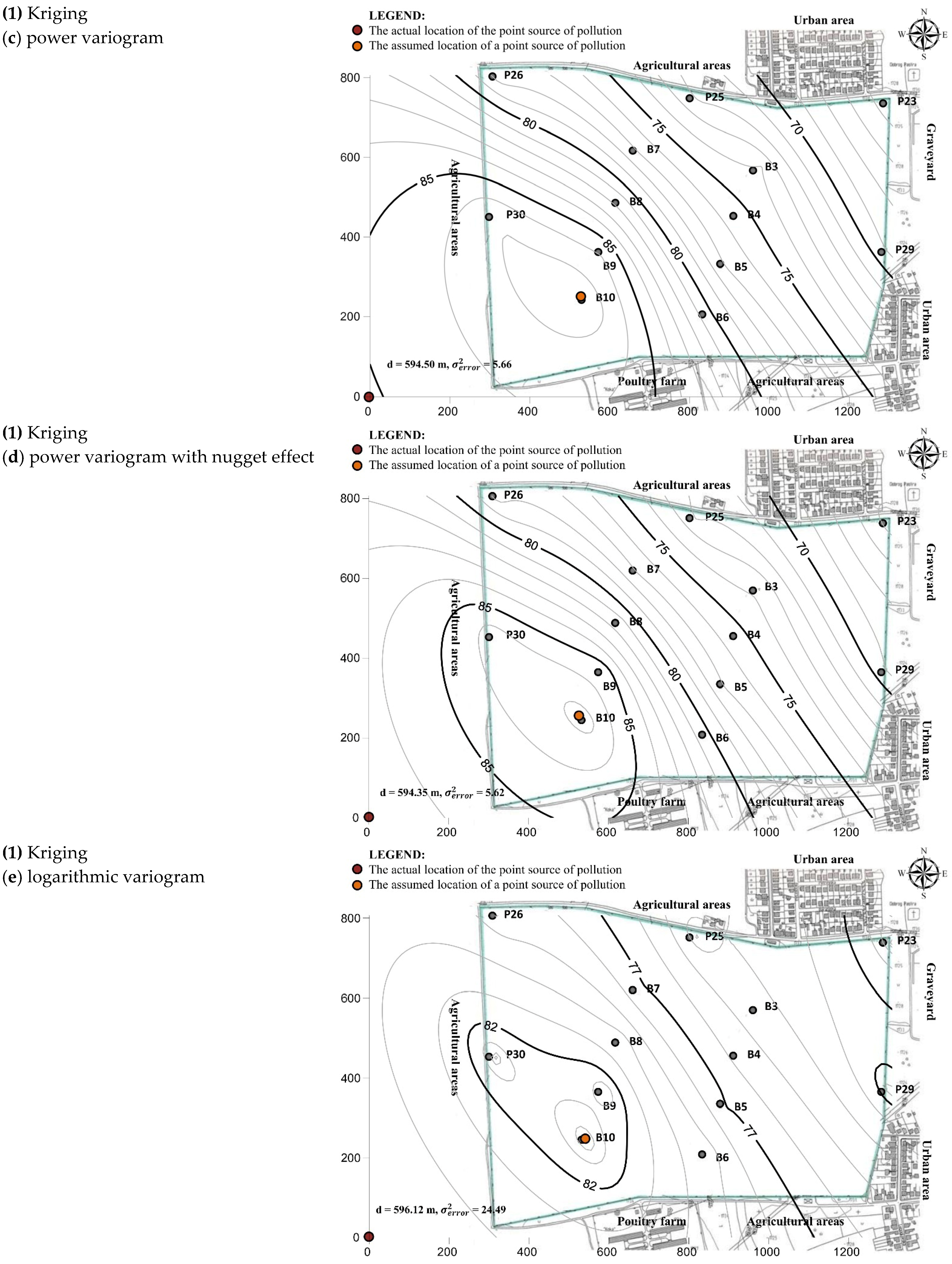
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Quevauviller, P.; Fouillac, A.M.; Grath, J.; Ward, R. Groundwater Monitoring; Wiley: Hoboken, NJ, USA, 2009. [Google Scholar] [CrossRef]
- EEA. Freshwater Quality—The European Environment—State and Outlook 2010; European Environment Agency: Copenhagen, Denmark, 2010. [Google Scholar]
- European Commission. Groundwater Protection in Europe: The New Groundwater Directive: Consolidating the EU Regulatory Framework; European Commission: Brussels, Belgium, 2009. [Google Scholar] [CrossRef]
- Alcamo, J. Water quality and its interlinkages with the Sustainable Development Goals. Curr. Opin. Environ. Sustain. 2019, 36, 126–140. [Google Scholar] [CrossRef]
- Neves, S.A.; Marques, A.C.; Patrício, M. Determinants of CO2 emissions in European Union countries: Does environmental regulation reduce environmental pollution? Econ. Anal. Policy 2020, 68, 114–125. [Google Scholar] [CrossRef]
- Wen, J.; Mughal, N.; Zhao, J.; Shabbir, M.S.; Niedbała, G.; Jain, V.; Anwar, A. Does globalization matter for environmental degradation? Nexus among energy consumption, economic growth, and carbon dioxide emission. Energy Policy 2021, 153, 112230. [Google Scholar] [CrossRef]
- Dyvak, M.; Rot, A.; Pasichnyk, R.; Tymchyshyn, V.; Huliiev, N.; Maslyiak, Y. Monitoring and Mathematical Modeling of Soil and Groundwater Contamination by Harmful Emissions of Nitrogen Dioxide from Motor Vehicles. Sustainability 2021, 13, 2768. [Google Scholar] [CrossRef]
- Kurwadkar, S.; Kanel, S.R.; Nakarmi, A. Groundwater pollution: Occurrence, detection, and remediation of organic and inorganic pollutants. Water Environ. Res. 2020, 92, 1659–1668. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, D.; Xu, H.; Ding, Z.; Shi, Y.; Lu, Z.; Cheng, Z. Groundwater pollution risk assessment based on groundwater vulnerability and pollution load on an isolated island. Chemosphere 2022, 289, 133134. [Google Scholar] [CrossRef]
- Plessis, A.D. Water Resources from a Global Perspective. In South Africa’s Water Predicament; Springer: Cham, Switzerland, 2023; pp. 1–25. [Google Scholar] [CrossRef]
- Kovač, I.; Šrajbek, M.; Kranjčević, L.; Novotni-Horčička, N. Nonlinear models of the dependence of nitrate concentrations on the pumping rate of a water supply system. Geosci. J. 2020, 24, 585–595. [Google Scholar] [CrossRef]
- Das, B.; Pal, S.C. Assessment of groundwater vulnerability to over-exploitation using MCDA, AHP, fuzzy logic and novel ensemble models: A case study of Goghat-I and II blocks of West Bengal, India. Environ. Earth Sci. 2020, 79, 104. [Google Scholar] [CrossRef]
- Umar, M.; Khan, S.N.; Arshad, A.; Aslam, R.A.; Khan, H.M.S.; Rashid, H.; Pham, Q.B.; Nasir, A.; Noor, R.; Khedher, K.M.; et al. A modified approach to quantify aquifer vulnerability to pollution towards sustainable groundwater management in Irrigated Indus Basin. Environ. Sci. Pollut. Res. 2022, 29, 27257–27278. [Google Scholar] [CrossRef]
- Addiscott, T.M.; Whitmore, A.P.; Powlson, D.S. Farming, Fertilizers and the Nitrate Problem; Oxford University Press: Oxford, UK, 1991. [Google Scholar]
- Foster, S.; Hirata, R.; Andreo, B. The aquifer pollution vulnerability concept: Aid or impediment in promoting groundwater protection? Hydrogeol. J. 2013, 21, 1389–1392. [Google Scholar] [CrossRef]
- Almasri, M.N.; Kaluarachchi, J.J. Modular neural networks to predict the nitrate distribution in ground water using the on-ground nitrogen loading and recharge data. Environ. Model. Softw. 2005, 20, 851–871. [Google Scholar] [CrossRef]
- Farhadi, H.; Fataei, E.; Sadeghi, M.K. The Relationship Between Nitrate Distribution in Groundwater and Agricultural Land use (Case study: Ardabil Plain, Iran). Anthropog. Pollut. J. 2020, 4, 50–56. [Google Scholar]
- Srivastav, A.L. Chemical fertilizers and pesticides: Role in groundwater contamination. In Agrochemicals Detection, Treatment and Remediation; Elsevier: Amsterdam, The Netherlands, 2020; pp. 143–159. [Google Scholar] [CrossRef]
- Yu, L.; Zheng, T.; Yuan, R.; Zheng, X. APCS-MLR model: A convenient and fast method for quantitative identification of nitrate pollution sources in groundwater. J. Environ. Manag. 2022, 314, 115101. [Google Scholar] [CrossRef]
- Müller, L.; Behrendt, A.; Schindler, U. Strukturaspekte der bodendecke und bodeneigenschaften zweier niederungsstandorte in Nordostdeutschland: Structure aspects of the soil landscape and soil properties of two lowland sites in North-East Germany. Arch. Agron. Soil Sci. 2004, 50, 289–307. [Google Scholar] [CrossRef]
- Krause, S.; Bronstert, A.; Zehe, E. Groundwater–surface water interactions in a North German lowland floodplain—Implications for the river discharge dynamics and riparian water balance. J. Hydrol. 2007, 347, 404–417. [Google Scholar] [CrossRef]
- Schmalz, B.; Tavares, F.; Fohrer, N. Assessment of nutrient entry pathways and dominating hydrological processes in lowland catchments. Adv. Geosci. 2007, 11, 107–112. [Google Scholar] [CrossRef]
- Krause, S.; Bronstert, A. An advanced approach for catchment delineation and water balance modelling within wetlands and floodplains. Adv. Geosci. 2005, 5, 1–5. [Google Scholar] [CrossRef]
- Davey, I.R.; Besien, T.J.; Evers, S.; Ward, R. Nitrates in Groundwater—Agricultural Nitrate Contamination in Groundwater in England and Wales: An Overview; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- He, X.-S.; Zhang, Y.-L.; Liu, Z.-H.; Wei, D.; Liang, G.; Liu, H.-T.; Xi, B.-D.; Huang, Z.-B.; Ma, Y.; Xing, B.-S. Interaction and coexistence characteristics of dissolved organic matter with toxic metals and pesticides in shallow groundwater. Environ. Pollut. 2020, 258, 113736. [Google Scholar] [CrossRef]
- Razowska-Jaworek, L.; Sadurski, A. Nitrates in Groundwater—Development of a Groundwater Abstraction Modelling Environment for Drinking Water Supply; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Sapek, B.; Sapek, A. Nitrates in Groundwater—Nitrate in Groundwater as an Indicator of Farmstead Impacts on the Environment; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Stockmarr, J.; Nyegaard, P. Nitrates in Groundwater—Nitrate in Danish Groundwater; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Li, P.; He, X.; Guo, W. Spatial groundwater quality and potential health risks due to nitrate ingestion through drinking water: A case study in Yan’an City on the Loess Plateau of northwest China. Hum. Ecol. Risk Assess. Int. J. 2019, 25, 11–31. [Google Scholar] [CrossRef]
- Yu, G.; Wang, J.; Liu, L.; Li, Y.; Zhang, Y.; Wang, S. The analysis of groundwater nitrate pollution and health risk assessment in rural areas of Yantai, China. BMC Public Health 2020, 20, 437. [Google Scholar] [CrossRef]
- Chambers, T.; Douwes, J.; Mannetje, A.; Woodward, A.; Baker, M.; Wilson, N.; Hales, S. Nitrate in drinking water and cancer risk: The biological mechanism, epidemiological evidence and future research. Aust. N. Z. J. Public Health 2022, 46, 105–108. [Google Scholar] [CrossRef] [PubMed]
- Brender, J.D. Human Health Effects of Exposure to Nitrate, Nitrite, and Nitrogen Dioxide. In Just Enough Nitrogen; Springer International Publishing: Cham, Switzerland, 2020; pp. 283–294. [Google Scholar] [CrossRef]
- Madison, R.J.; Brunett, J.O. Overview of the occurrence of nitrate in ground water in the United States. US Geol. Surv. Water-Supply Pap. 1984, 2275, 93–105. [Google Scholar]
- Rahman, A.; Mondal, N.C.; Tiwari, K.K. Anthropogenic nitrate in groundwater and its health risks in the view of background concentration in a semi arid area of Rajasthan, India. Sci. Rep. 2021, 11, 9279. [Google Scholar] [CrossRef] [PubMed]
- Manu, E.; Afrifa, G.Y.; Ansah-Narh, T.; Sam, F.; Loh, Y.S.A. Estimation of natural background and source identification of nitrate-nitrogen in groundwater in parts of the Bono, Ahafo and Bono East regions of Ghana. Groundw. Sustain. Dev. 2022, 16, 100696. [Google Scholar] [CrossRef]
- Nakić, Z.; Kovač, Z.; Parlov, J.; Perković, D. Ambient Background Values of Selected Chemical Substances in Four Groundwater Bodies in the Pannonian Region of Croatia. Water 2020, 12, 2671. [Google Scholar] [CrossRef]
- Eberts, M.; Thomas, M.A.; Jagucki, M.L. The Quality of Our Nation’s Waters: Factors Affecting Public-Supply-Well Vulnerability to Contamination: Understanding Observed Water Quality and Anticipating Future Water Quality; U.S. Department of the Interior, U.S. Geological Survey: Reston, VA, USA, 2013.
- Jurec, J.N.; Mesic, M.; Basic, F.; Kisic, I.; Zgorelec, Z. Nitrate concentration in drinking water from wells at three different locations in Northwest Croatia. Cereal Res. Commun. 2007, 35, 533–536. [Google Scholar] [CrossRef]
- Srajbek, M.; Kovac, I.; Novotni-Horcicka, N.; Kranjcevic, L. Assessment of average contributions of point and diffuse pollution sources to nitrate concentration in groundwater by nonlinear regression. Environ. Eng. Manag. J. 2020, 19, 95–104. [Google Scholar] [CrossRef]
- Kim, H.-R.; Yu, S.; Oh, J.; Kim, K.-H.; Lee, J.-H.; Moniruzzaman, M.; Kim, H.K.; Yun, S.-T. Nitrate contamination and subsequent hydrogeochemical processes of shallow groundwater in agro-livestock farming districts in South Korea. Agric. Ecosyst. Environ. 2019, 273, 50–61. [Google Scholar] [CrossRef]
- Šrajbek, M.; Kranjčević, L.; Kovač, I.; Biondić, R. Groundwater Nitrate Pollution Sources Assessment for Contaminated Wellfield. Water 2022, 14, 255. [Google Scholar] [CrossRef]
- Bronowicka-Mielniczuk, U.; Mielniczuk, J.; Obroślak, R.; Przystupa, W. A Comparison of Some Interpolation Techniques for Determining Spatial Distribution of Nitrogen Compounds in Groundwater. Int. J. Environ. Res. 2019, 13, 679–687. [Google Scholar] [CrossRef]
- Mustafa, J.S.; Mawlood, D.K. Mapping Groundwater Levels in Erbil Basin. Am. Sci. Res. J. Eng. Technol. Sci. 2023, 93, 21–38. [Google Scholar]
- Fan, X.; Min, T.; Dai, X. The Spatio-Temporal Dynamic Patterns of Shallow Groundwater Level and Salinity: The Yellow River Delta, China. Water 2023, 15, 1426. [Google Scholar] [CrossRef]
- Hajnrych, M.; Blachowski, J.; Worsa-Kozak, M. Study of groundwater temperature spatio-temporal variation in the city of Wroclaw. Preliminary results. IOP Conf. Ser. Earth Environ. Sci. 2023, 1189, 012028. [Google Scholar] [CrossRef]
- Lubis, R.F.; Yamano, M.; Delinom, R.; Martosuparno, S.; Sakura, Y.; Goto, S.; Miyakoshi, A.; Taniguchi, M. Assessment of urban groundwater heat contaminant in Jakarta, Indonesia. Environ. Earth Sci. 2013, 70, 2033–2038. [Google Scholar] [CrossRef]
- Taniguchi, M.; Shimada, J.; Fukuda, Y.; Yamano, M.; Onodera, S.-I.; Kaneko, S.; Yoshikoshi, A. Anthropogenic effects on the subsurface thermal and groundwater environments in Osaka, Japan and Bangkok, Thailand. Sci. Total Environ. 2009, 407, 3153–3164. [Google Scholar] [CrossRef] [PubMed]
- Previati, A.; Crosta, G.B. Characterization of the subsurface urban heat island and its sources in the Milan city area, Italy. Hydrogeol. J. 2021, 29, 2487–2500. [Google Scholar] [CrossRef]
- Benz, S.A.; Bayer, P.; Goettsche, F.M.; Olesen, F.S.; Blum, P. Linking Surface Urban Heat Islands with Groundwater Temperatures. Environ. Sci. Technol. 2016, 50, 70–78. [Google Scholar] [CrossRef]
- Nayak, A.; Matta, G.; Uniyal, D.P.; Kumar, A.; Kumar, P.; Pant, G. Assessment of potentially toxic elements in groundwater through interpolation, pollution indices, and chemometric techniques in Dehradun in Uttarakhand State. Environ. Sci. Pollut. Res. 2023. [Google Scholar] [CrossRef]
- Khan, M.; Almazah, M.M.A.; EIlahi, A.; Niaz, R.; Al-Rezami, A.Y.; Zaman, B. Spatial interpolation of water quality index based on Ordinary kriging and Universal kriging. Geomat. Nat. Hazards Risk 2023, 14, 2190853. [Google Scholar] [CrossRef]
- Kovač, I.; Kovačev-Marinčić, B.; Novotni-Horčička, N.; Mesec, J.; Vugrinec, J. Komparativna analiza koncentracije nitrata u gornjem i donjem sloju varaždinskog vodonosnika. Rad. Zavoda Znan. Varaždin 2017, 28, 41–57. [Google Scholar] [CrossRef]
- Novotni-Horčička, N.; Šrajbek, M.; Kovač, I. Nitrati u Regionalnom vodovodu Varaždin. In Voda i Javna Vodoopskrba; Hrvatski Zavod za Javno Zdravstvo (HZJZ): Zagreb, Croatia, 2010; pp. 123–131. [Google Scholar]
- Urumović, K. O kvartnom vodonosnom kopleksu u području Varaždina. Geološki Vjesn. 1971, 43, 109–118. [Google Scholar]
- Larva, O. Aquifer Vulnerability at Catchment Area of Varaždin Pumping Sites. Ph.D Thesis, Faculty of Mining, Geology and Petroleum Engineering, University of Zagreb, Zagreb, Croatia, 2008. [Google Scholar]
- Šrajbek, M. Nitrate Pollution Propagation in Groundwater and Wellfield Imapct Assessment. Ph.D Thesis, Faculty of Engineering, University of Rijeka, Rijeka, Croatia, 2021. [Google Scholar]
- European Union. Council Directive 91/676/EEC of 12 December 1991 concerning the protection of waters against pollution caused by nitrate from agricultural sources. Off. J. Eur. Commun. 1991, 375, 1–13. [Google Scholar]
- Gjetvaj, G. Identifikacija porijekla nitrata u podzemnim vodama Varaždinske regije. Hrvat. Vode 1993, 1, 247–252. [Google Scholar]
- Grđan, D.; Durman, P.; Kovačev-Marinčić, B. Odnos promjene režima i kvalitete podzemnih voda na crpilištima Varaždin i Bartolovec. Geološki Vjesn. 1991, 44, 301–308. [Google Scholar]
- Kovač, I. Statistical-Variographic Analysis of Ground Water Chemical Composition in Varaždin Region. Ph.D. Thesis, Faculty of Minning, Geology and Petroleum Engineering, University of Zagreb, Zagreb, Croatia, 2004. [Google Scholar]
- Arslan, H. Spatial and temporal mapping of groundwater salinity using ordinary kriging and indicator kriging: The case of Bafra Plain, Turkey. Agric. Water Manag. 2012, 113, 57–63. [Google Scholar] [CrossRef]
- Thomas, E.O. Spatial evaluation of groundwater quality using factor analysis and geostatistical Kriging algorithm: A case study of Ibadan Metropolis, Nigeria. Water Pract. Technol. 2023, 18, 592–607. [Google Scholar] [CrossRef]
- Belkhiri, L.; Tiri, A.; Mouni, L. Spatial distribution of the groundwater quality using kriging and Co-kriging interpolations. Groundw. Sustain. Dev. 2020, 11, 100473. [Google Scholar] [CrossRef]
- Gundogdu, K.S.; Guney, I. Spatial analyses of groundwater levels using universal kriging. J. Earth Syst. Sci. 2007, 116, 49–55. [Google Scholar] [CrossRef]
- Rostami, A.A.; Karimi, V.; Khatibi, R.; Pradhan, B. An investigation into seasonal variations of groundwater nitrate by spatial modelling strategies at two levels by kriging and co-kriging models. J. Environ. Manag. 2020, 270, 110843. [Google Scholar] [CrossRef]
- Arkoc, O. Modeling of spatiotemporal variations of groundwater levels using different interpolation methods with the aid of GIS, case study from Ergene Basin, Turkey. Model. Earth Syst. Environ. 2022, 8, 967–976. [Google Scholar] [CrossRef]
- Varouchakis, A.; Hristopulos, D.T. Comparison of stochastic and deterministic methods for mapping groundwater level spatial variability in sparsely monitored basins. Environ. Monit. Assess. 2013, 18, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Xiao, Y.; Gu, X.; Yin, S.; Shao, J.; Cui, Y.; Zhang, Q.; Niu, Y. Geostatistical interpolation model selection based on ArcGIS and spatio-temporal variability analysis of groundwater level in piedmont plains, northwest China. Springerplus 2016, 5, 425. [Google Scholar] [CrossRef] [PubMed]
- Elumalai, V.; Brindha, K.; Sithole, B.; Lakshmanan, E. Spatial interpolation methods and geostatistics for mapping groundwater contamination in a coastal area. Environ. Sci. Pollut. Res. 2017, 24, 11601–11617. [Google Scholar] [CrossRef]
- Ahmad, A.Y.; Saleh, I.A.; Balakrishnan, P.; Al-Ghouti, M.A. Comparison GIS-Based interpolation methods for mapping groundwater quality in the state of Qatar. Groundw. Sustain. Dev. 2021, 13, 100573. [Google Scholar] [CrossRef]
- Mirzaei, R.; Sakizadeh, M. Comparison of interpolation methods for the estimation of groundwater contamination in Andimeshk-Shush Plain, Southwest of Iran. Environ. Sci. Pollut. Res. 2016, 23, 2758–2769. [Google Scholar] [CrossRef]



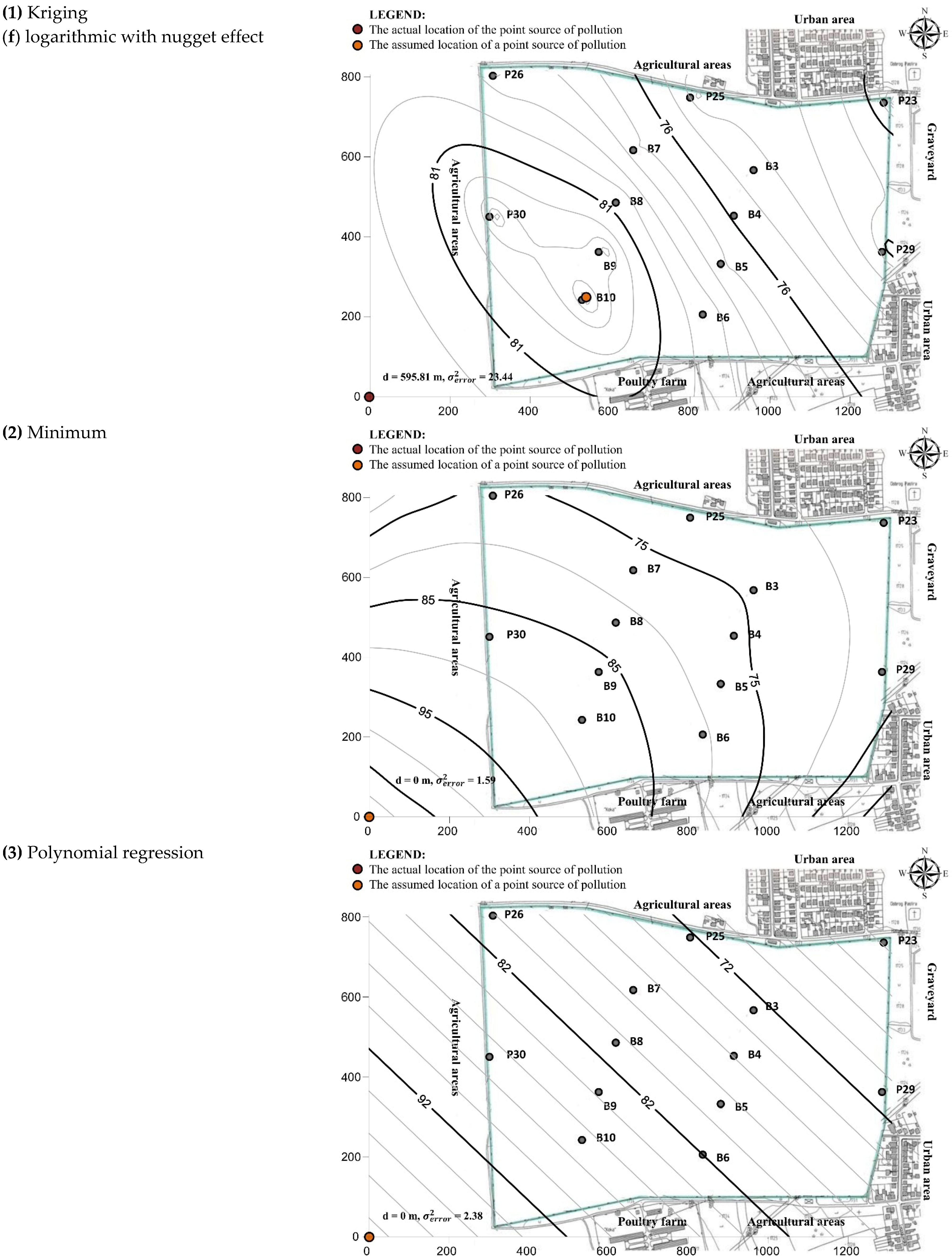

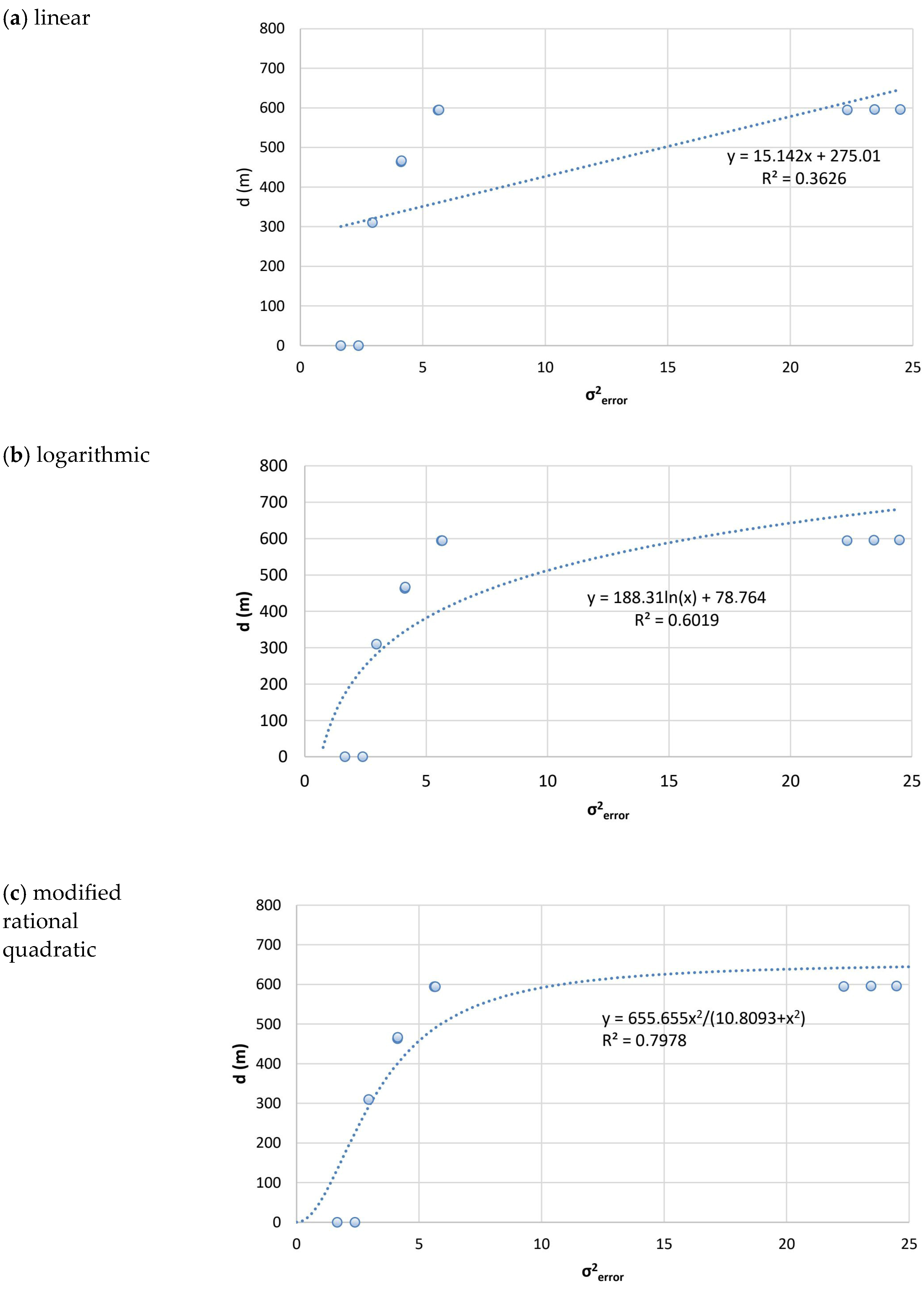

| B3 | B4 | B5 | B6 | B7 | B8 | B9 | B10 | P23 | P25 | P26 | P29 | P30 | ∑Δ2 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Average [mg/L NO3−] | 74.14 | 75.35 | 76.7 | 80.04 | 76.71 | 81.42 | 85.92 | 87.80 | 65.03 | 71.67 | 77.4 | 68.62 | 87.00 | ||
| Kriging–linear | 0.06 | 0.01 | −0.05 | −0.02 | −0.02 | 0.02 | 0.10 | 0.02 | −0.03 | −0.05 | −0.06 | −0.04 | 0.04 | 0.03 | 0.00 |
| Kriging–linear nugget | 0.06 | 0.01 | −0.05 | −0.02 | −0.02 | 0.03 | 0.10 | 0.02 | −0.03 | −0.05 | −0.06 | −0.04 | 0.03 | 0.03 | 0.00 |
| Kriging–power | 0.12 | 0.03 | −0.09 | −0.01 | −0.05 | 0.05 | 0.21 | 0.08 | −0.12 | −0.12 | −0.14 | −0.11 | 0.11 | 0.15 | 0.01 |
| Kriging–power nugget | 0.12 | 0.03 | −0.10 | −0.03 | −0.04 | 0.06 | 0.20 | 0.08 | −0.12 | −0.11 | −0.13 | −0.11 | 0.10 | 0.14 | 0.01 |
| Kriging–logarithmic | −0.07 | −0.18 | −0.28 | 0.61 | −0.40 | 0.69 | 2.16 | 1.43 | −2.70 | −1.29 | −0.56 | −1.99 | 1.90 | 24.68 | 1.90 |
| Kriging–logarithmic nugget | −0.02 | −0.14 | −0.25 | 0.52 | −0.34 | 0.56 | 1.86 | 0.98 | −2.20 | −1.06 | −0.46 | −1.68 | 1.51 | 16.53 | 1.27 |
| Minimum curvature | −0.01 | −0.10 | −0.10 | 0.06 | 0.03 | −0.04 | 0.01 | 0.00 | −0.25 | 0.03 | 0.08 | 0.24 | 0.01 | 0.15 | 0.01 |
| Polynomial regression | 1.72 | −0.24 | −1.84 | −1.56 | −0.33 | 1.14 | 2.52 | 1.25 | 1.67 | −0.21 | −2.52 | −1.87 | 0.27 | 30.92 | 2.38 |
| Radial basis function | 0.00 | 0.00 | −0.01 | 0.00 | 0.00 | 0.00 | 0.01 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
| Inverse distance to a power | −5.50 | −7.79 | −7.90 | −2.87 | −0.95 | −0.77 | 1.05 | 4.46 | −9.21 | −1.78 | 4.81 | −16.25 | 3.65 | 572.63 | 44.05 |
| Interpolation Method | ||
|---|---|---|
| Kriging–linear variogram | 4.13 | 466.61 |
| Kriging–linear variogram with nugget | 4.12 | 463.41 |
| Kriging–power variogram | 5.66 | 594.50 |
| Kriging–power variogram with nugget | 5.62 | 594.35 |
| Kriging–logarithmic variogram | 24.49 | 596.12 |
| Kriging-logarithmic variogram with nugget | 23.44 | 595.81 |
| Kriging–gaussian variogram | 51.87 | not applicable |
| Kriging–exponential variogram | 51.87 | not applicable |
| Minimum curvature | 1.65 | 0.00 |
| Polynomial regression | 2.38 | 0.00 |
| Radial basis function | 2.94 | 309.88 |
| Inverse distance to a power | 22.33 | 594.74 |
| Nearest neighbor | 22.26 | not applicable |
| Moving average | 23.01 | not applicable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kovač, I.; Šrajbek, M.; Klišanin, N.; Gilja, G. Analysis of the Distance between the Measured and Assumed Location of a Point Source of Pollution in Groundwater as a Function of the Variance of the Estimation Error. Hydrology 2023, 10, 199. https://doi.org/10.3390/hydrology10100199
Kovač I, Šrajbek M, Klišanin N, Gilja G. Analysis of the Distance between the Measured and Assumed Location of a Point Source of Pollution in Groundwater as a Function of the Variance of the Estimation Error. Hydrology. 2023; 10(10):199. https://doi.org/10.3390/hydrology10100199
Chicago/Turabian StyleKovač, Ivan, Marko Šrajbek, Nikolina Klišanin, and Gordon Gilja. 2023. "Analysis of the Distance between the Measured and Assumed Location of a Point Source of Pollution in Groundwater as a Function of the Variance of the Estimation Error" Hydrology 10, no. 10: 199. https://doi.org/10.3390/hydrology10100199
APA StyleKovač, I., Šrajbek, M., Klišanin, N., & Gilja, G. (2023). Analysis of the Distance between the Measured and Assumed Location of a Point Source of Pollution in Groundwater as a Function of the Variance of the Estimation Error. Hydrology, 10(10), 199. https://doi.org/10.3390/hydrology10100199










