Crystallite Size Effects on Electrical Properties of Nickel Chromite (NiCr2O4) Spinel Ceramics: A Study of Structural, Magnetic, and Dielectric Transitions
Abstract
1. Introduction
2. Materials and Methods
3. Results
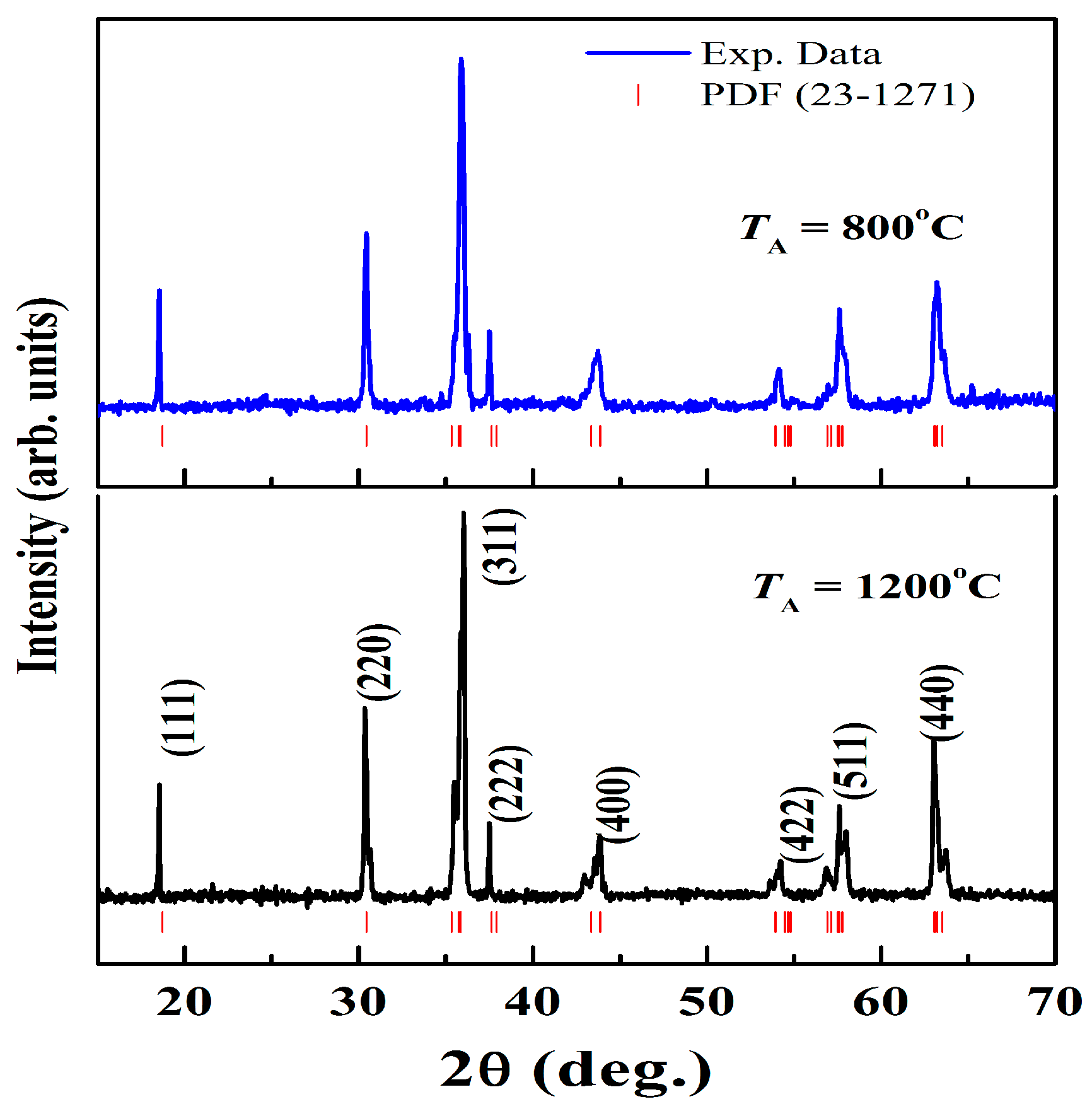
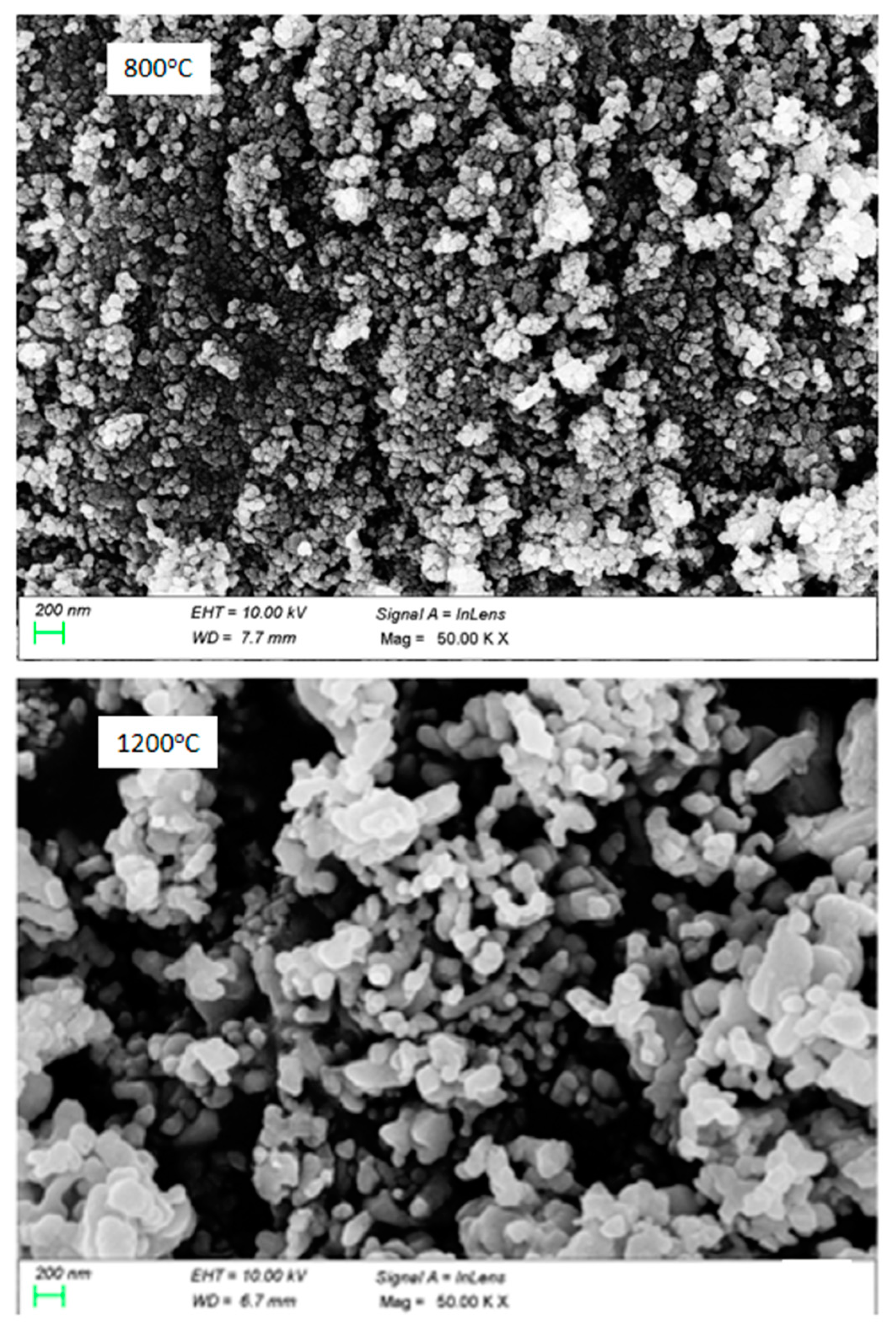
3.1. Structural Characterization
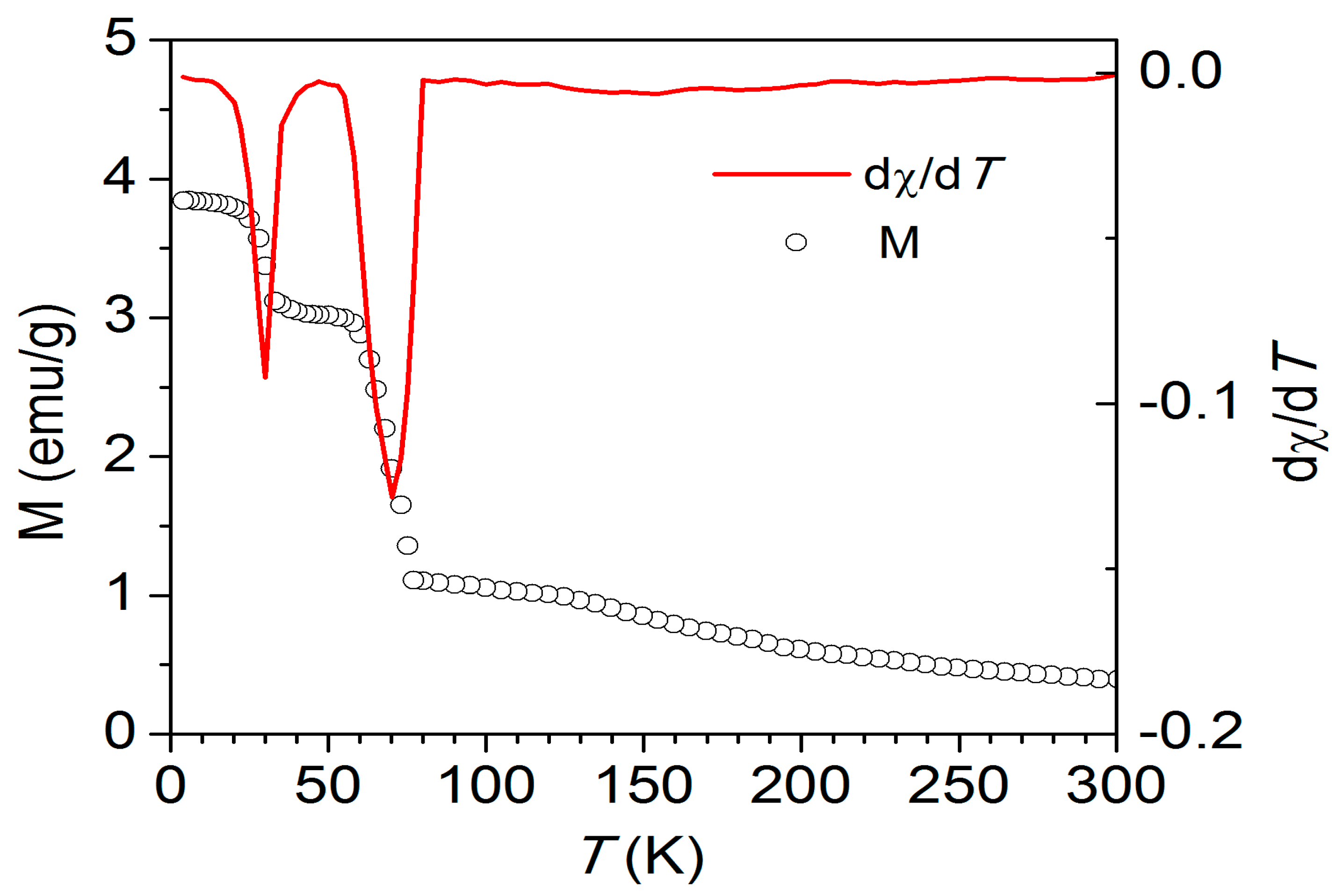
3.2. Magnetic Characterization
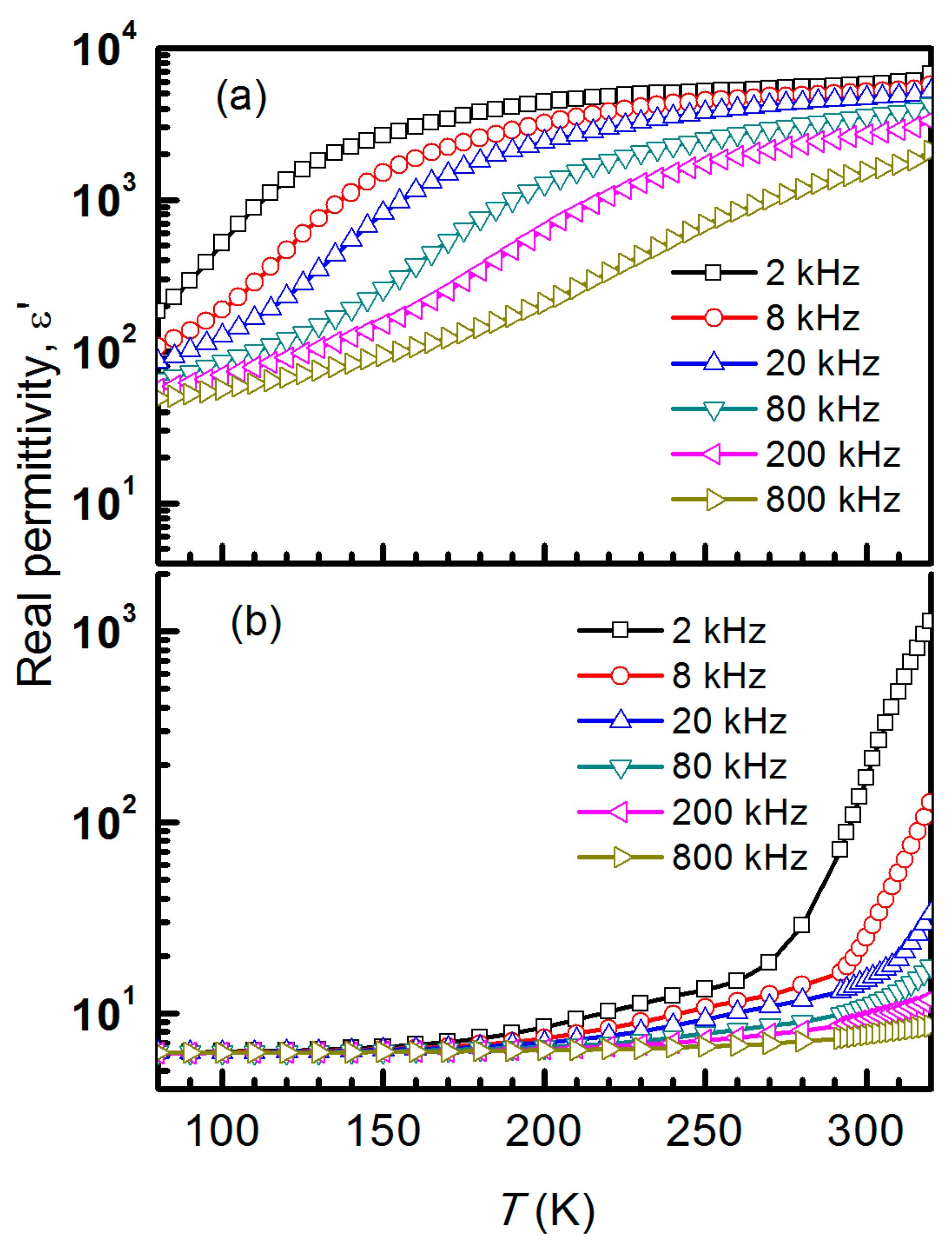
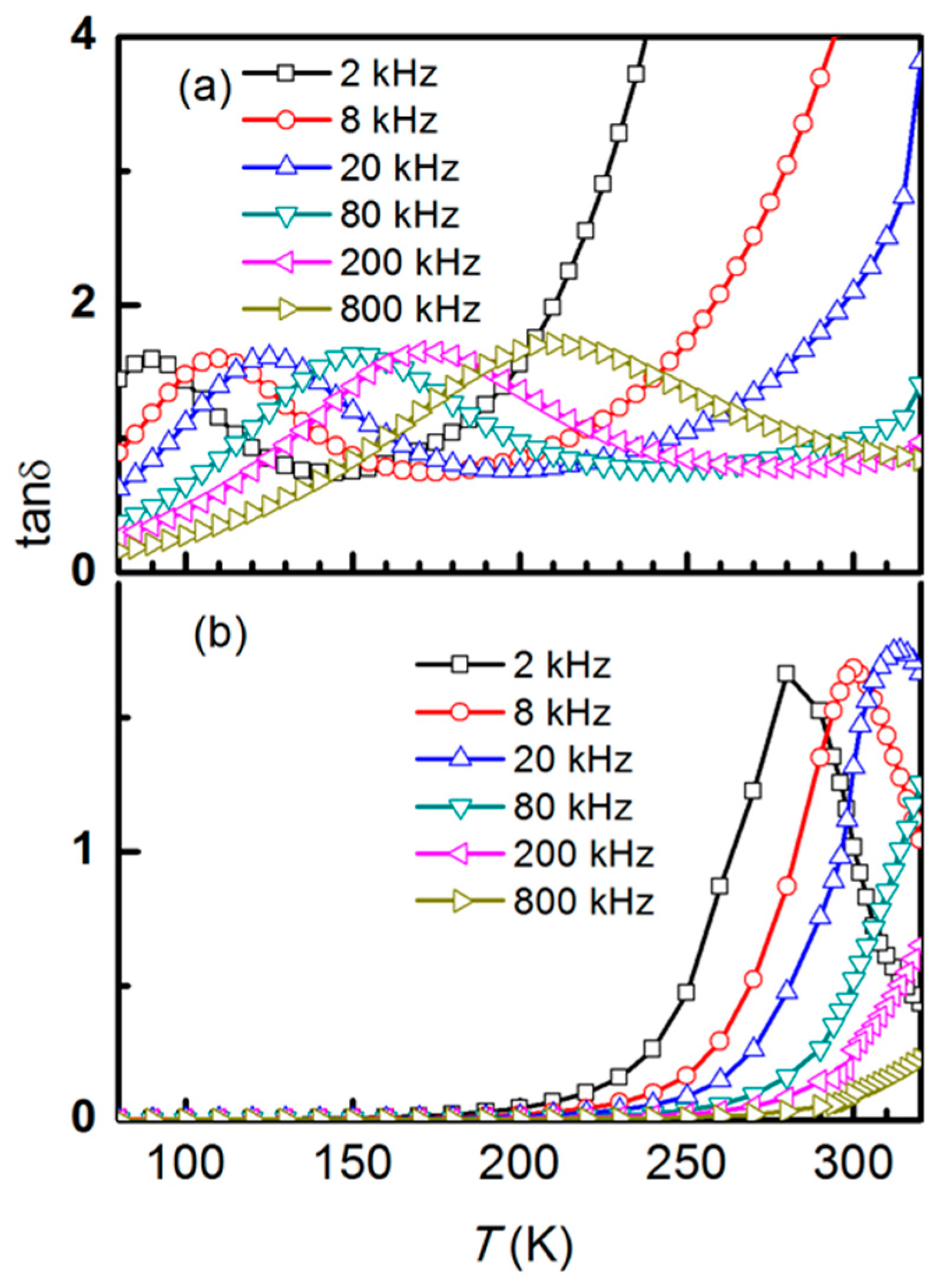
3.3. Dielectric Characterization
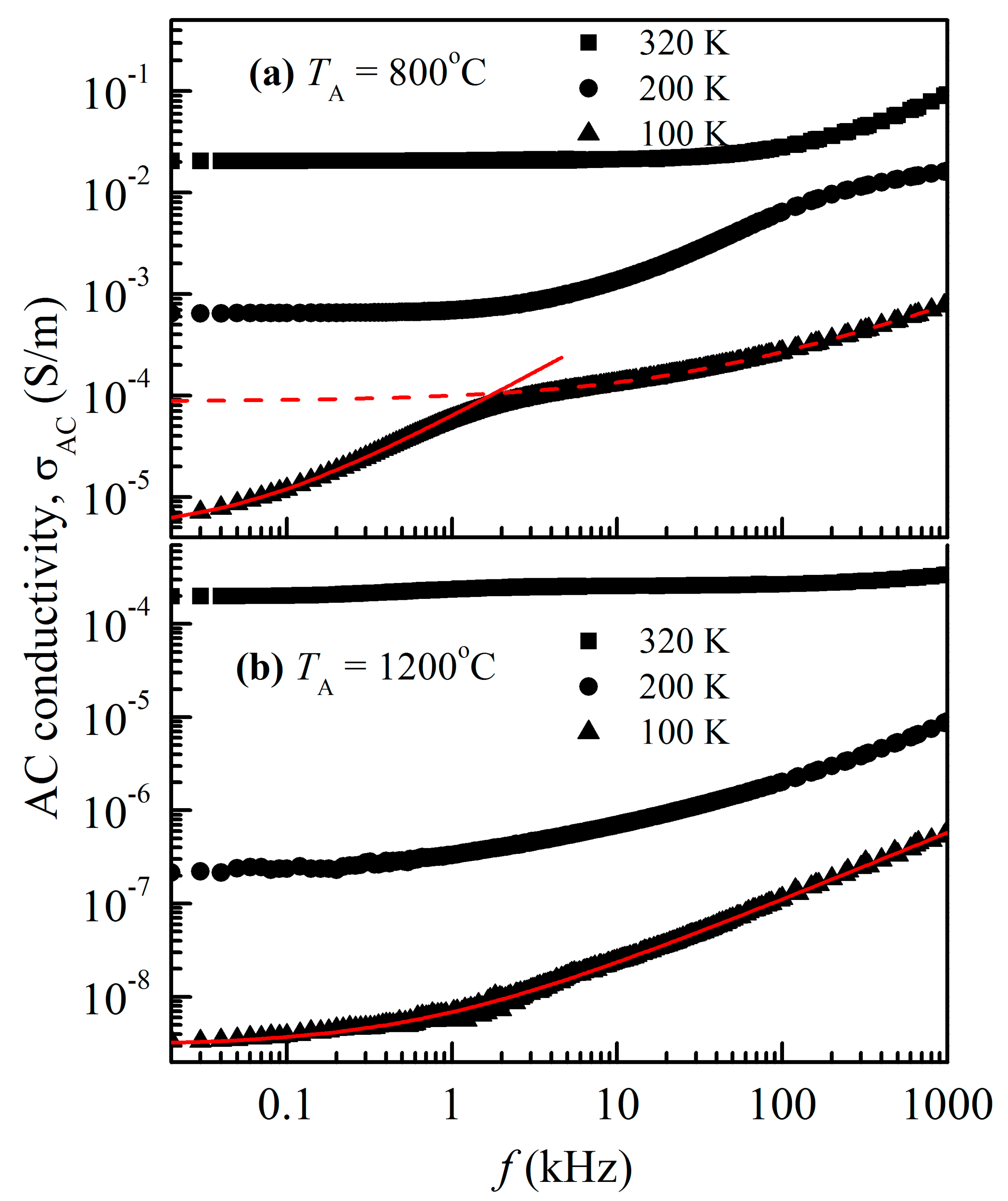
3.4. AC Conductivity
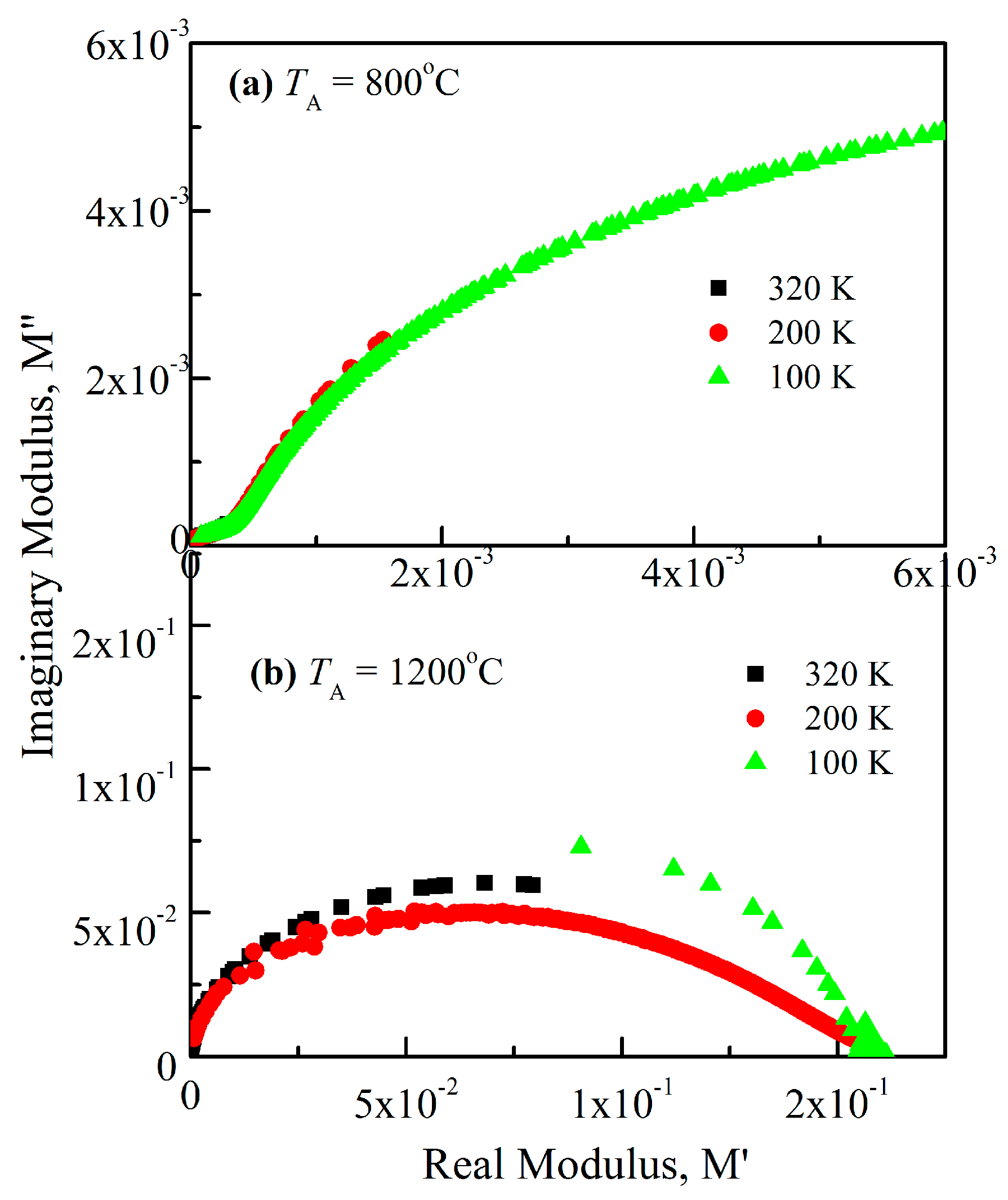
3.5. Electric Modulus
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, D.C.; Ihm, S.K. Application of spinel-type cobalt chromite as a novel catalyst for combustion of chlorinated organic pollutants. Environ. Sci. Technol. 2001, 35, 222–226. [Google Scholar] [CrossRef]
- Yamasaki, Y.; Miyasaka, S.; Kaneko, Y.; He, J.P.; Arima, T.; Tokura, Y. Magnetic reversal of the ferroelectric polarization in a multiferroic spinel oxide. Phys. Rev. Lett. 2006, 96, 207204. [Google Scholar] [CrossRef] [PubMed]
- Mufti, N.; Nugroho, A.A.; Blake, G.R.; Palstra, T.T.M. Magnetodielectric coupling in frustrated spin systems: The spinels MCr2O4 (M = Mn, Co and Ni). J. Phys. Condens. Matter. 2010, 22, 075902. [Google Scholar] [CrossRef]
- Suchomel, M.R.; Shoemaker, D.P.; Ribaud, L.; Kemei, M.C.; Seshadri, R. Spin-induced symmetry breaking in orbitally ordered NiCr2O4 and CuCr2O4. Phys. Rev. B 2012, 86, 054406. [Google Scholar] [CrossRef]
- Hu, W.; Qin, N.; Wu, G.; Li, S.; Bao, D. Opportunity of spinel ferrite materials in nonvolatile memory device applications based on their resistive switching performances. J. Am. Chem. Soc. 2012, 134, 14658. [Google Scholar] [CrossRef] [PubMed]
- O’Neill, H.S.C.; Dollase, W.A. Crystal structures and cation distributions in simple spinels from powder XRD structural refinements: MgCr2O4, ZnCr2O4, Fe3O4 and the temperature dependence of the cation distribution in ZnAl2O4. Phys. Chem. Miner. 1994, 20, 541–555. [Google Scholar] [CrossRef]
- Kocsis, V.; Bordács, S.; Varjas, D.; Penc, K.; Abouelsayed, A.; Kuntscher, C.A.; Ohgushi, K.; Tokura, Y.; Kézsmárki, I. Magnetoelasticity in ACr2O4 spinel oxides (A= Mn, Fe, Co, Ni, and Cu). Phys. Rev. B 2013, 87, 064416. [Google Scholar] [CrossRef]
- Sparks, T.D.; Kemei, M.C.; Barton, P.T.; Seshadri, R.; Mun, E.D.; Zapf, V.S. Magnetocapacitance as a sensitive probe of magnetostructural changes in NiCr2O4. Phys. Rev. B 2014, 89, 024405. [Google Scholar] [CrossRef]
- Klemme, S.; van Miltenbur, J.C. Thermodynamic properties of nickel chromite (NiCr2O4) based on adiabatic calorimetry at low temperatures. Phys. Chem. Miner. 2002, 29, 663–667. [Google Scholar] [CrossRef]
- Ishibashi, H.; Yasumi, T. Structural transition of spinel compound NiCr2O4 at ferrimagnetic transition temperature. J. Magn. Magn. Mater. 2007, 310, e610. [Google Scholar] [CrossRef]
- Tomiyasu, K.; Kagomiya, I. Magnetic structure of NiCr2O4 studied by neutron scattering and magnetization measurements. J. Phys. Soc. Japan. 2004, 73, 2539–2542. [Google Scholar] [CrossRef]
- Rathi, A.; Babu, P.D.; Rout, P.K.; Awana, V.P.S.; Tripathi, V.K.; Nagarajan, R.; Sivaiah, B.; Pant, R.P.; Basheed, G.A. Anomalous nano-magnetic effects in non-collinear spinel chromite NiCr2O4. J. Magn. Magn. Mater. 2019, 474, 585–590. [Google Scholar] [CrossRef]
- Bhowmik, R.N.; Ranganathan, R.; Nagarajan, R. Magnetic enhancement in antiferromagnetic nanoparticle of CoRh2O4. Phys. Rev. B 2004, 69, 054430. [Google Scholar] [CrossRef]
- Pandey, G.C.; Nemkovski, K.; Su, Y.; Rath, C. Evidence of anomalous conventional and spontaneous exchange bias, high coercivity in Fe doped NiCr2O4 spinel. Dalton Trans. 2020, 49, 4502–4517. [Google Scholar] [CrossRef]
- Orlandi, M.O.; Ramirez, M.A.; Foschini, C.R.; Felix, A.A.; Varela, J.A. Giant dielectric constant materials and their applications. In Sol-Gel Processing for Conventional and Alternative Energy. Advances in Sol-Gel Derived Materials and Technologies; Aparicio, M., Jitianu, A., Klein, L., Eds.; Springer: Boston, MA, USA, 2012; pp. 123–146. [Google Scholar] [CrossRef]
- Sadashivappa, P.K.; Venkatachalam, R.; Pothu, R.; Boddula, R.; Banerjee, P.; Naik, R.; Radwan, A.B.; Al-Qahtani, N. Progressive review of functional nanomaterials-based polymer nanocomposites for efficient EMI shielding. J. Compos. Sci. 2023, 7, 77. [Google Scholar] [CrossRef]
- Banerjee, P.; Franco, A., Jr. Rare earth and transition metal doped BiFeO3 ceramics: Structural, magnetic and dielectric characterization. J. Mater. Sci. Mater. Electron. 2016, 27, 6053–6059. [Google Scholar] [CrossRef]
- Aruna, S.T.; Mukasyan, A.S. Combustion Synthesis and Nanomaterials. Curr. Opin. Solid State Mater. Sci. 2008, 12, 44–50. [Google Scholar] [CrossRef]
- Hussein, S.S.; Al-Shakarchi, E.K. Sol gel auto combustion method to prepare nanostructures LiZnCu ferrite. Ceram. Int. 2024, 50, 17913. [Google Scholar] [CrossRef]
- Gyulasaryan, H.; Kuzanyan, A.; Manukyan, A.; Mukasyan, A.S. Combustion synthesis of magnetic nanomaterials for biomedical applications. Nanomaterials 2023, 13, 1902. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, P.; Franco, A. Role of higher valent substituent on the dielectric and optical properties of Sr0.8Bi2.2Nb2O9 ceramics. Mater. Chem. Phys. 2019, 225, 213–218. [Google Scholar] [CrossRef]
- Bokov, D.; Jalil, A.T.; Chupradit, S.; Suksatan, W.; Ansari, M.J.; Shewael, I.H.; Gabdrakhman, V.H.; Kainfar, E. Nanomaterial by sol-gel method: Synthesis and Application. Adv. Mat. Sci. Eng. 2021, 2021, 21. [Google Scholar] [CrossRef]
- Bitra, H.C.R.; Rao, A.V.; Babu, K.S.; Rao, G.N. Synthesis and enhanced dielectric properties of copper oxide nanoparticles. Mater. Chem. Phys. 2020, 254, 123379. [Google Scholar] [CrossRef]
- Ahmad, M.P.; Rao, A.V.; Babu, K.S.; Rao, G.N. Effect of carbon doping on structural and dielectric properties of zinc oxide. J. Adv. Dielectr. 2020, 10, 2050017. [Google Scholar] [CrossRef]
- Shanker, J.; Rao, G.N.; Venkataramana, K.; Babu, D.S. Investigation of structural and electrical properties of NdFeO3 perovskite nanocrystalline. Phys. Lett. A 2018, 382, 2974–2977. [Google Scholar] [CrossRef]
- Ptak, M.; Maczka, M.; Hermanowicz, K.; Pikul, A.; Hanuza, J. Particle size effects on the magnetic and phonon properties of multiferroic CoCr2O4. J. Sol. Stat. Chem. 2013, 199, 295–304. [Google Scholar] [CrossRef]
- Ali, A.A.; Shaaban, M.H. Electrical properties of LiBBaTe glass doped with Nd2O3. Solid State Sci. 2010, 12, 2148–2154. [Google Scholar] [CrossRef]
- Jonscher, A.K. On the origin of the universal dielectric response in condensed matter. Nature 1977, 267, 673–679. [Google Scholar] [CrossRef]
- Abdullah, M.H.; Yusoff, A.N. Complex impedance and dielectric properties of an MgZn ferrite. J. Alloy. Comp. 1996, 233, 129–135. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mamidipalli, N.R.; Tiyyagura, P.; Punna Rao, S.; Kothamasu, S.B.; Pothu, R.; Boddula, R.; Al-Qahtani, N. Crystallite Size Effects on Electrical Properties of Nickel Chromite (NiCr2O4) Spinel Ceramics: A Study of Structural, Magnetic, and Dielectric Transitions. ChemEngineering 2024, 8, 100. https://doi.org/10.3390/chemengineering8050100
Mamidipalli NR, Tiyyagura P, Punna Rao S, Kothamasu SB, Pothu R, Boddula R, Al-Qahtani N. Crystallite Size Effects on Electrical Properties of Nickel Chromite (NiCr2O4) Spinel Ceramics: A Study of Structural, Magnetic, and Dielectric Transitions. ChemEngineering. 2024; 8(5):100. https://doi.org/10.3390/chemengineering8050100
Chicago/Turabian StyleMamidipalli, Nagarjuna Rao, Papireddy Tiyyagura, Suryadevara Punna Rao, Suresh Babu Kothamasu, Ramyakrishna Pothu, Rajender Boddula, and Noora Al-Qahtani. 2024. "Crystallite Size Effects on Electrical Properties of Nickel Chromite (NiCr2O4) Spinel Ceramics: A Study of Structural, Magnetic, and Dielectric Transitions" ChemEngineering 8, no. 5: 100. https://doi.org/10.3390/chemengineering8050100
APA StyleMamidipalli, N. R., Tiyyagura, P., Punna Rao, S., Kothamasu, S. B., Pothu, R., Boddula, R., & Al-Qahtani, N. (2024). Crystallite Size Effects on Electrical Properties of Nickel Chromite (NiCr2O4) Spinel Ceramics: A Study of Structural, Magnetic, and Dielectric Transitions. ChemEngineering, 8(5), 100. https://doi.org/10.3390/chemengineering8050100





