Figure 1.
The 2D structure (flow domain) of the double-chamber parallel flexible valve micropump.
Figure 1.
The 2D structure (flow domain) of the double-chamber parallel flexible valve micropump.
Figure 2.
The dimensions of the microchannels.
Figure 2.
The dimensions of the microchannels.
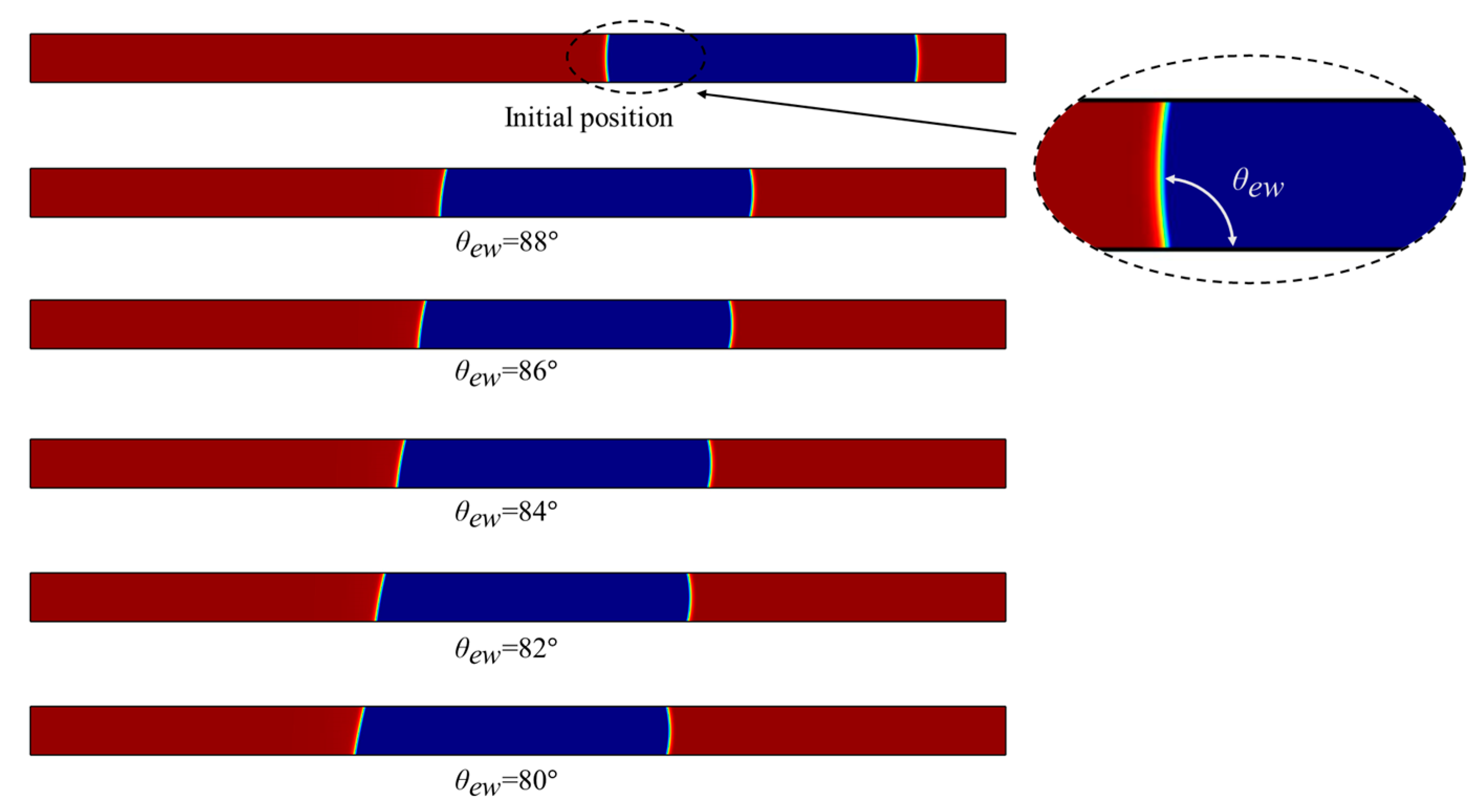
Figure 3.
Contact angle and motion of liquid droplets, blue is KCL droplet, and red is silicon oil.
Figure 3.
Contact angle and motion of liquid droplets, blue is KCL droplet, and red is silicon oil.
Figure 4.
The meshed geometry.
Figure 4.
The meshed geometry.
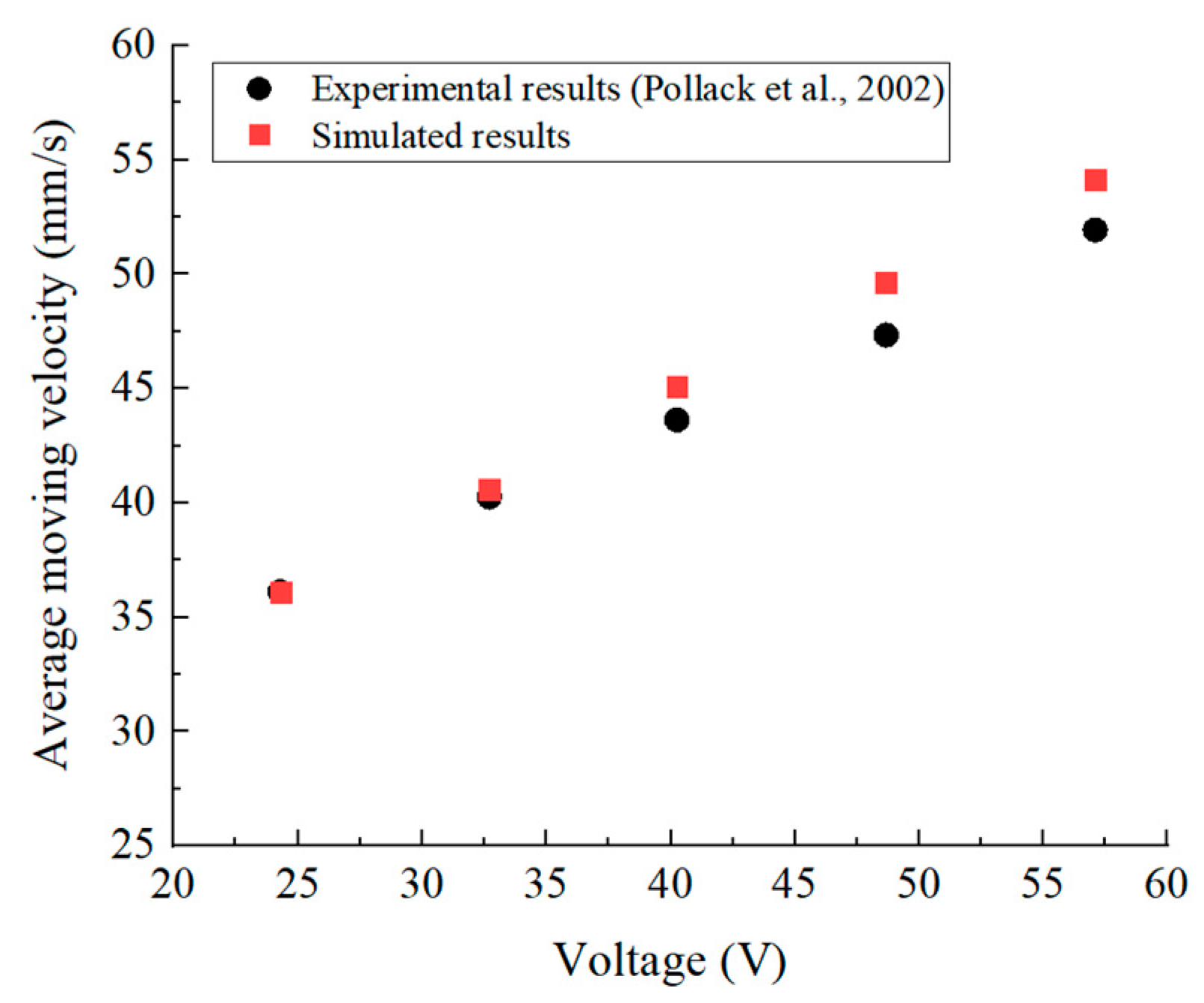
Figure 5.
A Comparison of droplet moving velocity between the simulation and the experiments [
17].
Figure 5.
A Comparison of droplet moving velocity between the simulation and the experiments [
17].
Figure 6.
Interface sharpness by varying grid resolution, blue is KCL droplet, and red is silicon oil.
Figure 6.
Interface sharpness by varying grid resolution, blue is KCL droplet, and red is silicon oil.
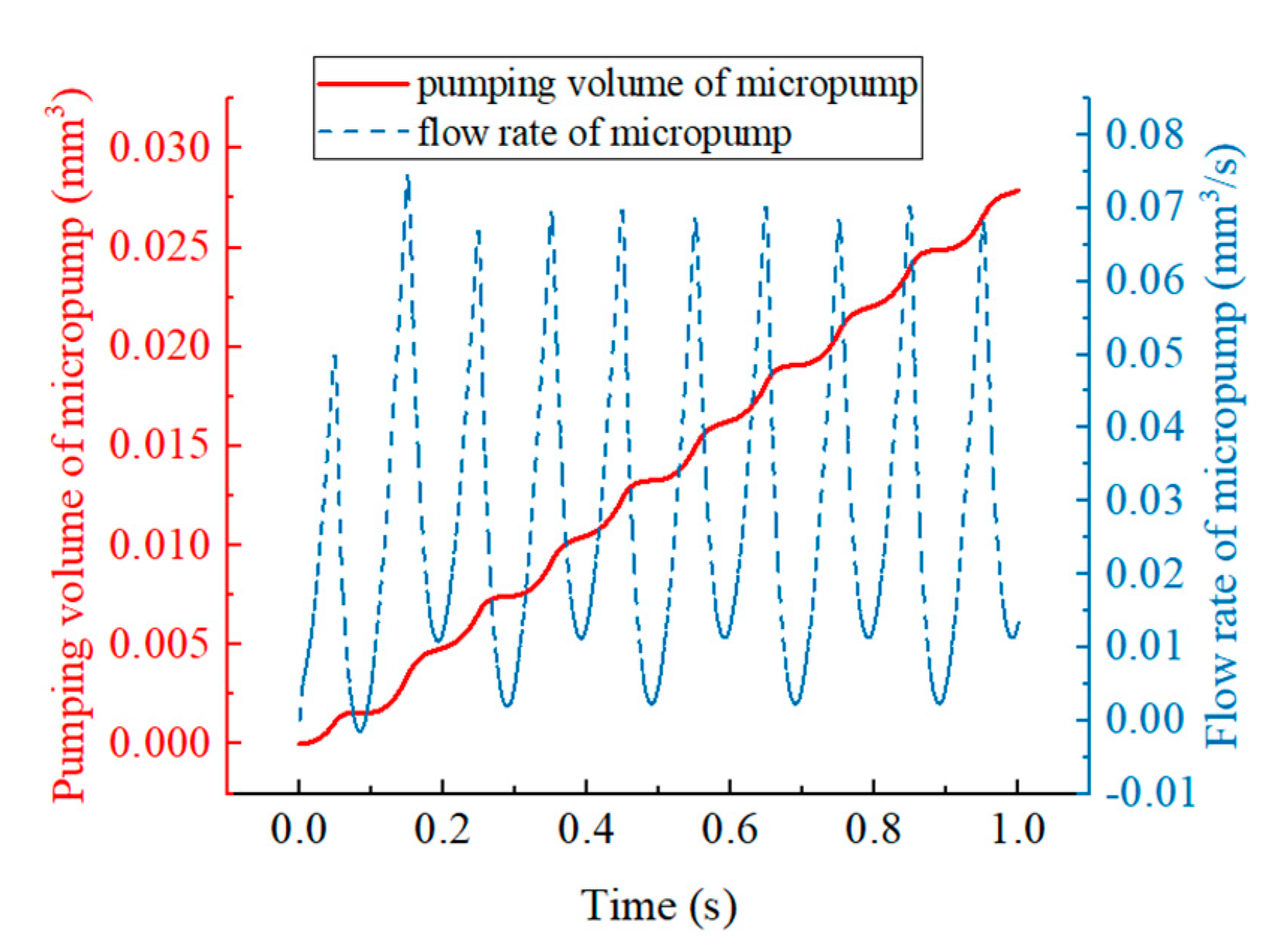
Figure 7.
The pumping volume and flow rate in the original micropump.
Figure 7.
The pumping volume and flow rate in the original micropump.
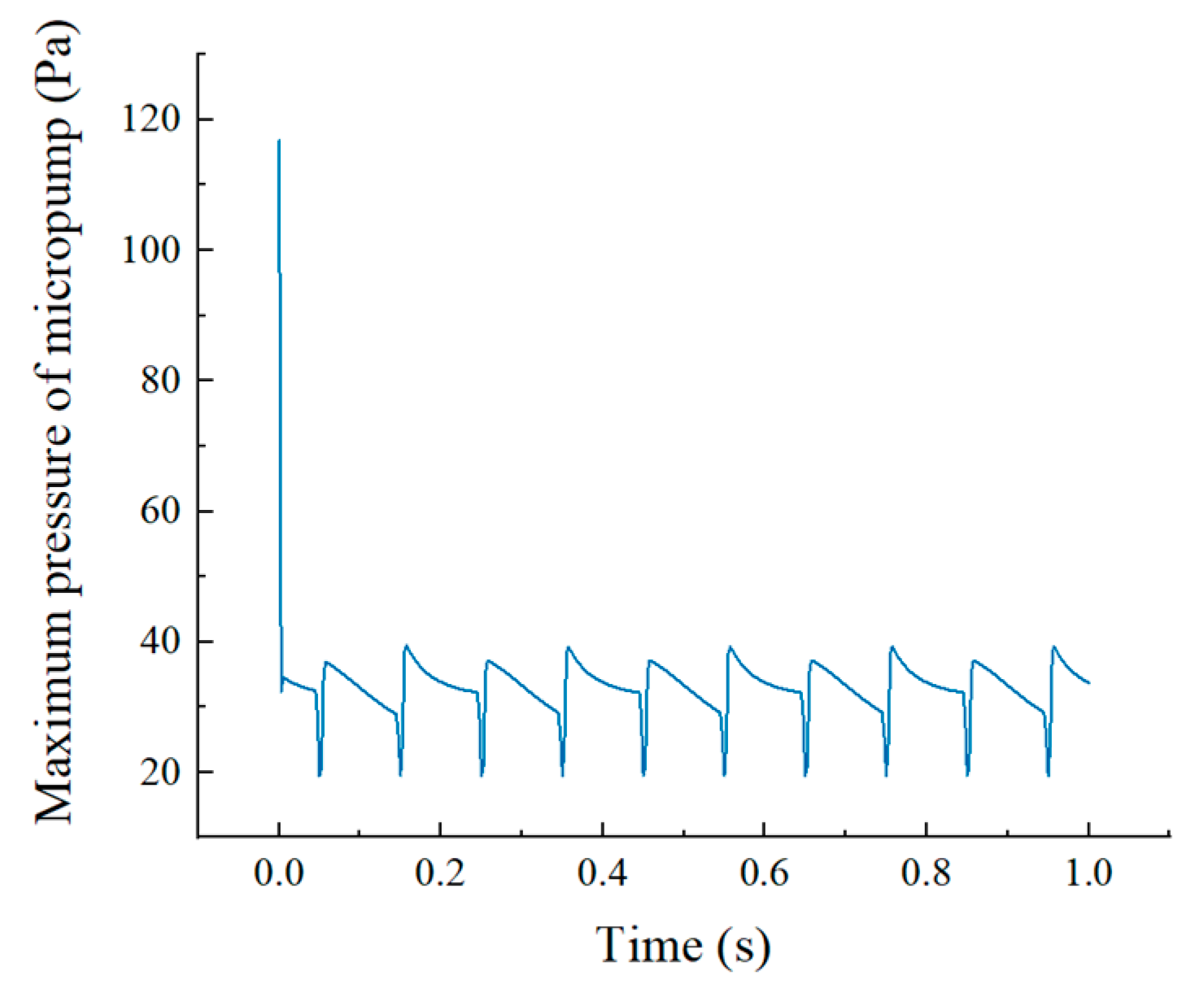
Figure 8.
The simulated maximum pressure with COMSOL.
Figure 8.
The simulated maximum pressure with COMSOL.
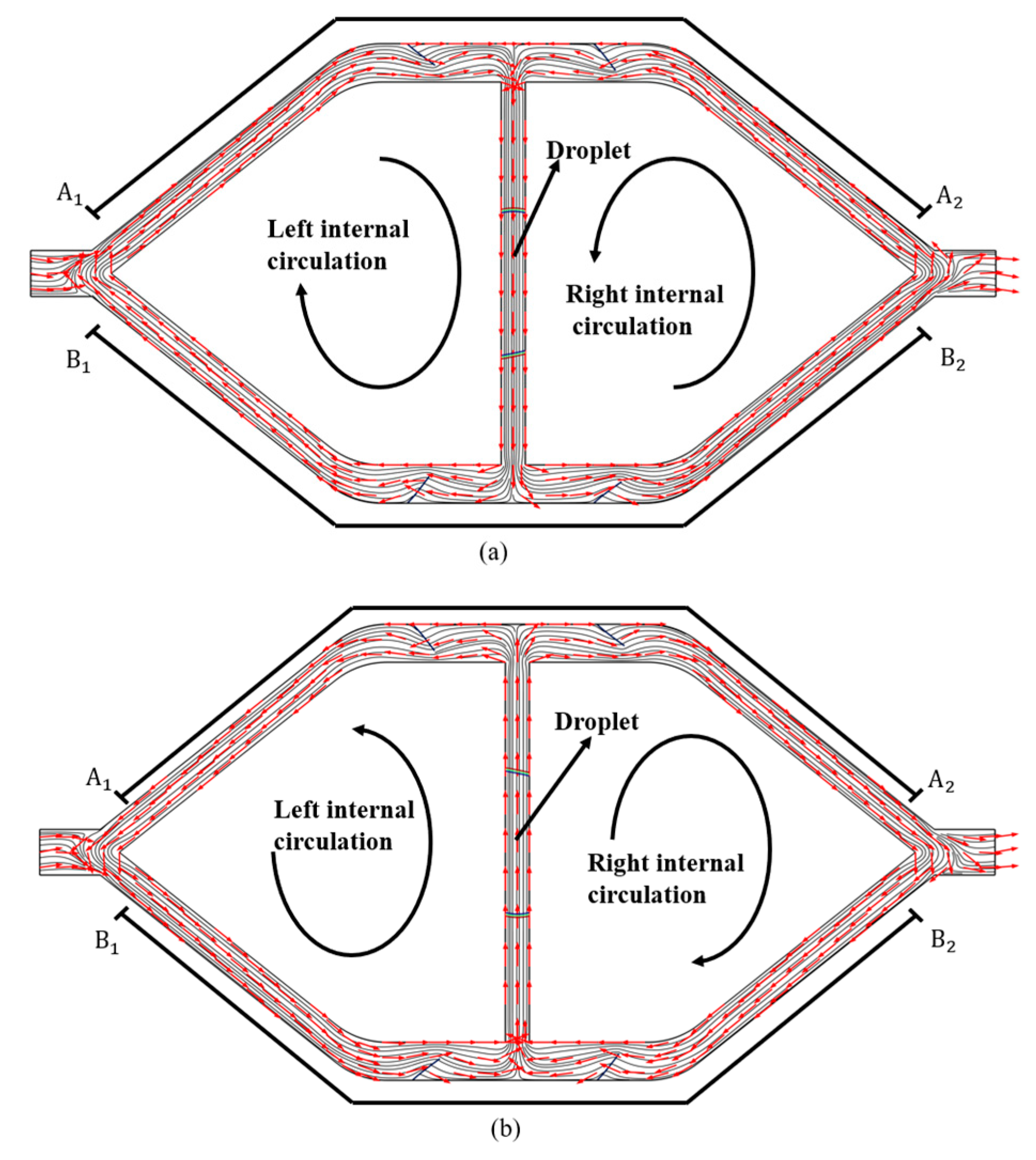
Figure 9.
The flow field inside the micropump while the KCL droplet is moving down (a) and up (b) in the pump chamber. The gray line is a streamline, and the red arrow is flowing direction.
Figure 9.
The flow field inside the micropump while the KCL droplet is moving down (a) and up (b) in the pump chamber. The gray line is a streamline, and the red arrow is flowing direction.
Figure 10.
The magnified view of internal flow on the micropump inlet and outlet. The yellow dots indicate the core of the vortices at each time instant.
Figure 10.
The magnified view of internal flow on the micropump inlet and outlet. The yellow dots indicate the core of the vortices at each time instant.
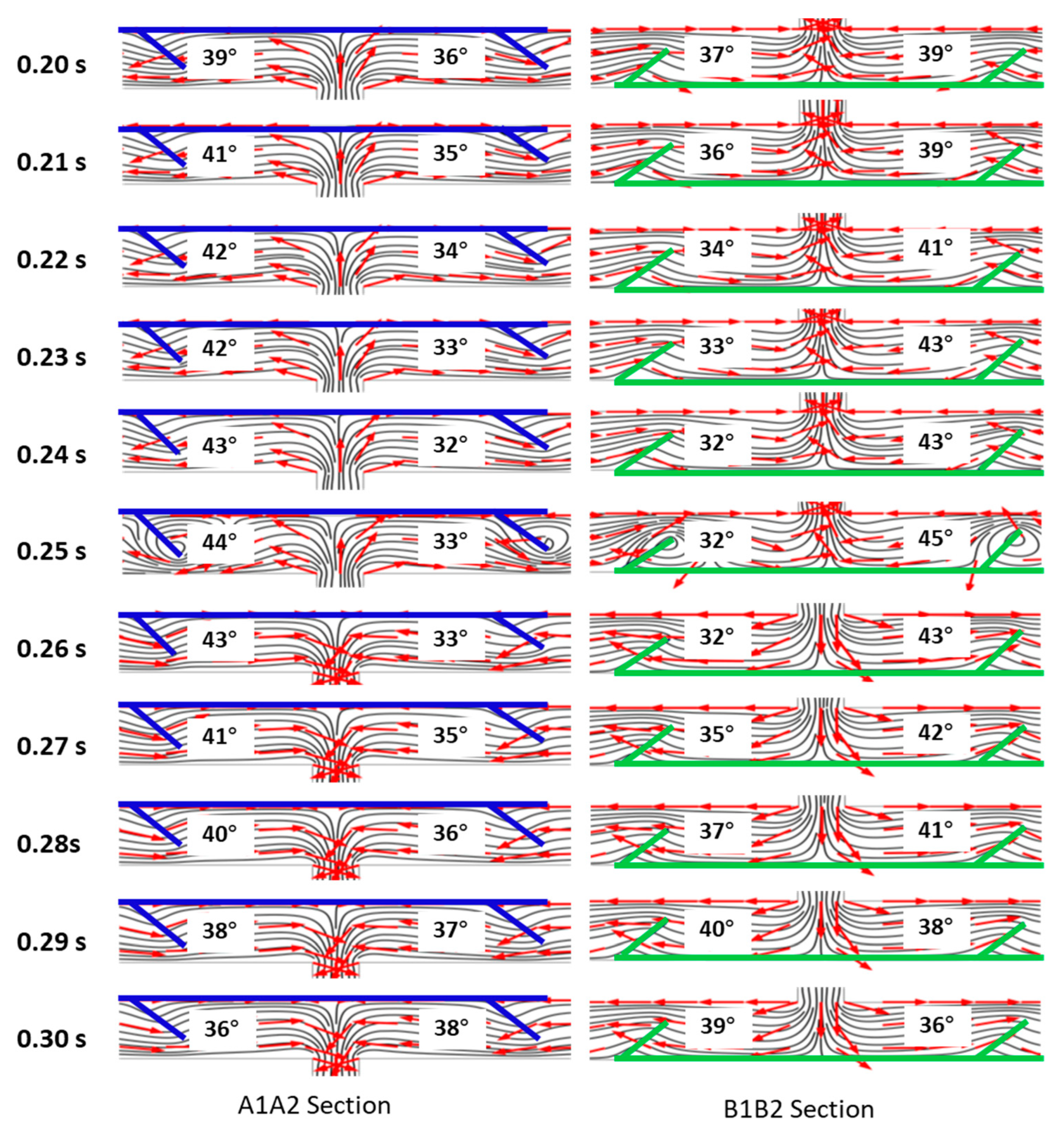
Figure 11.
The magnified view of the internal flow and the corresponding deformation of the flexible valves, blue is upper wall and flexible valve, and green is bottom wall and flexible valve.
Figure 11.
The magnified view of the internal flow and the corresponding deformation of the flexible valves, blue is upper wall and flexible valve, and green is bottom wall and flexible valve.
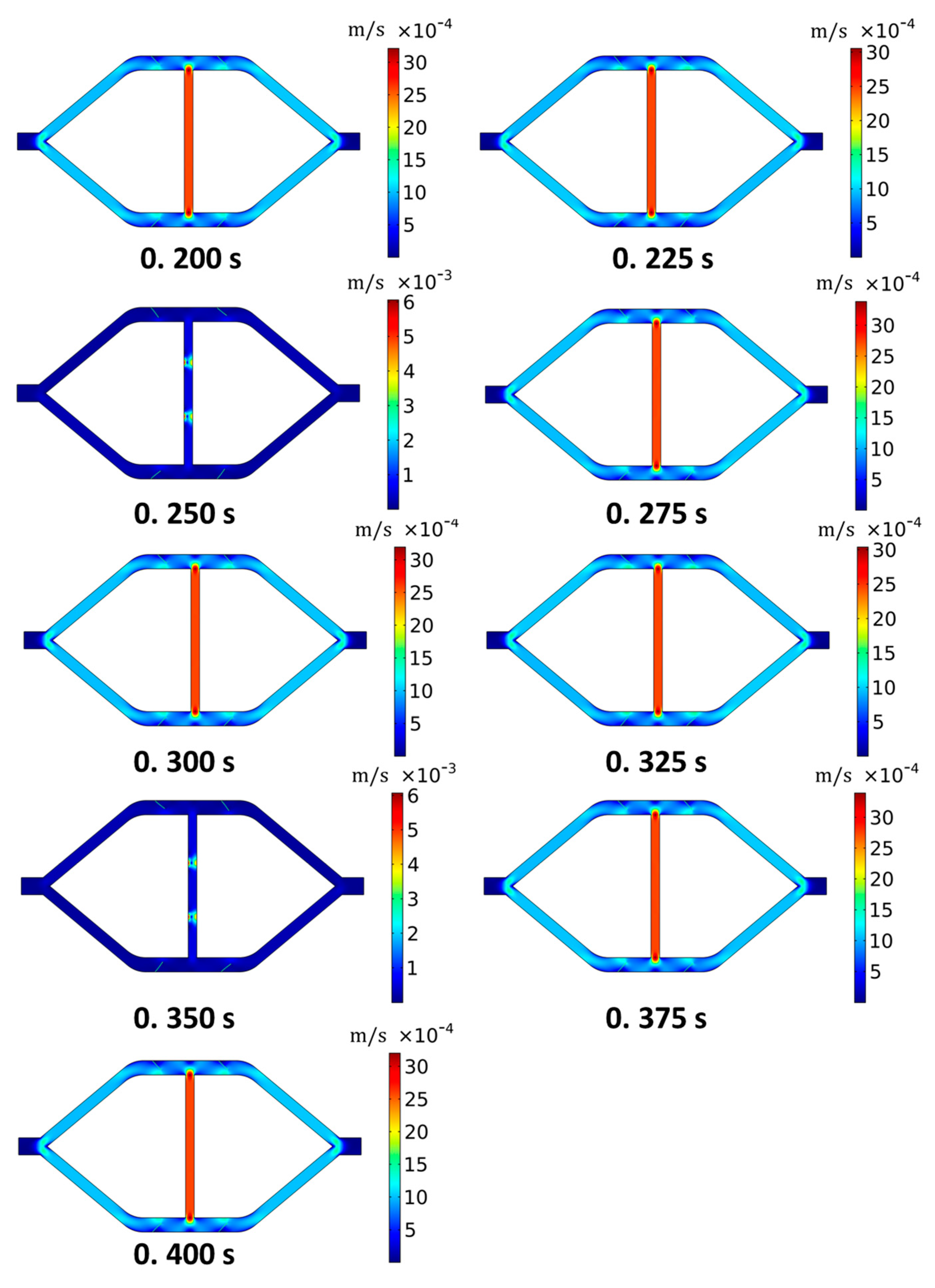
Figure 12.
The velocity contours of the parallel EWOD flexible valve pump.
Figure 12.
The velocity contours of the parallel EWOD flexible valve pump.
Figure 13.
The rectangular coordinate system at the inlet and outlet.
Figure 13.
The rectangular coordinate system at the inlet and outlet.
Figure 14.
The velocity distribution at the inlet (a,b) and outlet (c,d) of the micropump.
Figure 14.
The velocity distribution at the inlet (a,b) and outlet (c,d) of the micropump.
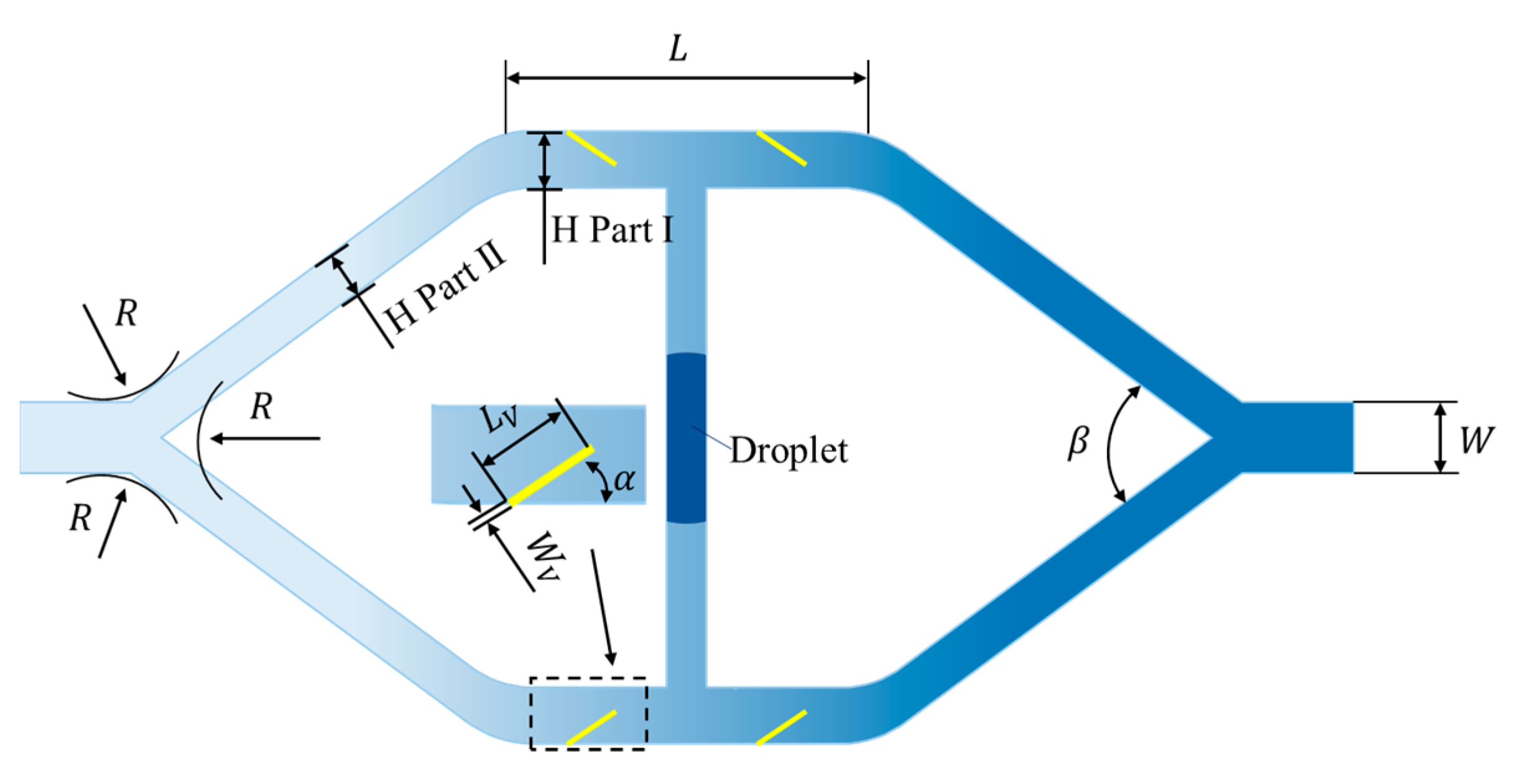
Figure 15.
The structural parameters of the micropump.
Figure 15.
The structural parameters of the micropump.
Figure 16.
The pumping volume and the maximum pressure in the micropump at different widths of inlet and outlet.
Figure 16.
The pumping volume and the maximum pressure in the micropump at different widths of inlet and outlet.
Figure 17.
The velocity distribution at different widths of the outlet, (a) is X-direction, and (b) is Y-direction.
Figure 17.
The velocity distribution at different widths of the outlet, (a) is X-direction, and (b) is Y-direction.
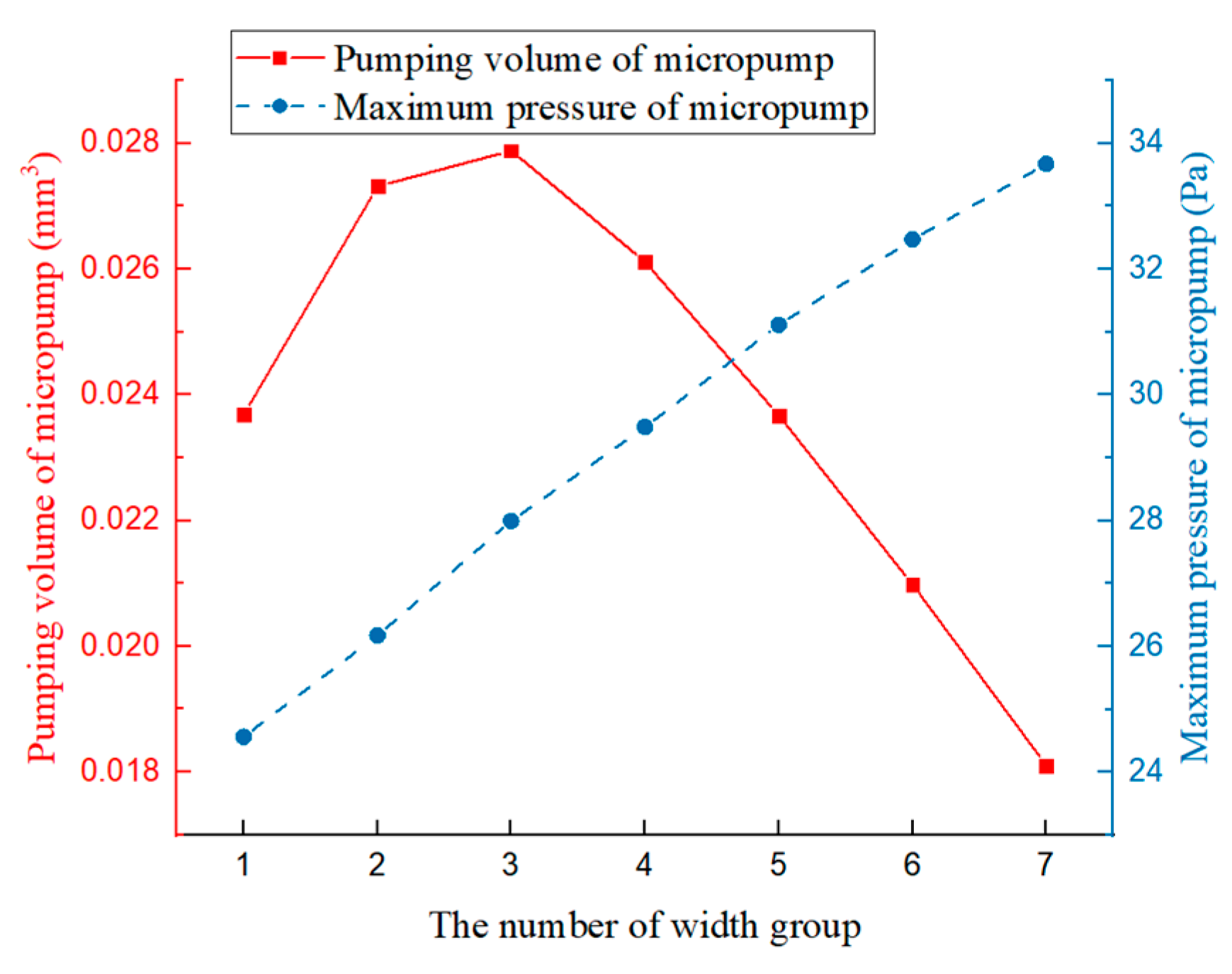
Figure 18.
The relationship of pumping volume and maximum pressure with different microchannel widths.
Figure 18.
The relationship of pumping volume and maximum pressure with different microchannel widths.
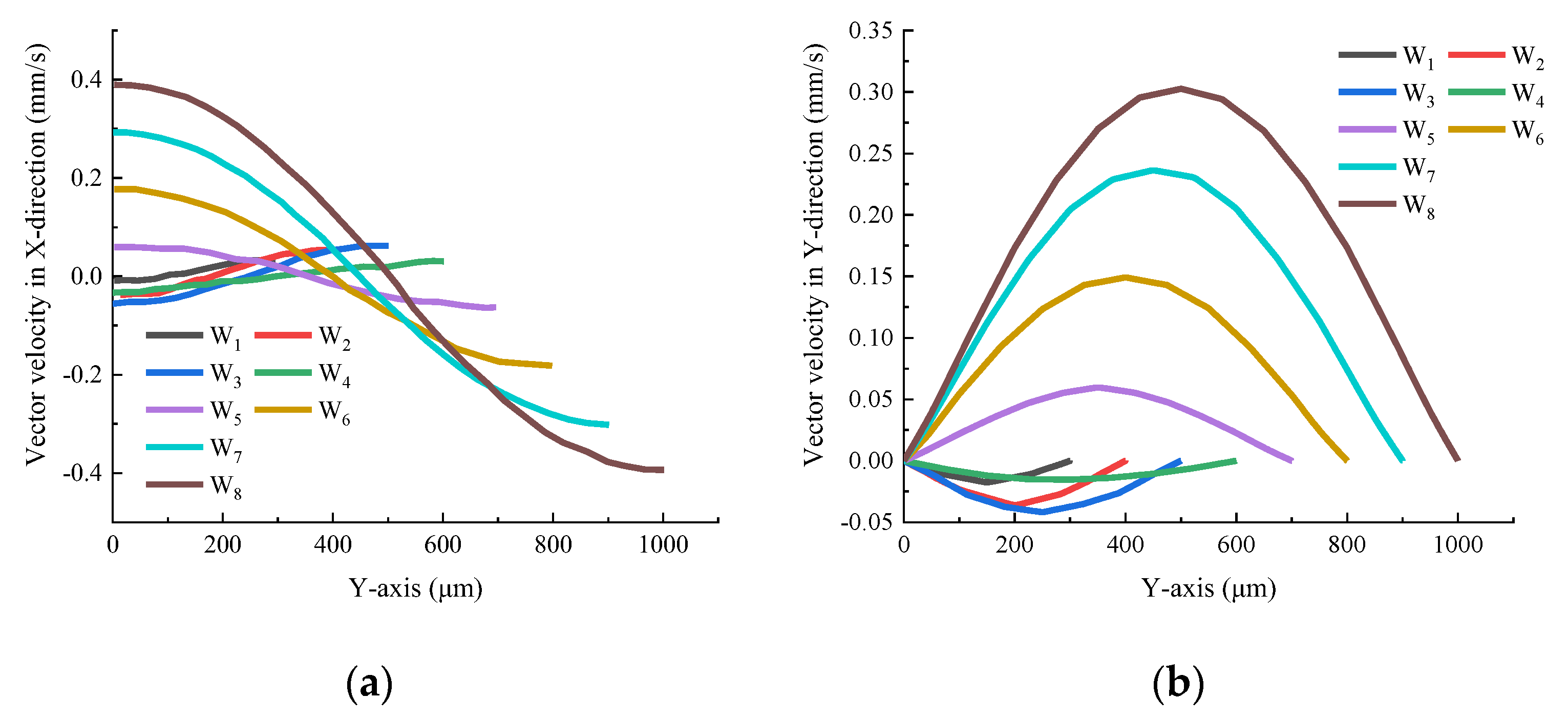
Figure 19.
The vector velocity distribution at the outlet with different microchannel widths, (a) is X-direction, and (b) is Y-direction.
Figure 19.
The vector velocity distribution at the outlet with different microchannel widths, (a) is X-direction, and (b) is Y-direction.
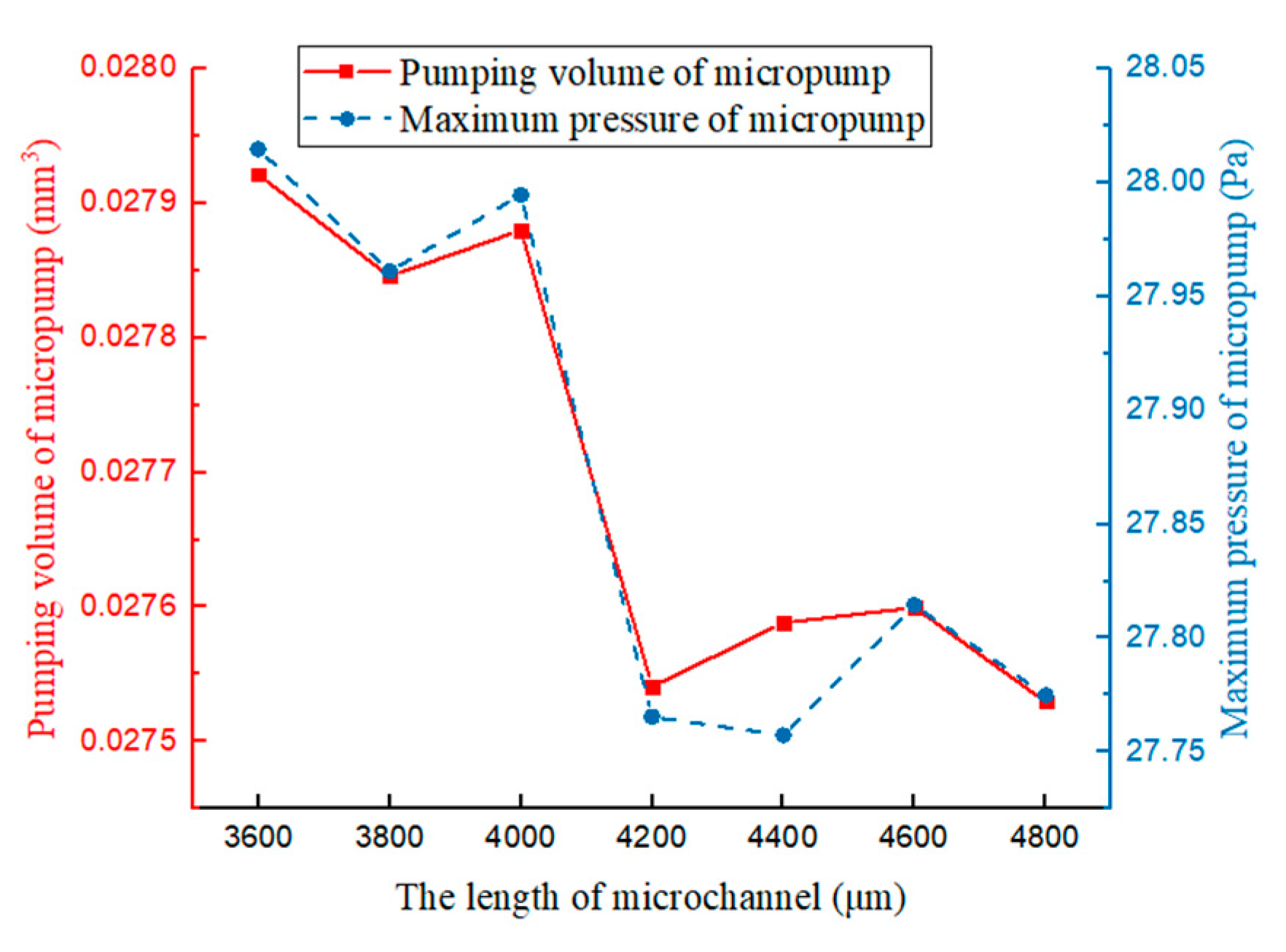
Figure 20.
The relationship of pumping volume and maximum pressure with different microchannel lengths.
Figure 20.
The relationship of pumping volume and maximum pressure with different microchannel lengths.
Figure 21.
The relationship of pumping volume and maximum pressure with a different fillet radius.
Figure 21.
The relationship of pumping volume and maximum pressure with a different fillet radius.
Figure 22.
The vector velocity distribution at the outlet with a different fillet radius, (a) is X-direction, and (b) is Y-direction.
Figure 22.
The vector velocity distribution at the outlet with a different fillet radius, (a) is X-direction, and (b) is Y-direction.
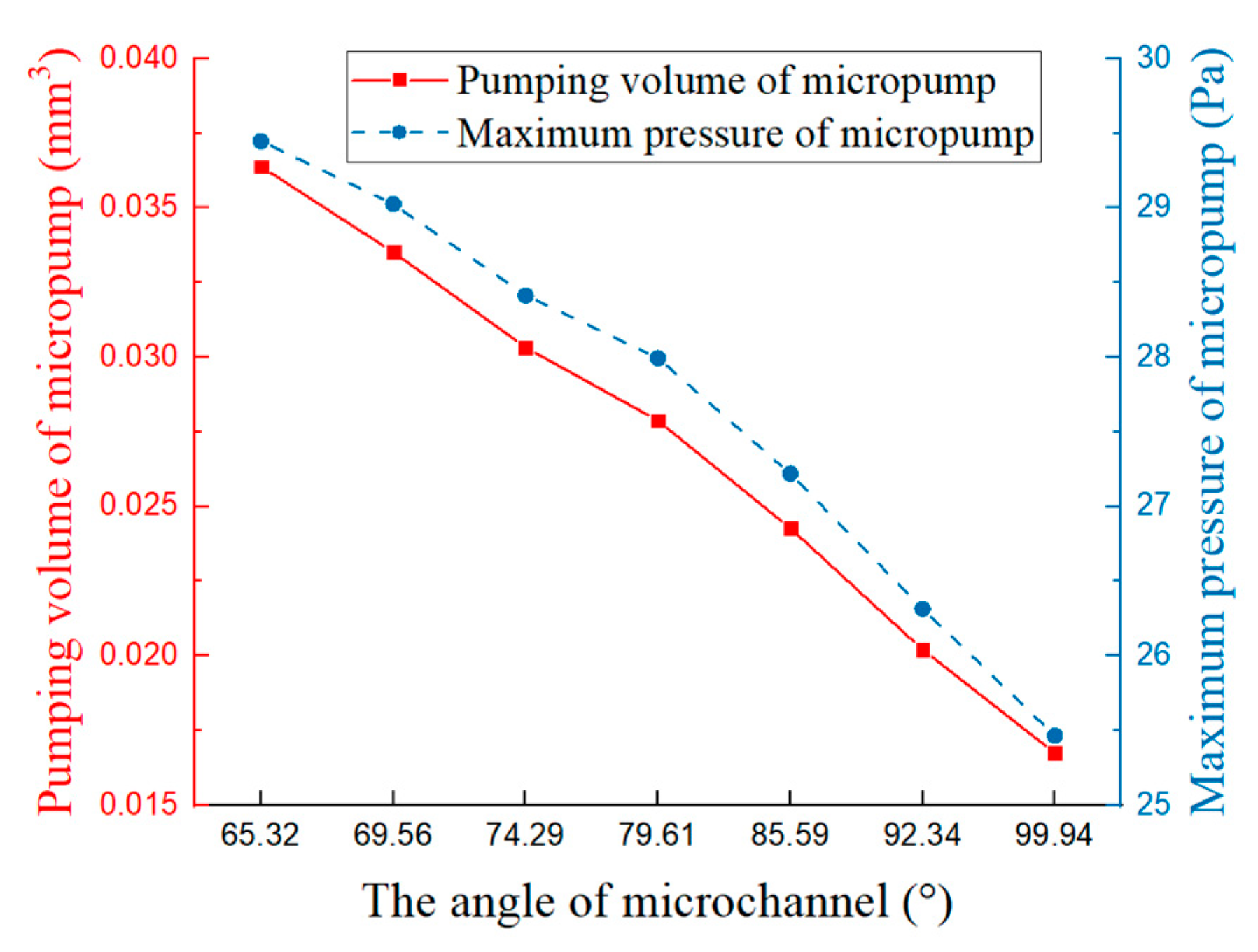
Figure 23.
The relationship of pumping volume and maximum pressure with different microchannel angles.
Figure 23.
The relationship of pumping volume and maximum pressure with different microchannel angles.
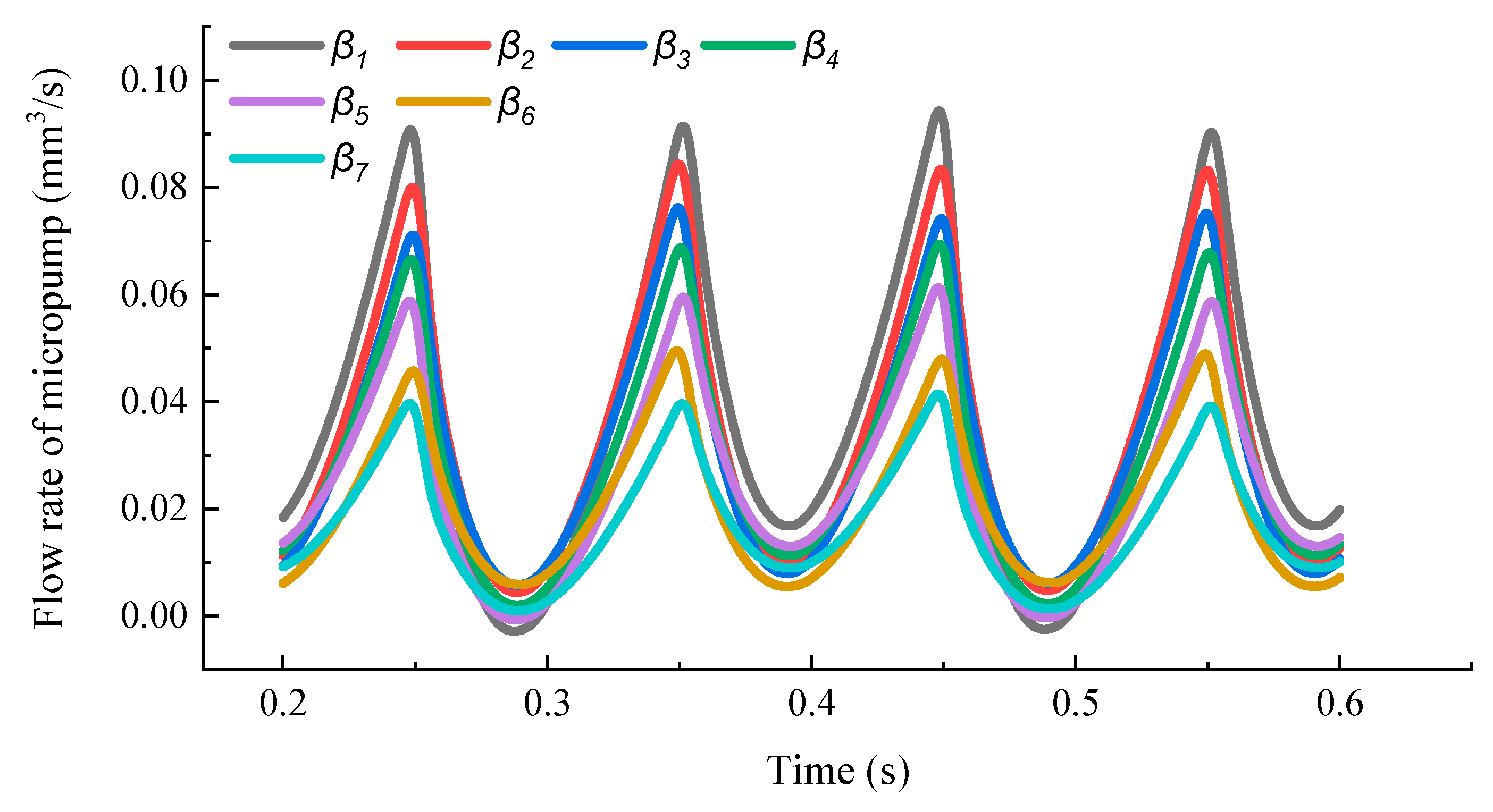
Figure 24.
The flow rate of the micropump with different microchannel angles.
Figure 24.
The flow rate of the micropump with different microchannel angles.
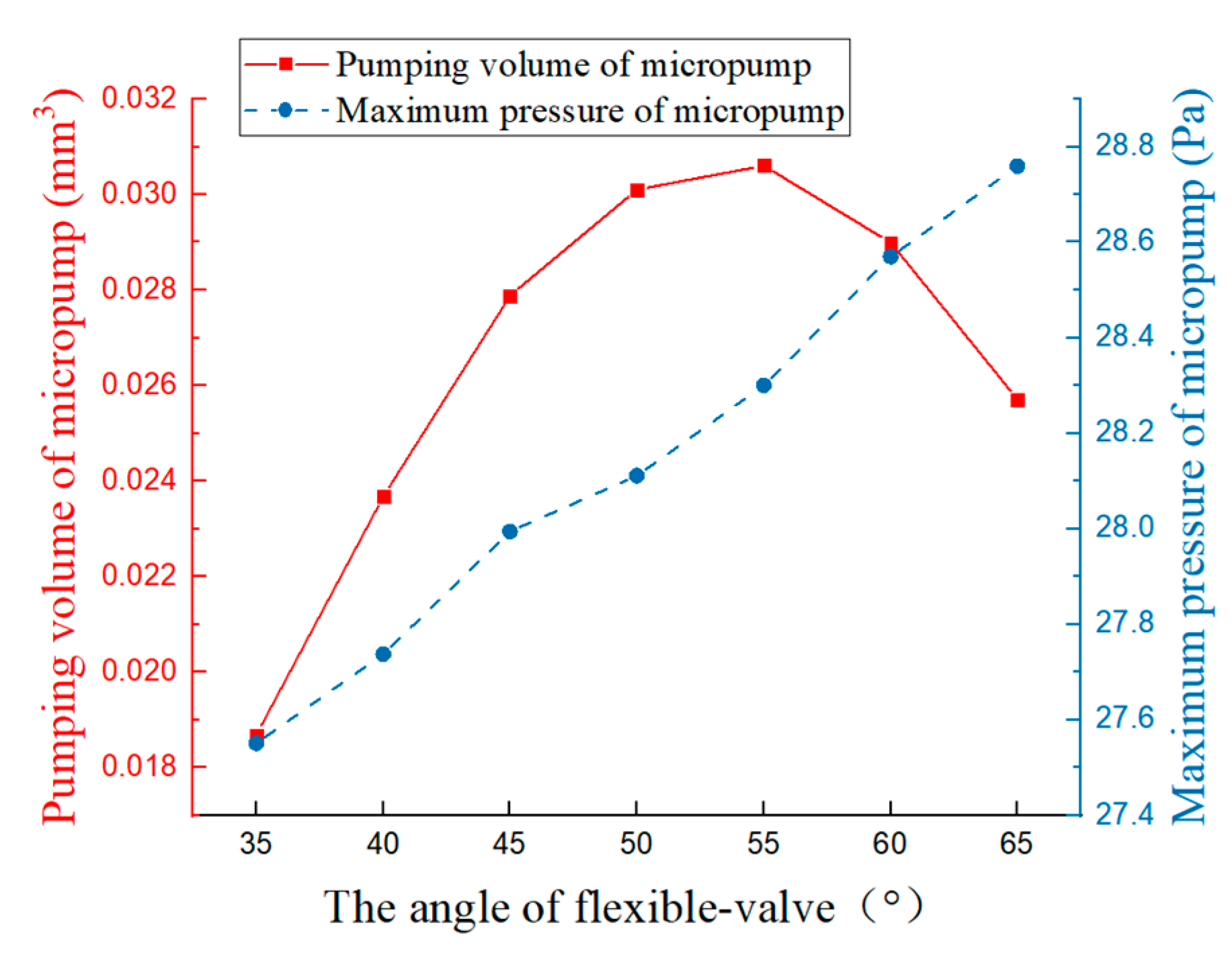
Figure 25.
The relationship of pumping volume and maximum pressure with different flexible valve angles.
Figure 25.
The relationship of pumping volume and maximum pressure with different flexible valve angles.
Figure 26.
The relationship of pumping volume and maximum pressure with different flexible valve width.
Figure 26.
The relationship of pumping volume and maximum pressure with different flexible valve width.
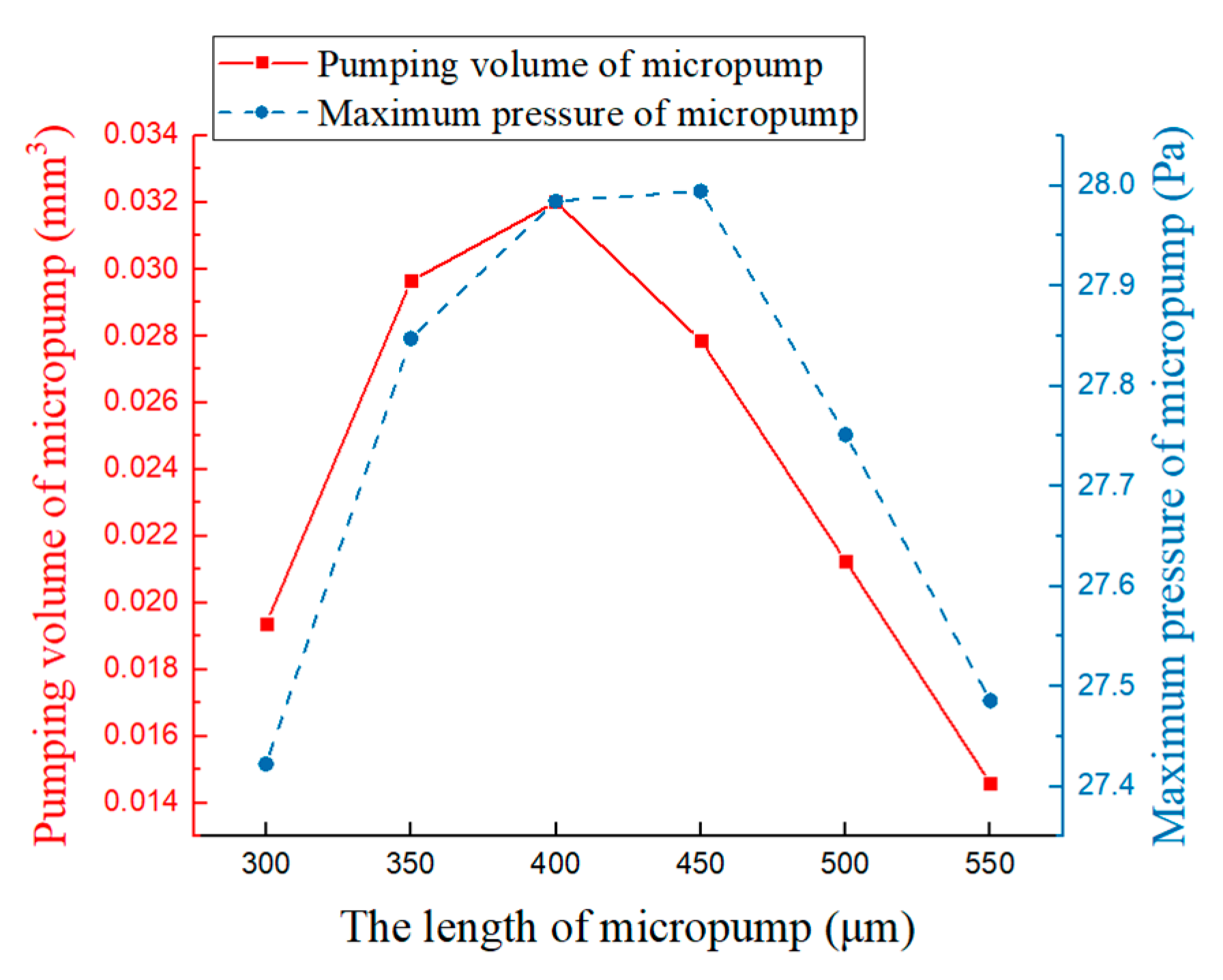
Figure 27.
The relationship of pumping volume and maximum pressure with different flexible valve lengths.
Figure 27.
The relationship of pumping volume and maximum pressure with different flexible valve lengths.
Figure 28.
The relationship of pumping volume and maximum pressure with different flexible valve material properties, (a) the effect of flexible valve density on the performance of micropumps, (b) the effect of flexible valve Young’s modulus on micropump performance, and (c) the effect of flexible valve Poisson’s ratio on micropump performance.
Figure 28.
The relationship of pumping volume and maximum pressure with different flexible valve material properties, (a) the effect of flexible valve density on the performance of micropumps, (b) the effect of flexible valve Young’s modulus on micropump performance, and (c) the effect of flexible valve Poisson’s ratio on micropump performance.
Figure 29.
The Pumping performance of in the optimized micropump, (a) is the pumping volume and flow rate, (b) is the maximum pressure.
Figure 29.
The Pumping performance of in the optimized micropump, (a) is the pumping volume and flow rate, (b) is the maximum pressure.
Table 1.
Table of basic physical characteristics of KCL solution and silicone oil.
Table 1.
Table of basic physical characteristics of KCL solution and silicone oil.
| Material | | | |
|---|
| KCL solution | 1000 | 0.87 | 2 |
| Silicone oil | 1000 | 0.1 |
Table 2.
A list of the parameters in the reference simulation before the optimization.
Table 2.
A list of the parameters in the reference simulation before the optimization.
| Items | W | H | L | R | β | α | Wv | Lv | E | ν |
|---|
| Value | 600 | 500 | 4000 | 0 | 79.61° | 45° | 20 | 450 | 3 × 105 Pa | 0.499 |
Table 3.
The width of the outlet and inlet of the micropump.
Table 3.
The width of the outlet and inlet of the micropump.
| Cases | | | | | | | | |
|---|
| Width () | 300 | 400 | 500 | 600 | 700 | 800 | 900 | 1000 |
Table 4.
The width parameters of the microchannel.
Table 4.
The width parameters of the microchannel.
| Cases | | | | | | | |
|---|
| Width of Part I () | 400 | 450 | 500 | 550 | 600 | 650 | 700 |
| Width of Part II () | 310 | 350 | 380 | 420 | 460 | 500 | 540 |
Table 5.
The length parameters of the microchannel.
Table 5.
The length parameters of the microchannel.
| Cases | | | | | | | |
|---|
| Length () | 3600 | 3800 | 4000 | 4200 | 4400 | 4600 | 4800 |
Table 6.
The fillet-radius parameters of the microchannel.
Table 6.
The fillet-radius parameters of the microchannel.
| Cases | | | | | | | |
|---|
| Radius () | 200 | 300 | 400 | 500 | 600 | 700 | 800 |
Table 7.
The angle parameters of the microchannel.
Table 7.
The angle parameters of the microchannel.
| Cases | | | | | | | |
|---|
| Angle () | 65.32 | 69.56 | 74.29 | 79.61 | 85.59 | 92.34 | 99.94 |
Table 8.
The angle parameters of the flexible valve.
Table 8.
The angle parameters of the flexible valve.
| Cases | | | | | | | |
|---|
| Angle () | 35 | 40 | 45 | 50 | 55 | 60 | 65 |
Table 9.
The width parameters of the flexible valve.
Table 9.
The width parameters of the flexible valve.
| Cases | | | | | | | |
|---|
| Width () | 10 | 15 | 20 | 25 | 30 | 35 | 40 |
Table 10.
The length parameters of the flexible valve.
Table 10.
The length parameters of the flexible valve.
| Cases | | | | | | |
|---|
| Length () | 300 | 350 | 400 | 450 | 500 | 550 |
Table 11.
The material properties of the flexible valve.
Table 11.
The material properties of the flexible valve.
| Cases | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|
Density
(kg/) | 700 | 750 | 800 | 850 | 900 | 950 | 1000 |
| Young’s modulus | | | | | | | |
| Poisson’s ratio | 0.379 | 0.399 | 0.419 | 0.439 | 0.459 | 0.479 | 0.499 |
Table 12.
The structural parameters of optimization.
Table 12.
The structural parameters of optimization.
| Cases | | | | | | | | | | | |
|---|
| Valve | 1000 | 500 380 | 3600 | 800 | | | 25 | 450 | 850 kg/ | 5 | 0.499 |