Optimization of Chemical Processes by the Hydrodynamic Simulation Method (HSM)
Abstract
:1. Introduction
2. Materials and Methods
2.1. Scale-Down Scale-Up (SDSU) Methodology in Process Development
2.2. The Use of the Hydrodynamic Simulations Method for Process Development and Optimization
3. Results
3.1. Example 1: Single-Phase Flow
3.2. Example 2: Two Phase Flow without Chemical Reaction
3.3. Example 3: Two-Phase Flow with Chemical Reaction
4. Conclusions
Author Contributions
Conflicts of Interest
References
- Levenspiel, O. Chemical reaction engineering. Ind. Eng. Chem. Res. 1999, 38, 4140–4143. [Google Scholar] [CrossRef]
- Faure, A.; York, P.; Rowe, R.C. Process control and scale-up of pharmaceutical wet granulation processes: A review. Eur. J. Pharm. Biopharm. 2001, 52, 269–277. [Google Scholar] [CrossRef]
- Schmidt, F.R. Optimization and scale up of industrial fermentation processes. Appl. Microbiol. Biotechnol. 2005, 68, 425–435. [Google Scholar] [CrossRef] [PubMed]
- Kunii, D.; Levenspiel, O. Fluidization Engineering; Howard Brenner: New York City, NY, USA, 1991; ISBN 9780409902334. [Google Scholar]
- Bentolila, M.; Novoa, R. Scale up Methodology for the Fine Chemical Industry—The Influence of the Mixing in the Process; VISIMIX Ltd.: Jerusalem, Israel, 2011. [Google Scholar]
- Harnby, N.; Edwards, M.F.; Nienow, A.W. Mixing in the Process Industries; Butterworth-Heinemann: Oxford, UK, 1997; ISBN 9780080536583. [Google Scholar]
- Holman, J. Heat Transfer; Mc Graw Hill: New York City, NY, USA, 2010. [Google Scholar]
- Dutta, B.K. Principles of mass transfer and separation processes. Can. J. Chem. Eng. 2009, 87, 818–819. [Google Scholar] [CrossRef]
- Paul, E.L.; Kresta, S.M. Handbook of Industrial Mixing; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar] [CrossRef]
- Ng, K.M.; Wibowo, C. Beyond process design: The emergence of a process development focus. Korean J. Chem. Eng. 2003, 20, 791–798. [Google Scholar] [CrossRef]
- Mah, R.S.H. Chemical Process Structures and Information Flows; Butterworths: Oxford, UK, 1990; ISBN 9781483278339. [Google Scholar]
- Berty, J.M. Changing Role of the Pilot Plant. Chem. Eng. Prog. 1979, 75, 48–51. [Google Scholar]
- Basu, P.K.; Mack, R.A.; Vinson, J.M. Consider a new approach to pharmaceutical process development. Chem. Eng. Prog. 1999, 95, 82–90. [Google Scholar]
- Genck, W.; Hasson, M.; Manoff, E.; Novoa, R.; Bentolila, M. Computer aided process engineering at chemagis. Pharm. Eng. 2011, 31, 30–38. [Google Scholar]
- Braginskii, L.N.; Kokotov, Y.V.; Gordeev, L.S. Characteristics of macroscale transfer during mixing in apparatus with reflecting partitions. Teor. Osn. Khimicheskoi Teknol. 1986, 20, 375–380. [Google Scholar]
- Barabash, V.M.; Bragisnkii, L.N.; Gorbacheva, G.V. Calculating the Gad Concentration in Apparatus Equiped with Agitator. Teor. Osn. Khimicheskoi Teknol. 1988, 21, 654–660. [Google Scholar]
- Barabash, V.M.; Braginskii, L.N.; Liv’yant, R.Z.; Sadovskii, V.L. Effect of near-wall turbulance on mixing hydrodynamics. Teor. Osn. Khimicheskoi Teknol. 1986, 20, 798–804. [Google Scholar]
- Barabash, V.M.; Bragisnkii, L.N.; Kozlova, E.G. Use Mixing Apparatus for Highly Concentrated Suspensions. Teor. Osn. Khimicheskoi Teknol. 1990, 24, 63–68. [Google Scholar]
- Rane, C.V.; Ekambara, K.; Joshi, J.B.; Ramkrishna, D. Effect of impeller design and power consumption on crystal size distribution. AIChE J. 2014, 60, 3596–3613. [Google Scholar] [CrossRef]
- Visimix Review of Some Mathematical Models Used in VisiMix. Available online: http://visimix.com/wp-content/uploads/2015/11/Review-of-the-Main-Mathematical-Models.pdf (accessed on 20 November 2017).

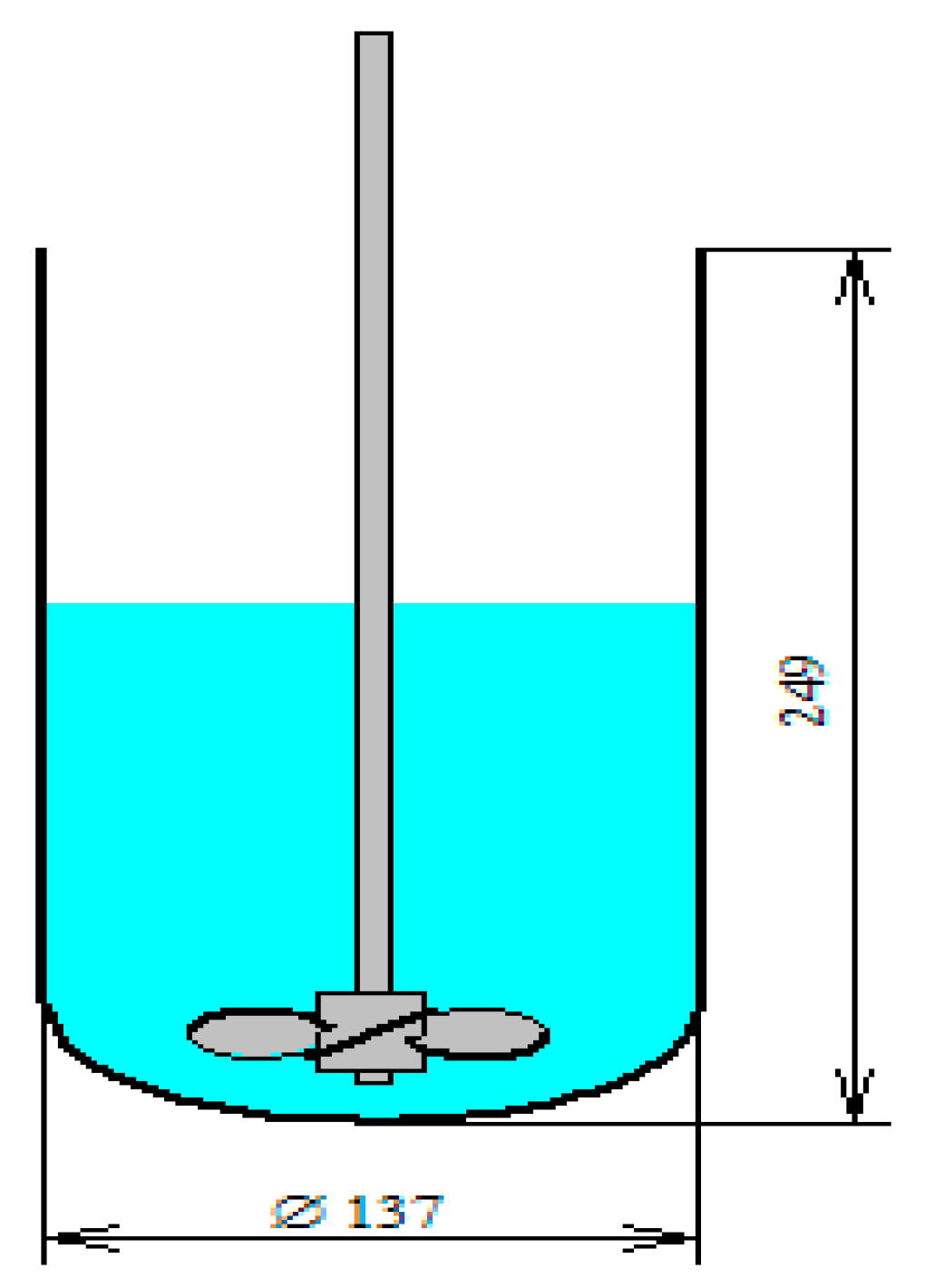
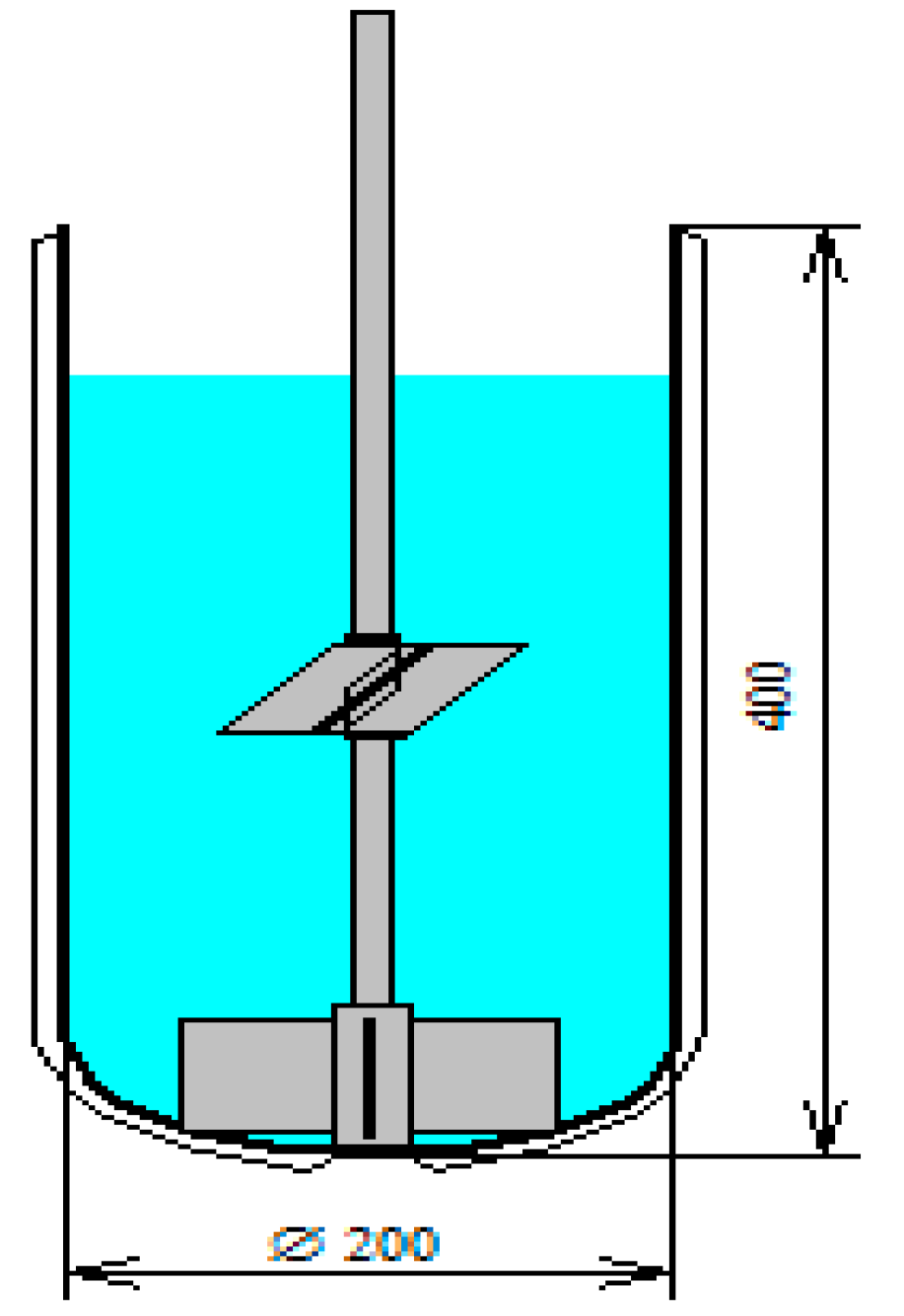
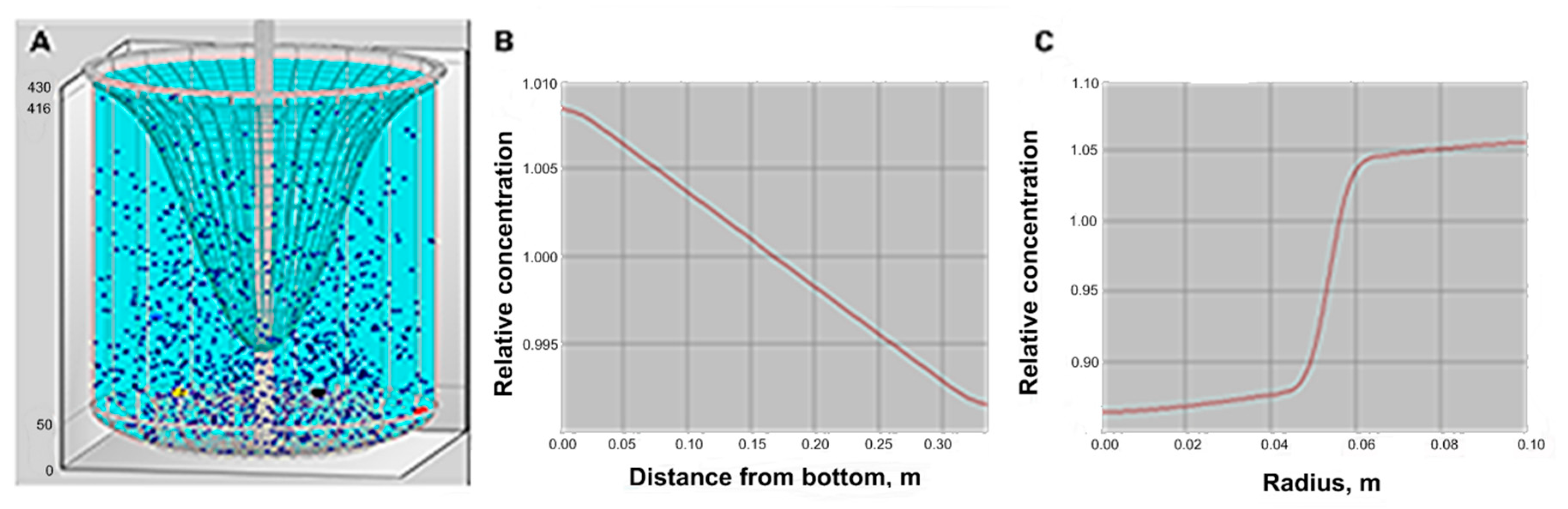
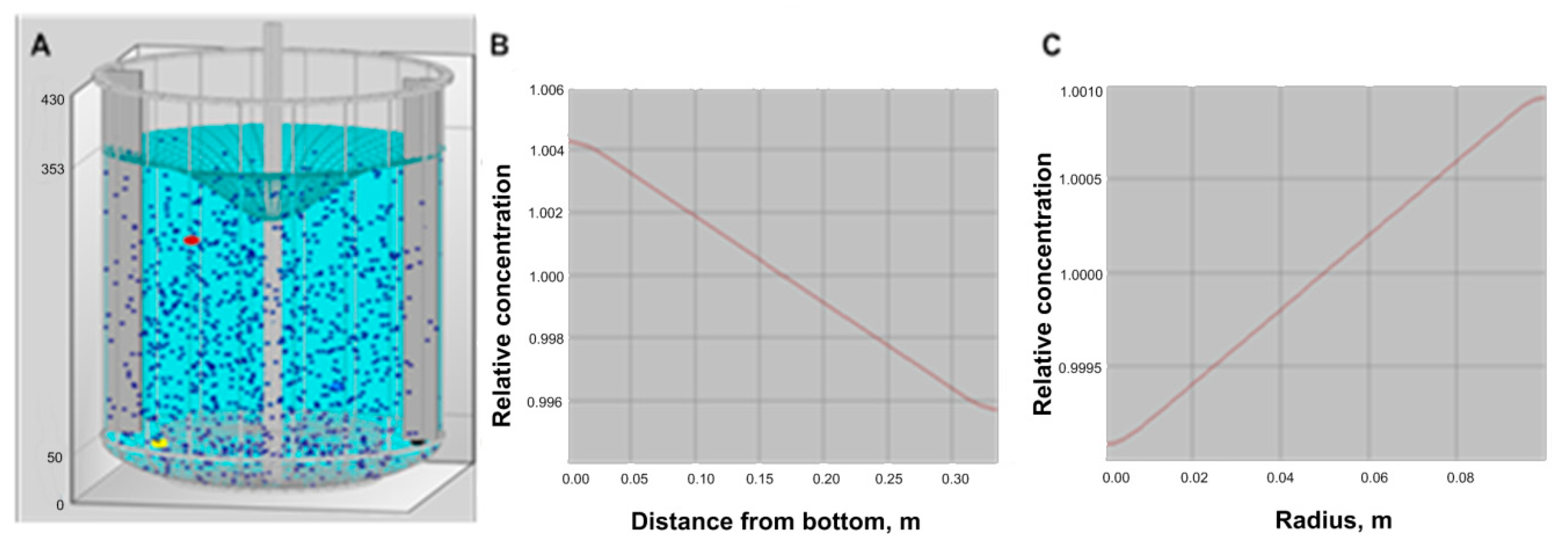
| Application | Key Process and Scale-Down Scale-Up Parameters |
|---|---|
| Newtonian Non Newtonian Hydrodynamics and Scale-up | Circulation flow rate |
| Local values of energy dissipation | |
| Turbulent Shear rates | |
| Blending-Single Phase mixing | Macro and micro mixing times |
| Max./Min. concentration difference ΔC | |
| Liquid-Solid Suspension, Crystallization, Dissolution | Max. local concentrations |
| Max. shear rate | |
| Crystal collision energy | |
| Liquid-Liquid Emulsification, Heterogeneous Synthesis | Drop size distribution |
| Specific mass transfer area | |
| Micro mixing time for disperse phase | |
| Liquid-Gas Gas injection, Absorption, Gas Liquid Reactors | Gas hold up |
| Specific mass transfer area | |
| Specific mass transfer coefficient | |
| Biotechnology | Oxygen mass transfer rate |
| Heat Transfer in Vessels with Different Heating/Cooling Devices | Media temperature |
| Heat transfer coefficient | |
| Specific heat/cool rate |
| General Hydrodynamics Main Characteristics | |||||
|---|---|---|---|---|---|
| Parameter | Unit | Reactor A | Reactor B | Reactor C | Reactor D |
| Rotation Speed | rpm | 45 | 45 | 200 | 500 |
| Mixing Power | W | 0.00224 | 0.0289 | 0.419 | 0.00712 |
| Reynolds for Flow | ---- | 3610 | 19800 | 42300 | 6470 |
| Tangential Velocity (Average) | m/s | 0.0241 | 0.0611 | 0.165 | 0.215 |
| Circulation Flow Rate | m3/s | 0.00042 | 0.000109 | 7.46 × 10−5 | 6.44 × 10−6 |
| Circulation Velocity (Average) | m/s | 0.0164 | 0.000616 | 0.000364 | 0.00226 |
| Turbulence Main Characteristics | |||||
| Rotation Speed | rpm | 45 | 45 | 200 | 500 |
| Energy Dissipation, Maximum | W | 0.0192 | 0.0306 | 8.14 | 8.97 |
| Energy Dissipation, Average | W | 0.000134 | 0.00029 | 0.00419 | 0.0285 |
| Energy Dissipation, Bulk Volume | W | 9.4 × 10−5 | 0.00021 | 0.00161 | 0.0123 |
| Turbulent Shear Rate Near Impeller | 1/s | 139 | 175 | 2860 | 3000 |
| Turbulent Shear Rate in Bulk Volume | 1/s | 9.74 | 14.5 | 40.3 | 111 |
| Single-Phase Mixing. Main Characteristics | |||||
| Rotation Speed | rpm | 45 | 45 | 200 | 500 |
| Macro Mixing Time | s | 102 | 122 | 198 | 27.2 |
| Mean period of circulation | s | 39.8 | 921 | 1340 | 38.8 |
| Micro Mixing Time | s | 103 | 69 | 24.9 | 9.02 |
| Parameter | Units | Value |
|---|---|---|
| General Basic Parameters | ||
| Circulation flow rate | m3/s | 2.39 × 10−5 |
| Energy dissipation in the bulk of volume | W/kg | 0.00506 |
| Microscale of turbulence in the bulk of volume | m | 0.000119 |
| Liquid-Solid Mixing | ||
| Complete suspension expected | ---- | YES |
| Maximum degree of non-uniformity-Axial | % | 15.6 |
| Maximum degree of non-uniformity-Radial | % | 57.4 |
| Maximum local concentration of solids | kg/m3 | 247 |
| Minimum local concentration of solids | kg/m3 | 73.2 |
| Parameter | Units | Value |
|---|---|---|
| General Basic Parameters | ||
| Circulation flow rate | m3/s | 0.00108 |
| Energy dissipation in the bulk of volume | W/kg | 0.0217 |
| Microscale of turbulence in the bulk of volume | m | 8.24 × 10−5 |
| Liquid-Solid Mixing | ||
| Complete suspension expected | ---- | YES |
| Maximum degree of non-uniformity-Axial | % | 4.27 |
| Maximum degree of non-uniformity-Radial | % | 0.166 |
| Maximum local concentration of solids | kg/m3 | 209 |
| Minimum local concentration of solids | kg/m3 | 191 |
| Gas Dispersion and Mass Transfer | ||
| Gas distribution | ----- | Satisfactory |
| Gas hold-up | ----- | 0.000247 |
| Sauter mean bubble diameter | m | 0.00197 |
| Estimated surface aeration rate | m/s | 0 |
| Specific mass transfer area | m2 | 0.754 |
| Specific mass transfer coefficient | 1/s | 0.000228 |
| Gas mass transfer rate | kg/h | 6.56 × 10−5 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bentolila, M.; Alshanski, I.; Novoa, R.; Gilon, C. Optimization of Chemical Processes by the Hydrodynamic Simulation Method (HSM). ChemEngineering 2018, 2, 21. https://doi.org/10.3390/chemengineering2020021
Bentolila M, Alshanski I, Novoa R, Gilon C. Optimization of Chemical Processes by the Hydrodynamic Simulation Method (HSM). ChemEngineering. 2018; 2(2):21. https://doi.org/10.3390/chemengineering2020021
Chicago/Turabian StyleBentolila, Moshe, Israel Alshanski, Roberto Novoa, and Chaim Gilon. 2018. "Optimization of Chemical Processes by the Hydrodynamic Simulation Method (HSM)" ChemEngineering 2, no. 2: 21. https://doi.org/10.3390/chemengineering2020021
APA StyleBentolila, M., Alshanski, I., Novoa, R., & Gilon, C. (2018). Optimization of Chemical Processes by the Hydrodynamic Simulation Method (HSM). ChemEngineering, 2(2), 21. https://doi.org/10.3390/chemengineering2020021




