Analysis of Cadmium Retention Mechanisms by a Smectite Clay in the Presence of Carbonates
Abstract
1. Introduction
2. Materials and Methods
2.1. Smectite Clay
2.2. Techniques Used for Cd Measurements
2.3. Sorption Tests
2.4. Sorption Modelling
3. Results
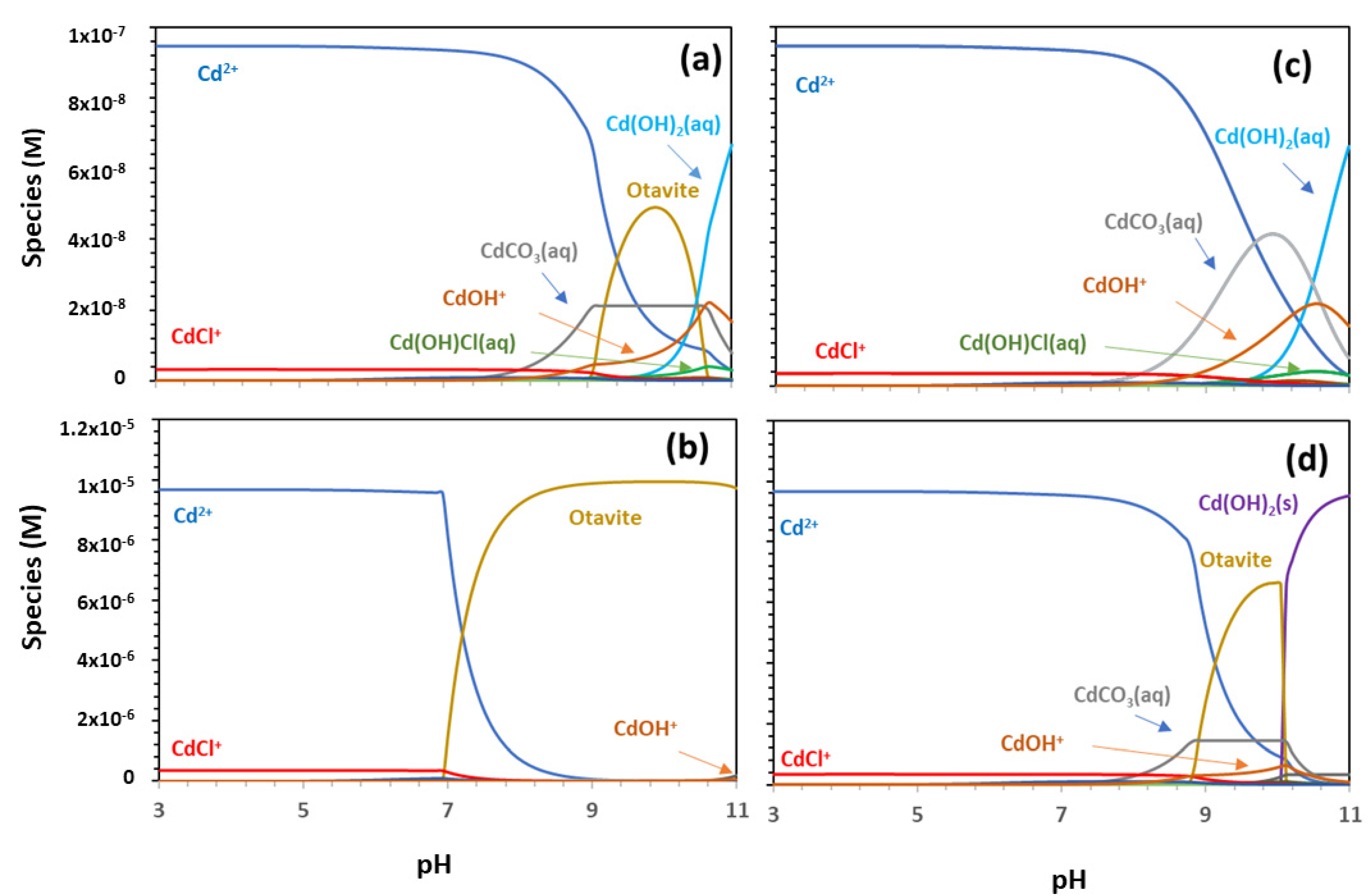
3.1. Cd Speciation
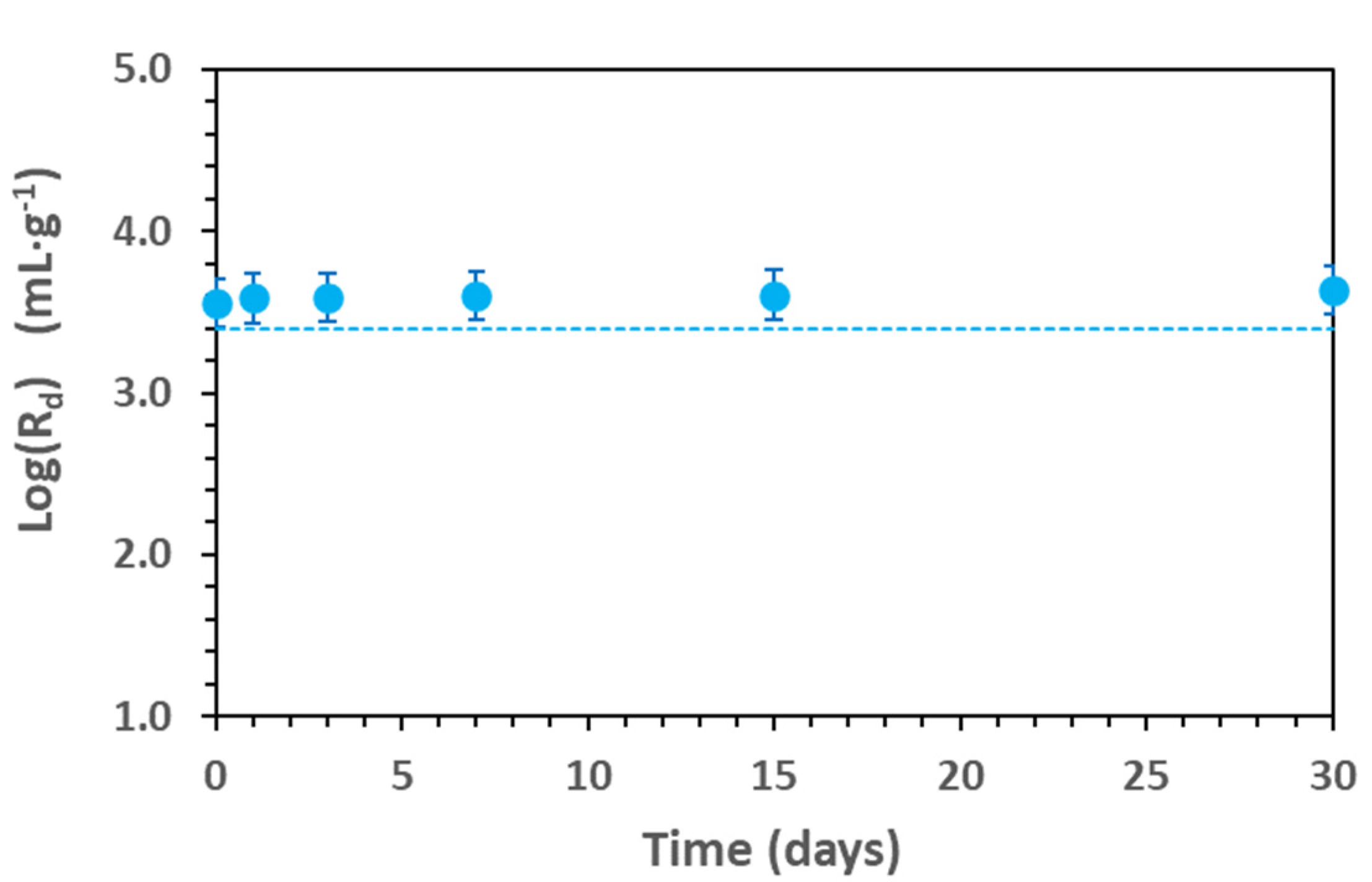
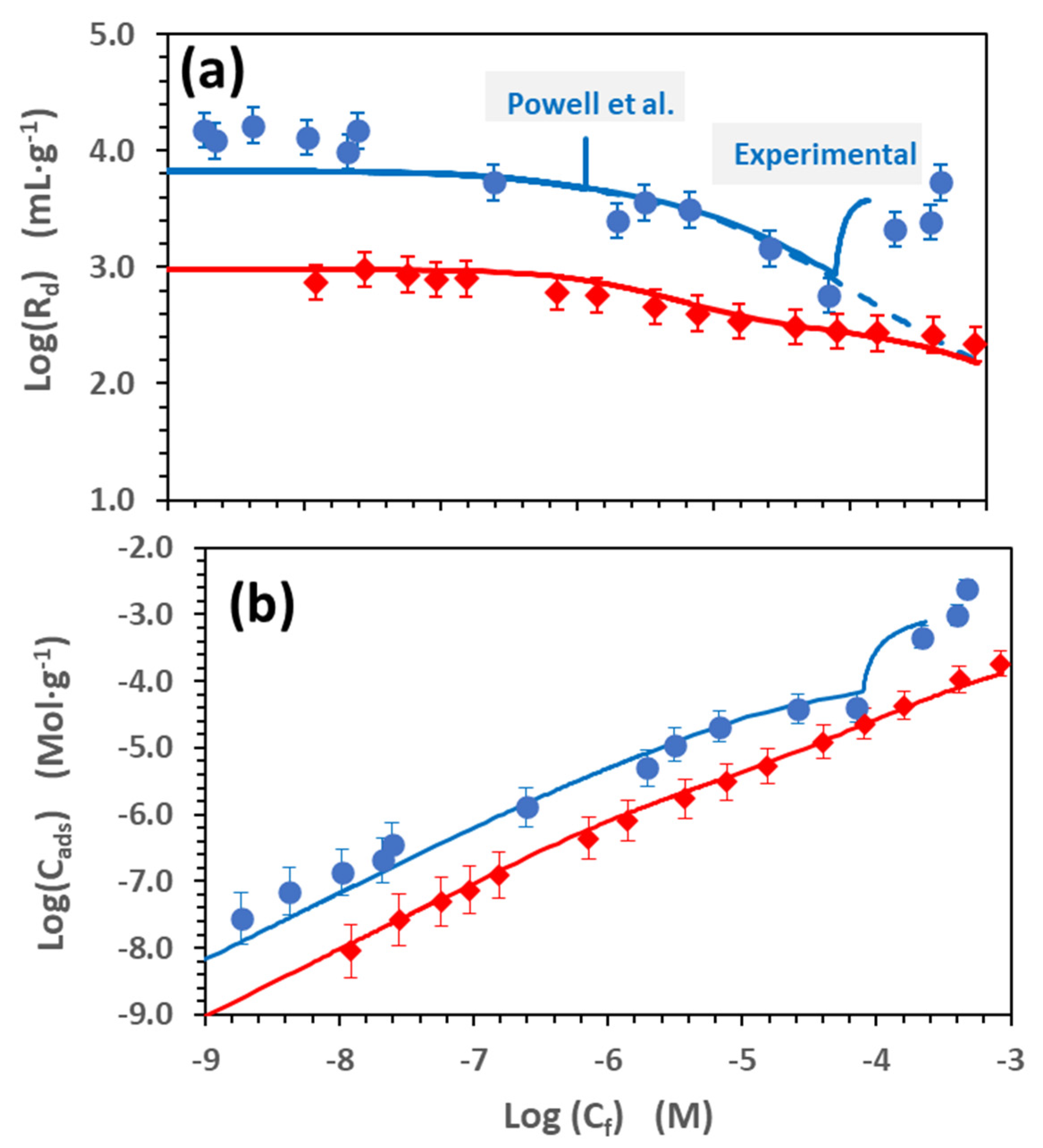
3.2. Sorption Tests
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- WHO. Exposure to Cadmium: A Major Public Health Concern; WHO/CED PHE/EPE/19.4.3.; World Health Organization: Geneva, Switzerland, 2019; Available online: https://www.who.int/publications/i/item/WHO-CED-PHE-EPE-19-4-3 (accessed on 22 December 2022).
- Sigel, A.; Sigel, H.; Sigel, R.K.O. (Eds.) Cadmium: From Toxicity to Essentiality Metal Ions; Life Sciences Series; Springer: Cham, Switzerland, 2013; Volume 11, 560p, ISBN 978-94-007-5178-1. [Google Scholar]
- Bouida, L.; Rafatullah, M.; Kerrouche, A.; Qutob, M.; Alosaimi, A.M.; Alorfi, H.S.; Hussein, M.A. A Review on Cadmium and lead Contamination: Sources, Fate, Mechanism, Health Effects and Remediation Methods. Water 2022, 14, 3432. [Google Scholar] [CrossRef]
- Rajendran, S.; Priya, T.A.K.; Khoo, K.S.; Hoang, T.K.A.; Ng, H.S.; Munawaroh, H.S.H.; Karaman, C.; Orooji, Y.; Sho, W.P.L. A critical review on various remediation approaches for heavy metal contaminants removal from contaminated soils. Chemosphere 2022, 287, 132369. [Google Scholar] [CrossRef] [PubMed]
- Bradl, H.B. Adsorption of heavy metals on soils and soils constituent. J. Colloids Interface Sci. 2004, 277, 1–18. [Google Scholar] [CrossRef] [PubMed]
- Sparks, D.L. Toxic metals in the environment: The role of surfaces. Elements 2005, 1, 193–197. [Google Scholar] [CrossRef]
- Gupta, S.S.; Bhattacharyya, K.G. Kinetics of adsorption of metal ions on inorganic materials: A review. Adv. Colloid Interface Sci. 2011, 162, 39–58. [Google Scholar] [CrossRef]
- Bhattacharyya, K.G.; Gupta, S.S. Adsorption of a few metals on natural and modified kaolinite and montmorillonite: A review. Adv. Colloid Interface Sci. 2008, 140, 114–131. [Google Scholar] [CrossRef] [PubMed]
- Keshu-Rani, M.; Yadav, J.; Meenu-Chaudhary, S.; Shanker, U. An updated review on synthetic approaches of green nanomaterials and their application for removal of water pollutants: Current challenges, assessment and future perspectives. J. Environ. Chem. Eng. 2021, 9, 106763. [Google Scholar] [CrossRef]
- Chen, G.; Shah, K.J.; Shi, L.; Chiang, P.-C. Removal of Cd(II) and Pb(II) ions from aqueous solutions by synthetic mineral adsorbent: Performance and mechanisms. Appl. Surf. Sci. 2017, 409, 296–305. [Google Scholar] [CrossRef]
- Ghorbel-Abid, I.; Galai, K.; Trabelsi-Ayadi, M. Retention of chromium(III) and cadmium(II) from aqueous solutions by illitic clay as low-cost adsorbent. Desalination 2010, 256, 190–195. [Google Scholar] [CrossRef]
- Wang, N.; Du, H.; Huang, Q.; Cai, P.; Rong, X.; Feng, X.; Chen, W. Surface complexation modelling of Cd(II) sorption onto montmorillonite, bacteria and their composite. Biogeoscience 2016, 13, 5557–5566. [Google Scholar] [CrossRef]
- Bedoui, K.; Bekri-Abbes, I.; Srasra, E. Removal of cadmium(II) from aqueous solution using pure smectite and lewatite S 100: The effect of time and metal concentration. Desalination 2008, 223, 269–273. [Google Scholar] [CrossRef]
- Choi, J. Geochemical modeling of cadmium sorption to soil as a function of soil properties. Chemosphere 2006, 63, 1824–1834. [Google Scholar] [CrossRef] [PubMed]
- Baker, H.M. A study of the binding strength and thermodynamic aspects of cadmium and lead ions with natural silicate minerals in aqueous solutions. Desalination 2009, 242, 115–127. [Google Scholar] [CrossRef]
- Rangel-Porras, G.; García-Magno, J.B.; Gonzalez Muñoz, M.P. Lead and cadmium immobilization on calcitic limestone materials. Desalination 2010, 262, 1–10. [Google Scholar] [CrossRef]
- Mobasherpour, I.; Salahi, E.; Pazouki, M. Removal of divalent cadmium cations by means of synthetic nano crystalline hydroxyapatite. Desalination 2011, 266, 142–148. [Google Scholar] [CrossRef]
- Li, Y.; Liu, J.; Wang, Y.; Tang, X.; Xu, J.; Liu, X. Contribution of components in natural soil to Cd and Pb competitive adsorption: Semi-quantitative to quantitative análisis. J. Hazard. Mater. 2023, 441, 129883. [Google Scholar] [CrossRef]
- Norris, S. Clays in Natural and Engineered Barriers for Radioactive Waste Confinement: An introduction. Geol. Soc. Lond. Spec. Publ. 2014, 400, 1–5. [Google Scholar] [CrossRef]
- Cuevas, J.; Leguey, S.; Garralón, A.; Rodriguez Rastrero, M.; Procopio, J.R.; Sevilla, M.T.; Sanchez Jiménez, N.; Rodriguez Abad, R.; Garrido, A. Behaviour of kaolinite and illite based clays as landfill barriers. Appl. Clay Sci. 2009, 42, 497–509. [Google Scholar]
- Sellin, P.; Leupin, O.X. The use of clays as an engineered barrier in radioactive-waste management: A review. Clays Clay Miner. 2013, 61, 477–498. [Google Scholar] [CrossRef]
- Payne, T.E.; Brendler, V.; Ochs, M.; Baeyens, B.; Brown, P.L.; Davis, J.A.; Ekberg, C.; Kulik, D.A.; Lutzenkirchen, J.; Missana, T.; et al. Guidelines for thermodynamic sorption modelling in the context of radioactive waste disposal. Environ. Model. Softw. 2013, 42, 143–156. [Google Scholar] [CrossRef]
- Missana, T.; Alonso, U.; Garcia-Gutierrez, M. Evaluation of component additive modelling approach for europium adsorption on 2:1 clays: Experimental, thermodynamic databases, and models. Chemosphere 2021, 272, 129877. [Google Scholar] [CrossRef]
- González Costa, J.J.; Reigosa, M.J.; Matías, J.M.; Covelo, E.F. Soil Cd, Cr, Cu, Ni, Pb and Zn sorption and retention models using SVM: Variable selection and competitive model. Sci. Total Environ. 2017, 593–594, 508–522. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Zhang, C.; Jiang, W.; Li, X.; Dai, Y.; Jia, H. Understanding the sorption behaviors of heavy metal ions in the interlayer and nanopore of montmorillonite: A molecular dynamics study. J. Hazard. Mater. 2021, 416, 125976. [Google Scholar] [CrossRef] [PubMed]
- Fernandez, A.M.; Baeyens, B.; Bradbury, M.H.; Rivas, P. Analysis of the pore water chemical composition of a Spanish compacted bentonite used in an engineered barrier. Phys. Chem. Earth 2004, 29, 105–118. [Google Scholar] [CrossRef]
- Shiao, S.-Y.; Egozy, Y.; Meyer, R.E. Adsorption of Cs(I), Sr(II), Eu(III), Co(II) and Cd(II) by Al2O3. J. Inorg. Nucl. Chem. 1981, 43, 3309–3315. [Google Scholar] [CrossRef]
- Boily, J.F.; Fein, J.B. Experimental study of cadmium-citrate co-adsorption onto gamma Al2O3. Geochim. Cosmochim. Acta 1996, 60, 2929–2938. [Google Scholar] [CrossRef]
- Crea, F.; Foti, C.; Milea, D.; Sammartano, S. Speciation of Cd in the Environment. In Cadmium: From Toxicity to Essentiality Metal Ions; Sigel, A., Sigel, H., Sigel, R.K.O., Eds.; Life Sciences Series 11; Springer: Chem, Switzerland, 2013; pp. 63–83. ISBN 978-94-007-5178-1. [Google Scholar]
- Undabeytia, T.; Nir, S.; Rytwo, G.; Morillo, E.; Maqueda, C. Modeling adsorption-desorption processes of Cd on montmorillonite. Clays Clay Miner. 1998, 46, 423–428. [Google Scholar] [CrossRef]
- Di Leo, P.; O’Brien, P. Nuclear Magnetic Resonance (NMR) study of Cd2+ sorption onto montmorillonite. Clays Clay Miner. 1999, 47, 761–768. [Google Scholar] [CrossRef]
- Saeki, K.; Kunito, T. Influence of chloride ions on cadmium adsorptions by oxides, hydroxides, oxyhydroxides, and phyllosilicates. Appl. Clay Sci. 2012, 62, 58–62. [Google Scholar] [CrossRef]
- Taylor, P.; Lumsdon, D.G.; Evans, L.J.; Bolton, K.A. The influence of pH and chloride on the retention of cadmium, lead, mercury, and zinc by soils. Soil Sediment Contam. 1995, 4, 137–150. [Google Scholar]
- El-Hefnawy, M.E.; Selim, E.M.; Assaad, F.F.; Ismail, A.I. The effect of chloride and sulfate ions on the adsorption of Cd2+ on clay and sandy loam egyptian soils. Sci. World J. 2014, 2014, 806252. [Google Scholar] [CrossRef] [PubMed]
- Huertas, F.; Fuentes-Santillana, J.L.; Jullien, F.; Rivas, P.; Linares, J.; Fariña, P.; Ghoreychi, M.; Jockwer, N.; Kickmaier, W.; Martínez, M.A.; et al. Full scale engineered barriers experiment for a deep geological repository for high-level radioactive waste in crystalline host rock. In EC Final REPORT EUR 2000; EU Publications: Luxembourg, 2000; p. 19147. [Google Scholar]
- Fernandez, A.M.; Kaufhold, S.; Sanchez-Ledesma, D.M.; Rey, J.J.; Melon, A.; Robredo, L.M.; Fernandez, S.; Labajo, M.A.; Clavero, M.A. Evolution of the THC conditions in the FEBEX in situ test after 18 years of experiment: Smectite crystallochemical modifications after interactions of the bentonite with a C-steel heater at 100 °C. Appl. Geochem. 2018, 98, 152–171. [Google Scholar] [CrossRef]
- Powell, K.J.; Brown, P.L.; Byrne, R.H.; Gajda, T.; Hefter, G.; Sjöberg, S.; Wanner, H. Chemical speciation of environmentally significant metals with inorganic ligands Part 4: The Cd2+ + OH−, Cl−, CO32−, SO42− and PO43− systems. Int. Union Pure Appl. Chem. 2011, 83, 1163–1214. [Google Scholar] [CrossRef]
- Delany, J.M.; Lundeen, S.R. The LLNL Thermochemical Data Base–Revised Data and File Format for the EQ3/6 Package; Lawrence Livermore National Lab: Livermore, CA, USA, 1991. [Google Scholar]
- Van der Lee, J.; De Windt, L. CHESS Tutorial and Cookbook; Technical Report LHM/RD/99/05; École des Mines de Paris: Fontainebleau, France, 1999. [Google Scholar]
- Missana, T.; Garcia-Gutierrez, M.; Alonso, U. Analysis and Modelling of Sorption Processes in Complex Materials; Colección Documentos CIEMAT: Madrid, Spain, 2019; ISBN 978-84-7834-819-0. [Google Scholar]
- Gaines, G.L.; Thomas, H.C. Adsorption studies on clay minerals. II. A formulation of the thermodynamics of exchange adsorption. J. Chem. Phys 1953, 21, 714–718. [Google Scholar] [CrossRef]
- Bradbury, M.H.; Baeyens, B. Sorption by Cation Exchange: Incorporation of a Cation Exchange Model into Geochemical Computer Codes; Technical Report Bericht 94-07; PSI: Villigen, Switzerland, 1994. [Google Scholar]
- Gamsjäger, H.; Magalhães, M.C.F.; Königsberger, E.; Sawada, K.; Churagulov, B.R.; Schmidt, P.; Zeng, D. IUPAC-NIST Solubility Data Series. 92. Metal Carbonates. Part 1. Solubility and Related Thermodynamic Quantities of Cadmium(II) Carbonate in Aqueous Systems. J. Phys. Chem. Ref. Data 2011, 40, 043104. [Google Scholar]
- Stipp, S.L.; Parks, G.A.; Nordstrom, D.K.; Leckie, J.O. Solubility-product constant and thermodynamic properties for synthetic otavite, CdCO3(s), and aqueous association constants for the Cd(II)-CO2-H2O system. Geochim. Cosmochim. Acta 1993, 57, 2699–2113. [Google Scholar] [CrossRef]
- Missana, T.; García-Gutiérrez, M. Adsorption of bivalent ions (Ca(II), Sr(II) and Co(II)) onto FEBEX bentonite. Phys. Chem. Earth 2007, 32, 559–567. [Google Scholar] [CrossRef]
- Mayordomo, N.; Alonso, U.; Missana, T. Effects of γ-alumina nanoparticles on strontium sorption in smectite: Additive model approach. Appl. Geochem. 2019, 100, 121–130. [Google Scholar] [CrossRef]
- Missana, T.; Benedicto, A.; García-Gutierrez, M.; Alonso, U. Modeling cesium retention onto Na-, Ca- and K-smectite: Effects of ionic strength, exchange and competing ions on the determination of selectivity coefficients. Geochem. Cosmochim. Acta 2014, 128, 266–277. [Google Scholar] [CrossRef]
- Missana, T.; Alonso, U.; Garcia-Gutierrez, M.; Albarran, N.; Lopez, T. Experimental Study and Modeling of Uranium (VI) Sorption onto a Spanish Smectite. In Scientific Basis for Nuclear Waste Management XXXII; Cambridge University Press: Cambridge, UK, 2009; Volume 1124, pp. 561–566. [Google Scholar]
- Gunneriusson, L. Composition and stabilityof Cd(II)-Chloro and Hydroxo Complexes at the goethite (α-FeOOH)/water interface. J. Colloid Interface Sci. 1994, 163, 484–492. [Google Scholar] [CrossRef]


| Aqueous Species | Definition | LogK |
|---|---|---|
| CdOH [+] | 1 Cd [2+], 1 H2O, -1 H[+] | −9.91 ± 0.1 |
| Cd(OH)2(aq) | 1 Cd [2+], 2 H2O, -2 H[+] | −20.19 ± 0.13 |
| Cd(OH)3[−] | 1 Cd [2+], 3 H2O, -3 H[+] | −33.5 ± 0.5 |
| Cd(OH)4[2−] | 1 Cd [2+], 4 H2O, -4 H[+] | −47.28 ± 0.15 |
| Cd2OH [3+] | 2 Cd [2+], 1 H2O, -1 H[+] | −8.73 ± 0.01 |
| Cd4(OH)4[4+] | 4 Cd [2+], 4 H2O, -4 H[+] | −31.8 |
| CdHCO3[+] | 1 Cd [2+], 1 HCO3[−] | 0.84–2.4/1.5 (*) |
| CdCO3(aq) | 1 Cd [2+], 1 HCO3[−], -1 H[+] | −5.9288 |
| Cd(CO3)2[2−] | 1 Cd [2+], 2 HCO3[−], -2H[+] | −14.4576 |
| CdCl[+] | 1 Cd [2+], 1 Cl[−] | 1.98 ± 0.06 |
| CdCl2(aq) | 1 Cd [2+], 2 Cl[−] | 2.64 ± 0.09 |
| CdCl3[−] | 1 Cd [2+], 3 Cl[−] | 2.3 ± 0.21 |
| Cd(OH)Cl(aq) | 1 Cd [2+], 1 Cl[−], 1 H2O, -1 H[+] | 7.4328 (*) |
| Solid Species | Composition | LogK |
| Cd(OH)2 | 1 Cd [2+], -2 H[+], 2 H2O | −13.72 ± 0.12 |
| CdCO3(s), otavite (**) | 1 Cd [2+], 1 HCO3[−], -1 H[+] | 1.7336 |
| Cd(OH)Cl | 1 Cd [2+], 1 Cl[−], -1 H[+], 1H2O | −3.543 |
| CdCl2 | 1 Cd [2+], 2 Cl[−] | 0.674 (*) |
| CdCl2:H2O | 1 Cd [2+], 2 Cl[−], 1 H2O | 1.6747 (*) |
| SPECIES | DEFINITION | LogK |
|---|---|---|
| SwOH2[+] | 1 SwOH, 1 H[+] | 5.3 |
| SsOH2[+] | 1 SsOH, 1 H[+] | 4.8 |
| SwO [−] | 1 SwOH, -1 H[+] | −8.4 |
| SsO [−] | 1 SsOH, -1 H[+] | −9.9 |
| SPECIES | DEFINITION | Mean LogK |
|---|---|---|
| X2Cd | 2 X-Na, 1 Cd [2+], -2 Na[+] | 3.5 |
| SwOCd[+] | 1 SwOH, -1 H[+], 1 Cd [2+] | −2.51 |
| SsOCd[+] | 1 SsOH, -1 H[+], 1 Cd [2+] | −1.4 |
| SwOHCd [2+] | 1 SwOH, 1 Cd [2+] | 4.14 |
| SsOHCd [2+] | 1 SsOH, 1 Cd [2+] | 6.1 |
| SsOCdOH | 1 SwOH, -2 H[+], 1 Cd [2+], 1 H2O | −11.66 |
| SwOCdOH | 1 SsOH, -2 H[+], 1 Cd [2+], 1 H2O | −11.86 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Missana, T.; Alonso, U.; Mayordomo, N.; García-Gutiérrez, M. Analysis of Cadmium Retention Mechanisms by a Smectite Clay in the Presence of Carbonates. Toxics 2023, 11, 130. https://doi.org/10.3390/toxics11020130
Missana T, Alonso U, Mayordomo N, García-Gutiérrez M. Analysis of Cadmium Retention Mechanisms by a Smectite Clay in the Presence of Carbonates. Toxics. 2023; 11(2):130. https://doi.org/10.3390/toxics11020130
Chicago/Turabian StyleMissana, Tiziana, Ursula Alonso, Natalia Mayordomo, and Miguel García-Gutiérrez. 2023. "Analysis of Cadmium Retention Mechanisms by a Smectite Clay in the Presence of Carbonates" Toxics 11, no. 2: 130. https://doi.org/10.3390/toxics11020130
APA StyleMissana, T., Alonso, U., Mayordomo, N., & García-Gutiérrez, M. (2023). Analysis of Cadmium Retention Mechanisms by a Smectite Clay in the Presence of Carbonates. Toxics, 11(2), 130. https://doi.org/10.3390/toxics11020130






