Quantitative Analysis of Molecular Mobility in Amorphous Lactose Above Tg: A Novel Insight from Molecular Dynamic Simulation to Strength Parameter
Abstract
1. Introduction
2. Materials and Methods
2.1. MD Simulation
2.2. Mm Measurement
2.3. Mobility Coefficient Calculation
2.4. Activation Energy Calculation
2.5. Strength Parameter Measurement
2.6. Statistical Analysis
3. Results
3.1. Mobility Trajectories
3.2. Mobility Coefficients and Activation Energy
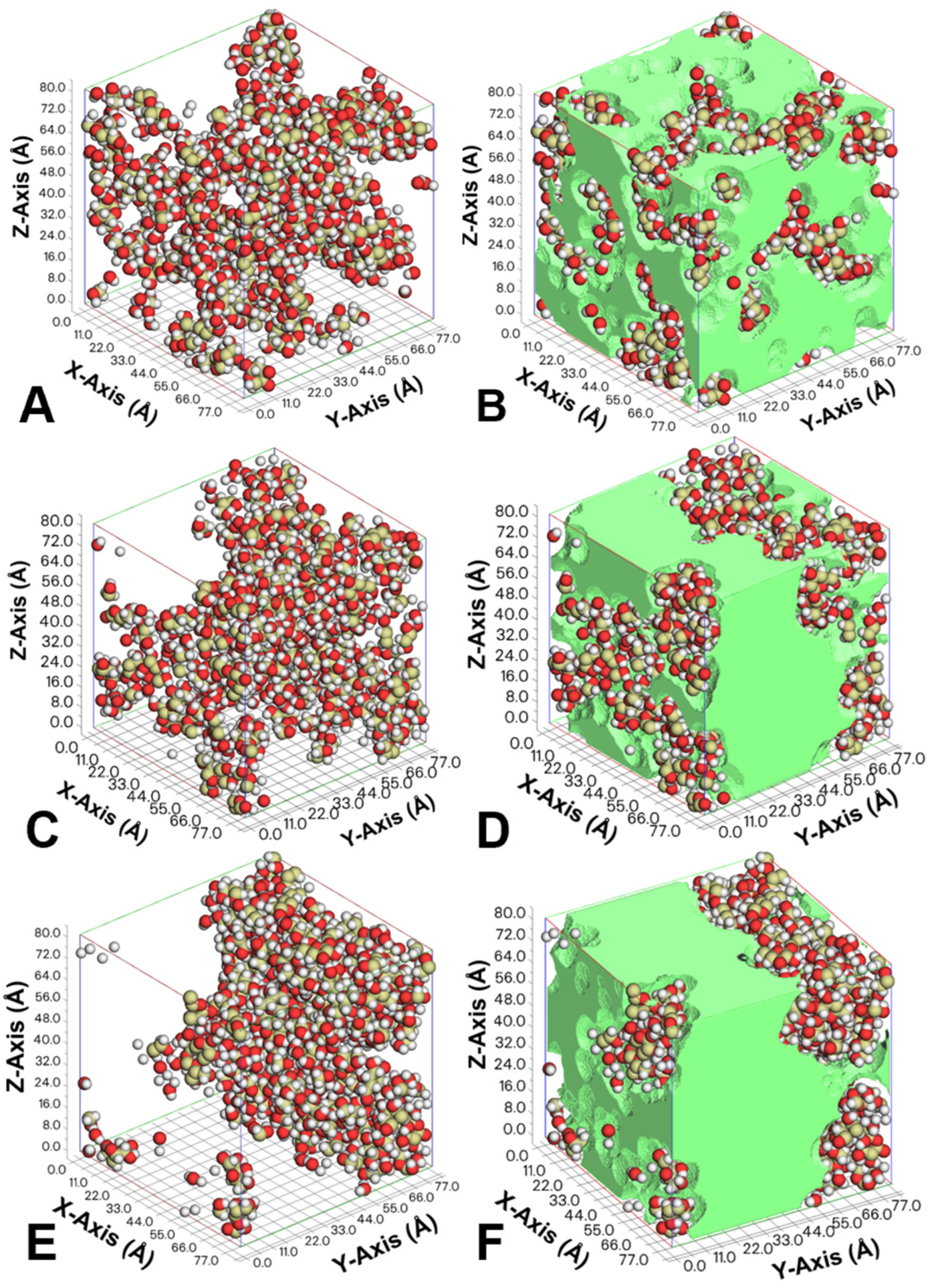
3.3. Free Volume in Cells
3.4. S Parameter and Mm
3.5. Lactose Mobility Pathways
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Aganovic, K.; Hertel, C.; Rudi, F.V.; Johne, R.; Schlüter, O.; Schwarzenbolz, U.; Jäger, H.; Holzhauser, T.; Bergmair, J.; Roth, A.; et al. Aspects of High Hydrostatic Pressure Food Processing: Perspectives on Technology and Food Safety. Compr. Rev. Food Sci. Food Saf. 2021, 20, 3225–3266. [Google Scholar] [CrossRef]
- Garcia, M.A.V.T.; Garcia, C.F.; Faraco, A.A.G. Pharmaceutical and Biomedical Applications of Native and Modified Starch: A Review. Starch—Stärke 2020, 72, 1900270. [Google Scholar] [CrossRef]
- Rahman, M.S.; Roos, Y.H. Glass Transition and Phase Transitions in Food and Biological Materials; Ahmed, J., Ed.; Wiley: Hoboken, NJ, USA, 2017. [Google Scholar]
- Fan, F.; Roos, Y.H. Structural Relaxations of Amorphous Lactose and Lactose-Whey Protein Mixtures. J. Food Eng. 2016, 173, 106–115. [Google Scholar] [CrossRef]
- Penkov, N.V. Relationships between Molecular Structure of Carbohydrates and Their Dynamic Hydration Shells Revealed by Terahertz Time-Domain Spectroscopy. Int. J. Mol. Sci. 2021, 22, 11969. [Google Scholar] [CrossRef]
- Roudaut, G.; Simatos, D.; Champion, D.; Contreras-Lopez, E.; Le Meste, M. Molecular Mobility around the Glass Transition Temperature: A Mini Review. Innov. Food Sci. Emerg. Technol. 2004, 5, 127–134. [Google Scholar] [CrossRef]
- Champion, D.; Le Meste, M.; Simatos, D. Towards an Improved Understanding of Glass Transition and Relaxations in Foods: Molecular Mobility in the Glass Transition Range. Trends Food Sci. Technol. 2000, 11, 41–55. [Google Scholar] [CrossRef]
- Fan, F.; Xiang, P.; Zhao, L. Vibrational Spectra Analysis of Amorphous Lactose in Structural Transformation: Water/Temperature Plasticization, Crystal Formation, and Molecular Mobility. Food Chem. 2021, 341, 128215. [Google Scholar] [CrossRef]
- Tiwari, R.; Ludescher, R.D. Molecular Mobility in a Homologous Series of Amorphous Solid Glucose Oligomers. Food Chem. 2012, 132, 1814–1821. [Google Scholar] [CrossRef]
- Slade, L.; Levine, H.; Reid, D.S. Beyond Water Activity: Recent Advances Based on an Alternative Approach to the Assessment of Food Quality and Safety. Crit. Rev. Food Sci. Nutr. 1991, 30, 115–360. [Google Scholar] [CrossRef]
- Fan, F.; Roos, Y.H. Glass Transition-Associated Structural Relaxations and Applications of Relaxation Times in Amorphous Food Solids: A Review. Food Eng. Rev. 2017, 9, 257–270. [Google Scholar] [CrossRef]
- Maidannyk, V.A.; Roos, Y.H. Modification of the WLF Model for Characterization of the Relaxation Time-Temperature Relationship in Trehalose-Whey Protein Isolate Systems. J. Food Eng. 2016, 188, 21–31. [Google Scholar] [CrossRef]
- Cavallo, V.; Roggero, A.; Fina, A.; Gerard, J.-F.; Pruvost, S. P(MMA-Co-MAA)/Cellulose Nanofibers Composites: Effect of Hydrogen Bonds on Molecular Mobility. Carbohydr. Polym. 2024, 346, 122579. [Google Scholar] [CrossRef] [PubMed]
- Brunk, E.; Rothlisberger, U. Mixed Quantum Mechanical/Molecular Mechanical Molecular Dynamics Simulations of Biological Systems in Ground and Electronically Excited States. Chem. Rev. 2015, 115, 6217–6263. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.-W.; Li, B.; Lin, Q.-B.; Hu, C.-Y. Two-Phase Molecular Dynamics Model to Simulate the Migration of Additives from Polypropylene Material to Food. Int. J. Heat Mass Transf. 2018, 122, 694–706. [Google Scholar] [CrossRef]
- Fan, F.; Roos, Y.H. Structural Strength and Crystallization of Amorphous Lactose in Food Model Solids at Various Water Activities. Innov. Food Sci. Emerg. Technol. 2017, 40, 27–34. [Google Scholar] [CrossRef]
- Carpin, M.; Bertelsen, H.; Bech, J.K.; Jeantet, R.; Risbo, J.; Schuck, P. Caking of Lactose: A Critical Review. Trends Food Sci. Technol. 2016, 53, 1–12. [Google Scholar] [CrossRef]
- Huang, Z.; Li, K.; Ma, L.; Chen, F.; Liao, X.; Hu, X.; Ji, J. Flavor Release from Lactose/Protein Matrix during Storage: Effects of Lactose Crystallization and Powder Microstructure. LWT 2021, 141, 110857. [Google Scholar] [CrossRef]
- Eslami, H.; Materzok, T.; Müller-Plathe, F. Molecular Structure and Dynamics in Wet Gecko β-Keratin. ACS Biomater. Sci. Eng. 2023, 9, 257–268. [Google Scholar] [CrossRef]
- Lu, T.; Chen, F. Multiwfn: A Multifunctional Wavefunction Analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef]
- Lu, T. A Comprehensive Electron Wavefunction Analysis Toolbox for Chemists, Multiwfn. J. Chem. Phys. 2024, 161, 082503. [Google Scholar] [CrossRef]
- Karuth, A.; Alesadi, A.; Xia, W.; Rasulev, B. Predicting Glass Transition of Amorphous Polymers by Application of Cheminformatics and Molecular Dynamics Simulations. Polymer 2021, 218, 123495. [Google Scholar] [CrossRef]
- Cui, T.; Wu, X.; Mou, T.; Fan, F. Water Usability as a Descriptive Parameter of Thermodynamic Properties and Water Mobility in Food Solids. NPJ Sci. Food 2023, 7, 30. [Google Scholar] [CrossRef] [PubMed]
- Roe, D.R.; Cheatham, T.E. PTRAJ and CPPTRAJ: Software for Processing and Analysis of Molecular Dynamics Trajectory Data. J. Chem. Theory Comput. 2013, 9, 3084–3095. [Google Scholar] [CrossRef] [PubMed]
- Kumar, M.; Madhumita, M.; Prabhakar, P.K.; Basu, S. Refractance Window Drying of Food and Biological Materials: Status on Mechanisms, Diffusion Modelling and Hybrid Drying Approach. Crit. Rev. Food Sci. Nutr. 2024, 64, 3458–3481. [Google Scholar] [CrossRef]
- Pittia, P.; Sacchetti, G. Antiplasticization Effect of Water in Amorphous Foods. A Review. Food Chem. 2008, 106, 1417–1427. [Google Scholar] [CrossRef]
- Hourigan, J.A.; Lifran, E.V.; Vu, L.T.T.; Listiohadi, Y.; Sleigh, R.W. Lactose: Chemistry, Processing, and Utilization. In Advances in Dairy Ingredients; Wiley: Hoboken, NJ, USA, 2013; pp. 21–41. [Google Scholar]
- Bliznyuk, V.N.; Assender, H.E.; Briggs, G.A.D. Surface Glass Transition Temperature of Amorphous Polymers. A New Insight with SFM. Macromolecules 2002, 35, 6613–6622. [Google Scholar] [CrossRef]
- Barnett, A.; Karnes, J.J.; Lu, J.; Major, D.R.; Oakdale, J.S.; Grew, K.N.; McClure, J.P.; Molinero, V. Exponential Water Uptake in Ionomer Membranes Results from Polymer Plasticization. Macromolecules 2022, 55, 6762–6774. [Google Scholar] [CrossRef]
- Eslami, H.; Müller-Plathe, F. Self-Assembly Pathways of Triblock Janus Particles into 3D Open Lattices. Small 2024, 20, 2306337. [Google Scholar] [CrossRef]
- Nakamura, T.; Ishiyama, T. Molecular Dynamics Study of Hydrogen Bond Structure and Tensile Strength for Hydrated Amorphous Cellulose. Biomacromolecules 2024, 25, 7249–7259. [Google Scholar] [CrossRef]
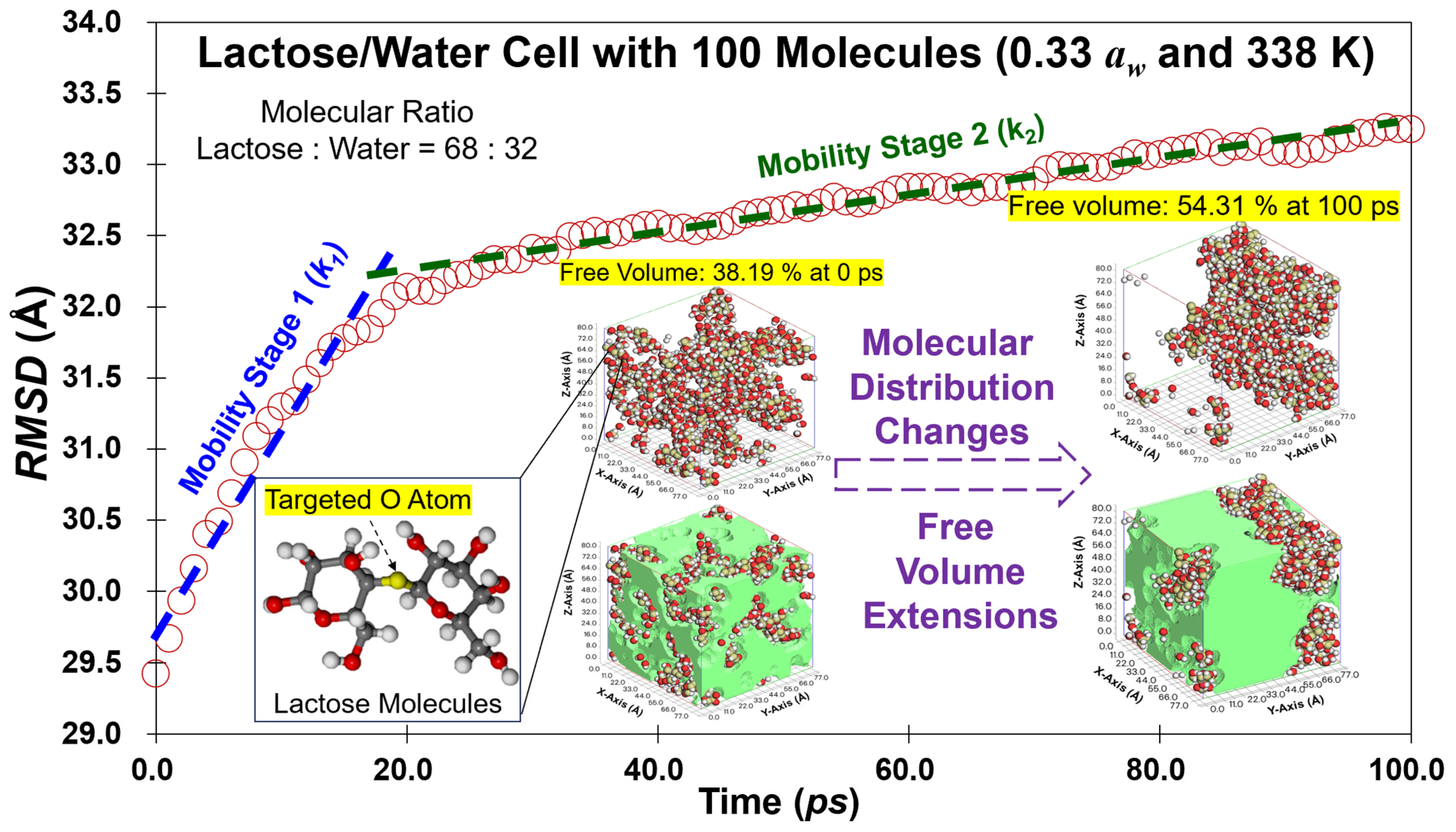


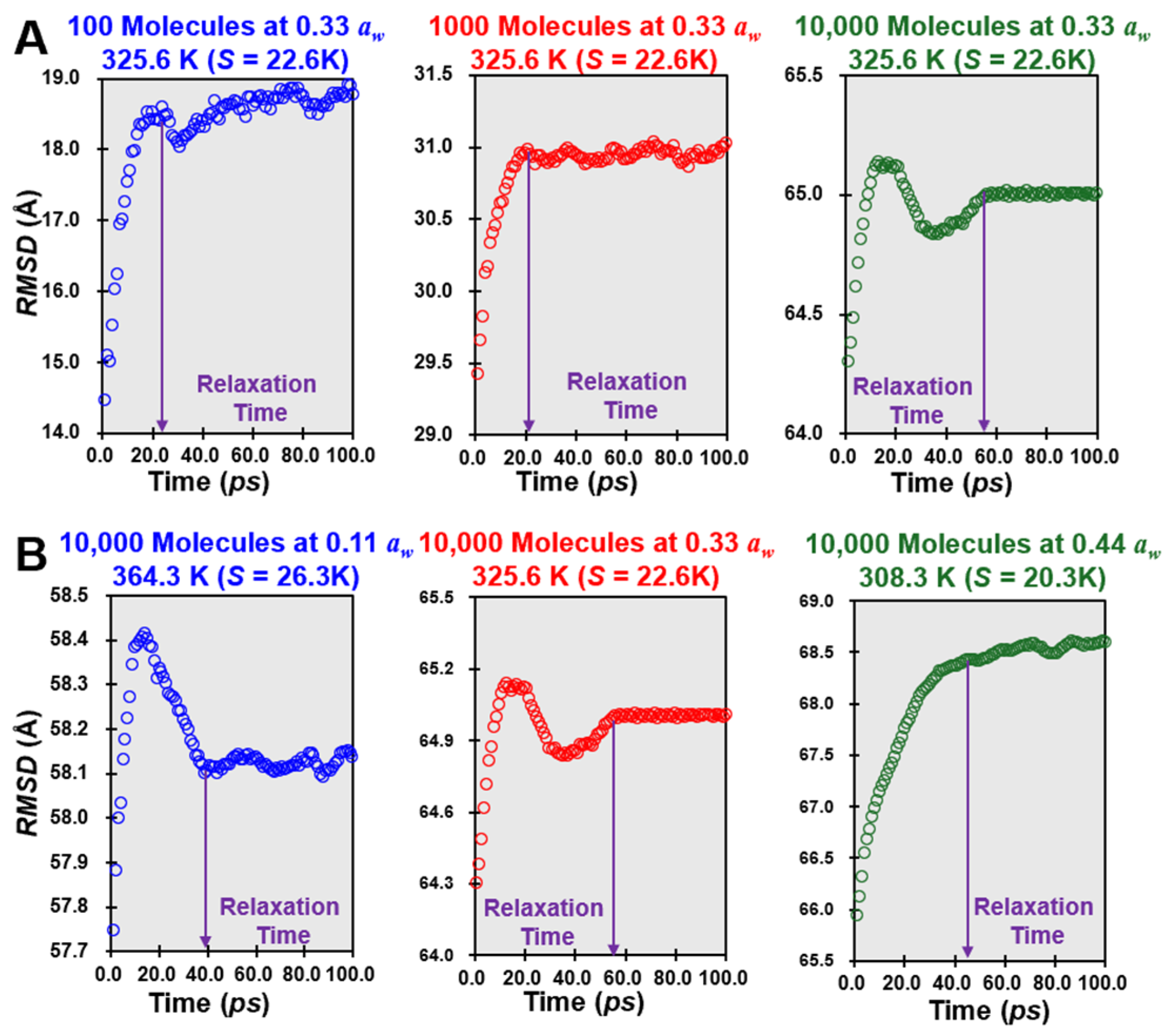

| Simulated aw | Ratio (L:W) | T − Tg (K) | k1 | R12 | k2 | Dm1 (Å/ps) | Dm2 (Å/ps) | Ea1 (kJ/mol) | Ea2 (kJ/mol) |
|---|---|---|---|---|---|---|---|---|---|
| 0.11 | 68:32 | 0 | −0.2432 | 0.9589 | 0.0118 | 0.0405 | 0.0002 | 10.9878 | 12.8061 |
| 10 | −0.2931 | 0.9618 | 0.0098 | 0.0489 | 0.0016 | ||||
| 20 | −0.3219 | 0.9527 | 0.0061 | 0.0537 | 0.0010 | ||||
| 30 | −0.3371 | 0.9691 | 0.0011 | 0.0562 | 0.0002 | ||||
| 0.22 | 57:43 | 0 | −0.1687 | 0.9846 | 0.0027 | 0.0298 | 0.0005 | 8.3431 | 10.1664 |
| 10 | −0.1090 | 0.8231 | 0.0055 | 0.0317 | 0.0009 | ||||
| 20 | −0.4255 | 0.9885 | 0.0013 | 0.0350 | 0.0005 | ||||
| 30 | −0.2963 | 0.9864 | 0.0038 | 0.0394 | 0.0011 | ||||
| 0.33 | 52:48 | 0 | −0.1066 | 0.8014 | 0.0041 | 0.0282 | 0.0003 | 8.1669 | 12.7744 |
| 10 | −0.1861 | 0.8166 | 0.0005 | 0.0294 | 0.0011 | ||||
| 20 | −0.1910 | 0.9586 | 0.0013 | 0.0335 | 0.0006 | ||||
| 30 | −0.2386 | 0.9319 | 0.0038 | 0.0364 | 0.0013 | ||||
| 0.44 | 38:62 | 0 | −0.1742 | 0.9536 | 0.0007 | 0.0274 | 0.00003 | 6.9418 | 15.3543 |
| 10 | −0.1739 | 0.9472 | 0.0055 | 0.0290 | 0.00008 | ||||
| 20 | −0.1499 | 0.9396 | 0.0013 | 0.0317 | 0.00005 | ||||
| 30 | −0.2963 | 0.9324 | 0.0038 | 0.0361 | 0.00003 |
| Mimic Water Activities (aw) | Simulant Cell Size (Ångstrom) | Cell Density (g/cm3) | Temperature (K) | Time (ps) | Free Volume (Ångstrom3) | Percentage (%) |
|---|---|---|---|---|---|---|
| 0.11 | 42.9 × 42.9 × 42.9 | 0.5 | 338 | 0 | 32,200.939 | 40.65 |
| 20 | 42,319.684 | 53.42 | ||||
| 100 | 44,176.873 | 55.77 | ||||
| 348 | 0 | 32,067.512 | 40.48 | |||
| 20 | 41,528.707 | 52.42 | ||||
| 100 | 44,364.481 | 56.00 | ||||
| 358 | 0 | 32,067.512 | 40.48 | |||
| 20 | 40,812.000 | 51.52 | ||||
| 100 | 44,188.707 | 55.78 | ||||
| 368 | 0 | 32,067.512 | 40.48 | |||
| 20 | 42,010.641 | 53.03 | ||||
| 100 | 44,653.483 | 56.37 | ||||
| 0.22 | 40.7 × 40.7 × 40.7 | 0.5 | 313 | 0 | 27,575.943 | 40.93 |
| 20 | 33,886.857 | 50.30 | ||||
| 100 | 36,417.298 | 54.06 | ||||
| 323 | 0 | 27,575.943 | 40.93 | |||
| 20 | 35,110.157 | 52.12 | ||||
| 100 | 36,336.005 | 53.98 | ||||
| 333 | 0 | 27,575.943 | 40.93 | |||
| 20 | 34,992.405 | 51.94 | ||||
| 100 | 37,323.439 | 55.40 | ||||
| 343 | 0 | 27,575.943 | 40.93 | |||
| 20 | 35,689.584 | 52.98 | ||||
| 100 | 36,637.622 | 54.38 | ||||
| 0.33 | 39.6 × 39.6 × 39.6 | 0.5 | 303 | 0 | 25,547.001 | 41.21 |
| 20 | 31,309.400 | 50.51 | ||||
| 100 | 33,234.957 | 53.62 | ||||
| 313 | 0 | 25,547.001 | 41.21 | |||
| 20 | 32,613.698 | 52.62 | ||||
| 100 | 33,937.488 | 54.75 | ||||
| 323 | 0 | 25,547.001 | 41.21 | |||
| 20 | 31,759.772 | 51.24 | ||||
| 100 | 33,362.598 | 53.82 | ||||
| 333 | 0 | 25,547.001 | 41.21 | |||
| 20 | 32,519.532 | 52.46 | ||||
| 100 | 33,265.822 | 53.67 | ||||
| 0.44 | 36.0 × 36.0 × 36.0 | 0.5 | 286 | 0 | 18,752.591 | 39.98 |
| 20 | 24,228.779 | 51.65 | ||||
| 100 | 24,856.389 | 52.99 | ||||
| 296 | 0 | 18,752.591 | 39.98 | |||
| 20 | 23,792.236 | 50.72 | ||||
| 100 | 25,020.057 | 53.34 | ||||
| 306 | 0 | 18,752.591 | 39.98 | |||
| 20 | 24,456.596 | 52.14 | ||||
| 100 | 25,199.910 | 53.72 | ||||
| 316 | 0 | 18,752.591 | 39.98 | |||
| 20 | 24,338.806 | 51.89 | ||||
| 100 | 25,474.984 | 54.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, F.; Liu, H.; Xu, Y.; Mou, T. Quantitative Analysis of Molecular Mobility in Amorphous Lactose Above Tg: A Novel Insight from Molecular Dynamic Simulation to Strength Parameter. Foods 2025, 14, 928. https://doi.org/10.3390/foods14060928
Fan F, Liu H, Xu Y, Mou T. Quantitative Analysis of Molecular Mobility in Amorphous Lactose Above Tg: A Novel Insight from Molecular Dynamic Simulation to Strength Parameter. Foods. 2025; 14(6):928. https://doi.org/10.3390/foods14060928
Chicago/Turabian StyleFan, Fanghui, Huan Liu, Yier Xu, and Tian Mou. 2025. "Quantitative Analysis of Molecular Mobility in Amorphous Lactose Above Tg: A Novel Insight from Molecular Dynamic Simulation to Strength Parameter" Foods 14, no. 6: 928. https://doi.org/10.3390/foods14060928
APA StyleFan, F., Liu, H., Xu, Y., & Mou, T. (2025). Quantitative Analysis of Molecular Mobility in Amorphous Lactose Above Tg: A Novel Insight from Molecular Dynamic Simulation to Strength Parameter. Foods, 14(6), 928. https://doi.org/10.3390/foods14060928







