Effect of Reduced Atmosphere Sintering on Blocking Grain Boundaries in Rare-Earth Doped Ceria
Abstract
:1. Introduction
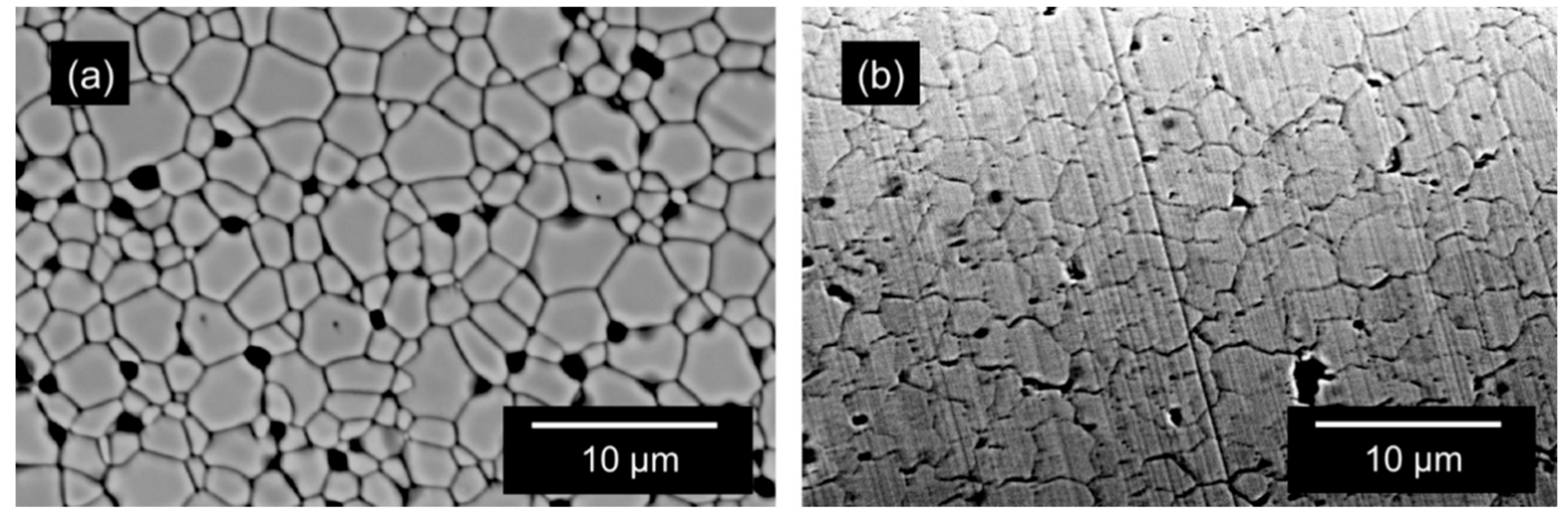
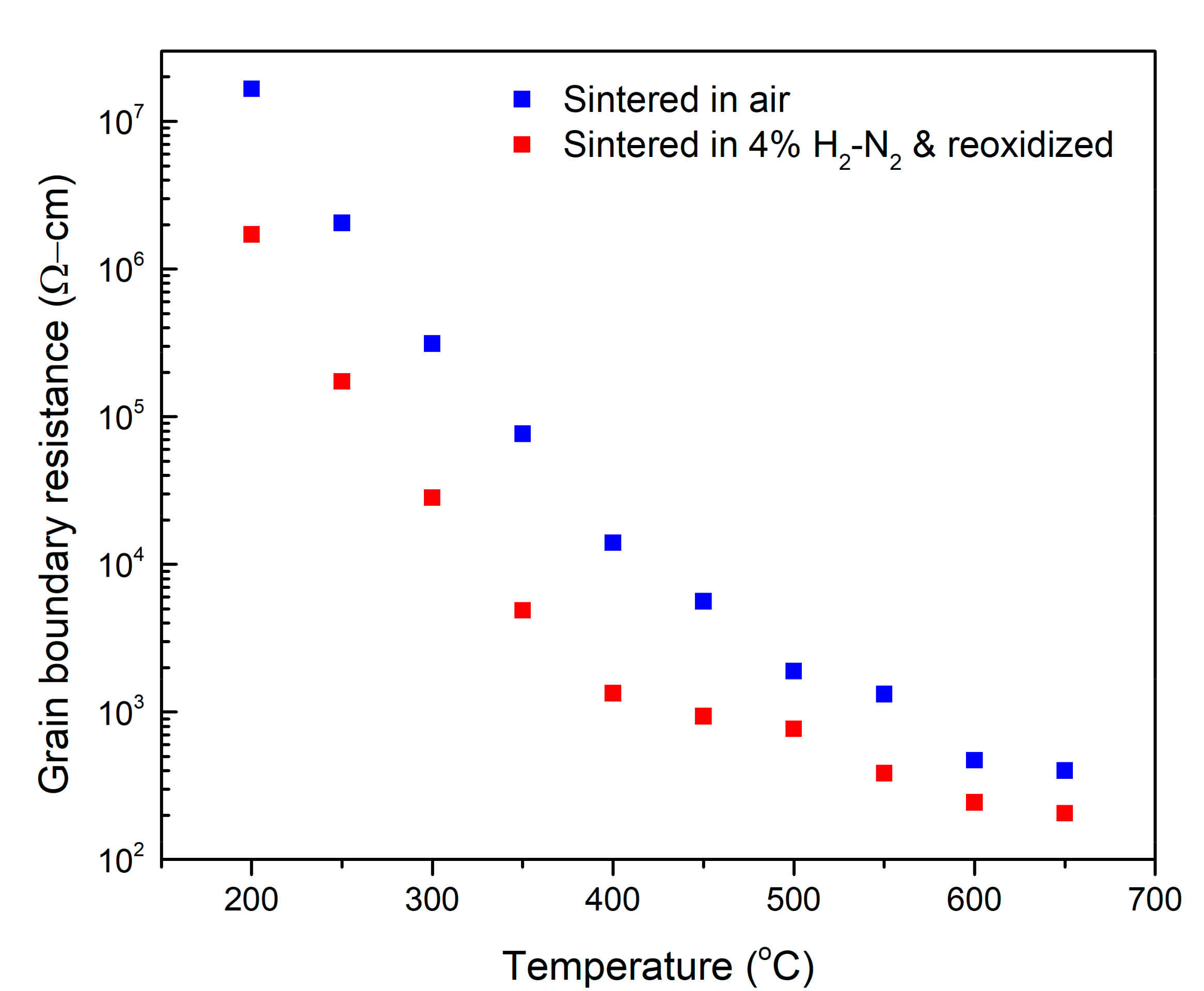
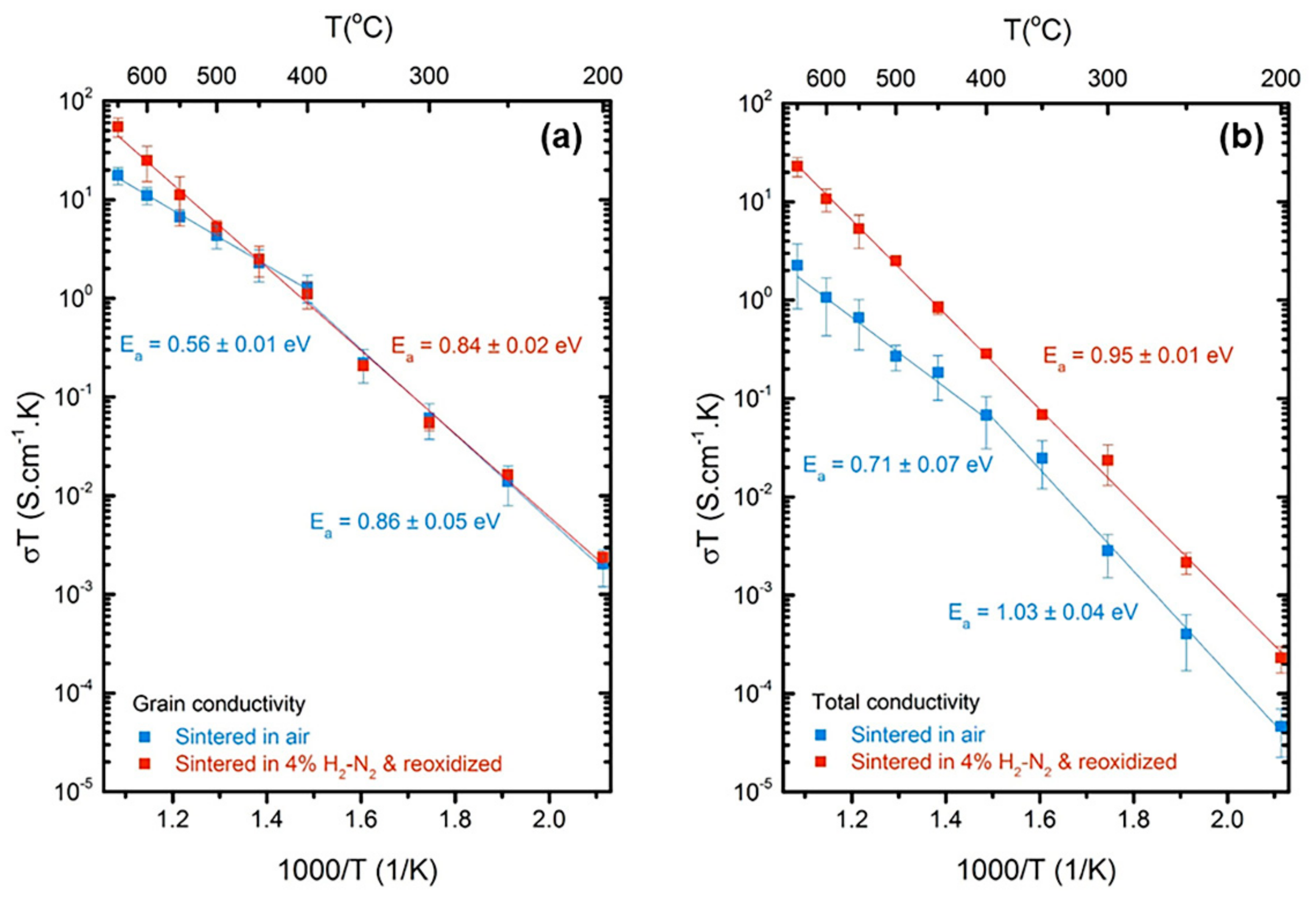
2. Results
3. Discussion
4. Materials and Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wachsman, E.D.; Lee, K.T. Lowering the temperature of solid oxide fuel cells. Science 2011, 334, 935–939. [Google Scholar] [CrossRef] [PubMed]
- Brett, D.J.; Atkinson, A.; Brandon, N.P.; Skinner, S.J. Intermediate temperature solid oxide fuel cells. Chem. Soc. Rev. 2008, 37, 1568–1578. [Google Scholar] [CrossRef] [PubMed]
- Inaba, H.; Tagawa, H. Ceria-based solid electrolytes. Solid State Ion. 1996, 83, 1–16. [Google Scholar] [CrossRef]
- Tuller, H.; Nowick, A. Doped ceria as a solid oxide electrolyte. J. Electrochem. Soc. 1975, 122, 255. [Google Scholar] [CrossRef]
- Eguchi, K.; Setoguchi, T.; Inoue, T.; Arai, H. Electrical properties of ceria-based oxides and their application to solid oxide fuel cells. Solid State Ion. 1992, 52, 165–172. [Google Scholar] [CrossRef]
- Bae, J.; Hong, S.; Koo, B.; An, J.; Prinz, F.B.; Kim, Y.-B. Influence of the grain size of samaria-doped ceria cathodic interlayer for enhanced surface oxygen kinetics of low-temperature solid oxide fuel cell. J. Eur. Ceram. Soc. 2014, 34, 3763–3768. [Google Scholar] [CrossRef]
- Bae, J.; Yang, H.; Son, J.; Koo, B.; Kim, Y.B. Enhanced Oxygen Reduction Reaction in Nanocrystalline Surface of Samaria-Doped Ceria via Randomly Distributed Dopants. J. Am. Ceram. Soc. 2016, 99, 4050–4056. [Google Scholar] [CrossRef]
- Omar, S.; Wachsman, E.D.; Nino, J.C. A co-doping approach towards enhanced ionic conductivity in fluorite-based electrolytes. Solid State Ion. 2006, 177, 3199–3203. [Google Scholar] [CrossRef]
- Xia, C.; Liu, M. Microstructures, conductivities, and electrochemical properties of Ce0. 9Gd0.1O2 and GDC–Ni anodes for low-temperature SOFCs. Solid State Ion. 2002, 152, 423–430. [Google Scholar] [CrossRef]
- Baure, G.; Zhou, H.; Chung, C.-C.; Buck, M.N.; Stozhkova, M.A.; Jones, J.L.; Nino, J.C. Comparison of the in-and across-plane ionic conductivity of highly oriented neodymium doped ceria thin films. Acta Mater. 2018, 147, 10–15. [Google Scholar] [CrossRef]
- Huang, H.; Gür, T.M.; Saito, Y.; Prinz, F. High ionic conductivity in ultrathin nanocrystalline gadolinia-doped ceria films. Appl. Phys. Lett. 2006, 89, 143107. [Google Scholar] [CrossRef]
- Avila-Paredes, H.J.; Choi, K.; Chen, C.-T.; Kim, S. Dopant-concentration dependence of grain-boundary conductivity in ceria: A space-charge analysis. J. Mater. Chem. 2009, 19, 4837–4842. [Google Scholar] [CrossRef]
- Tuller, H.L. Ionic conduction in nanocrystalline materials. Solid State Ion. 2000, 131, 143–157. [Google Scholar] [CrossRef] [Green Version]
- Christie, G.; Van Berkel, F. Microstructure—Ionic conductivity relationships in ceria-gadolinia electrolytes. Solid State Ion. 1996, 83, 17–27. [Google Scholar] [CrossRef]
- Guo, X.; Sigle, W.; Maier, J. Blocking grain boundaries in yttria-doped and undoped ceria ceramics of high purity. J. Am. Ceram. Soc. 2003, 86, 77–87. [Google Scholar] [CrossRef]
- Kim, S.K.; Khodorov, S.; Chen, C.-T.; Kim, S.; Lubomirsky, I. How to interpret current–voltage relationships of blocking grain boundaries in oxygen ionic conductors. Phys. Chem. Chem. Phys. 2013, 15, 8716–8721. [Google Scholar] [CrossRef] [PubMed]
- Gerhardt, R.; Nowick, A.S. Grain-boundary effect in ceria doped with trivalent cations: I, electrical measurements. J. Am. Ceram. Soc. 1986, 69, 641–646. [Google Scholar] [CrossRef]
- Omar, S. Enhanced Ionic Conductivity of Ceria-Based Compounds for the Electrolyte Application in SOFCs. Ph.D. Thesis, University of Florida, Gainesville, FL, USA, 2008. [Google Scholar]
- Gerhardt, R.; Nowick, A.; Mochel, M.; Dumler, I. Grain-boundary effect in ceria doped with trivalent cations: II, Microstructure and microanalysis. J. Am. Ceram. Soc. 1986, 69, 647–651. [Google Scholar] [CrossRef]
- Li, F.; Ohkubo, T.; Chen, Y.; Kodzuka, M.; Ye, F.; Ou, D.; Mori, T.; Hono, K. Laser-assisted three-dimensional atom probe analysis of dopant distribution in Gd-doped CeO2. Scr. Mater. 2010, 63, 332–335. [Google Scholar] [CrossRef]
- Diercks, D.R.; Tong, J.; Zhu, H.; Kee, R.; Baure, G.; Nino, J.C.; O’Hayre, R.; Gorman, B.P. Three-dimensional quantification of composition and electrostatic potential at individual grain boundaries in doped ceria. J. Mater. Chem. A 2016, 4, 5167–5175. [Google Scholar] [CrossRef]
- Kim, M.; Duscher, G.; Browning, N.D.; Sohlberg, K.; Pantelides, S.T.; Pennycook, S.J. Nonstoichiometry and the electrical activity of grain boundaries in SrTiO3. Phys. Rev. Lett. 2001, 86, 4056. [Google Scholar] [CrossRef] [Green Version]
- McGibbon, M.; Browning, N.; Chisholm, M.; McGibbon, A.; Pennycook, S.; Ravikumar, V.; Dravid, V. Direct determination of grain boundary atomic structure in SrTiO3. Science 1994, 266, 102–104. [Google Scholar] [CrossRef] [PubMed]
- Browning, N.; Buban, J.; Moltaji, H.; Pennycook, S.; Duscher, G.; Johnson, K.; Rodrigues, R.; Dravid, V.P. The influence of atomic structure on the formation of electrical barriers at grain boundaries in SrTiO3. Appl. Phys. Lett. 1999, 74, 2638–2640. [Google Scholar] [CrossRef]
- Guo, X.; Waser, R. Electrical properties of the grain boundaries of oxygen ion conductors: Acceptor-doped zirconia and ceria. Prog. Mater. Sci. 2006, 51, 151–210. [Google Scholar] [CrossRef]
- Fisher, C.A.; Matsubara, H. Molecular dynamics investigations of grain boundary phenomena in cubic zirconia. Comput. Mater. Sci. 1999, 14, 177–184. [Google Scholar] [CrossRef]
- Cantwell, P.R.; Tang, M.; Dillon, S.J.; Luo, J.; Rohrer, G.S.; Harmer, M.P. Grain boundary complexions. Acta Mater. 2014, 62, 1–48. [Google Scholar] [CrossRef]
- Schottky, W. Zur halbleitertheorie der sperrschicht-und spitzengleichrichter. Z. Phys. 1939, 113, 367–414. [Google Scholar] [CrossRef]
- Mott, N.F. The theory of crystal rectifiers. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1939, 171, 27–38. [Google Scholar]
- Guo, X.; Sigle, W.; Fleig, J.; Maier, J. Role of space charge in the grain boundary blocking effect in doped zirconia. Solid State Ion. 2002, 154, 555–561. [Google Scholar] [CrossRef]
- Guo, X.; Maier, J. Grain boundary blocking effect in zirconia: A Schottky barrier analysis. J. Electrochem. Soc. 2001, 148, E121. [Google Scholar] [CrossRef]
- Göbel, M.C.; Gregori, G.; Guo, X.; Maier, J. Boundary effects on the electrical conductivity of pure and doped cerium oxide thin films. Phys. Chem. Chem. Phys. 2010, 12, 14351–14361. [Google Scholar] [CrossRef]
- Frechero, M.A.; Rocci, M.; Sánchez-Santolino, G.; Kumar, A.; Salafranca, J.; Schmidt, R.; Díaz-Guillén, M.; Durá, O.; Rivera-Calzada, A.; Mishra, R. Paving the way to nanoionics: Atomic origin of barriers for ionic transport through interfaces. Sci. Rep. 2015, 5, 17229. [Google Scholar] [CrossRef] [Green Version]
- Durá, O.; De La Torre, M.L.; Vázquez, L.; Chaboy, J.; Boada, R.; Rivera-Calzada, A.; Santamaria, J.; Leon, C. Ionic conductivity of nanocrystalline yttria-stabilized zirconia: Grain boundary and size effects. Phys. Rev. B 2010, 81, 184301. [Google Scholar] [CrossRef] [Green Version]
- Lee, W.; Jung, H.J.; Lee, M.H.; Kim, Y.B.; Park, J.S.; Sinclair, R.; Prinz, F.B. Oxygen surface exchange at grain boundaries of oxide ion conductors. Adv. Funct. Mater. 2012, 22, 965–971. [Google Scholar] [CrossRef]
- Coppola, N.; Polverino, P.; Carapella, G.; Sacco, C.; Galdi, A.; Montinaro, D.; Maritato, L.; Pianese, C. Optimization of the electrical performances in Solid Oxide Fuel Cells with room temperature sputter deposited Gd0.1ce0.9o1.95 buffer layers by controlling their granularity via the in-air annealing step. Int. J. Hydrogen Energy 2020, 45, 12997–13008. [Google Scholar] [CrossRef]
- Ciacchi, F.; Nightingale, S.; Badwal, S. Microwave sintering of zirconia-yttria electrolytes and measurement of their ionic conductivity. Solid State Ion. 1996, 86, 1167–1172. [Google Scholar] [CrossRef]
- Mahboob, S.; Prasad, G.; Kumar, G. Impedance and ac conductivity studies on Ba (Nd0.2Ti0.6Nb0.2)O3 ceramic prepared through conventional and microwave sintering route. Bull. Mater. Sci. 2006, 29, 347–355. [Google Scholar] [CrossRef]
- Kabir, A.; Santucci, S.; Van Nong, N.; Varenik, M.; Lubomirsky, I.; Nigon, R.; Muralt, P.; Esposito, V. Effect of oxygen defects blocking barriers on gadolinium doped ceria (GDC) electro-chemo-mechanical properties. Acta Mater. 2019, 174, 53–60. [Google Scholar] [CrossRef]
- Cologna, M.; Rashkova, B.; Raj, R. Flash Sintering of Nanograin Zirconia in <5 s at 850 C. J. Am. Ceram. Soc. 2010, 93, 3556–3559. [Google Scholar] [CrossRef]
- Yu, M.; Grasso, S.; Mckinnon, R.; Saunders, T.; Reece, M.J. Review of flash sintering: Materials, mechanisms and modelling. Adv. Appl. Ceram. 2017, 116, 24–60. [Google Scholar] [CrossRef] [Green Version]
- An, J.; Bae, J.; Hong, S.; Koo, B.; Kim, Y.-B.; Gür, T.M.; Prinz, F.B. Grain boundary blocking of ionic conductivity in nanocrystalline yttria-doped ceria thin films. Scr. Mater. 2015, 104, 45–48. [Google Scholar] [CrossRef] [Green Version]
- Bae, J.; Lim, Y.; Park, J.-S.; Lee, D.; Hong, S.; An, J.; Kim, Y.-B. Thermally-induced dopant segregation effects on the space charge layer and ionic conductivity of nanocrystalline gadolinia-doped ceria. J. Electrochem. Soc. 2016, 163, F919. [Google Scholar] [CrossRef]
- Esposito, V.; He, Z.; Zhang, W.; Prasad, A.S.; Glasscock, J.A.; Chatzichristodoulou, C.; Ramousse, S.; Kaiser, A. Enhanced mass diffusion phenomena in highly defective doped ceria. Acta Mater. 2013, 61, 6290–6300. [Google Scholar] [CrossRef] [Green Version]
- Inaba, H.; Nakajima, T.; Tagawa, H. Sintering behaviors of ceria and gadolinia-doped ceria. Solid State Ion. 1998, 106, 263–268. [Google Scholar] [CrossRef]
- Zhang, T.; Ma, J.; Chan, S.; Kilner, J. Improvements in Sintering Behavior and Grain-Boundary Conductivity of Ceria-Based Electrolytes by a Small Addition of Fe2O3. J. Electrochem. Soc. 2004, 151, J84. [Google Scholar] [CrossRef]
- Higashi, K.; Sonoda, K.; Ono, H.; Sameshima, S.; Hirata, Y. Synthesis and sintering of rare-earth-doped ceria powder by the oxalate coprecipitation method. J. Mater. Res. 1999, 14, 957–967. [Google Scholar] [CrossRef]
- Sameshima, S.; Ono, H.; Higashi, K.; Sonoda, K.; Hirata, Y. Microstructure of rare-earth-doped ceria prepared by oxalate coprecipitation method. J. Ceram. Soc. Jpn. 2000, 108, 985–988. [Google Scholar] [CrossRef] [Green Version]
- Hong, S.J.; Virkar, A.V. Lattice parameters and densities of rare-earth oxide doped ceria electrolytes. J. Am. Ceram. Soc. 1995, 78, 433–439. [Google Scholar] [CrossRef]
- ASTM B962-15. Standard Test Methods for Density of Compacted or Sintered Powder Metallurgy (PM) Products Using Archimedes’ Principle; ASTM International: West Conshohocken, PA, USA, 2015. [Google Scholar]
- Basu, S.; Devi, P.S.; Maiti, H.S. Synthesis and properties of nanocrystalline ceria powders. J. Mater. Res. 2004, 19, 3162–3171. [Google Scholar] [CrossRef]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy Theory, Experiment, and Applications, 2nd ed.; John Wiley &Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Abram, E.; Sinclair, D.; West, A. A strategy for analysis and modelling of impedance spectroscopy data of electroceramics: Doped lanthanum gallate. J. Electroceram. 2003, 10, 165–177. [Google Scholar] [CrossRef]
- Hume-Rothery, W. Atomic Theory for Students of Metallurgy; The Institute of Metals: London, UK, 1952. [Google Scholar]
- Bevan, D. Ordered intermediate phases in the system CeO2 Ce2O3. J. Inorg. Nucl. Chem. 1955, 1, 49–59. [Google Scholar] [CrossRef]
- Bevan, D.; Kordis, J. Mixed oxides of the type MO2 (fluorite)—M2O3—I oxygen dissociation pressures and phase relationships in the system CeO2 Ce2O3 at high temperatures. J. Inorg. Nucl. Chem. 1964, 26, 1509–1523. [Google Scholar] [CrossRef]
- Hayles, J.; Bao, H. The reduction and oxidation of ceria: A natural abundance triple oxygen isotope perspective. Geochim. Cosmochim. Acta 2015, 159, 220–230. [Google Scholar] [CrossRef]
- Galusek, D.; Ghillányová, K. Ceramic oxides. In Ceramic Science and Technology; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2010; Volume 2, pp. 43–48. [Google Scholar]
- ASTM E112-13. Standard Test Methods for Determining Average Grain Size; ASTM International: West Conshohocken, PA, USA, 2003. [Google Scholar]
- McLachlan, D.; Hwang, J.-H.; Mason, T.O. Evaluating dielectric impedance spectra using effective media theories. J. Electroceram. 2000, 5, 37–51. [Google Scholar] [CrossRef]
- Starikov, E. ‘Meyer-Neldel Rule’: True history of its development and its intimate connection to classical thermodynamics. J. Appl. Solut. Chem. Model. 2014, 3, 15–31. [Google Scholar] [CrossRef]
- Segal, D. Chemical synthesis of ceramic materials. J. Mater. Chem. 1997, 7, 1297–1305. [Google Scholar] [CrossRef]
- Spiridigliozzi, L.; Dell’Agli, G.; Biesuz, M.; Sglavo, V.M.; Pansini, M. Effect of the Precipitating Agent on the Synthesis and Sintering Behavior of 20 mol% Sm-Doped Ceria. Adv. Mater. Sci. Eng. 2016, 2016, 6096123. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sulekar, S.; Mehr, M.; Kim, J.H.; Nino, J.C. Effect of Reduced Atmosphere Sintering on Blocking Grain Boundaries in Rare-Earth Doped Ceria. Inorganics 2021, 9, 63. https://doi.org/10.3390/inorganics9080063
Sulekar S, Mehr M, Kim JH, Nino JC. Effect of Reduced Atmosphere Sintering on Blocking Grain Boundaries in Rare-Earth Doped Ceria. Inorganics. 2021; 9(8):63. https://doi.org/10.3390/inorganics9080063
Chicago/Turabian StyleSulekar, Soumitra, Mehrad Mehr, Ji Hyun Kim, and Juan Claudio Nino. 2021. "Effect of Reduced Atmosphere Sintering on Blocking Grain Boundaries in Rare-Earth Doped Ceria" Inorganics 9, no. 8: 63. https://doi.org/10.3390/inorganics9080063
APA StyleSulekar, S., Mehr, M., Kim, J. H., & Nino, J. C. (2021). Effect of Reduced Atmosphere Sintering on Blocking Grain Boundaries in Rare-Earth Doped Ceria. Inorganics, 9(8), 63. https://doi.org/10.3390/inorganics9080063





