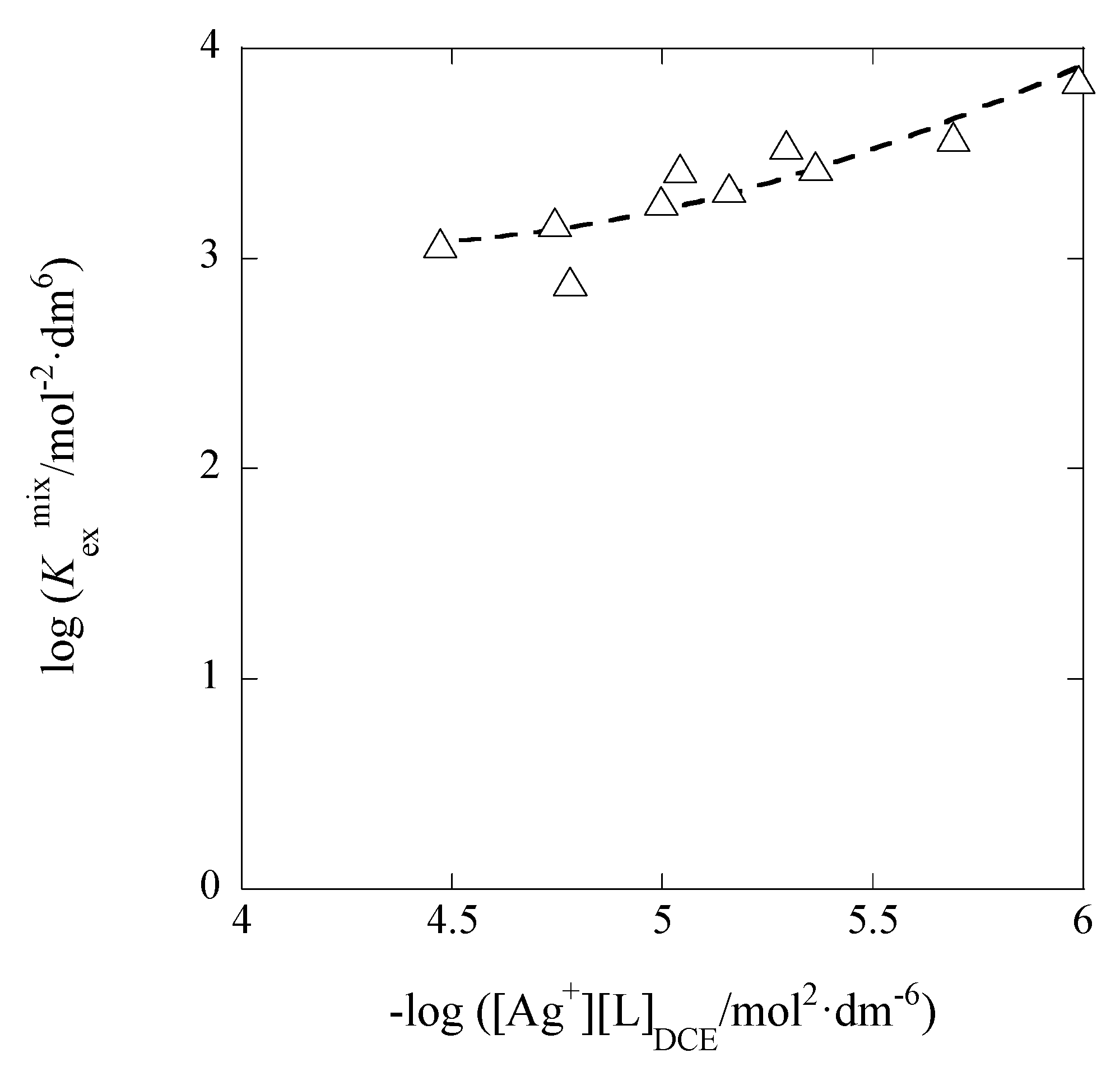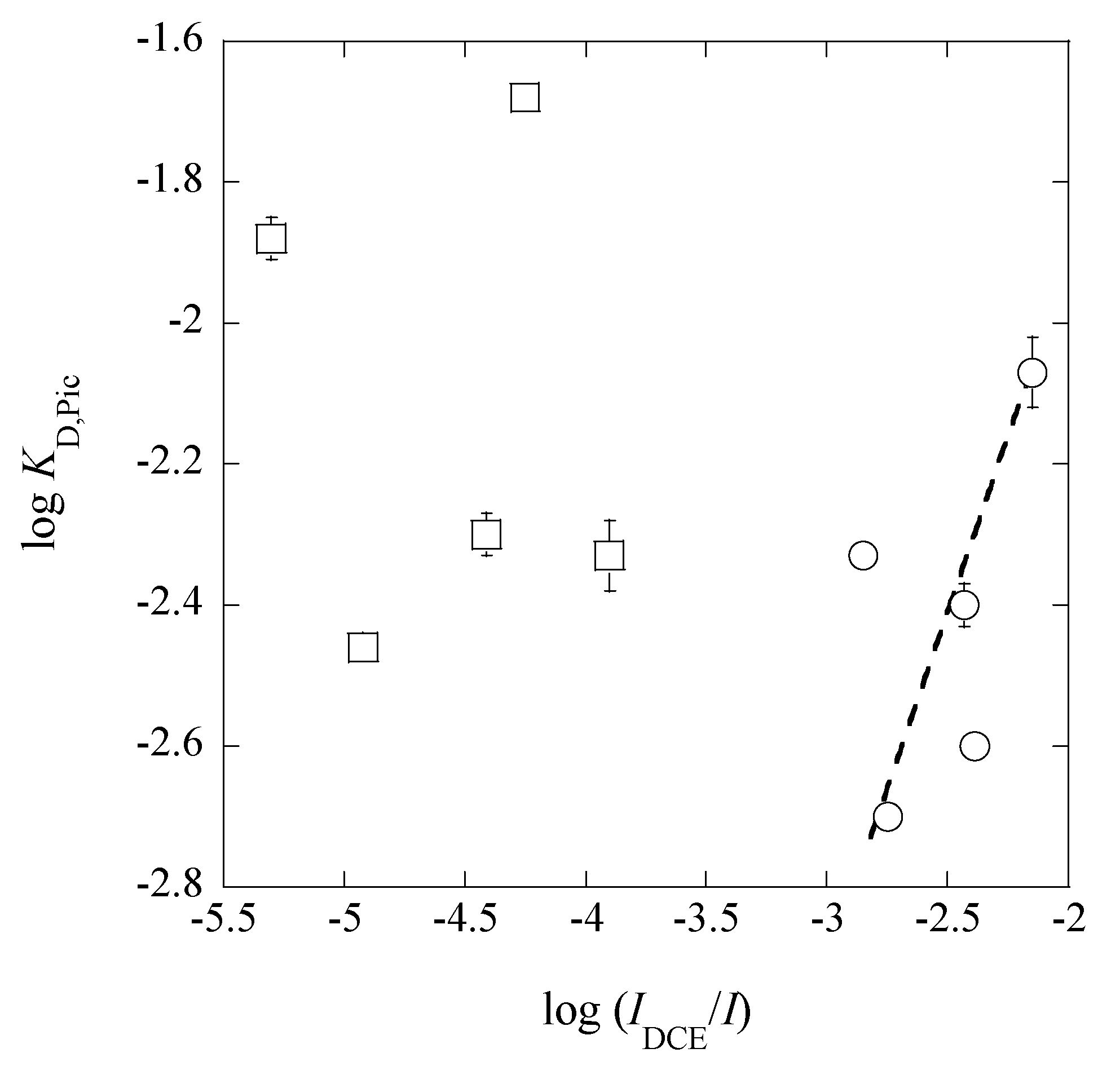Silver(I) Extraction with Benzo-18-Crown-6 Ether from Water into 1,2-Dichloroethane: Analyses on Ionic Strength of the Phases and their Equilibrium Potentials

Abstract

Extraction constants (K_ex & K_ex±) for the extraction of silver picrate (AgPic) by benzo-18-crown-6 ether (B18C6) into 1,2-dichloroethane (DCE) were determined at 298 K and various ionic strength (I)-values of a water phase with or without excess HNO₃. Here the symbols, K_ex and K_ex±, were defined as [AgLPic]_DCE/P and [AgL⁺]_DCE[Pic⁻]_DCE/P with P = [Ag⁺][L]_DCE[Pic⁻] and L = B18C6, respectively; [ ]_DCE refers to the concentration of the corresponding species in the DCE phase at equilibrium. Simultaneously, K_D,Pic (= [Pic⁻]_DCE/[Pic⁻]) and K_1,DCE (= K_ex/K_ex±) values for given I and I_DCE values were determined, where the symbol I_DCE shows I of the DCE phase. Also, equilibrium potential differences (Δφ_eq) based on the Pic⁻ transfer at the water/DCE interface were obtained from the analysis of the K_D,Pic [= K_D,Pic^S exp{−(F/RT) Δφ_eq}] values; the symbol K_D,Pic^S shows K_D,Pic at Δφ_eq = 0 V. On the basis of these results, I dependences of logK_ex and logK_ex± and I_DCE ones of logK_1,DCE and logK_ex± were examined. Extraction experiments of AgClO₄ and AgNO₃ by B18C6 into DCE were done for comparison. The logK_ex±-versus-Δφ_eq plot for the above Ag(I) extraction systems with Pic⁻, ClO₄⁻, and NO₃⁻ gave a good positive correlation.

1. Introduction

It is well known that crown compounds (L) extract alkali and alkaline-earth metal ions (M^z+, z = 1, 2) from water (w) into various diluents [1,2,3,4]. In many extraction experiments, extraction constants for L have been determined so far [1,2,3,4,5,6,7,8]. For example, the two representative constants, K_ex and K_ex±, for the extraction of a univalent metal salt (M^IA) by L have been defined as [MLA]_org/P [2,3] and [ML⁺]_org[A⁻]_org/P [1,4] with P = [M⁺][L]_org[A⁻], respectively. Generally, the K_ex value is effective for the evaluation of an extraction-ability and -selectivity of L against M⁺ into low-polar diluents, while the K_ex± value is for those of L into high-polar ones. Here, the subscript ″org″ denotes an organic phase and A⁻ does a univalent pairing anion. For the latter K_ex±, its thermodynamic equilibrium constants have been reported [4]. For the former K_ex, its thermodynamic treatment seems to be few. The authors were not able to find out the study with respect to a dependence of logK_ex on the ionic strength (I) of the w phase.

Presences of equilibrium potential differences (Δφ_eq) between aqueous and diluent solutions have been recently reported for the extraction of some M⁺ or M²⁺ with L [5,6,7]. This symbol Δφ_eq was defined as ∑{inner potential (φ ) of ionic species in the w phase} − ∑{φ of those in the org or diluent phase} [8], according to the definition [9,10], φ_w − φ_org, of an interfacial equilibrium potential-difference in the electrochemistry at liquid/liquid interfaces. In the above studies, an approximation method [5,6,7,8] for the Δφ_eq determination, namely the method with a use of a ″conditional″ distribution constant (K_D,A) of A⁻ into the org phase, has been described in comparison with its more-precise method with solving higher-degree equations [6]. Here the ″conditional″ is due to the fact that the K_D,A values change depending on the Δφ_eq ones, even at fixed pressure and temperature. However, it is still not clarified whether the Δφ_eq values determined by the K_D,A values equal those coming from the distribution of M⁺ into the org phases or not.

In the present paper, we determined the K_ex, K_ex±, and K_D,A values [6,8] at 298 K by the extraction experiments into 1,2-dichloroethane (DCE) with silver picrate (AgPic) and benzo-18-crown-6 ether (B18C6), in order to elucidate mainly the above two subjects for I and Δφ_eq. The same experiments were performed under the condition of the presence of excess HNO₃ in the w phases. Then, an ion-pair formation constant (K_1,DCE/mol⁻¹·dm³) for Ag(B18C6)⁺Pic⁻ in the DCE phase, DCE saturated with water, and the Δφ_eq values were calculated from the relations, K_1,DCE = K_ex/K_ex± [6,8] and Δφ_eq = −(2.303RT/F){logK_D,A − log(K_D,A standardized at Δφ_eq = 0 V)} [11], respectively. Here, R, T, and F are usual meanings. On the basis of these data, the dependences of logK_ex and logK_ex± on the I values and those of logK_1,DCE and logK_ex± on the I values (I_DCE) of the DCE phases were examined. Moreover, a relation between the Δφ_eq values determined by the K_D,A ones and the conditional distribution constants (K_D,Ag) of Ag⁺ into the DCE phases was discussed indirectly. For comparison, the K_ex± and K_ex values were experimentally determined at 298 K for the AgClO₄- and AgNO₃-B18C6 extraction into DCE. As basic data, the K_D,Ag^S value was determined in terms of a simple Ag⁺Pic⁻ extraction experiment into DCE. The symbol K_D,Ag^S denotes the distribution constant of Ag⁺ into the DCE phase standardized at Δφ_eq = 0 V, that is, the standard distribution one.

2. Results

2.1. Determination of LogK_D,Ag^S

According to our previous paper [12], the K_D,M^S value has been obtained from a plot of D_A′ versus [A⁻] based on the equation

D_A′ = [A]_t,org/[A⁻] = K_ex,MA[A⁻] + K_{D, ±}

(1)

with

K_{D, ±}² = K_D,M^S·K_D,A^S = K_D,M·K_D,A

(2)

and K_ex,MA = K_MA,org(K_{D, ±})² (= [MA]_org/[M⁺][A⁻]), where K_MA,org is [MA]_org/[M⁺]_org[A⁻]_org and [A]_t,org denotes a total concentration, [MA]_org + [A⁻]_org, of A(−I) in the org phase. A regression analysis of the plot (see Figure 1) yields a straight line with a slope of K_ex,AgPic and an intercept of K_{D, ±}. We call this K_{D, ±} a mean distribution constant.

Using the K_D,Pic^S value (= 10^−1.011 [12]), we immediately can obtain the K_D,Ag^S one from Equation (2). The thus-determined values were logK_{D, ±} = −3.74 ± 0.04, logK_ex,AgPic = −1.49 ± 0.05, and logK_AgPic,DCE = 5.992 ± 0.008 and then the logK_D,Ag^S value became −6.47 ± 0.04 from the logarithmic form of Equation (2). This K_D,Ag^S value was used only for the K_AgL,DCE calculation (see

Table 1. Fundamental data for the extraction with AgPic, AgClO₄, or AgNO₃ (= AgA) and B18C6 (= L) into DCE at 298 K.

A− (I 1/10−3)	logKex±	logKD,A [Δφeq/V]	logKex	logK1,DCE (IDCE1/10−5)	logKAgL,DCE
Pic− (2.5)	0.33 ± 0.03	−2.40 ± 0.03 [0.082]	5.31 ± 0.03	4.98 ± 0.05 (0.93)	7.81
(2.7)2	0.51	−2.60 [0.094]	5.336	4.82 (1.1)	7.99 3
(2.8)2	0.25	−2.33 [0.078]	5.17	4.92 (0.40)	7.73 3
(3.1)	0.40 ± 0.09	−2.07 ± 0.05 [0.063]	4.93 ± 0.05	4.53 ± 0.10 (2.2)	7.88
(3.6) 2	0.17	−2.70 [0.10]	5.55	5.38 (0.64)	7.65 3
ClO4− (2.8)	−1.24 ± 0.02	−2.13 ± 0.11 [−0.032]	2.99 ± 0.11	4.23 ± 0.11 (2.1)	8.07
NO3− (3.1)	−4.40 ± 0.07	−3.47 ± 0.04 [−0.14]	1.04 ± 0.03	5.44 ± 0.08 (0.11)	7.98

¹ Unit: mol·dm⁻³; ² Values in this line were cited from Ref. [6]; ³ Values re-calculated from the relation logK_AgL,DCE = logK_ex± − logK_D,Ag^S·K_D,Pic^S = logK_ex± + 7.481, since the K_D,Ag^S value was re-determined in this study.

and

Table 2. Fundamental data for the extraction with AgPic and B18C6 (= L) into DCE in the presence of excess HNO₃ in the water phases at 298 K.

I/10−2 mol·dm−3	logKex±	logKD,Pic [Δφeq/V]	logKex	logK1,DCE (IDCE 1/10−6)	logKAgL,DCE
2.4	0.38 ± 0.10	−2.33 ± 0.05 [0.078]	5.35 ± 0.04	4.97 ± 0.11 (3.0)	7.87
5.0	0.00 ± 0.06	−2.30 ± 0.03 [0.076]	5.41 ± 0.03	5.41 ± 0.07 (2.0)	7.49
9.7 2	−0.13	−1.68 [0.040]	5.07	5.20 (5.5)	7.35 3
11	−0.23 ± 0.06	−2.26 ± 0.02 [0.074]	5.45 ± 0.02	5.68 ± 0.06 (0.97)	7.26
26	−0.78 ± 0.06	−1.88 ± 0.03 [0.051]	5.11 ± 0.02	5.89 ± 0.07 (1.3)	6.70

¹ Unit: see I; ² See the footer 2 in Table 1; ³ See the footer 3 in Table 1.

).

2.2. Composition Determination of Complex Species Extracted into DCE

Compositions of species extracted into DCE have been determined by a plot of 2logD or log(D/[A⁻]) versus log[L]_org [2,7,13]. When the slope of both plots is in unity, it independently gives the compositions of ML⁺ with A⁻ or MLA as the extracted major species. Among their plots, experimental slopes of all the log(D/[Pic⁻])-versus-log[B18C6]_org plots were less than unity, suggesting the dissociation of Ag(B18C6)Pic in the DCE phases [13]. On the other hand, the 2logD-versus-log[L]_org plots were in the slope ranges of 1.01–1.11 for the AgPic extraction with L = B18C6, in the slopes of 1.09 for the AgClO₄ one and of 1.08 for the AgNO₃ one (Figure 2). These results indicate the AgB18C6⁺ extraction into DCE with A⁻ = Pic⁻, ClO₄⁻, or NO₃⁻. That is, the extraction systems were accompanied with the dissociation process, Ag(B18C6)A_DCE $\rightleftharpoons$ AgB18C6⁺_DCE + A⁻_DCE (see Appendix).

2.3. Determination of Various Equilibrium Constants for the Extraction Systems

For the determination of K_D,A, K_ex±, and K_ex, the following extraction-constant parameter (K_ex^mix/mol⁻²·dm⁶) has been employed [7,8,11].

logK_ex^mix = log{([MLA]_org + [ML⁺]_org + [M⁺]_org)/P}

(3)

Rearranging this equation, we immediately obtain

logK_ex^mix ≈ log{K_ex + (K_D,A/[M⁺][L]_org)}

(3a)

≈ log{K_ex + (K_ex±/P)^1/2}

(3b)

under the assumption of [MLA]_org + [ML⁺]_org >> [M⁺]_org. So we can determine the K_D,A and K_ex± values from the plots of logK_ex^mix versus −log([M⁺][L]_org) {see Equation (3a)} and −(1/2)logP {see Equation (3b)}, respectively, together with the K_ex values. Figure 3 and Figure 4 show examples for such plots. Additionally, the ion-pair formation constant, K_1,org, for MLA in the org phase was calculated from K_1,org = K_ex/K_ex± for a given I_org on average.

Similarly, a complex formation constant (K_AgB18C6,DCE/mol⁻¹·dm³) for AgB18C6⁺ in the DCE phase was estimated from the thermodynamic relation of K_AgB18C6,DCE = K_ex±/(K_D,Ag^S·K_D,Pic^S). Table 1 and Table 2 list the logarithmic K_D,A, K_ex±, K_ex, K_1,DCE, and K_AgB18C6,DCE values thus-obtained, together with the average I and I_DCE values.

2.4. Determination of Equilibrium Potential Differences between the Water and DCE Phases

The logK_D,A values were obtained from the plots based on Equation (3a). Next, the equilibrium potential differences Δφ_eq can be evaluated from using the following equation [11]:

Δφ_eq = −(2.303RT/F)(logK_D,A − logK_D,A^S)

(4)

with

logK_D,A^S = (F/2.303RT)Δφ_A°′

(4a)

where the symbols, K_D,A^S and Δφ_A°′, are called the standard distribution constant of A⁻ into the org phase, namely K_D,A at Δφ_eq = 0 V, and a standard formal potential for the A⁻ transfer at the w/diluent interface, respectively; see Section 2.1 for the K_D,Pic^S value. The thus-evaluated values are listed in Table 1 and Table 2.

Strictly speaking, Δφ_eq and Δφ_A⁰′ are the equilibrium potential differences between the two bulk phases. However, they can be regarded approximately as the equilibrium potential differences at the liquid/liquid interfaces [9,10].

3. Discussion

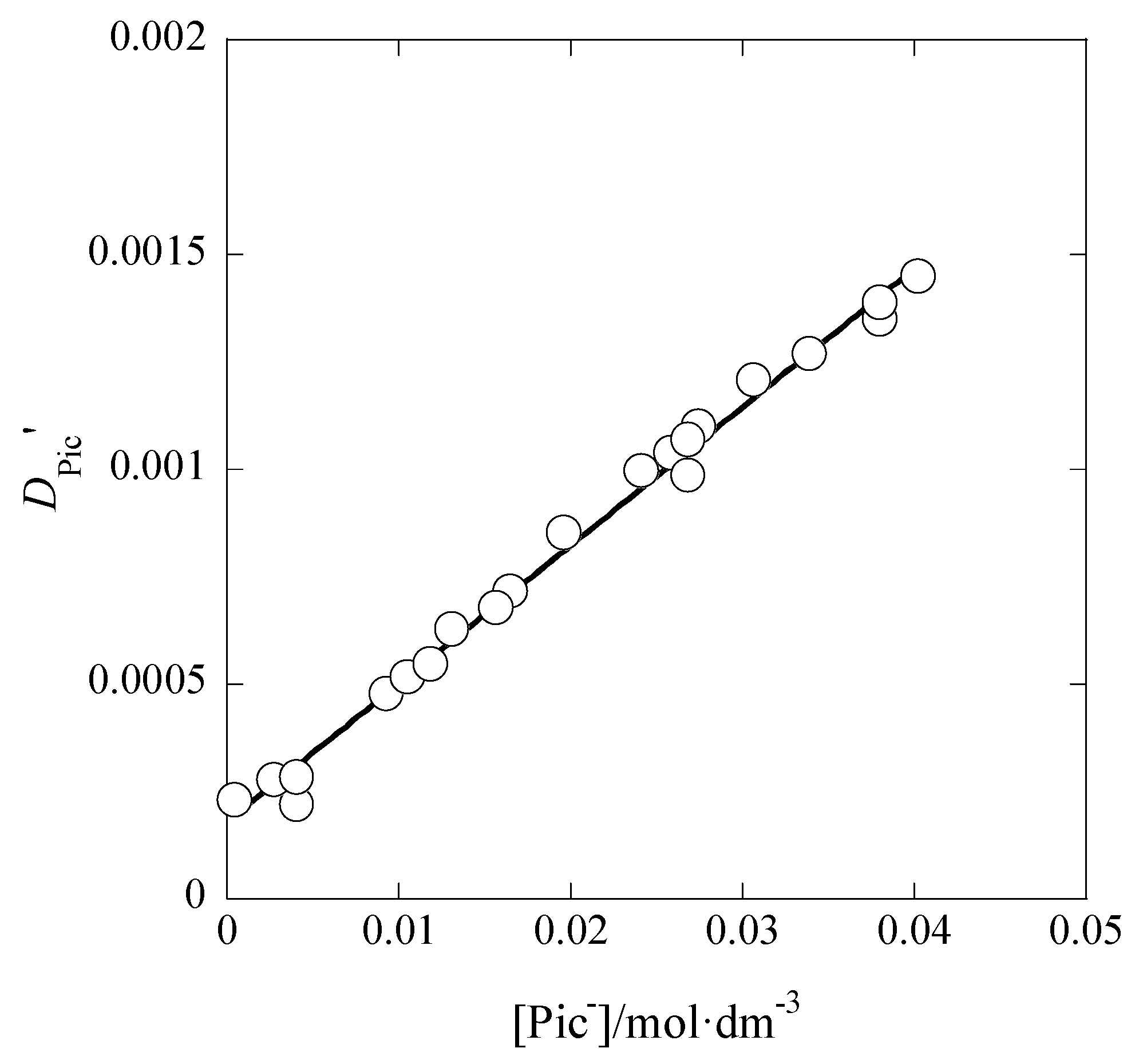
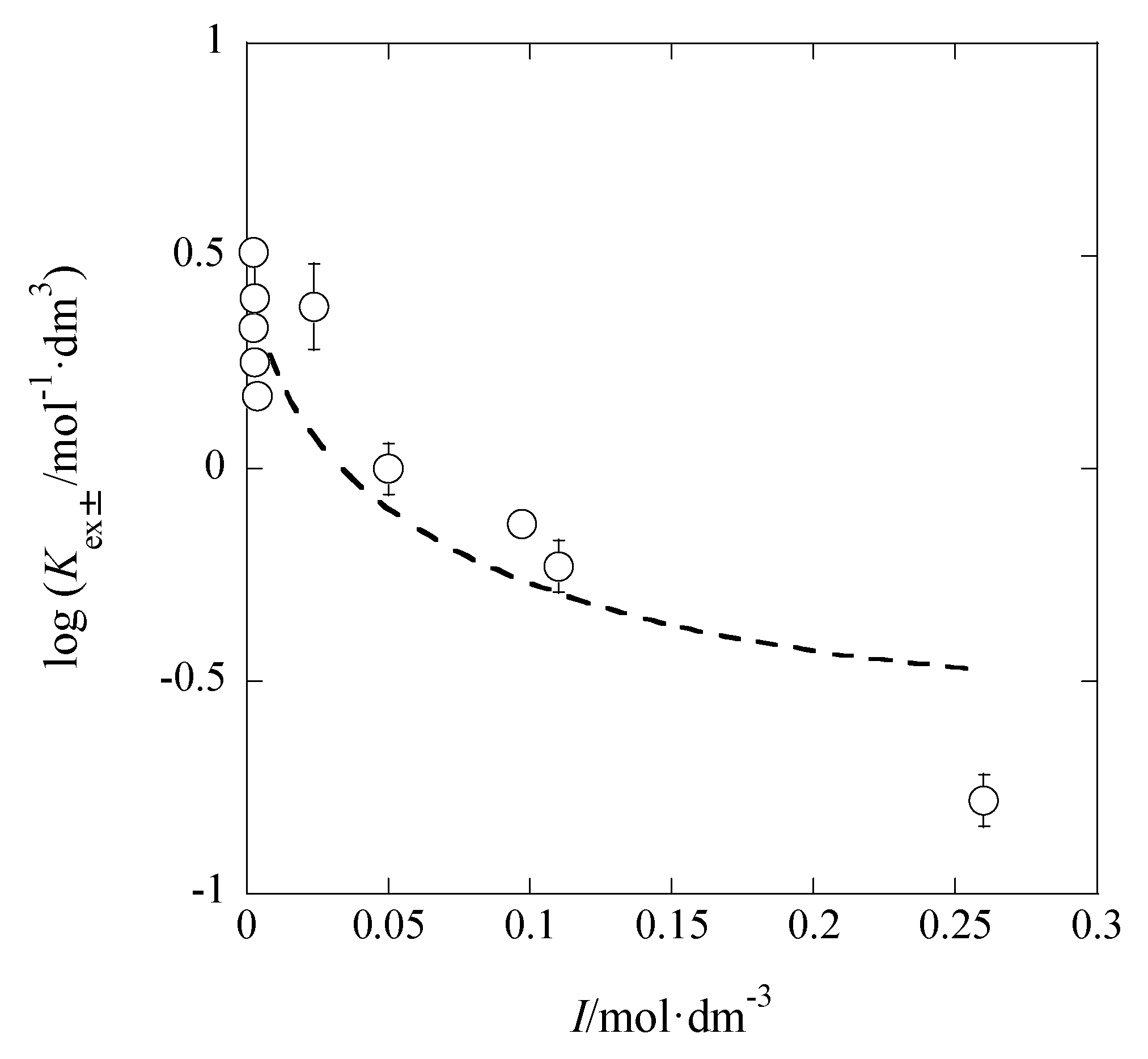
3.1. I Dependences of LogK_ex and LogK_ex±

The dependences of logK_ex and logK_ex± on I are considered below. On the basis of their definitions, the K_ex value can be dependent on [M⁺] and [A⁻], while, in addition to these concentrations, the K_ex± value can be on [ML⁺]_org and [A⁻]_org. Therefore, K_ex is mainly a function of I, while K_ex± is a function with the two parameters, I and I_org; the relations [14] of [M⁺] = a_M/y₊(I) and [A⁻]_org = a_A,org/y_−,org(I_org) hold as examples (see below for the symbols a and y). The I dependence of logK_ex± is of an approximate.

Figure 5 shows the logK_ex-versus-I plot for the AgPic-B18C6 extraction system with DCE; in the plot, the average value of I was employed as I (x-axis) of each system. Using the extended Debye-Hückel (DH) equation [14], the extraction constant (K_ex⁰) at I → 0 mol·dm⁻³ is expressed as

logK_ex⁰ = log([MLA]_org/a_M[L]_orga_A) = logK_ex − log(y₊y₋)

$$= \log K_{\mathrm{ex}}+ 2A\sqrt{I}/(1 +Bå\sqrt{I})$$

(5)

where the symbols, a_j and y, denote the activity of species j (= M⁺, A⁻) and its activity coefficient with I, respectively [14]. Rearranging Equation (5), the following equation was obtained immediately:

$$\log K_{\mathrm{ex}}= \log {K_{\mathrm{ex}}}^0- 2A\sqrt{I}/(1 +Bå\sqrt{I})$$

(5a)

The regression analysis of the plot in Figure 5 based on this equation yielded the regression line with log(K_ex⁰/mol⁻²·dm⁶) = 5.28 ± 0.25 and Bå = 44 ± 661 mol^−1/2·dm^3/2 Å at r = 0.024 and N (number of data) = 10, the fixed A value (= 0.5114) in pure water, and 298 K. Considering the error of the experimental Bå value, it is difficult to discuss the Bå or å value in this result. When the three parameters, logK_ex⁰, A, and Bå, had been used for the regression analysis, it gave the results of logK_ex⁰ > 0, A < 0, and Bå < 0. Consequently, we gave up such an analysis.

Similarly, the extraction constant (K_ex±⁰) at I → 0 is expressed as

logK_ex±⁰ = log(a_ML,orga_A,org/a_M[L]_orga_A)

= logK_ex± + log(y_ML,orgy_−,org) − log(y₊y₋)

(6)

where the subscript “ML” means the complex ion ML⁺. Rearranging this equation, we can immediately obtain

$$\log K_{\mathrm{ex}\pm }= \log {K_{\mathrm{ex}\pm }}^0\prime - 2A\sqrt{I}/(1 +Bå\sqrt{I}),$$

(6a)

where K_ex±⁰′ denotes K_ex±⁰/(y_ML,orgy_−,org) (= [ML⁺]_org[A⁻]_org/a_M[L]_orga_A). Unfortunately, the analysis of the plot based on Equation (6a) did not yield the suitable result which satisfies the condition of Bå > 0.

On the other hand, using the Davies equation without Bå instead of the extended DH equation [14], logK_ex±⁰′ = 0.60 ± 0.11 and A = 2.05 ± 0.38 mol^−1/2·dm^3/2 were obtained (Figure 6). The Davies equation is logy = −A$z^2\{\sqrt{I}$/(1 + $\sqrt{I}$) − 0.3I} [14], where z shows a formal charge of ionic species with a sign (refer to the Introduction).

The analysis of the logK_ex-versus-I plot by the Davies equation yielded logK_ex⁰ = 5.29 ± 0.11 with A = 0.08 ± 0.40 mol^−1/2·dm^3/2. Within the calculation error of ±0.3, this logK_ex⁰ value was in accord with 5.3 determined by the DH equation (see above in this section).

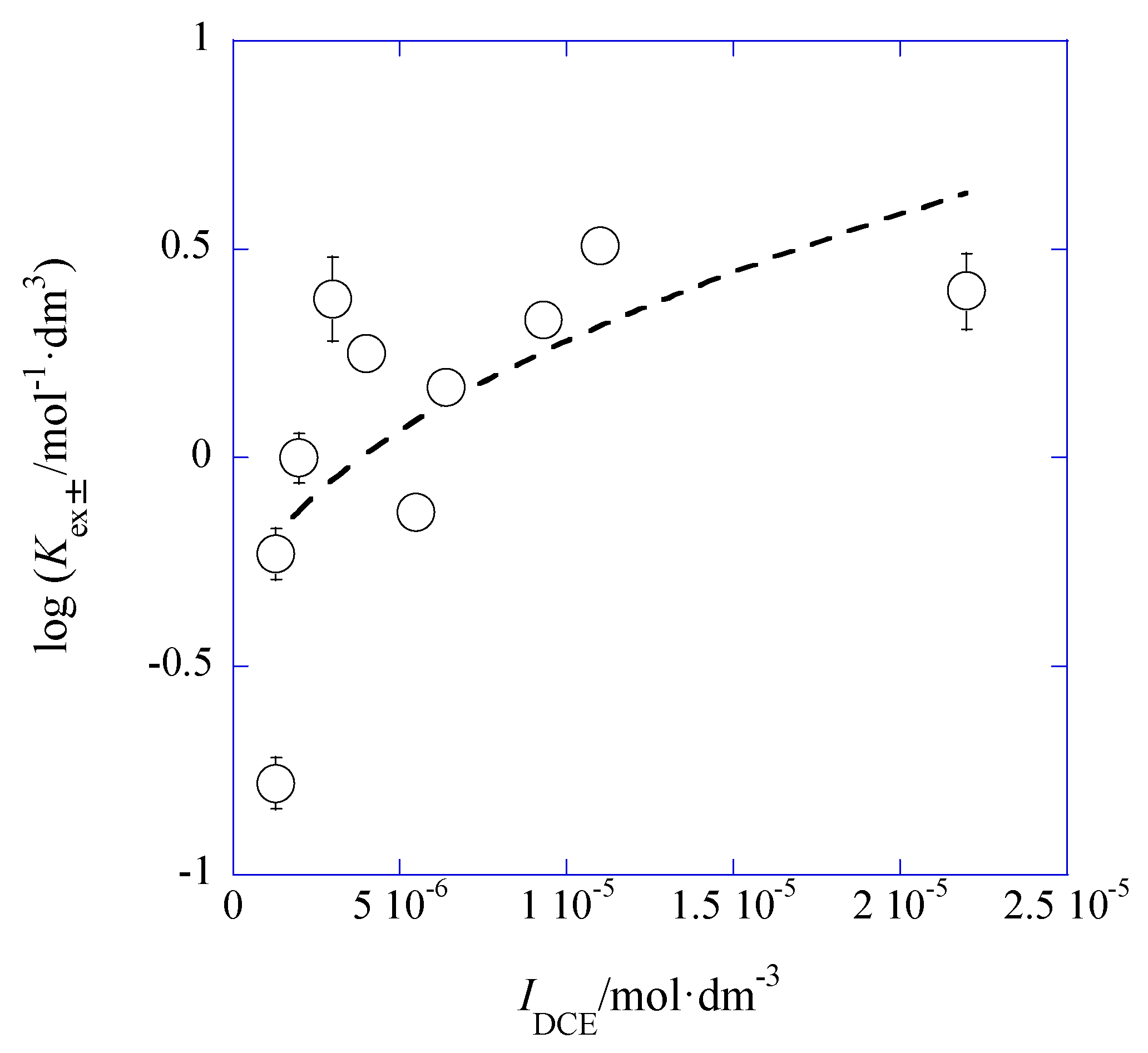
3.2. I_DCE Dependence of LogK_ex±

Applying the DH limiting law [14] for the system and rearranging Equation (6) at org = DCE, we can easily obtain

$$\log K_{\mathrm{ex}\pm }\approx \log {K_{\mathrm{ex}\pm }}^0\prime \prime + 2A_{\mathrm{DCE}}\sqrt{I_{\mathrm{DCE}}}.$$

(6b)

Hence, a plot of logK_ex± versus I_DCE yields logK_ex±⁰′′ and A_DCE values immediately. Here, K_ex±⁰′′ is defined as a_ML,DCEa_A,DCE/([M⁺][L]_DCE[A⁻]) (=y₊y₋K_ex±⁰). Figure 7 shows its plot for the AgPic-18C6 extraction systems with DCE.

Also the average values of I_DCE were used for the plot (see Section 3.1) and the y₊y₋ value in K_ex±⁰′′ was estimated on average (N = 10) to be 0.76 ± 0.14. This product was calculated from the ion size parameters, a(Ag⁺) = 2.5 and a(Pic⁻) = 7 Å, in water [15]. A plot analysis gave logK_ex±⁰′′ = −0.45 ± 0.24 and A_DCE = 116 ± 46 mol^−1/2·dm^3/2 at r = 0.665. Accordingly, introducing y₊y₋ in logK_ex±⁰′′ = logy₊y₋ + logK_ex±⁰, the logK_ex±⁰ value became –0.36 ± 0.24. The experimental A_DCE value was much larger than its theoretical one (= 10.6 mol^−1/2·dm^3/2) for a pure DCE at 298 K. This difference between these A_DCE values may be due to simple errors caused by the narrow experimental I_DCE-range of (0.097–2.2) × 10⁻⁵ mol·dm⁻³ or to the condition where the diluent DCE was saturated with water.

3.3. I_DCE Dependences of LogK_1,DCE

The thermodynamic ion-pair formation constant (K_1,org⁰) at I_org → 0 is described as

logK_1,org⁰ = log([MLA]_org/a_ML,orga_A,org) = logK_1,org − log(y_ML,orgy_−,org)

(7)

Rearranging this equation at org = DCE and ML⁺ = AgB18C6⁺ can give the following equation:

$$\log K_{1,\mathrm{DCE}}= \log {K_{1,\mathrm{DCE}}}^0+ \log (y_{\mathrm{AgB}18\mathrm{C}6,\mathrm{DCE}}{y}_{-,\mathrm{DCE}}) \approx \log {K_{1,\mathrm{DCE}}}^0- 2A_{\mathrm{DCE}}\sqrt{I_{\mathrm{DCE}}}$$

(7a)

A plot of logK_1,DCE versus I_DCE is shown in Figure 8. The plot analysis yielded the regression line with logK_1,DCE⁰ = 5.89 ± 0.19 and A_DCE = 152 ± 37 mol^−1/2·dm^3/2 at r = 0.821 and N = 10. This A_DCE value overlaps with the value (= 116) determined above (see Section 3.2), within the calculation error (= 46) and much larger than the theoretical one too. The authors cannot clearly explain the larger experimental A_DCE values, as similar to Section 3.2.

The logarithmic value, logK_1,DCE^av, of simple average-K_1,DCE one was 5.36 ± 0.42 in the I_DCE range of (0.097-2.2) × 10⁻⁵ mol·dm⁻³ at N = 10 and was smaller than the logK_1,DCE⁰ value (= 5.9 at I → 0). Although the experimental I_DCE values were adequately small (I_DCE << 0.001), the magnitude of K_1,DCE decreased with an increase in I_DCE. Also, the logK_1,DCE^av value was smaller than the logK_AgPic,DCE one (= 6.0, see Section 2.1). From the logK_ex±⁰′ value (= 0.6) in Section 3.1 and the logK_ex±⁰ one (= −0.36) in 3.2, we obtained log(y_AgB18C6,DCEy⁻_,DCE) (= logK_ex±⁰ − logK_ex±⁰′) = −0.96 ± 0.27. Hence, the logK_1,DCE value was estimated to be 4.93 {= logK_1,DCE⁰ + log(y_AgB18C6,DCEy_−,DCE) = 5.89−0.96}, being somewhat smaller than the logK_1,DCE^av value (= 5.4). These facts indicate that the logK_1,DCE^av value is not properly reflective of the logK_1,DCE one in Equation (7).

On the other hand, the log(K_AgB18C6,DCE/mol⁻¹·dm³) values were calculated from the relation logK_ML,org = logK_ex± − logK_D,M^S⋅K_D,A^S for a given I_DCE. Here, we assumed that, considering the smaller I_DCE values, the ratio, y_Ml,DCE/y_+,DCE, of the activity coefficients in the thermodynamic complex-formation constant, K_ML,DCE⁰, equals unity. Accordingly, the approximation that an average value among the K_AgB18C6,DCE ones equals the K_AgB18C6,DCE⁰ value becomes valid. Consequently, as its logarithmic value, 7.77 ± 0.25 was obtained on average (N = 12) at 298 K.

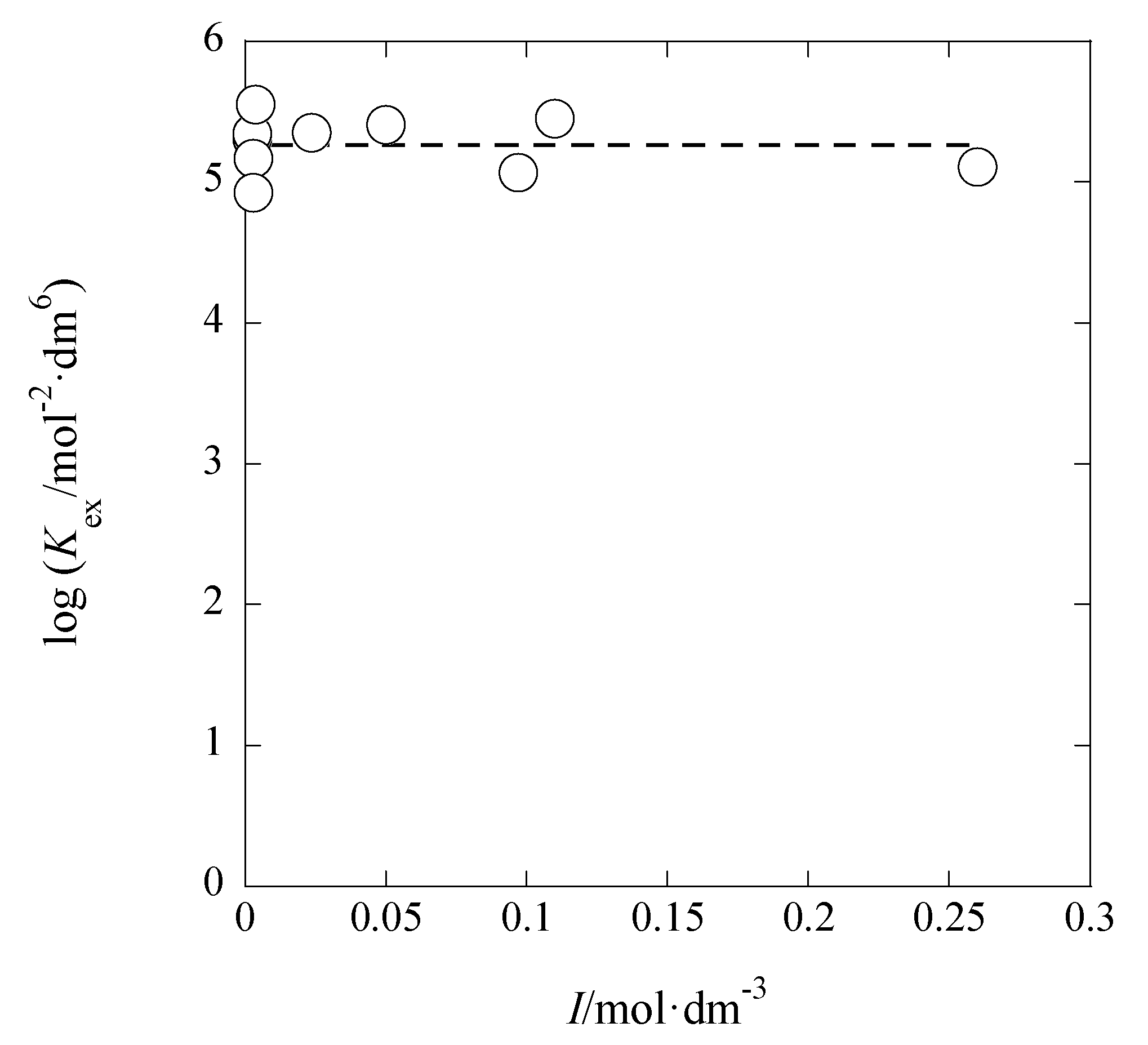
3.4. A Trend between LogK_D,Pic and Log(I_DCE/I)

From a plot of logK_D,Pic versus log(I_DCE/I), we obtained a theoretical line of logK_D,Pic = log(I_DCE/I) − (0.09 ± 0.12) at r = 0.398 (Figure 9) under the condition of the fixed slope of unity, except for the points in the I range of 0.024-0.26 mol·dm⁻³. This trend suggests that the K_D,Pic values are basically proportional to the ratios of (I_DCE/I), as reported before [16]. The deviation of the five points in Figure 9 can come from an effect of the higher I values in (I_DCE/I). An answer to this deviation is explained as follows.

For the case of only MA (or mixture of MX with small excess organic acid, HA) in w phase with L, we can propose the following equation for I and I_org.

I = (1/2)([M⁺] + [ML⁺] + [A⁻]) = [A⁻]

(8)

with the charge balance equation of [M⁺] + [ML⁺] = [A⁻]. Similarly, the authors can obtain

I_org = (1/2)([M⁺]_org + [ML⁺]_org + [A⁻]_org) ≈ [A⁻]_org

(8a)

in the org phase. The assumption that [AgB18C6⁺]_DCE is much larger than [Ag⁺]_DCE, namely [AgB18C6⁺]_DCE ≈ [Pic⁻]_DCE, was employed for the experiments. From Equations (8) and (8a), one can see easily the experimental relation that (I_DCE/I) is basically proportional to K_D,Pic.

On the other hand, for the present case of mixture of MX with the small excess HA and excess HX (strong acid) in the w phase with L, the corresponding equations are

I = (1/2)([M⁺] + [H⁺] + [ML⁺] + [HL⁺] + [A⁻] + [X⁻]) ≈ [A⁻] + [X⁻]

(9)

and

I_org = (1/2)([M⁺]_org + [H⁺]_org + [ML⁺]_org + [HL⁺]_org + [A⁻]_org + [X⁻]_org) ≈ [A⁻]_org

(9a)

with the assumptions that [M⁺] + [ML⁺] + [H⁺] ≈ [A⁻] + [X⁻] and [M⁺]_org + [H⁺]_org + [ML⁺]_org + [HL⁺]_org ≈ [A⁻]_org (>> [X⁻]_org), respectively. Therefore, Equations (9) and (9a) give the experimental relation of

(I_DCE/I) ≈ [Pic⁻]_DCE/([Pic⁻] + [NO₃⁻]) = K_D,Pic/(1 + [NO₃⁻]/[Pic⁻])

(9b)

and then its ratio becomes smaller than the K_D,Pic value in the case of [NO₃⁻] >> [Pic⁻]. When [NO₃⁻] nearly equals [Pic⁻] in Equation (9b), the log(I_DCE/I) value deviates from the logK_D,Pic one by +0.3: that is, (I_DCE/I) ≈ K_D,Pic/2. However, such a deviation is comparable to experimental errors. Thus, Equation (9b) explains well the deviation of the five points from the regression line in Figure 9. Obviously, the deviation becomes larger, when an excess of HX, such as HNO₃, was added in the w phase and X⁻ less distribute into the org phase than A⁻ does (for example, see the K_D,Pic^S & K_D,NO3^S values in

Table 3. Log K_ex± values evaluated from the logK_D,A^S and logK_Ag/AgL^S values at B18C6 (= L), DCE, and 298 K.

A−	logKD,AS 1	logKex±
		Evaluated 2	Experimental
Cl−	−7.99, −8.135 3	−6.69	- 4
Br−	−6.57, −6.74 3	−5.27	- 4
N3−	−6.42	−5.12	- 4
NO3−	−5.91, −5.94 3	−4.61, −4.64 3	−4.40
I−	−4.50, −4.62 3	−3.20	- 4
SCN−	−4.21, −4.47 3	−2.91	- 4
MnO4−	−3.33	−2.03	- 5
ClO4−	−2.84, −3.00 3	−1.54, −1.70 3	−1.24
Pic− 6	−1.01 1	- 7	0.35 ± 0.12 8 −0.36 ± 0.24 9

¹ Ref. [12]; ² Values calculated from logK_ex± = 1.30 + logK_D,A^S {see Equation (11)}. They have the error of 0.3 at least because of the standard deviation of logK_Ag/AgL^S; ³ Ref. [17]; ⁴ Not determinable, probably because of precipitation etc. See the text; ⁵ Not determined here; ⁶ The K_Ag/AgL^S value was determined, based on the data of the extraction experiments. See Table 1; ⁷ Not evaluated; ⁸ Average value in the I range of (2.5–3.6) × 10⁻³ mol·dm⁻³. See Table 1; ⁹ Value at I_DCE → 0. See the text.

).

3.5. Δφ_eq Dependences of LogK_M/ML and LogK_ex±

The logK_M/ML, defined as log([ML⁺]_org/[M⁺][L]_org) [8], can be resolved as follows and calculated from logK_ex± − logK_D,A.

logK_M/ML = logK_D,M + logK_ML,org = 16.90Δφ_eq + logK_D,M^S·K_ML,org

(10)

at 298 K with

logK_D,M = (F/2.303RT)Δφ_eq + logK_D,M^S

(10a)

Here, the term of log(K_D,M^S⋅K_ML,org) has to be a constant, because these two equilibrium constants are independent of Δφ_eq; logK_ML,org = −(F/2.303RT) (standard formal potential of the ML⁺ formation in the org phase) [8]. The magnitude of the K_M/ML (or K_M/ML^S) value shows an incorporation-ability into the org phase of L against M⁺. Then, the plot of logK_M/ML versus Δφ_eq based on Equation (10) can yield a straight line with the slope of 17 V⁻¹ and the intercept of logK_D,M^S⋅K_ML,org.

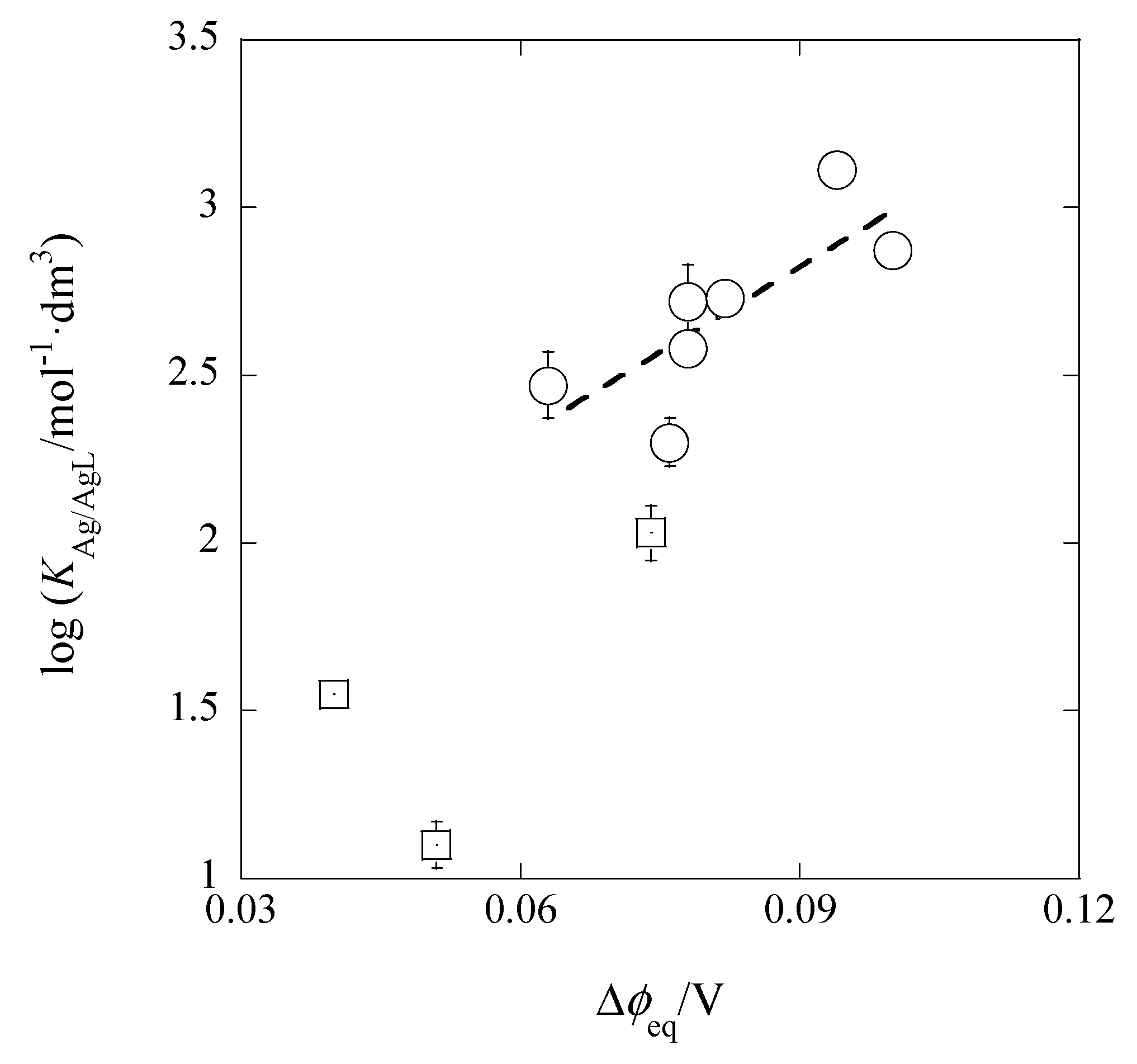
Figure 10 shows its plot, of which the experimental regression line was logK_M/ML = (16.8 ± 6.1)Δφ_eq,Pic + (1.30 ± 0.50) at r = 0.775 and N = 7. Here, the three data (the squares in Figure 10) were neglected from the calculation of the line, because their I values were much larger than the values of the extraction systems without the presence of excess HNO₃ in the w phase (see Table 1 and Table 2). The Δφ_eq,Pic values show the Δφ_eq ones obtained from the experimental logK_D,Pic values. The predictable intercept value was calculated to be 1.30 (= logK_D,Ag^S + logK_AgB18C6,DCE = −6.47 + 7.77) ± 0.25, being in accord with the experimental value. Similarly, the slope value was in good agreement with its theoretical one (= 17). These facts indicate that the Δφ_eq,Pic values essentially correspond to the Δφ_eq ones in logK_D,Ag {see Equation (10a)}. In other words, the relation of logK_Ag/AgB18C6 = (slope) × Δφ_eq,Pic + logK_D,Ag^S·K_AgB18C6,DCE is satisfied.

A plot of logK_ex± versus Δφ_eq for the A⁻ = Pic⁻, ClO₄⁻, and NO₃⁻ systems also gave a regression line with a slope of 19.3 ± 2.0 V⁻¹ and an intercept of −1.23 ± 0.18 at r = 0.974 and N = 7 in the narrow I range (see Table 1 for their basic data). This slope was very close to the theoretical value (= 17). As similar to the previous results [11], these results indicate that the plot satisfies the relation of logK_ex± = 16.90Δφ_eq + logK_D,A + logK_D,Ag^S·K_AgB18C6,DCE (= 16.90Δφ_eq + logK_D,A + 1.30). The (logK_D,A + 1.3) term corresponds to the intercept [11] within ±0.3 at least.

3.6. Evaluation of LogK_ex± Based on LogK_D,A^S

Using the logK_M/ML^S and logK_D,A^S values, we can immediately evaluate the logK_ex± value as follows. A thermodynamic cycle gives

logK_ex± = logK_M/ML^S + logK_D,A^S = logK_M/ML + logK_D,A

(11)

by using Equations (10a) and (4). It is difficult to accurately determine the K_D,M^S {or K_D,M(Δφ_eq): the function of Δφ_eq, see Equation (10a)} and K_ML,org values. On the other hand, it is comparatively easy to determine the K_M/ML^S value. So, if the logK_M/ML^S {or logK_M/ML(Δφ_eq)} value is determined for given ML⁺ and diluent, then the logK_ex± values can be calculated from Equation (11) with the logK_D,A^S {or logK_D,A(Δφ_eq)} ones. In this study, we determined the logK_Ag/AgL^S value to be 1.30 from the data (see Table 1) of the AgPic-B18C6 extraction systems with DCE. Calculated logK_ex± values for some A⁻ are listed in Table 3, together with several experimental values.

The determination of the K_ex± values will be experimentally difficult for the Cl⁻ to I⁻ extraction systems, because of the precipitation [18] of their silver salts. The same is true of the SCN⁻ extraction system, because of its low solubility product (= 1.0 × 10⁻¹² mol²·dm⁻⁶ [18]) against Ag⁺ in water. Also, AgN₃, which is a white insoluble crystal, is explosive [19]. Therefore, the experimental K_ex± values were determined at 298 K for the ClO₄⁻ and NO₃⁻ extraction systems (see Table 1). Considering the differences (0.03–0.26) between the logK_D,A^S values at a fixed A⁻ in Table 3 and the standard deviation (= 0.3) of the logK_Ag/AgB18C6^S value, these experimentally-obtained logK_ex± values are very close to the values evaluated here.

Similar results were obtained for the NaA-B18C6 extraction into DCE. Their logK_ex± values evaluated from logK_Na/NaB18C6^S = 0.53, which were calculated here, were −2.8 for A⁻ = MnO₄⁻ and −2.3 for ClO₄⁻. Their experimental logK_ex± values were −2.23 [13] at I = 0.0077 mol·dm⁻³ for MnO₄⁻ and −3.65 ± 0.07 at 0.074 for ClO₄⁻ of which the value was re-calculated from the data reported before [8]. These differences, ≤ ∣1.4∣, between the evaluated and experimental values were larger than those for the AgA-B18C6 extraction systems.

The above fact indicates that the logK_ex± values can be evaluated from a sum of the logK_Ag/AgB18C6^S and logK_D,A^S (or logK_Ag/AgB18C6 & logK_D,A) ones. Namely, the order, A⁻ = NO₃⁻ < ClO₄⁻ << Pic⁻, in logK_ex± for a given extraction system at fixed AgB18C6⁺ and DCE is fundamentally predicted from that of logK_D,A^S (see Table 3). Thus, for the systems where the extraction experiments are difficult, the present procedure becomes easy to evaluate the logK_ex± values. Also, the experimental intercepts (≈ logK_ex± [13]) of the straight lines in Figure 2 support this order: the intercepts were −4.05 for A⁻ = NO₃⁻, −0.92 for ClO₄⁻, and 2.20 for Pic⁻.

4. Materials and Methods

4.1. Materials

An aqueous solution of a commercial Ba(OH)₂·8H₂O (≥98%, Wako Pure Chemical Industries, Tokyo, Japan) and a solution with 2 equivalents of HPic·nH₂O (≥99.5%, Wako Pure Chemical Industries, Tokyo, Japan) were mixed, that of Ag₂SO₄ (≥99.5%, Kanto Chemicals Co. Ltd., Tokyo, Japan) was added in the mixture, and immediately BaSO₄ precipitated. After standing the mixture overnight, the thus-obtained yellow solution with the precipitate was filtered and then its filtrate was evaporated by a rotary evaporator (type RE1-N, Iwaki, Japan) in order to deposit a fine yellow crystal. The crystal obtained was filtered and dried in vacuo for 21 h. Found: 97.29% for Ag by the AAS measurements at 328.1 nm; 101% for Pic⁻ by spectrophotometric measurements at 355.0 nm. An amount of water in the AgPic crystal was not detected by a Karl-Fischer titration. This crystal was employed only for the AgPic extraction experiments without B18C6.

Concentrations of the aqueous solution with AgNO₃ (≥99.8%, Kanto Chemicals Co. Ltd., Tokyo, Japan) and that with AgClO₄ (97%, Aldrich, Missouri, MO, USA) were determined by a precipitation titration with NaCl (99.98% ± 0.01%, Wako: standard reagent for volumetric analysis, Wako Pure Chemical Industries, Tokyo, Japan). A commercial DCE (guaranteed-pure reagent, Kanto Chemicals Co. Ltd., Tokyo, Japan) was treated with the same procedure as that described previously [13,16]. All other chemicals used in this study were of guaranteed-pure reagent grade.

4.2. Extraction Experiments

Procedures for both the AgPic extraction experiments and the AgA extraction ones with B18C6 into DCE were essentially the same as those [8,20] reported before. The latter experiments were performed by using mixtures of AgNO₃ with HPic in the w phases. The total concentration range of Ag(I) was 0.00041 to 0.043 mol·dm⁻³ for the AgPic extraction and the ranges of AgNO₃, HPic, and B18C6 were (1.5 or 5.0) × 10⁻⁴, (3.3 or 3.4) × 10⁻³, and (0.4–7.5) × 10⁻⁴ mol·dm⁻³, respectively, for the Ag(I) extraction with B18C6. The extraction of AgB18C6⁺ with NO₃⁻ or Ag(B18C6)NO₃ was negligible, compared with that of AgB18C6⁺ with Pic⁻ or Ag(B18C6)Pic (see Figure 2 or the logK_ex± and logK_ex values in Table 1). After the extraction operations, the w phases were in the pH ranges of 2.68–2.74 at the system of I = 0.0025 mol·dm⁻³ and 2.70–3.37 at that of 0.0031 (see Table 1).

For the AgPic extraction by B18C6 into DCE in the presence of “excess HNO₃“ in the w phases, the total concentrations of AgNO₃ and HPic were fixed at 1.5 × 10⁻⁴ and 0.0033 or 0.0034 mol·dm⁻³, respectively. Under this condition, the total concentration, [HNO₃]_t, of HNO₃ in the w phase was changed in the range of 0.025 to 0.25 mol·dm⁻³. After the extraction operations, the w phases were in the pH ranges of 1.64 and 1.65 at [HNO₃]_t = 0.025 mol·dm⁻³, 1.34–1.38 at 0.050, 1.06 at 0.10, and 0.62–0.68 at 0.25.

Used apparatus, such as the atomic absorption spectrophotometer, UV-V, centrifuge, mechanical shaker, and pH meter with the glass electrode, were the same as those [8,16] described previously.

5. Conclusions

The I or I_DCE dependences of the logK_ex± and logK_ex values for the present extraction systems were clarified experimentally. Their experimental equations were logK_ex = 5.3 – 2×0.51$\sqrt{I}/(1+44\sqrt{I}$) and logK_ex± = 0.6 − 2×2.0$\left\{\sqrt{I}/\left(1+\sqrt{I}\right)-0.3I\right\}$ = −0.5 + 2×116$\sqrt{I_{\mathrm{DCE}}}$. However, the magnitudes of these changes in logK_ex± or logK_ex with I or I_DCE were insignificant in practical separation. It was also suggested that the style of M(I) employed in the extraction experiments with L, either the simple salt MPic or the mixture of MX with HPic and excess HX, largely control whether the logK_D,Pic values are dependent on the log(I_DCE/I ) ones or not. The logK_ex±-versus-Δφ_eq plot for the Pic⁻, ClO₄⁻, and NO₃⁻ systems yielded the good positive correlation. On the basis of the plot of logK_Ag/AgB18C6 versus Δφ_eq,Pic, it was indirectly proved that the Δφ_eq values obtained from the K_D,Pic ones is common to those from the K_D,Ag values. Moreover, the logK_ex± values were predicted from the sum of the logK_D,A^S and logK_Ag/AgB18C6^S ones for given MB18C6⁺ and DCE at least. At the same time, the order in K_ex± reflected that in K_D,A^S.