La2C2@D5(450)-C100: Calculated High Energy Gain in Encapsulation
Abstract
1. Introduction
2. Calculations
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bochvar, D.A.; Galpern, E.G. Hypothetical systems: Carbododecahedron, s-Icosahedron, and carbo-s-Icosahedron. Dokl. Akad. Nauk SSSR 1973, 209, 610–612. [Google Scholar]
- Pitzer, K.S.; Clementi, E. Large molecules in carbon vapor. J. Am. Chem. Soc. 1959, 81, 4477–4485. [Google Scholar] [CrossRef]
- Slanina, Z. Remark on the present applicability of quantum chemistry to the calculations of equilibrium and rate constants of chemical reactions. Radiochem. Radioanal. Lett. 1975, 22, 291–298. [Google Scholar]
- Slanina, Z.; Lee, S.-L.; Yu, C.-H. Computations in treating fullerenes and carbon aggregates. Rev. Comput. Chem. 1996, 8, 1–62. [Google Scholar]
- Zhao, X.; Slanina, Z.; Goto, H. Theoretical studies on the relative stabilities of C96 IPR fullerenes. J. Phys. Chem. A 2004, 108, 4479–4484. [Google Scholar] [CrossRef]
- Zhao, X.; Slanina, Z. C98 IPR isomers: Gibbs-energy based relative stabilities. J. Mol. Struct. (Theochem) 2003, 636, 195–201. [Google Scholar] [CrossRef]
- Zhao, X.; Goto, H.; Slanina, Z. C100 IPR fullerenes: Temperature-dependent relative stabilities based on the Gibbs function. Chem. Phys. 2004, 306, 93–104. [Google Scholar] [CrossRef]
- Diener, M.D.; Alford, J.M. Isolation and properties of small-bandgap fullerenes. Nature 1998, 393, 668–671. [Google Scholar] [CrossRef]
- Mercado, B.Q.; Jiang, A.; Yang, H.; Wang, Z.; Jin, H.; Liu, Z.; Olmstead, M.M.; Balch, A.L. Isolation and structural characterization of the molecular nanocapsule Sm2@D3d(822)-C104. Angew. Chem. Int. Ed. 2009, 48, 9114–9116. [Google Scholar] [CrossRef]
- Beavers, C.M.; Jin, H.; Yang, H.; Wang, Z.; Wang, X.; Ge, H.; Liu, Z.; Mercado, B.Q.; Olmstead, M.M.; Balch, A.L. La2C90 to La2C138: Isolation and crystallographic characterization of La2@D5(450)-C100. J. Am. Chem. Soc. 2011, 133, 15338–15341. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Fortea, A.; Balch, A.L.; Poblet, J.M. Endohedral metallofullerenes: A unique host-guest association. Chem. Soc. Rev. 2011, 40, 3551–3563. [Google Scholar] [CrossRef] [PubMed]
- Popov, A.A.; Yang, S.; Dunsch, L. Endohedral fullerenes. Chem. Rev. 2013, 113, 5989–6113. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.; Wang, S.; Troyanov, S.I. The most stable isomers of giant fullerenes C102 and C104 captured as Chlorides, C102(603)Cl18/20 and C104(234)Cl16/18/20/22. Chem. Eur. J. 2014, 20, 6875–6878. [Google Scholar] [CrossRef] [PubMed]
- Cai, W.; Bao, L.; Zhao, S.; Xie, Y.; Akasaka, T.; Lu, X. Anomalous compression of D5(450)-C100 by encapsulating La2C2 Cluster Instead of La2. J. Am. Chem. Soc. 2015, 137, 10292–10296. [Google Scholar] [CrossRef] [PubMed]
- Cai, W.; Li, F.-F.; Bao, L.; Xie, Y.; Lu, X. Isolation and crystallographic characterization of La2C2@Cs(574)-C102 and La2C2@C2(816)-C104: Evidence for the top-down formation mechanism of fullerenes. J. Am. Chem. Soc. 2016, 138, 6670–6675. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Yang, S.; Kemnitz, E.; Troyanov, S.I. New giant fullerenes identified as chloro derivatives: Isolated-pentagon-rule C108(1771)Cl12 and C106(1155)Cl24 as well as nonclassical C104Cl24. Inorg. Chem. 2016, 55, 5741–5743. [Google Scholar] [CrossRef] [PubMed]
- Pan, C.; Bao, L.; Yu, X.; Fang, H.; Xie, Y.; Akasaka, T.; Lu, X. Facile Access to Y2C2n (2n = 92–130) and crystallographic characterization of Y2C2@C1(1660)-C108: A giant nanocapsule with a linear carbide cluster. ACS Nano 2018, 12, 2065–2069. [Google Scholar] [CrossRef] [PubMed]
- Slanina, Z.; Uhlík, F.; Pan, C.; Akasaka, T.; Lu, X.; Adamowicz, L. Computed stabilization for a giant fullerene endohedral: Y2C2@C1(1660)-C108. Chem. Phys. Lett. 2018, 710, 147–149. [Google Scholar] [CrossRef]
- Shao, N.; Gao, Y.; Yoo, S.; An, W.; Zeng, X.C. Search for lowest-energy fullerenes: C98 to C110. J. Phys. Chem. A 2006, 110, 7672–7676. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Cao, X.Y.; Dolg, M. Segmented contraction scheme for small-core lanthanide pseudopotential basis sets. J. Mol. Struct. (Theochem) 2002, 581, 139–147. [Google Scholar] [CrossRef]
- Boys, S.F.; Bernardi, F. The calculation of small molecular interactions by the difference of separate total energies. Some procedures with reduced errors. Mol. Phys. 1970, 19, 553–566. [Google Scholar] [CrossRef]
- Slanina, Z.; Uhlík, F.; Lee, S.-L.; Adamowicz, L.; Akasaka, T.; Nagase, S. Computed stabilities in metallofullerene series: Al@C82, Sc@C82, Y@C82, and La@C82. Int. J. Quant. Chem. 2011, 111, 2712–2718. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Rev. D.01; Gaussian Inc.: Wallingford, CT, USA, 2013. [Google Scholar]
- Slanina, Z.; Uhlík, F.; Nagase, S.; Akasaka, T.; Adamowicz, L.; Lu, X. Eu@C72: Computed Comparable Populations of Two Non-IPR Isomers. Molecules 2017, 22, 1053. [Google Scholar] [CrossRef] [PubMed]
- Okubo, S.; Kato, T.; Inakuma, M.; Shinohara, H. Separation and characterization of ESR-active lanthanum endohedral fullerenes. New Diam. Front. Carbon Technol. 2001, 11, 285–294. [Google Scholar]
- Hehre, W.J. A Guide to Molecular Mechanics and Quantum Chemical Calculations; Wavefunction: Irvine, CA, USA, 2003; p. 435. [Google Scholar]
- Jensen, F. Introduction to Computational Chemistry; Wiley: Chichester, UK, 2017; p. 319. [Google Scholar]
- Slanina, Z.; Uhlík, F.; Lu, X.; Akasaka, T.; Lemke, K.H.; Seward, T.M.; Nagase, S.; Adamowicz, L. Calculations of the water-dimer encapsulations into C84. Fullerenes Nanotub. Carbon Nanostruct. 2016, 24, 1–7. [Google Scholar] [CrossRef]
- Yu, P.; Shen, W.; Bao, L.; Pan, C.; Slanina, Z.; Lu, X. Trapping an unprecedented Ti3C3 unit inside the icosahedral C80 fullerene: A crystallographic survey. Chem. Sci. 2019, 10, 10925–10930. [Google Scholar] [CrossRef] [PubMed]
- Slanina, Z.; Uhlík, F.; Lee, S.-L.; Wang, B.-C.; Adamowicz, L.; Suzuki, M.; Haranaka, M.; Feng, L.; Lu, X.; Nagase, S.; et al. Towards relative populations of non-isomeric metallofullerenes: La@C76(Td) vs. La2@C76(Cs,17490). Fullerenes Nanotub. Carbon Nanostruct. 2014, 22, 299–306. [Google Scholar] [CrossRef]
- Slanina, Z.; Uhlík, F.; Adamowicz, L. Theoretical predictions of fullerene stabilities. In Handbook of Fullerene Science and Technology; Lu, X., Akasaka, T., Slanina, Z., Eds.; Springer: Singapore, 2022; pp. 111–179. [Google Scholar]
- Slanina, Z. Breakdown of the conventional formula for the partition function of free internal rotation. J. Phys. Chem. 1982, 86, 4782–4786. [Google Scholar] [CrossRef]
- Slanina, Z.; Zhao, X.; Kurita, N.; Gotoh, H.; Uhlík, F.; Rudziński, J.M.; Lee, K.H.; Adamowicz, L. Computing the relative gas-phase populations of C60 and C70: Beyond the traditional scale. J. Mol. Graph. Mod. 2001, 19, 216–221. [Google Scholar] [CrossRef] [PubMed]
- Slanina, Z.; Uhlík, F.; Hu, S.; Akasaka, T.; Lu, X.; Adamowicz, L. Calculated isomeric populations of Er@C82. Fullerenes Nanotub. Carbon Nanostruct. 2024, 32. [Google Scholar] [CrossRef]
- Chase, M.W., Jr.; Davies, C.A.; Downery, J.R., Jr.; Frurip, D.J.; McDonald, R.A.; Syverud, A.N. JANAF thermochemical tables, Third edition, Vols. 1,2. J. Phys. Chem. Ref. Data 1985, 14 (Suppl. 1), 646. [Google Scholar]
- Gueorguiev, G.K.; Stafström, S.; Hultman, L. Nano-wire formation by self-assembly of silicon-metal cage-like molecules. Chem. Phys. Lett. 2008, 458, 170–174. [Google Scholar] [CrossRef]
- Basiuk, V.A.; Basiuk, E.V. Noncovalent complexes of Ih-C80 fullerene with phthalocyanines. Fullerenes Nanotub. Carbon Nanostruct. 2018, 26, 69–75. [Google Scholar] [CrossRef]
- Basiuk, V.A.; Tahuilan-Anguiano, D.E. Complexation of free-base and 3d transition metal(II) phthalocyanines with endohedral fullerene Sc3N@C80. Chem. Phys. Lett. 2019, 722, 146–152. [Google Scholar] [CrossRef]
- Wang, S.; Chang, Q.; Zhang, G.; Li, F.; Wang, X.; Yang, S.; Troyanov, S.I. Structural studies of giant empty and endohedral fullerenes. Front. Chem. 2020, 8, 607712. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Zhao, R.; Dang, J.; Zhao, X. Theoretical study on the stabilities, electronic structures, and reaction and formation mechanisms of fullerenes and endohedral metallofullerenes. Coor. Chem. Rev. 2022, 471, 214762. [Google Scholar] [CrossRef]
- Li, Y.B.; Biswas, R.; Kopcha, W.P.; Dubroca, T.; Abella, L.; Sun, Y.; Crichton, R.A.; Rathnam, C.; Yang, L.T.; Yeh, Y.-W.; et al. Structurally defined water-soluble metallofullerene derivatives towards biomedical applications. Angew. Chem. Int. Ed. Engl. 2023, 62, e202211704. [Google Scholar] [CrossRef] [PubMed]
- Menon, A.; Kaur, R.; Guldi, D.M. Merging carbon nanostructures with porphyrins. In Handbook of Fullerene Science and Technology; Lu, X., Akasaka, T., Slanina, Z., Eds.; Springer: Singapore, 2022; pp. 219–264. [Google Scholar]
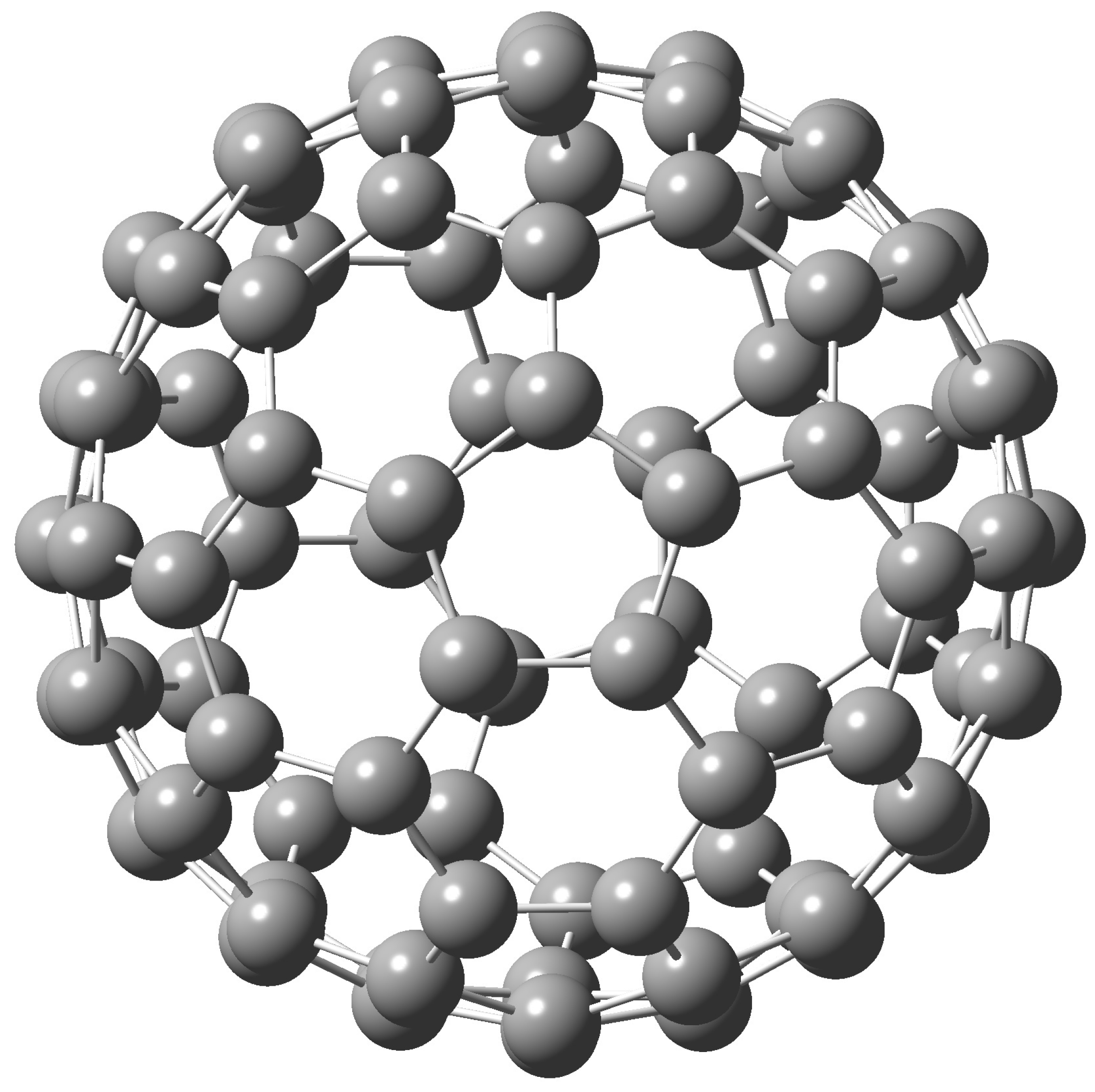
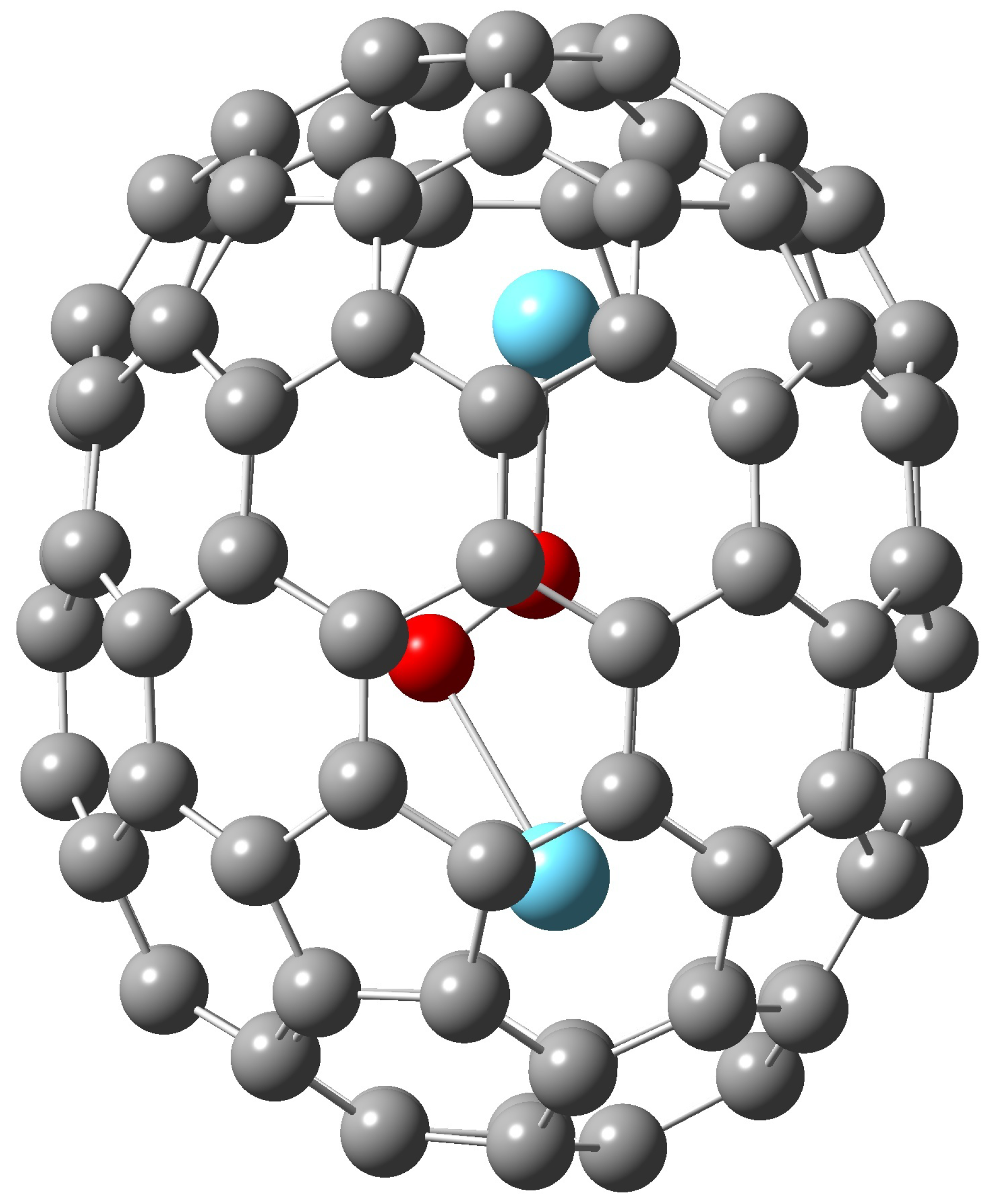
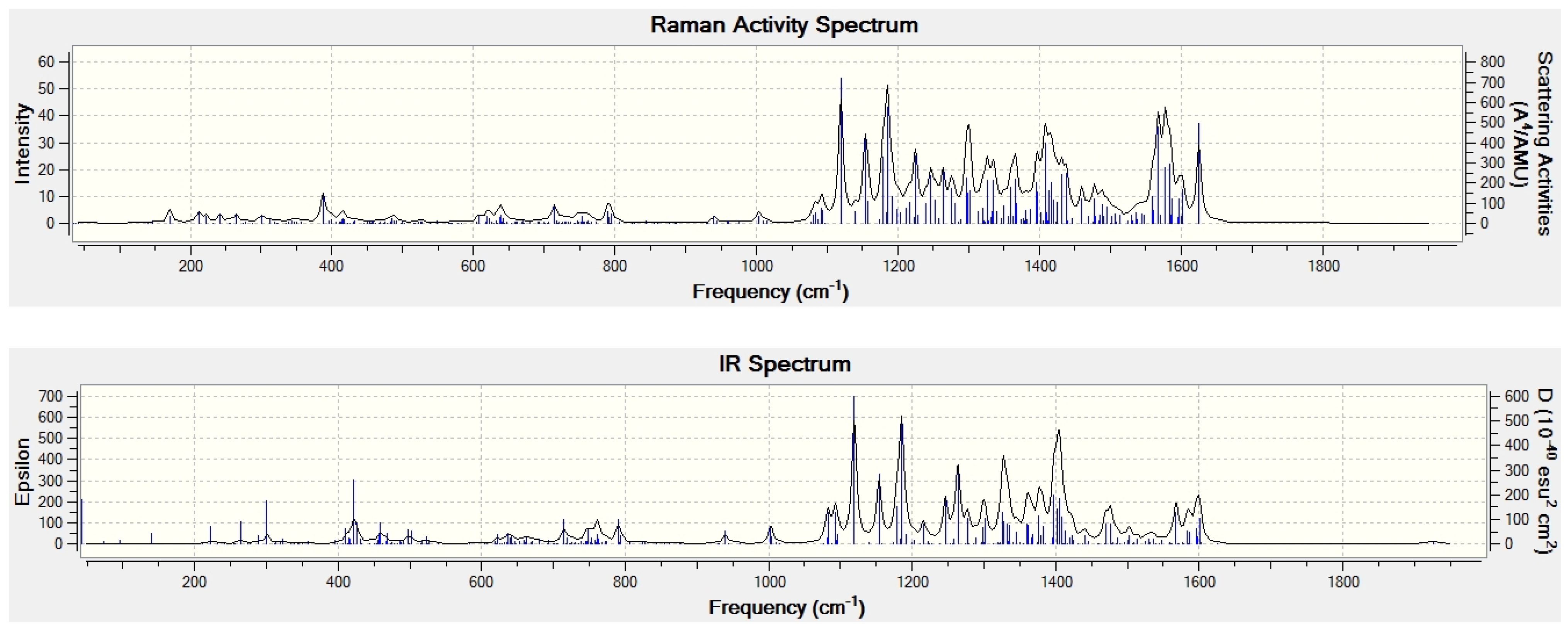
| Energy Term | (kcal/mol/atom) |
|---|---|
| without BSSE | −148.7 |
| with BSSE | −143.2 |
| with BSSE & steric | −140.2 |
| −138.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Slanina, Z.; Uhlík, F.; Akasaka, T.; Lu, X.; Adamowicz, L. La2C2@D5(450)-C100: Calculated High Energy Gain in Encapsulation. Inorganics 2024, 12, 196. https://doi.org/10.3390/inorganics12070196
Slanina Z, Uhlík F, Akasaka T, Lu X, Adamowicz L. La2C2@D5(450)-C100: Calculated High Energy Gain in Encapsulation. Inorganics. 2024; 12(7):196. https://doi.org/10.3390/inorganics12070196
Chicago/Turabian StyleSlanina, Zdeněk, Filip Uhlík, Takeshi Akasaka, Xing Lu, and Ludwik Adamowicz. 2024. "La2C2@D5(450)-C100: Calculated High Energy Gain in Encapsulation" Inorganics 12, no. 7: 196. https://doi.org/10.3390/inorganics12070196
APA StyleSlanina, Z., Uhlík, F., Akasaka, T., Lu, X., & Adamowicz, L. (2024). La2C2@D5(450)-C100: Calculated High Energy Gain in Encapsulation. Inorganics, 12(7), 196. https://doi.org/10.3390/inorganics12070196









