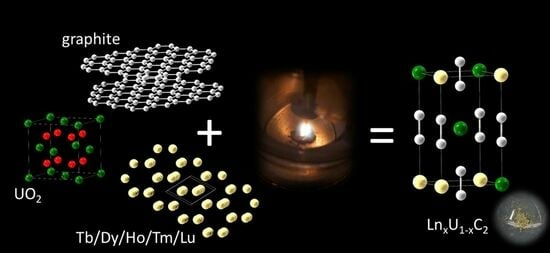
Solid Solutions LnxU1−xC2 with Ln = Tb, Dy, Ho, Tm, and Lu Showing Ideal Vegard Behavior
Abstract
:1. Introduction
- Valence changes are only observed for ytterbium but not for europium, which is solely found in its divalent state in these carbides.
- Ideal Vegard behavior is only observed for metal cations with very similar ionic radii, i.e., EuxSr1−xC2.
- No ternary phases with defined compositions have been found in any of these investigations.
- For large differences in the ionic radii of the constituting metal cations, either no complete miscibility was found, i.e., a miscibility gap was observed, or the cubic high-temperature modification was already observed at room temperature to reduce the lattice strain.
2. Results and Discussion
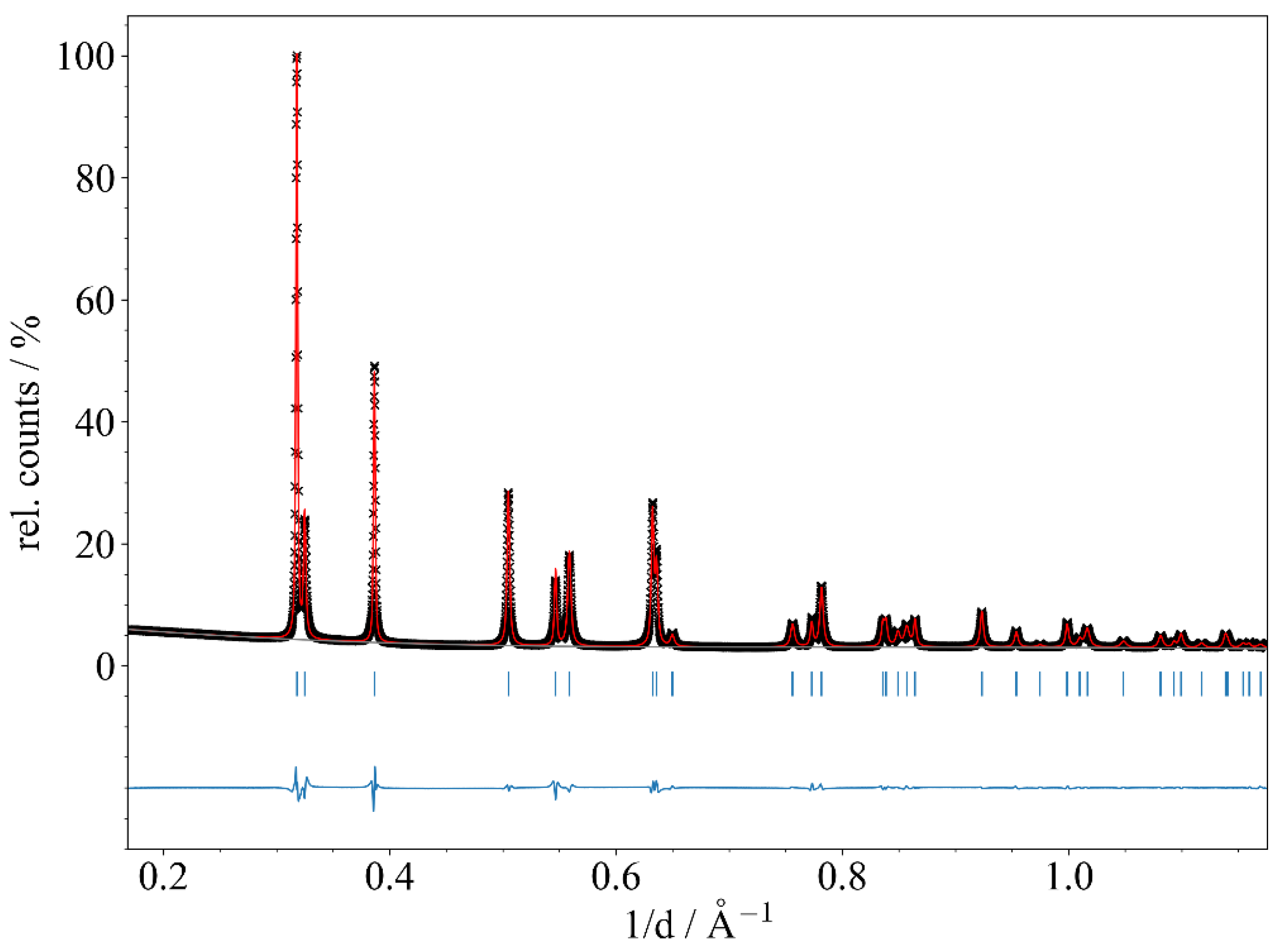
2.1. Synthesis and Structure Analysis
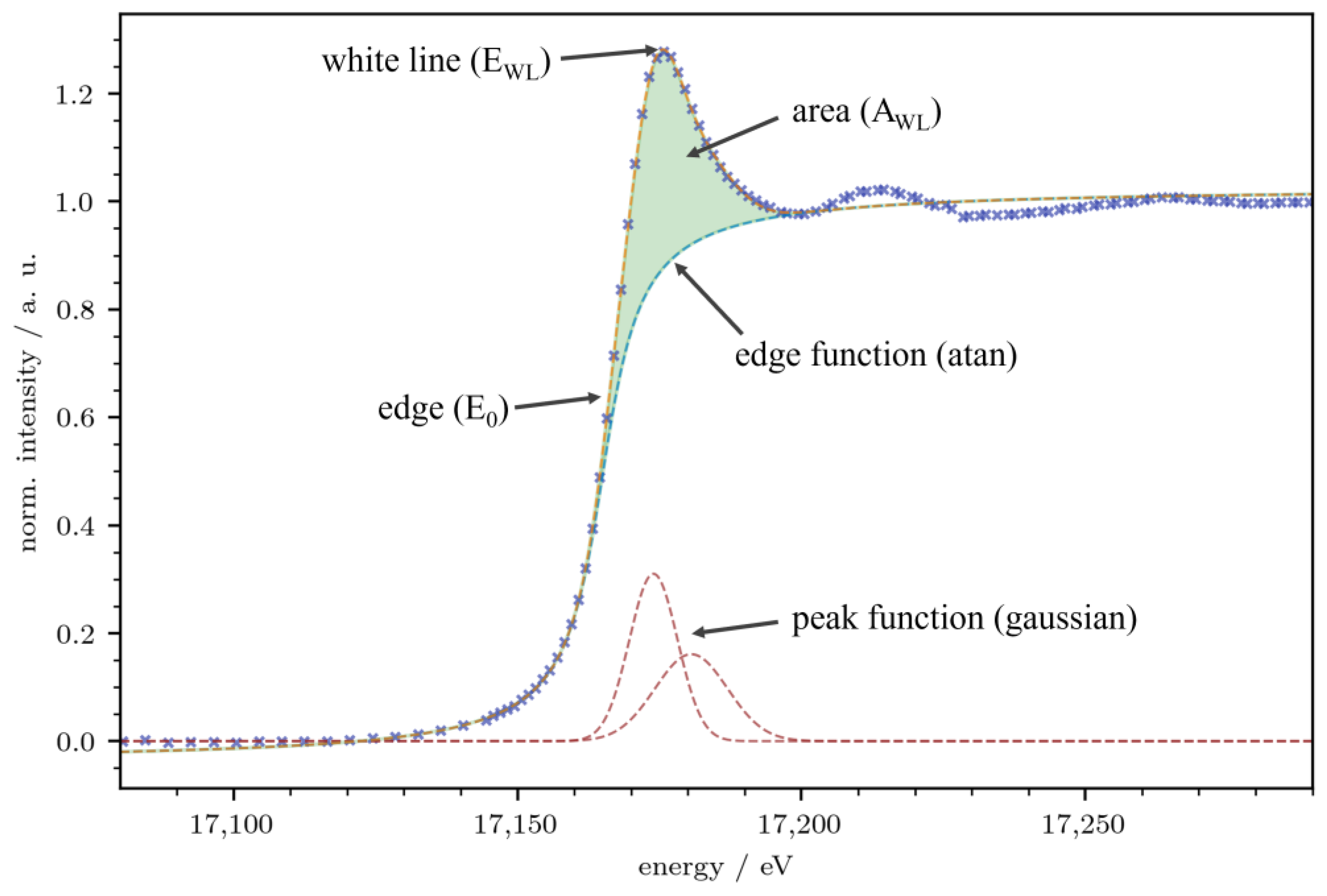
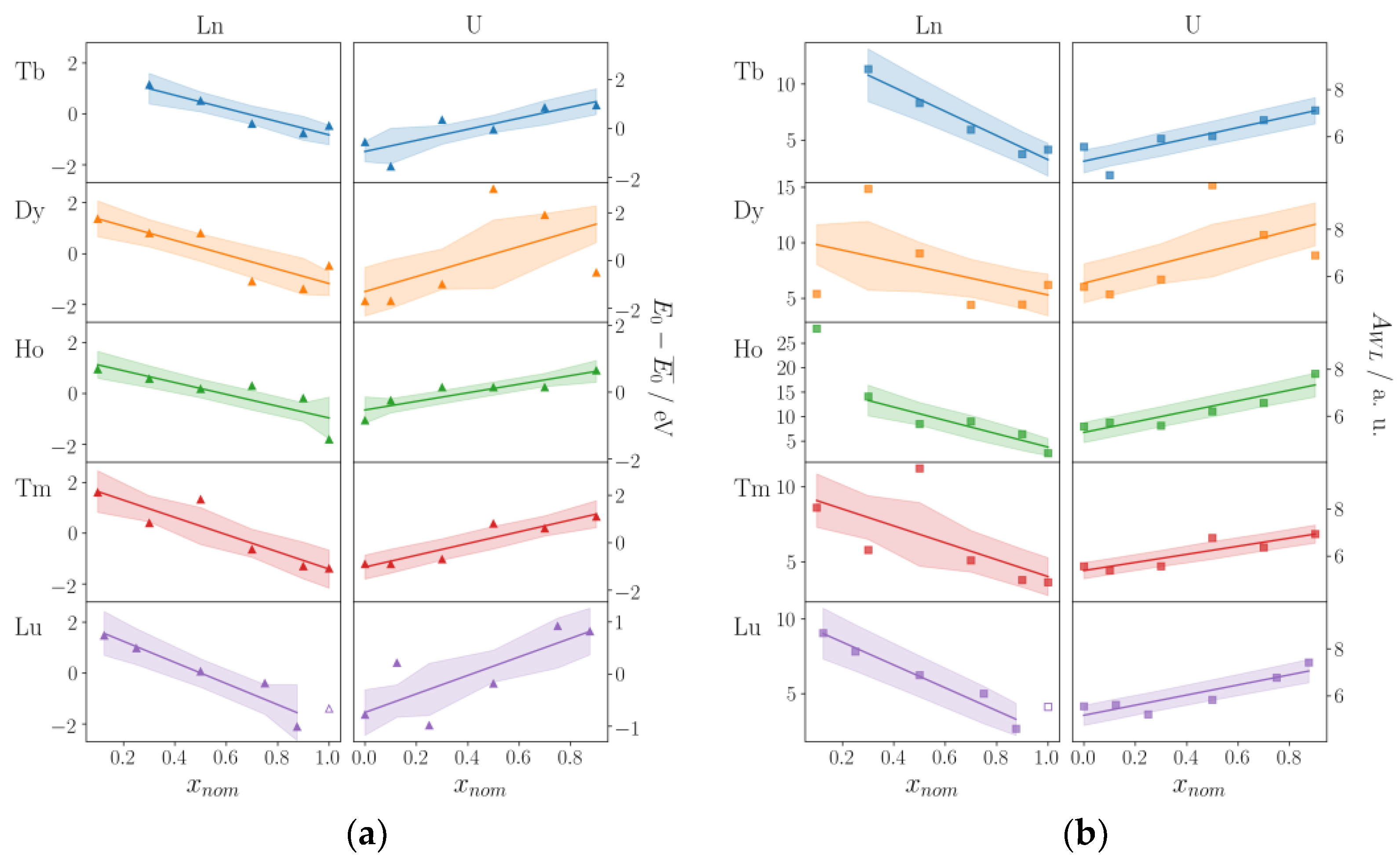
2.2. XANES Investigations
3. Materials and Methods
3.1. Synthesis
3.2. X-ray Powder Diffraction (XRPD)
3.3. Synchrotron Powder Diffraction
- (a)
- Beamline P02.1 of the DESY synchrotron radiation facility, Hamburg/Germany [36]: all samples were sealed in glass capillaries (∅ = 0.3 mm) in an argon atmosphere and were measured with the beamline area detector Varex XRD 4343CT (150 × 150 µm2 pixel size, 2880 × 2880 pixel area, CsI scintillator directly deposited on amorphous Si photodiodes) with a wavelength of λ = 0.20735 Å at room temperature. The resulting 2-dimensional TIFF images were processed into 1-dimensional diffraction data using the integration software Dioptas [37]. A LaB6 (NIST 660c) standard was measured in addition to conducting the detector calibration, which is required to perform the azimuthal integration with the Dioptas software. The WinXPow software package [35] was used for raw data handling and visual inspection of the data. The final diffraction patterns and refinements were visualized with Matplotlib [38] using a self-written Python script [39].
- (b)
- Beamline BL 09 of the DELTA synchrotron facility, Dortmund/Germany [40]: the measurements were performed in glass capillaries (sealed in an argon atmosphere, Ø = 0.3 mm) at room temperature with a wavelength of 0.4959 Å using a PILATUS 100 K detector (steps of 0.0825° in 2θ, 10 s integration time per data point, recording time: ~70 min). The WinXPow software package [35] was used for raw data handling and visual inspection of the data. The final diffraction patterns and refinements were visualized with Matplotlib [38] using a self-written Python script [39].
3.4. Le Bail Fits
3.5. EDX Analysis
3.6. XANES
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Von Stackelberg, M. Untersuchungen über Carbide. Z. Phys. Chem. Abt. B 1930, 9, 437–475. [Google Scholar] [CrossRef]
- Ruschewitz, U. Binary and ternary carbides of alkali and alkaline-earth metals. Coord. Chem. Rev. 2003, 244, 115–136. [Google Scholar] [CrossRef]
- Atoji, M. Neutron Diffraction Studies of CaC2, YC2, LaC2, CeC2, TbC2, YbC2, LuC2, and UC2. J. Chem. Phys. 1961, 35, 1950–1960. [Google Scholar] [CrossRef]
- Rundle, R.E.; Baenziger, N.C.; Wilson, A.S.; McDonald, R.A. The Structures of the Carbides, Nitrides and Oxides of Uranium. J. Am. Chem. Soc. 1948, 70, 99–105. [Google Scholar] [CrossRef]
- Jilek, R.E.; Bauer, E.; Burrell, A.K.; McCleskey, T.M.; Jia, Q.X.; Scott, B.L.; Beaux, M.F.; Durakiewicz, T.; Joyce, J.J.; Rector, K.D.; et al. Preparation of Epitaxial Uranium Dicarbide Thin Films by Polymer-Assisted Deposition. Chem. Mater. 2013, 25, 4373–4377. [Google Scholar] [CrossRef]
- Bernal, J.D.; Djatlowa, E.; Kasarnowsky, I.; Reichstein, S.; Ward, A.G. The Structure of Strontium and Barium Peroxides SrO2 and BaO2. Z. Kristallogr. 1935, 92, 344–354. [Google Scholar] [CrossRef]
- Ziegler, M.; Rosenfeld, M.; Kaenzig, W.; Fischer, P. Strukturuntersuchungen an Alkalihyperoxiden. Helv. Phys. Acta 1976, 49, 57–90. [Google Scholar] [CrossRef]
- Auffermann, G.; Prots, Y.; Kniep, R. SrN and SrN2: Diazenides by Synthesis under High N2-Pressure. Angew. Chem. Int. Ed. Engl. 2001, 40, 547–549. [Google Scholar] [CrossRef]
- Hunt, E.B.; Rundle, R.E. The Structure of Thorium Dicarbide by X-Ray and Neutron Diffraction. J. Am. Chem. Soc. 1951, 73, 4777–4781. [Google Scholar] [CrossRef]
- Knapp, M.; Ruschewitz, U. Structural Phase Transitions in CaC2. Chem.–Eur. J. 2001, 7, 874–880. [Google Scholar] [CrossRef]
- Vohn, V.; Knapp, M.; Ruschewitz, U. Synthesis and Crystal Structure of SrC2. J. Solid State Chem. 2000, 151, 111–116. [Google Scholar] [CrossRef]
- Vohn, V.; Kockelmann, W.; Ruschewitz, U. On the synthesis and crystal structure of BaC2. J. Alloys Compds. 1999, 284, 132–137. [Google Scholar] [CrossRef]
- Wandner, D.; Link, P.; Heyer, O.; Mydosh, J.; Ahmida, M.A.; Abd-Elmeguid, M.M.; Speldrich, M.; Lueken, H.; Ruschewitz, U. Structural Phase Transitions in EuC2. Inorg. Chem. 2010, 49, 312–318. [Google Scholar] [CrossRef] [PubMed]
- Bredig, M.A. The polymorphism of calcium carbide. J. Phys. Chem. 1942, 46, 801–819. [Google Scholar] [CrossRef]
- Link, P.; Wandner, D.; Schellenberg, I.; Pöttgen, R.; Paulus, M.; Sahle, C.J.; Sternemann, C.; Ruschewitz, U. EuxSr1-xC2 (0 < x < 1): A Dicarbide Solid Solution with Perfect Vegard Behavior. Z. Anorg. Allg. Chem. 2010, 636, 2276–2281. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. 1976, A32, 751–767. [Google Scholar] [CrossRef]
- Link, P.; Glatzel, P.; Kvashnina, K.; Trots, D.M.; Smith, R.I.; Ruschewitz, U. Structure Induced Yb Valence Changes in the Solid Solution YbxCa1-xC2. Inorg. Chem. 2013, 52, 7020–7030. [Google Scholar] [CrossRef]
- Link, P.; Glatzel, P.; Kvashina, K.; Smith, R.I.; Ruschewitz, U. Yb Valence States in YbC2: A HERFD-XANES Spectroscopic Investigation. Inorg. Chem. 2011, 50, 5587–5595. [Google Scholar] [CrossRef]
- Busch, S. Gemischtkationische Dicarbide der Lanthanoide, Alkali- und Erdalkalimetalle, Kristallstrukturen und Phasenumwandlungen. Ph.D. Thesis, University of Cologne, Cologne, Germany, 2016. [Google Scholar]
- Pioro, I.; Kirillov, P.; Mendez-Vilas, A. Materials and Processes for Energy: Communicating Current Research and Technological Developments, 1st ed.; Formatex Research Center: Norristown, PA, USA, 2013; pp. 818–830. [Google Scholar]
- Wallace, T.C.; Krikorian, N.H.; Stone, P.L. The High Carbon Portion of the Uranium-Gadolinium-Carbon System. J. Electrochem. Soc. 1964, 111, 1404–1408. [Google Scholar] [CrossRef]
- McColm, I.J.; Colquhoun, I.; Clark, N.J. The cubic-tetragonal transformation in metal dicarbides—I The uranium-lanthanum and uranium-cerium dicarbide systems. J. Inorg. Nucl. Chem. 1972, 34, 3809–3814. [Google Scholar] [CrossRef]
- Jones, D.W.; McColm, I.J.; Yerkess, J. Tetragonal and cubic crystal structures of some binary and ternary metal dicarbides in the series Ce-Er, Ce-Lu, U-La, and U-Ce. J. Solid State Chem. 1991, 92, 301–311. [Google Scholar] [CrossRef]
- Asakura, H.; Hosokawa, S.; Teramura, K.; Tanaka, T. Local Structure Study of Lanthanide Elements by X-Ray Absorption Near Edge Structure Spectroscopy. Chem. Rec. 2019, 19, 1420–1431. [Google Scholar] [CrossRef] [PubMed]
- Vitova, T.; Kvashnina, K.O.; Nocton, G.; Sukharina, G.; Denecke, M.A.; Butorin, S.M.; Mazzanti, M.; Caciuffo, R.; Soldatov, A.; Behrends, T.; et al. High energy resolution x-ray absorption spectroscopy study of uranium in varying valence states. Phys. Rev. B 2010, 82, 235118. [Google Scholar] [CrossRef]
- Wilkins, M.C.D.; Townsend, L.T.; Stennett, M.C.; Kvashnina, K.O.; Corkhill, C.L.; Hyatt, N.C. A multimodal X-ray spectroscopy investigation of uranium speciation in ThTi2O6 compounds with the brannerite structure. Sci. Rep. 2023, 13, 12776. [Google Scholar] [CrossRef] [PubMed]
- Shi, H.; Zhang, P.; Li, S.-S.; Wang, B.; Sun, B. First-principles study of UC2 and U2C3. J. Nucl. Mater. 2010, 396, 218. [Google Scholar] [CrossRef]
- Wong, J.; Lytle, F.W.; Messmer, R.P.; Maylotte, D.H. K-edge absorption spectra of selected vanadium compounds. Phys. Rev. B 1984, 30, 5596. [Google Scholar] [CrossRef]
- George, G.N.; Gorbaty, M.L. Sulfur K-edge X-ray absorption spectroscopy of petroleum asphaltenes and model compounds. J. Am. Chem. Soc. 1989, 111, 3182–3186. [Google Scholar] [CrossRef]
- Tromp, M.; Moulin, J.; Reid, G.; Evans, J. Cr K-Edge XANES Spectroscopy: Ligand and Oxidation State Dependence—What is Oxidation State? AIP Conf. Proc. 2007, 882, 699–701. [Google Scholar] [CrossRef]
- Kosog, B.; La Pierre, H.S.; Denecke, M.A.; Heinemann, F.W.; Meyer, K. Oxidation State Delineation via U LIII-Edge XANES in a Series of Isostructural Uranium Coordination Complexes. Inorg. Chem. 2012, 51, 7940–7944. [Google Scholar] [CrossRef]
- Qi, B.; Perez, I.; Ansari, P.H.; Lu, F.; Croft, M. L2 and L3 measurements of transition-metal 5d orbital occupancy, spin-orbit effects, and chemical bonding. Phys. Rev. B 1987, 36, 2972. [Google Scholar] [CrossRef]
- Bès, R.; Rivenet, M.; Solari, P.-L.; Kvashnina, K.O.; Scheinost, A.C.; Martin, P.M. Use of HERFD–XANES at the U L3- and M4-Edges To Determine the Uranium Valence State on [Ni(H2O)4]3[U(OH,H2O)(UO2)8O12(OH)3]. Inorg. Chem. 2016, 55, 4260–4270. [Google Scholar] [CrossRef]
- Pöttgen, R.; Gulden, T.; Simon, A. Miniaturisierte Lichtbogenapparatur für den Laborbedarf. GIT Labor-Fachzeitschrift 1999, 43, 133–136. [Google Scholar]
- WinXPow, version 3.03; Stoe & Cie GmbH: Darmstadt, Germany, 2010.
- Dippel, A.-C.; Liermann, H.-P.; Delitz, J.T.; Walter, P.; Schulte-Schrepping, H.; Seeck, O.; Franz, H. Beamline P02.1 at PETRA III for high-resolution and high-energy powder diffraction. J. Synchrotron Radiat. 2015, 22, 675–687. [Google Scholar] [CrossRef] [PubMed]
- Prescher, C.; Prakapenka, V.B. DIOPTAS: A program for reduction of two-dimensional X-ray diffraction data and data exploration. High Pressure Res. 2015, 35, 223–230. [Google Scholar] [CrossRef]
- Hunter, J.D. Matplotlib: A 2D Graphics Environment. Comput. Sci. Eng. 2007, 9, 90–95. [Google Scholar] [CrossRef]
- Van Rossum, G.; Drake, F.L. Python 3 Reference Manual; CreateSpace: Scotts Valley, CA, USA, 2009. [Google Scholar]
- Krywka, C.; Sternemann, C.; Paulus, M.; Javid, N.; Winter, R.; Al-Sawalmih, A.; Yi, S.; Raabe, D.; Tolan, M. The small-angle and wide-angle X-ray scattering set-up at beamline BL9 of DELTA. J. Synchrotron Radiat. 2007, 14, 244–251. [Google Scholar] [CrossRef] [PubMed]
- Coelho, A.A.; Evans, J.; Evans, I.; Kern, A.; Parsons, S. The TOPAS symbolic computation system. Powder Diffr. 2011, 26, S22–S25. [Google Scholar] [CrossRef]
- Stephens, P.W. Phenomenological model of anisotropic peak broadening in powder diffraction. J. Appl. Crystallogr. 1999, 32, 281–289. [Google Scholar] [CrossRef]
- Frahm, R.; Wagner, R.; Herdt, A.; Lützenkirchen-Hecht, D. XAS at the materials science X-ray beamline BL8 at the DELTA storage ring. J. Phys. Conf. Ser. 2009, 190, 012040. [Google Scholar] [CrossRef]
- Lützenkirchen-Hecht, D.; Wagner, R.; Szillat, S.; Hüsecken, A.K.; Istomin, K.; Pietsch, U.; Frahm, R. The multi-purpose hard X-ray beamline BL10 at the DELTA storage ring. J. Synchrotron Radiat. 2014, 21, 819–826. [Google Scholar] [CrossRef] [PubMed]
- Ravel, B.; Newville, M. ATHENA, ARTEMIS, HEPHAESTUS: Data analysis for X-ray absorption spectroscopy using IFEFFIT. J. Synchrotron Radiat. 2005, 12, 537–541. [Google Scholar] [CrossRef]
- Harris, C.R.; Millman, K.J.; van der Walt, S.J.; Gommers, R.; Virtanen, P.; Cournapeau, D.; Wieser, E.; Taylor, J.; Berg, S.; Smith, N.J.; et al. Array programming with NumPy. Nature 2020, 585, 357–362. [Google Scholar] [CrossRef] [PubMed]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental algorithms for scientific computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef] [PubMed]
- Guda, A.A.; Guda, S.A.; Martini, A.; Kravtsova, A.N.; Algasov, A.; Bugaev, A.; Kubrin, S.P.; Guda, L.V.; Šot, P.; van Bokhoven, J.A.; et al. Understanding X-ray absorption spectra by means of descriptors and machine learning algorithms. npj Comput. Mater. 2021, 7, 203. [Google Scholar] [CrossRef]
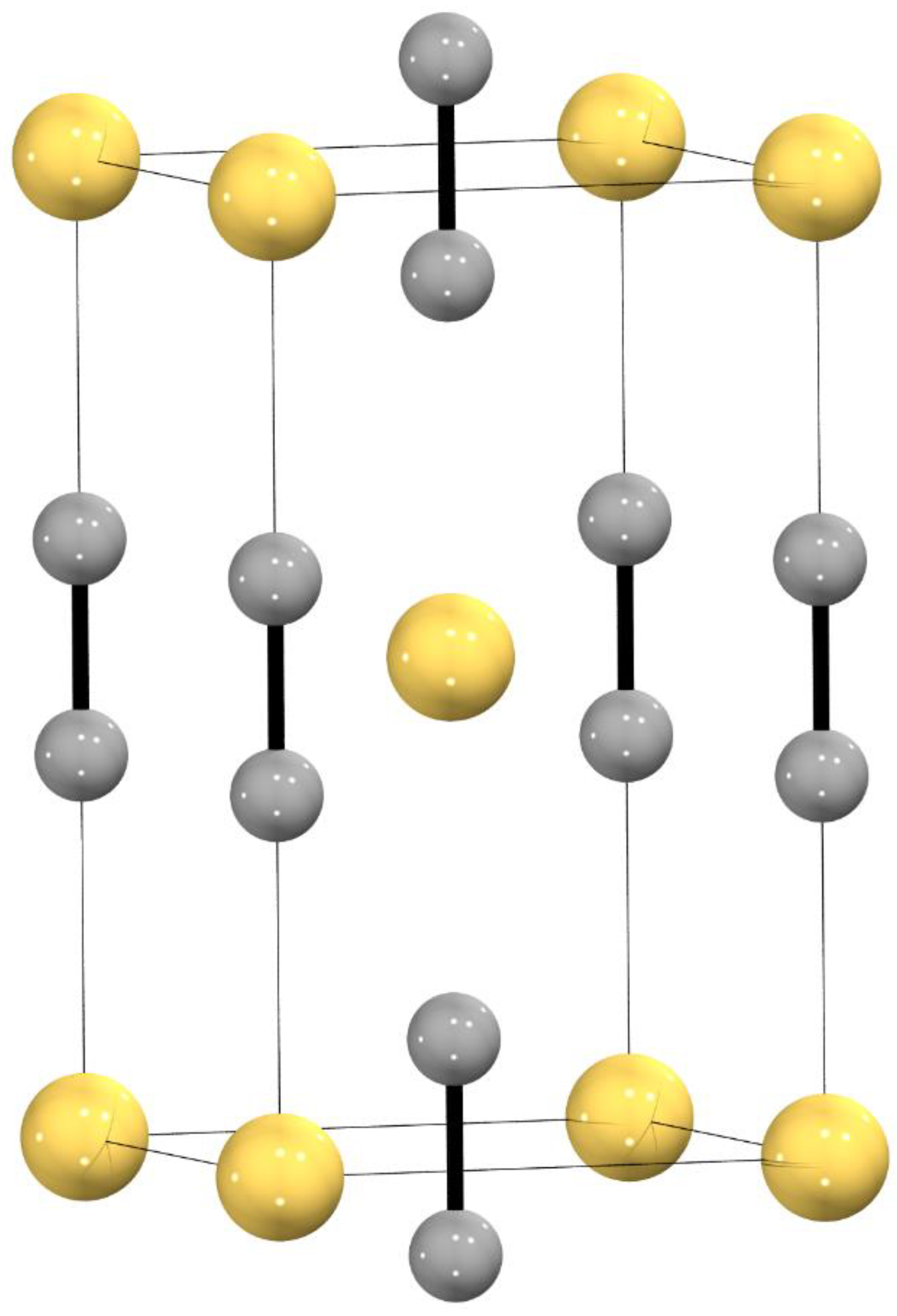
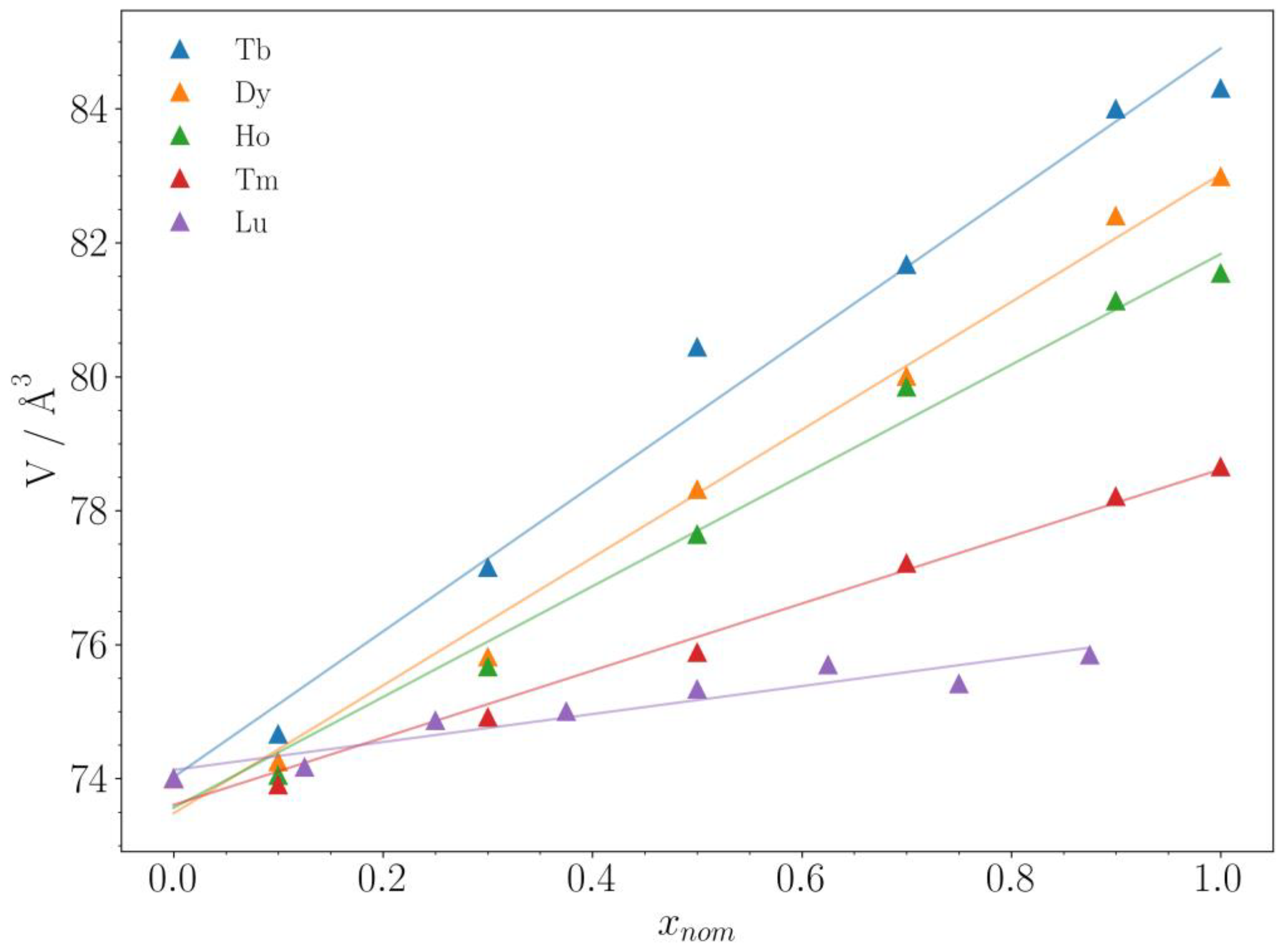
| La | Ce | Gd | Tb | Dy | Ho | Tm | Lu | |
|---|---|---|---|---|---|---|---|---|
| r(Ln3+)/pm | 103.2 | 101 | 93.8 | 92.3 | 91.2 | 90.1 | 88 | 86.1 |
| r(Ln3+)/r(U4+) | 1.16 | 1.13 | 1.05 | 1.04 | 1.02 | 1.01 | 0.99 | 0.97 |
| solid solution | discont. | discont. | Vegard | Vegard | Vegard | Vegard | Vegard | Vegard |
| boiling point Ln/°C | 3464 | 3443 | 3273 | 3230 | 2567 | 2720 | 1950 | 3330 |
| reference | [22,23] | [22,23] | [21] | this work | this work | this work | this work | this work |
| Ln | Slope of E0 = f(xnom)/eV·x−1 | Slope of AWL = f(xnom)/a.u.·x−1 | ||
|---|---|---|---|---|
| Ln-LIII Edge | U-LIII Edge | Ln-LIII Edge | U-LIII Edge | |
| Tb | −2.6(6) | 2.2(7) | −11(2) | 2.38(7) |
| Dy | −2.5(9) | 2.9(24) | −5(5) | 3(2) |
| Ho | −2.4(11) | 1.2(4) | −12(29) | 2.2(11) |
| Tm | −3.2(6) | 2.3(5) | −6(3) | 1.6(5) |
| Lu | −3.7(6) | 1.8(7) | −6.5(11) | 2.2(6) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tobeck, C.; Wende, H.; Ruschewitz, U. Solid Solutions LnxU1−xC2 with Ln = Tb, Dy, Ho, Tm, and Lu Showing Ideal Vegard Behavior. Inorganics 2023, 11, 457. https://doi.org/10.3390/inorganics11120457
Tobeck C, Wende H, Ruschewitz U. Solid Solutions LnxU1−xC2 with Ln = Tb, Dy, Ho, Tm, and Lu Showing Ideal Vegard Behavior. Inorganics. 2023; 11(12):457. https://doi.org/10.3390/inorganics11120457
Chicago/Turabian StyleTobeck, Christian, Heiko Wende, and Uwe Ruschewitz. 2023. "Solid Solutions LnxU1−xC2 with Ln = Tb, Dy, Ho, Tm, and Lu Showing Ideal Vegard Behavior" Inorganics 11, no. 12: 457. https://doi.org/10.3390/inorganics11120457
APA StyleTobeck, C., Wende, H., & Ruschewitz, U. (2023). Solid Solutions LnxU1−xC2 with Ln = Tb, Dy, Ho, Tm, and Lu Showing Ideal Vegard Behavior. Inorganics, 11(12), 457. https://doi.org/10.3390/inorganics11120457