Effect of Doping Different Cu Valence States in HfO2 on Resistive Switching Properties of RRAM
Abstract
:1. Introduction
2. Calculation Theory: Model and Method
3. Results and Analysis
3.1. Valence Determination
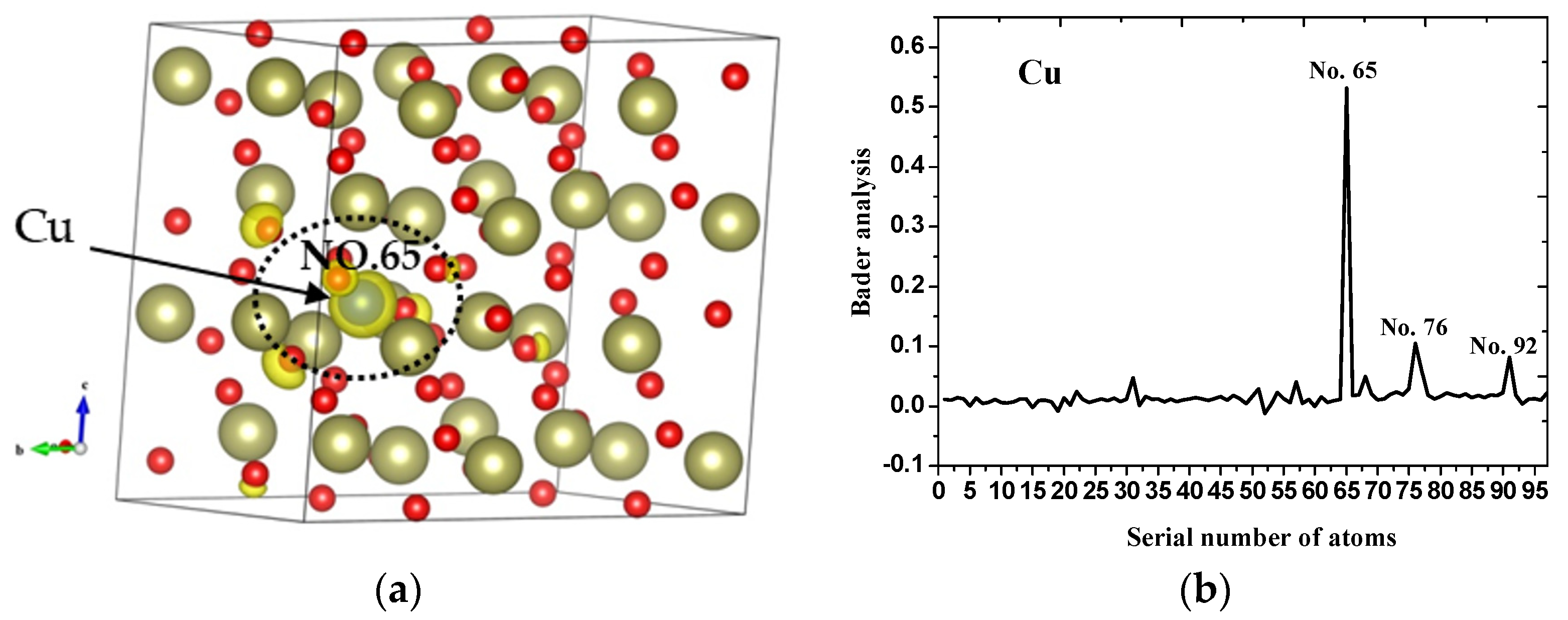
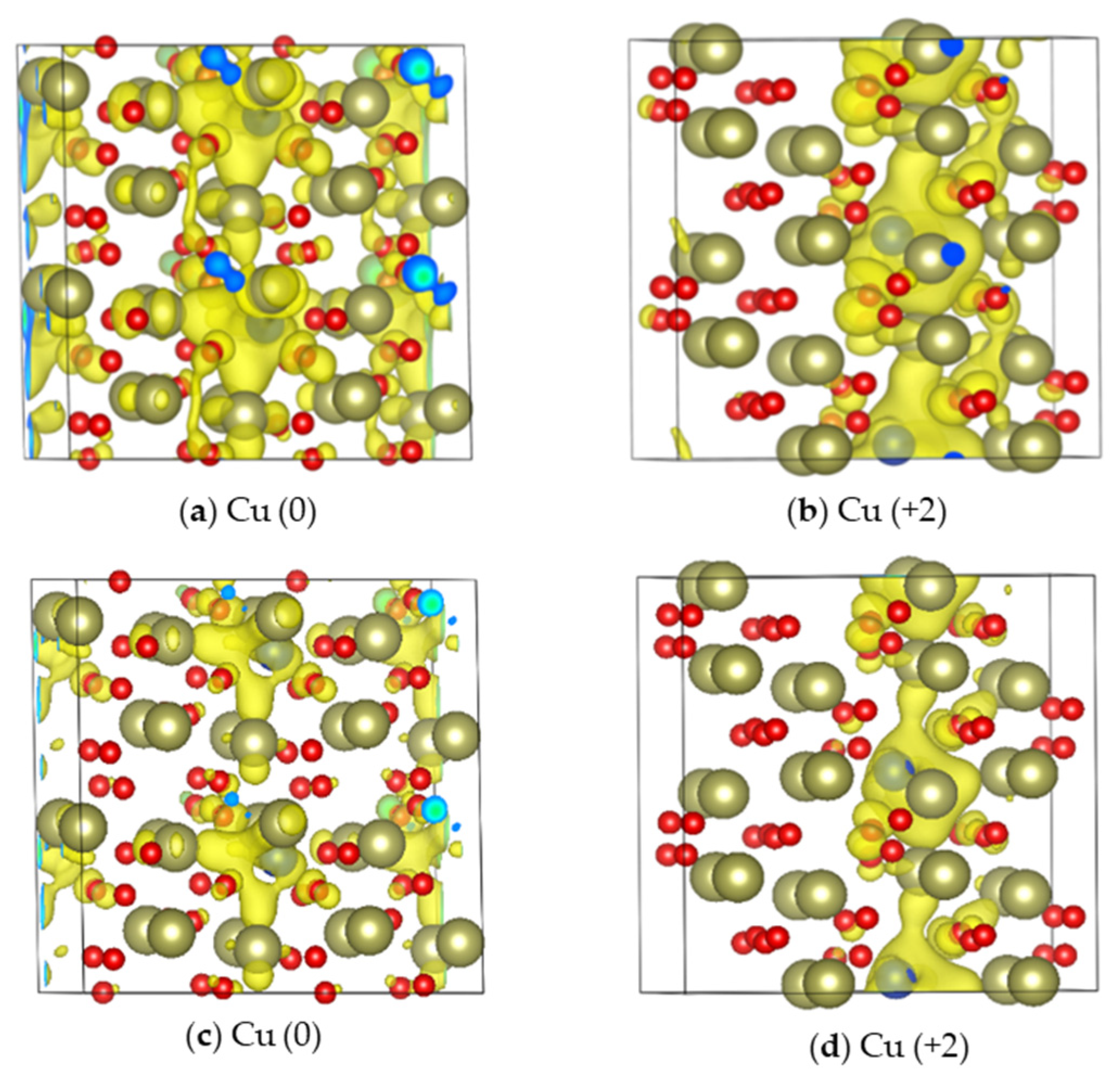
3.2. Charge Density Difference and Bader Analysis
3.3. Mulliken Population Analysis of Different Valence States
3.4. Influence of Conductive Channels on the Valence States
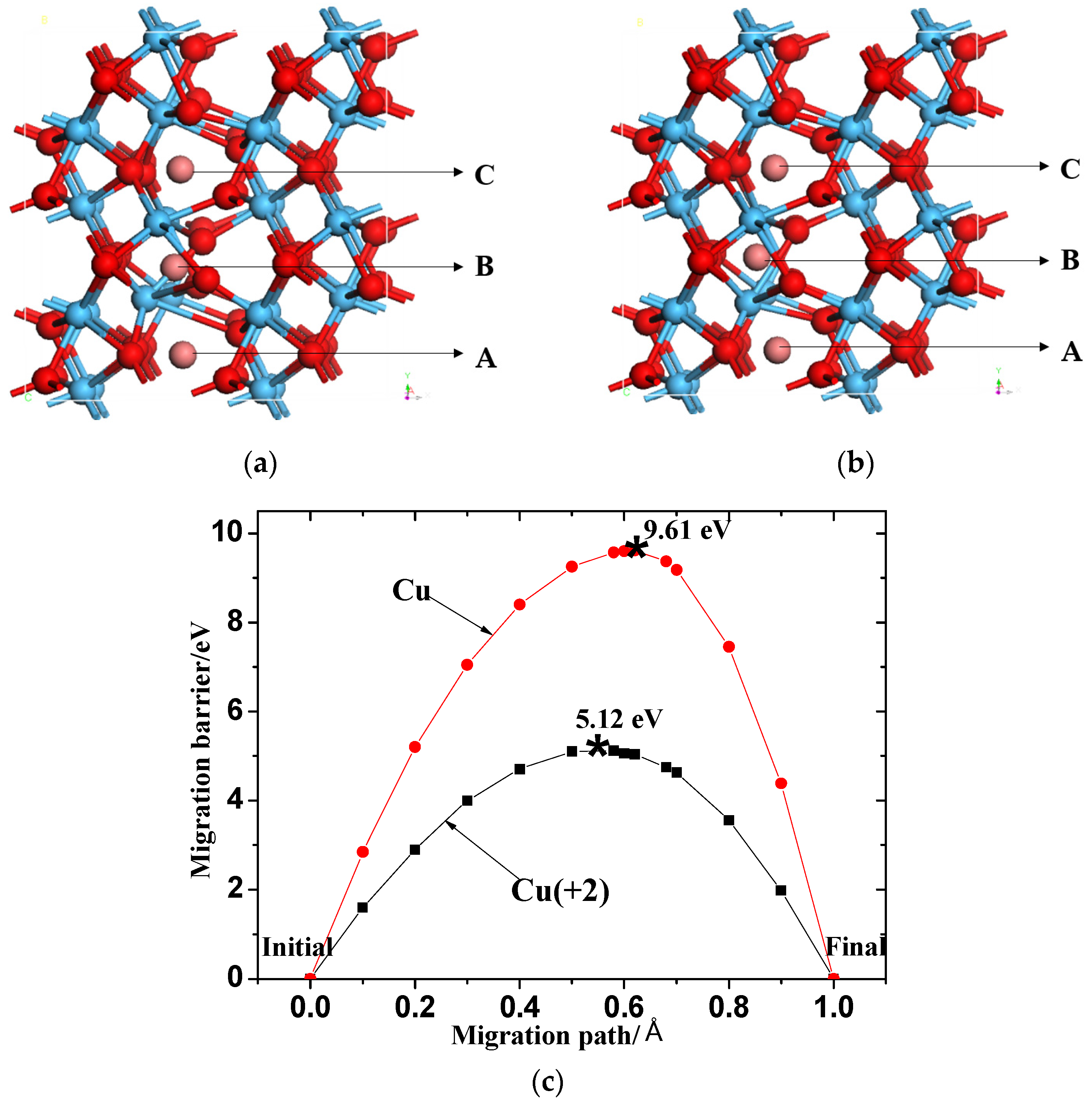
3.5. Comparative Analysis of Migration Barriers
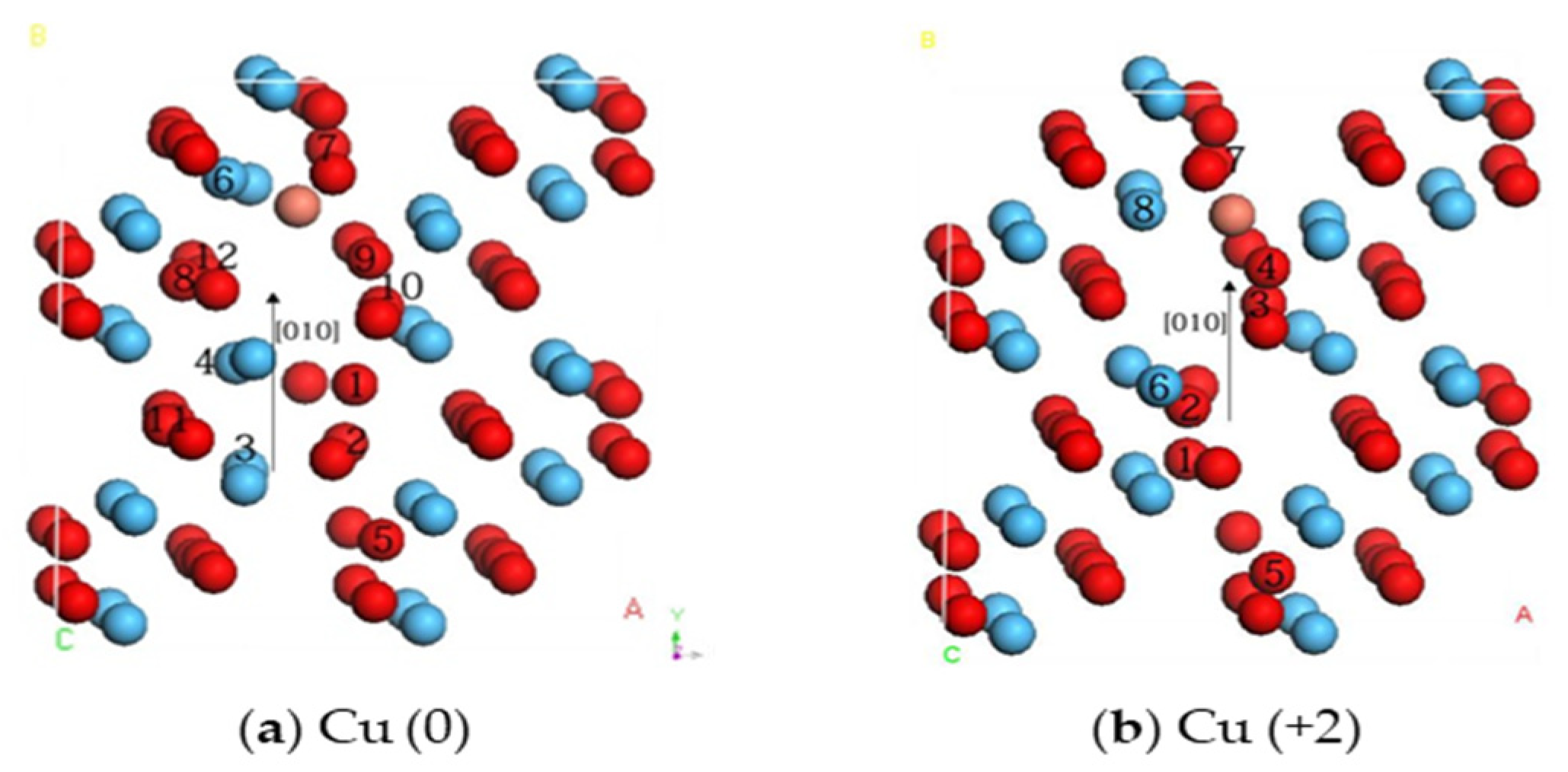
3.6. Effects of Different Valence Systems on Stability
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chiang, C.-C.; Ostwal, V.; Wu, P.; Pang, C.-S.; Zhang, F.; Chen, Z.; Appenzeller, J. Memory applications from 2D materials. Appl. Phys. Rev. 2021, 8, 021306. [Google Scholar] [CrossRef]
- Valov, L.; Michael, N.K. Cation-based resistance change memory. Phys. D Appl. Phys. 2013, 46, 074005. [Google Scholar] [CrossRef]
- Milo, V.; Zambelli, C.; Olivo, P.; Pérez, E.; Mahadevaiah, M.K.; Ossorio, O.G.; Wenger, C.; Ielmini, D. Multilevel HfO2-based RRAM devices for low-power neuromorphic networks. APL Mater. 2019, 7, 081120. [Google Scholar] [CrossRef] [Green Version]
- Jena, A.K.; Sahoo, A.K.; Mohanty, J. Effects of magnetic field on resistive switching in multiferroic based Ag/BiFeO3/FTO RRAM device. Appl. Phys. Lett. 2020, 116, 092901. [Google Scholar] [CrossRef]
- Yang, Y.; Pan, F.; Liu, Q.; Liu, M.; Zeng, F. Fully Room-Temperature-Fabricated Nonvolatile Resistive Memory for Ultrafast and High-Density Memory Application. Nano Lett. 2009, 9, 1636–1643. [Google Scholar] [CrossRef] [PubMed]
- Lanza, M.; Wong, H.-S.P.; Pop, E.; Ielmini, D.; Shi, Y. Recommended methods to study resistive switching devices. Adv. Electron. Mater. 2019, 5, 1800143. [Google Scholar] [CrossRef] [Green Version]
- Frascaroli, J.; Volpe, F.G.; Brivio, S.; Spiga, S. Effect of Al doping on the retention behavior of HfO2 resistive switching memories. Microelectron. Eng. 2015, 147, 104–107. [Google Scholar] [CrossRef]
- Trapatseli, M.; Khiat, A.; Cortese, S.; Serb, A.; Carta, D.; Prodromakis, T. Engineering the switching dynamics of TiOx-based RRAM with Al doping. J. Appl. Phys. 2016, 120, 025108. [Google Scholar] [CrossRef]
- Liu, Q.; Long, S.; Lv, H.; Wang, W.; Niu, J.; Huo, Z.; Chen, J.; Liu, M. Controllable Growth of Nanoscale Conductive Filaments in Solid-Electrolyte-Based ReRAM by Using a Metal Nanocrystal Covered Bottom Electrode. ACS Nano 2010, 4, 6162–6168. [Google Scholar] [CrossRef]
- Maoxiu, Z.; Qiang, Z.; Wei, Z.; Liu, Q.; Dai, Y. The conductive path in HfO2: First principles study. J. Semicond. 2012, 33, 072002. [Google Scholar]
- Waser, B.R.; Dittmann, R.; Staikov, G.; Szot, K. Redox-based resistive switching memories–nanoionic mechanisms, prospects, and challenges. Adv. Mater. 2009, 21, 2632–2663. [Google Scholar] [CrossRef]
- Xiao, B.; Watanabe, S. Interface Structure in Cu/Ta2O5/Pt Resistance Switch: A First-Principles Study. ACS Appl. Mater. Interfaces 2015, 7, 519–525. [Google Scholar] [CrossRef] [PubMed]
- Xiao, B.; Gu, T.K.; Tada, T.; Watanabe, S. Conduction paths in Cu/amorphous-Ta2O5/Pt atomic switch: First-principles studies. J. Appl. Phys. 2014, 115, 034503. [Google Scholar] [CrossRef] [Green Version]
- Traore, B.; Blaise, P.; Vianello, E.; Grampeix, H.; Jeannot, S.; Perniola, L.; De Salvo, B.; Nishi, Y. On the Origin of Low-Resistance State Retention Failure in HfO2-Based RRAM and Impact of Doping/Alloying. IEEE Trans. Electron. Devices 2015, 62, 4029–4036. [Google Scholar] [CrossRef]
- Ielmini, D.; Wong, H.-S.P. In-memory computing with resistive switching devices. Nat. Electron. 2018, 1, 333–343. [Google Scholar] [CrossRef]
- Cavallini, M.; Hemmatian, Z.; Riminucci, A.; Prezioso, M.; Morandi, V.; Murgia, M. Regenerable Resistive Switching in Silicon Oxide Based Nanojunctions. Adv. Mater. 2012, 24, 1197–1201. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Can. Metall. Q. 1996, 54, 11169. [Google Scholar]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1997, 77, 3865. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gritsenko, V.; Nekrashevich, S.; Vasilev, V.; Shaposhnikov, A. Electronic structure of memory traps in silicon nitride. Microelectron. Eng. 2009, 86, 1866–1869. [Google Scholar] [CrossRef]

| Electron Affinity Energy | Ability of Gained Electron | Ability of Lost Electron |
|---|---|---|
| Doping Cu | 0.540 | 3.994 |
| Valence State | Cu (0) | Cu (+1) | Cu (+2) |
|---|---|---|---|
| Formation energy (eV) | 4.813 | 2.547 | 1.863 |
| Mulliken Population Analysis | Mulliken Population | Bond Length | ||
|---|---|---|---|---|
| Hf-O | Cu-O | Hf-O | Cu-O | |
| Cu (0) | 0.3696 | 0.107 | 2.1392 | 2.1601 |
| Cu (+2) | 0.3706 | 0.0229 | 2.1389 | 2.3718 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Chen, J.; Hong, Y. Effect of Doping Different Cu Valence States in HfO2 on Resistive Switching Properties of RRAM. Inorganics 2022, 10, 85. https://doi.org/10.3390/inorganics10060085
Yang J, Chen J, Hong Y. Effect of Doping Different Cu Valence States in HfO2 on Resistive Switching Properties of RRAM. Inorganics. 2022; 10(6):85. https://doi.org/10.3390/inorganics10060085
Chicago/Turabian StyleYang, Jin, Jun Chen, and Yingzheng Hong. 2022. "Effect of Doping Different Cu Valence States in HfO2 on Resistive Switching Properties of RRAM" Inorganics 10, no. 6: 85. https://doi.org/10.3390/inorganics10060085
APA StyleYang, J., Chen, J., & Hong, Y. (2022). Effect of Doping Different Cu Valence States in HfO2 on Resistive Switching Properties of RRAM. Inorganics, 10(6), 85. https://doi.org/10.3390/inorganics10060085







