Reaching the Maximal Unquenched Orbital Angular Momentum L = 3 in Mononuclear Transition-Metal Complexes: Where, When and How?
Abstract
1. Introduction
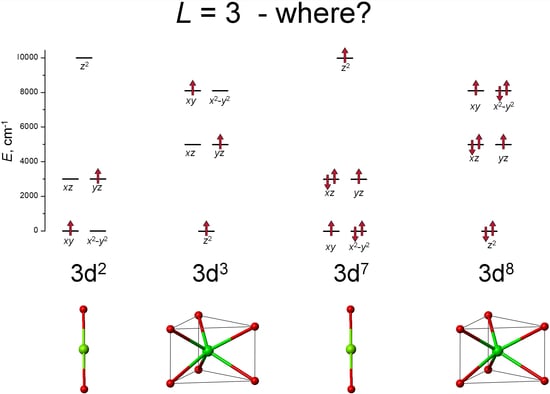
2. Results
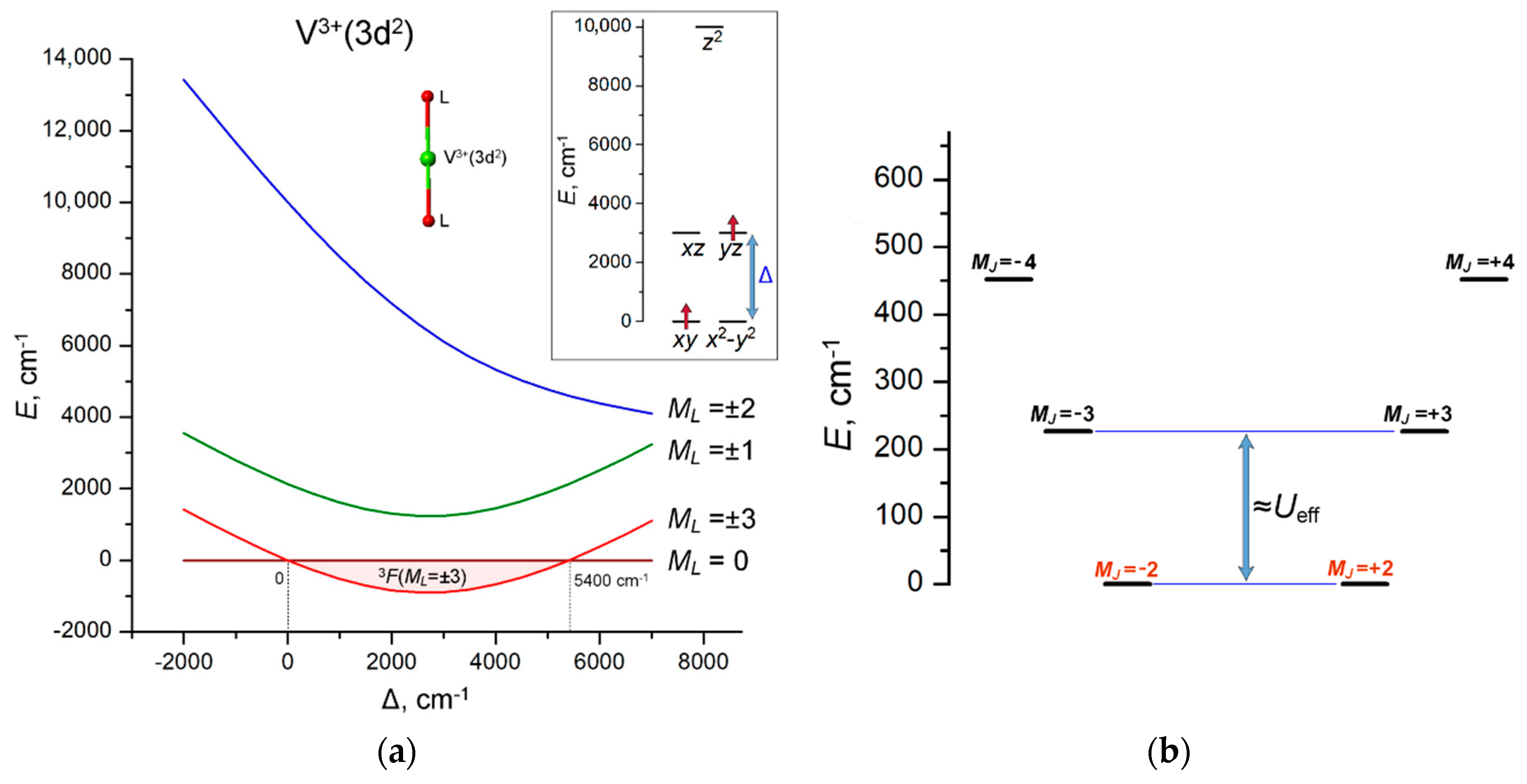
2.1. 3d2 Complexes (V3+, Cr4+)
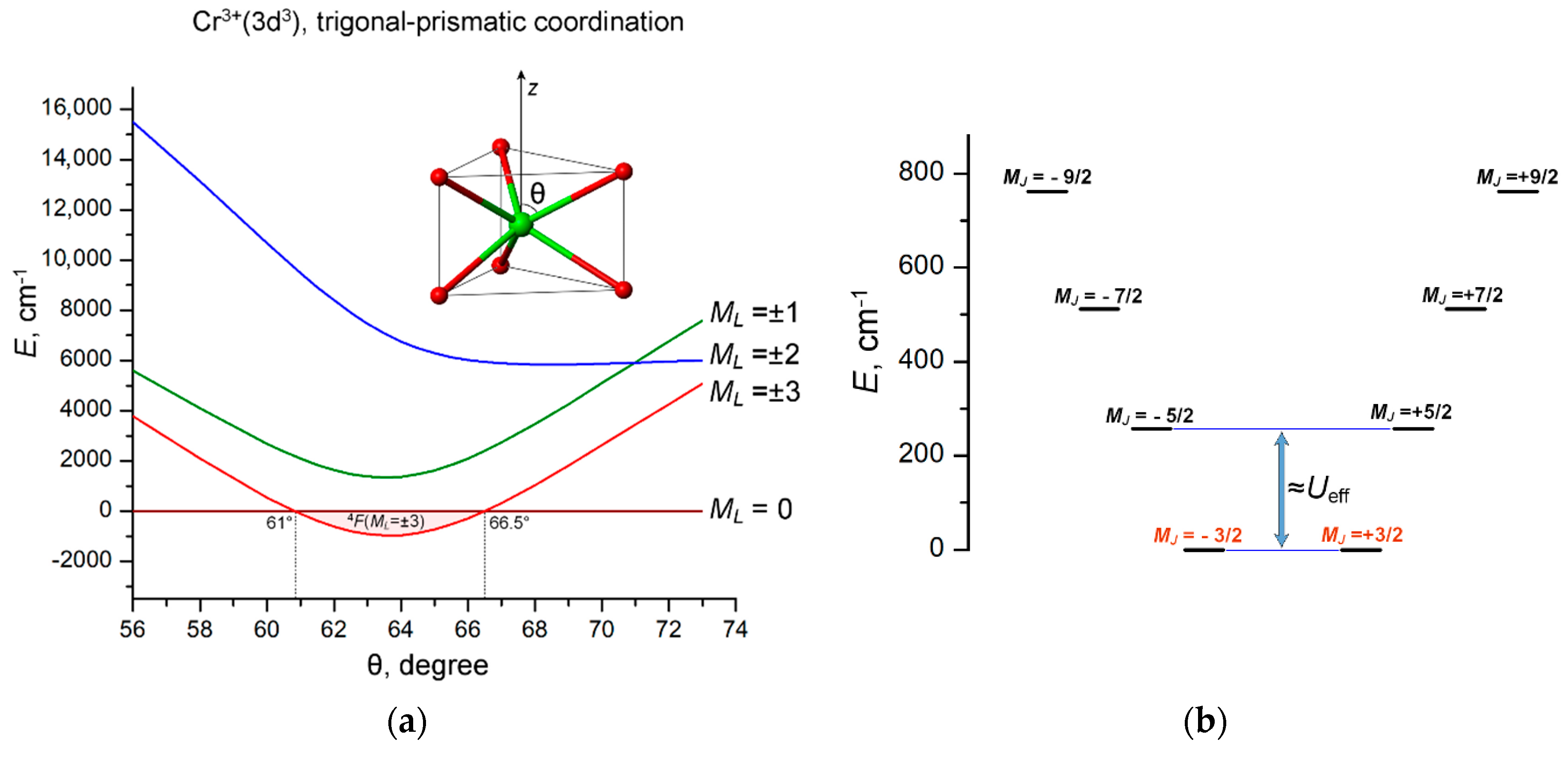
2.2. 3d3 Complexes (Cr3+, Mn4+)
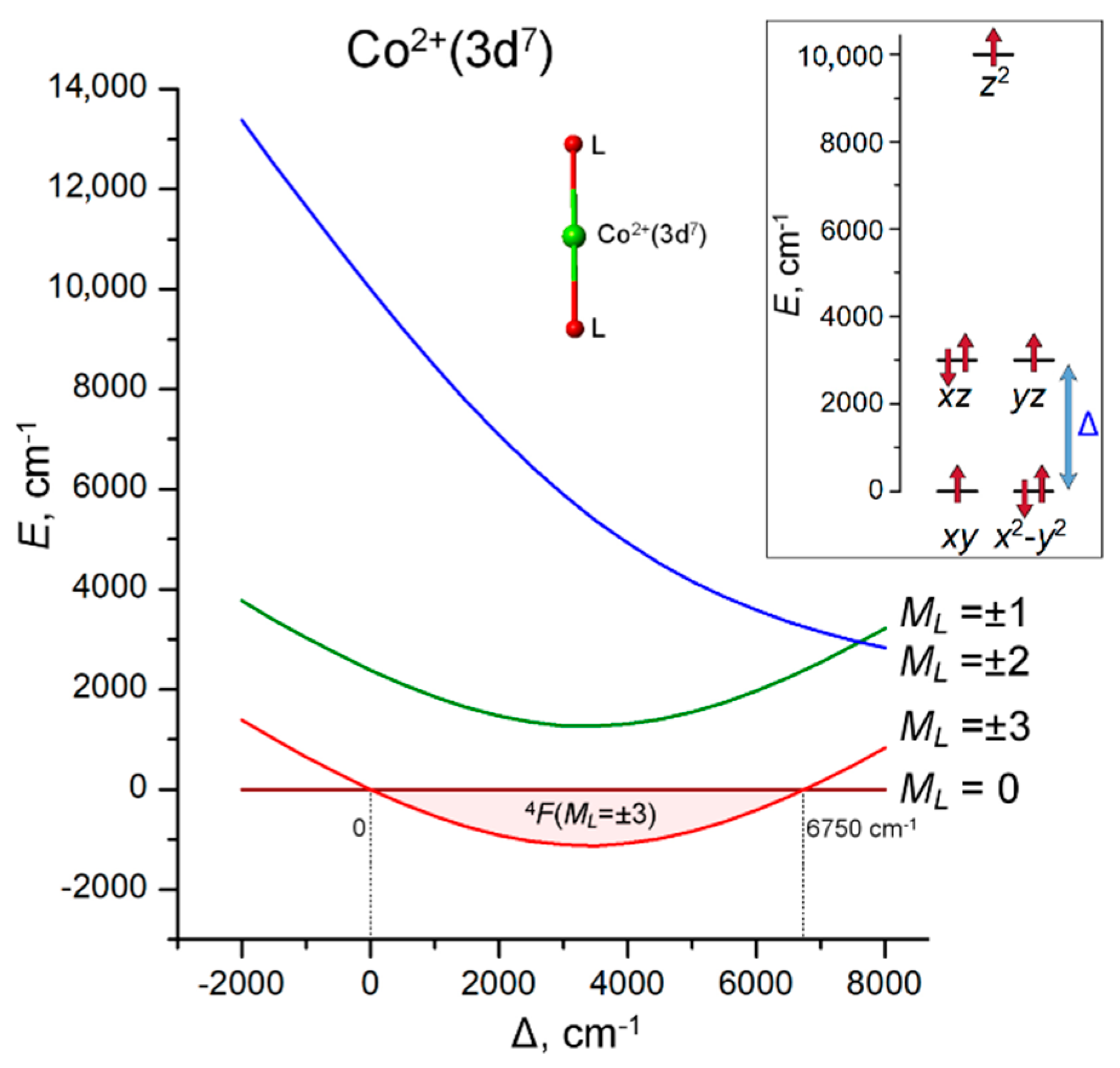
2.3. 3d7 Complexes (Fe+,Co2+)
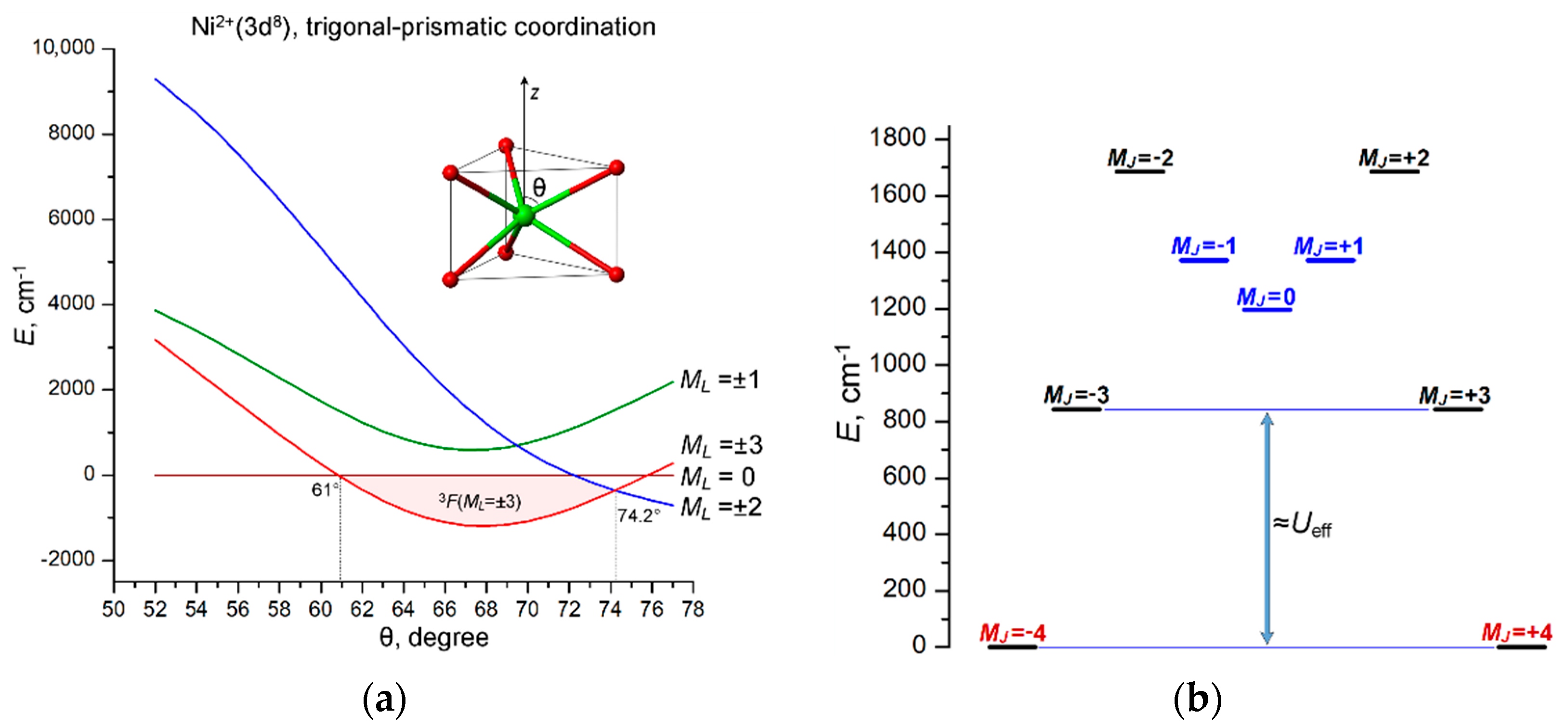
2.4. 3d8 Complexes (Ni2+, Co1+)
3. Materials and Methods
Ligand Field and Angular Overlap Model Calculations
4. Discussion and Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sessoli, R.; Gatteschi, D.; Caneschi, A.; Novak, M.A. Magnetic bistability in a metal-ion cluster. Nature 1993, 365, 141–143. [Google Scholar] [CrossRef]
- Gatteschi, D.; Sessoli, R. Quantum tunneling of magnetization and related phenomena in molecular materials. Angew. Chem. Int. Ed. 2003, 42, 268–297. [Google Scholar] [CrossRef] [PubMed]
- Gatteschi, D.; Sessoli, R.; Villain, J. Molecular Nanomagnets; Oxford University Press: Oxford, UK, 2006. [Google Scholar] [CrossRef]
- Leuenberger, M.N.; Loss, D. Quantum computing in molecular magnets. Nature 2001, 410, 789–793. [Google Scholar] [CrossRef] [PubMed]
- Bogani, L.; Wernsdorfer, W. Molecular spintronics using single-molecule magnets. Nat. Mater. 2008, 7, 179. [Google Scholar] [CrossRef] [PubMed]
- Bartolomé, J.; Luis, F.; Fernández, J.F. (Eds.) Molecular Magnets: Physics and Applications; NanoScience and Technology; Springer: Berlin/Heidelberg, Germany, 2014; pp. 1–395. ISBN 1010:9783642406089. [Google Scholar]
- McInnes, E.J.L.; Winpenny, R.E.P. 4.14—Molecular Magnets. Comprehensive Inorganic Chemistry II; Elsevier: Edinburgh, UK, 2013; Volume 4, pp. 371–396. [Google Scholar] [CrossRef]
- Feng, M.; Tong, M.-L. Single Ion Magnets from 3d to 5f: Developments and Strategies. Chem.—Eur. J. 2018, 24, 7574–7594. [Google Scholar] [CrossRef]
- Coronado, E. Molecular magnetism: From chemical design to spin control in molecules, materials and devices. Nat. Rev. Mat. 2020, 5, 87–104. [Google Scholar] [CrossRef]
- Caneschi, A.; Gatteschi, D.; Lalioti, N.; Sangregorio, C.; Sessoli, R.; Venturi, G.; Vindigni, A.; Rettori, A.; Pini, M.G.; Novak, M.A. Cobalt(II)-Nitronyl Nitroxide Chains as Molecular Magnetic Nanowires. Angew. Chem. Int. Ed. 2001, 40, 1760–1763. [Google Scholar] [CrossRef]
- Coulon, C.; Miyasaka, H.; Clérac, R. Single-Chain Magnets: Theoretical Approach and Experimental Systems. In Single-Molecule Magnets and Related Phenomena. Structure and Bonding; Winpenny, R., Ed.; Springer: Berlin/Heidelberg, Germany, 2006; Volume 122, pp. 163–206. [Google Scholar] [CrossRef]
- Sun, H.-L.; Wang, Z.-M.; Gao, S. Strategies towards Single-Chain Magnets. Coord. Chem. Rev. 2010, 254, 1081–1100. [Google Scholar] [CrossRef]
- Ishikawa, N.; Sugita, M.; Ishikawa, T.; Koshihara, S.-Y.; Kaizu, Y. Lanthanide Double-Decker Complexes Functioning as Magnets at the Single-Molecular Level. J. Am. Chem. Soc. 2003, 125, 8694–8695. [Google Scholar] [CrossRef]
- Freedman, D.E.; Harman, W.H.; Harris, T.D.; Long, G.J.; Chang, C.J.; Long, J.R. Slow magnetic relaxation in a high-spin iron(II) complex. J. Am. Chem. Soc. 2010, 132, 1224–1225. [Google Scholar] [CrossRef]
- Harman, W.H.; Harris, T.D.; Freedman, D.E.; Fong, H.; Chang, A.; Rinehart, J.D.; Ozarowski, A.; Sougrati, M.T.; Grandjean, F.; Long, G.J.; et al. Slow magnetic relaxation in a family of trigonal pyramidal iron(II) pyrrolide complexes. J. Am. Chem. Soc. 2010, 132, 18115–18126. [Google Scholar] [CrossRef]
- Rinehart, J.D.; Long, J.R. Exploiting Single-Ion Anisotropy in the Design of f-Element Single-Molecule Magnets. Chem. Sci. 2011, 2, 2078–2085. [Google Scholar] [CrossRef]
- Woodruff, D.N.; Winpenny, R.E.P.; Layfield, R.A. Lanthanide Single-Molecule Magnets. Chem. Rev. 2013, 113, 5110–5148. [Google Scholar] [CrossRef]
- Liddle, S.T.; van Slageren, J. Improving f-Element Single Molecule Magnets. Chem. Soc. Rev. 2015, 44, 6655–6669. [Google Scholar] [CrossRef]
- Zhang, P.; Zhang, L.; Tang, J. Lanthanide single molecule magnets: Progress and perspective. Dalton Trans. 2015, 44, 3923–3929. [Google Scholar] [CrossRef]
- Layfield, R.; Murugesu, M. Lanthanides and Actinides in Molecular Magnetism; John Wiley & Sons: New York, NY, USA, 2015; pp. 1–368. ISBN 978-3-527-33526-8. [Google Scholar]
- Lu, J.; Guo, M.; Tang, J. Recent Developments in Lanthanide Single-Molecule Magnets. Chem.—Asian J. 2017, 12, 2772–2779. [Google Scholar] [CrossRef]
- Liu, J.-L.; Chen, Y.-C.; Tong, M.-L. Symmetry Strategies for High Performance Lanthanide-Based Single-Molecule Magnets. Chem. Soc. Rev. 2018, 47, 2431–2453. [Google Scholar] [CrossRef]
- Feltham, H.L.C.; Brooker, S. Review of Purely 4f and Mixed-Metal nd-4f Single-Molecule Magnets Containing only One Lanthanide Ion. Coord. Chem. Rev. 2014, 276, 1–33. [Google Scholar] [CrossRef]
- Layfield, R.A. Organometallic Single-Molecule Magnets. Organometallics 2014, 33, 1084–1099. [Google Scholar] [CrossRef]
- Craig, G.A.; Murrie, M. 3d single-ion magnets. Chem. Soc. Rev. 2015, 44, 2135–2147. [Google Scholar] [CrossRef]
- Frost, J.M.; Harriman, K.L.M.; Murugesu, M. The rise of 3-d single-ion magnets in molecular magnetism: Towards materials from molecules? Chem. Sci. 2016, 7, 2470–2491. [Google Scholar] [CrossRef] [PubMed]
- Wyborne, B.G. Spectroscopic Properties of Rare Earths; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1965; pp. 1–241. ISBN 978-0470965078. [Google Scholar]
- Liu, J.; Chen, Y.-C.; Liu, J.-L.; Vieru, V.; Ungur, L.; Jia, J.-H.; Chibotaru, L.F.; Lan, Y.; Wernsdorfer, W.; Gao, S.; et al. A stable pentagonal bipyramidal Dy(III) single-ion magnet with a record magnetization reversal barrier over 1000 K. J. Am. Chem. Soc. 2016, 138, 5441–5450. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.-S.; Chilton, N.F.; Winpenny, R.E.P.; Zheng, Y.-Z. On approaching the limit of molecular magnetic anisotropy: A near-perfect pentagonal bipyramidal dysprosium(III) single-molecule magnet. Angew. Chem. Int. Ed. 2016, 55, 16071–16074. [Google Scholar] [CrossRef] [PubMed]
- Goodwin, C.A.P.; Ortu, F.; Reta, D.; Chilton, N.F.; Mills, D.P. Molecular magnetic hysteresis at 60 kelvin in dysprosocenium. Nature 2017, 548, 439–442. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.-S.; Day, B.M.; Chen, Y.-C.; Tong, M.-L.; Mansikkamäki, A.; Layfield, R.A. Magnetic Hysteresis up to 80 Kelvin in a Dysprosium Metallocene Single-Molecule Magnet. Science 2018, 362, 1400–1403. [Google Scholar] [CrossRef]
- Zhu, Z.; Tang, J. Lanthanide single-molecule magnets with high anisotropy barrier: Where to from here? Natl. Sci. Rev. 2022, nwac194. [Google Scholar] [CrossRef]
- Bar, A.K.; Pichon, C.; Sutter, J.-P. Magnetic Anisotropy in Two- to Eight-Coordinated Transition–Metal Complexes: Recent Developments in Molecular Magnetism. Coord. Chem. Rev. 2016, 308, 346–380. [Google Scholar] [CrossRef]
- Boca, R. Zero-field splitting in metal complexes. Coord. Chem. Rev. 2004, 248, 757–815. [Google Scholar] [CrossRef]
- Marriott, K.E.R.; Bhaskaran, L.; Wilson, C.; Medarde, M.; Ochsenbein, S.T.; Hill, S.; Murrie, M. Pushing the limits of magneticanisotropy in trigonal bipyramidal Ni(II). Chem. Sci. 2015, 6, 6823–6828. [Google Scholar] [CrossRef]
- Novikov, V.V.; Pavlov, A.A.; Nelyubina, Y.V.; Boulon, M.-E.; Varzatskii, O.; Voloshin, Y.Z.; Winpenny, R.E.P. A Trigonal Prismatic Mononuclear Cobalt(II) Complex Showing Single-Molecule Magnet Behavior. J. Am. Chem. Soc. 2015, 137, 9792–9795. [Google Scholar] [CrossRef]
- Yao, B.; Singh, M.K.; Deng, Y.-F.; Wang, Y.-N.; Dunbar, K.R.; Zhang, Y.-Z. Trigonal Prismatic Cobalt(II) Single-Ion Magnets: Manipulating the Magnetic Relaxation Through Symmetry Control. Inorg. Chem. 2020, 59, 8505–8513. [Google Scholar] [CrossRef]
- Sutter, J.P.; Béreau, V.; Jubault, V.; Bretosh, K.; Pichon, C.; Duhayon, C. Magnetic anisotropy of transition metal and lanthanide ions in pentagonal bipyramidal geometry. Chem. Soc. Rev. 2022, 51, 3280–3313. [Google Scholar] [CrossRef]
- Zadrozny, J.M.; Atanasov, M.; Bryan, A.M.; Lin, C.-Y.; Rekken, B.D.; Power, P.P.; Neese, F.; Long, J.R. Slow magnetization dynamics in a series of two-coordinate iron(II) complexes. Chem. Sci. 2013, 4, 125–138. [Google Scholar] [CrossRef]
- Zadrozny, J.M.; Xiao, D.J.; Atanasov, M.; Long, G.J.; Grandjean, F.; Neese, F.; Long, J.R. Magnetic blocking in a linear iron(I) complex. Nat. Chem. 2013, 5, 577–581. [Google Scholar] [CrossRef]
- Yao, X.-N.; Du, J.-Z.; Zhang, Y.-Q.; Leng, X.-B.; Yang, M.-W.; Jiang, S.-D.; Wang, Z.-X.; Ouyang, Z.-W.; Deng, L.; Wang, B.-W.; et al. Two-Coordinate Co(II) Imido Complexes as Outstanding Single-Molecule Magnets. J. Am. Chem. Soc. 2017, 139, 373–380. [Google Scholar] [CrossRef]
- Bunting, P.C.; Atanasov, M.; Damgaard-Møller, E.; Perfetti, M.; Crassee, I.; Orlita, M.; Overgaard, J.; van Slageren, J.; Neese, F.; Long, J.R. A linear cobalt(II) complex with maximal orbital angular momentum from a non-Aufbau ground state. Science 2018, 362, eaat7319. [Google Scholar] [CrossRef]
- Rau, G.; Baumann, S.; Rusponi, S.; Donati, F.; Stepanow, S.; Gragnaniello, L.; Dreiser, J.; Piamonteze, C.; Nolting, F.; Gangopadhyay, S.; et al. Reaching the magnetic anisotropy limit of a 3d metal atom. Science 2014, 344, 988–992. [Google Scholar] [CrossRef] [PubMed]
- Powell, P.P. Stable Two-Coordinate, Open-Shell (d1–d9) Transition Metal Complexes. Chem. Rev. 2012, 112, 3482–3507. [Google Scholar] [CrossRef]
- Reber, C.; Güdel, H.U. Near-infrared luminescence spectroscopy of Al2O3:V3+ and YP3O9:V3+. Chem. Phys. Lett. 1989, 154, 425–431. [Google Scholar] [CrossRef]
- Schaeffer, C.E.; Jorgensen, C.K. The angular overlap model, an attempt to revive the ligand field approaches. Mol. Phys. 1965, 9, 401–412. [Google Scholar] [CrossRef]
- Schäffer, C.E. A perturbation representation of weak covalent bonding. Struct. Bonding. 1968, 5, 68–95. [Google Scholar] [CrossRef]
- Jorgensen, C.K. The Nephelauxetic Series. Prog. Inorg. Chem. 1962, 4, 73–124. [Google Scholar] [CrossRef]
- Mironov, V.S.; Bazhenova, T.A.; Manakin, Y.V.; Lyssenko, K.A.; Talantsev, A.D.; Yagubskii, E.B. A new Mo(IV) complex with the pentadentate (N3O2) Schiff-base ligand: The first non-cyanide pentagonal–bipyramidal paramagnetic 4d complex. Dalton Trans. 2017, 46, 14083–14087. [Google Scholar] [CrossRef] [PubMed]
- Manakin, Y.V.; Mironov, V.S.; Bazhenova, T.A.; Lyssenko, K.A.; Gilmutdinov, I.F.; Bikbaev, K.S.; Masitov, A.A.; Yagubskii, E.B. (Et4N)[MoIII(DAPBH)Cl2], the first pentagonal-bipyramidal Mo(III) complex with a N3O2-type Schiff-base ligand: Manifestation of unquenched orbital momentum and Ising-type magnetic anisotropy. Chem. Commun. 2018, 54, 10084–10087. [Google Scholar] [CrossRef]
- Bazhenova, T.A.; Zorina, L.V.; Simonov, S.V.; Mironov, V.S.; Maximova, O.V.; Spillecke, L.; Koo, C.; Klingeler, R.; Manakin, Y.V.; Vasiliev, A.N.; et al. The first pentagonal-bipyramidal vanadium(iii) complexes with a Schiff-base N3O2 pentadentate ligand: Synthesis, structure and magnetic properties. Dalton Trans. 2020, 49, 15287–15298. [Google Scholar] [CrossRef]
- Campanella, A.J.; Ozvat, T.M.; Zadrozny, J.M. Ligand design of zero-field splitting in trigonal prismatic Ni(ii) cage complexes. Dalton Trans. 2022, 51, 3341–3348. [Google Scholar] [CrossRef]
- Nehrkorn, J.; Veber, S.L.; Zhukas, L.A.; Novikov, V.V.; Nelyubina, Y.V.; Voloshin, Y.Z.; Holldack, K.; Stoll, S.; Schnegg, A. Determination of Large Zero-Field Splitting in High-Spin Co(I) Clathrochelates. Inorg. Chem. 2018, 57, 15330–15340. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mironov, V.S. Reaching the Maximal Unquenched Orbital Angular Momentum L = 3 in Mononuclear Transition-Metal Complexes: Where, When and How? Inorganics 2022, 10, 227. https://doi.org/10.3390/inorganics10120227
Mironov VS. Reaching the Maximal Unquenched Orbital Angular Momentum L = 3 in Mononuclear Transition-Metal Complexes: Where, When and How? Inorganics. 2022; 10(12):227. https://doi.org/10.3390/inorganics10120227
Chicago/Turabian StyleMironov, Vladimir S. 2022. "Reaching the Maximal Unquenched Orbital Angular Momentum L = 3 in Mononuclear Transition-Metal Complexes: Where, When and How?" Inorganics 10, no. 12: 227. https://doi.org/10.3390/inorganics10120227
APA StyleMironov, V. S. (2022). Reaching the Maximal Unquenched Orbital Angular Momentum L = 3 in Mononuclear Transition-Metal Complexes: Where, When and How? Inorganics, 10(12), 227. https://doi.org/10.3390/inorganics10120227