Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles
Abstract
:1. Introduction
2. Quantum Interference in a V-Type Quantum Emitter
3. Computational Method
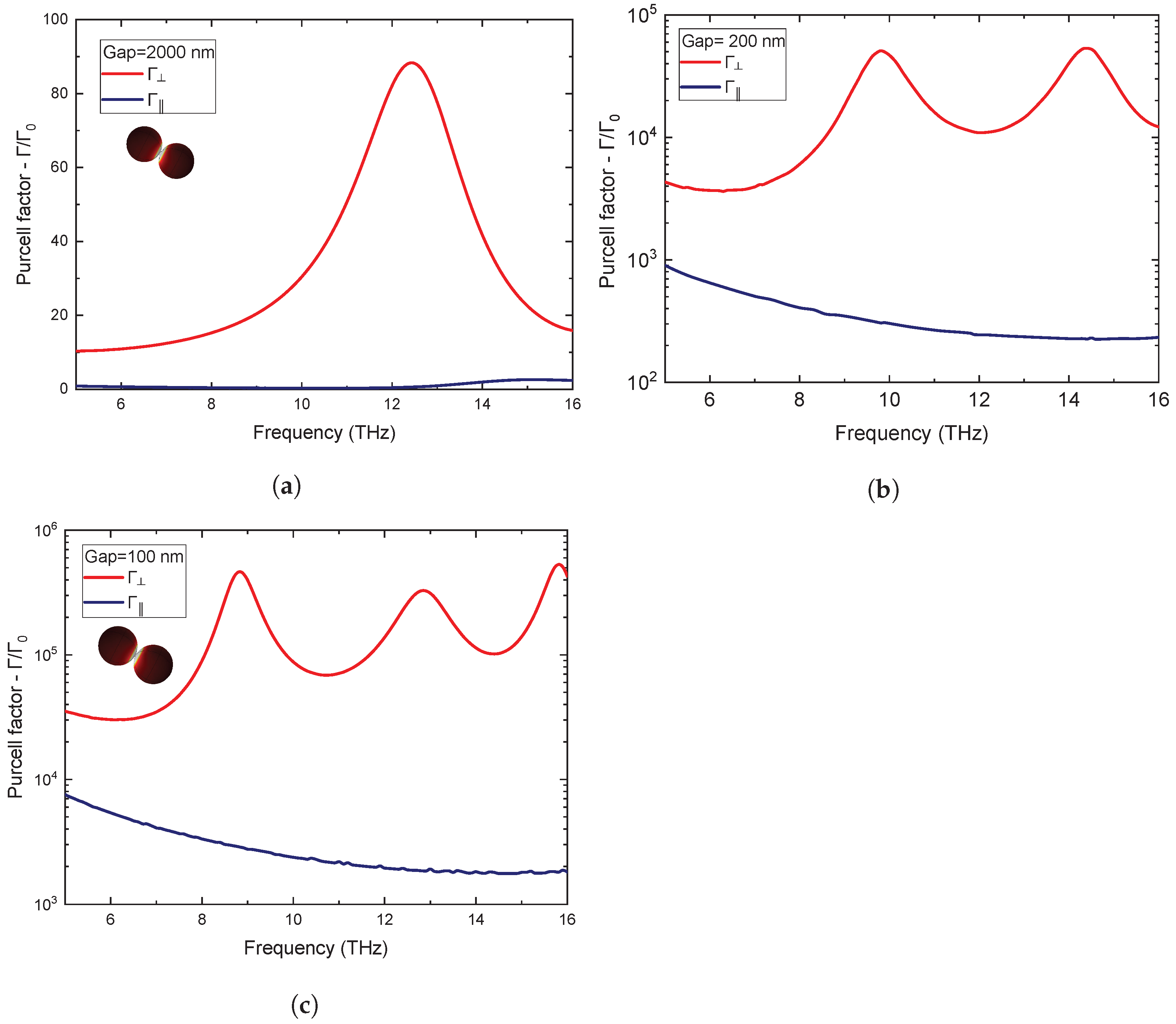
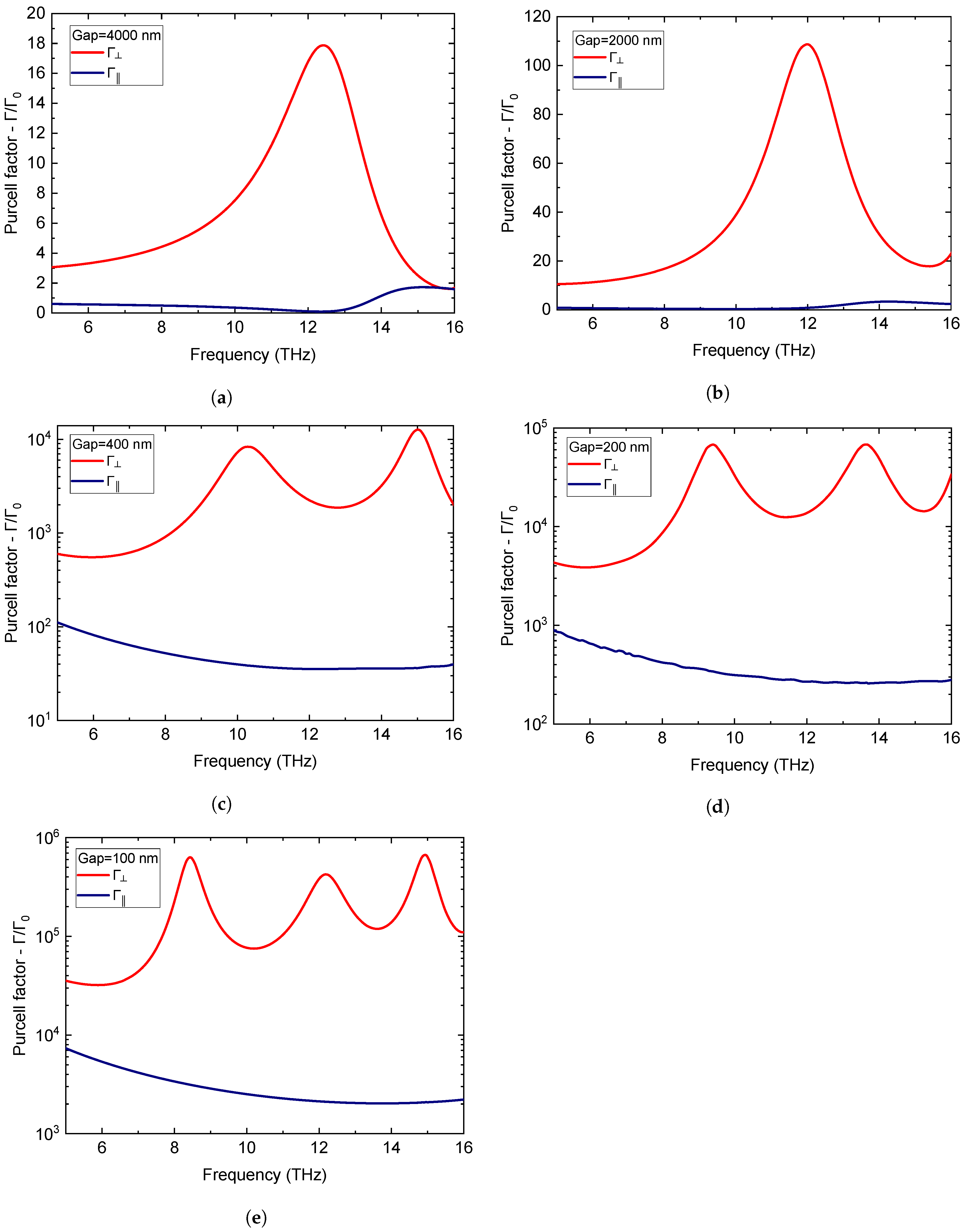
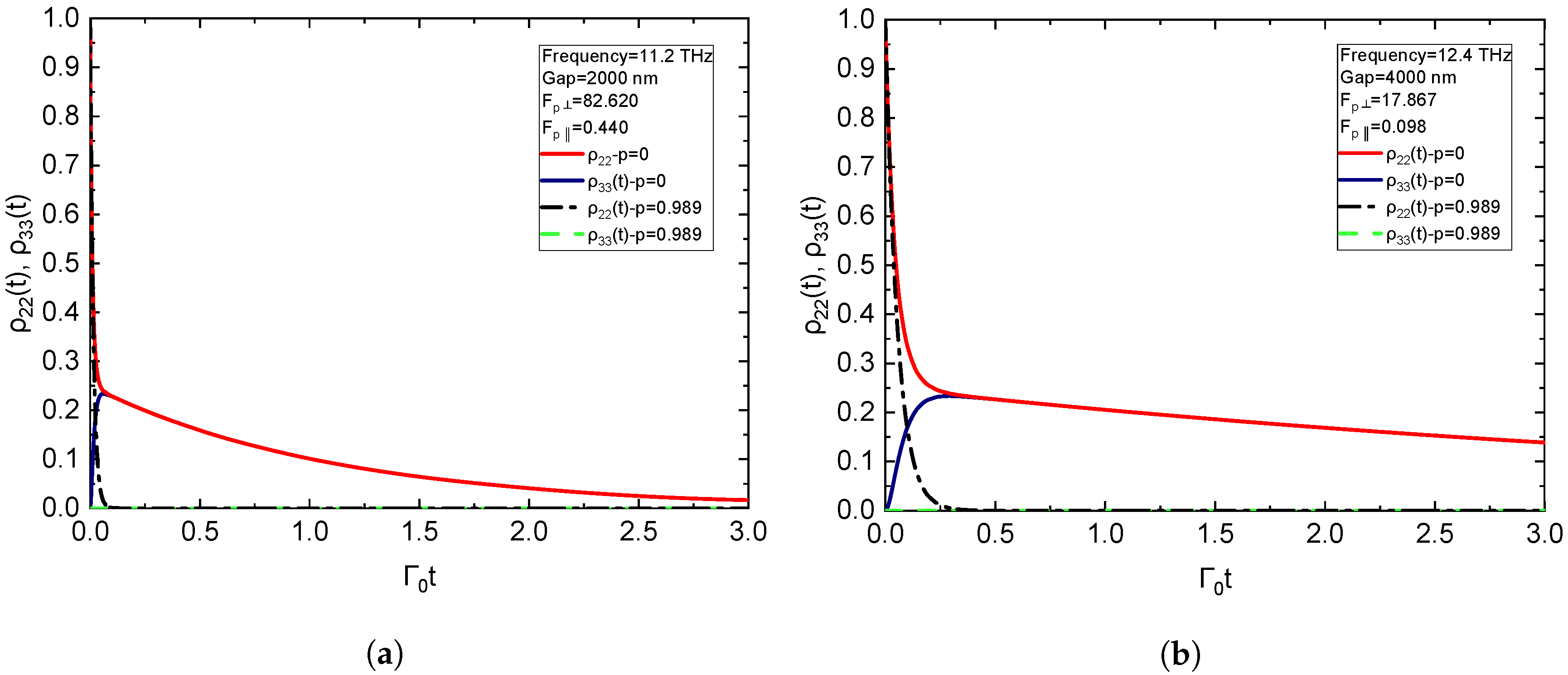
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Purcell, E.M. Spontaneous emission probabilities at radio frequencies. Phys. Rev. 1946, 69, 681. [Google Scholar]
- Drexhage, K.H. Influence of a dielectric interface on fluorescence decay time. J. Luminesc. 1970, 1, 693–701. [Google Scholar] [CrossRef]
- Drexhage, K.H. Interaction of light with monomolecular dye lasers. Prog. Opt. 1974, 12, 163–232. [Google Scholar]
- Barnes, W.; Horsley, S.; Vos, W. Classical antennae, quantum emitters, and densities of optical states. J. Opt. 2019, 22, 073501. [Google Scholar] [CrossRef] [Green Version]
- Iwase, H.; Englund, D.; Vučković, J. Analysis of the Purcell effect in photonic and plasmonic crystals with losses. Opt. Express 2010, 18, 16546–16560. [Google Scholar] [CrossRef] [PubMed]
- Ivanov, K.A.; Morozov, K.M.; Pozina, G.; Gubaydullin, A.R.; Girshova, E.I.; Kaliteevski, M.A. Control of the surface plasmon dispersion and Purcell effect at the metamaterial-dielectric interface. Sci. Rep. 2020, 10, 20828. [Google Scholar]
- Adl, H.P.; Gorji, S.; Habil, M.K.; Suárez, I.; Chirvony, V.S.; Gualdrón-Reyes, A.F.; Mora-Seró, I.; Valencia, L.M.; de la Mata, M.; Hernández-Saz, J.; et al. Purcell Enhancement and Wavelength Shift of Emitted Light by CsPbI3 Perovskite Nanocrystals Coupled to Hyperbolic Metamaterials. ACS Photonics 2020, 7, 3152–3160. [Google Scholar] [CrossRef]
- Karanikolas, V.; Paspalakis, E. Plasmon-induced quantum interference near carbon nanostructures. J. Phys. Chem. C 2018, 122, 1478. [Google Scholar] [CrossRef]
- Yannopapas, V.; Vitanov, N.V. Spontaneous emission of two-level atoms placed within clusters of metallic nanoparticles. J. Phys. Cond. Matter 2007, 19, 096210. [Google Scholar] [CrossRef]
- Yannopapas, V.; Paspalakis, E.; Vitanov, N.V. Plasmon-induced enhancement of quantum interference near metallic nanostructures. Phys. Rev. Lett. 2009, 103, 063602. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Simulating quantum interference in spontaneous decay near plasmonic nanostructures: Population dynamics. Phys. Rev. A 2011, 83, 055805. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Modifying free-space spontaneous emission near a plasmonic nanostructure. Phys. Rev. A 2011, 83, 023819. [Google Scholar] [CrossRef]
- Tame, M.; McEnery, K.; Özdemir, Ş.; Lee, J.; Maier, S.A.; Kim, M.S. Quantum plasmonics. Nat. Phys. 2013, 9, 329–340. [Google Scholar] [CrossRef] [Green Version]
- Chikkaraddy, R.; de Nijs, B.; Benz, F.; Barrow, S.J.; Scherman, O.A.; Rosta, E.; Demetriadou, A.; Fox, P.; Hess, O.; Baumberg, J.J. Single-molecule strong coupling at room temperature in plasmonic nanocavities. Nature 2016, 535, 127–130. [Google Scholar] [CrossRef] [Green Version]
- Gonçalves, P.; Christensen, T.; Rivera, N.; Jauho, A.P.; Mortensen, N.A.; Soljačić, M. Plasmon-emitter interactions at the nanoscale. Nat. Commun. 2020, 11, 366. [Google Scholar] [CrossRef] [Green Version]
- Dubroka, A.; Caha, O.; Hroncek, M.; Friš, P.; Orlita, M.; Holý, V.; Steiner, H.; Bauer, G.; Springholz, G.; Humlicek, J. Interband absorption edge in the topological Bi(Te1−xSex)3. Phys. Rev. B 2017, 96, 235202. [Google Scholar] [CrossRef] [Green Version]
- Rider, M.S.; Sokolikova, M.; Hanham, S.M.; Navarro-Cia, M.; Haynes, P.D.; Lee, D.K.K.; Daniele, M.; Guidi, M.C.; Mattevi, C.; Lupi, S.; et al. Experimental signature of a topological quantum dot. Nanoscale 2020, 12, 22817–22825. [Google Scholar] [CrossRef]
- Siroki, G.; Lee, D.; Haynes, P. Single-electron induced surface plasmons on a topological nanoparticle. Nat. Commun. 2016, 7, 12375. [Google Scholar] [CrossRef] [Green Version]
- Colas des Francs, G.; Barthes, J.; Bouhelier, A.; Weeber, J.C.; Dereux, A.; Cuche, A.; Girard, C. Plasmonic Purcell factor and coupling efficiency to surface plasmons. Implications for addressing and controlling optical nanosources. J. Opt. 2016, 18, 094005. [Google Scholar] [CrossRef] [Green Version]
- Chatzidakis, G.D.; Yannopapas, V. Strong electromagnetic coupling in dimers of topological-insulator nanoparticles and quantum emitters. Phys. Rev. B 2020, 101, 165410. [Google Scholar] [CrossRef]
- Karaoulanis, D.; Paspalakis, E.; Yannopapas, V. Quantum interference near bismuth-chalcogenide microstructures. J. Opt. Soc. Am. B 2021, 38, 3301–3308. [Google Scholar] [CrossRef]
- Vladimirova, Y.V.; Zadkov, V.N. Quantum Optics in Nanostructures. Nanomaterials 2021, 11, 1919. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, G.S. Anisotropic Vacuum-Induced Interference in Decay Channels. Phys. Rev. Lett. 2000, 84, 5500–5503. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Paspalakis, E.; Keitel, C.H.; Knight, P.L. Fluorescence control through multiple interference mechanisms. Phys. Rev. A 1998, 58, 4868. [Google Scholar] [CrossRef] [Green Version]
- Ficek, Z.; Swain, S. Simulating quantum interference in a three-level system with perpendicular transition dipole moments. Phys. Rev. A 2004, 69, 023401. [Google Scholar] [CrossRef] [Green Version]
- Zhu, S.-Y.; Scully, M.O. Spectral line elimination and spontaneous emission cancellation via quantum interference. Phys. Rev. Lett. 1996, 76, 388–391. [Google Scholar] [CrossRef]
- Menon, S.; Agarwal, G.S. Gain components in the Autler-Townes doublet from quantum interferences in decay channels. Phys. Rev. A 1999, 61, 013807. [Google Scholar] [CrossRef] [Green Version]
- Hughes, S.; Agarwal, G.S. Anisotropy-Induced Quantum Interference and Population Trapping between Orthogonal Quantum Dot Exciton States in Semiconductor Cavity Systems. Phys. Rev. Lett. 2017, 118, 063601. [Google Scholar] [CrossRef] [Green Version]
- Das, S.; Agarwal, G.S. Protecting bipartite entanglement by quantum interferences. Phys. Rev. A 2010, 81, 052341. [Google Scholar] [CrossRef] [Green Version]
- Iliopoulos, N.; Terzis, A.F.; Yannopapas, V.; Paspalakis, E. Prolonging entanglement dynamics near periodic plasmonic nanostructures. Phys. Rev. B 2017, 96, 075405. [Google Scholar] [CrossRef]
- Sangshekan, B.; Sahrai, M.; Asadpour, S.H. Controllable atom-photon entanglement via quantum interference near plasmonic nanostructure. Sci. Rep. 2022, 12, 677. [Google Scholar] [CrossRef] [PubMed]
- Zhou, P.; Swain, S. Quantum interference in probe absorption: Narrow resonances, transparency, and gain without population inversion. Phys. Rev. Lett. 1997, 78, 832–835. [Google Scholar] [CrossRef]
- Paspalakis, E.; Gong, S.-Q.; Knight, P.L. Spontaneous emission induced coherent effects in absorption and dispersion of a V-type three-level atom. Opt. Commun. 1998, 152, 293–298. [Google Scholar] [CrossRef]
- Zhou, P.; Swain, S. Ultranarrow spectral lines via quantum interference. Phys. Rev. Lett. 1996, 77, 3995. [Google Scholar] [CrossRef]
- Wang, C.-L.; Kang, Z.-H.; Tian, S.-C.; Jiang, Y.; Gao, J.-Y. Effect of spontaneously generated coherence on absorption in a V-type system: Investigation in dressed states. Phys. Rev. A 2009, 79, 043810. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Transparency and slow light in a four-level quantum system near a plasmonic nanostructure. Phys. Rev. A 2012, 86, 053811. [Google Scholar] [CrossRef]
- Li, L.; Nie, W.; Chen, A. Transparency and tunable slow and fast light in a nonlinear optomechanical cavity. Sci. Rep. 2016, 6, 35090. [Google Scholar] [CrossRef] [Green Version]
- Frogley, M.D.; Dynes, J.F.; Beck, M.; Faist, J.; Phillips, C.C. Gain without inversion in semiconductor nanostructures. Nat. Mater 2006, 5, 175–178. [Google Scholar] [CrossRef]
- Paspalakis, E.; Kylstra, N.J.; Knight, P.L. Transparency induced via decay interference. Phys. Rev. Lett. 1999, 82, 2079. [Google Scholar] [CrossRef] [Green Version]
- Bortman-Arbiv, D.; Wilson-Gordon, A.D.; Friedmann, H. Phase control of group velocity: From subluminal to superluminal light propagation. Phys. Rev. A 2001, 63, 043818. [Google Scholar] [CrossRef]
- Gurudev Dutt, M.V.; Cheng, J.; Li, B.; Xu, X.; Li, X.; Berman, P.R.; Steel, D.G.; Bracker, A.S.; Gammon, D.; Economou, S.E.; et al. Stimulated and spontaneous optical generation of electron spin coherence in charged GaAs quantum dots. Phys. Rev. Lett. 2005, 94, 227403. [Google Scholar] [CrossRef] [Green Version]
- Niu, Y.-P.; Gong, S.-Q. Enhancing Kerr nonlinearity via spontaneously generated coherence. Phys. Rev. A 2006, 73, 053811. [Google Scholar] [CrossRef]
- Asadpour, S.H.; Sahrai, M.; Soltani, A.; Hamedi, H.R. Enhanced Kerr nonlinearity via quantum interference from spontaneous emission. Phys. Lett. A 2012, 376, 147–152. [Google Scholar] [CrossRef]
- Yannopapas, V.; Vitanov, N.V. Electromagnetic Green’s tensor and local density of states calculations for collections of spherical scatterers. Phys. Rev. B 2007, 75, 115124. [Google Scholar] [CrossRef]
- Rosolen, G.; Maes, B.; Chen, P.Y.; Sivan, Y. Overcoming the bottleneck for quantum computations of complex nanophotonic structures: Purcell and Förster resonant energy transfer calculations using a rigorous mode-hybridization method. Phys. Rev. B. 2020, 101, 155401. [Google Scholar] [CrossRef] [Green Version]
- Gallinet, B.; Butet, J.; Martin, O.J. Numerical methods for nanophotonics: Standard problems and future challenges. Laser Photonics Rev. 2015, 9, 577–603. [Google Scholar] [CrossRef]
- Pelton, M. Modified spontaneous emission in nanophotonic structures. Nat. Photon. 2015, 9, 427–435. [Google Scholar] [CrossRef]
- Thanopulos, I.; Yannopapas, V.; Paspalakis, E. Non-Markovian dynamics in plasmon-induced spontaneous emission interference. Phys. Rev. B 2017, 95, 075412. [Google Scholar] [CrossRef] [Green Version]
- Leng, H.; Szychowski, B.; Daniel, M.-C.; Pelton, M. Strong coupling and induced transparency at room temperature with single quantum dots and gap plasmons. Nat. Commun. 2018, 9, 4012. [Google Scholar] [CrossRef]
- Agarwal, G.S. Spectroscopy of strongly coupled atom–cavity systems: A topical review. J. Mod. Opt. 1998, 45, 449–470. [Google Scholar] [CrossRef]
- Dovzhenko, D.S.; Ryabchuk, S.V.; Rakovich, Y.P.; Nabiev, I.R. Light–matter interaction in the strong coupling regime: Configurations, conditions, and applications. Nanoscale 2018, 10, 3589–3605. [Google Scholar] [CrossRef] [PubMed]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kyvelos, N.; Tsigaridas, G.; Paspalakis, E.; Yannopapas, V. Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles. Photonics 2022, 9, 596. https://doi.org/10.3390/photonics9090596
Kyvelos N, Tsigaridas G, Paspalakis E, Yannopapas V. Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles. Photonics. 2022; 9(9):596. https://doi.org/10.3390/photonics9090596
Chicago/Turabian StyleKyvelos, Nikolaos, Georgios Tsigaridas, Emmanuel Paspalakis, and Vassilios Yannopapas. 2022. "Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles" Photonics 9, no. 9: 596. https://doi.org/10.3390/photonics9090596
APA StyleKyvelos, N., Tsigaridas, G., Paspalakis, E., & Yannopapas, V. (2022). Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles. Photonics, 9(9), 596. https://doi.org/10.3390/photonics9090596






