Planar Waveguide-Based Fiber Spectrum Analyzer Mountable to Commercial Camera
Abstract
:1. Introduction
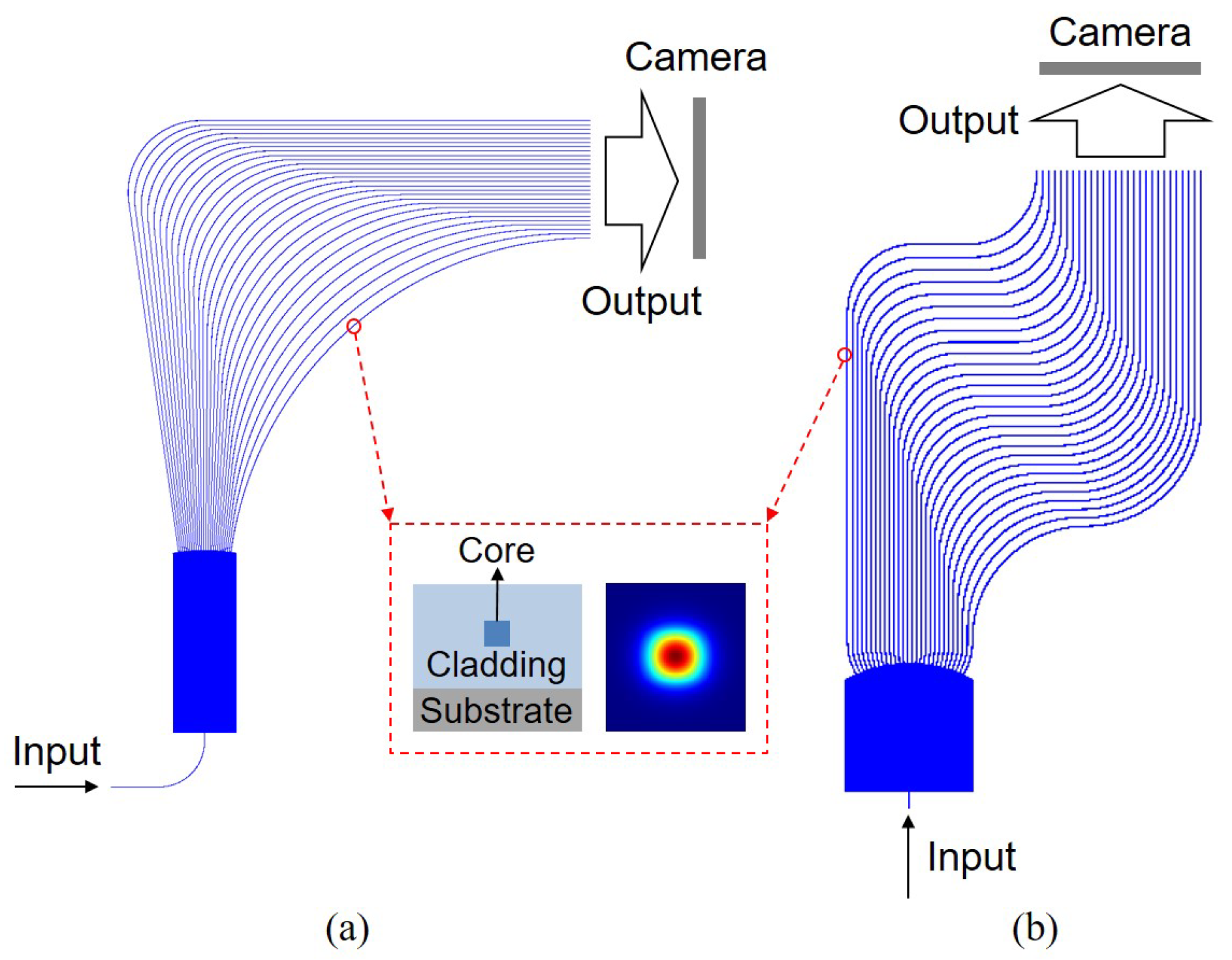
2. Device Structure and Operation Principle
2.1. Device Structure and Waveguide Length Difference
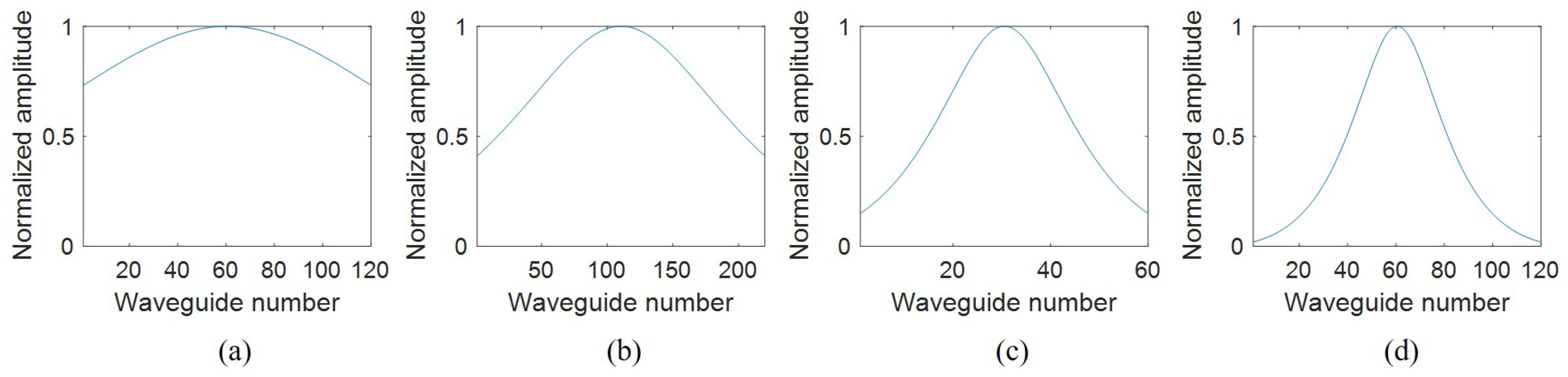
2.2. Calculation of Structure Parameters
2.2.1. Detailed Geometric Structures of WSLs
2.2.2. Derivation for the Formulas of Structural Parameters
2.2.3. Calculation of Structural Parameters Based on the Formulas
2.3. Calculation of Focal Depth
3. Device Function Definition
3.1. Waveguide Lens Only
3.2. WSLs with Different Focal Lengths to Camera
3.3. WSLs with High Spectral Resolution
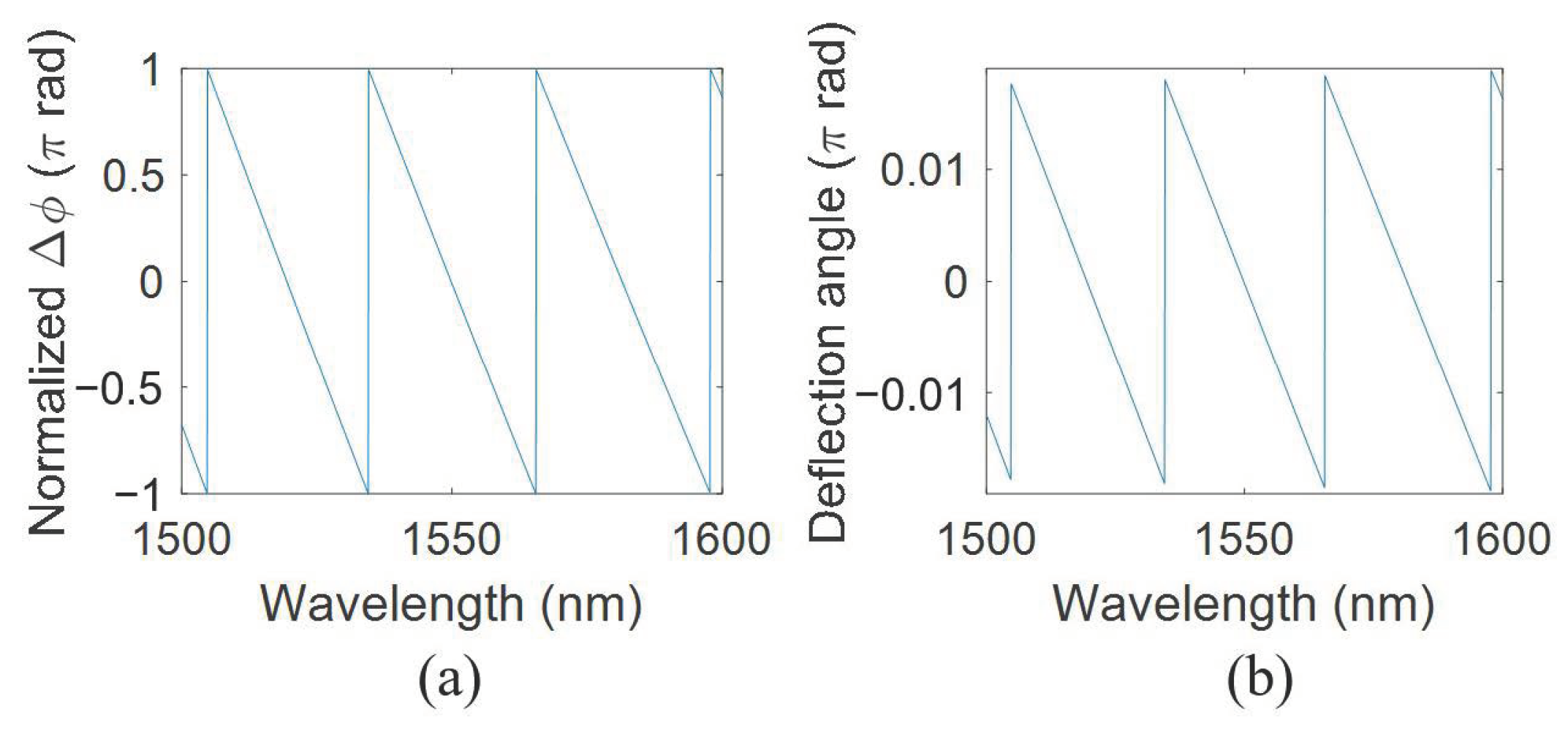
3.4. WSL with Large FSR
4. Device Fabrication and Testing Results
4.1. Device Fabrication
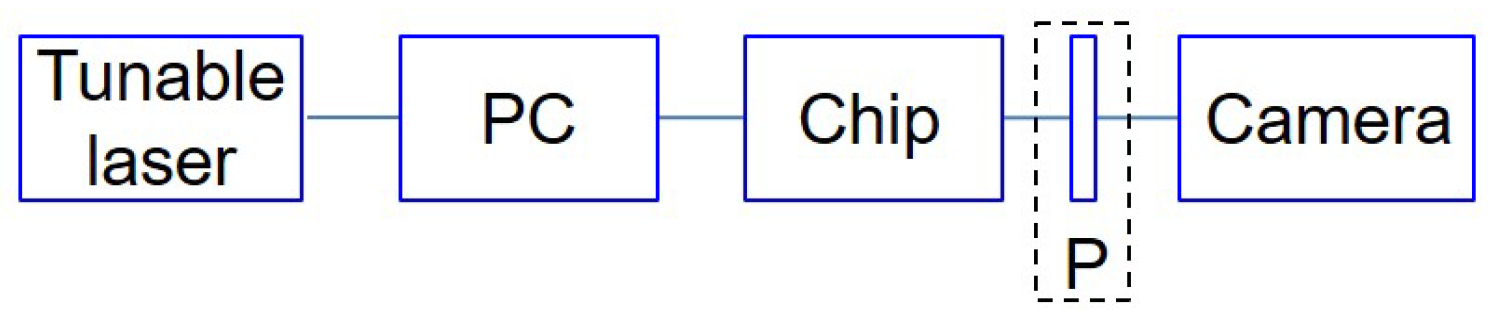
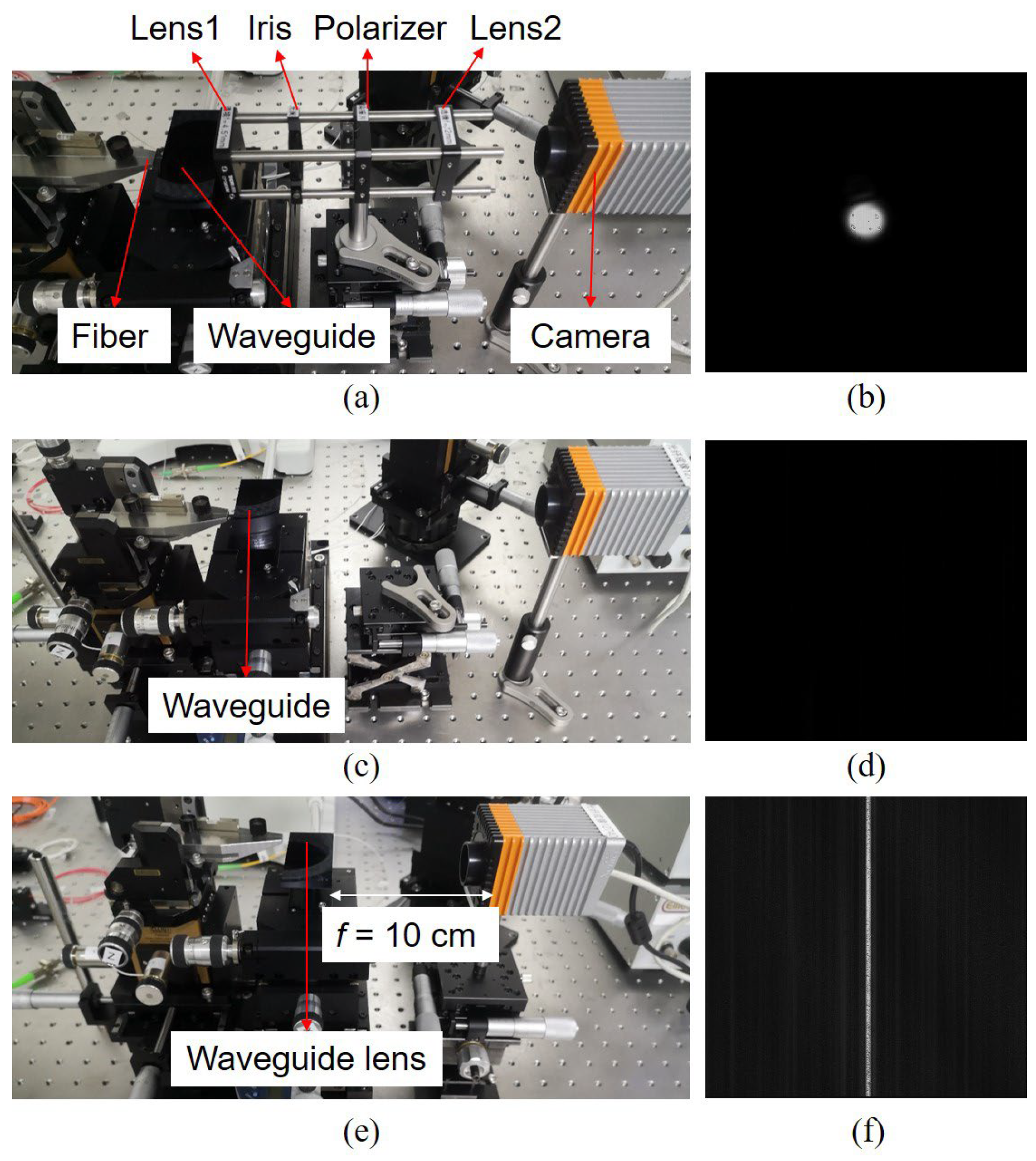
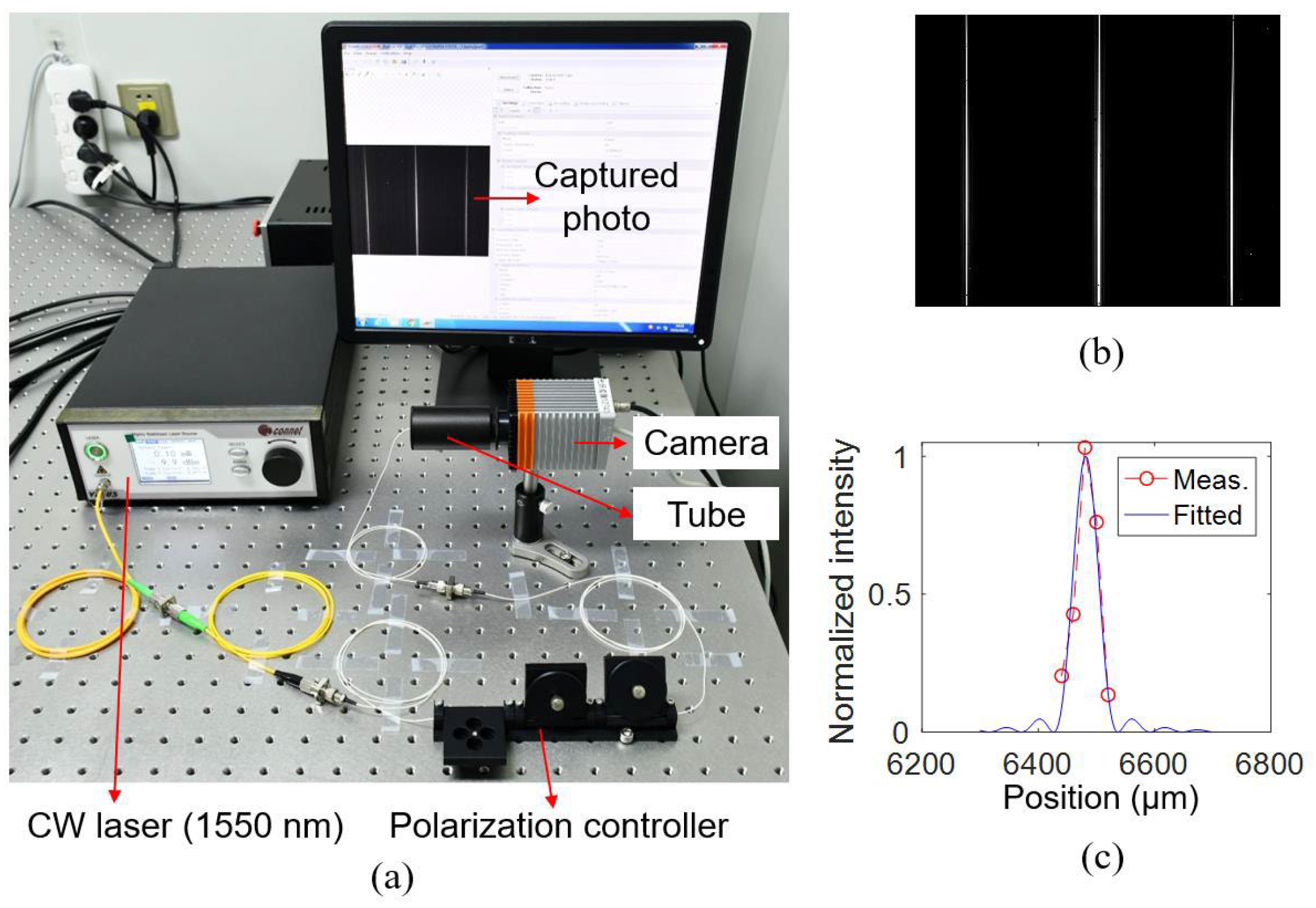
4.2. Experimental Setup
4.3. Waveguide Lens
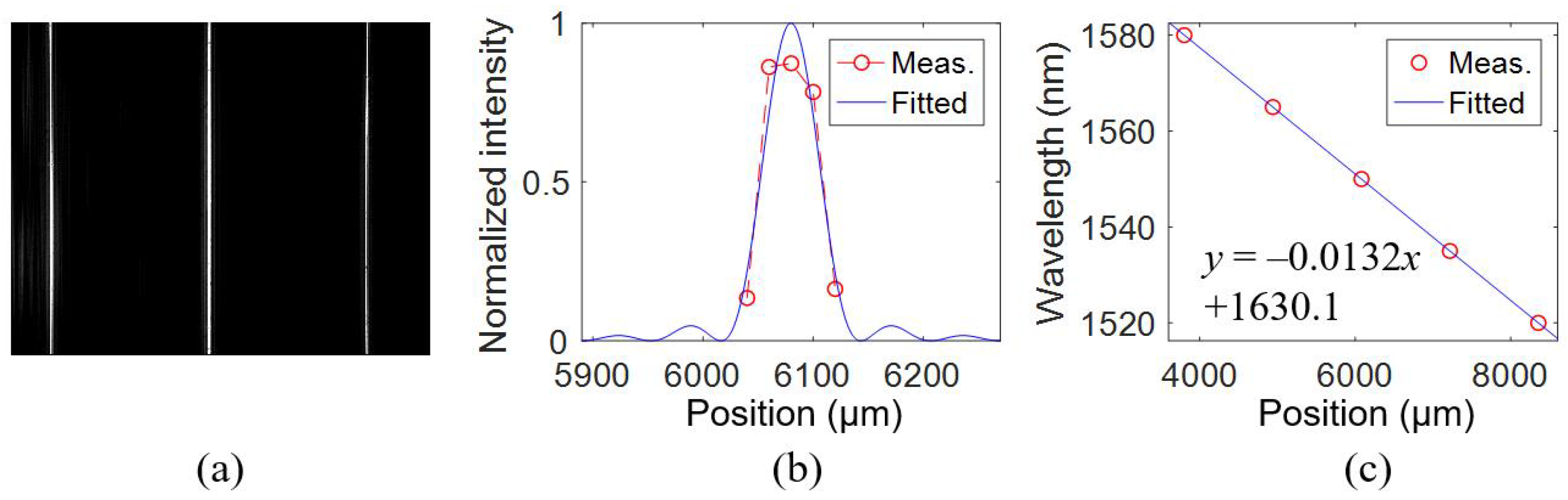
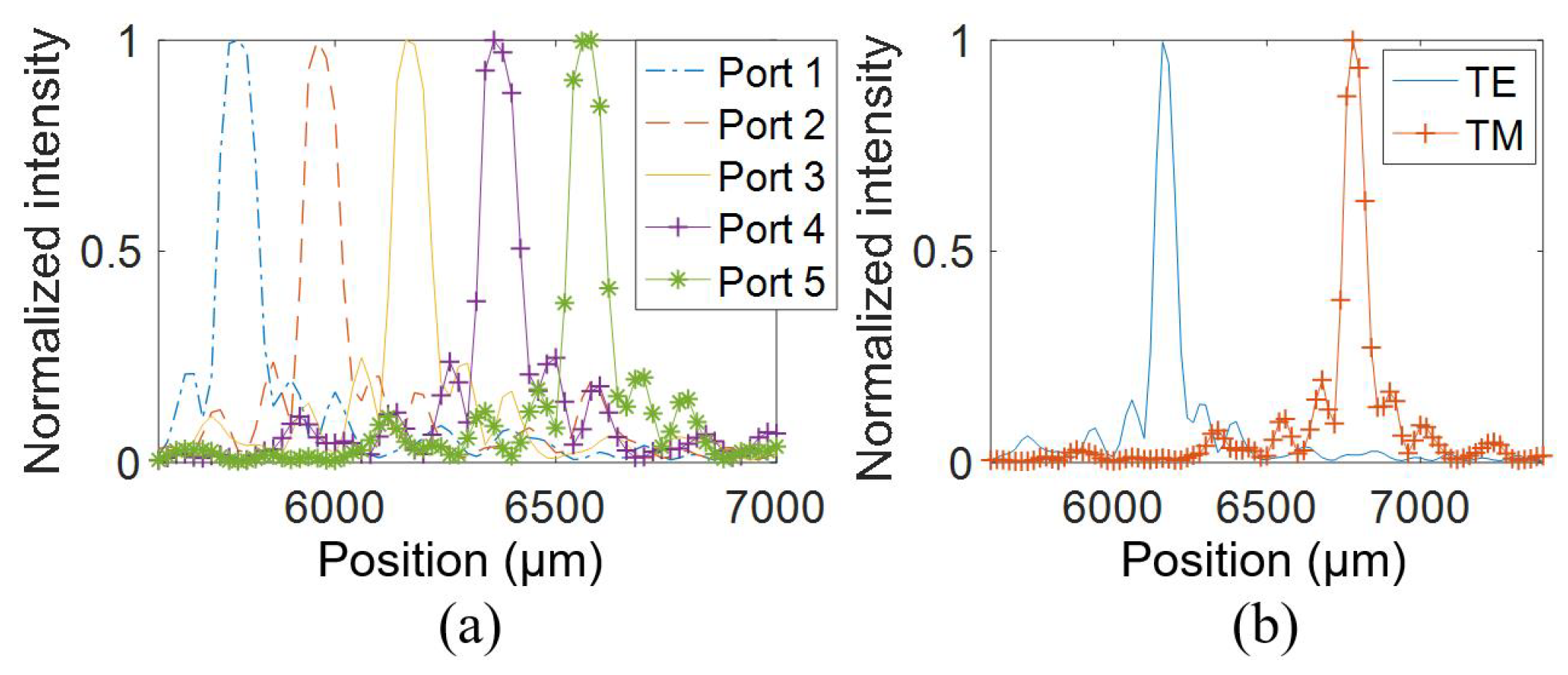
4.4. WSLs with Different Focal Lengths
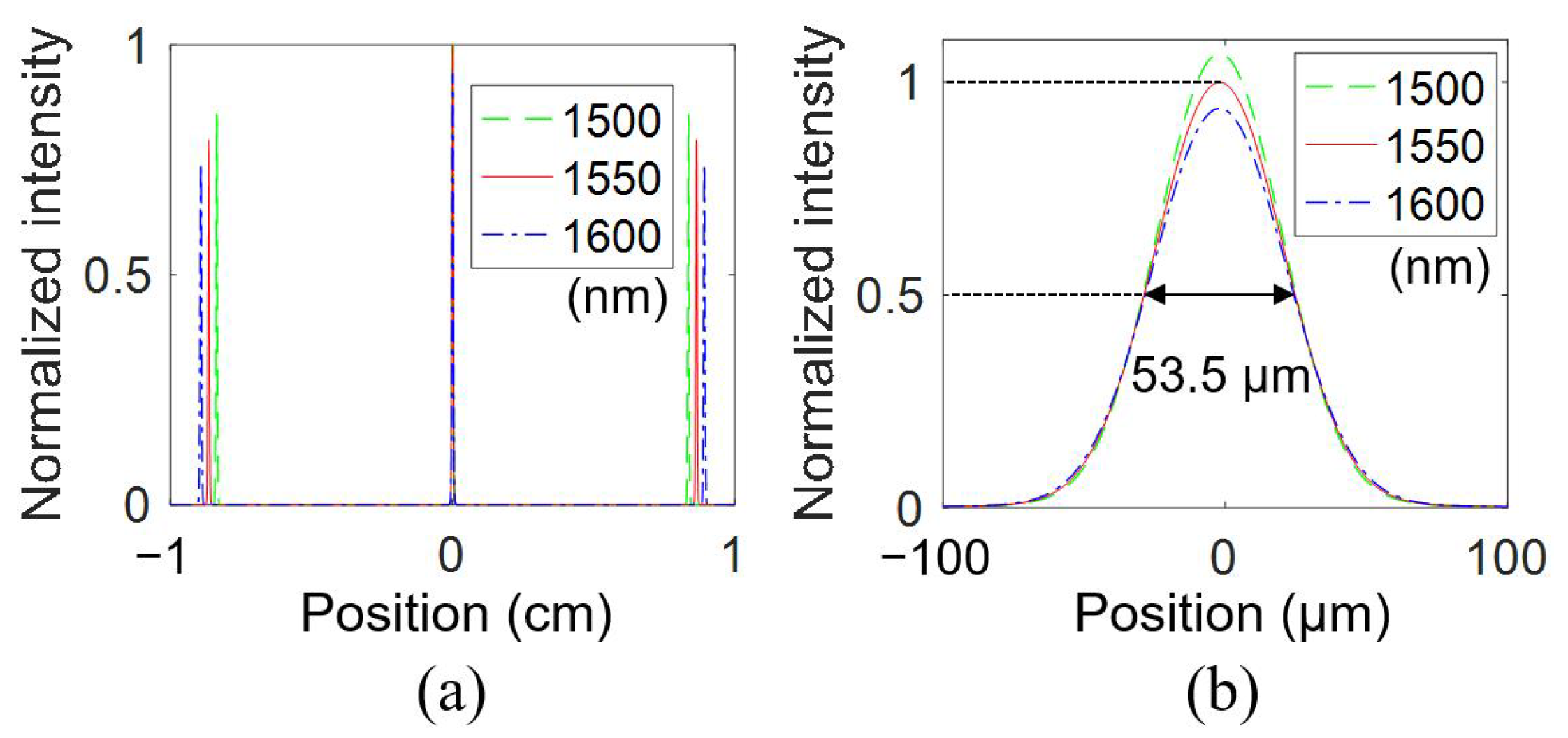
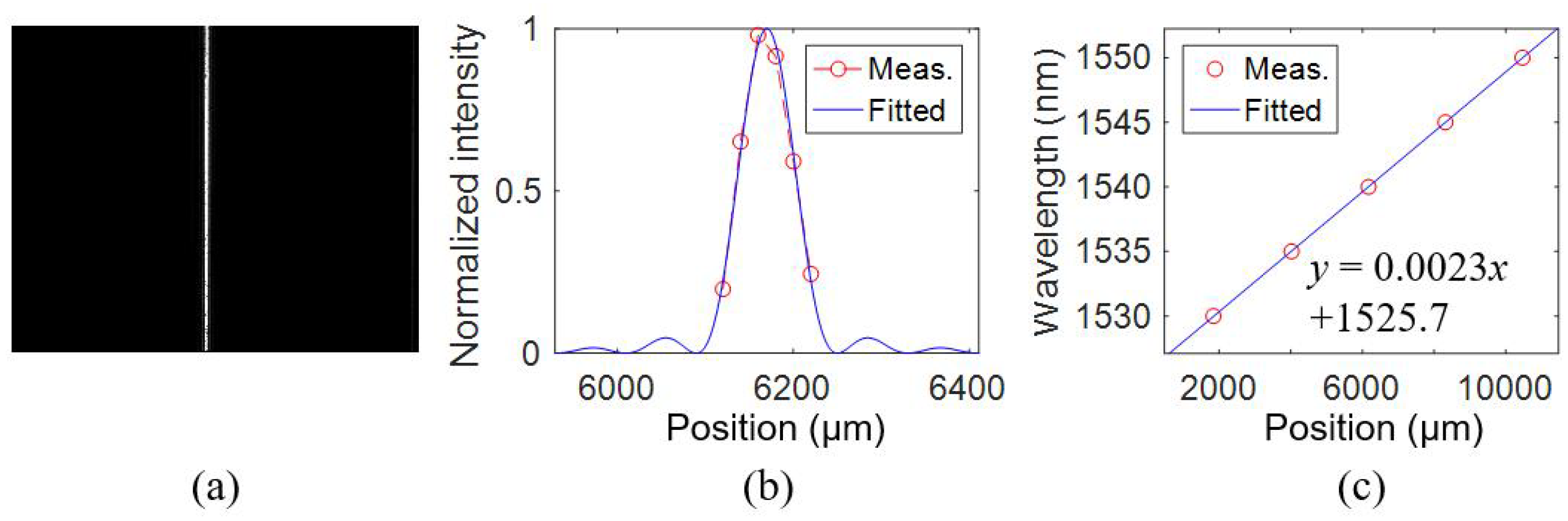
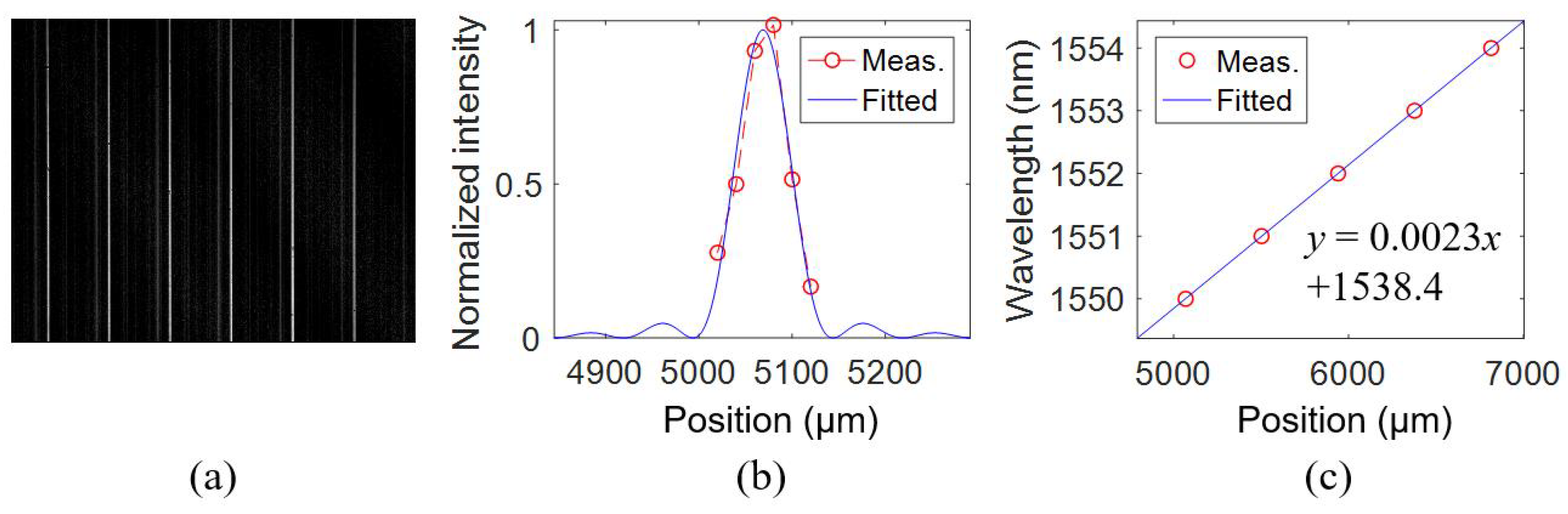
4.5. WSLs with High Spectral Resolution
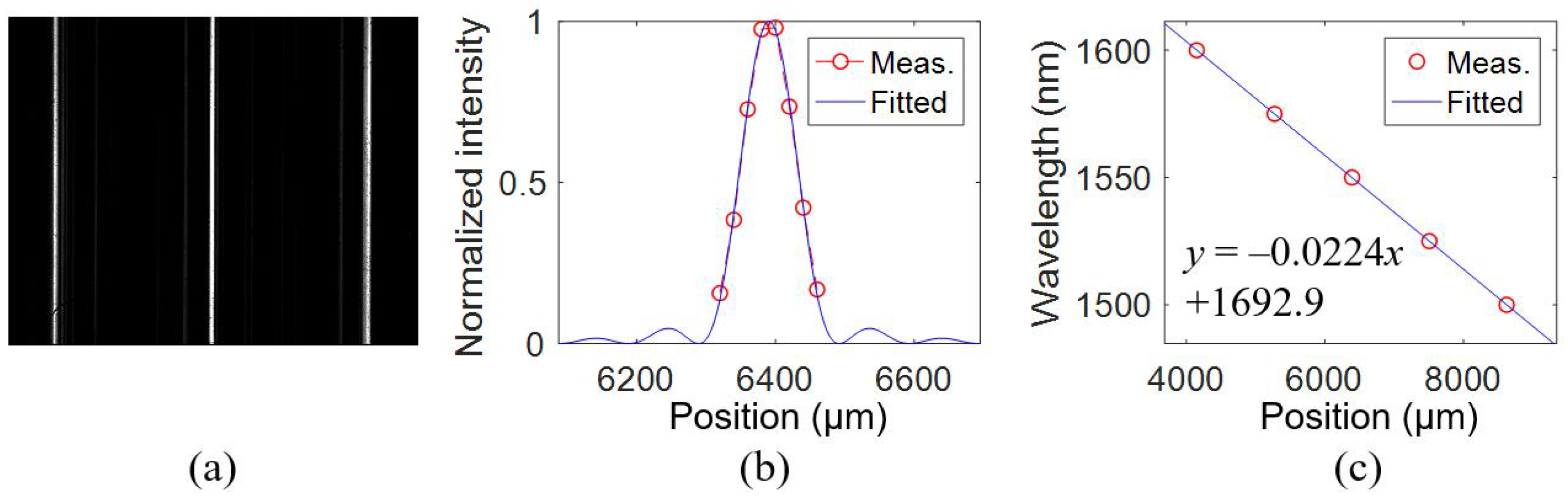
4.6. WSL with Large FSR
4.7. Measured Spectra of a Multiwavelength Laser
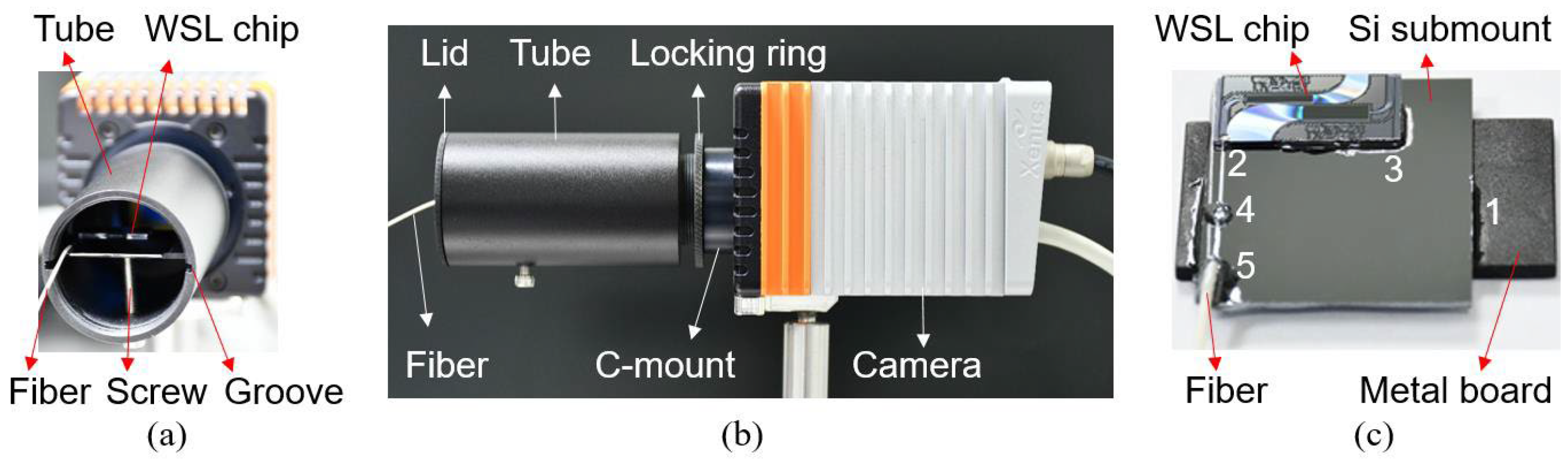
5. Mounting the WSL Chip to a Camera
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gutzler, R.; Garg, M.; Ast, C.R.; Kuhnke, K.; Kern, K. Light–Matter Interaction at Atomic Scales. Nat. Rev. Phys. 2021, 3, 441–453. [Google Scholar] [CrossRef]
- Gu, C.; Zuo, Z.; Luo, D.; Deng, Z.; Liu, Y.; Hu, M.; Li, W. Passive Coherent Dual-Comb Spectroscopy Based on Optical-Optical Modulation with Free Running Lasers. PhotoniX 2020, 1, 7. [Google Scholar] [CrossRef]
- Peng, Y.; Shi, C.; Zhu, Y.; Gu, M.; Zhuang, S. Terahertz Spectroscopy in Biomedical Field: A Review on Signal-to-Noise Ratio Improvement. PhotoniX 2020, 1, 12. [Google Scholar] [CrossRef] [Green Version]
- Gatkine, P.; Veilleux, S.; Dagenais, M. Astrophotonic Spectrographs. Appl. Sci. 2019, 9, 290. [Google Scholar] [CrossRef] [Green Version]
- Wijk, A.V.; Doerr, C.R.; Ali, Z.; Karabiyik, M.; Akca, B.I. Compact Ultrabroad-Bandwidth Cascaded Arrayed Waveguide Gratings. Opt. Express 2020, 28, 14618–14626. [Google Scholar] [CrossRef] [PubMed]
- Akca, B.I.; Doerr, C.R. Interleaved Silicon Nitride AWG Spectrometers. IEEE Photon. Technol. Lett. 2019, 30, 90–93. [Google Scholar] [CrossRef]
- Nikbakth, H.; Karabiyik, M.; Akca, B.I. Ultrawide-Bandwidth On-Chip Spectrometer Design Using Band-Pass Filters. Opt. Express 2020, 28, 23003–23011. [Google Scholar] [CrossRef]
- Zou, J.; Ma, X.; Xia, X.; Hu, J.; Wang, C.; Zhang, M.; Lang, T.; He, J. High Resolution and Ultra-Compact On-Chip Spectrometer Using Bidirectional Edge-Input Arrayed Waveguide Grating. IEEE/OSA J. Lightw. Technol. 2020, 38, 4447–4453. [Google Scholar] [CrossRef]
- Zhang, Z.; Mettbach, N.; Zawadzki, C.; Wang, J.; Schmidt, D.; Brinker, W.; Grote, N.; Schell, M.; Keil, N. Polymer-Based Photonic Toolbox: Passive Components, Hybrid Integration and Polarisation Control. IET Optoelectronics 2011, 5, 226–232. [Google Scholar] [CrossRef]
- Yun, S.; Han, Y.; Kim, S.; Shin, J.; Park, S.; Lee, D.; Lee, S.; Baek, Y. Compact Hybrid-Integrated 4 × 80-Gbps TROSA Module Using Optical Butt-Coupling of DML/SI-PD and Silica AWG Chips. IEEE/OSA J. Lightw. Technol. 2021, 39, 2468–2475. [Google Scholar] [CrossRef]
- Luo, X.; Song, J.; Tu, X.; Fang, Q.; Jia, L.; Huang, Y.; Liow, T.; Yu, M.; Lo, G. Silicon-Based Traveling-Wave Photodetector Array (Si-TWPDA) with Parallel Optical Feeding. Opt. Express 2014, 22, 20020–20026. [Google Scholar] [CrossRef] [PubMed]
- Lv, Q.; Han, Q.; Pan, P.; Ye, H.; Yin, D.; Yang, X. Monolithic Integration of a InP AWG and InGaAs Photodiodes on InP Platform. Opt. Laser Technol. 2017, 90, 122–127. [Google Scholar] [CrossRef]
- Słowikowski, M.; Kazmierczak, A.; Stopinski, S.; Bieniek, M.; Szostak, S.; Matuk, K.; Augustin, L.; Piramidowicz, R. Photonic Integrated Interrogator for Monitoring the Patient Condition During MRI Diagnosis. Sensors 2021, 21, 4238. [Google Scholar] [CrossRef]
- Stoll, A.; Zhang, Z.; Haynes, R.; Roth, M. High-Resolution Arrayed-Waveguide-Gratings in Astronomy: Design and Fabrication Challenges. Photonics 2017, 4, 30. [Google Scholar] [CrossRef] [Green Version]
- Jiang, X.; Yang, Z.; Wu, L.; Dang, Z.; Ding, Z.; Liu, Z.; Chang, Q.; Zhang, Z. Fiber Spectrum Analyzer Based on Planar Waveguide Array Aligned to a Camera without Lens. arXiv 2022, arXiv:2205.03054. Available online: http://arxiv.org/abs/2205.03054 (accessed on 1 June 2022).
- Wang, Z.; Li, T.; Soman, A.; Mao, D.; Kananen, T.; Gu, T. On-Chip Wavefront Shaping with Dielectric Metasurface. Nat. Commun. 2019, 10, 3547. [Google Scholar] [CrossRef]
- Acoleyen, K.V.; Bogaerts, W.; Jágerská, J.; Thomas, N.L.; Houdré, R.; Baets, R. Off-Chip Beam Steering with a One-Dimensional Optical Phased Array on Silicon-on-Insulator. Opt. Lett. 2009, 34, 1477–1479. [Google Scholar] [CrossRef]
- Chung, S.; Abediasl, H.; Hashemi, H. A Monolithically Integrated Large-Scale Optical Phased Array in Silicon-on-Insulator CMOS. IEEE J. Solid-State Circuits 2018, 53, 275–296. [Google Scholar] [CrossRef]
- Ochoa, N.A. Alternative Approach to Evaluate the Rayleigh-Sommerfeld Diffraction Integrals Using Tilted Spherical Waves. Opt. Express 2017, 25, 12008–12019. [Google Scholar] [CrossRef]
- Jiang, X.; Yang, Z.; Liu, Z.; Dang, Z.; Ding, Z.; Chang, Q.; Zhang, Z. 3D Integrated Wavelength Demultiplexer Based on a Square-Core Fiber and Dual-Layer Arrayed Waveguide Gratings. Opt. Express 2021, 29, 2090–2098. [Google Scholar] [CrossRef]
- Xu, W.; Zhou, L.; Lu, L.; Chen, J. Aliasing-Free Optical Phased Array Beam-Steering with a Plateau Envelope. Opt. Express 2019, 27, 3354–3368. [Google Scholar] [CrossRef] [PubMed]
- Strandman, C.; Bäcklund, Y. Bulk Silicon Holding Structures for Mounting of Optical Fibers in V-Grooves. J. Microelectromechanical Syst. 1997, 6, 35–40. [Google Scholar] [CrossRef]




| Parameter | WG Lens | WSL1 | WSL2 | WSL3 | WSL4 |
|---|---|---|---|---|---|
| N | 220 | 120 | 220 | 60 | 120 |
| FSR (nm) | ~ 1 | 64 | 31 | 4.5 | 112 |
| ΔL (μm) | 0 | 25.7 | 53.0 | 365.1 | 14.6 |
| f (cm) | 10 | 5 | 10 | 10 | 10 |
| d (μm) | 18 | 16.5 | 13.5 | 66 | 33 |
| Ro (μm) | 3000 | 6000 | 6000 | 6000 | 6000 |
| Do (μm) | 12 | 8 | 8 | 48 | 36 |
| Lo (μm) | 200 | 200 | 200 | 600 | 500 |
| R (μm) | 1500 | ~ | ~ | ~ | 1500 |
| L1 (μm) | 0 | 0 | 0 | 4000 | 500 |
| K1 (μm) | 0 | 20 | 20 | 20 | 0 |
| R1 (μm) | 2700 | 3000 | 13,500 | 21,000 | 2800 |
| Calculated ΔD (μm) | ~ | 4702.2 | 11,500.4 | 2348.6 | 4698.3 |
| Simulated FWHM (nm) | ~ | 0.50 | 0.14 | 0.09 | 1.47 |
| Focal depth (mm) | 4.30 | 4.51 | 7.48 | 4.17 | 4.9 |
| Measured FWHM (nm) | ~ | 0.74 | 0.16 | 0.15 | 2.00 |
| Measured FSR (nm) | ~ | 64.5 | 29.8 | 4.4 | 112 |
| Size (mm2) | 9.5 × 16.0 | 4.2 × 13.3 | 17.4 × 22.8 | 30.3 × 32.8 | 11.1 × 20.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, X.; Zhang, Z. Planar Waveguide-Based Fiber Spectrum Analyzer Mountable to Commercial Camera. Photonics 2022, 9, 456. https://doi.org/10.3390/photonics9070456
Jiang X, Zhang Z. Planar Waveguide-Based Fiber Spectrum Analyzer Mountable to Commercial Camera. Photonics. 2022; 9(7):456. https://doi.org/10.3390/photonics9070456
Chicago/Turabian StyleJiang, Xinhong, and Ziyang Zhang. 2022. "Planar Waveguide-Based Fiber Spectrum Analyzer Mountable to Commercial Camera" Photonics 9, no. 7: 456. https://doi.org/10.3390/photonics9070456
APA StyleJiang, X., & Zhang, Z. (2022). Planar Waveguide-Based Fiber Spectrum Analyzer Mountable to Commercial Camera. Photonics, 9(7), 456. https://doi.org/10.3390/photonics9070456






