Design and Modelling of Metal-Oxide Nanodisk Arrays for Structural Colors and UV-Blocking Functions in Solar Cell Glass Covers
Abstract
:1. Introduction
2. Simulation Setup
3. Results and Discussion
3.1. Scattering Cross-Section (SCS) of TiO2 Nanodisk Resonator
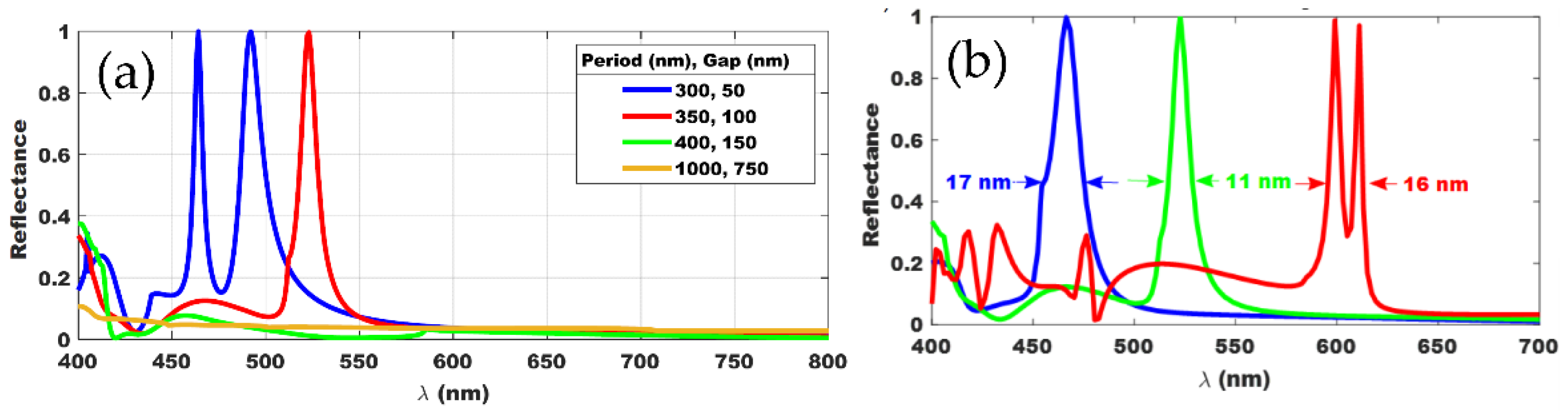
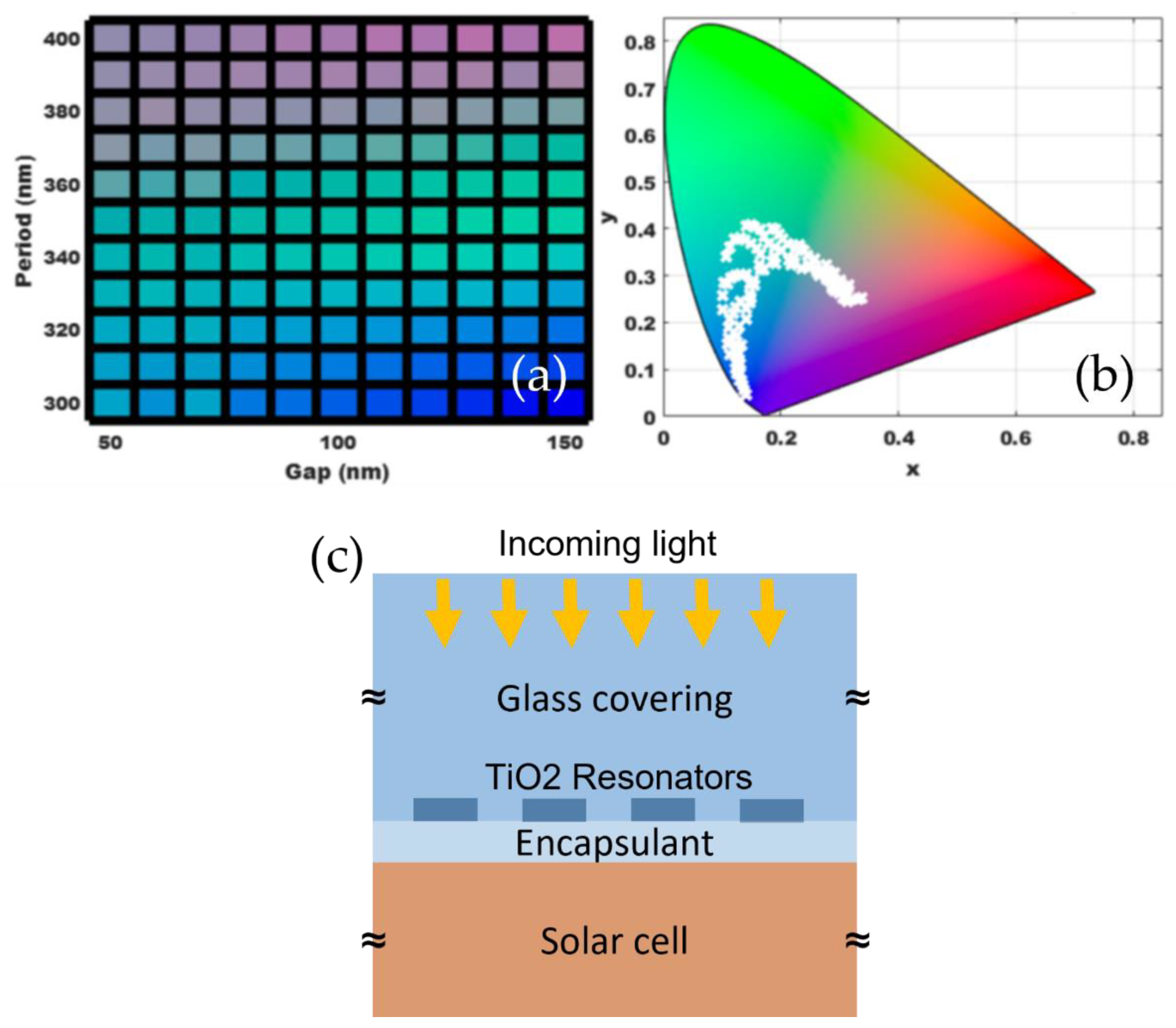
3.2. Reflectance from TiO2 Nanodisk Array
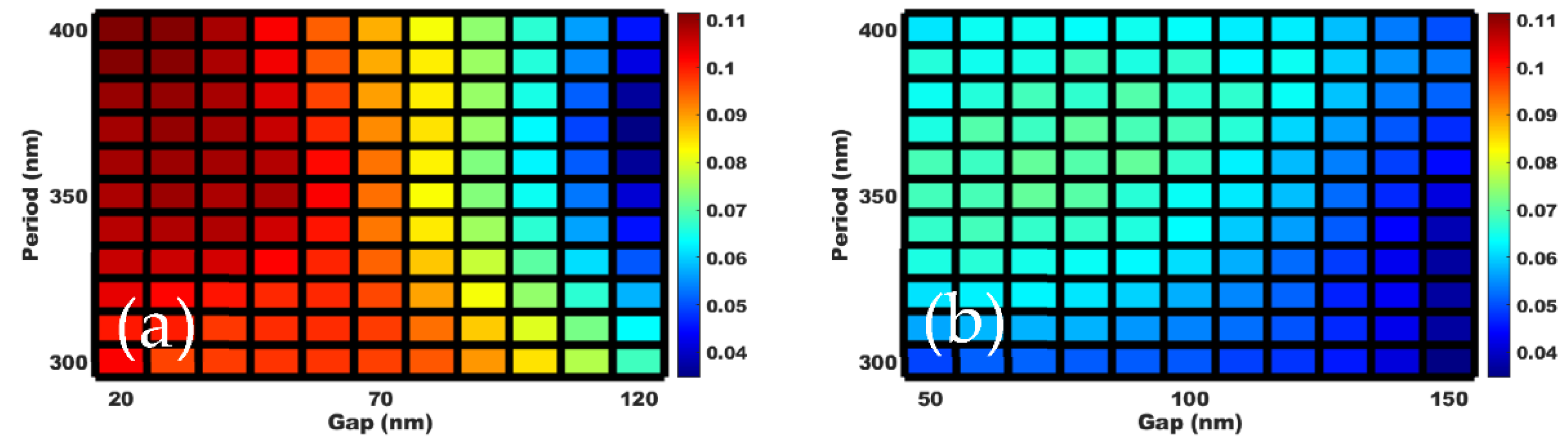
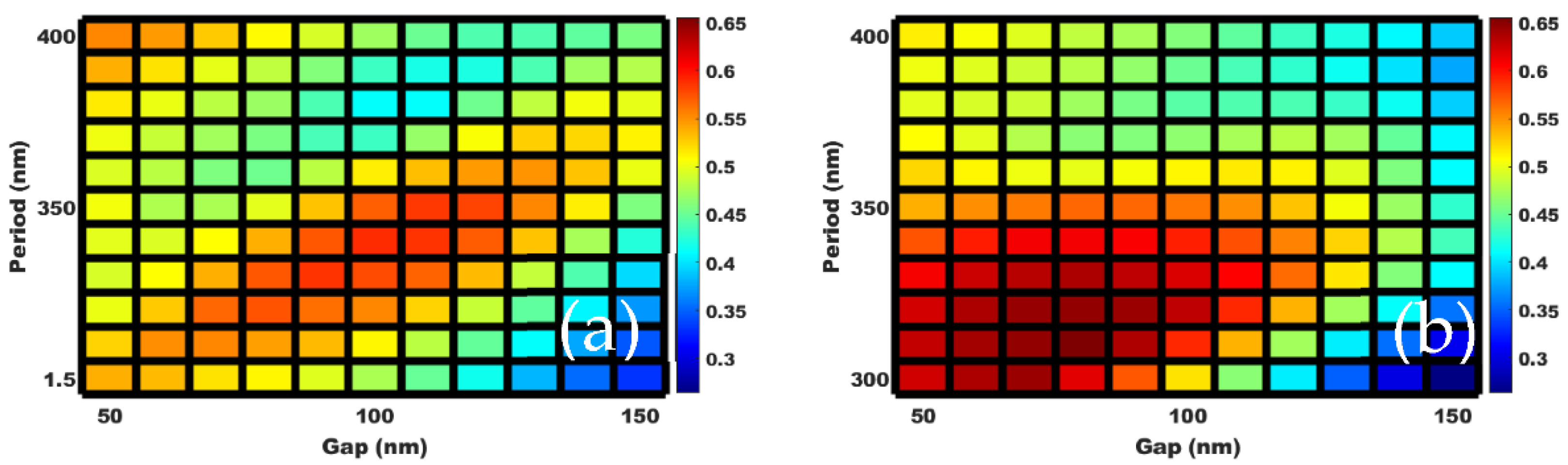
3.3. Assessment of TiO2 Resonator Array as Efficient Color and UV Absorber Coating
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- IRENA. Renewable Energy Statistics 2019; The International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2019; ISBN 978-92-9260-137-9. [Google Scholar]
- IRENA. Off-Grid Renewable Energy Solutions: Global and Regional Status and Trends; The International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2018; ISBN 978-92-9260-076-1. [Google Scholar]
- Ballif, C.; Perret-Aebi, L.E.; Lufkin, S.; Rey, E. Integrated Thinking for Photovoltaics in Buildings. Nat. Energy 2018, 3, 438–442. [Google Scholar] [CrossRef]
- Heinstein, P.; Ballif, C.; Perret-Aebi, L.E. Building Integrated Photovoltaics (BIPV): Review, Potentials, Barriers and Myths. Green 2013, 3, 125–156. [Google Scholar] [CrossRef]
- Traverse, C.J.; Pandey, R.; Barr, M.C.; Lunt, R.R. Emergence of Highly Transparent Photovoltaics for Distributed Applications. Nat. Energy 2017, 2, 849–860. [Google Scholar] [CrossRef]
- Yu, W.; Shen, L.; Jia, X.; Liu, Y.; Guo, W.; Ruan, S. Improved Color Rendering Index of Low Band Gap Semi-Transparent Polymer Solar Cells Using One-Dimensional Photonic Crystals. RSC Adv. 2015, 5, 54638–54644. [Google Scholar] [CrossRef]
- Park, H.J.; Xu, T.; Lee, J.Y.; Ledbetter, A.; Guo, L.J. Photonic Color Filters Integrated with Organic Solar Cells for Energy Harvesting. ACS Nano 2011, 5, 7055–7060. [Google Scholar] [CrossRef]
- Lee, K.T.; Jang, J.Y.; Zhang, J.; Yang, S.M.; Park, S.; Park, H.J. Highly Efficient Colored Perovskite Solar Cells Integrated with Ultrathin Subwavelength Plasmonic Nanoresonators. Sci. Rep. 2017, 7, 10640. [Google Scholar] [CrossRef] [Green Version]
- Kristensen, A.; Yang, J.K.W.; Bozhevolnyi, S.I.; Link, S.; Nordlander, P.; Halas, N.J.; Mortensen, N.A. Plasmonic Colour Generation. Nat. Rev. Mater. 2016, 2, 16088. [Google Scholar] [CrossRef]
- Moitra, P.; Slovick, B.A.; Li, W.; Kravchencko, I.I.; Briggs, D.P.; Krishnamurthy, S.; Valentine, J. Large-Scale All-Dielectric Metamaterial Perfect Reflectors. ACS Photonics 2015, 2, 692–698. [Google Scholar] [CrossRef]
- Cordaro, A.; Van De Groep, J.; Raza, S.; Pecora, E.F.; Priolo, F.; Brongersma, M.L. Antireflection High-Index Metasurfaces Combining Mie and Fabry-Pérot Resonances. ACS Photonics 2019, 6, 453–459. [Google Scholar] [CrossRef] [Green Version]
- Gargas, D.J.; Moore, M.C.; Ni, A.; Chang, S.W.; Zhang, Z.; Chuang, S.L.; Yang, P. Whispering Gallery Mode Lasing from Zinc Oxide Hexagonal Nanodisks. ACS Nano 2010, 4, 3270–3276. [Google Scholar] [CrossRef]
- Yang, B.; Liu, W.; Li, Z.; Cheng, H.; Choi, D.Y.; Chen, S.; Tian, J. Ultrahighly Saturated Structural Colors Enhanced by Multipolar-Modulated Metasurfaces. Nano Lett. 2019, 19, 4221–4228. [Google Scholar] [CrossRef] [PubMed]
- Dong, Z.; Ho, J.; Yu, Y.F.; Fu, Y.H.; Paniagua-Dominguez, R.; Wang, S.; Kuznetsov, A.I.; Yang, J.K.W. Printing beyond SRGB Color Gamut by Mimicking Silicon Nanostructures in Free-Space. Nano Lett. 2017, 17, 7620–7628. [Google Scholar] [CrossRef] [PubMed]
- Frolov, A.Y.; Verellen, N.; Li, J.; Zheng, X.; Paddubrouskaya, H.; Denkova, D.; Shcherbakov, M.R.; Vandenbosch, G.A.E.; Panov, V.I.; Van Dorpe, P.; et al. Near-Field Mapping of Optical Fabry-Perot Modes in All-Dielectric Nanoantennas. Nano Lett. 2017, 17, 7629–7637. [Google Scholar] [CrossRef]
- Sun, S.; Zhou, Z.; Duan, Z.; Xiao, S.; Song, Q. All-Dielectric Full-Color Printing with TiO2 Metasurfaces. ACS Nano 2017, 11, 4445–4452. [Google Scholar] [CrossRef]
- Spinelli, P.; Verschuuren, M.A.; Polman, A. Broadband Omnidirectional Antireflection Coating Based on Subwavelength Surface Mie Resonators. Nat. Commun. 2012, 3, 692. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Neder, V.; Luxembourg, S.L.; Polman, A. Efficient Colored Silicon Solar Modules Using Integrated Resonant Dielectric Nanoscatterers. Appl. Phys. Lett. 2017, 111, 073902. [Google Scholar] [CrossRef]
- Kempe, M. Encapsulant Materials for PV Modules. Photovolt. Sol. Energy 2017, 478–490. [Google Scholar] [CrossRef]
- Peike, C.; Peike, C.; Hädrich, I.; Weiß, K.; Dürr, I.; Ise, F. Overview of PV Module Encapsulation Materials. Photovolt. Int. 2013, 19, 85–92. [Google Scholar]
- Czanderna, A.W.; Pern, F.J. Encapsulation of PV Modules Using Ethylene Vinyl Acetate Copolymer as a Pottant: A Critical Review. Sol. Energy Mater. Sol. Cells 1996, 43, 101–181. [Google Scholar] [CrossRef]
- Kempe, M.D.; Jorgensen, G.J.; Terwilliger, K.M.; McMahon, T.J.; Kennedy, C.E.; Borek, T.T. Acetic Acid Production and Glass Transition Concerns with Ethylene-Vinyl Acetate Used in Photovoltaic Devices. Sol. Energy Mater. Sol. Cells 2007, 91, 315–329. [Google Scholar] [CrossRef]
- Johansson, W.; Peralta, A.; Jonson, B.; Anand, S.; Österlund, L.; Karlsson, S. Transparent TiO2 and ZnO Thin Films on Glass for UV Protection of PV Modules. Front. Mater. 2019, 6, 259. [Google Scholar] [CrossRef]
- Yang, B.; Liu, W.; Li, Z.; Cheng, H.; Chen, S.; Tian, J. Polarization-Sensitive Structural Colors with Hue-and-Saturation Tuning Based on All-Dielectric Nanopixels. Adv. Opt. Mater. 2018, 6, 1–8. [Google Scholar] [CrossRef]
- Shapturenka, P.; Birkholz, F.; Zakaria, N.I.; DenBaars, S.P.; Gordon, M.J. Quasiordered, Subwavelength TiO 2 Hole Arrays with Tunable, Omnidirectional Color Response. J. Vac. Sci. Technol. A 2020, 38, 053403. [Google Scholar] [CrossRef]
- Sanchez-Sobrado, O.; Mendes, M.J.; Haque, S.; Mateus, T.; Araujo, A.; Aguas, H.; Fortunato, E.; Martins, R. Colloidal-Lithographed TiO2 Photonic Nanostructures for Solar Cell Light Trapping. J. Mater. Chem. C 2017, 5, 6852–6861. [Google Scholar] [CrossRef]
- Visser, D.; Chen, D.Y.; Désières, Y.; Ravishankar, A.P.; Anand, S. Embossed Mie Resonator Arrays Composed of Compacted TiO2 Nanoparticles for Broadband Anti-Reflection in Solar Cells. Sci. Rep. 2020, 10, 12527. [Google Scholar] [CrossRef]
- Lumerical Solutions FDTD. Available online: https://www.lumerical.com/products/fdtd/ (accessed on 6 April 2022).
- Sarkar, S.; Gupta, V.; Kumar, M.; Schubert, J.; Probst, P.T.; Joseph, J.; König, T.A.F. Hybridized Guided-Mode Resonances via Colloidal Plasmonic Self-Assembled Grating. ACS Appl. Mater. Interfaces 2019, 11, 13752–13760. [Google Scholar] [CrossRef] [Green Version]
- Alaee, R.; Rockstuhl, C.; Fernandez-Corbaton, I. An Electromagnetic Multipole Expansion beyond the Long-Wavelength Approximation. Opt. Commun. 2018, 407, 17–21. [Google Scholar] [CrossRef] [Green Version]
- Alaee, R.; Filter, R.; Lehr, D.; Lederer, F.; Rockstuhl, C. A Generalized Kerker Condition for Highly Directive Nanoantennas. Opt. Lett. 2015, 40, 2645. [Google Scholar] [CrossRef]
- Staude, I.; Miroshnichenko, A.E.; Decker, M.; Fofang, N.T.; Liu, S.; Gonzales, E.; Dominguez, J.; Luk, T.S.; Neshev, D.N.; Brener, I.; et al. Tailoring Directional Scattering through Magnetic and Electric Resonances in Subwavelength Silicon Nanodisks. ACS Nano 2013, 7, 7824–7832. [Google Scholar] [CrossRef]
- Butakov, N.A.; Schuller, J.A. Designing Multipolar Resonances in Dielectric Metamaterials. Sci. Rep. 2016, 6, 38487. [Google Scholar] [CrossRef] [Green Version]
- van de Groep, J.; Polman, A. Designing Dielectric Resonators on Substrates: Combining Magnetic and Electric Resonances. Opt. Express 2013, 21, 26285. [Google Scholar] [CrossRef] [PubMed]
- Rybin, M.V.; Filonov, D.S.; Samusev, K.B.; Belov, P.A.; Kivshar, Y.S.; Limonov, M.F. Phase Diagram for the Transition from Photonic Crystals to Dielectric Metamaterials. Nat. Commun. 2015, 6, 10102. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Rinnerbauer, V.; Wang, I.; Stelmakh, V.; Joannopoulos, J.D.; Soljačić, M. Structural Colors from Fano Resonances. ACS Photonics 2015, 2, 27–32. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amores, A.P.; Ravishankar, A.P.; Anand, S. Design and Modelling of Metal-Oxide Nanodisk Arrays for Structural Colors and UV-Blocking Functions in Solar Cell Glass Covers. Photonics 2022, 9, 273. https://doi.org/10.3390/photonics9050273
Amores AP, Ravishankar AP, Anand S. Design and Modelling of Metal-Oxide Nanodisk Arrays for Structural Colors and UV-Blocking Functions in Solar Cell Glass Covers. Photonics. 2022; 9(5):273. https://doi.org/10.3390/photonics9050273
Chicago/Turabian StyleAmores, Albert Peralta, Ajith Padyana Ravishankar, and Srinivasan Anand. 2022. "Design and Modelling of Metal-Oxide Nanodisk Arrays for Structural Colors and UV-Blocking Functions in Solar Cell Glass Covers" Photonics 9, no. 5: 273. https://doi.org/10.3390/photonics9050273
APA StyleAmores, A. P., Ravishankar, A. P., & Anand, S. (2022). Design and Modelling of Metal-Oxide Nanodisk Arrays for Structural Colors and UV-Blocking Functions in Solar Cell Glass Covers. Photonics, 9(5), 273. https://doi.org/10.3390/photonics9050273




