Numerical Study on a Bound State in the Continuum Assisted Plasmonic Refractive Index Sensor
Abstract
:1. Introduction
2. Design of a BIC-Assisted Plasmonic Sensor
3. Results
3.1. Sensitivity Characterization and Refractive Index Sensing
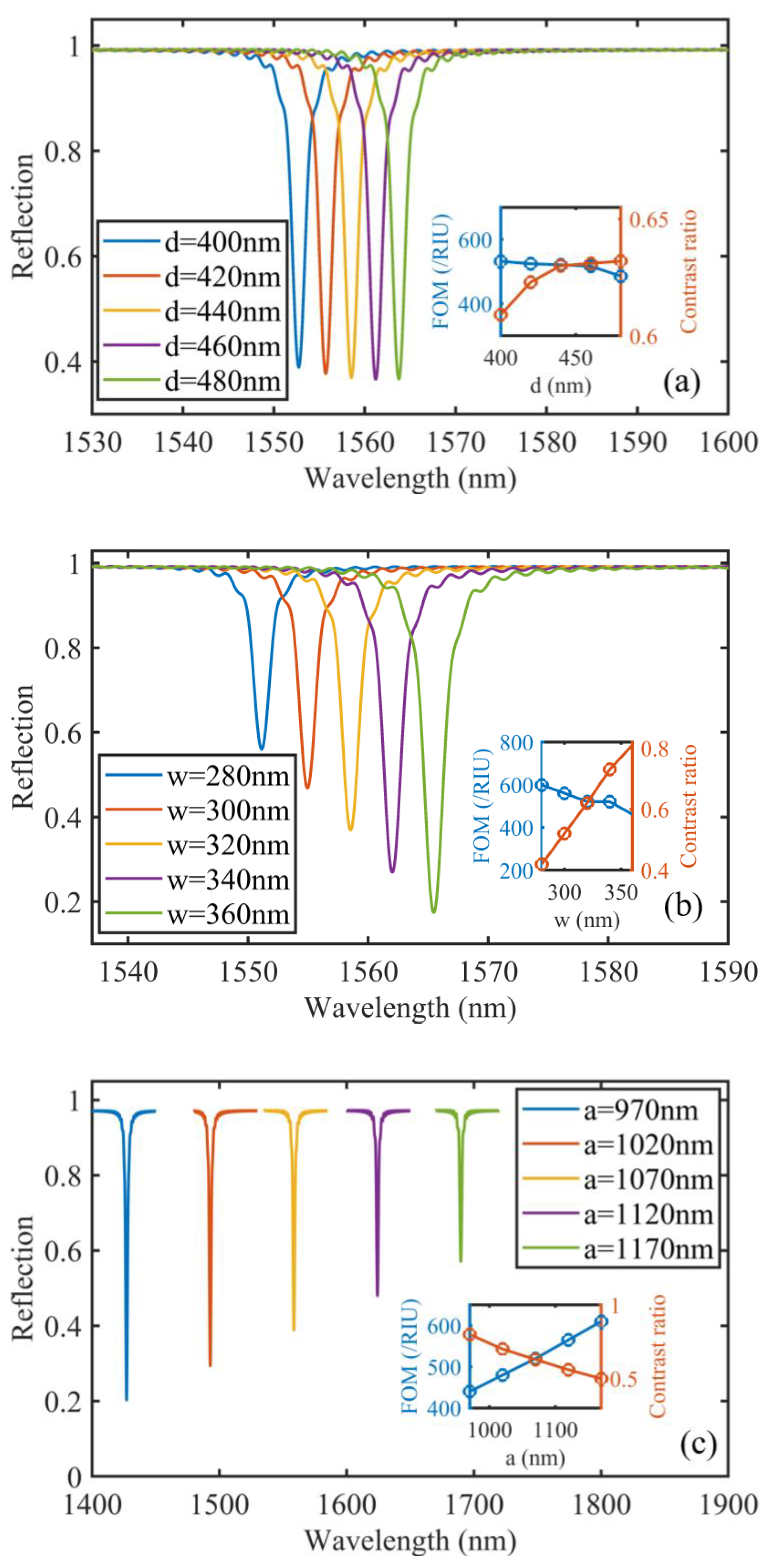
3.2. Performance Change with the Thickness of the Silver Layer
3.3. Performance Change with the Grating Variables
3.4. Performance Change with the Grating Shape Deviation
4. Discussion
| Ref. | Sensitivity (nm/RIU) | FWHM (nm) | FOM (/RIU) |
|---|---|---|---|
| Sharma et al. [31] | 461.53 | 14.8 | 31.18 |
| Lu et al. [32] | 497.83 | 0.904 | 551 |
| Chau et al. [33] | 1200 | 45 | 26.67 |
| Sun et al. [34] | 526.0 | 7.2 | 73.10 |
| Li et al. [35] | 404.295 | 8.04 | 50.30 |
| Sreekanth et al. [36] He et al. [37] | 30,000 815 | 50.8 360.6 | 590 2.26 |
| This paper @1559 nm | 938 | 1.8 | 521 |
| This paper @1905 nm | 1264 | 0.18 | 7022 |
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Reather, H. Surface Plasmons on Smooth and Rough Surfaces and on Gratings. Springer Tracts Mod. Phys. 1988, 111, 345–398. [Google Scholar]
- Pandey, A.K.; Sharma, A.K.; Basu, R. Fluoride glass-based surface plasmon resonance sensor in infrared region: Performance evaluation. J. Phys. D Appl. Phys. 2017, 50, 185103. [Google Scholar] [CrossRef]
- Sharma, S.; Kumari, R.; Varshney, S.K.; Lahiri, B. Optical biosensing with electromagnetic nanostructures. Rev. Phys. 2020, 5, 100044. [Google Scholar] [CrossRef]
- Suido, Y.; Yamamoto, Y.; Thomas, G.; Ajiki, Y.; Kan, T. Extension of the measurable wavelength range for a near-infrared spectrometer using a plasmonic Au grating on a si substrate. Micromachines 2019, 10, 403. [Google Scholar] [CrossRef] [Green Version]
- Chen, W.; Kan, T.; Ajiki, Y.; Matsumoto, K.; Shimoyama, I. NIR spectrometer using a Schottky photodetector enhanced by grating-based SPR. Opt. Express 2016, 24, 25797–25804. [Google Scholar] [CrossRef]
- Dhawan, A.; Gerhold, M.D.; Muth, J.F. Plasmonic structures based on subwavelength apertures for chemical and biological sensing applications. IEEE Sens. J. 2008, 8, 942–950. [Google Scholar] [CrossRef]
- Fore, S.; Yuen, Y.; Hesselink, L.; Huser, T. Pulsed-interleaved excitation FRET measurements on single duplex DNA molecules inside C-shaped nanoapertures. Nano Lett. 2007, 7, 1749–1756. [Google Scholar] [CrossRef]
- Zaman, M.A.; Padhy, P.; Hesselink, L. Solenoidal optical forces from a plasmonic Archimedean spiral. Phys. Rev. A 2019, 100, 013857. [Google Scholar] [CrossRef]
- Palermo, G.; Sreekanth, K.V.; Maccaferri, N.; Lio, G.E.; Nicoletta, G.; De Angelis, F.; Hinczewski, M.; Strangi, G. Hyperbolic dispersion metasurfaces for molecular biosensing. Nanophotonics 2021, 10, 295–314. [Google Scholar] [CrossRef]
- Ferraro, A.; Lio, G.E.; Hmina, A.; Palermo, G.; Djouda, J.M.; Maurer, T.; Caputo, R. Tailoring of plasmonic functionalized metastructures to enhance local heating release. Nanophotonics 2021, 10, 3907–3916. [Google Scholar] [CrossRef]
- Von Neumann, J.; Wigner, E. On some peculiar discrete eigenvalues. Phys. Z. 1929, 30, 465–467. [Google Scholar]
- Plotnik, Y.; Peleg, O.; Dreisow, F.; Heinrich, M.; Nolte, S.; Szameit, A.; Segev, M. Experimental observation of optical bound states in the continuum. Phys. Rev. Lett. 2011, 107, 183901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fan, K.; Shadrivov, I.V.; Padilla, W.J. Dynamic bound states in the continuum. Optica 2019, 6, 169–173. [Google Scholar] [CrossRef]
- Hsu, C.W.; Zhen, B.; Stone, A.D.; Joannopoulos, J.D.; Soljačić, M. Bound states in the continuum. Nat. Rev. Mater. 2016, 1, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Molina, M.I.; Miroshnichenko, A.E.; Kivshar, Y.S. Surface bound states in the continuum. Phys. Rev. Lett. 2012, 108, 070401. [Google Scholar] [CrossRef] [Green Version]
- Song, Y.; Jiang, N.; Liu, L.; Hu, X.; Zi, J. Cherenkov radiation from photonic bound states in the continuum: Towards compact free-electron lasers. Phys. Rev. Appl. 2018, 10, 064026. [Google Scholar] [CrossRef]
- Kodigala, A.; Lepetit, T.; Gu, Q.; Bahari, B.; Fainman, Y.; Kanté, B. Lasing action from photonic bound states in continuum. Nature 2017, 541, 196–199. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, T.; Tian, J.; Sun, J.; Li, S.; De Leon, I.; Zaccaria, R.P.; Peng, L.; Gao, F.; Lin, X. Quasi-BIC laser enabled by high-contrast grating resonator for gas detection. Nanophotonics 2021, 11, 297–304. [Google Scholar] [CrossRef]
- Hwang, M.-S.; Lee, H.-C.; Kim, K.-H.; Jeong, K.-Y.; Kwon, S.-H.; Koshelev, K.; Kivshar, Y.; Park, H.-G. Ultralow-threshold laser using super-bound states in the continuum. Nat. Commun. 2021, 12, 4135. [Google Scholar] [CrossRef]
- Romano, S.; Lamberti, A.; Masullo, M.; Penzo, E.; Cabrini, S.; Rendina, I.; Mocella, V. Optical biosensors based on photonic crystals supporting bound states in the continuum. Materials 2018, 11, 526. [Google Scholar] [CrossRef] [Green Version]
- Romano, S.; Zito, G.; Torino, S.; Calafiore, G.; Penzo, E.; Coppola, G.; Cabrini, S.; Rendina, I.; Mocella, V. Label-free sensing of ultralow-weight molecules with all-dielectric metasurfaces supporting bound states in the continuum. Photonics Res. 2018, 6, 726–733. [Google Scholar] [CrossRef]
- Li, Z.; Xiang, Y.; Xu, S.; Dai, X. Ultrasensitive terahertz sensing in all-dielectric asymmetric metasurfaces based on quasi-BIC. JOSA B 2022, 39, 286–291. [Google Scholar] [CrossRef]
- Azzam, S.I.; Shalaev, V.M.; Boltasseva, A.; Kildishev, A.V. Formation of bound states in the continuum in hybrid plasmonic-photonic systems. Phys. Rev. Lett. 2018, 121, 253901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cao, J.; Sun, Y.; Kong, Y.; Qian, W. The sensitivity of grating-based SPR sensors with wavelength interrogation. Sensors 2019, 19, 405. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.M.; Boltasseva, A. Searching for better plasmonic materials. Laser Photonics Rev. 2010, 4, 795–808. [Google Scholar] [CrossRef] [Green Version]
- Johnson, P.B.; Christy, R.-W. Optical constants of the noble metals. Phys. Rev. B 1972, 6, 4370. [Google Scholar] [CrossRef]
- Pastrňák, J.; Roskovcová, L. Refraction index measurements on AlN single crystals. Phys. Status Solidi B 1966, 14, K5–K8. [Google Scholar] [CrossRef]
- Yeh, Y.-L. Real-time measurement of glucose concentration and average refractive index using a laser interferometer. Opt. Lasers Eng. 2008, 46, 666–670. [Google Scholar] [CrossRef]
- Iqbal, T.; Khalil, S.; Ijaz, M.; Riaz, K.N.; Khan, M.I.; Shakil, M.; Nabi, A.G.; Javaid, M.; Abrar, M.; Afsheen, S. Optimization of 1D plasmonic grating of nanostructured devices for the investigation of plasmonic bandgap. Plasmonics 2019, 14, 775–783. [Google Scholar] [CrossRef]
- Blaber, M.G.; Arnold, M.D.; Ford, M.J. A review of the optical properties of alloys and intermetallics for plasmonics. J. Phys. Condens. Matter 2010, 22, 143201. [Google Scholar] [CrossRef]
- Sharma, A.K.; Pandey, A.K. Metal oxide grating based plasmonic refractive index sensor with Si layer in optical communication band. IEEE Sens. J. 2019, 20, 1275–1282. [Google Scholar] [CrossRef]
- Lu, X.; Zheng, G.; Zhou, P. High performance refractive index sensor with stacked two-layer resonant waveguide gratings. Results Phys. 2019, 12, 759–765. [Google Scholar] [CrossRef]
- Chou Chau, Y.-F.; Chou Chao, C.-T.; Huang, H.J.; Kooh, M.R.R.; Kumara, N.; Lim, C.M.; Chiang, H.-P. Perfect dual-band absorber based on plasmonic effect with the cross-hair/nanorod combination. Nanomaterials 2020, 10, 493. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sun, P.; Zhou, C.; Jia, W.; Wang, J.; Xiang, C.; Xie, Y.; Zhao, D. Narrowband absorber based on magnetic dipole resonances in two-dimensional metal–dielectric grating for sensing. Opt. Commun. 2020, 459, 124946. [Google Scholar] [CrossRef]
- Li, Y.; Liu, Y.; Liu, Z.; Tang, Q.; Shi, L.; Chen, Q.; Du, G.; Wu, B.; Liu, G.; Li, L. Grating-assisted ultra-narrow multispectral plasmonic resonances for sensing application. Appl. Phys. Express 2019, 12, 072002. [Google Scholar] [CrossRef]
- Sreekanth, K.V.; Alapan, Y.; ElKabbash, M.; Ilker, E.; Hinczewski, M.; Gurkan, U.A.; De Luca, A.; Strangi, G. Extreme sensitivity biosensing platform based on hyperbolic metamaterials. Nat. Mater. 2016, 15, 621–627. [Google Scholar] [CrossRef] [Green Version]
- He, W.; Feng, Y.; Hu, Z.-D.; Balmakou, A.; Khakhomov, S.; Deng, Q.; Wang, J. Sensors with multifold nanorod metasurfaces array based on hyperbolic metamaterials. IEEE Sens. J. 2019, 20, 1801–1806. [Google Scholar] [CrossRef]






| w (nm) | d (nm) | a (nm) | h (nm) | Incident Angle (deg) |
|---|---|---|---|---|
| 320 | 440 | 1070 | 100 | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, S.; Chang, C.; Zhou, P.; Zou, Y. Numerical Study on a Bound State in the Continuum Assisted Plasmonic Refractive Index Sensor. Photonics 2022, 9, 224. https://doi.org/10.3390/photonics9040224
Tang S, Chang C, Zhou P, Zou Y. Numerical Study on a Bound State in the Continuum Assisted Plasmonic Refractive Index Sensor. Photonics. 2022; 9(4):224. https://doi.org/10.3390/photonics9040224
Chicago/Turabian StyleTang, Shulin, Chang Chang, Peiji Zhou, and Yi Zou. 2022. "Numerical Study on a Bound State in the Continuum Assisted Plasmonic Refractive Index Sensor" Photonics 9, no. 4: 224. https://doi.org/10.3390/photonics9040224
APA StyleTang, S., Chang, C., Zhou, P., & Zou, Y. (2022). Numerical Study on a Bound State in the Continuum Assisted Plasmonic Refractive Index Sensor. Photonics, 9(4), 224. https://doi.org/10.3390/photonics9040224





