Implementation of Logic Gates in an Erbium-Doped Fiber Laser (EDFL): Numerical and Experimental Analysis
Abstract
1. Introduction
2. Materials and Methods
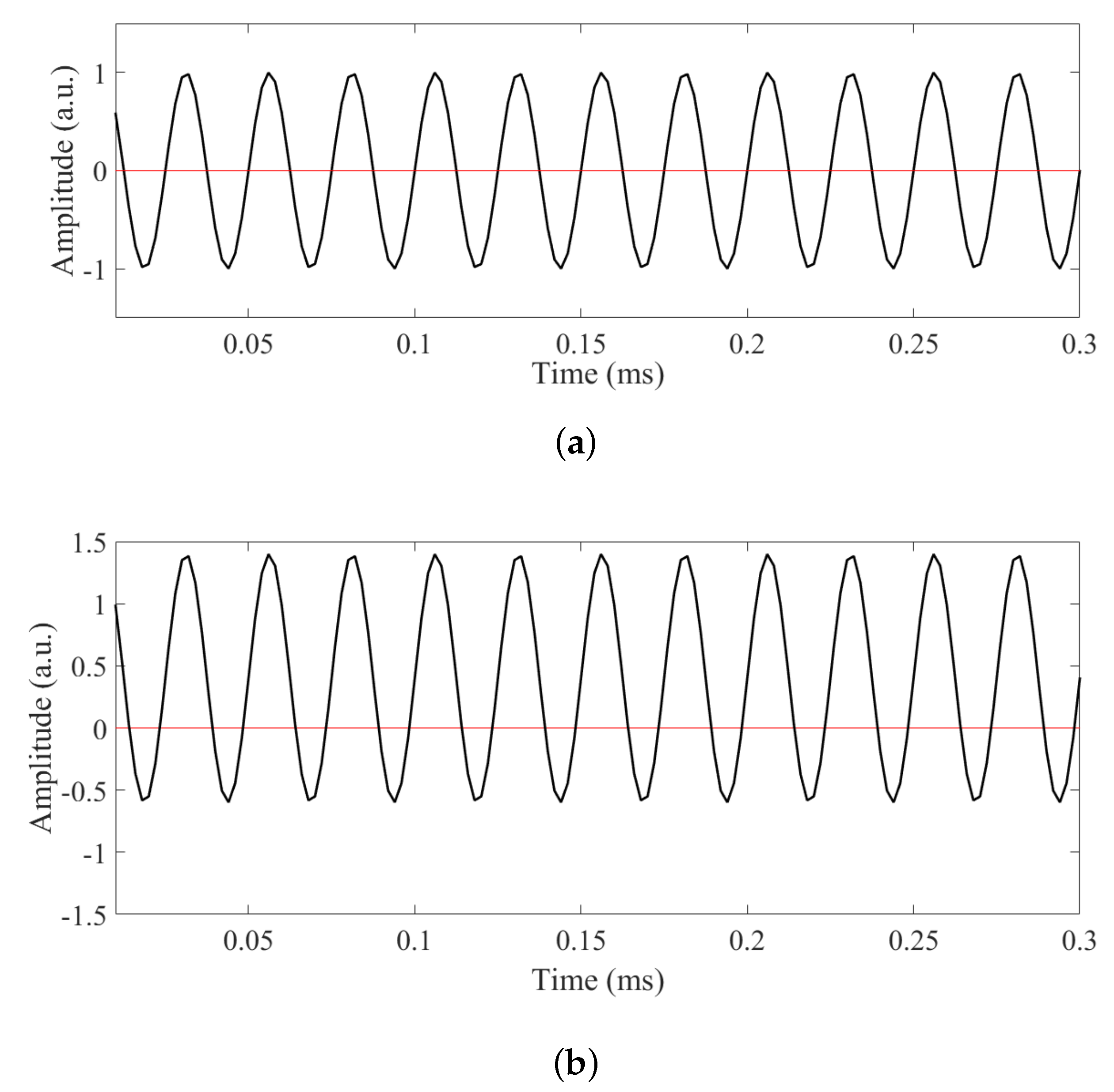
2.1. Numerical Model
2.2. Experimental Set-Up
2.3. Methods
3. Results
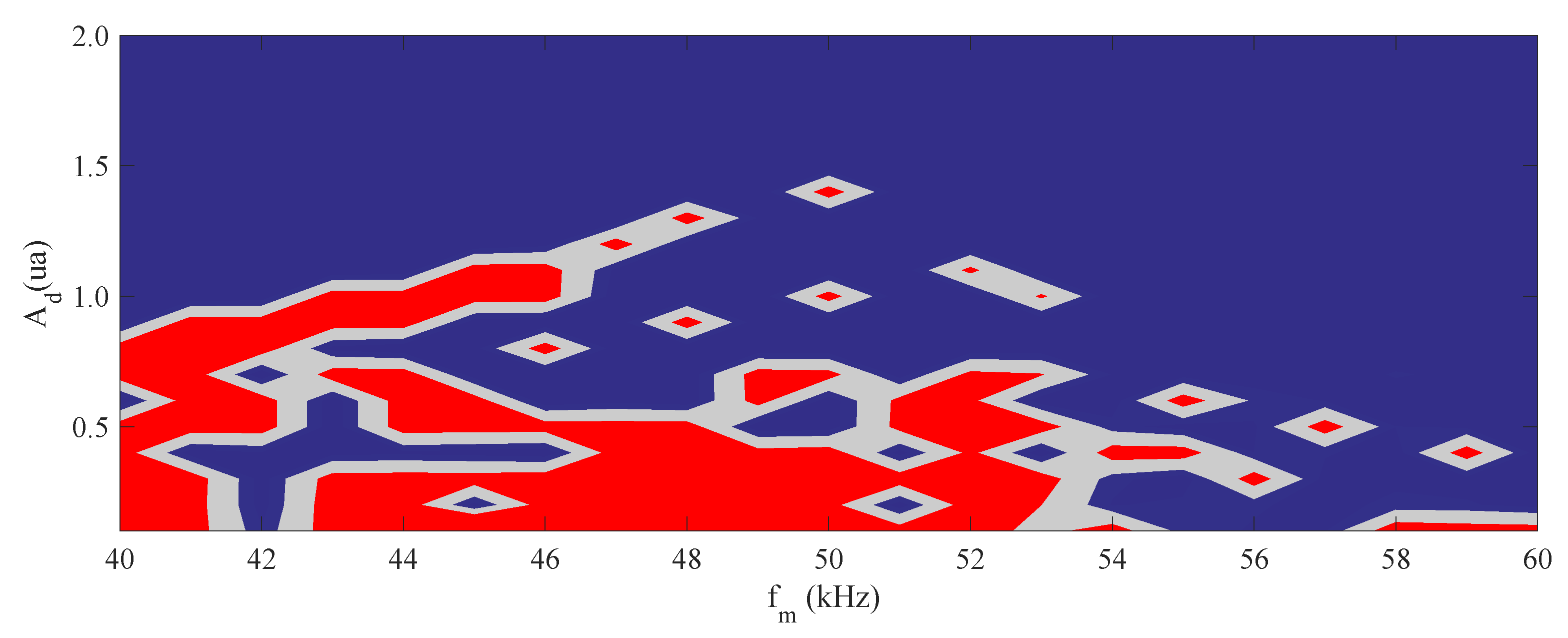
3.1. Numerical Results
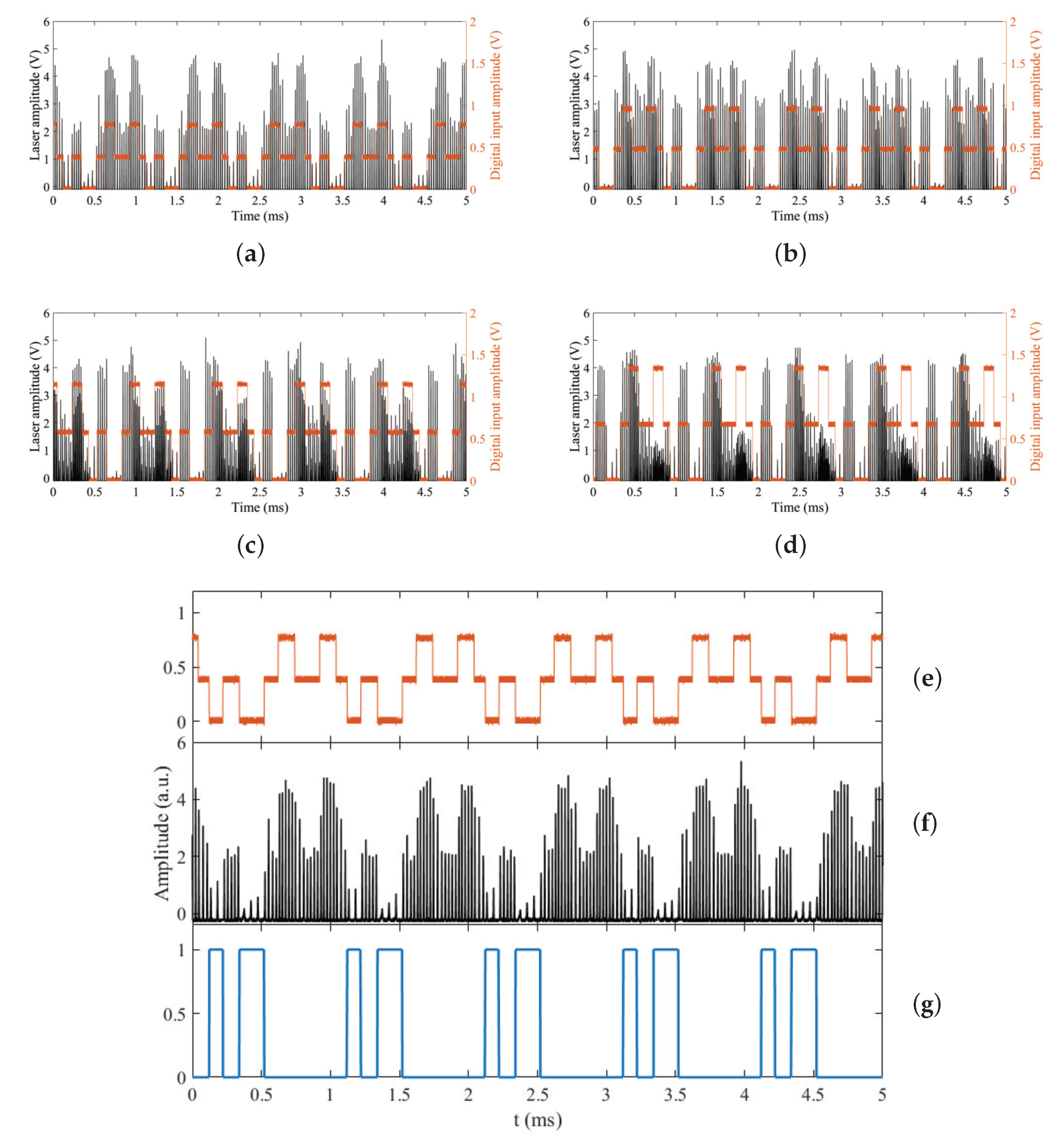
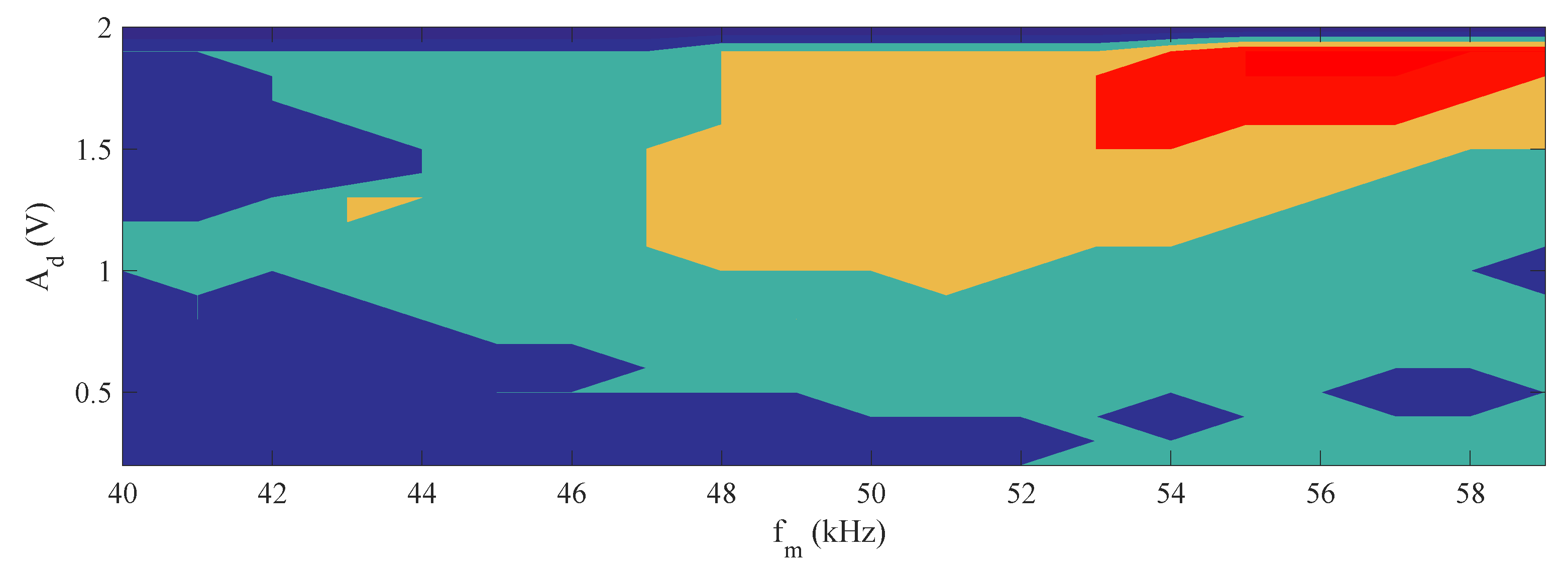
3.2. Experimental Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Digonnet, M.J.; Gaeta, C. Theoretical analysis of optical fiber laser amplifiers and oscillators. Appl. Opt. 1985, 24, 333–342. [Google Scholar] [CrossRef] [PubMed]
- Digonnet, M. Theory of operation of three-and four-level fiber amplifiers and sources. In Proceedings of the Fiber Laser Sources and Amplifiers; SPIE: Cardiff, Wales, 1990; Volume 1171, pp. 8–26. [Google Scholar]
- Sola, I.; Martín, J.; Ávarez, J.; Jarabo, S. Erbium doped fibre characterisation by laser transient behaviour analysis. Opt. Commun. 2001, 193, 133–140. [Google Scholar] [CrossRef]
- Tang, C.L.; Statz, H.; deMars, G. Spectral output and spiking behavior of solid-state lasers. J. Appl. Phys. 1963, 34, 2289–2295. [Google Scholar] [CrossRef]
- Magallón, D.A.; Jaimes-Reátegui, R.; García-López, J.H.; Huerta-Cuellar, G.; López-Mancilla, D.; Pisarchik, A.N. Control of Multistability in an Erbium-Doped Fiber Laser by an Artificial Neural Network: A Numerical Approach. Mathematics 2022, 10, 3140. [Google Scholar] [CrossRef]
- Arecchi, F.T.; Harrison, R.G. Instabilities and Chaos in Quantum Optics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 34. [Google Scholar]
- Lacot, E.; Stoeckel, F.; Chenevier, M. Dynamics of an erbium-doped fiber laser. Phys. Rev. A 1994, 49, 3997. [Google Scholar] [CrossRef]
- Saucedo-Solorio, J.M.; Pisarchik, A.N.; Kir’yanov, A.V.; Aboites, V. Generalized multistability in a fiber laser with modulated losses. JOSA B 2003, 20, 490–496. [Google Scholar] [CrossRef]
- Pisarchik, A.N.; Barmenkov, Y.O.; Kir’yanov, A.V. Experimental characterization of the bifurcation structure in an erbium-doped fiber laser with pump modulation. IEEE J. Quantum Electron. 2003, 39, 1567–1571. [Google Scholar] [CrossRef]
- Meucci, R.; Marc Ginoux, J.; Mehrabbeik, M.; Jafari, S.; Clinton Sprott, J. Generalized multistability and its control in a laser. Chaos Interdiscip. J. Nonlinear Sci. 2022, 32, 083111. [Google Scholar] [CrossRef]
- Sinha, S.; Ditto, W.L. Dynamics based computation. Phys. Rev. Lett. 1998, 81, 2156. [Google Scholar] [CrossRef]
- Cafagna, D.; Grassi, G. Chaos-based SR flip–flop via chua’s circuit. Int. J. Bifurc. Chaos 2006, 16, 1521–1526. [Google Scholar] [CrossRef]
- Munakata, T.; Sinha, S.; Ditto, W.L. Chaos computing: Implementation of fundamental logical gates by chaotic elements. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2002, 49, 1629–1633. [Google Scholar] [CrossRef]
- Murali, K.; Sinha, S.; Mohamed, I.R. Chaos computing: Experimental realization of NOR gate using a simple chaotic circuit. Phys. Lett. A 2005, 339, 39–44. [Google Scholar] [CrossRef]
- Murali, K.; Sinha, S.; Ditto, W.L.; Bulsara, A.R. Reliable logic circuit elements that exploit nonlinearity in the presence of a noise floor. Phys. Rev. Lett. 2009, 102, 104101. [Google Scholar] [CrossRef]
- Campos-Cantón, I.; Pecina-Sánchez, J.; Campos-Cantón, E.; Rosu, H.C. A simple circuit with dynamic logic architecture of basic logic gates. Int. J. Bifurc. Chaos 2010, 20, 2547–2551. [Google Scholar] [CrossRef]
- Pando L, C.; Meucci, R.; Ciofini, M.; Arecchi, F. CO2 laser with modulated losses: Theoretical models and experiments in the chaotic regime. Chaos Interdiscip. J. Nonlinear Sci. 1993, 3, 279–285. [Google Scholar] [CrossRef]
- Pando, C.; Acosta, G.L.; Meucci, R.; Ciofini, M. Highly dissipative Hénon map behavior in the four-level model of the CO2 laser with modulated losses. Phys. Lett. A 1995, 199, 191–198. [Google Scholar] [CrossRef]
- Singh, K.P.; Sinha, S. Enhancement of “logical” responses by noise in a bistable optical system. Phys. Rev. E 2011, 83, 046219. [Google Scholar] [CrossRef]
- Murali, K.; Sinha, S.; Kohar, V.; Kia, B.; Ditto, W.L. Chaotic attractor hopping yields logic operations. PLoS ONE 2018, 13, e0209037. [Google Scholar] [CrossRef]
- Murali, K.; Sinha, S.; Kohar, V.; Ditto, W.L. Harnessing tipping points for logic operations. Eur. Phys. J. Spec. Top. 2021, 230, 3403–3409. [Google Scholar] [CrossRef]
- Jaimes-Reátegui, R.; Afanador-Delgado, S.; Sevilla-Escoboza, R.; Huerta-Cuellar, G.; García-López, J.H.; López-Mancilla, D.; Castañeda-Hernández; Pisarchik, A.N. Optoelectronic flexible logic gate based on a fiber laser. Eur. Phys. J. Spec. Top. 2014, 223, 2837–2846. [Google Scholar] [CrossRef]
- García-López, J.H.; Jaimes-Reátegui, R.; Afanador-Delgado, S.M.; Sevilla-Escoboza, R.; Huerta-Cuéllar, G.; López-Mancilla, D.; Chiu-Zarate, R.; Castañeda-Hernández, C.E.; Pisarchik, A.N. Experimental and Numerical Study of an Optoelectronics Flexible Logic Gate Using a Chaotic Doped Fiber Laser. In Recent Development in Optoelectronic Devices; IntechOpen: London, UK, 2018; pp. 97–114. [Google Scholar]
- Reategui, R.; Kir’yanov, A.; Pisarchik, A.; Barmenkov, Y.O.; Il’ichev, N. Experimental study and modeling of coexisting attractors and bifurcations in an erbium-doped fiber laser with diode-pump modulation. Laser Phys. 2004, 14, 1277–1281. [Google Scholar]
- Pisarchik, A.N.; Kir’yanov, A.V.; Barmenkov, Y.O.; Jaimes-Reátegui, R. Dynamics of an erbium-doped fiber laser with pump modulation: Theory and experiment. JOSA B 2005, 22, 2107–2114. [Google Scholar] [CrossRef]
- Pisarchik, A.N.; Jaimes-Reátegui, R.; Sevilla-Escoboza, R.; Huerta-Cuellar, G.; Taki, M. Rogue waves in a multistable system. Phys. Rev. Lett. 2011, 107, 274101. [Google Scholar] [CrossRef] [PubMed]
- Barba-Franco, J.; Romo-Muñoz, L.; Jaimes-Reátegui, R.; García-López, J.; Cuellar, G.H.; Pisarchik, A. Electronic equivalent of a pump-modulated erbium-doped fiber laser. Integration, 2022; in press. [Google Scholar] [CrossRef]
- Reategui, R.J. Dynamic of Complex Systems with Parametric Modulation: Duffing Oscillators and a Fiber Laser. Ph.D. Thesis, Centro de Investigaciones en Optica, Leon, Mexico, 2004. [Google Scholar]
- Doedel, E.J.; Carlos, L.; Pando, L. Isolas of periodic passive Q-switching self-pulsations in the three-level: Two-level model for a laser with a saturable absorber. Phys. Rev. E 2011, 84, 056207. [Google Scholar] [CrossRef]
- Doedel, E.J.; Carlos, L.; Pando, L. Multiparameter bifurcations and mixed-mode oscillations in Q-switched CO2 lasers. Phys. Rev. E 2014, 89, 052904. [Google Scholar] [CrossRef]
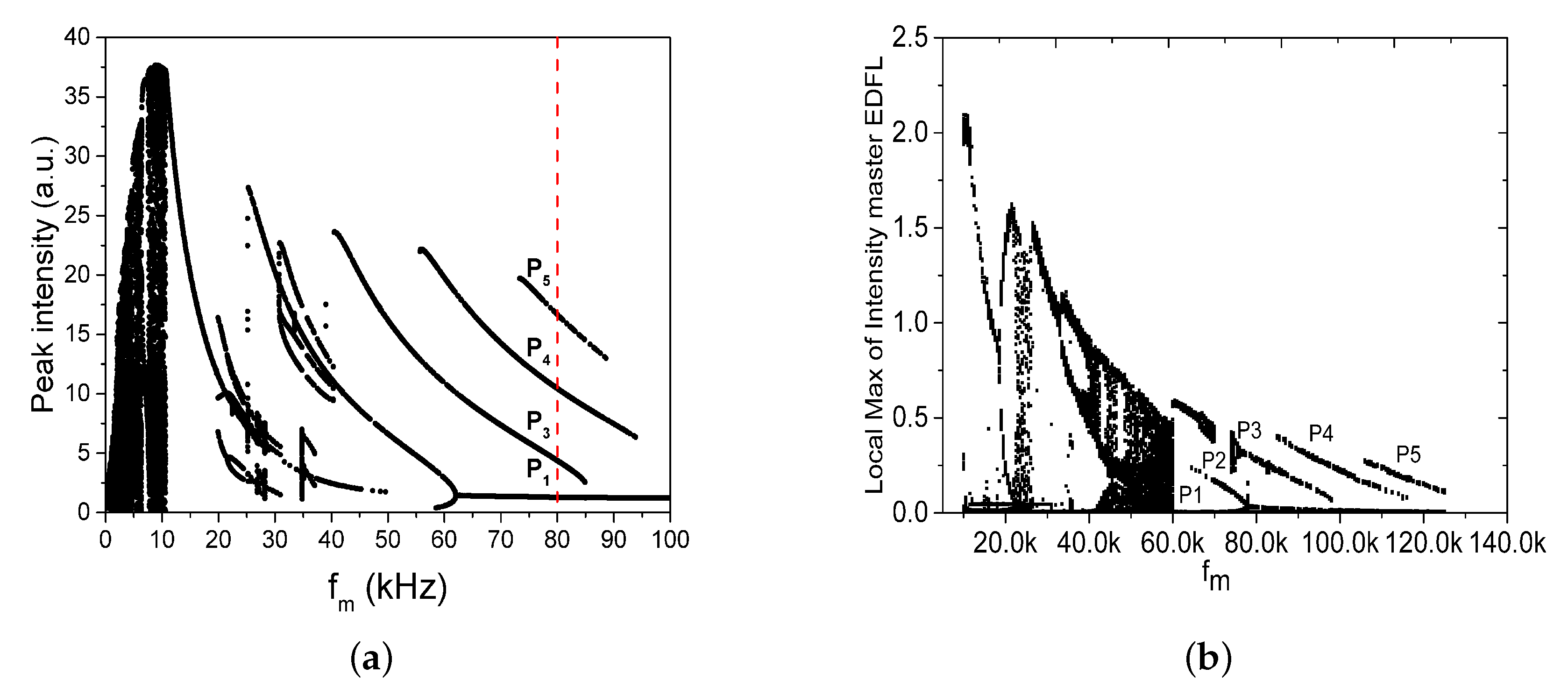
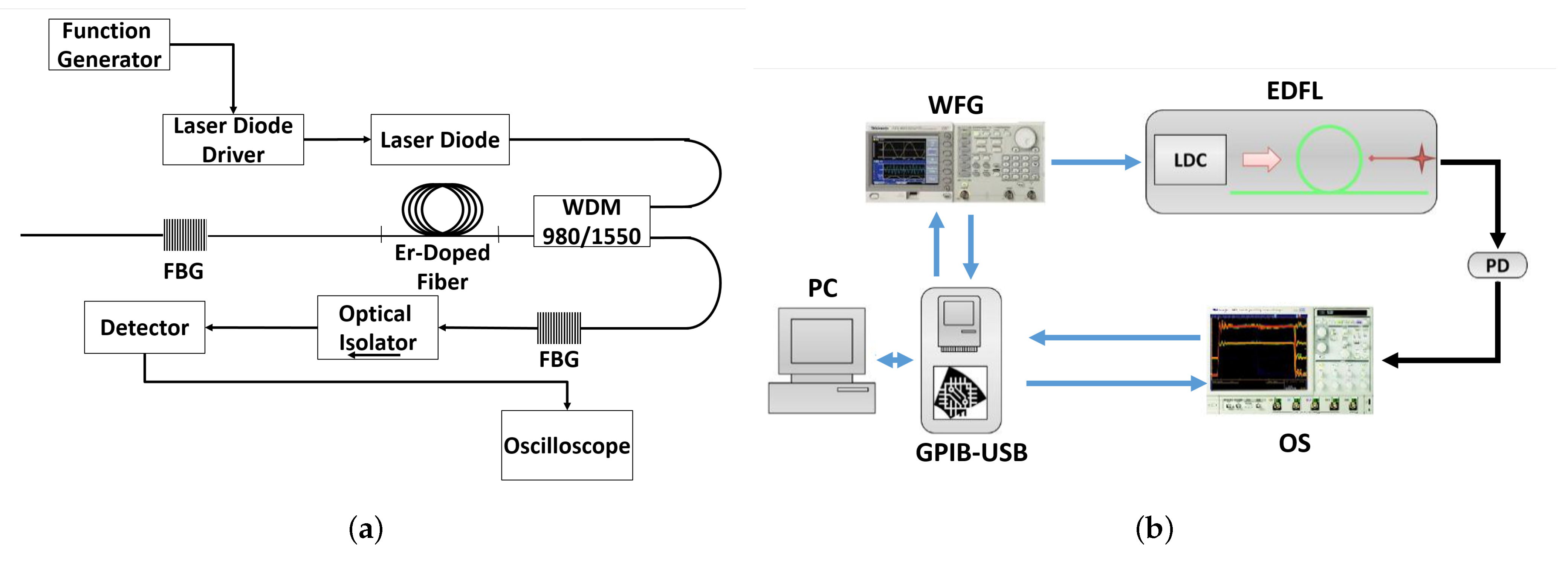

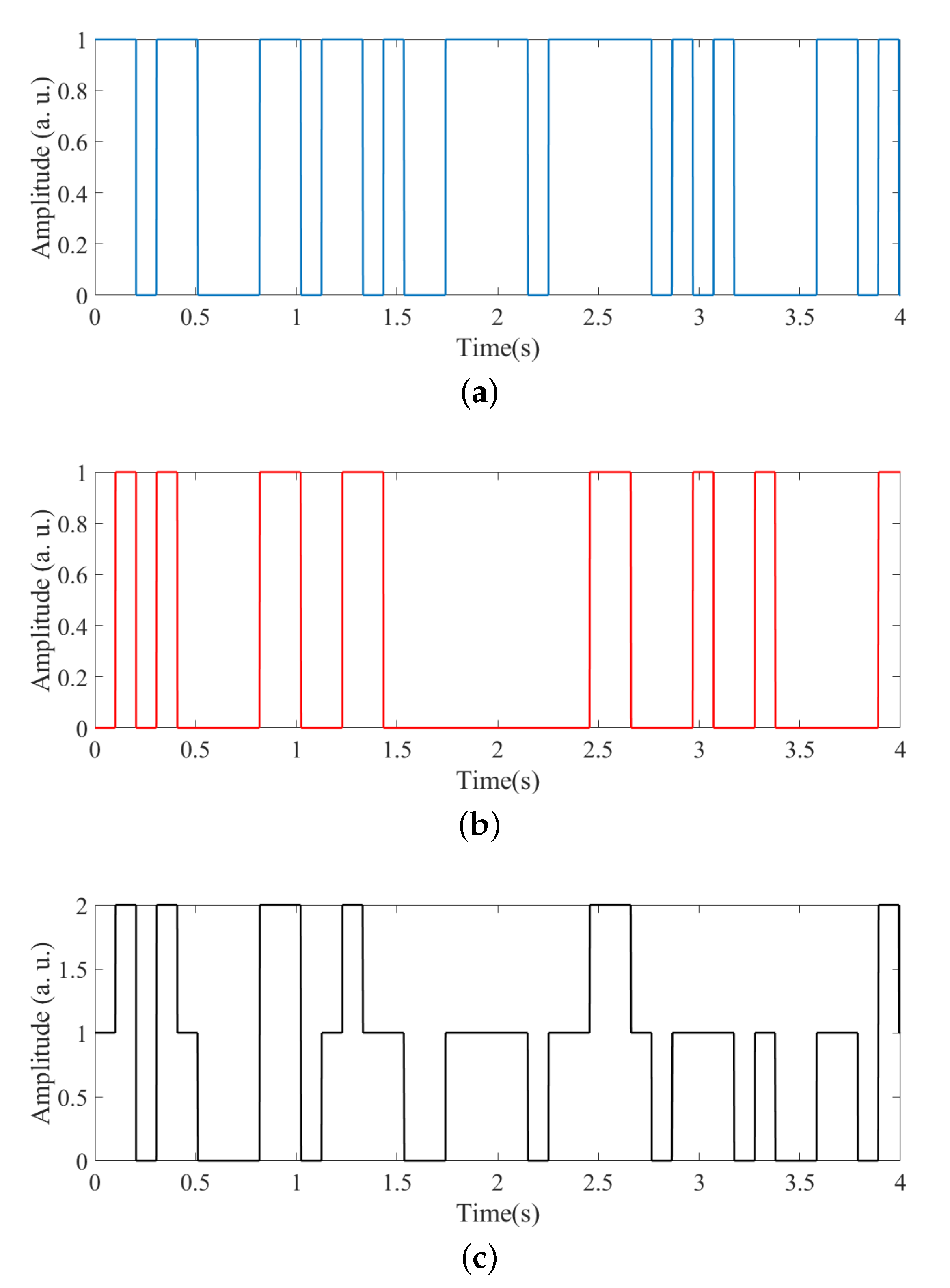
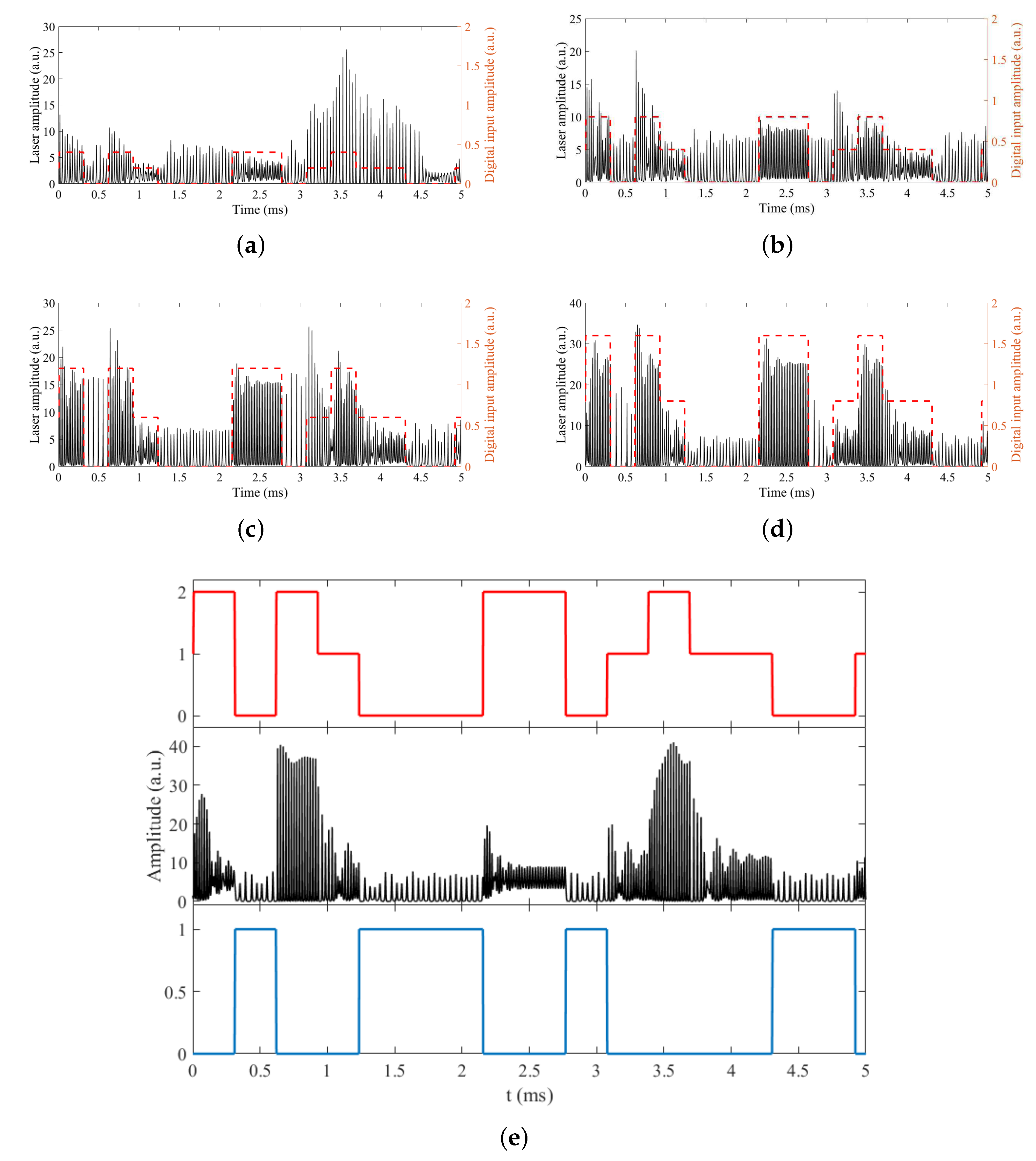
| a | 6.6207 × 10 |
| b | 7.4151 × 10 |
| c | |
| d | 4.0763 × 10 |
| e | 506 |
| Numeric NAND Logic Gate | |||
|---|---|---|---|
| 0 | |||
| Numeric Logic Gates for Different Input Values | |||
|---|---|---|---|
| V, kHz NAND | fm = 42 kHz XNOR | fm = 46 kHz NOR | |
| 1 | 1 | 1 | |
| 1 | 0 | 0 | |
| 0 | 1 | 0 | |
| Experimental Logic Gates for Different Input Values | ||
|---|---|---|
fm = 44 kHz XNOR | fm = 46 kHz NAND | |
| 1 | 1 | |
| 1 | 0 | |
| 0 | 1 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Afanador Delgado, S.M.; Echenausía Monroy, J.L.; Huerta Cuellar, G.; García López, J.H.; Reátegui, R.J. Implementation of Logic Gates in an Erbium-Doped Fiber Laser (EDFL): Numerical and Experimental Analysis. Photonics 2022, 9, 977. https://doi.org/10.3390/photonics9120977
Afanador Delgado SM, Echenausía Monroy JL, Huerta Cuellar G, García López JH, Reátegui RJ. Implementation of Logic Gates in an Erbium-Doped Fiber Laser (EDFL): Numerical and Experimental Analysis. Photonics. 2022; 9(12):977. https://doi.org/10.3390/photonics9120977
Chicago/Turabian StyleAfanador Delgado, Samuel Mardoqueo, José Luis Echenausía Monroy, Guillermo Huerta Cuellar, Juan Hugo García López, and Rider Jaimes Reátegui. 2022. "Implementation of Logic Gates in an Erbium-Doped Fiber Laser (EDFL): Numerical and Experimental Analysis" Photonics 9, no. 12: 977. https://doi.org/10.3390/photonics9120977
APA StyleAfanador Delgado, S. M., Echenausía Monroy, J. L., Huerta Cuellar, G., García López, J. H., & Reátegui, R. J. (2022). Implementation of Logic Gates in an Erbium-Doped Fiber Laser (EDFL): Numerical and Experimental Analysis. Photonics, 9(12), 977. https://doi.org/10.3390/photonics9120977








