Measurement of Stress Optical Coefficient for Silicone Adhesive Based on Terahertz Time Domain Spectroscopy
Abstract
1. Introduction
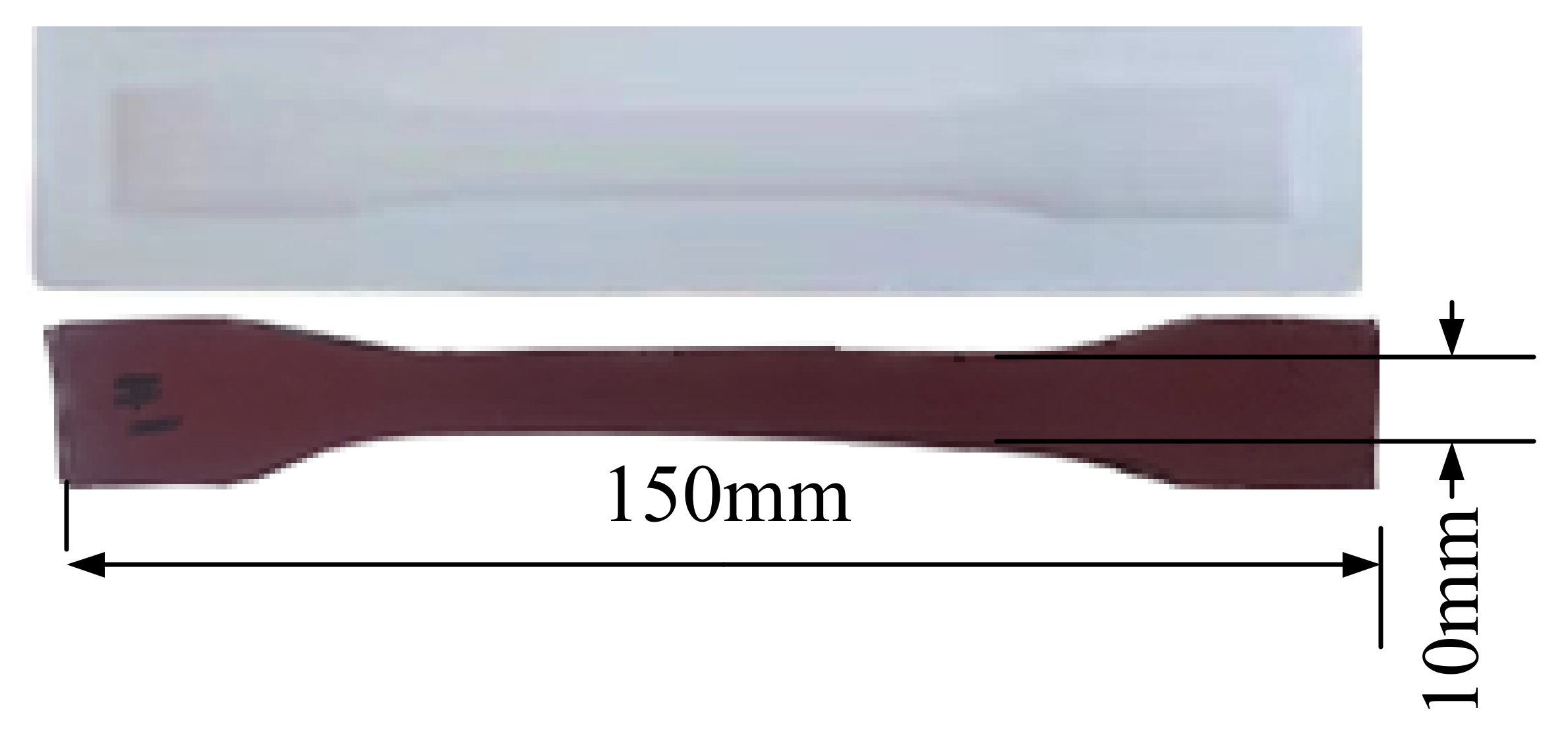
2. Materials and Experiments
3. Methods and Models
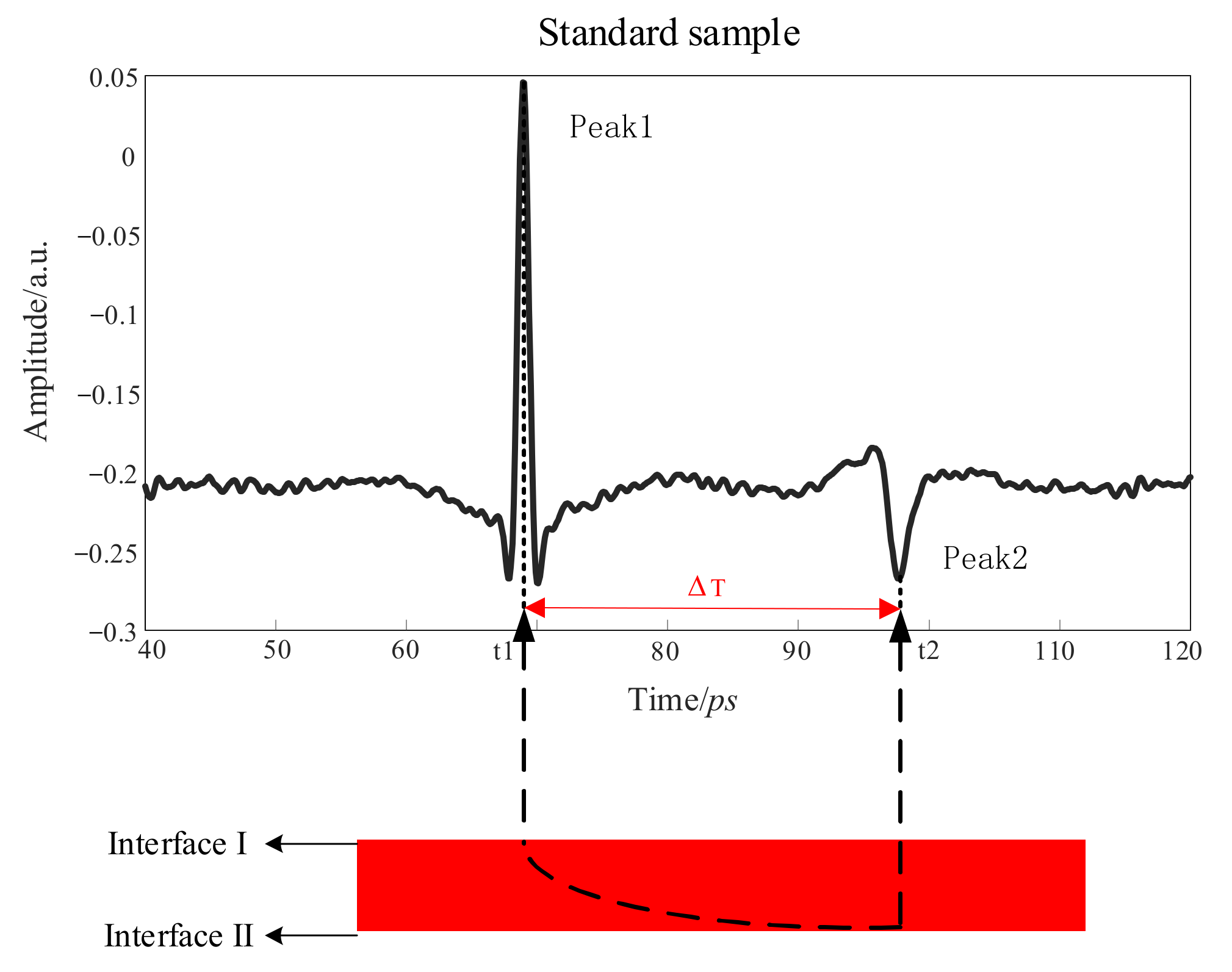
3.1. Model of Stress Measurement
3.2. Calculation Model of Stress Optical Coefficient of Transmission THz-TDS
3.3. Calculation Model of Stress Optical Coefficient of Reflection THz-TDS
4. Results and Discussion
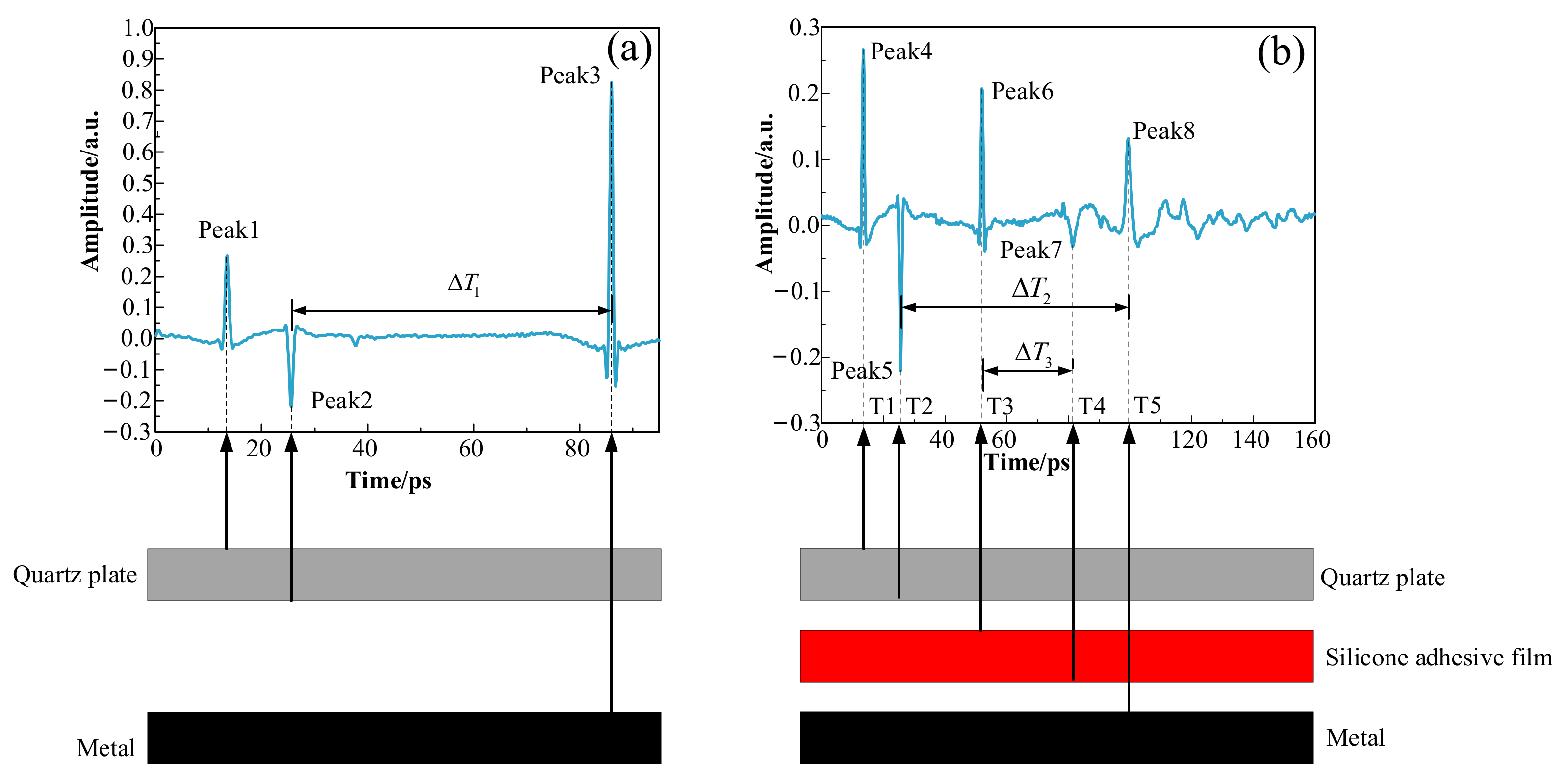
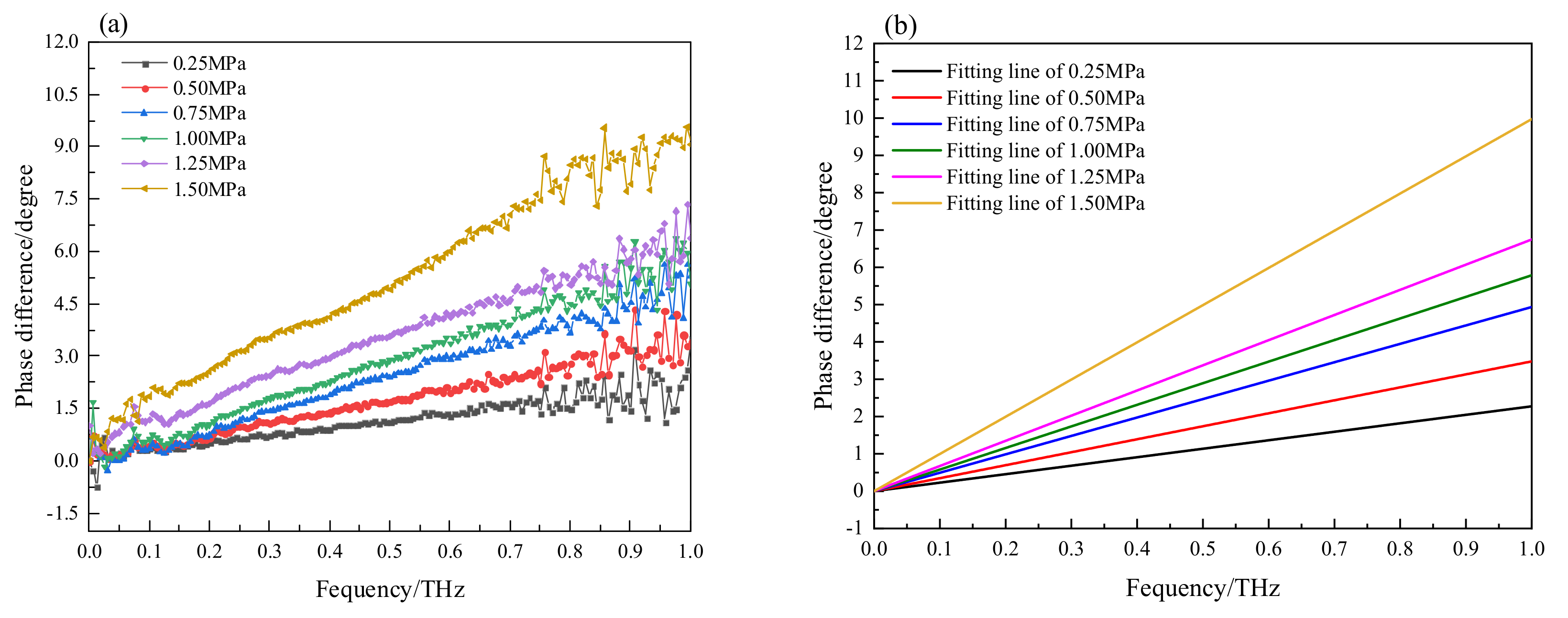
4.1. Measurement of Stress Optical Coefficient of Silicone Adhesive in Transmission System
4.2. Measurement of Stress Optical Coefficient of Silicone Adhesive in Reflection System
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yang, S.; Gu, L.; Gibson, R.F. Nondestructive detection of weak joints in adhesively bonded composite structures. Compos. Struct. 2001, 51, 63–71. [Google Scholar] [CrossRef]
- Romano, M.G.; Guida, M.; Marulo, F.; Giugliano Auricchio, M.; Russo, S. Characterization of adhesives bonding in aircraft structures. Materials 2020, 13, 4816. [Google Scholar] [CrossRef] [PubMed]
- Abdel-Monsef, S.; Renart, J.; Carreras, L.; Maimí, P.; Turon, A. Environmental effects on the cohesive laws of the composite bonded joints. Compos. Part A 2022, 155, 106798. [Google Scholar] [CrossRef]
- Amancio-Filho, S.T.; dos Santos, J.F. Joining of polymers and polymer–metal hybrid structures: Recent developments and trends. Polym. Eng. Sci. 2009, 49, 1461–1476. [Google Scholar] [CrossRef]
- Giese-Hinz, J.; Kothe, C.; Louter, C.; Weller, B. Mechanical and chemical analysis of structural silicone adhesives with the influence of artificial aging. Int. J. Adhes. Adhes. 2021, 117, 103019. [Google Scholar] [CrossRef]
- Zhong, Y.-F.; Ren, J.J.; Li, L.-J.; Zhang, J.; Zhang, D.; Gu, J.; Xue, J.; Chen, Q. Terahertz data analytics-based bonding interface damage characterization in a multilayer structural composites under cyclic loading. Polym. Test. 2022, 116, 107785. [Google Scholar] [CrossRef]
- Matejícek, J.; Sampath, S.; Dubsky, J. X-ray residual stress measurement in metallic and ceramic plasma sprayed coatings. J. Therm. Spray Technol. 1998, 7, 489–496. [Google Scholar] [CrossRef]
- Loechelt, G.H.; Cave, N.G.; Menéndez, J. Measuring the tensor nature of stress in silicon using polarized off-axis Raman spectroscopy. Appl. Phys. Lett. 1995, 66, 3639–3641. [Google Scholar] [CrossRef]
- Jiang, P.; Fan, X.L.; Sun, Y.L.; Wang, H.T.; Su, L.C.; Wang, T.J. Thermal-cycle dependent residual stress within the crack-susceptible zone in thermal barrier coating system. J. Am. Ceram. Soc. 2018, 101, 4256–4261. [Google Scholar] [CrossRef]
- James, D.F.; Phillips, J. Manual on Experimental Stress Analysis; Society for Experimental Mechanics: Bethel, CT, USA, 1989. [Google Scholar]
- Dally, J.; Riley, W. Experimental Stress Analysis; McGraw-Hill: New York, NY, USA, 1978. [Google Scholar]
- Zhang, D.D.; Ren, J.J.; Gu, J.; Li, L.J.; Zhang, J.; Xiong, W.; Zhong, Y.; Zhou, T. Nondestructive testing of bonding defects in multilayered ceramic matrix composites using THz time domain spectroscopy and imaging. Compos. Struct. 2020, 251, 112624. [Google Scholar] [CrossRef]
- Beiranvand, B.; Sobolev, A.S.; Larionov, M.Y.; Kuzmin, L.S. A Distributed Terahertz Metasurface with Cold-Electron Bolometers for Cosmology Missions. Appl. Sci. 2021, 11, 4459. [Google Scholar] [CrossRef]
- Behrokh, B.; Sobolev, A.S.; Anton, V.; Kudryashov, A.V. Composite right/left-handed transmission line with array of thermocouples for generating terahertz radiation. Appl. Phys. 2020, 92, 20502. [Google Scholar] [CrossRef]
- Han, C.; Wang, Y.; Li, Y.Q.; Chen, Y.; Abbasi, N.A.; Kurner, T.; Molisch, A.F. Terahertz Wireless Channels: A Holistic Survey on Measurement, Modeling, and Analysis. IEEE Commun. Surv. Tutor. 2022, 24, 1670–1707. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Han, C.; Hu, Z.; Nie, S.; Jornet, J.M. Terahertz Band Communication: An Old Problem Revisited and Research Directions for the Next Decade. IEEE Trans. Commun. 2022, 70, 4250–4285. [Google Scholar] [CrossRef]
- Zhang, L.L.; Zhong, H.; Deng, C.; Zhang, C.L.; Zhao, Y.J. Terahertz polarization imaging with birefringent materials. Opt. Commun. 2010, 283, 4993–4995. [Google Scholar] [CrossRef]
- Schemmel, P.; Diederich, G.; Moore, A.J. Direct stress optic coefficients for YTZP ceramic and PTFE at GHz frequencies. Opt. Express 2016, 24, 8110–8119. [Google Scholar] [CrossRef] [PubMed]
- Moriwaki, A.; Okano, M.; Watanabe, S. Internal triaxial strain imaging of visibly opaque black rubbers with terahertz polarization spectroscopy. APL Photonics 2017, 2, 106101. [Google Scholar] [CrossRef]
- Song, W.; Li, L.-A.; Wang, Z.; Wang, S.; He, M.; Han, J.; Cong, L.; Deng, Y. Experimental verification of the uniaxial stress-optic law in the terahertz frequency regime. Opt. Lasers Eng. 2014, 52, 174–177. [Google Scholar] [CrossRef]
- Wang, Z.; Kang, K.; Wang, S.; Li, L.; Xu, N.; Han, J.; He, M.; Wu, L.; Zhang, W.; Jia, G.; et al. Determination of plane stress state using terahertz time-domain spectroscopy. Sci. Rep. 2016, 6, 36308. [Google Scholar] [CrossRef] [PubMed]
- Sotta, P.; Albouy, P.A.; Abou Taha, M.; Moreaux, B.; Fayolle, C. Crosslinked elastomers: Structure–property relationships and stress-optical law. Polymers 2021, 14, 9. [Google Scholar] [CrossRef] [PubMed]
- Muller, R.; Vergnes, B. Validity of the stress optical law and application of birefringence to polymer complex flows. Rheol. Ser. 1996, 5, 257–284. [Google Scholar] [CrossRef]
- Decruppe, J.; Humbert, C. Stress Optical Coefficient of Viscoelastic Solutions; Steinkopff: Heidelberg, Germany, 1998. [Google Scholar]



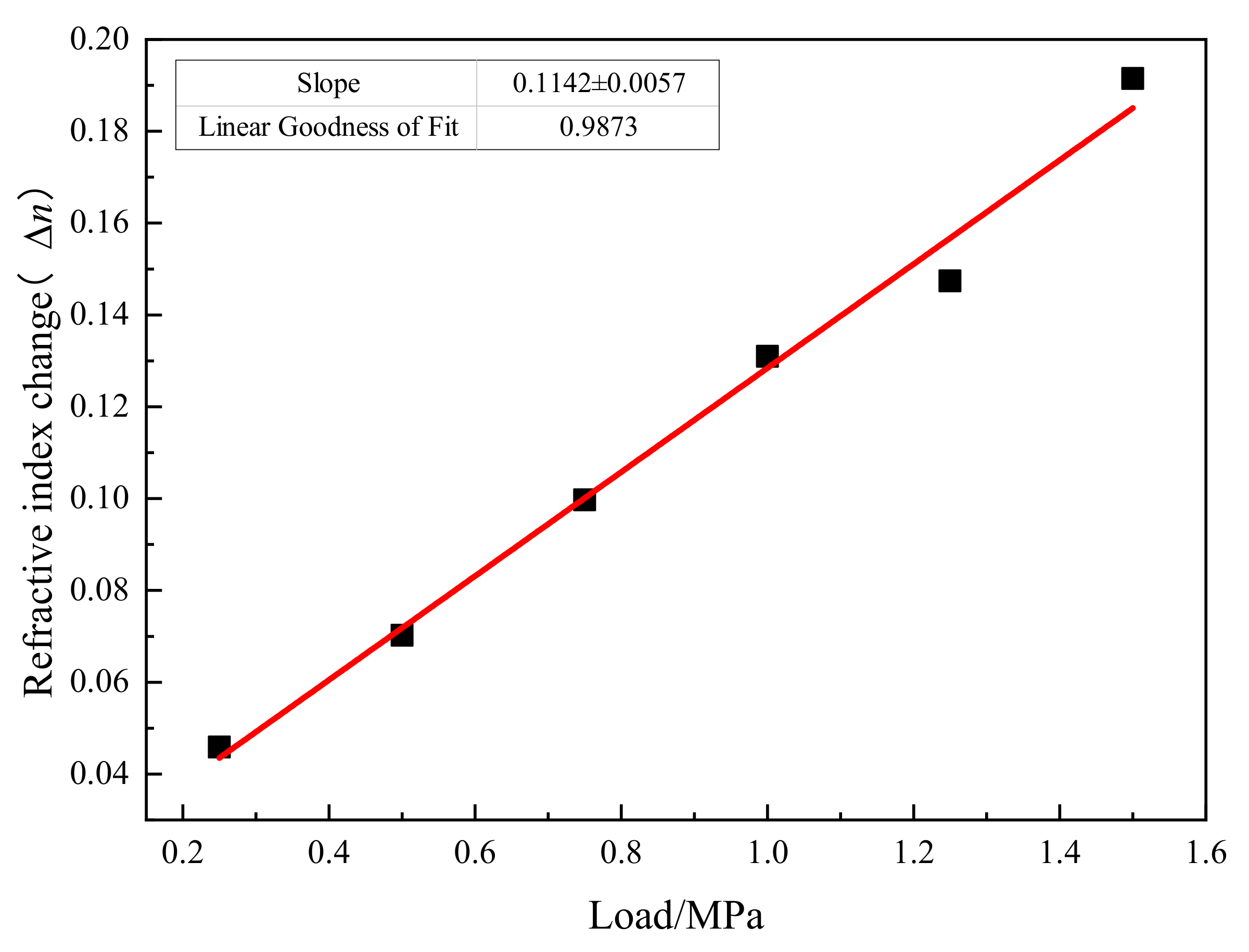

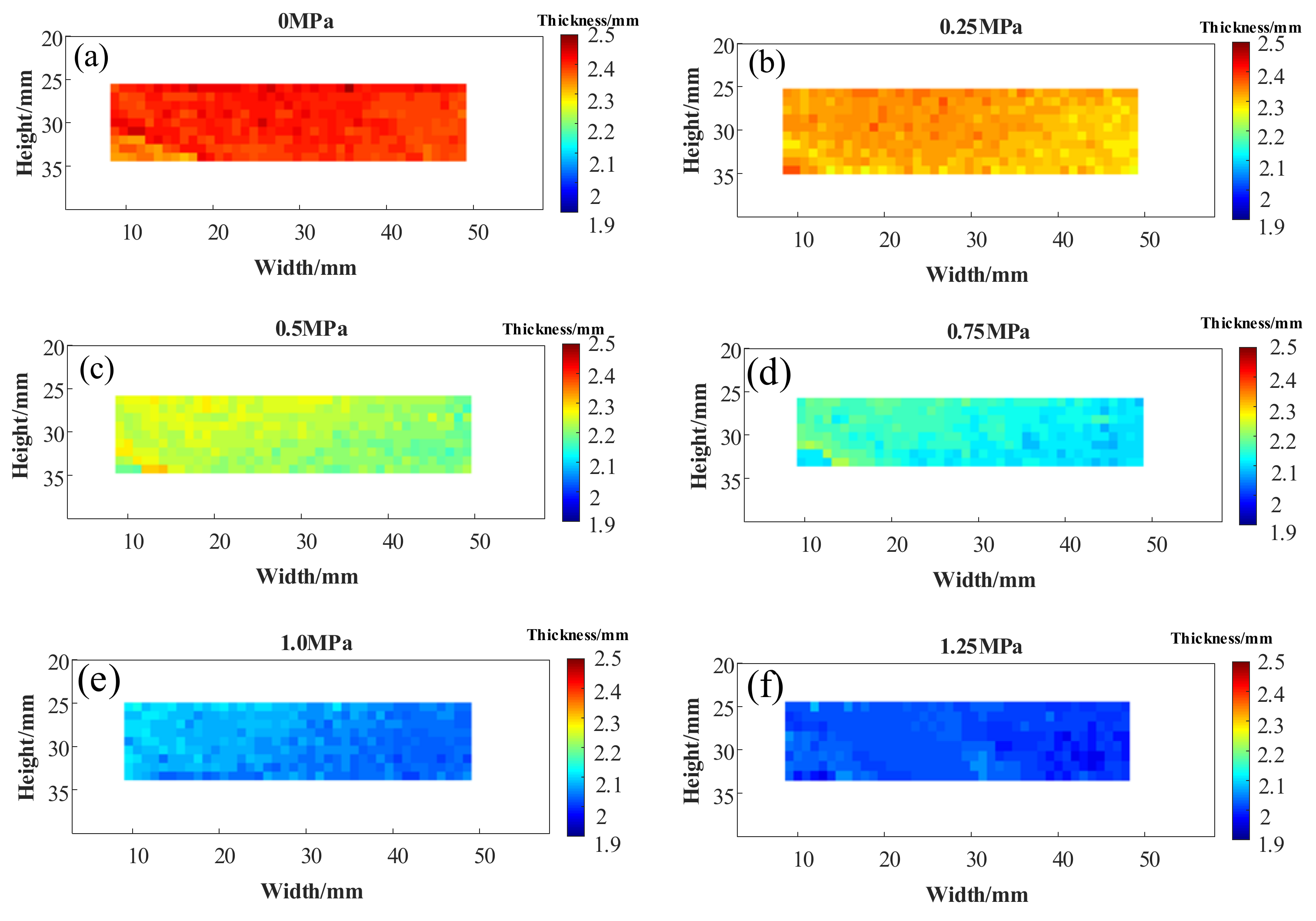

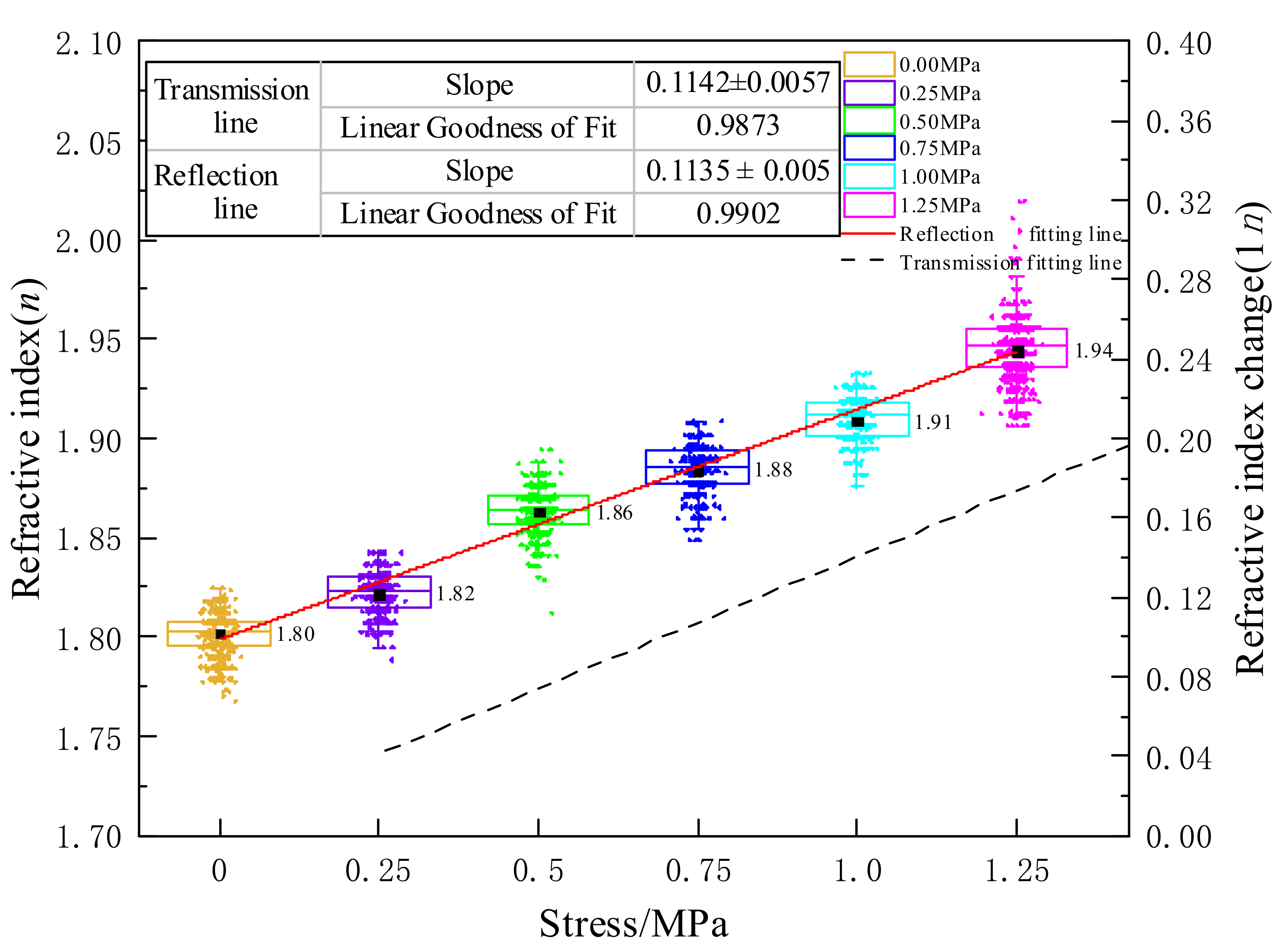
| Tensile Strength | Elastic Modulus (E) | Poisson Ratio (μ) | |
|---|---|---|---|
| RTV560 | 4.8 Mpa | 1.2 Gpa | 0.48 |
| 0.25 MPa | 0.5 MPa | 0.75 MPa | 1.0 MPa | 1.25 MPa | 1.5 MPa | |
|---|---|---|---|---|---|---|
| Slope | 2.274 ± 0.037 | 3.478 ± 0.030 | 4.936 ± 0.030 | 5.787 ± 0.036 | 6.746 ± 0.052 | 9.972 ± 0.069 |
| Linear Goodness of Fit | 0.9595 | 0.9878 | 0.9936 | 0.9936 | 0.9905 | 0.9924 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, Y.-F.; Ren, J.-J.; Li, L.-J.; Zhang, J.-Y.; Zhang, D.-D.; Gu, J.; Xue, J.-W.; Chen, Q. Measurement of Stress Optical Coefficient for Silicone Adhesive Based on Terahertz Time Domain Spectroscopy. Photonics 2022, 9, 929. https://doi.org/10.3390/photonics9120929
Zhong Y-F, Ren J-J, Li L-J, Zhang J-Y, Zhang D-D, Gu J, Xue J-W, Chen Q. Measurement of Stress Optical Coefficient for Silicone Adhesive Based on Terahertz Time Domain Spectroscopy. Photonics. 2022; 9(12):929. https://doi.org/10.3390/photonics9120929
Chicago/Turabian StyleZhong, Yi-Fan, Jiao-Jiao Ren, Li-Juan Li, Ji-Yang Zhang, Dan-Dan Zhang, Jian Gu, Jun-Wen Xue, and Qi Chen. 2022. "Measurement of Stress Optical Coefficient for Silicone Adhesive Based on Terahertz Time Domain Spectroscopy" Photonics 9, no. 12: 929. https://doi.org/10.3390/photonics9120929
APA StyleZhong, Y.-F., Ren, J.-J., Li, L.-J., Zhang, J.-Y., Zhang, D.-D., Gu, J., Xue, J.-W., & Chen, Q. (2022). Measurement of Stress Optical Coefficient for Silicone Adhesive Based on Terahertz Time Domain Spectroscopy. Photonics, 9(12), 929. https://doi.org/10.3390/photonics9120929




