Nanoparticle Shape Optimization for Tamm-Plasmon-Polariton-Based Organic Solar Cells in the Visible Spectral Range
Abstract
1. Introduction
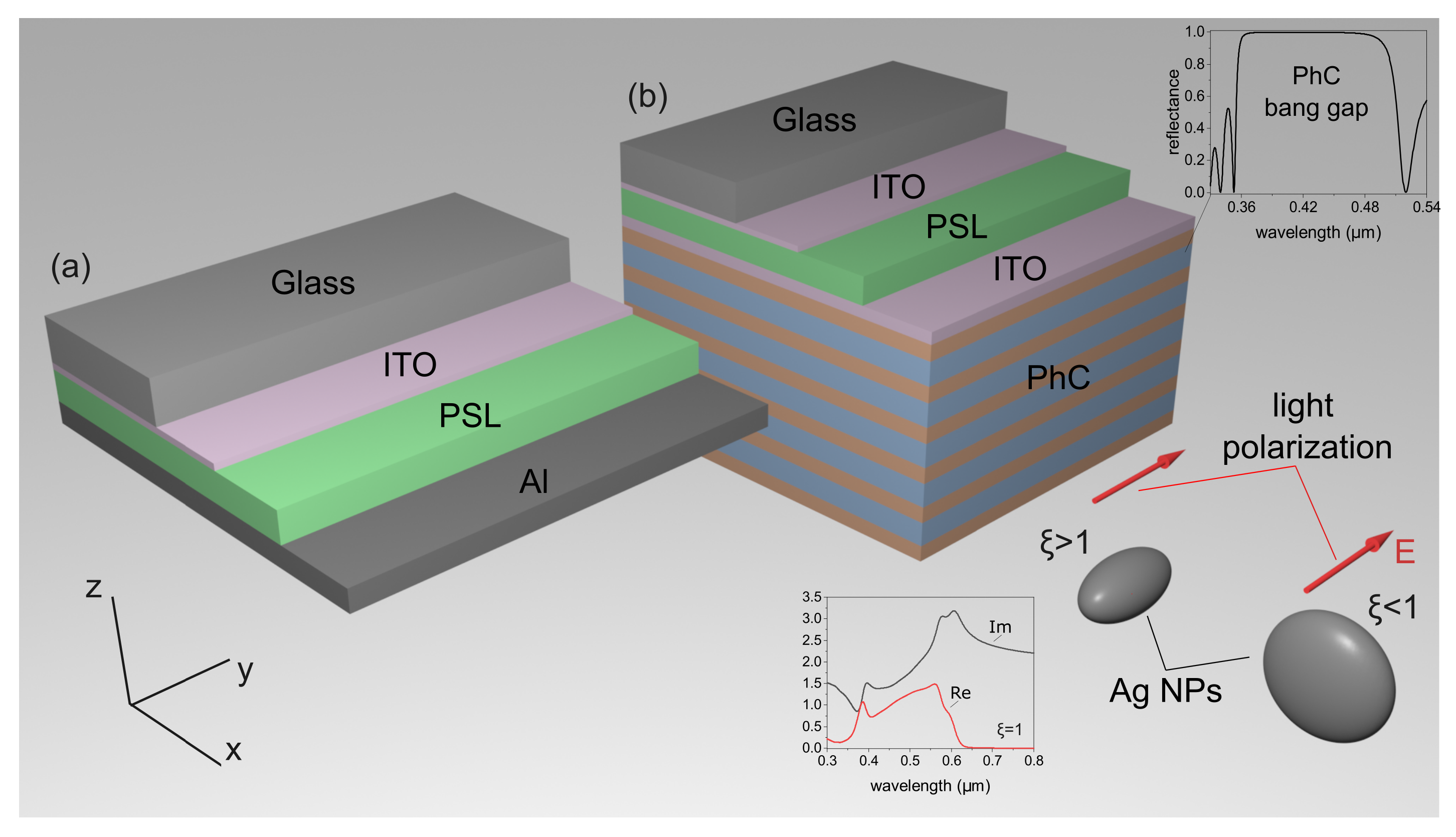
2. Description of the Model
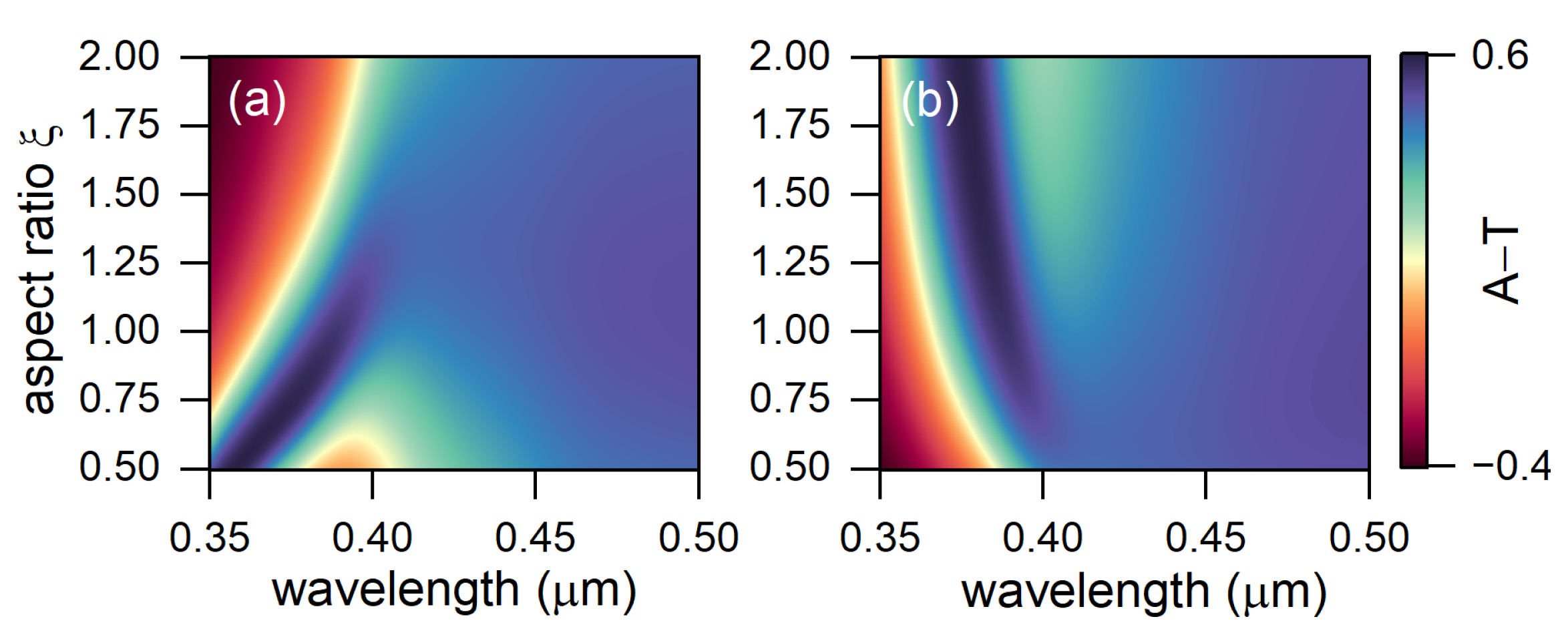
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vinogradov, A.P.; Dorofeenko, A.V.; Merzlikin, A.M.; Lisyansky, A. Surface states in photonic crystals. Physics-Uspekhi 2010, 53, 243–256. [Google Scholar] [CrossRef]
- Kavokin, A.V.; Shelykh, I.A.; Malpuech, G. Lossless interface modes at the boundary between two periodic dielectric structures. Phys. Rev. B 2005, 72, 233102. [Google Scholar] [CrossRef]
- Kaliteevski, M.; Iorsh, I.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Kavokin, A.V.; Shelykh, I.A. Tamm plasmon-polaritons: Possible electromagnetic states at the interface of a metal and a dielectric Bragg mirror. Phys. Rev. B 2007, 76, 165415. [Google Scholar] [CrossRef]
- Pyatnov, M.; Bikbaev, R.; Timofeev, I.; Ryzhkov, I.; Vetrov, S.; Shabanov, V. Broadband Tamm Plasmons in Chirped Photonic Crystals for Light-Induced Water Splitting. Nanomaterials 2022, 12, 928. [Google Scholar] [CrossRef] [PubMed]
- Wu, F.; Wu, X.; Xiao, S.; Liu, G.; Li, H. Broadband wide-angle multilayer absorber based on a broadband omnidirectional optical Tamm state. Opt. Express 2021, 29, 23976. [Google Scholar] [CrossRef] [PubMed]
- Xue, C.H.; Wu, F.; Jiang, H.T.; Li, Y.; Zhang, Y.W.; Chen, H. Wide-angle Spectrally Selective Perfect Absorber by Utilizing Dispersionless Tamm Plasmon Polaritons. Sci. Rep. 2016, 6, 39418. [Google Scholar] [CrossRef]
- Juneau-Fecteau, A.; Savin, R.; Boucherif, A.; Fréchette, L.G. A practical Tamm plasmon sensor based on porous Si. AIP Adv. 2021, 11, 065305. [Google Scholar] [CrossRef]
- Xu, W.H.; Chou, Y.H.; Yang, Z.Y.; Liu, Y.Y.; Yu, M.W.; Huang, C.H.; Chang, C.T.; Huang, C.Y.; Lu, T.C.; Lin, T.R.; et al. Tamm Plasmon-Polariton Ultraviolet Lasers. Adv. Photonics Res. 2021, 3, 2100120. [Google Scholar] [CrossRef]
- Bikbaev, R.G.; Maksimov, D.N.; Chen, K.P.; Timofeev, I.V. Double-Resolved Beam Steering by Metagrating-Based Tamm Plasmon Polariton. Materials 2022, 15, 6014. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Zhu, Y.; Wang, W.; Li, Y.; Gao, R.; Yu, P.; Xu, H.; Wang, Z. Broadband Tamm plasmon-enhanced planar hot-electron photodetector. Nanoscale 2020, 12, 23945–23952. [Google Scholar] [CrossRef]
- Bikbaev, R.G.; Vetrov, S.Y.; Timofeev, I.V.; Shabanov, V.F. Tamm Plasmon Polaritons for Light Trapping in Organic Solar Cells. Dokl. Phys. 2020, 65, 161–163. [Google Scholar] [CrossRef]
- Zhang, X.L.; Song, J.F.; Li, X.B.; Feng, J.; Sun, H.B. Optical Tamm states enhanced broad-band absorption of organic solar cells. Appl. Phys. Lett. 2012, 101, 243901. [Google Scholar] [CrossRef]
- Bikbaev, R.G.; Vetrov, S.Y.; Timofeev, I.V.; Shabanov, V.F. Photosensitivity and reflectivity of the active layer in a Tamm-plasmon-polariton-based organic solar cell. Appl. Opt. 2021, 60, 3338. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.X. Organic Solar Cells: Recent Progress and Challenges. ACS Energy Lett. 2019, 4, 2537–2539. [Google Scholar] [CrossRef]
- Yang, F.; Huang, Y.; Li, Y.; Li, Y. Large-area flexible organic solar cells. Npj Flex. Electron. 2021, 5, 30. [Google Scholar] [CrossRef]
- Li, Y.; Huang, W.; Zhao, D.; Wang, L.; Jiao, Z.; Huang, Q.; Wang, P.; Sun, M.; Yuan, G. Recent Progress in Organic Solar Cells: A Review on Materials from Acceptor to Donor. Molecules 2022, 27, 1800. [Google Scholar] [CrossRef] [PubMed]
- Bikbaev, R.G.; Pykhtin, D.A.; Vetrov, S.Y.; Timofeev, I.V.; Shabanov, V.F. Nanostructured photosensitive layer for Tamm-plasmon-polariton-based organic solar cells. Appl. Opt. 2022, 61, 5049. [Google Scholar] [CrossRef]
- Stelling, C.; Singh, C.R.; Karg, M.; König, T.A.F.; Thelakkat, M.; Retsch, M. Plasmonic nanomeshes: Their ambivalent role as transparent electrodes in organic solar cells. Sci. Rep. 2017, 7, 42530. [Google Scholar] [CrossRef]
- Malitson, I.H. Interspecimen Comparison of the Refractive Index of Fused Silica. J. Opt. Soc. Am. 1965, 55, 1205–1209. [Google Scholar] [CrossRef]
- DeVore, J.R. Refractive Indices of Rutile and Sphalerite. J. Opt. Soc. Am. 1951, 41, 416–419. [Google Scholar] [CrossRef]
- Colours in metal glasses, in metallic films and in metallic solutions—II. Proc. R. Soc. London. Ser. A Contain. Pap. A Math. Phys. Character 1905, 76, 370–373. [CrossRef]
- Chen, L.; Mao, S.; Wang, P.; Yao, Z.; Du, Z.; Zhu, Z.; Belfiore, L.A.; Tang, J. Visible Light Driven Hot-Electron Injection by Pd Nanoparticles: Fast Response in Metal–Semiconductor Photodetection. Adv. Opt. Mater. 2020, 9, 2001505. [Google Scholar] [CrossRef]
- Lee, D.; Han, S.G.; Mun, J.; Yang, K.; Kim, S.H.; Rho, J.; Cho, K.; Oh, D.X.; Jeong, M.S. Elucidating the photoluminescence-enhancement mechanism in a push-pull conjugated polymer induced by hot-electron injection from gold nanoparticles. Photonics Res. 2021, 9, 131. [Google Scholar] [CrossRef]
- Yeh, P. Electromagnetic propagation in birefringent layered media. J. Opt. Soc. Am. 1979, 69, 742. [Google Scholar] [CrossRef]
- Vyunishev, A.M.; Bikbaev, R.G.; Svyakhovskiy, S.E.; Timofeev, I.V.; Pankin, P.S.; Evlashin, S.A.; Vetrov, S.Y.; Myslivets, S.A.; Arkhipkin, V.G. Broadband Tamm plasmon polariton. J. Opt. Soc. Am. B 2019, 36, 2299–2305. [Google Scholar] [CrossRef]
- Kim, K.; Lee, S. Detailed balance analysis of plasmonic metamaterial perovskite solar cells. Opt. Express 2019, 27, A1241. [Google Scholar] [CrossRef] [PubMed]
- Sandhu, S.; Yu, Z.; Fan, S. Detailed balance analysis of nanophotonic solar cells. Opt. Express 2013, 21, 1209. [Google Scholar] [CrossRef] [PubMed]
- Kirchartz, T.; Pieters, B.E.; Kirkpatrick, J.; Rau, U.; Nelson, J. Recombination via tail states in polythiophene:fullerene solar cells. Phys. Rev. B 2011, 83. [Google Scholar] [CrossRef]




| (A/m) | (A/m) | |||
|---|---|---|---|---|
| 0.5 | 51.5 | 26.1 | 56.9 | 28.6 |
| 1 | 55.6 | 27.9 | 55.6 | 27.9 |
| 1.5 | 56.5 | 28.4 | 51.3 | 25.8 |
| 2 | 57.0 | 28.6 | 48.4 | 24.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bikbaev, R.G.; Vetrov, S.Y.; Timofeev, I.V.; Shabanov, V.F. Nanoparticle Shape Optimization for Tamm-Plasmon-Polariton-Based Organic Solar Cells in the Visible Spectral Range. Photonics 2022, 9, 786. https://doi.org/10.3390/photonics9110786
Bikbaev RG, Vetrov SY, Timofeev IV, Shabanov VF. Nanoparticle Shape Optimization for Tamm-Plasmon-Polariton-Based Organic Solar Cells in the Visible Spectral Range. Photonics. 2022; 9(11):786. https://doi.org/10.3390/photonics9110786
Chicago/Turabian StyleBikbaev, Rashid G., Stepan Ya. Vetrov, Ivan V. Timofeev, and Vasily F. Shabanov. 2022. "Nanoparticle Shape Optimization for Tamm-Plasmon-Polariton-Based Organic Solar Cells in the Visible Spectral Range" Photonics 9, no. 11: 786. https://doi.org/10.3390/photonics9110786
APA StyleBikbaev, R. G., Vetrov, S. Y., Timofeev, I. V., & Shabanov, V. F. (2022). Nanoparticle Shape Optimization for Tamm-Plasmon-Polariton-Based Organic Solar Cells in the Visible Spectral Range. Photonics, 9(11), 786. https://doi.org/10.3390/photonics9110786







