Effective Control of the Optical Bistability of a Three-Level Quantum Emitter near a Nanostructured Plasmonic Metasurface
Abstract
:1. Introduction
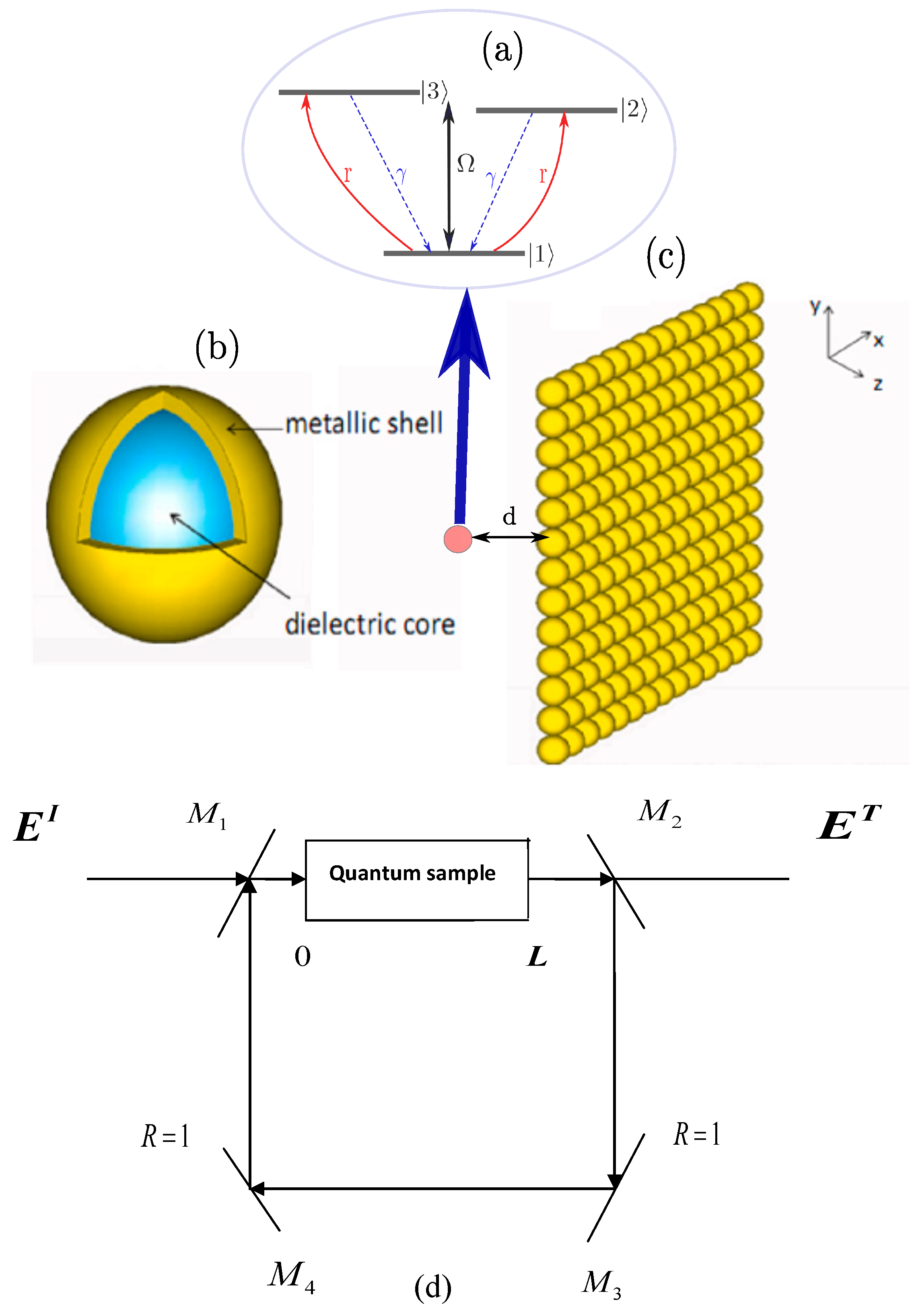
2. Model and Equations
2.1. Hamiltonian and Master Equations
2.2. Susceptibility
2.3. Optical Bistability in a Unidirectional Ring Cavity
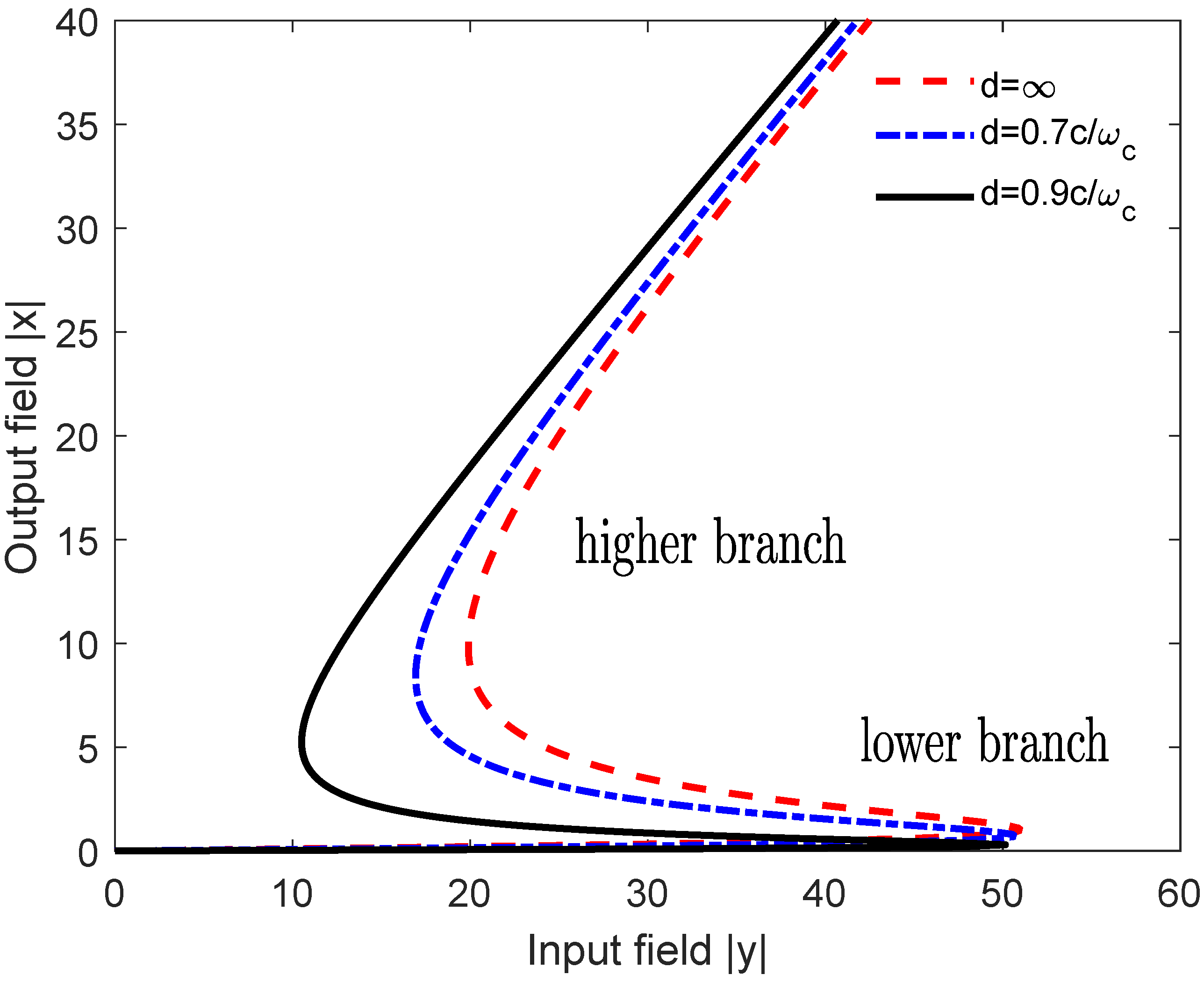
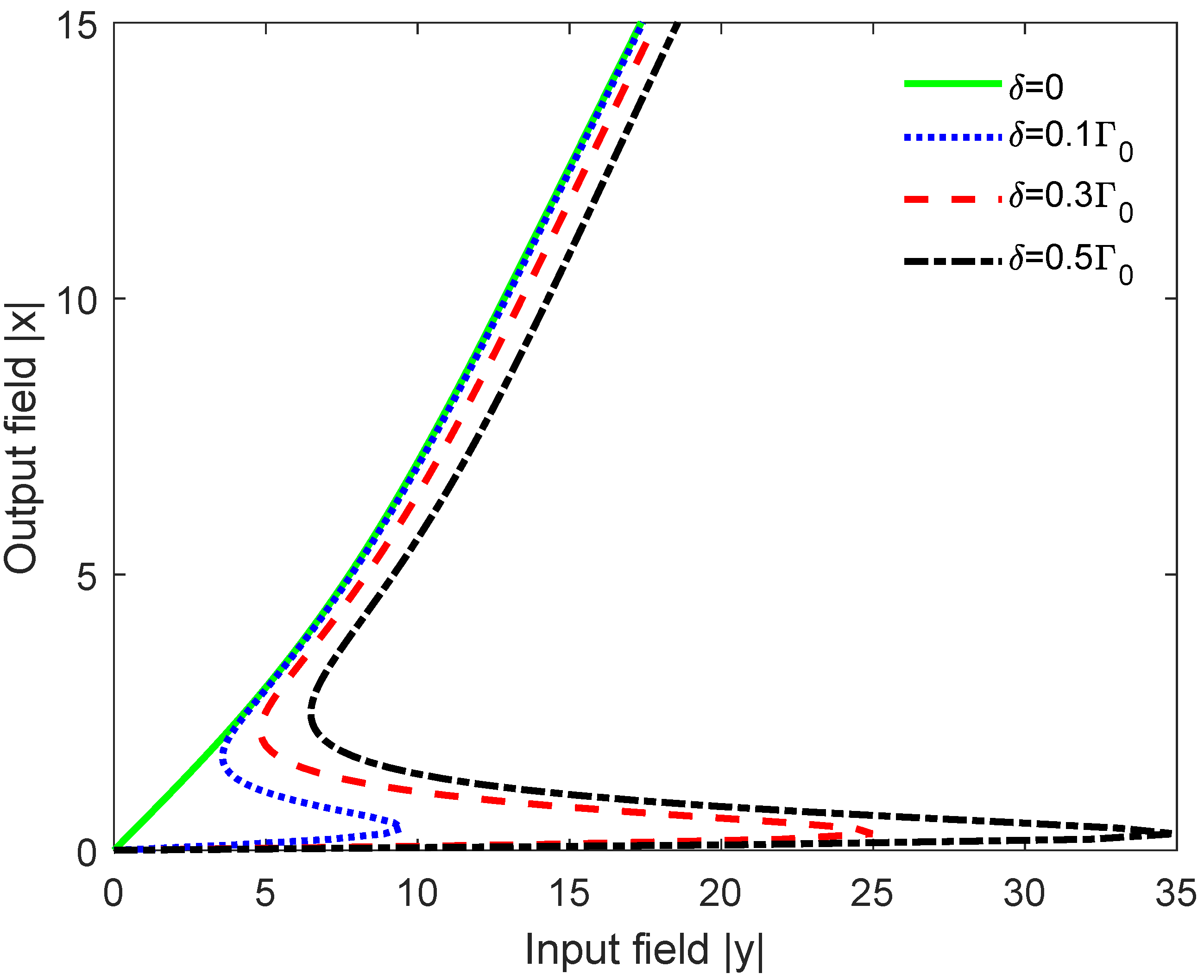
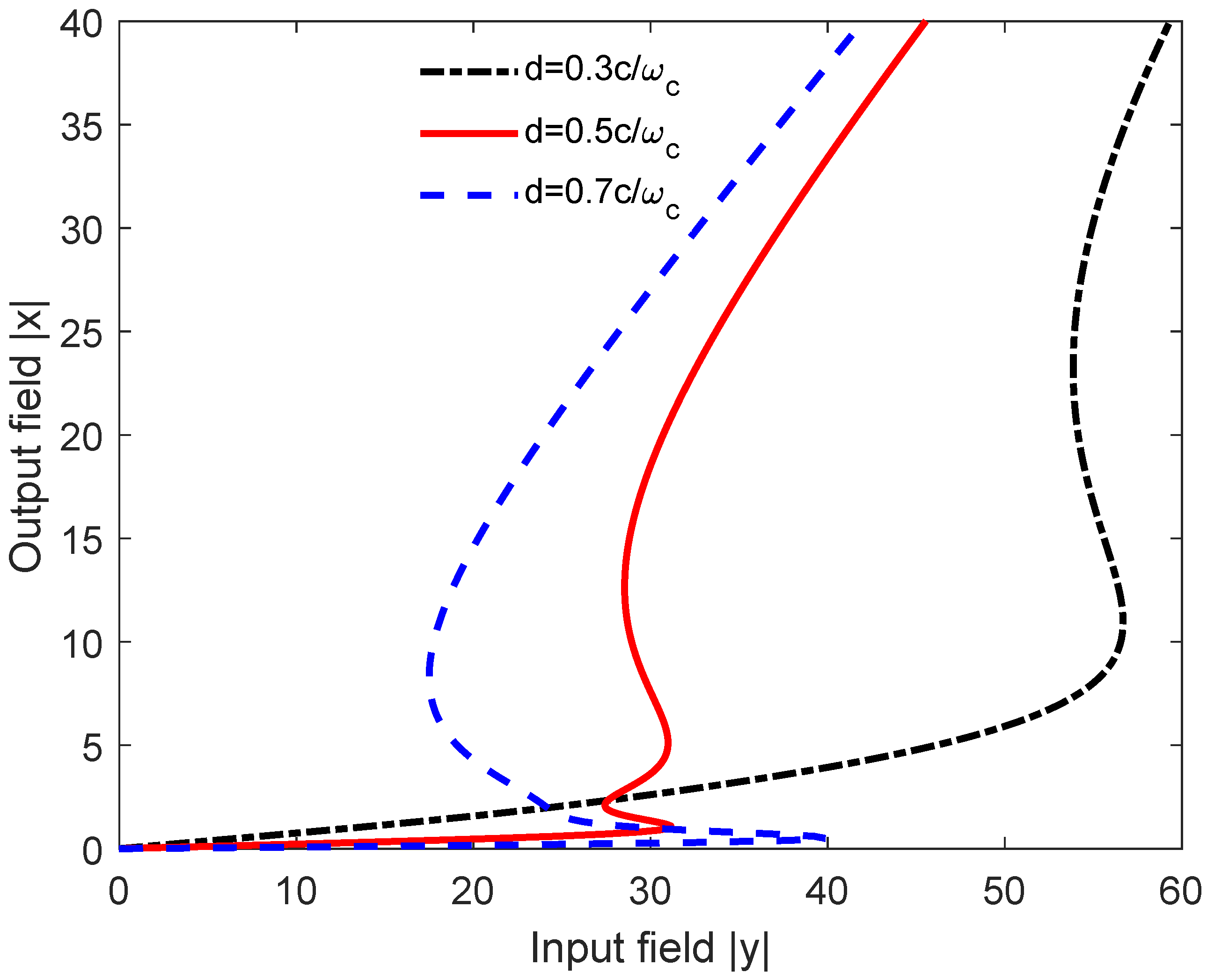
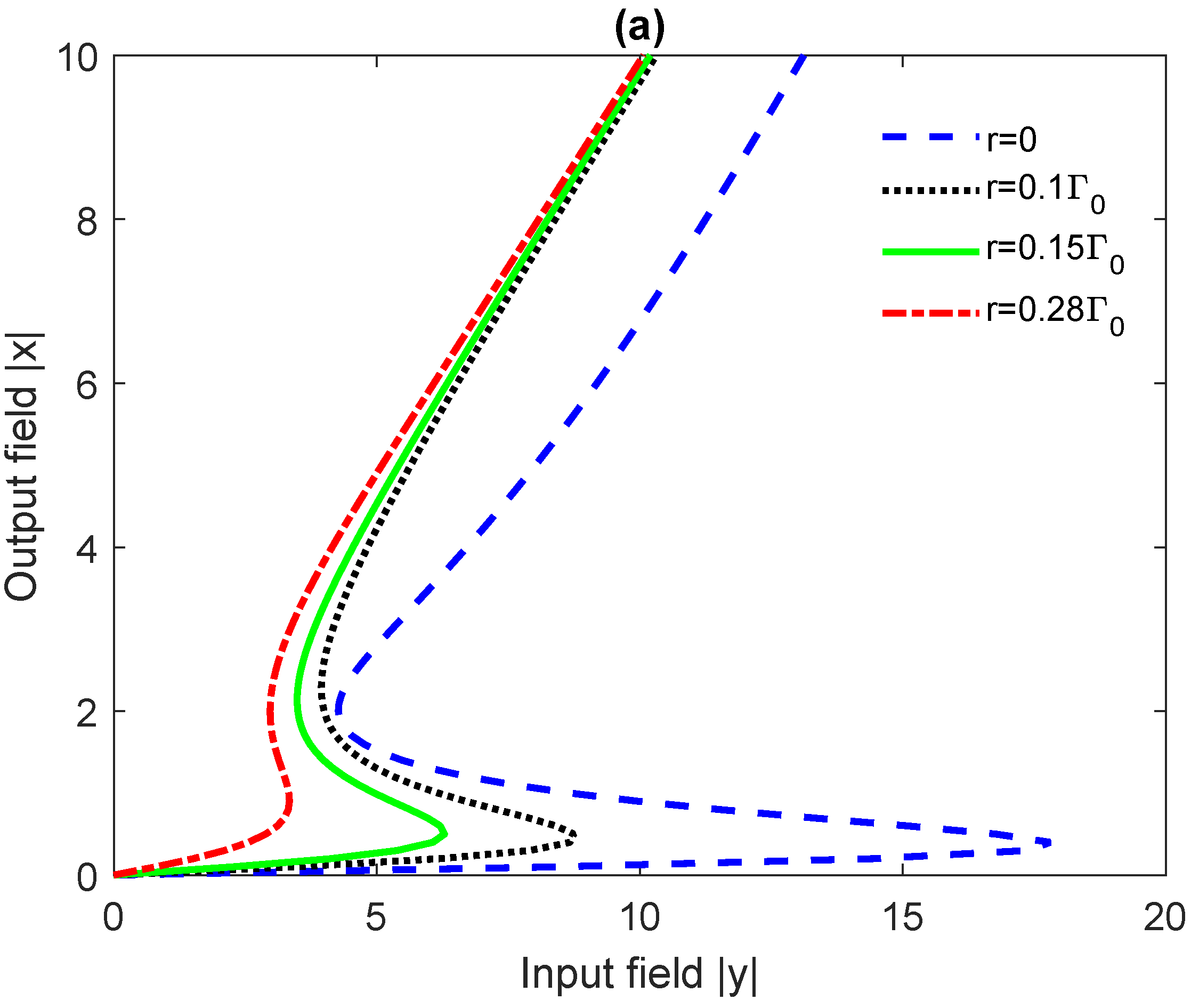
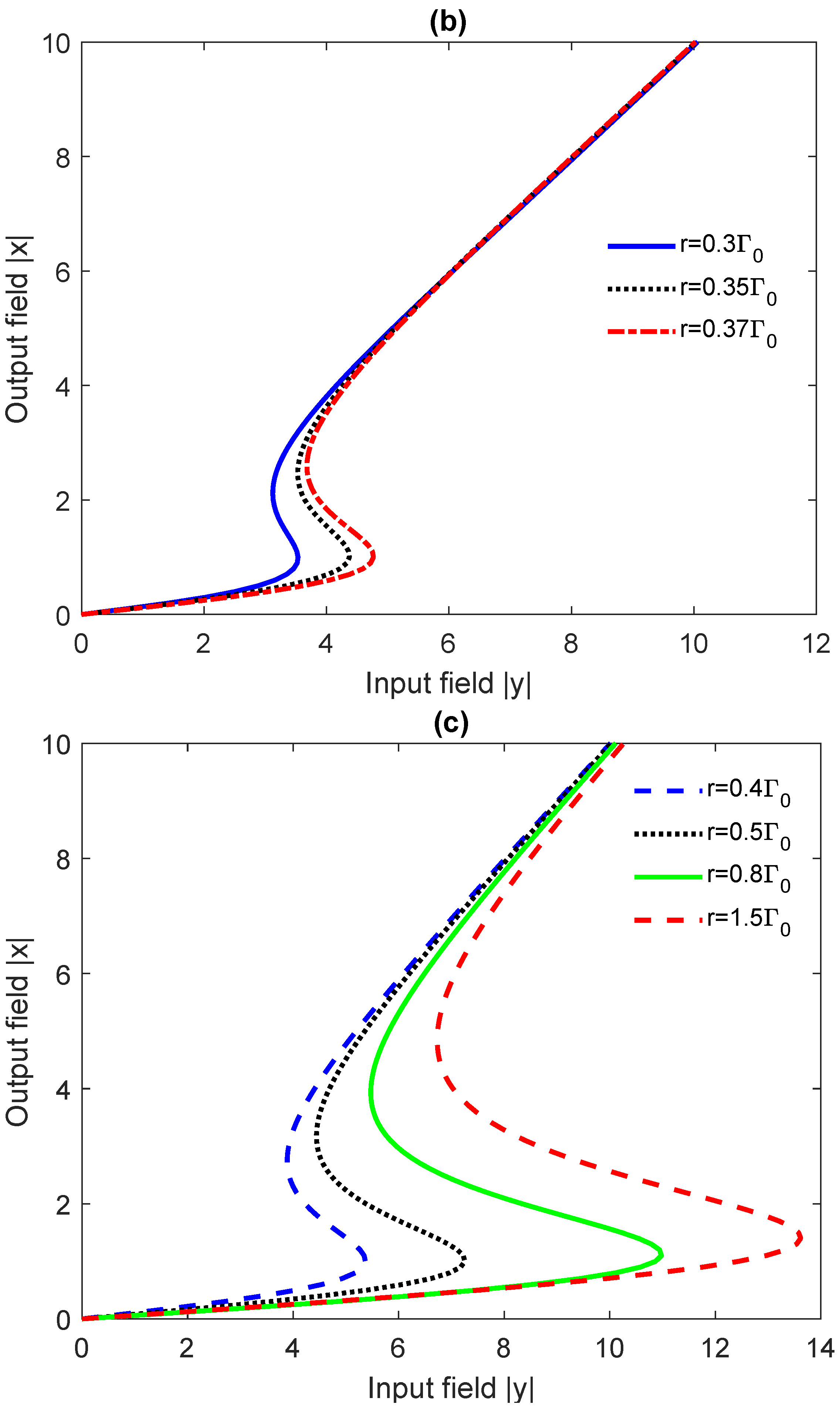
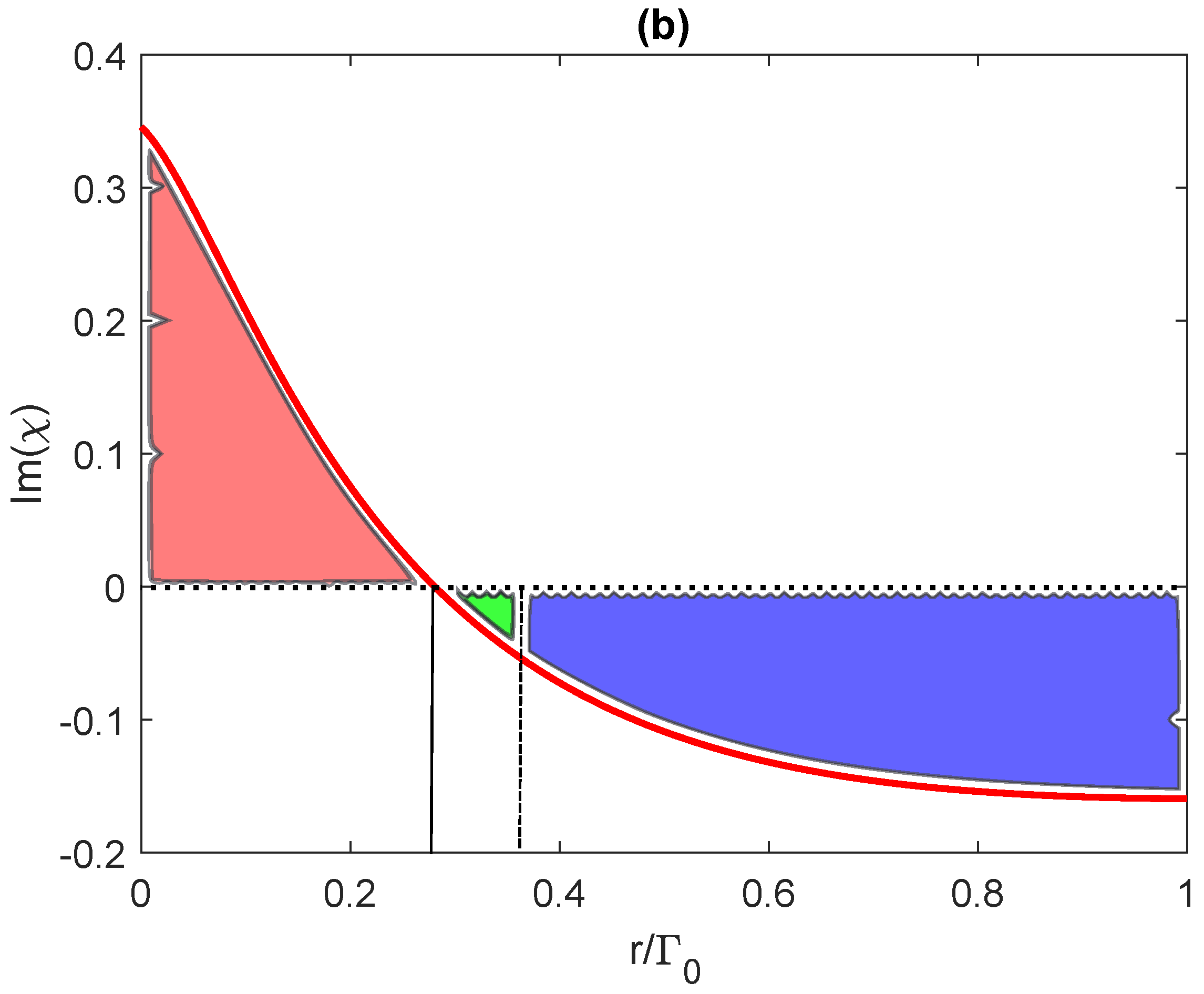
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gibbs, H.M.; McCall, S.L.; Venkatesan, T.N.C. Differential Gain and Bistability Using a Sodium-Filled Fabry-Perot Interferometer. Phys. Rev. Lett. 1976, 36, 1135. [Google Scholar] [CrossRef]
- Rosenberger, A.T.; Orozco, L.A.; Kimble, H.J. Observation of absorptive bistability with two-level atoms in a ring cavity. Phys. Rev. A 1983, 28, 2569. [Google Scholar] [CrossRef] [Green Version]
- Harshawardhan, W.; Agarwal, G.S. Controlling optical bistability using electromagnetic-field-induced transparency and quantum interferences. Phys. Rev. A 1996, 53, 1812. [Google Scholar] [CrossRef] [PubMed]
- Chang, H.; Wu, H.; Xie, C.; Wang, H. Controlled Shift of Optical Bistability Hysteresis Curve and Storage of Optical Signals in a Four-Level Atomic System. Phys. Rev. Lett. 2004, 93, 213901. [Google Scholar] [CrossRef] [Green Version]
- Cheng, D.C.; Liu, C.P.; Gong, S.Q. Optical bistability via amplitude and phase control of a microwave field. Opt. Commun. 2006, 263, 111. [Google Scholar] [CrossRef]
- Cheng, D.C.; Liu, C.P.; Gong, S.Q. Optical bistability and multistability via the effect of spontaneously generated coherence in a three-level ladder-type atomic system. Phys. Lett. A 2004, 332, 244. [Google Scholar] [CrossRef]
- Li, J.-H. Controllable optical bistability in a four-subband semiconductor quantum well system. Phys. Rev. B 2007, 75, 155329. [Google Scholar] [CrossRef]
- Wang, Z.; Xu, M. Control of the switch between optical multistability and bistability in three-level V-type atoms. Opt. Commun. 2009, 282, 1574. [Google Scholar] [CrossRef]
- Sahrai, M.; Asadpour, S.; Sadighi-Bonabi, R. Optical bistability via quantum interference from incoherent pumping and spontaneous emission. J. Lumin. 2011, 131, 2395. [Google Scholar] [CrossRef]
- Hamedi, H.R.; Khaledi-Nasab, A.; Raheli, A.; Sahrai, M. Coherent control of optical bistability and multistability via double dark resonances (DDRs). Opt. Commun. 2014, 312, 117. [Google Scholar] [CrossRef]
- Hamedi, H.R.; Sahrai, M.; Khoshsima, H.; Juzeliunas, G. Optical bistability forming due to a Rydberg state. J. Opt. Soc. Am. B 2017, 34, 1923. [Google Scholar] [CrossRef]
- Fleischhauer, M.; Imamoglu, A.; Marangos, J.P. Electromagnetically induced transparency: Optics in coherent media. Rev. Mod. Phys. 2005, 77, 633. [Google Scholar] [CrossRef] [Green Version]
- Yannopapas, V.; Paspalakis, E.; Vitanov, N.V. Plasmon-Induced Enhancement of Quantum Interference near Metallic Nanostructures. Phys. Rev. Lett. 2009, 103, 063602. [Google Scholar] [CrossRef] [PubMed]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Simulating quantum interference in spontaneous decay near plasmonic nanostructures: Population dynamics. Phys. Rev. A 2011, 83, 055805. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Modifying free-space spontaneous emission near a plasmonic nanostructure. Phys. Rev. A 2011, 83, 023819. [Google Scholar] [CrossRef]
- Gu, Y.; Wang, L.; Ren, P.; Zhang, J.; Zhang, T.; Martin, O.J.F.; Gong, Q. Polarized linewidth-controllable double-trapping electromagnetically induced transparency spectra in a resonant plasmon nanocavity. Nano Lett. 2012, 12, 2488. [Google Scholar] [CrossRef] [Green Version]
- Artuso, R.D.; Bryant, G.W. Strongly coupled quantum dot-metal nanoparticle systems: Exciton-induced transparency, discontinuous response, and suppression as driven quantum oscillator effects. Phys. Rev. B 2010, 82, 195419. [Google Scholar] [CrossRef]
- Zhang, W.; Govorov, A.O.; Bryant, G.W. Semiconductor-Metal Nanoparticle Molecules: Hybrid Excitons and the Nonlinear Fano Effect. Phys. Rev. Lett. 2006, 97, 146804. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Singh, M.R.; Schindel, D.G.; Hatef, A. Dipole-dipole interaction in a quantum dot and metallic nanorod hybrid system. Appl. Phys. Lett. 2011, 99, 181106. [Google Scholar] [CrossRef]
- Kosionis, S.G.; Terzis, A.F.; Yannopapas, V.; Paspalakis, E. Nonlocal Effects in Energy Absorption of Coupled Quantum Dot–Metal Nanoparticle Systems. J. Phys. Chem. C 2012, 116, 23663. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Transparency and slow light in a four-level quantum system near a plasmonic nanostructure. Phys. Rev. A 2012, 86, 053811. [Google Scholar] [CrossRef]
- Paspalakis, E.; Evangelou, S.; Yannopapas, V.; Terzis, A.F. Phase-dependent optical effects in a four-level quantum system near a plasmonic nanostructure. Phys. Rev. A 2013, 88, 053832. [Google Scholar] [CrossRef]
- Wang, L.; Gu, Y.; Chen, H.; Zhang, J.-Y.; Cui, Y.; Gerardot, B.; Gong, Q.-H. Polarized linewidth-controllable double-trapping electromagnetically induced transparency spectra in a resonant plasmon nanocavity. Sci. Rep. 2013, 3, 2879. [Google Scholar] [CrossRef]
- Wang, Z.-P.; Yu, B.-L. Plasmonic Control of Refractive Index Without Absorption in Metallic Photonic Crystals Doped with Quantum Dots. Plasmonics 2018, 13, 567. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Modification of Kerr nonlinearity in a four-level quantum system near a plasmonic nanostructure. J. Mod. Optic. 2014, 61, 1458. [Google Scholar] [CrossRef]
- Chen, H.; Ren, J.; Gu, Y.; Zhao, D.; Zhang, J.; Gong, Q. Nanoscale Kerr Nonlinearity Enhancement Using Spontaneously Generated Coherence in Plasmonic Nanocavity. Sci. Rep. 2016, 5, 18315. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hamedi, H.R.; Yannopapas, V.; Mekys, A.; Paspalakis, E. Control of Kerr nonlinearity in a four-level quantum system near a plasmonic nanostructure. Phys. E Low-Dimens. Syst. Nanostruct. 2021, 130, 114662. [Google Scholar] [CrossRef]
- Kosionis, S.G.; Paspalakis, E. Control of Self-Kerr Nonlinearity in a Driven Coupled Semiconductor Quantum Dot–Metal Nanoparticle Structure. J. Phys. Chem. C 2019, 123, 7308. [Google Scholar] [CrossRef]
- Singh, S.K.; Abak, M.K.; Tasgin, M.E. Enhancement of four-wave mixing via interference of multiple plasmonic conversion paths. Phys. Rev. B 2016, 93, 035410. [Google Scholar] [CrossRef] [Green Version]
- Paspalakis, E.; Evangelou, S.; Kosionis, S.G.; Terzis, A.F. Strongly modified four-wave mixing in a coupled semiconductor quantum dot-metal nanoparticle system. J. Appl. Phys. 2014, 115, 083106. [Google Scholar] [CrossRef]
- Sadeghi, S.M. Gain without inversion in hybrid quantum dot–metallic nanoparticle systems. Nanotechnology 2010, 21, 455401. [Google Scholar] [CrossRef] [PubMed]
- Kosionis, S.G.; Terzis, A.F.; Sadeghi, S.M.; Paspalakis, E. Optical response of a quantum dot-metal nanoparticle hybrid interacting with a weak probe field. J. Phys. Condens. Matter 2013, 25, 045304. [Google Scholar] [CrossRef] [PubMed]
- Sadeghi, S.M. Ultrafast plasmonic field oscillations and optics of molecular resonances caused by coherent exciton-plasmon coupling. Phys. Rev. A 2013, 88, 013831. [Google Scholar] [CrossRef]
- Zhao, D.; Gu, Y.; Wu, J.; Zhang, J.; Zhang, T.; Gerardot, B.D.; Gong, Q. Quantum-dot gain without inversion: Effects of dark plasmon-exciton hybridization. Phys. Rev. B 2014, 89, 245433. [Google Scholar] [CrossRef] [Green Version]
- Carreño, F.; Antón, M.A.; Yannopapas, V.; Paspalakis, E. Control of the absorption of a four-level quantum system near a plasmonic nanostructure. Phys. Rev. B 2017, 95, 195410. [Google Scholar] [CrossRef]
- Kosionis, S.G.; Paspalakis, E. Pump-probe optical response of semiconductor quantum dot–metal nanoparticle hybrids. J. Appl. Phys. 2018, 124, 223104. [Google Scholar] [CrossRef]
- Malyshev, A.V.; Malyshev, V.A. Optical bistability and hysteresis of a hybrid metal-semiconductor nanodimer. Phys. Rev. B 2011, 84, 035314. [Google Scholar] [CrossRef]
- Nugroho, B.S.; Malyshev, V.A.; Knoester, J. Tailoring optical response of a hybrid comprising a quantum dimer emitter strongly coupled to a metallic nanoparticle. Phys. Rev. B 2015, 92, 165432. [Google Scholar] [CrossRef] [Green Version]
- Carreno, F.; Anton, M.A.; Paspalakis, E. Nonlinear optical rectification and optical bistability in a coupled asymmetric quantum dot-metal nanoparticle hybrid. J. Appl. Phys. 2018, 124, 113107. [Google Scholar] [CrossRef]
- Nugroho, B.S.; Iskandar, A.A.; Malyshev, V.A.; Knoester, J. Bistable optical response of a nanoparticle heterodimer: Mechanism, phase diagram, and switching time. J. Chem. Phys. 2013, 139, 014303. [Google Scholar] [CrossRef] [Green Version]
- Mohammadzadeh, A.; Miri, M. Optical response of hybrid semiconductor quantum dot-metal nanoparticle system: Beyond the dipole approximation. J. Appl. Phys. 2018, 123, 043111. [Google Scholar] [CrossRef] [Green Version]
- Li, J.-B.; Kim, N.-C.; Cheng, M.-T.; Zhou, L.; Hao, Z.-H.; Wang, Q.-Q. Optical bistability and nonlinearity of coherently coupled exciton-plasmon systems. Optic Express 2012, 20, 1856. [Google Scholar] [CrossRef]
- Nugroho, B.S.; Iskandar, A.A.; Malyshev, V.A.; Knoester, J. Plasmon-assisted two-photon absorption in a semiconductor quantum dot–metallic nanoshell composite. Phys. Rev. B 2020, 102, 045405. [Google Scholar] [CrossRef]
- Tan, Y.; Xia, X.S.; Liao, X.L.; Li, J.B.; Zhong, H.H.; Liang, S.; Xiao, S.; Liu, L.H.; Luo, J.H.; He, M.D.; et al. A highly-flexible bistable switch based on a suspended monolayer Z-shaped graphene nanoribbon nanoresonator. Carbon 2020, 157, 724. [Google Scholar] [CrossRef]
- Li, J.B.; Liang, S.; Xiao, S.; He, M.D.; Kim, N.C.; Chen, L.Q.; Wu, G.H.; Peng, Y.X.; Luo, X.Y.; Guo, Z.P. Four-wave mixing signal enhancement and optical bistability of a hybrid metal nanoparticle-quantum dot molecule in a nanomechanical resonator. Opt. Express 2016, 24, 2360. [Google Scholar] [CrossRef] [PubMed]
- Asadpour, S.H.; Soleimani, H. Optical bistability and multistability in a four-level quantum system in the presence of plasmonic nanostructure. Phys. E-Low-Dimens. Syst. Nanostruct. 2016, 75, 112. [Google Scholar] [CrossRef]
- Solookinejad, G.; Jabbari, M.; Nafar, M.; Ahmadi, E.; Asadpour, S.H. Incoherent control of optical bistability and multistability in a hybrid system: Metallic nanoparticle-quantum dot nanostructure. J. Appl. Phys. 2018, 124, 063102. [Google Scholar] [CrossRef]
- Tohari, M.M.; Alqahtani, M.M.; Lyras, A. Optical Multistability in the Metal Nanoparticle–Graphene Nanodisk–Quantum Dot Hybrid Systems. Nanomaterials 2020, 10, 1687. [Google Scholar] [CrossRef]
- Tohari, M.M. Terahertz Optical Bistability in the Metal Nanoparticles-Graphene Nanodisks-Quantum Dots Hybrid Systems. Nanomaterials 2020, 10, 2173. [Google Scholar] [CrossRef]
- Evangelou, S. Tailoring second-order nonlinear optical effects in coupled quantum dot-metallic nanosphere structures using the Purcell effect. Microelectron. Eng. 2019, 215, 111019. [Google Scholar] [CrossRef]
- Singh, M.R. Enhancement of the second-harmonic generation in a quantum dot–metallic nanoparticle hybrid system. Nanotechnology 2014, 24, 125701. [Google Scholar] [CrossRef]
- Jha, P.K.; Wang, Y.; Ren, X.; Zhang, X. Quantum-coherence-enhanced transient surface plasmon lasing. J. Opt. 2017, 19, 054002. [Google Scholar] [CrossRef]
- Xu, X.; Broussier, A.; Ritacco, T.; Nahra, M.; Geoffray, F.; Issa, A.; Jradi, S.; Bachelot, R.; Couteau, C.; Blaize, S. Towards the integration of nanoemitters by direct laser writing on optical glass waveguides. Phot. Res. 2020, 8, 1541. [Google Scholar] [CrossRef]
- Ge, D.; Marguet, S.; Issa, A.; Jradi, S.; Nguyen, T.H.; Nahra, M.; Béal, J.; Deturche, R.; Chen, H.; Blaize, S.; et al. Hybrid plasmonic nano-emitters with controlled single quantum emitter positioning on the local excitation field. Nat. Commun. 2020, 11, 3414. [Google Scholar] [CrossRef] [PubMed]
- Lio, G.E.; Madrigal, J.B.; Xu, X.; Peng, Y.; Pierini, S.; Couteau, C.; Jradi, S.; Bachelot, R.; Caputo, R.; Blaize, S. Integration of Nanoemitters onto Photonic Structures by Guided Evanescent-Wave Nano-Photopolymerization. J. Phys. Chem. C 2019, 123, 14669. [Google Scholar] [CrossRef]
- Lio, G.E.; Ferraro, A.; Ritacco, T.; Aceti, D.M.; De Luca, A.; Giocondo, M.; Caputo, R. Leveraging on ENZ Metamaterials to Achieve 2D and 3D Hyper-Resolution in Two-Photon Direct Laser Writing. Adv. Mater. 2021, 33, 202008644. [Google Scholar] [CrossRef]
- Fofang, N.T.; Grady, N.K.; Fan, Z.; Govorov, A.O.; Halas, N.J. Plexciton Dynamics: Exciton—Plasmon Coupling in a J-Aggregate—Au Nanoshell Complex Provides a Mechanism for Nonlinearity. Nano Lett. 2011, 11, 1556. [Google Scholar] [CrossRef]
- Agarwal, G.S. Anisotropic Vacuum-Induced Interference in Decay Channels. Phys. Rev. Lett. 2000, 84, 5500. [Google Scholar] [CrossRef] [Green Version]
- Kiffner, M.; Macovei, M.; Evers, J.; Keitel, C.H. Chapter 3—Vacuum-Induced Processes in Multilevel Atoms. Prog. Opt. 2010, 55, 85. [Google Scholar]
- Yang, Y.; Xu, J.; Chen, H.; Zhu, S. Quantum Interference Enhancement with Left-Handed Materials. Phys. Rev. Lett. 2008, 100, 043601. [Google Scholar] [CrossRef] [PubMed]
- Li, G.X.; Evers, J.; Keitel, C.H. Spontaneous emission interference in negative-refractive-index waveguides. Phys. Rev. B 2009, 80, 045102. [Google Scholar] [CrossRef] [Green Version]
- Jha, P.K.; Ni, X.; Wu, C.; Wang, Y.; Zhang, X. Metasurface-Enabled Remote Quantum Interference. Phys. Rev. Lett. 2015, 115, 025501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hughes, S.; Agarwal, G.S. Anisotropy-Induced Quantum Interference and Population Trapping between Orthogonal Quantum Dot Exciton States in Semiconductor Cavity Systems. Phys. Rev. Lett. 2017, 118, 063601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Karanikolas, V.; Paspalakis, E. Plasmon-Induced Quantum Interference near Carbon Nanostructures. J. Phys. Chem. C 2018, 122, 14788. [Google Scholar] [CrossRef]
- Zhang, S.; Ni, W.; Kou, X.; Yeung, M.H.; Sun, L.; Wang, J.; Yan, C. Formation of Gold and Silver Nanoparticle Arrays and Thin Shells on Mesostructured Silica Nanofibers. Adv. Funct. Mater. 2007, 17, 3258. [Google Scholar] [CrossRef]
- Liu, J.; Dong, H.; Li, Y.; Zhan, P.; Zhu, M.; Wang, Z. A Facile Route to Synthesis of Ordered Arrays of Metal Nanoshells with a Controllable Morphology. Jpn. J. Appl. Phys. 2006, 45, L582. [Google Scholar] [CrossRef]
- Yang, S.; Cai, W.; Kong, L.; Lei, Y. Surface Nanometer-Scale Patterning in Realizing Large-Scale Ordered Arrays of Metallic Nanoshells with Well-Defined Structures and Controllable Properties. Adv. Funct. Mater. 2010, 20, 2527–2533. [Google Scholar] [CrossRef]
- Sainidou, R.; Stefanou, N.; Modinos, A. Green’s function formalism for phononic crystals. Phys. Rev. B 2004, 69, 064301. [Google Scholar] [CrossRef]
- Yannopapas, V.; Vitanov, N. Electromagnetic Green’s tensor and local density of states calculations for collections of spherical scatterers. Phys. Rev. B 2007, 75, 115124. [Google Scholar] [CrossRef]
- Bonifacio, R.; Lugiato, L.A. Optical bistability and cooperative effects in resonance fluorescence. Phys. Rev. A 1978, 18, 1129. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamedi, H.R.; Paspalakis, E.; Yannopapas, V. Effective Control of the Optical Bistability of a Three-Level Quantum Emitter near a Nanostructured Plasmonic Metasurface. Photonics 2021, 8, 285. https://doi.org/10.3390/photonics8070285
Hamedi HR, Paspalakis E, Yannopapas V. Effective Control of the Optical Bistability of a Three-Level Quantum Emitter near a Nanostructured Plasmonic Metasurface. Photonics. 2021; 8(7):285. https://doi.org/10.3390/photonics8070285
Chicago/Turabian StyleHamedi, Hamid R., Emmanuel Paspalakis, and Vassilios Yannopapas. 2021. "Effective Control of the Optical Bistability of a Three-Level Quantum Emitter near a Nanostructured Plasmonic Metasurface" Photonics 8, no. 7: 285. https://doi.org/10.3390/photonics8070285
APA StyleHamedi, H. R., Paspalakis, E., & Yannopapas, V. (2021). Effective Control of the Optical Bistability of a Three-Level Quantum Emitter near a Nanostructured Plasmonic Metasurface. Photonics, 8(7), 285. https://doi.org/10.3390/photonics8070285







